In this paper, a type of complex algebraic differential equations (CADEs) is considered formulating by
α[φ(z)φ"(z)+(φ′(z))2]+amφm(z)+am−1φm−1(z)+...+a1φ(z)+a0=0.
The conformal analysis (angle-preserving) of the CADEs is investigated. We present sufficient conditions to obtain analytic solutions of the CADEs. We show that these solutions are subordinated to analytic convex functions in terms of ez. Moreover, we investigate the connection estimates (coefficient bounds) of CADEs by employing the majorization method. We achieve that the coefficients bound are optimized by Bernoulli numbers.
1.
Introduction
An algebraic differential equation is a differential equation that can be formulated by consequence with differential algebra. There are different directions to study this class involving complex domains. These studies are considered the second-order homogeneous linear differential equation [1], meropmorphic solution by using Painlevé analysis [2], univalent symmetric solution by applying a special case of Painlevé analysis [3], fractional calculus of CADEs [4,5,6], Nevanlinna method, for normal classes, and algebraic differential equations [7], irregular and regular singular solutions, by utilizing special functions such as Zeta function [8], numerical solution [9] and quantum studies [10].
The geometric behavior of classes of CADEs is studied in different views. Phong [11] presented a solution of a class of CADEs driven by string theories. The class is also motivating from the view of non-Kahler geometry and the theory of non-linear partial differential equations. Brodsky [12] analyzed a class of CADEs by utilizing the concept of Bourbaki geometric theory with applications in multi-agent system. Seilera and Seib [13] employed the differential geometric theory to recognize the solution of a class of CADEs. Fenyes [14] introduced a complete analysis of solution of a class of CADEs using the quasiconformal geometry. Kravchenko et al. [15] studied the analytic solution by using the geometry behavior of Liouville transformation. More studies of analytic solutions of CADEs can be located in [16,17,18].
Here, we proceed to study a class of CADEs geometrically. Our tools are based on some concepts from the geometric function theory and univalent function theory. For an analytic function φ which defined in the open unit disk ∪={z∈C:|z|<1}, we formulate the following CADE as follows:
Our aim is to present sufficient conditions to obtain its analytic solutions. We show that these solutions are subordinated to analytic convex functions in terms of ez. Moreover, we investigate the coefficient bounds of CADE by employing the majorization method. We achieve that the coefficients bound are optimized by Bernoulli numbers.
2.
Materials and method
A special class of CADEs is studied in [2] taking the structure
where α and aı∈C,ı=0,...,m are constants such that
Here, we rearrange (2.1) and investigate the geometric properties by including it in some classes of normalized analytic functions in ∪. Then the solution is majorized by employing special function in ∪. Eq (2.1) implies the homogeneous form when α≠0
Fenyes [14] studied a special case of Eq (2.1) as follows: (φ′(z))2=q/2, where q indicates the potential energy, by using the Liouville transformation. The same technique is used by Vladislav et al [15] to analyze the equation φ″(z)+cφ(z)=0.
To study Eq (2.2) geometrically, we need the next concepts.
Definition 2.1. An analytic function φ is subordinated to an analytic function ψ, written φ≺ψ, if occurs an analytic function h with |h(z)|≤|z| such that φ=(ψ(h)) (see [19]). The Ma-Minda classes S∗(ρ) and K(ρ) of starlike and convex functions respectively indicated by (zφ′(z)φ(z))≺ρ(z) and (1+zφ″(z)φ′(z))≺ρ(z), where ρ has a positive real part in ∪,ρ(0)=1,|ρ′(0)|>1 and maps ∪ onto a starlike-domain with respect to one and symmetric based on the real axis.
Our study is indicated by using the above inequality to define the following special class.
Definition 2.2. A function of normalized expansion φ(z)=z+∑∞n=2φnzn,z∈∪ is called in the class M(ρ) if and only if
It is clear that P(0)=1. In the sequel, we shall consider a starlike function with positive real part such as ez and a convex function (univalent)
as well as
is convex univalent in ∪ (see [19], P415). Note that the coefficients are converging to the Bernoulli numbers. Moreover, the real part of the function ϱe(z)=(ez−1)/z satisfies the inequality
Hence, ℜ(eηz−1ηz)≥1ρe(−1)=12.
Our computation is based on analytic technique of Caratheodory functions which are used in [20]. This is the first step. The second step is to majorize Λmφ(z) by a special type of ρ(z),z∈∪ denoted by Λmφ(z)≪ρ(z). Note that two functions are under majorization if and only if |λȷ|≤|ρȷ| for all ȷ=1,2,..., where λȷ and ρȷ are the coefficients of Λmφ(z) and ρ(z) respectively. In this case, we illustrate sufficient conditions of the coefficient bounds of Λmφ(z), for different values of m=0,1,.., using a Caratheodory function.
Majorization-subordination theory creates by Biernacki who exposed in 1936 that if f(z) is subordinate in ∪ to F(z) (F(z) is the normalized function in ∪). In the following works, Goluzin, Tao Shah, Lewandowski and MacGregor studied numerous connected problems, but continuously under the condition that the dominant function F(z) is |z|<0.12. In 1951, Goluzin presented that if f(z) is majorized by a univalent function F(z), then f′(z) is majorized by F′(z) in |z|<0.12. He conjectured that majorization would continuously arise for |z|<3–√8 and this was shown by Tao Shah in 1958. Later Campbell proved the same result for a parametric class of univalent function (see [21]).
3.
Results
In this place, we illustrate our computational results.
Theorem 3.1. Let the function φ∈∧ achieving the inequality
where P(z)=(zφ′(z)φ(z))(zφ″(z)φ′(z)+zφ′(z)φ(z)). Then
when γ≥maxγk,
●
and
●
and
●
and
Proof. Case I: k=0⇒1+γ(zP′(z))≺z+√z2+1.
Define a function Γγ:∪→C admitting the structure
Clearly, Γγ(z) is analytic in ∪ satisfying Γγ(0)=1 and it is a solution of the differential equation
Consequently, we have O(z):=γ(zΓ′γ(z))=z+√z2+1−1 is starlike in ∪. Then for H(z):=O(z)+1, we conclude that
Then Miller-Mocanu Lemma (see [19], P132) implies that
To complete this case, we only request to show that Γγ(z)≺ρe(z). Obviously, the function Γγ(z) is increasing in the interval (−1,1) that is achieving the inequality
Since the function ρe(z) satisfies the inequality for real ϑ,
then the following inequality holds
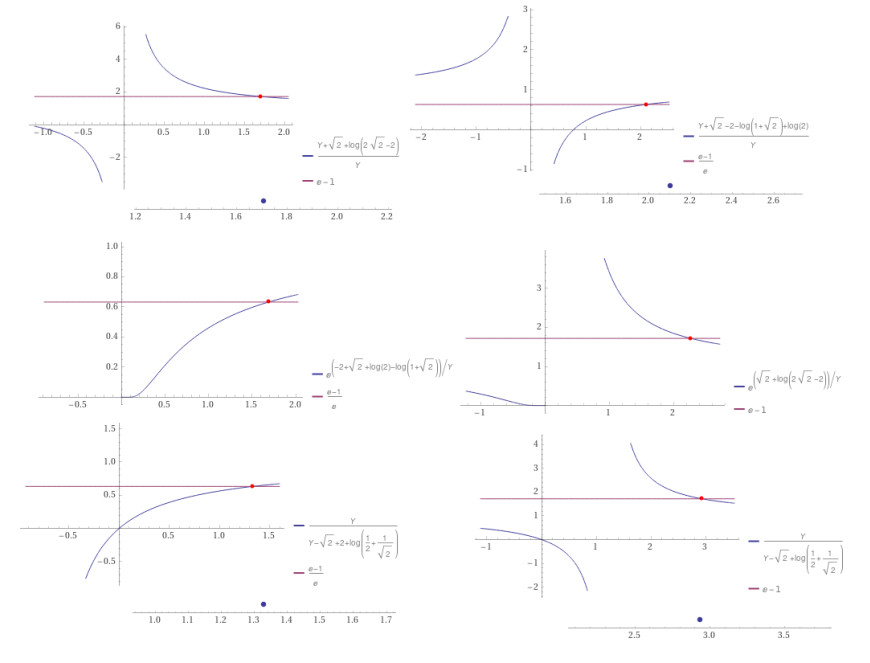
if γ achieves the upper and lower bounds (see Fig 1-first row)
and
This leads to the subordination inequalities
Case II: k=1⇒1+γ(zP′(z)P(z))≺z+√z2+1.
Define a function Πγ:∪→C formulating the structure
Clearly, Πγ(z) is analytic in ∪ satisfying Πγ(0)=1 and it is a solution of the differential equation
By using O(z)=z+√z2+1−1, which is starlike in ∪ and H(z)=O(z)+1, we get
Then again, according to Miller-Mocanu Lemma, we have
Accordingly, the next inequality carries
if γ admits the upper and lower bounds (see Figure 1-second row)
and
This yields to the subordination inequalities
Case III: k=2⇒1+γ(zP′(z)P2(z))≺z+√z2+1.
Define a function Θγ:∪→C formulating the structure
Clearly, Θγ(z) is analytic in ∪ satisfying Θγ(0)=1 and it is a solution of the differential equation
By using O(z)=z+√z2+1−1, which is starlike in ∪ and H(z)=O(z)+1, we get
Then again, according to Miller-Mocanu Lemma, we have
Accordingly, we have
if γ2 admits the upper and lower bounds (see Figure 1-third row)
and
This brings the subordination inequalities
Next result studies the subordination with respect to the function ϱe(z)=ez−1z,z∈∪.
Theorem 3.2. Let the the assumptions of Theorem 3.1 hold. Then
when γ≥maxγk,
●
and
●
and
●
and
Proof. Consider the convex univalent function ϱe(z)=ez−1z. It is clear that ϱ(0)=1 with a positive real part. Moreover it satisfies the inequality
By the proof of Theorem 3.1, we have the following inequality
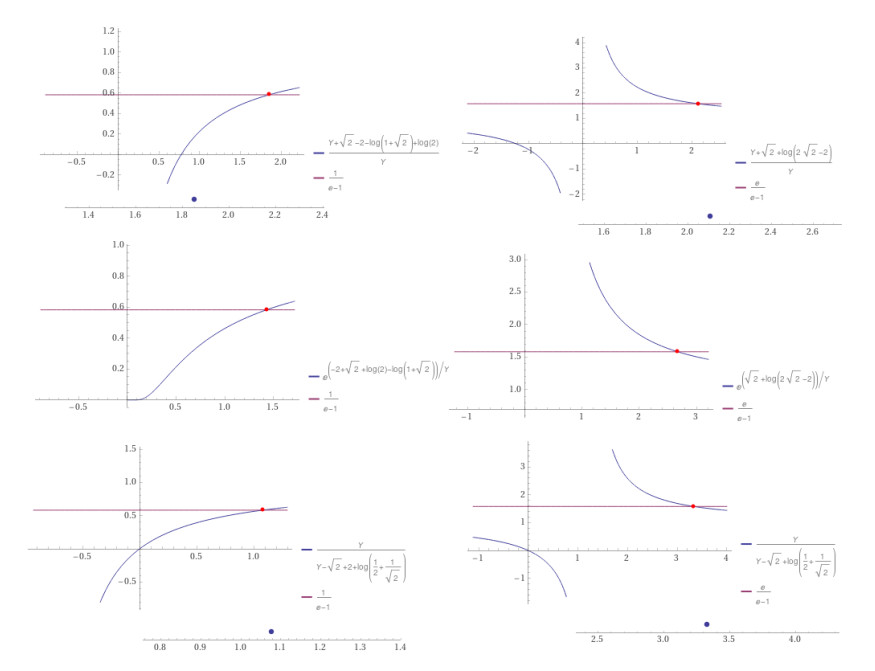
if γ has the upper and lower bounds (see Figure 2-first row)
and
This leads to the subordination inequalities (see Figure 2-second row)
Similarly, we have
and
This yields to the subordination inequalities
Finally, we have the upper and lower bounds (see Figure 2-third row)
and
This brings the subordination inequalities
4.
Discussion
We proceed to include the term Λmφ(z)=amφm(z)+am−1φm−1(z)+...+a1φ(z)+a0 for some m to study the behavior of solutions of Eq (2.1). Dividing Eq (2.1) by α≠0, we have
We have the following result
Theorem 4.1. Consider the CADEs (4.1), with α=−1 and a0=1. If φ∈M(ρ) is a convex univalent function in ∪ satisfying the condition of Theorem 3.1 then the constant connections aı achieving the following values
Proof. From Eq (4.1) together with Theorem 3.1, we have Λmφ(z)≺ρe(z). Since φ is convex univalent in ∪ then it takes the extreme function structure φ(z)=z/(1−z)=z+z2+.... Therefore, we have
In addition, we have
where Bn is the Bernoulli numbers satisfying the inequality
Compering the coefficients of Λmφ(z) and ρe(z), we have
Next result indicates the value of constant coefficients of Λmφ when φ is starlike in ∪.
Theorem 4.2. Consider the CADE (4.1), with α=−1 and a0=1. If φ∈M(ρ) is a starlike function in ∪ satisfying the condition of Theorem 3.1 then the constant connections aı achieving the following values
Proof. Obviously, from the assumptions, we have Λmφ(z)≺ρe(z). Since φ is starlike in ∪ then it admits the extreme function structure φ(z)=z/(1−z)2=z+2z2+.... Therefore, we have
Compering the coefficients of Λmφ(z) and ρe(z), we have
Remark 4.3.
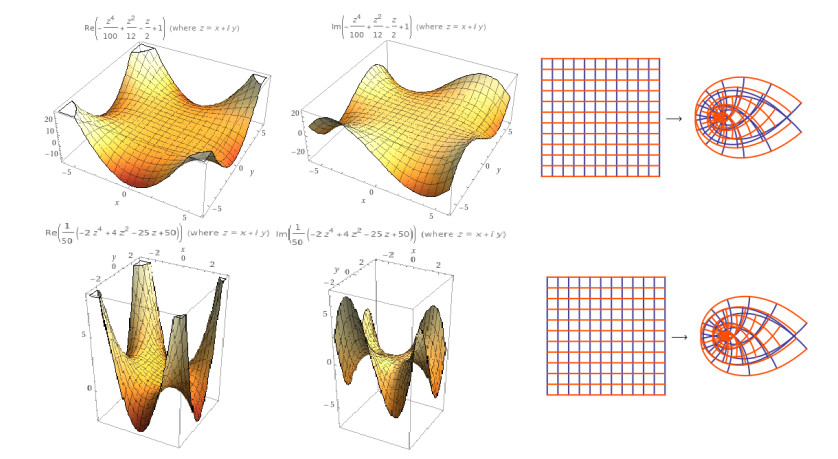
● Note that Theorems 4.1 and 4.2 show that Λmφ(z) accumulates at m=5, which leads to the expansion structure (see Figure 3)
and
● One can generalize Theorems 4.1 and 4.2 in terms of α for all values. In this case, we obtain the constant coefficients Aı=aı−α provided α≠0
and
respectively.
● Results in [2] indicate that for n=4, the coefficients satisfy A4≠0 by using Painlevé analysis, which did not apply in case n≥5. While, the majorization-subordination analysis indicates that for n≥5, the coefficients are converged by Bernoulli numbers.
5.
Conclusions
A class of non-linear complex algebraic differential equations (CADEs) is investigated in view of geometric function theory. We defined a class of normalized functions including the structure of CADEs. Based on the subordination inequality, we introduced the values of constant coefficients. As proceeding works in this direction, one can generalize Eq (2.1) in terms of differential operators including fractional differential and convolution operator in the open unit disk. Or can be realized by a quantum calculus.
Acknowledgments
The authors would like to thanks the reviewers for the deep comments to improve our work. Also, we express our thanks to editorial office for their advice.
Conflict of interest
The authors declare no conflict of interest.









 DownLoad:
DownLoad: