Ordering results of extreme order statistics from dependent and heterogeneous modified proportional (reversed) hazard variables

1.   Introduction

In reliability theory, to model the lifetime data with different hazard shapes, it is desirable to introduce flexible families of distributions, and to this end, there are two methods have been commonly used to characterise lifetime distribution with considerable flexibility. One method is to adopt the well-known families of distributions, for example, Gamma, Weibull and Log-normal, which have been studied quite extensively in the literature, for more discussions on this topic, we refer readers to ^[1,2,3]. Marshall and Olkin ^[4] developed a new method to introduce one parameter to a base distribution results in a new family of distribution with more flexibility. For example, for a baseline distribution function $F$ with support $\mathbb R^+ = (0, \infty)$ and corresponding survival function $\bar F$, the new distribution functions can be defined as

Marshall and Olkin ^[4] originally proposed the family of distributions in $(1.1)$ and studied it for the case when $F$ is a Weibull distribution. When $F$ has probability density and hazard rate functions as $f$ and $h_F$, respectively, then the hazard rate function of $G$ is given by

Therefore, one can observe that if $h_F(x)$ is decreasing (increasing) in $x$, then for $0 < \alpha\le1(\alpha\ge1)$, $h_F(x; \alpha)$ is also decreasing in $x$. Moreover, one can observe that $h_F(x)\le h_F(x; \alpha)$ for $0 < \alpha\le1$, and $h_F(x; \alpha)\le h_F(x)$ for $\alpha\ge1$. For this reason, the parameter $\alpha$ in $(1.1)$ is referred to as a tilt parameter (see ^[5]). Note that (1.1) is equivalent to (1.2) if $\alpha$ in (1.1) is changed to $1/\alpha$. The proportional hazard rates (PHR) and the proportional reversed hazard rates (PRHR) models have important applications in reliability and survival analysis. The random variables $X_{1}, \cdots, X_{n}$ are said to follow: (ⅰ) PHR model if $X_{i}$ has the survival function $\bar F_{X_{i}}(x) = \bar F^{\lambda_{i}}(x)$, $i = 1, \cdots, n$, where $\bar F$ is the baseline survival function and $(\lambda_1, \cdots, \lambda_n)$ is the frailty vector; (ii) PRHR model if $X_{i}$ has the distribution function $F_{X_{i}}(x) = F^{\beta_{i}}(x)$, $i = 1, \cdots, n$, where $F$ is the baseline distribution and $(\beta_1, \cdots, \beta_n)$ is the resilience vector. It is well-known that the Exponential, Weibull, Lomax and Pareto distributions are special cases of the PHR model, and Fréchet distribution is a special case of the PRHR model. Balakrishnan et al. ^[6] introduced two new statistical models by adding a parameter to PHR and PRHR models, which are regarded as the baseline distributions in $G(x; \alpha)$ and $H(x; \alpha)$, respectively. The two new models are referred to as the modified proportional hazard rates (MPHR) and modified proportional reversed hazard rates (MPRHR) models, respectively. These are given by

where $\lambda$ and $\beta$ are the proportional hazard rate and proportional reversed hazard rate parameters, respectively. We denote $X \sim MPHR(\alpha, \lambda; \bar F)$ and $X\sim MPRHR(\alpha, \beta; F)$ if X has the distribution functions $G(x; \alpha, \lambda)$ and $H(x; \alpha, \beta)$, respectively. For the case $\lambda = \beta = 1$, (1.3) and (1.4) simply reduce to (1.1) and (1.2), respectively. For the case $\alpha = 1$, (1.3) and (1.4) simply reduce to the PHR and PRHR models, respectively. According to the Theorem 2.1 of Navarro et al.^[14], $(1.3)$ and $(1.4)$ can be rewritten the distorted distribution of $h_1$ and $h_2$, respectively, where

if $\lambda = \beta = 1$, (1.5) just as the distorted distributions of (1.1) and (1.2), respectively. For some special models, please refer to multiple-outlier models (^[7], ^[8]), extended exponential and extend Weibull distribution (^[4], ^[9]), extended Pareto distribution (^[10]) and extended Lomax distribution (^[11]).

Order statistics play an important role in reliability theory, auction theory, operations research, and many applied probability areas. $X_{k:n}$ denotes the $k$th smallest of random variables $X_1, \dots, X_n, \; k = 1, \dots, n.$ In reliability theory, $X_{k:n}$ characterizes the lifetime of a $(n-k+1)$-out-of-$n$ system, which works if at least $n-k+1$ of all the $n$ components function normally. Specifically, $X_{1:n}$ and $X_{n:n}$ denote the lifetimes of series and parallel systems, respectively. In auction theory, $X_{1:n}$ and $X_{n:n}$ represent the final price of the first-price procurement auction and the first-price sealed-bid auction (see ^[12]), respectively. In the past decades, researchers devoted themselves to stochastic comparisons of order statistics from heterogeneous independent or dependent samples. For example. Belzunce et al. ^[13] established some results and applications concerning the likelihood ratio order of random vectors of order statistics in the case of independent but not necessarily identically distributed observations and for the case of possible dependent observations. Navarro et al.^[14] obtained ordering properties for coherent systems with possibly dependent identically distributed components. Balakrishnan and Zhao^[15] studied the stochastic comparison of order statistics from independent and heterogeneous proportional hazard rates models, gamma variables, geometric variables, and negative binomial variables in the stochastic orders and majorization orders. For more discussions related to order statistics, one may refer to ^{[16,17,18,19,20]}. Besides, many authors have studied stochastic comparisons of order statistics from heterogeneous samples following some families of lifetime distributions. For example, Fang et al.^[21] conducted stochastic comparisons on sample extremes of dependent and heterogeneous observations from PHR and PRHR models. Balakrishnan et al. ^[6] introduced MPHR and MPRHR model, and established some stochastic comparisons between the corresponding order statistics with independent samples. Li and Li ^[22] developed sufficient conditions for the (reversed) hazard rate order on maximums (minimums) of samples following PRHR (PHR) model under Archimedean copula. Das and Kayal ^[23] introduced a scale parameter into the MPHR and MPRHR models lead to new models, which are called as modified proportional hazard rate scale (MPHRS) and modified proportional reversed hazard rate scale (MPRHRS) models, respectively, and obtained some stochastic comparison results on independent samples in term of the usual stochastic, (reversed) hazard rate orders. Barmalzan et al. ^[24] discussed the hazard rate order and reversed hazard rate order of series and parallel systems with dependent components following either MPHR or MPRHR models under Archimedean copula. Motivated by the work of Balakrishnan et al.^[6], this paper devotes to studying stochastic comparisons of sample extremes arising from heterogeneous and dependent MPHR and MPRHR models, and we derive the usual stochastic, (reversed) hazard rate orders of extremes with the heterogeneity considered in the model parameters.

The remaining part of the paper is organized as follows: Section 2 recalls some basic concepts and notations that will be used in the sequel. Section 3 deals with stochastic comparisons between minimums of MPRHR (MPHR) sample with Archimedean (survival) copula, respectively. Section 4 presents the corresponding results on maximums of the sample. Section 5 summarizes our research findings and future directions.

2.   Preliminaries

In this section, let us first review some basic concepts that will be used in the sequel. Let $X$ and $Y$ be two random variables with distribution functions $F(x)$ and $G(x)$, survival functions $\bar F(x) = 1- F(x)$ and $\bar G(x) = 1-G(x)$, probability density functions $f(x)$ and $g(x)$, the hazard rate functions $h_X(x) = f(x) /\bar F(x)$ and $h_Y(x) = g(x)/\bar G(x)$, and reversed hazard rate functions $r_X(x) = f(x)/F(x)$ and $r_Y(x) = g(x)/G(x)$, respectively.

Definition 1. $X$ is said to be smaller than $Y$ in the sense of

(i) the usual stochastic order (denoted by $X\leq_{st}Y$) if $\bar{F}(x)\le \bar{G}(x)$ for all $x \in \mathbb R^+$;

(ii) the hazard rate order (denoted by $X\leq_{hr}Y$) if $h_{X}(x)\ge h_{Y}(x)$ for all $x \in \mathbb R^+$ or equivalently, if $\bar{G}(x)/\bar{F}(x)$ is increasing in $x \in \mathbb R^+$;

(iii) the reversed hazard rate order (denoted by $X\leq_{rh}Y$) if $r_{X}(x)\le r_{Y}(x)$ for all $x \in \mathbb R^+$ or equivalently, if $G(x)/ F(x)$ is increasing in $x \in \mathbb R^+$.

It is well known that the hazard rate and reversed hazard rate orders imply the usual stochastic order, but the converse is not true. For a comprehensive discussion on various stochastic orders and their applications, one may refer to Shaked and Shanthikumar^[25] and Li and Li ^[26].

Next, we introduce the notion of the weak majorization order. For two real vectors $\boldsymbol x = (x_1, ..., x_n)$ and $\boldsymbol y = (y_1, ..., y_n)\in \mathbb R^n$, $x_{(1)} \le x_{(2)} \le\dots\le x_{(n)}$ and $y_{(1)} \le y_{(2)} \le\dots\le y_{(n)}$ denote the increasing arrangement of the components of $\boldsymbol x$ and $\boldsymbol y$, respectively. Denote $\mathcal I_n = \{1, \dots, n\}$.

Definition 2. The vector $\boldsymbol x$ is said to be weakly supermajorized by the vector $\boldsymbol y$ (write as $\boldsymbol x \overset{w}\preceq \boldsymbol y$) if $\sum_{i = 1}^j x_{(i)}\ge \sum_{i = 1}^j y_{(i)},$ for all $j\in\mathcal I_n$.

Now, let us review the concept of Archimedean Copulas.

Definition 3. For a decreasing and continuous function $\psi:[0, +\infty ]\mapsto [0, 1]$ such that $\psi(0) = 1$ and $\psi(+\infty) = 0,$ let $\phi = \psi^{-1}$ be the pseudo-inverse. Then

is said to be an Archimedean copula with generator $\psi$ if $(-1)^k\psi^{k}(x)\ge 0$ for $k = 0, \dots, n-2$ and $(-1)^{n-2}\psi^{n-2}(x)$ is decreasing and convex.

For detailed discussions on copulas and its applications, one may refer to Nelsen ^[27].

The following lemmas are useful to establish the main results.

Lemma 1. (^[28]) Let $I\subseteq \mathbb R$ be an open interval, a continuously differentiable $h:I^n\rightarrow \mathbb R$ is Schur-convex(Schur-concave) if and only if $h$ is symmetric on $I^n$ and for all $i\neq j$

Lemma 2. (^[29]) For a real function $h$ on $\mathcal {A}\subseteq\mathbb R^n$, $\boldsymbol x \overset{w}\preceq\boldsymbol y$ implies $h(\boldsymbol x) \le h(\boldsymbol y)$ if and only if $h$ is decreasing and Schur-convex on $\mathcal A$.

$h(x)$ is Schur-concave on $\mathcal {A}$ if and only if $-h(x)$ is Schur-convex. For more details on majorization and Schur-convexity (concavity), please refer to ^[29].

Lemma 3. For two $n$-dimensional Archimedean copulas $C_{\psi_1}$ and $C_{\psi_2}$, if $\phi_2 \circ \psi_1$ is super-additive, then $C_{\psi_1}(\boldsymbol u)\le C_{\psi_2}(\boldsymbol u)$ for all $\boldsymbol u\in [0, 1]^n$.

Throughout this paper, all concerned random variables are assumed to be absolutely continuous and nonnegative, and the terms increasing and decreasing stand for non-decreasing and non-increasing, respectively. Denote $\boldsymbol e_i = (\underbrace{0, \dots, 0, 1, }_{i}\underbrace{0, \dots, 0}_{n-i})$ for $i\in \mathcal I_n$, and “$\overset{sgn}{ = }$” means equality of sign.

3.   Minimum of sample

In this section, we carry out stochastic comparison of smallest order statistics from dependent and heterogeneous MPHR and MPRHR samples, and the heterogeneity has been considered in the model parameters.

3.1.   On samples of MPHR

In this subsection, we deal with the case of MPHR samples in the sense of the usual stochastic and hazard rate orders. Let $\textbf{X} = (X_1, \dots, X_n)$ be the random vector, we denote $\boldsymbol X \sim MPHR (\mathit{\boldsymbol{\alpha}}; \boldsymbol \lambda; \bar F; \psi)$ the sample follows MPHR model, where $\bar F$ is the baseline survival function, $\psi$ is generator of the associated Archimedean survival copula, and $\mathit{\boldsymbol{\alpha}} = (\alpha_1, \dots, \alpha_n)$ and $\mathit{\boldsymbol{\lambda}} = (\lambda_1, \dots, \lambda_n)$ are the tilt parameter vector and modified proportional hazard rate vector, respectively.

Next, we establish sufficient conditions for the usual stochastic order, whenever the modified proportional hazard rate parameters may be different with the tilt parameters being equal.

Theorem 1. For $\mathit{\boldsymbol{X}} \sim MPHR(\alpha; \mathit{\boldsymbol{\lambda}}; \bar F; \psi_1)$ and $\mathit{\boldsymbol{Y}} \sim MPHR(\alpha; \mathit{\boldsymbol{\mu}}; \bar F; \psi_2)$, where $0 < \alpha\le1$. If $\psi_1$ or $\psi_2$ is log-concave, and $\phi_2 \circ \psi_1$ is super-additive, then $\mathit{\boldsymbol{\lambda}}\overset{w}\preceq \mathit{\boldsymbol{\mu}}$ implies

Proof. The survival function of $X_{1:n}$ can be expressed as

Assume that $\psi_1$ is log-concave. In order to obtain the required result, according to Lemma 2, it is sufficient to show that $J_1({\alpha}, \mathit{\boldsymbol{\lambda}}, \psi_1, \bar F(x))$ is decreasing in ${\lambda_i}$ and Schur-convex in $\mathit{\boldsymbol{\lambda}}$ for given $x\ge0$ and $0 < \alpha\le1$. Taking the partial derivative of $J_1({\alpha}, \mathit{\boldsymbol{\lambda}}, \psi_1, \bar F(x))$ with respect to ${\lambda_i}$, $i\in\mathcal I_n$, we have

That is, $J_1({\alpha}, \mathit{\boldsymbol{\lambda}}, \psi_1, \bar F(x))$ is decreasing in $\lambda_i$. Furthermore, for $i\not = j,$ we have

where

Since the log-concavity of $\psi_1$ implies the increasing property of ${\psi_1}/{\psi_1'}$, and consider that $\phi_1({\alpha(\bar F(x))^{\lambda}}/{(1 -\bar\alpha(\bar F(x))^{\lambda})})$ is increasing in $\lambda,$ then we have

On the other hand, ${1}/{(1 -\bar\alpha(\bar F(x))^{\lambda})}$ is nonnegative and decreasing in $\lambda.$ Consequently, $h_1(\lambda)$ is increasing in $\lambda$, which in turn implies that

Thus, Schur-convexity of $J_1({\alpha}, \mathit{\boldsymbol{\lambda}}, \psi_1, \bar F(x))$ follows from Lemma 1. Due to Lemma 2, $\mathit{\boldsymbol{\lambda}}\overset{w}\preceq \mathit{\boldsymbol{\mu}}$ implies $J_1({\alpha}, \mathit{\boldsymbol{\lambda}}, \psi_1, \bar F(x)) \le J_1({\alpha}, \mathit{\boldsymbol{\mu}}, \psi_1, \bar F(x))$, and note that $\phi_2 \circ \psi_1$ is super-additive, by Lemma 3, we have $J_1({\alpha}, \mathit{\boldsymbol{\mu}}, \psi_1, \bar F(x)) \le J_1({\alpha}, \mathit{\boldsymbol{\mu}}, \psi_2, \bar F(x))$. Hence, it holds that

As a consequence, we conclude that $\ X_{1:n}\le_{st}Y_{1:n}.$ For the case of $\psi_2$ is log-concave, the proof can be obtained in a similar way. Then we complete the proof.

Remark 1. When $\alpha = 1$, MPHR model reduces to PHR model, which just the result established in Theorem 4.1 (ii) of ^[21].

The next example illustrates the result of Theorem 1.

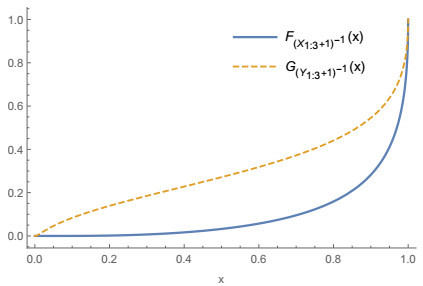
Example 1. Consider the case of $n = 3$. Let $\bar F(x) = e^{-(a x)^b}$, $a > 0$, $0 < b\le1$, and generators $\psi_1(x) = e^{\frac{1-e^{x}}{\theta_1}}$, $0 < \theta_1\le 1$, $\psi_2(x) = (\theta_2{x}+1)^{-1/\theta_2}$, $\theta_2 > 0$. Set $\alpha = 0.4, a = 1.2, b = 0.5, \theta_1 = 0.1, \theta_2 = 1.2, \; \mathit{\boldsymbol{\lambda}} = (0.4, 0.5, 0.6)\overset{w}\preceq(0.3, 0.4, 0.5) = \mathit{\boldsymbol{\mu}}$. One can check chat $\psi_1(x)$ is log-concave. It can be seen that $[\phi_2 \circ \psi_1(x)]'' = e^{x}\big(e^{\frac{1}{\theta_1}-\frac{e^{x}}{\theta_1}}\big)^{-\theta_2}\big(\theta_1+e^{x}\theta_2\big){\theta_1}^{-2}\ge0$, that is, $\phi_2 \circ \psi_1(x)$ is convex function in $x$, which implies that $\phi_2 \circ \psi_1$ is super-additive. To plot the whole of survival curves of $X_{1:3}$ and $Y_{1:3}$ on $[0, \infty)$, we perform the transformation $(x+1)^{-1}:[0, \infty)\longmapsto[ 0, 1]$. Then it is obvious that $X_{1:3}\le_{st}Y_{1:3}$ is equivalent to ${({Y_{1:3}+1})^{-1}}\le_{st} ({X_{1:3}+1})^{-1}$. As is seen in Figure 1, the distribution curve of ${({X_{1:3}+1})^{-1}}$ is always beneath that of ${({Y_{1:3}+1})^{-1}}$, that is, $X_{1:3}\le_{st}Y_{1:3}$, which coincides with the result of Theorem 1.

The following counterexample shows that the condition $\psi_1(x)$ or $\psi_2(x)$ is log-concave given in the above Theorem 1 can't be dropped.

Counterexample 1. Under the setup of Example 1, let generators $\psi_1(x) = (\theta_1{x}+1)^{-1/\theta_1}, \psi_2(x) = (\theta_2{x}+1)^{-1/\theta_2}$, $\theta_i > 0, i = 1, 2.$ Note that $[\log {\psi_i(x)}]'' = \theta_i(1+\theta_i{x})^{-2}\ge0$ for $x\ge 0$, thus, $\psi_i(x)$ is log-convex. Take $\theta_1 = 10, \theta_2 = 1.2.$ As is seen in Figure 2, the survival function $\bar F_{X_{1:3}}(x)$ is not always beneath that of $\bar G_{Y_{1:3}}(x)$, that is, neither $X_{1:3}\leq_{st}Y_{1:3}$ nor $X_{1:3}\geq_{st}Y_{1:3}$.

The following theorem presents the usual stochastic order on sample minimum, here, we assume that the two samples have common modified proportional hazard rates parameters.

Theorem 2. For $\mathit{\boldsymbol{X}} \sim MPHR (\mathit{\boldsymbol{\alpha}}; \lambda; \bar F; \psi_1)$ and ${\bf {Y}} \sim MPHR (\mathit{\boldsymbol{\beta}}; \lambda; \bar F; \psi_2)$. If $\phi_1 \circ \psi_2$ is super-additive, then $\mathit{\boldsymbol{\alpha}}\overset{w}\preceq \mathit{\boldsymbol{\beta}}$ implies

Proof. The survival function of $X_{1:n}$ can be written as

To obtain the required result, it suffices to show that the $J_2(\mathit{\boldsymbol{\alpha}}, \lambda, \psi_1, \bar F(x))$ is increasing in ${\alpha_i}$ and Schur-concave in $\mathit{\boldsymbol{\alpha}}$, $i\in \mathcal{I}_n$. Differentiating $J_2(\mathit{\boldsymbol{\alpha}}, \lambda, \psi_1, \bar F(x))$ with respect to $\alpha_i$, we obtain

That is, $J_2(\mathit{\boldsymbol{\alpha}}, \lambda, \psi_1, \bar F(x))$ is increasing in $\alpha_i$. Furthermore, for $i\not = j,$ we have

where

By the decreasing and convexity of $\psi_1$, it holds that

Hence, $h_2(\alpha)$ is negative and decreasing in $\alpha,$ or equivalently, ${1}/{h_2(\alpha)}$ is negative and increasing in $\alpha$. Then, for $i\not = j,$

It follows from Lemma 1 that $J_2(\mathit{\boldsymbol{\alpha}}, \lambda, \psi_1, \bar F(x))$ is Schur-concave, which is equivalent to $-J_2(\mathit{\boldsymbol{\alpha}}, \lambda, \psi_1, \bar F(x))$ is Schur-convex. According to Lemma 2, $\mathit{\boldsymbol{\alpha}}\overset{w}\preceq \mathit{\boldsymbol{\beta}}$ implies $-J_2(\mathit{\boldsymbol{\alpha}}, \lambda, \psi_1, \bar F(x))\le -J_2(\mathit{\boldsymbol{\beta}}, {\lambda}, \psi_1, \bar F(x)),$ and note that $\phi_1 \circ \psi_2$ is super-additive, it holds by Lemma 3 that $J_2(\mathit{\boldsymbol{\beta}}, {\lambda}, \psi_1, \bar F(x)) \ge J_2(\mathit{\boldsymbol{\beta}}, {\lambda}, \psi_2, \bar F(x))$. Hence,

That is, $\ X_{1:n}\ge_{st}Y_{1:n}.$ The desired result then follows.

The following example demonstrates the theoretical result of Theorem 2.

Example 2. Let $\bar F(x) = e^{-(a x)^b}, a > 0$, $0 < b\le1$, and generators $\psi_1(x) = (\theta {x}+1)^{-1/\theta}$, $\theta > 0, \; \psi_2(x) = e^{-{x}}$. Take $n = 3, \lambda = 0.4, a = 0.5, b = 0.8, \theta = 0.7$ and $\mathit{\boldsymbol{\alpha}} = (0.3, 0.5, 0.7)\overset{w}\preceq(0.2, 0.4, 0.5) = \mathit{\boldsymbol{\beta}}.$ It is easy to check that the conditions of Theorem 2 are all satisfied. The distribution functions of ${({X_{1:3}+1})^{-1}}$ and ${({Y_{1:3}+1})^{-1}}$ are displayed in Figure 3, which confirms $X_{1:3}\ge_{st}Y_{1:3}.$

The next theorem gives sufficient conditions guaranteeing the hazard rate order between two modified proportional hazard rates models with the same modified proportional hazard rates parameters and the heterogeneous tilt parameters.

Theorem 3. For $\mathit{\boldsymbol{X}} \sim MPHR (\mathit{\boldsymbol{\alpha}}; \lambda; \bar F; \psi)$ and $\mathit{\boldsymbol{Y}} \sim MPHR (\mathit{\boldsymbol{\beta}}; \lambda; \bar F; \psi)$ with log-concave $\psi$, and $-{\psi'}/{\psi}$ is log-convex. If $\mathit{\boldsymbol{\alpha}}\overset{w}\preceq \mathit{\boldsymbol{\beta}}$, then

Proof. For convenience, let us give the following facts for any $x\ge 0$.

$\varrho1$: $\psi (x) \; \ge0$ and $\psi'(x) \; \le 0$.

$\varrho2$: The log-concave $\psi$ implies the decreasing $\psi'/\psi$ and hence $\psi''(x)\psi (x)\le [\psi'(x)]^2$.

$\varrho3$: The log-convex $-\psi'/\psi$ implies that $\{\psi''(x)\psi(x)-[\psi'(x)]^2\}/{\psi(x)\psi'(x)}\ge 0$ increases in $x\ge 0$.

Denote $h(x)$ the hazard rate function corresponding to the baseline survival function $\bar F(x)$. The survival function of $X_{1:n}$ can be written as

and the hazard rate function of $X_{1:n}$ is

Likewise, $Y_{1:n}$ gets the hazard rate function $h_{Y_{1:n}}(x) = L_1(x, \mathit{\boldsymbol{\beta}}, \lambda, \psi)$ for $x \ge 0.$ Further denote

Then, for any $s, t\in \mathcal I_n$ with $s\not = t$ and all $u_i\mathcal \in[0, 1]$ ($i\in \mathcal I_n$), we have

By $\varrho3$, it holds that $C_1(\boldsymbol \alpha, x)\ge C_1(\alpha_s\mathit{\boldsymbol{e}}_s, x)$, and in combination $\varrho1$ with $\varrho2$, we have

Therefore, $L_1(x, \mathit{\boldsymbol{\alpha}}, \lambda, \psi)$ is decreasing in $\alpha_i$ for any $i\in\mathcal I_n.$ Furthermore, for $s, t\in \mathcal I_n$ with $s\not = t,$ we obtain

As ${1}/{h_2(\alpha)}$ is increasing in $\alpha$, it holds that

Likewise, for $\alpha_s \ge \alpha_t,$ we have

Consider that ${1}/{(1-\bar\alpha_i(\bar F(x))^{\lambda})^2}\ge0$ is decreasing in $\alpha_i$, $\varrho2$ implies that $B_1(\alpha_i\mathit{\boldsymbol{e}}_i, x)$ is increasing in $\alpha_i$, and $B_1(\alpha_t\mathit{\boldsymbol{e}}_t, x)\le B_1(\alpha_s\mathit{\boldsymbol{e}}_s, x)\le 0$ for $\alpha_s\ge \alpha_t$. Then

Note that ${ 1}/{(1-\bar\alpha_i(\bar F(x))^{\lambda})}\ge0$ is decreasing in $\alpha_i$ and ${1}/{h_2(\alpha_i)}$ is increasing in $\alpha_i$. It is clear that

is increasing in $\alpha_i$ for $\alpha_s\ge \alpha_t$. By $\varrho3$, for $\alpha_s\ge \alpha_t$, we have $C_1(\boldsymbol \alpha, x)-C_1(\alpha_t\mathit{\boldsymbol{e}}_t, x)\ge C_1(\boldsymbol \alpha, x)- C_1(\alpha_s\mathit{\boldsymbol{e}}_s, x)\ge0.$ It holds that

Combing (3.1)-(3.4), we conclude that $L_1(x, \mathit{\boldsymbol{\alpha}}, {\lambda}, \psi)$ is Schur-convex with respect to $\mathit{\boldsymbol{\alpha}}$. Thus, according to Lemma 1 and Lemma 2, $\mathit{\boldsymbol{\alpha}}\overset{w}\preceq \mathit{\boldsymbol{\beta}}$ implies $h_{X_{1:n}}(x) = L_1(x, \mathit{\boldsymbol{\alpha}}, {\lambda}, \psi)\le L_1(x, \mathit{\boldsymbol{\beta}}, {\lambda}, \psi) = h_{Y_{1:n}}(x)$ for all $x$, that is, $X_{1:n}\ge_{hr} Y_{1:n}.$

Remark 2. For two independent samples, we have $\psi(x) = e^{-{x}}$, thus Theorem 3 serves as a generalization of Theorem 3.4 (ii) of ^[6] to the case of dependent samples with Archimedean survival copulas.

We present the following example to illustrate the result of Theorem 3.

Example 3. Let $\bar F(x) = e^{-(a x)^b}, a > 0$, $0 < b\le1$, and generator $\psi(x) = e^{\frac{1-e^{x}}{\theta}}$, $0 < \theta\le 0.5(3-\sqrt5)$. Set $n = 3, \lambda = 0.03, a = 5, b = 0.007, \theta = 0.1$ and $\mathit{\boldsymbol{\alpha}} = (0.8, 0.6, 0.4)\overset{w}\preceq(0.7, 0.5, 0.3) = \mathit{\boldsymbol{\beta}}$. One can verify that the conditions of Theorem 3 are all satisfied. Figure 4 plots the reversed hazard rate functions of ${({X_{1:3}+1})^{-1}}$ and ${({Y_{1:3}+1})^{-1}}$, and it is obvious that $X_{1:3}\ge_{hr}Y_{1:3}$ is equivalent to ${({X_{1:3}+1})^{-1}}\le_{rh} ({Y_{1:3}+1})^{-1}$, which asserts $X_{1:3}\ge_{hr} Y_{1:3}.$

3.2.   On samples of MPRHR

In this subsection, we consider the case of MPRHR samples. Let $\textbf{X} = (X_1, \dots, X_n)$ be the random vector, and $\boldsymbol X \sim MPRHR (\mathit{\boldsymbol{\alpha}}; \mathit{\boldsymbol{\beta}}; F;\psi)$, where $F$ is the baseline distribution function, $\psi$ is generator of the associated Archimedean copula, and $\mathit{\boldsymbol{\alpha}} = (\alpha_1, \dots, \alpha_n)$ and $\mathit{\boldsymbol{\beta}} = (\beta_1, \dots, \beta_n)$ are the tilt parameter vector and modified proportional reversed hazard rate vector, respectively.

Next, we present two results on the heterogeneity among parameters in terms of the usual stochastic order. The proofs can be obtained along the same way with that of Theorem 1, and thus omitted here.

Theorem 4. For $\mathit{\boldsymbol{X}} \sim MPRHR(\alpha; \mathit{\boldsymbol{\beta}}; F; \psi_1)$ and $\mathit{\boldsymbol{Y}} \sim MPRHR (\alpha; \mathit{\boldsymbol{\gamma}}; F; \psi_2)$, where $0 < \alpha\le1$. If $\psi_1$ or $\psi_2$ is log-concave, and $\phi_1 \circ \psi_2$ is super-additive, then $\mathit{\boldsymbol{\beta}}\overset{w}\preceq \mathit{\boldsymbol{\gamma}}$ implies

Theorem 5. For $\mathit{\boldsymbol{X}} \sim MPRHR (\mathit{\boldsymbol{\alpha}}; \beta; F; \psi_1)$ and $\mathit{\boldsymbol{Y}} \sim MPRHR (\mathit{\boldsymbol{\upsilon}}; \beta; F; \psi_2)$. If $\psi_1$ or $\psi_2$ is log-concave, and $\phi_2 \circ \psi_1$ is super-additive, then $\mathit{\boldsymbol{\alpha}}\overset{w}\preceq \mathit{\boldsymbol{\upsilon}}$ implies

The next example is provided to illustrate the result of Theorem 4.

Example 4. Let $\bar F(x) = e^{-(a x)^b}, a > 0$, $0 < b\le1$, and generators $\psi_1(x) = (\theta_1 {x}+1)^{-1/\theta_1}$, $\theta_1 > 0, \; \psi_2(x) = e^{\frac{1-e^{x}}{\theta_2}}$, $0 < \theta_2\le1$. Set $n = 3, \alpha = 0.3, \theta_1 = 0.8, \theta_2 = 0.9, a = 0.1, b = 0.9, \; \mathit{\boldsymbol{\beta}} = (0.3, 0.3, 0.4)\overset{w}\preceq(0.2, 0.2, 0.3) = \mathit{\boldsymbol{\gamma}}$. It is easy to check that all conditions of Theorem 4 are satisfied. The distribution functions of $({X_{1:3}+1})^{-1}$ and $({Y_{1:3}+1})^{-1}$ are plotted in Figure 5, from which we can confirm that $\ X_{1:3}\ge_{st} Y_{1:3}.$

The following theorem compares two sample minimums with common modified proportional reversed hazard rates parameters and heterogeneous tilt parameters in the sense of the hazard rate order. The proof is similar to that of Theorem 3 and hence omitted.

Theorem 6. For $\mathit{\boldsymbol{X}} \sim MPRHR (\mathit{\boldsymbol{\alpha}}; \beta; F; \psi)$ and $\mathit{\boldsymbol{Y}} \sim MPRHR (\mathit{\boldsymbol{\upsilon}}; \beta; F; \psi)$ with log-concave $\psi$, and $-{\psi'}/{\psi }$ is log-convex. If $\boldsymbol \alpha \overset{w}\preceq \boldsymbol \upsilon$, then

Remark 3. It is mentioned that Theorem 6 generalizes Theorem 4.5 (ii) of ^[6] to the case of dependent samples with Archimedean copulas.

Remark 4. According to the Theorem 2.1 of Navarro et al.^[10], the coherent system reliability function can be written as $\bar F_{X}(x) = h(\bar F(x))$, where $h$ only depends on structure function and on the survival copula of $(X_1, \dots, X_n)$. Owing to the complexity of coherent system, we only studied the series system in Section 3 by distored distribution. Based on Archimedean copula $K$, we obtained the distorted function representations of series system as follows:

where $h_3(u; \boldsymbol \alpha, \boldsymbol \lambda)$ and $h_4(u; \boldsymbol \alpha, \boldsymbol \beta)$ are obtained by MPHR and MPRHR models. In $(3.5)$, if $\alpha_i = \alpha (i = 1, \dots, n),$ we can obtain representation of Theorem 1, when $\lambda_i = \lambda (i = 1, \dots, n)$, we can obtain representation of Theorem 2 and Theorem 3. As for in other Theorem 4, Theorem 5, and Theorem 6, we can obtain in similar way by $(3.6)$.

4.   Maximum of sample

In parallel to the previous section, here, we consider the largest order statistics from dependent and heterogeneous MPHR and MPRHR samples.

4.1.   On samples of MPHR

In this subsection, we deal with the case of MPHR samples in the sense of the usual stochastic and reversed hazard rate orders. The first theorem establishes sufficient conditions for the usual stochastic order, which can be verified in a similar method with that of Theorem 1, and thus omitted for brevity.

Theorem 7. For $\mathit{\boldsymbol{X}} \sim MPHR(\alpha; \mathit{\boldsymbol{\lambda}}; \bar F; \psi_1)$ and $\mathit{\boldsymbol{Y}} \sim MPHR(\alpha; \mathit{\boldsymbol{\mu}}; \bar F; \psi_2)$, where $0 < \alpha\le1$. If $\psi_1$ or $\psi_2$ is log-concave, and $\phi_1 \circ \psi_2$ is super-additive, then $\mathit{\boldsymbol{\lambda}}\overset{w}\preceq \mathit{\boldsymbol{\mu}}$ implies

In the following, we give a numerical example to illustrate the effectiveness of Theorem 7.

Example 5. Under the setup of Example 4, set $n = 3, \alpha = 0.9, \theta_1 = 0.9, \theta_2 = 2, a = 0.5, b = 0.3, \; \mathit{\boldsymbol{\lambda}} = (4, 5, 6)\overset{w}\preceq(3, 4, 5) = \mathit{\boldsymbol{\mu}}$. One can check that the conditions of Theorem 7 are satisfied. The survival functions of ${({X_{3:3}+1})^{-1}}$ and ${({Y_{3:3}+1})^{-1}}$ are plotted in Figure 6, which verifies that $F_{X_{3:3}}(x) \ge G_{Y_{3:3}}(x)$. That is, \ $X_{3:3}\le_{st}Y_{3:3}$.

The following theorem conducts stochastic comparisons of samples with heterogeneous tilt parameters in the sense of the usual stochastic order.

Theorem 8. For $\mathit{\boldsymbol{X}} \sim MPHR (\mathit{\boldsymbol{\alpha}}; \lambda; \bar F; \psi_1)$ and ${\bf {Y}} \sim MPHR (\mathit{\boldsymbol{\beta}}; \lambda; \bar F; \psi_2)$. If $\psi_1$ or $\psi_2$ is log-concave, and $\phi_2 \circ \psi_1$ is super-additive, then $\mathit{\boldsymbol{\alpha}}\overset{w}\preceq\mathit{\boldsymbol{\beta}}$ implies

Proof. The distribution function $X_{n:n}$ is given by

Without loss of generality, we assume that $\psi_1$ is log-concave. In order to obtain the desired result, we just need to prove that $J_3(\mathit{\boldsymbol{\alpha}}, {\lambda}, \psi_1, \bar F(x))$ is decreasing in ${\alpha_i}$ and Schur-convex in $\mathit{\boldsymbol{\alpha}}$, $i \in \mathcal{I}_n$. Taking the partial derivative of $J_3(\mathit{\boldsymbol{\alpha}}, \lambda, \psi_1, \bar F(x))$ with respect to $\alpha_i$, we get

That is, $J_3(\mathit{\boldsymbol{\alpha}}, {\lambda}, \psi_1, \bar F(x))$ is decreasing in $\alpha_i$. Furthermore, for $i\not = j,$ it holds that

where

Since the log-concavity of $\psi_1$ implies the increasing property of ${\psi_1}/{\psi_1'}$, and consider that $\phi_1({1-(\bar F(x))^{\lambda}}/{(1 -\bar \alpha(\bar F(x))^{\lambda})})$ is increasing in $\alpha,$ then we have

is negative and is increasing in $\alpha$ for every fixed $x\ge0$. In addition, ${1}/{(1 -\bar\alpha(\bar F(x))^{\lambda})}\ge0$ is decreasing in $\alpha$. Consequently, $h_3(\alpha)$ is increasing in $\alpha$ which in turn implies that

Therefore, $J_3(\mathit{\boldsymbol{\alpha}}, {\lambda}, \psi_1, \bar F(x))$ is decreasing in ${\alpha_i}$ and Schur-convex in $\mathit{\boldsymbol{\alpha}}.$ By Lemma 2, $\mathit{\boldsymbol{\alpha}}\overset{w}\preceq \mathit{\boldsymbol{\beta}}$ implies $J_3(\mathit{\boldsymbol{\alpha}}, {\lambda}, \psi_1, \bar F(x))\le J_3(\mathit{\boldsymbol{\beta}}, {\lambda}, \psi_1, \bar F(x)),$ and note that $\phi_2 \circ \psi_1$ is super-additive, we can conclude by Lemma 3 that $J_3(\mathit{\boldsymbol{\beta}}, {\lambda}, \psi_1, \bar F(x)) \le J_3(\mathit{\boldsymbol{\beta}}, {\lambda}, \psi_2, \bar F(x))$. Hence,

which implies that $\ X_{n:n}\ge_{st}Y_{n:n}.$ The proof can be completed.

The following numerical example is provided to demonstrate the theoretical result of Theorem 8.

Example 6. Let $\bar F(x) = e^{-(a x)^b}, a > 0$, $0 < b\le1$, and generators $\psi_1(x) = e^{-{x}}$, $\psi_2(x) = (\theta {x}+1)^{-1/\theta}$, $\theta > 0.$ Take $n = 3, \lambda = 0.8, a = 0.5, b = 0.2, \theta = 2$ and $\mathit{\boldsymbol{\alpha}} = (0.3, 0.5, 0.7)\overset{w}\preceq(0.2, 0.4, 0.6) = \mathit{\boldsymbol{\beta}}.$ One can check chat the conditions of Theorem 8 are satisfied. The survival functions of ${({X_{3:3}+1})^{-1}}$ and ${({Y_{3:3}+1})^{-1}}$ are plotted in Figure 7, that is $X_{3:3}\ge_{st}Y_{3:3}$, which verifies the result of Theorem 8.

In the next theorem, we provide a sufficient condition to obtain the reversed hazard rate order between two modified proportional hazard rates models when the modified proportional hazard rates parameters are equal and the tilt parameters are heterogeneous.

Theorem 9. For $\mathit{\boldsymbol{X}} \sim MPHR (\mathit{\boldsymbol{\alpha}}; \lambda; \bar F; \psi)$ and $\mathit{\boldsymbol{Y}} \sim MPHR (\mathit{\boldsymbol{\beta}}; \lambda; \bar F; \psi)$ with log-concave $\psi$. Assume that $-{\psi'}/{\psi}$ is log-convex. If $\mathit{\boldsymbol{\alpha}}\overset{w}\preceq \mathit{\boldsymbol{\beta}}$, then

Proof. The distribution function $X_{n:n}$ is given by

and the reversed hazard rate function of $X_{n:n}$ is

Similiarly, $Y_{n:n}$ gets the reversed hazard rate function $r_{Y_{n:n}}(x) = L_2(x, \mathit{\boldsymbol{\beta}}, \lambda, \psi)$ for $x \ge 0.$ Further denote

Note that, for $s, t \in \mathcal I_n$ with $s\not = t$, and $u_i\in[0, 1]$ ($i\in \mathcal I_n$), we have

By $\varrho1$, $\varrho2$ and $C_1(\boldsymbol \alpha, x)\ge C_1(\alpha_s\mathit{\boldsymbol{e}}_s, x)$, it holds that

As a result, $L_2(x, \mathit{\boldsymbol{\alpha}}, \lambda, \psi)$ is increasing in $\alpha_i,$ for any $i\in\mathcal I_n$, and for $s, t \in \mathcal I_n$ with $s\not = t$,

Since $h_3(\alpha)$ is increasing in $\alpha$, it holds that

Obviously, for $\alpha_s\ge \alpha_t,$

Note that ${1}/{(1-\bar\alpha_i(\bar F(x))^{\lambda})^2}\ge0$ is decreasing in $\alpha_i$, $\varrho2$ implies that $B_1(\alpha_i\mathit{\boldsymbol{e}}_i, x)$ is increasing in $\alpha_i$, and $B_1(\alpha_t\mathit{\boldsymbol{e}}_t, x)\le B_1(\alpha_s\mathit{\boldsymbol{e}}_s, x)\le 0$ for $\alpha_s\ge \alpha_t$. Then, we have

Since ${\alpha_i}/{(1-\bar\alpha_i(\bar F(x))^{\lambda})}$ and ${h_3(\alpha_i)}$ are increasing in $\alpha_i$, and by $\varrho3$, we have $C_1(\boldsymbol \alpha, x)-C_1(\alpha_t\mathit{\boldsymbol{e}}_t, x)\ge C_1(\boldsymbol \alpha, x)- C_1(\alpha_s\mathit{\boldsymbol{e}}_s, x)\ge0$ for $\alpha_s\ge \alpha_t$. It is verified that

By (4.1)-(4.4), it is plain that $L_2(x, \mathit{\boldsymbol{\alpha}}, \lambda, \psi)$ is increasing in $\alpha_i$ and Schur-concave with respect to $\mathit{\boldsymbol{\alpha}}.$ According to Lemma 1 and Lemma 2, $\mathit{\boldsymbol{\alpha}}\overset{w}\preceq \mathit{\boldsymbol{\beta}}$ implies $-r_{X_{n:n}}(x) = -L_2(x, \mathit{\boldsymbol{\alpha}}, {\lambda}, \psi) \le -L_2(x, \mathit{\boldsymbol{\beta}}, {\lambda}, \psi) = -r_{Y_{n:n}}(x)$ for all $x$, that is, $\ X_{n:n}\ge_{rh} Y_{n:n}.$ This completes the proof.

Remark 5. Theorem 9 extends Theorem 3.4 (i) of ^[6] to the case of dependent samples with Archimedean survival copulas.

The following example illustrates the result of Theorem 9.

Example 7. Let $\bar F = e^{-(a x)^b}, a > 0, 0 < b\le 1$, and $\psi(x) = e^{\frac{1-e^{x}}{\theta}}$, $0 < \theta\le 0.5(3-\sqrt5).$ Set $n = 3, \lambda = 3, a = 6, b = 0.08, \theta = 0.3$ and $\mathit{\boldsymbol{\alpha}} = (0.8, 0.6, 0.4)\overset{w}\preceq(0.7, 0.5, 0.3) = \mathit{\boldsymbol{\beta}}$. These satisfy all conditions of Theorem 9, and the hazard rate functions of ${({X_{3:3}+1})^{-1}}$ and ${({Y_{3:3}+1})^{-1}}$ are displayed in Figure 8, which confirms that $\ X_{3:3}\ge_{rh} Y_{3:3}.$

4.2.   On samples of MPRHR

In this subsection, we consider the case of MPRHR samples. The first two theorems present the comparison results on the heterogeneity among parameters in terms of the usual stochastic order. The proofs can be completed in a similar way with that of Theorem 1, and thus are omitted.

Theorem 10. For $\mathit{\boldsymbol{X}} \sim MPRHR(\alpha; \mathit{\boldsymbol{\beta}}; F; \psi_1)$ and $\mathit{\boldsymbol{Y}} \sim MPRHR(\alpha; \mathit{\boldsymbol{\gamma}}; F; \psi_2)$, where $0 < \alpha\le1$. If $\psi_1$ or $\psi_2$ is log-concave, and $\phi_2 \circ \psi_1$ is super-additive, then $\mathit{\boldsymbol{\beta}}\overset{w}\preceq \mathit{\boldsymbol{\gamma}}$ implies

Proof. The distribution function of $X_{n:n}$ can be represented as

Suppose that $\psi_1$ is log-concave. To establish the desired result, along with Lemma 2, it suffices to show that for fixed $x\ge0$ and $0 < \alpha\le1$. $J_4({\alpha}, \mathit{\boldsymbol{\beta}}, \psi_1, F(x))$ is decreasing in ${\beta_i}$ and Schur-convex in $\mathit{\boldsymbol{\beta}}$. The partial derivative of $J_4({\alpha}, \mathit{\boldsymbol{\beta}}, \psi_1, F(x))$ with respect to ${\beta_i}$ is

Which clearly shows that $J_4({\alpha}, \mathit{\boldsymbol{\beta}}, \psi_1, F(x))$ is decreasing in $\beta_i$. Now, for $i\not = j,$ we get

where

As dicussed in the proof of Theorem 1, for each fixded $x > 0$, we have

Moreover, we readily observe that ${1}/{(1 -\bar\alpha(F(x))^{\beta})}$ is nonnegative and decreasing in $\beta$ for $0 < \alpha\le1$. Upon combining these observations, we find $h_4(\beta)$ to be increasing in $\beta$. Consequently

Therefore, Schur-convexity of $J_4({\alpha}, \mathit{\boldsymbol{\beta}}, \psi_1, F(x))$ follows from Lemma 1. According to Lemma 2, $\mathit{\boldsymbol{\beta}}\overset{w}\preceq \mathit{\boldsymbol{\gamma}}$ implies $J_4({\alpha}, \mathit{\boldsymbol{\beta}}, \psi_1, F(x)) \le J_4({\alpha}, \mathit{\boldsymbol{\gamma}}, \psi_1, F(x))$, and the assumption $\phi_2 \circ \psi_1$ is super-additive, by Lemma 3, we have $J_4({\alpha}, \mathit{\boldsymbol{\gamma}}, \psi_1, F(x)) \le J_4({\alpha}, \mathit{\boldsymbol{\gamma}}, \psi_2, F(x))$. So, we obtain

It is clear that we conclude $F_{X_{n:n}}(x)\le F_{Y_{n:n}}(x)$, that is, $\ X_{n:n}\ge_{st}Y_{n:n}.$ For the case of $\psi_2$ is log-concave, the proof can be obtained in a similar way. This completes the desired proof.

Remark 6. Theorem 10 generalizes the result of Theorem 5.1 (ii) of ^[21] to the case of MPHR model.

Theorem 11. For $\mathit{\boldsymbol{X}} \sim MPRHR (\mathit{\boldsymbol{\alpha}}; \beta; F; \psi_1)$ and $\mathit{\boldsymbol{Y}} \sim MPRHR (\mathit{\boldsymbol{\upsilon}}; \beta; F; \psi_2)$. If $\phi_1 \circ \psi_2$ is super-additive, then $\mathit{\boldsymbol{\alpha}}\overset{w}\preceq \mathit{\boldsymbol{\upsilon}}$ implies

The following example is provided to illustrate the Theorem 11.

Example 8. Under the setup of Example 2, take $n = 3, \lambda = 0.8, a = 0.5, b = 0.4, \theta = 0.6$ and $\mathit{\boldsymbol{\alpha}} = (0.4, 0.5, 0.6)\overset{w}\preceq(0.3, 0.4, 0.5) = \mathit{\boldsymbol{\upsilon}}.$ These satisfy all conditions of Theorem 11, and the survival functions of ${({X_{3:3}+1})^{-1}}$ and ${({Y_{3:3}+1})^{-1}}$ are poltted in Figure 9, which asserts ${X_{3:3}} \le_{st}{Y_{3:3}}$.

Now, we present comparison result of sample having common modified proportional reversed hazard rates parameters and heterogeneous tilt parameters in terms of the reversed hazard rate order.

Theorem 12. For $\mathit{\boldsymbol{X}} \sim MPRHR (\mathit{\boldsymbol{\alpha}}; \beta; F; \psi)$ and $\mathit{\boldsymbol{Y}} \sim MPRHR (\mathit{\boldsymbol{\upsilon}}; \beta; F; \psi)$ with log-concave $\psi$, and $-{\psi'}/{\psi}$ is log-convex. If $\boldsymbol \alpha \overset{w}\preceq \boldsymbol \upsilon$, then

Remark 7. It should be pointed out that Theorem 12 extends Theorem 4.5 (i) of ^[6] to the case of dependent samples with Archimedean copulas.

Remark 8. Similarly, according to the Remark 2, based on Archimedean copula $K$, we obtained the distorted function representations of parallel system as follows:

where $h_5(u; \boldsymbol \alpha, \boldsymbol \lambda)$ and $h_6(u; \boldsymbol \alpha, \boldsymbol \beta)$ are obtained by MPHR and MPRHR models. In $(4.5)$, when $\alpha_i = \alpha(i = 1, \dots, n),$ we can obtain representation of Theorem 7, if $\lambda_i = \lambda (i = 1, \dots, n)$, we can obtain representation of Theorem 8 and Theorem 9. As for in other Theorem 10, Theorem 11, and Theorem 12, we can obtain in similar way by $(4.6)$.

5.   Conclusion

In this paper, we study stochastic comparisons on minimums and maximums from heterogeneous MPRHR (MPHR) samples with Archimedean (survival) copulas. Some ordering results are established for the usual stochastic, hazard rate and reversed rate orders on the smallest and largest order statistics. These results generalize some known results in the literature. As a further study, it is of interest to consider other stochastic orders such as likelihood ratio order, star order and dispersive order.

Acknowledgments

The authors would like to thank the two anonymous reviewers and the Editor for their valuable and constructive comments and suggestions to improve the presentation of this manuscript. This research was supported by the National Natural Science Foundation of China (11861058, 71471148).

Conflict of interest

The authors declare no conflict of interest.