Explicit solutions to the Sharma-Tasso-Olver equation

Mohammed Aly Abdou; Loubna Ouahid; Saud Owyed; A. M. Abdel-Baset; Mustafa Inc; Mehmet Ali Akinlar; Yu-Ming Chu; Mohammed Aly Abdou; Loubna Ouahid; Saud Owyed; A. M. Abdel-Baset; Mustafa Inc; Mehmet Ali Akinlar; Yu-Ming Chu

doi:10.3934/math.2020465

AIMS Mathematics

2020, Volume 5, Issue 6: 7272-7284. doi: 10.3934/math.2020465

Previous Article Next Article

Research article

Explicit solutions to the Sharma-Tasso-Olver equation

1.
Physics Department, College of Science, University of Bisha, Bisha 61922, P. O Box 344, Kingdom of Saudi Arabia
2.
Theoretical Research Group, Physics Department, Faculty of Science, Mansoura University, 35516 Mansoura, Egypt
3.
Mathematics Department, College of Science, University of Bisha, Bisha 61922, P. O Box 344, Kingdom of Saudi Arabia
4.
Physics Department, Assiut University, Assiut 71516, Egypt
5.
Department of Mathematics, Firat University, Elazig, Turkey
6.
Department of Medical Research, China Medical University Hospital, China Medical University, Taichung, Taiwan
7.
Department of Mathematical Engineering, Yildiz Technical University, Istanbul, Turkey
8.
Department of Mathematics, Huzhou University, Huzhou 313000, China
9.
Hunan Provincial Key Laboratory of Mathematical Modeling and Analysis in Engineering, Changsha University of Science & Technology, Changsha 410114, China

Received: 01 May 2020 Accepted: 10 September 2020 Published: 17 September 2020
MSC : 35A09, 35E05

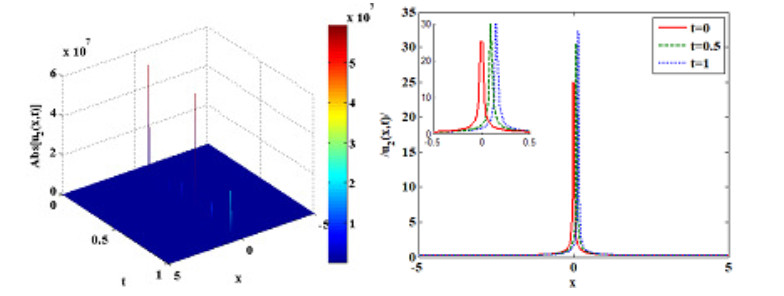
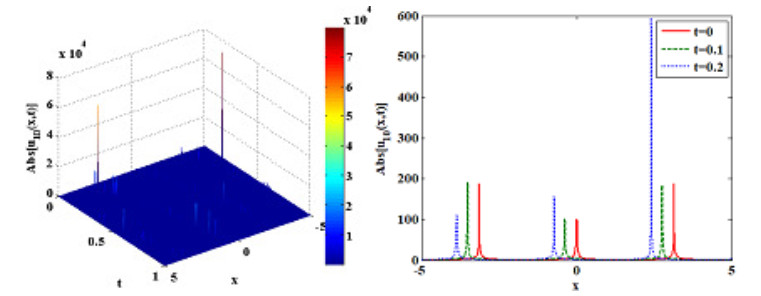
We present new exact traveling wave solutions of generalized Sharma-Tasso-Olver (STO) with variable coefficients using three different methods, namely the extended F-expansion, the new sub-equations, and generalized Kudryashov expansion. We obtain new solutions with the form of solitons, triangular and rational functions. Computational results indicate that these methods are very useful and easily applicable for solving diverse types of differential equations in nonlinear science.

Keywords:

extended F-expansion,
new sub-equations,
generalized Kudryashov expansion method,
Sharma-Tasso-Olver equation

Citation: Mohammed Aly Abdou, Loubna Ouahid, Saud Owyed, A. M. Abdel-Baset, Mustafa Inc, Mehmet Ali Akinlar, Yu-Ming Chu. Explicit solutions to the Sharma-Tasso-Olver equation[J]. AIMS Mathematics, 2020, 5(6): 7272-7284. doi: 10.3934/math.2020465

Related Papers:

[1]	Yunmei Zhao, Yinghui He, Huizhang Yang . The two variable (φ/φ, 1/φ)-expansion method for solving the time-fractional partial differential equations. AIMS Mathematics, 2020, 5(5): 4121-4135. doi: 10.3934/math.2020264
[2]	Emad H. M. Zahran, Ahmet Bekir, Reda A. Ibrahim, Ratbay Myrzakulov . The new soliton solution types to the Myrzakulov-Lakshmanan-XXXII-equation. AIMS Mathematics, 2024, 9(3): 6145-6160. doi: 10.3934/math.2024300
[3]	Ghazala Akram, Saima Arshed, Maasoomah Sadaf, Hajra Mariyam, Muhammad Nauman Aslam, Riaz Ahmad, Ilyas Khan, Jawaher Alzahrani . Abundant solitary wave solutions of Gardner's equation using three effective integration techniques. AIMS Mathematics, 2023, 8(4): 8171-8184. doi: 10.3934/math.2023413
[4]	J. Vanterler da C. Sousa, E. Capelas de Oliveira, L. A. Magna . Fractional calculus and the ESR test. AIMS Mathematics, 2017, 2(4): 692-705. doi: 10.3934/Math.2017.4.692
[5]	M. A. Habib, H. M. Shahadat Ali, M. Mamun Miah, M. Ali Akbar . The generalized Kudryashov method for new closed form traveling wave solutions to some NLEEs. AIMS Mathematics, 2019, 4(3): 896-909. doi: 10.3934/math.2019.3.896
[6]	Yaya Wang, Md Nurul Raihen, Esin Ilhan, Haci Mehmet Baskonus . On the new sine-Gordon solitons of the generalized Korteweg-de Vries and modified Korteweg-de Vries models via beta operator. AIMS Mathematics, 2025, 10(3): 5456-5479. doi: 10.3934/math.2025252
[7]	Hammad Alotaibi . Solitary waves of the generalized Zakharov equations via integration algorithms. AIMS Mathematics, 2024, 9(5): 12650-12677. doi: 10.3934/math.2024619
[8]	Chaudry Masood Khalique, Oke Davies Adeyemo, Kentse Maefo . Symmetry solutions and conservation laws of a new generalized 2D Bogoyavlensky-Konopelchenko equation of plasma physics. AIMS Mathematics, 2022, 7(6): 9767-9788. doi: 10.3934/math.2022544
[9]	Abdulah A. Alghamdi . Analytical discovery of dark soliton lattices in (2+1)-dimensional generalized fractional Kundu-Mukherjee-Naskar equation. AIMS Mathematics, 2024, 9(8): 23100-23127. doi: 10.3934/math.20241123
[10]	Elsayed M. E. Zayed, Mona El-Shater, Khaled A. E. Alurrfi, Ahmed H. Arnous, Nehad Ali Shah, Jae Dong Chung . Dispersive optical soliton solutions with the concatenation model incorporating quintic order dispersion using three distinct schemes. AIMS Mathematics, 2024, 9(4): 8961-8980. doi: 10.3934/math.2024437

Abstract

1. Introduction

Research on exact solutions of nonlinear differential equations with variable coefficients has been a significant area for recent decades, see e.g. ^{[1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25]}. We consider nonlinear STO equation with variable coefficients ^[26,27].

$\begin{equation} u_{t} +f\left(t\right)\left(uu_{x} +\frac{1}{3} u^{3} \right)_{x} +g\left(t\right)u_{xxx} = 0, \end{equation}$

(1.1)

In which $f\left(t\right)\ne 0$ , $g\left(t\right)\ne 0$ are functions of $t$ . In the scientific literature there are a various number of effective methods for the exact solutions of nolinear PDEs. Among these methods, similarity reduction ^[1], Adomian decomposition ^[13], Backlund transformation ^[2], Painleve expansion ^[3], homogeneous balance ^[15], Jacobi elliptic function ^[5,6], tanh function ^[16], F-expansion ^{[17,18,19,20]}, variational iteration ^[9,10,11,12], homotopy analysis ^[14] and Exp-function ^[21,22,23]. Riemann-Hilbert method ^{[28,29,30,31]}, Lie symmetry ^[32], Hirota bilinear ^[33], Darboux method ^[34], variable-coefficient fractional Y-expansion method ^[35], Riccati equation method ^[36], fractional riccati method ^[37], fractional dual-function method ^[38]. Noether symmetries ^[39], Kudryashov method ^[40,41], Simplest equation method ^[42].

In order to study the traveling wave propagation solution of STO ^[43,44], let us introduce:

$\begin{equation} \zeta = x+\frac{\omega }{\alpha } \int _{0}^{t}g\left(t'\right) dt', \; u\left(x, t\right) = u\left(\zeta \right), \end{equation}$

(1.2)

in which $\alpha$ is a parameter and $\omega$ is wave speed. By Eq (1.2), Eq (1.1) is written

$\begin{equation} \frac{\omega }{\alpha } u_{\zeta } +u_{\zeta \zeta \zeta } +3\left(uu_{\zeta } +\frac{1}{3} u^{3} \right)_{\zeta } = 0, \end{equation}$

(1.3)

in which $f\left(t\right)$ and $g\left(t\right)$ satisfy $f\left(t\right) = 3g\left(t\right)$ . Integrating Eq (1.3), we get

$\begin{equation} \frac{\omega }{\alpha } u+u_{\zeta \zeta } +3\left(uu_{\zeta } +\frac{1}{3} u^{3} \right) = 0. \end{equation}$

(1.4)

In this study we get solitary wave and the periodic wave solutions by using algebraic direct method, Sub-equations method and F-expansion method. In the next two sections, the new proposed methods are presented and different types of exact solutions of STO are written down. Section 4 is devoted to the conclusion.

2. Methodology

Let Z be a polynomial function of $x$ , and $t$ . Consider the nonlinear PDE

$\begin{equation} Z\left(u, u_{x} , u_{t} , u_{xx, ...} \right) = 0. \end{equation}$

(2.1)

Let

$\begin{equation} u\left(x, t\right) = u\left(\zeta \right), \zeta = k\left(x+\lambda t\right), \end{equation}$

(2.2)

where $k$ , $\lambda$ are constants. Inserting Eq (2.2) into Eq (2.1), we get the ODE in terms of $u\left(\xi \right)$

$\begin{equation} \chi \left(u, ku', \lambda ku', k^{2} u'', ...\right) = 0. \end{equation}$

(2.3)

2.1. Extended F-Expansion method

Let the solution be written as

$\begin{equation} u\left(\zeta \right) = a_{0} +\sum\limits _{i = -M}^{M}a_{i} \chi ^{i} \left(\zeta \right), \end{equation}$

(2.4)

in which $a_{0}$ and $a_{i}$ are constants, and $M\ne 0$ is a natural number and $\chi \left(\zeta \right)$ satisfies

$\begin{equation} \chi '\left(\zeta \right) = A+B\chi \left(\zeta \right)+C\chi ^{2} \left(\zeta \right), \end{equation}$

(2.5)

where, $\chi '\left(\zeta \right) = \frac{d\chi }{d\zeta }$ and $A, \, B, \, C$ are parameters.

In order to solve Eq (1.4) via F-expansion method, equating $u_{\xi \xi }$ with $u^{3}$ yields $M = 1$ . Hence, Eq (2.4) reads

$\begin{equation} u\left(\zeta \right) = a_{0} +a_{1} \chi \left(\zeta \right)+\frac{a_{-1} }{\chi \left(\zeta \right)} , \end{equation}$

(2.6)

in which $a_{0}$ , $a_{1}$ and $a_{-1}$ are constants. Inserting Eq (2.6) into the reduced Eq (1.4) yields:

Case (1.1): $a_{-1} = 0$ , $a_{1} = 1$ , $a_{0} = -1$ , $\alpha = \alpha$ and $\omega = -\alpha$ . Using the transformation (1.2), the corresponding solution in terms of the original coordinates is as follows

$\begin{equation} u_{1} \left(x, t\right) = -\frac{1}{2} +\frac{1}{4} \tanh \left(x-\int _{0}^{t}g\left(t'\right) dt'\right) \end{equation}$

(2.7)

where $g\left(t\right)$ is an arbitrary function.

Figure 1. (a) Three-dimensional mesh of

$Abs[u_{1} \left(x, t\right)]$ versus

$t$ and

$x$ , (b) variation

$Abs[u_{1} \left(x, t\right)]$ with the normalized propagation position

$x$ for different values of the time.

DownLoad: Full-Size Img PowerPoint

Case (1.2): $a_{-1} = 0$ , $a_{1} = -1$ , $a_{0} = \frac{1}{2}$ and $\omega = -\frac{\alpha }{4}$ . Using the transformation (1.2), the corresponding solution in terms of the original coordinates is as follows

$\begin{equation} u_{2} \left(x, t\right) = \frac{1}{4} \coth \left(x-\frac{1}{4} \int _{0}^{t}g\left(t'\right) dt'\right), \end{equation}$

(2.8)

where $g\left(t\right)$ is an arbitrary function.

Figure 2. (a) Three-dimensional mesh of

$Abs[u_{2} \left(x, t\right)]$ versus

$t$ and

$x$ , (b) variation

$Abs[u_{2} \left(x, t\right)]$ with the normalized propagation position

$x$ for different values of the time.

DownLoad: Full-Size Img PowerPoint

Case (1.3): $a_{-1} = \frac{1}{2}$ , $a_{1} = \frac{1}{2}$ , $a_{0} = -1$ , $\alpha = \alpha$ and $\omega = -4\alpha$ . Using the transformation (1.2), the corresponding solutions in terms of the original coordinates is

$\begin{equation} \begin{array}{l} {u_{3} \left(x, t\right) = -1+\frac{1}{2\left[\coth \left(x-4\int _{0}^{t}g\left(t'\right) dt'\right)\pm \csc h\left(x-4\int _{0}^{t}g\left(t'\right) dt'\right)\right]} } \\ {{\rm \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; }+\frac{1}{2} \left[\coth \left(x-4\int _{0}^{t}g\left(t'\right) dt'\right)\pm \csc h\left(x-4\int _{0}^{t}g\left(t'\right) dt'\right)\right]} \end{array} , \end{equation}$

(2.9)

$\begin{equation} \begin{array}{l} {u_{4} \left(x, t\right) = -1+\frac{1}{2\left[\tanh \left(x-4\int _{0}^{t}g\left(t'\right) dt'\right)\pm i\sec h\left(x-4\int _{0}^{t}g\left(t'\right) dt'\right)\right]} } \\ {{\rm \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; }+\frac{1}{2} \left[\tanh \left(x-4\int _{0}^{t}g\left(t'\right) dt'\right)\pm i\sec h\left(x-4\int _{0}^{t}g\left(t'\right) dt'\right)\right]} \end{array}, \end{equation}$

(2.10)

where $g\left(t\right)$ is an arbitrary function.

Case (1.4): $a_{-1} = 1$ , $a_{1} = 1$ , $a_{0} = -2$ , $\alpha = \alpha$ and $\omega = -16\alpha$ . From the transformation (1.2), the corresponding solution in terms of the original coordinates is as follows

$\begin{equation} u_{5} \left(x, t\right) = -2+\coth \left(x-16\int _{0}^{t}g\left(t'\right) dt'\right)+\tanh \left(x-16\int _{0}^{t}g\left(t'\right) dt'\right), \end{equation}$

(2.11)

where $g\left(t\right)$ is an arbitrary function.

Case (2.1): $a_{-1} = 1$ , $a_{1} = -1$ , $a_{0} = 2i$ , $\alpha = \alpha$ and $\omega = 16\alpha$ . Using the transformation (1.2), the corresponding solution in terms of the original coordinates is taken as

$\begin{equation} u_{6} \left(x, t\right) = 2i+\cot \left(x+16\int _{0}^{t}g\left(t'\right) dt'\right)-\tanh \left(x+16\int _{0}^{t}g\left(t'\right) dt'\right), \end{equation}$

(2.12)

where $g\left(t\right)$ is an arbitrary function.

Figure 3. (a) Three-dimensional mesh of

$Abs[u_{6} \left(x, t\right)]$ versus

$t$ and

$x$ , (b) variation

$Abs[u_{6} \left(x, t\right)]$ with the normalized propagation position

$x$ for different values of the time.

DownLoad: Full-Size Img PowerPoint

Case (2.2): $a_{-1} = A$ , $a_{1} = 0$ , $a_{0} = \frac{B}{2}$ , $\alpha = \alpha$ and $\omega = -\frac{B^{2} \alpha }{4}$ . By means of Eq (1.2), the corresponding solution in terms of the original coordinates gives

$\begin{equation} u_{7} \left(x, t\right) = \frac{B}{2} +\frac{AB}{\exp \left\{B\left[x-\frac{B^{2} }{4} \int _{0}^{t}g\left(t'\right) dt'\right]\right\}-A} , \end{equation}$

(2.13)

where $g\left(t\right)$ is an arbitrary function.

Case (2.3): $a_{-1} = -1$ , $a_{1} = 1$ , $a_{0} = 2i$ , $\alpha = \alpha$ and $\omega = 16\alpha$ . Using the transformation (1.2), the corresponding solution in terms of the original coordinates admits to

$\begin{equation} u_{8} \left(x, t\right) = 2i-\frac{1}{\cot \left(x+16\int _{0}^{t}g\left(t'\right) dt'\right)} +\cot \left(x+16\int _{0}^{t}g\left(t'\right) dt'\right), \end{equation}$

(2.14)

where $g\left(t\right)$ is an arbitrary function.

Case (2.4): $a_{-1} = \frac{1}{2}$ , $a_{1} = -\frac{1}{2}$ , $a_{0} = \pm i$ , $\alpha = \alpha$ and $\omega = 4\alpha$ . Making use the transformation (1.2), the corresponding solutions in terms of the original coordinates yields

$\begin{equation} \begin{array}{l} {u_{9} \left(x, t\right) = \pm i+\frac{1}{2\left[\sec \left(x+4\int _{0}^{t}g\left(t'\right) dt'\right)+\tan \left(x+4\int _{0}^{t}g\left(t'\right) dt'\right)\right]} } \\ {{\rm \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; }-\frac{1}{2} \left[\sec \left(x+4\int _{0}^{t}g\left(t'\right) dt'\right)+\tan \left(x+4\int _{0}^{t}g\left(t'\right) dt'\right)\right]} \end{array}, \end{equation}$

(2.15)

$\begin{equation} \begin{array}{l} {u_{10} \left(x, t\right) = \pm i+\frac{1}{2\left[\csc \left(x+4\int _{0}^{t}g\left(t'\right) dt'\right)-\cot \left(x+4\int _{0}^{t}g\left(t'\right) dt'\right)\right]} } \\ {{\rm \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; }-\frac{1}{2} \left[\csc \left(x+4\int _{0}^{t}g\left(t'\right) dt'\right)-\cot \left(x+4\int _{0}^{t}g\left(t'\right) dt'\right)\right]} \end{array}, \end{equation}$

(2.16)

where $g\left(t\right)$ is an arbitrary function.

Figure 4. (a) Three-dimensional mesh of

$Abs[u_{10} \left(x, t\right)]$ versus

$t$ and

$x$ , (b) variation

$Abs[u_{10} \left(x, t\right)]$ with the normalized propagation position

$x$ for different values of the time.

DownLoad: Full-Size Img PowerPoint

2.2. New sub-equation method

In view this method (MAE) ^[24], affirms the general solution as the form as

$\begin{equation} u\left(\varsigma \right) = a_{0} +\sum\limits _{j = 1}^{N}a_{j} A^{j\, f\left(\varsigma \right)} +\sum\limits _{j = 1}^{N}b_{j} A^{-j\, f\left(\varsigma \right)} , \end{equation}$

(2.17)

The parameters $a_{j}$ , $b_{j}$ are arbitrary constants and $f\left(\varsigma \right)$ satisfy the following auxiliary equation

$\begin{equation} f'\left(\varsigma \right) = \frac{\alpha +\beta A^{-\, f\left(\varsigma \right)} +\sigma A^{f\left(\varsigma \right)} }{\ln \left(A\right)} , \end{equation}$

(2.18)

in which $\alpha$ , $\beta$ , $\sigma$ are arbitrary constants and $A > 0$ , $A\ne 1$ .

To solve Eq (1.4), we employ Eq (2.17) to get solutions taking into consideration the homogeneous balance between $u^{3}$ and $u''$ in Eq (1.4) that results N = 1. Set N = 1 in Eq (2.17), we get

$\begin{equation} u\left(\varsigma \right) = a_{0} +a_{1} A^{f\left(\varsigma \right)} +b_{1} A^{-f\left(\varsigma \right)} , \end{equation}$

(2.19)

According to (MAE) method, writing Eq (2.19) in Eq (1.4) with the help of Eq (2.18), we get

Case 1: $\left\{w = \left(4\alpha \sigma -\beta ^{2} \right)\delta, \; \delta = \delta, \; a_{0} = -\frac{1}{2} \beta \pm \frac{1}{2} \sqrt{\beta ^{2} -4\alpha \sigma }, \; a_{1} = -\sigma, \; b_{1} = 0\right\}$

Case 2: $\left\{w = -\frac{1}{4} \delta \beta ^{2} +\delta \alpha \sigma, \; \delta = \delta, \; a_{0} = \frac{1}{2} \beta, \; \; a_{1} = 0, \; b_{1} = \alpha \right\}$

Case 3: $\left\{w = -\delta \beta ^{2} +4\delta \alpha \sigma, \; \delta = \delta, \; a_{0} = \beta, \; \; a_{1} = 0, \; b_{1} = 2\alpha \right\}$

Case 4: $\left\{w = \left(4\alpha \sigma -\beta ^{2} \right)\delta, \; \delta = \delta, \; a_{0} = \frac{1}{2} \beta \pm \frac{1}{2} \sqrt{\beta ^{2} -4\alpha \sigma }, \; a_{1} = 0, \; b_{1} = \alpha \right\}$

Case 5: $\left\{w = -\delta \beta ^{2} +4\delta \alpha \sigma, \; \delta = \delta, \; a_{0} = 0, \; \; a_{1} = -\sigma, \; b_{1} = \alpha \right\}$

In view of case [1], exact solutions of Eq (1.1) are given a

when $\beta ^{2} -4\alpha \sigma < 0$ , and $\sigma \ne 0$ ,

$u_{1} \left(\varsigma \right) = -\frac{1}{2} \beta \pm \frac{1}{2} \sqrt{\beta ^{2} -4\alpha \sigma } -\sigma \frac{-\beta +\sqrt{4\alpha \sigma -\beta ^{2} } \tan \left(\frac{1}{2} \sqrt{4\alpha \sigma -\beta ^{2} } \zeta \right)}{2\sigma } ,$

$\begin{equation} u_{2} \left(\varsigma \right) = -\frac{1}{2} \beta \pm \frac{1}{2} \sqrt{\beta ^{2} -4\alpha \sigma } +\sigma \frac{\beta +\sqrt{4\alpha \sigma -\beta ^{2} } \cot \left(\frac{1}{2} \sqrt{4\alpha \sigma -\beta ^{2} } \zeta \right)}{2\sigma } . \end{equation}$

(2.20)

If $\beta ^{2} -4\alpha \sigma > 0$ , and $\sigma \ne 0$ , we have

$u_{3} \left(\varsigma \right) = -\frac{1}{2} \beta \pm \frac{1}{2} \sqrt{\beta ^{2} -4\alpha \sigma } +\sigma \frac{\beta +\sqrt{\beta ^{2} -4\alpha \sigma } \tanh \left(\frac{1}{2} \sqrt{4\alpha \sigma -\beta ^{2} } \zeta \right)}{2\sigma } ,$

$\begin{equation} u_{4} \left(\varsigma \right) = -\frac{1}{2} \beta \pm \frac{1}{2} \sqrt{\beta ^{2} -4\alpha \sigma } +\sigma \frac{\beta +\sqrt{\beta ^{2} -4\alpha \sigma } \tanh \left(\frac{1}{2} \sqrt{4\alpha \sigma -\beta ^{2} } \zeta \right)}{2\sigma } . \end{equation}$

(2.21)

If $\beta ^{2} -4\alpha \sigma = 0$ , and $\sigma \ne 0$ ,

$\begin{equation} u_{5} \left(\varsigma \right) = -\frac{1}{2} \beta \pm \frac{1}{2} \sqrt{\beta ^{2} -4\alpha \sigma } +\sigma \frac{2+\beta \zeta }{2\sigma \zeta } . \end{equation}$

(2.22)

Similarly as before, according to case [4], new exact solution s of Eq (1.1) is:

As long as $\beta ^{2} -4\alpha \sigma < 0$ , and $\sigma \ne 0$ , we have

$u_{6} \left(\varsigma \right) = \frac{1}{2} \beta \pm \frac{1}{2} \sqrt{\beta ^{2} -4\alpha \sigma } +\alpha \frac{2\sigma }{-\beta +\sqrt{4\alpha \sigma -\beta ^{2} } \tan \left(\frac{1}{2} \sqrt{4\alpha \sigma -\beta ^{2} } \zeta \right)} ,$

$\begin{equation} u_{7} \left(\varsigma \right) = \frac{1}{2} \beta \pm \frac{1}{2} \sqrt{\beta ^{2} -4\alpha \sigma } -\alpha \frac{2\sigma }{\beta +\sqrt{4\alpha \sigma -\beta ^{2} } \cot \left(\frac{1}{2} \sqrt{4\alpha \sigma -\beta ^{2} } \zeta \right)} . \end{equation}$

(2.23)

if $\beta ^{2} -4\alpha \sigma > 0$ and $\sigma \ne 0$ , admits to

$u_{8} \left(\varsigma \right) = \frac{1}{2} \beta \pm \frac{1}{2} \sqrt{\beta ^{2} -4\alpha \sigma } -\alpha \frac{2\sigma }{\beta +\sqrt{\beta ^{2} -4\alpha \sigma } \tanh \left(\frac{1}{2} \sqrt{\beta ^{2} -4\alpha \sigma } \zeta \right)} ,$

$\begin{equation} u_{9} \left(\varsigma \right) = \frac{1}{2} \beta \pm \frac{1}{2} \sqrt{\beta ^{2} -4\alpha \sigma } -\alpha \frac{2\sigma }{\beta +\sqrt{\beta ^{2} -4\alpha \sigma } \coth \left(\frac{1}{2} \sqrt{\beta ^{2} -4\alpha \sigma } \zeta \right)} . \end{equation}$

(2.24)

if $\beta ^{2} -4\alpha \sigma = 0$ and $\sigma \ne 0$ ,

$u_{10} \left(\varsigma \right) = \frac{1}{2} \beta \pm \frac{1}{2} \sqrt{\beta ^{2} -4\alpha \sigma } -\alpha \frac{2\sigma \zeta }{2+\beta \zeta } ,$

$\begin{equation} \zeta = x+\frac{\omega }{\alpha } \int _{0}^{t}g\left(t'\right) dt'. \end{equation}$

(2.25)

3. Generalized Kudryashov expansion method

In view this method ^[25], suppose that the solution of Eq (1.4) is written as:

$\begin{equation} u\left(\zeta \right) = \sum \limits_{i = -N}^{N}a_{i} \psi ^{i} \left(\zeta \right), \end{equation}$

(3.1)

where $a_{i}$ are constants to be calculated afterward and verifies:

$\begin{equation} \psi '\left(\varsigma \right) = \ln \left(A\right)\left[\alpha +\beta \psi \left(\varsigma \right)+\gamma \psi ^{2} \left(\varsigma \right)\right], \end{equation}$

(3.2)

where A, $\alpha$ , $\beta$ and $\gamma$ are constants.

Equating $u''\left(\varsigma \right)$ and $u^{3} \left(\varsigma \right)$ , we get N = 1, thus Eq (3.1) leads to:

$\begin{equation} u\left(\varsigma \right) = a_{0} +a_{1} \psi \left(\varsigma \right)+\frac{a_{-1} }{\psi \left(\varsigma \right)} , \end{equation}$

(3.3)

Now, we have:

Case [1]: $a_{0} = 0$ , $a_{1} = -\sigma \ln \left(A\right)$ , $a_{-1} = \alpha \ln \left(A\right)$

Case [2]: $a_{0} = \left(-\frac{\beta }{2} +\frac{\sqrt{\beta ^{2} -4\alpha \sigma } }{2} \right)\ln \left(A\right)$ , $a_{1} = -\sigma \ln \left(A\right)$ , $a_{-1} = 0$

In view of case [1], new exact travelling wave solutions of Eq (1.1) are

$\begin{equation} u_{i} (\zeta ) = -\sigma \ln \left(A\right)\psi _{i} \left(\zeta \right)+\frac{\alpha \ln \left(A\right)}{\psi _{i} \left(\zeta \right)} . \end{equation}$

(3.4)

According to case [2], exact solutions of Eq (1.1) are:

$\begin{equation} u_{i} (\zeta ) = \left(-\frac{\beta }{2} +\frac{\sqrt{\beta ^{2} -4\alpha \sigma } }{2} \right)\ln \left(A\right)-\sigma \ln \left(A\right)\psi _{i} \left(\zeta \right), \end{equation}$

(3.5)

where $\psi _{i} \left(\varsigma \right)$ is:

Family 1. In case of $\Delta = \beta ^{2} -4\alpha \sigma < 0$ , $\sigma \ne 0$ , $\psi _{i} \left(\varsigma \right)$ reads

$\begin{equation} \psi _{1} \left(\varsigma \right) = -\frac{\beta }{2\sigma } +\frac{\sqrt{-\Delta } }{2\sigma } \tan _{A} \left(\frac{\sqrt{-\Delta } }{2} \varsigma \right), \end{equation}$

(3.6)

$\begin{equation} \psi _{2} \left(\varsigma \right) = -\frac{\beta }{2\sigma } -\frac{\sqrt{-\Delta } }{2\sigma } \cot _{A} \left(\frac{\sqrt{-\Delta } }{2} \varsigma \right), \end{equation}$

(3.7)

$\begin{equation} \psi _{3} \left(\varsigma \right) = -\frac{\beta }{2\sigma } +\frac{\sqrt{-\Delta } }{4\sigma } \tan _{A} \left(\frac{\sqrt{-\Delta } }{4} \varsigma \right)-\frac{\sqrt{-\Delta } }{4\sigma } \cot _{A} \left(\frac{\sqrt{-\Delta } }{4} \varsigma \right), \end{equation}$

(3.8)

Family 2. In case of $\Delta = \beta ^{2} -4\alpha \sigma > 0$ , $\sigma \ne 0$ , $\psi _{i} \left(\varsigma \right)$ reads

$\begin{equation} \psi _{4} \left(\varsigma \right) = -\frac{\beta }{2\sigma } -\frac{\sqrt{\Delta } }{2\sigma } \tanh _{A} \left(\frac{\sqrt{\Delta } }{2} \varsigma \right), \end{equation}$

(3.9)

$\begin{equation} \psi _{5} \left(\varsigma \right) = -\frac{\beta }{2\sigma } -\frac{\sqrt{\Delta } }{2\sigma } \coth _{A} \left(\frac{\sqrt{\Delta } }{2} \varsigma \right), \end{equation}$

(3.10)

$\begin{equation} \psi _{6} \left(\varsigma \right) = -\frac{\beta }{2\sigma } -\frac{\sqrt{\Delta } }{2\sigma } \coth _{A} \left(\sqrt{\Delta } \varsigma \right)\pm \frac{\sqrt{pq\Delta } }{2\sigma } \csc h_{A} \left(\sqrt{\Delta } \varsigma \right), \end{equation}$

(3.11)

$\begin{equation} \psi _{7} \left(\varsigma \right) = -\frac{\beta }{2\sigma } -\frac{\sqrt{\Delta } }{2\sigma } \tanh _{A} \left(\sqrt{\Delta } \varsigma \right)\pm i\frac{\sqrt{pq\Delta } 2\sigma }{S} \sec h_{A} \left(\sqrt{\Delta } \varsigma \right), \end{equation}$

(3.12)

Family 3. In the limiting cas if $\alpha \sigma > 0$ , $\beta = 0$ , then

$\begin{equation} \psi _{8} \left(\varsigma \right) = \sqrt{\frac{\alpha }{\sigma } } \tan _{A} \left(\sqrt{\alpha \sigma } \varsigma \right), \end{equation}$

(3.13)

$\begin{equation} \psi _{9} \left(\varsigma \right) = -\sqrt{\frac{\alpha }{\sigma } } \cot _{A} \left(\sqrt{\alpha \sigma } \varsigma \right), \end{equation}$

(3.14)

$\begin{equation} \psi _{10} \left(\varsigma \right) = \sqrt{\frac{\alpha }{\sigma } } \tan _{A} \left(2\sqrt{\alpha \sigma } \varsigma \right)\pm \sqrt{pq\frac{\alpha }{\sigma } } \sec _{A} \left(2\sqrt{\alpha \sigma } \varsigma \right), \end{equation}$

(3.15)

$\begin{equation} \psi _{11} \left(\varsigma \right) = -\sqrt{\frac{\alpha }{\sigma } } \cot _{A} \left(2\sqrt{\alpha \sigma } \varsigma \right)\pm \sqrt{pq\frac{\alpha }{\sigma } } \csc _{A} \left(2\sqrt{\alpha \sigma } \varsigma \right), \end{equation}$

(3.16)

Family 4. when $\sigma = -\alpha$ , $\beta = 0$ , then

$\begin{equation} \psi _{12} \left(\varsigma \right) = -\tanh _{A} \left(\alpha \varsigma \right), \end{equation}$

(3.17)

$\begin{equation} \psi _{13} \left(\varsigma \right) = -\coth _{A} \left(\alpha \varsigma \right), \end{equation}$

(3.18)

$\begin{equation} \psi _{14} \left(\varsigma \right) = -\tanh _{A} \left(2\alpha \varsigma \right)\pm i\sqrt{pq} \sec h_{A} \left(2\alpha \varsigma \right), \end{equation}$

(3.19)

Family 5. when $\beta = k$ , $\sigma = mk$ , $\beta = \alpha = 0$ , $\beta = k$ , $\alpha = mk$ , $\sigma = 0$ , then

$\begin{equation} \psi _{16} \left(\varsigma \right) = \frac{pA^{k\varsigma } }{q-mpA^{k\varsigma } } , \end{equation}$

(3.20)

$\begin{equation} \psi _{17} \left(\varsigma \right) = \frac{-1}{\sigma \varsigma \ln \left(A\right)} , \end{equation}$

(3.21)

$\begin{equation} \psi _{18} \left(\varsigma \right) = A^{k\varsigma } -m, \end{equation}$

(3.22)

$\begin{equation} \zeta = x+\frac{\omega }{\alpha } \int _{0}^{t}g\left(t'\right) dt', \end{equation}$

(3.23)

where $\sinh _{A} \left(\varsigma \right) = \frac{pA^{\varsigma } -qA^{-\varsigma } }{2}$ , $\cosh _{A} \left(\varsigma \right) = \frac{pA^{\varsigma } +qA^{-\varsigma } }{2}$ , $\tanh _{A} \left(\varsigma \right) = \frac{pA^{\varsigma } -qA^{-\varsigma } }{pA^{\varsigma } +qA^{-\varsigma } }$ ,

$\begin{equation} \coth _{A} \left(\varsigma \right) = \frac{pA^{\varsigma } +qA^{-\varsigma } }{pA^{\varsigma } -qA^{-\varsigma } } , \tan _{A} \left(\varsigma \right) = -i\frac{pA^{i\varsigma } -qA^{-i\varsigma } }{pA^{i\varsigma } +qA^{-i\varsigma } } , \cot _{A} \left(\varsigma \right) = i\frac{pA^{i\varsigma } +qA^{-i\varsigma } }{pA^{i\varsigma } -qA^{-i\varsigma } } . \end{equation}$

(3.24)

4. Conclusions

Methods of the extended sub-equation, direct algebraic and F-expansion have been successfully applied to solve the variable coefficient STO equation with its fission and fusion. Using the F-expansion method, one may able to classify ten types of solutions in terms of the arbitrary function $g\left(t\right)$ . The advantage of the presence of that arbitrary function $g\left(t\right)$ , enable us to construct a wide range classes of solutions according to the different choices of $g\left(t\right)$ and any initial condition may be persuaded.

On the other hand, using different mathematical methods may lead us to another type of solutions. For example, applying the improved tanh method, one obtains the following type of solution

$\begin{equation} u\left(x, t\right) = \pm \left(\sec \left(x+\alpha \int _{0}^{t}g\left(t'\right) dt'\right)\pm \tan \left(x+\alpha \int _{0}^{t}g\left(t'\right) dt'\right)\right), \end{equation}$

(4.1)

that maps to the triangular periodic solution where $\omega = \alpha$ . In addition, one may also obtain the numerous soliton like solutions,

$\begin{equation} u\left(x, t\right) = \pm \frac{1}{2} \frac{\tanh \left(x+\alpha \int _{0}^{t}g\left(t'\right) dt'\right)}{1\pm \sec h\left(x+\alpha \int _{0}^{t}g\left(t'\right) dt'\right)} . \end{equation}$

(4.2)

where $\omega = -\alpha$ and $g\left(t\right)$ is an arbitrary function of $t$ .

Application of these methods to fractal order PDEs may be seen in, e.g. ^{[25,26,27,45,46,47,48,49,50,51,52,53]}. We will investigate the applicability of these methods to fractional stochastic differential equations in a future work.

Appendix

(A, B, C) values and $F\left(\xi \right)$ in $F' = A+BF\left(\xi \right)+CF^{2} \left(\xi \right)$ .

$A$	$B$	$C$	$\chi \left(\zeta \right)$
$0$	$1$	$-1$	$\chi \left(\zeta \right) = \frac{1}{2} +\frac{1}{2} \tanh \left(\frac{\zeta }{2} \right)$
$0$	$-1$	$1$	$\chi \left(\zeta \right) = \frac{1}{2} -\frac{1}{2} \coth \left(\frac{\zeta }{2} \right)$
$\frac{1}{2}$	$0$	$-\frac{1}{2}$	$\chi \left(\zeta \right) = \coth \left(\zeta \right)\pm \csc h\left(\zeta \right)$ , $\tan \left(\zeta \right)\pm i\sec h\left(\zeta \right)$
$1$	$0$	$-1$	$\chi \left(\zeta \right) = \tanh \left(\zeta \right)$ , $\coth \left(\zeta \right)$
$\frac{1}{2}$	$0$	$\frac{1}{2}$	$\chi \left(\zeta \right) = \sec \left(\zeta \right)+\tan \left(\zeta \right)$ , $\csc \left(\zeta \right)-\cot \left(\zeta \right)$
$-\frac{1}{2}$	$0$	$-\frac{1}{2}$	$\chi \left(\zeta \right) = \sec \left(\zeta \right)-\tan \left(\zeta \right)$ , $\csc \left(\zeta \right)+\cot \left(\zeta \right)$
$1\left(-1\right)$	$0$	$1\left(-1\right)$	$\chi \left(\zeta \right) = \tan \left(\zeta \right)$ , $\cot \left(\zeta \right)$
$0$	$0$	$\ne 0$	$\chi \left(\zeta \right) = \frac{-1}{C\zeta +\lambda }$
Constant	$0$	$0$	$\chi \left(\zeta \right) = A\zeta$
Constant	$\ne 0$	$0$	$\chi \left(\zeta \right) = \frac{\exp \left(B\zeta \right)-A}{B}$

Acknowledgments

The work was supported by the Natural Science Foundation of China (Grant Nos. 61673169, 11301127, 11701176, 11626101, 11601485).

Conflict of interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.

References

[1]	R. Hirota, Exact solutions of the KdV equation for multiple collisions of solitons, Phys. Rev. Lett., 27 (1971), 1192-1-1192-3. doi: 10.1103/PhysRevLett.27.1192
[2]	M. Wadati, K. Konno, Simple derivation of Bucklund transformation from Riccati form of inverse method, Prog. Theor. Phys., 53 (1975), 1652-1656. doi: 10.1143/PTP.53.1652
[3]	F. Cariello, M. Tabor, Similarity reductions from extended Painlev expansions for nonintegrable evolution equations, Physica D: Nonlinear Phenomena, 53 (2003), 59-70.
[4]	M. J. Abolowitz, P. A. Clarkson, Solitons nonlinear evolution equations and inverse scattering, London: Combridge University Press, 1991.
[5]	M. A. Abdou, A. Elhanbaly, Construction of periodic and solitary wave solutions by the extended Jacobi elliptic function expansion method, Commun. Nonliner. Sci., 12 (2007), 1229-1241. doi: 10.1016/j.cnsns.2006.01.013
[6]	S. A. El-Wakil, M. A. Abdou, A. Elhanbaly, New solitons and periodic wave solutions for nonlinear evolution equations, Phys. Lett. A, 353 (2006), 40-47. doi: 10.1016/j.physleta.2005.12.055
[7]	S. A. El-Wakil, M. A. Abdou, The extended mapping method and its applications for nonlinear evolutions equations, Phys. Lett. A, 358 (2006), 275-282. doi: 10.1016/j.physleta.2006.05.040
[8]	M. A. Abdou, S. Zhang, New periodic wave solution via extended mapping method, Commun. Nonliner. Sci., 14 (2009), 2-11. doi: 10.1016/j.cnsns.2007.06.010
[9]	M. A. Abdou, On the variational iteration method, Phys. Lett. A, 366 (2007), 61-68. doi: 10.1016/j.physleta.2007.01.073
[10]	E. M. Abulwafa, M. A. Abdou, A. A. Mahmoud, The solution of nonlinear coagulation problem with mass loss, Chaos, Solitons Fractals, 29 (2006), 313-330. doi: 10.1016/j.chaos.2005.08.044
[11]	H. Ji-Huan, Some asymptotic methods for strongly nonlinear equations, Int. J. Modern Phys. B, 20 (2006), 1141-1199. doi: 10.1142/S0217979206033796
[12]	H. Ji-Huan, Non perturbative method for strongly nonlinear problems, dissertation, de Verlag im Internet GmbH, Berlin, 2006.
[13]	M. Abdou, A. Elhanbaly, Decomposition method for solving a system of coupled fractional time nonlinear equations, Phys. Scripta, 73 (2006), 338-348. doi: 10.1088/0031-8949/73/4/005
[14]	S. A. El-Wakil, M. A. Abdou, New applications of the homotopy analysis method, Zeitschrift fur Naturforschung, 63 (2008), 1-8.
[15]	S. A. El-Wakil, E. M.Abulwafa, A. Elhanbaly, et al. The extended homogeneous balance method and its applications for a class of nonlinear evolution equations, Chaos, Solitons Fractals, 33 (2007), 1512-1522. doi: 10.1016/j.chaos.2006.03.010
[16]	S. A. El-Wakil, M. A. Abdou, New exact travelling wave solutions using Modified extended tanh function method, Chaos, Solitons Fractals, 31 (2007), 840-852. doi: 10.1016/j.chaos.2005.10.032
[17]	I. Liu, K. Yang, The extended F-expansion method and exact solutions of nonlinear PDEs, Chaos, Solitons Fractals, 22 (2004), 111-121. doi: 10.1016/j.chaos.2003.12.069
[18]	M. A. Abdou, An improved generalized F-expansion method and its applicatuions, J. Comput. Appl. Math., 214 (2008), 202-208. doi: 10.1016/j.cam.2007.02.030
[19]	M. A. Abdou, The extended F-expansion method and its application for a class of nonlinear evolution equations, Chaos, Solitons Fractals, 31 (2007), 95-104. doi: 10.1016/j.chaos.2005.09.030
[20]	M. A. Abdou, Further improved F-expansion and new exact solutions for nonlinear evolution equations, J. Nonlinear Dynamics, 52 (2007), 277-288.
[21]	H. Ji-Huan, M. A. Abdou, New periodic solutions for nonlinear evolution equations using Exp function method, Chaos, Solitons Fractals, 34 (2007), 1421-1429. doi: 10.1016/j.chaos.2006.05.072
[22]	S. A. El-Wakil, M. A. Abdou, A. Hendi, New periodic wave solutions via Exp-function method, Phys. Lett. A, 372 (2008), 830-840. doi: 10.1016/j.physleta.2007.08.033
[23]	M. A. Abdou, Generalized solitary and periodic solutions for nonlinear partial differential equations by the Exp-function method, J. Nonlinear Dynamics, 52 (2008), 1-9. doi: 10.1007/s11071-007-9250-1
[24]	M. S. Osman, D. Lu, M. M. A. Khater, et al. Complex wave structures for abundant solutions related to the complex Ginzburg-Landau model, Optik, 192 (2019), 162927-1-162927-5. doi: 10.1016/j.ijleo.2019.06.027
[25]	S. Owyed, M. A. Abdou, A. H. Abdel-Aty, et al. New optical soliton solutions of nolinear evolution equation describing nonlinear dispersion, Commun. Theor. Phys., 71 (2019), 1063-1068. doi: 10.1088/0253-6102/71/9/1063
[26]	M. A. Abdou, On the quantum Zakharov Kuznetsov equation, Int. J. Nonlinear Sci., 26 (2018), 89-96.
[27]	S. Owyed, M. A. Abdou, A. H. Abdel-Aty, et al. Optical solitons solutions for perturbed time fractional nonlinear Schrodinger equation via two strategic algorithms, Aims Math., Available from: http://www.aimspress.com/journal/Math,2020, accepted and in press.
[28]	J. J. Yang, S. F. Tian, W. Q. Peng, et al. The N-coupled higher-order nonlinear Schrödinger equation: Riemann-Hilbert problem and multi-soliton solutions, Math. Meth. Appl. Sci., (2019), 1-15.
[29]	W. Q. Peng, S. F. Tian, T. T. Zhang, Initial value problem for the pair transition coupled nonlinear Schrödinger equations via the Riemann-Hilbert method, Complex Analy. Operator Theory, 14 (2020), 1-15. doi: 10.1007/s11785-019-00958-3
[30]	T. Y. Xu, S. F. Tian, W. Q. Peng, Riemann-Hilbert approach for multisoliton solutions of generalized coupled fourth-order nonlinear Schrödinger equations, Math. Meth. Appl. Sci., 43 (2019), 865-880.
[31]	W. Q. Peng, S. F. Tian, X. B. Wang, et al. Riemann-Hilbert method and multi-soliton solutions for three-component coupled nonlinear Schrödinger equations, J. Geom. Phys., 146 (2019), 103508-1-103508-9. doi: 10.1016/j.geomphys.2019.103508
[32]	S. F. Tian, Lie symmetry analysis, conservation laws and solitary wave solutions to a fourth-order nonlinear generalized Boussinesq water wave equation, Appl. Math. Lett., 100 (2020), 106056-1-106056-7. doi: 10.1016/j.aml.2019.106056
[33]	L. D. Zhang, S. F. Tian, W. Q. Peng, et al. The dynamics of lump, lumpoff and Rogue wave solutions of (2+1)-dimensional Hirota-Satsuma-Ito equations, East Asian J. Appl. Math., 10 (2020), 243-255.
[34]	C. Q. Dai, J. F. Zhang, Controlling effect of vector and scalar crossed double-Ma breathers in a partially nonlocal nonlinear medium with a linear potential, Nonlinear Dyn., 100 (2020), 1621-1628. doi: 10.1007/s11071-020-05603-9
[35]	G. Z. Wu, C. Q. Dai, Nonautonomous soliton solutions of variable-coefficient fractional nonlinear Schrödinger equation, Appl. Math. Lett., 106 (2020), 106365-1-106365-6. doi: 10.1016/j.aml.2020.106365
[36]	C. Q. Dai, Y. Fan, N. Zhang, Re-observation on localized waves constructed by variable separation solutions of (1+1)-dimensional coupled integrable dispersionless equations via the projective Riccati equation method, Appl. Math. Lett., 96 (2019), 20-26. doi: 10.1016/j.aml.2019.04.009
[37]	C. Q. Dai, Y. Fan, Y. Y. Wang, Three-dimensional optical solitons formed by the balance between different-order nonlinearities and high-order dispersion/diffraction in parity-time symmetric potentials, Nonlinear Dyn., 98 (2019), 489-499. doi: 10.1007/s11071-019-05206-z
[38]	B. H. Wang, P. H. Lu, C. Q. Dai, et al. Vector optical soliton and periodic solutions of a coupled fractional nonlinear Schrödinger equation, Results Phys., 17 (2020), 103036-1-103036-7. doi: 10.1016/j.rinp.2020.103036
[39]	B. Muatjetjeja, S. O. Mbusi, A. R. Adem, Noether symmetries of a generalized coupled Lane-Emden-Klein-Gordon-Fock system with central symmetry, Symmetry, 12 (2020), 1-6.
[40]	B. Muatjetjeja, A. R. Adem, S. Oscar. Mbusi, Traveling wave solutions and conservation laws of a generalized Kudryashov-Sinelshchikov equation, J. Appl. Anal., 25 (2019), 211-217. doi: 10.1515/jaa-2019-0022
[41]	A. R. Adem, B. Muatjetjeja, Conservation laws and exact solutions for a 2D Zakharov-Kuznetsov equation, Appl. Math. Lett., 48 (2015), 109-117. doi: 10.1016/j.aml.2015.03.019
[42]	A. R. Adem, C. M. Khalique, Conserved quantities and solutions of a (2 + 1)-dimensional Haragus-Courcelle-Il'ichev model, Comput. Math. Appl., 71 (2016), 1129-1136. doi: 10.1016/j.camwa.2016.01.021
[43]	C. A Garzon, On exact solutions for a generalized Burgers-Sharma-Tasso-Olver equation with forcing term, Commun. Appl. Analy., 21 (2017), 127-134.
[44]	A. H. Salas, Exact solutions to a generalized sharma-Tasso-Olver equation, Appl. Math. Sci., 5 (2011), 2289-2295.
[45]	S. Owyed, M. A. Abdou, A. H. Abdel-Aty, et al. Numerical and approximate solutions for coupled time fractional nonlinear evolutions equations via reduced differential transform method, Chaos, Solitons Fractals, 131 (2020), 109474.
[46]	M. A. Abdou, A. Soliman, New exact travelling wave solutions for fractal order space time FPDEs descring Transmisssion line, Results Phys., 9 (2018), 1497.
[47]	M. A. Abdou, Fractional reduced differentional transform method and its applications, Int. J. Nonlinear Sci., 26 (2018), 55-64.
[48]	M. A. Abdou, New exact solutions for space-time fractal order nonlinear dynamics of microtubules via the generalized Kudryashov method, Acta (2018), submitted.
[49]	M. A. Abdou, An anylatical approach for space-time fractal order nonlinear dynamics of microtubules, Waves in random media and complex media, Available from: https://doi.org/10.1080/17455030.2018.1517951.
[50]	M. A. Abdou, On the fractional order space-time nonlinear equations arising in plasma physics, Indian J. Phys., 93 (2019), 537-541. doi: 10.1007/s12648-018-1342-x
[51]	M. A. Abdou, A new analytical method for space-time fractional nonlinear differential equations arising in plasma physics, J. Ocean Eng. Sci., 2 (2017), 1-5. doi: 10.1016/j.joes.2016.11.001
[52]	S. Owyed, M. A. Abdou, Abdel-Haleem Abdel-Aty, et al. New optical soliton solutions of nolinear evolution equation describing nonlinear dispersion, Commun. Theor. Phys., 71 (2019), 1063-1068. doi: 10.1088/0253-6102/71/9/1063
[53]	Luu Vu Cam Hoan, S. Owyed, M. Inc, et al. New explicit optical solitons of fractional nonlinear evolution equation via three different methods, Results Phy., (2020), doi: https://doi.org/10.1016/j.rinp.2020.103209.

This article has been cited by:

1.	M. Akher Chowdhury, M. Mamun Miah, H.M. Shahadat Ali, Yu-Ming Chu, M.S. Osman, An investigation to the nonlinear (2 + 1)-dimensional soliton equation for discovering explicit and periodic wave solutions, 2021, 23, 22113797, 104013, 10.1016/j.rinp.2021.104013
2.	Loubna Ouahid, Plenty of soliton solutions to the DNA Peyrard-Bishop equation via two distinctive strategies, 2021, 96, 0031-8949, 035224, 10.1088/1402-4896/abdc57
3.	Awatif A. Hendi, Loubna Ouahid, Saud Owyed, M.A. Abdou, New periodic solutions for Ginzburg-Landau in three different derivatives via extended Jacobian elliptic function method, 2021, 22113797, 104152, 10.1016/j.rinp.2021.104152
4.	Loubna Ouahid, M. A. Abdou, S. Owyed, Sachin Kumar, New optical soliton solutions via two distinctive schemes for the DNA Peyrard–Bishop equation in fractal order, 2021, 35, 0217-9849, 2150444, 10.1142/S0217984921504443
5.	Hamood Ur Rehman, Aziz Ullah Awan, ElSayed M. Tag-ElDin, Sharifah E. Alhazmi, Mansour F. Yassen, Rizwan Haider, Extended hyperbolic function method for the (2 +1)-dimensional nonlinear soliton equation, 2022, 40, 22113797, 105802, 10.1016/j.rinp.2022.105802
6.	M. Raheel, Mustafa Inc, E. Tala-Tebue, K. H. Mahmoud, Breather, kink and rogue wave solutions of Sharma-Tasso-Olver-like equation, 2022, 54, 0306-8919, 10.1007/s11082-022-03933-z
7.	Loubna Ouahid, M. A. Abdou, S. Owyed, A. M. Abdel-Baset, M. Inc, Multi-waves interaction and optical solitons for Heisenberg models of fractal order, 2022, 96, 0973-1458, 2963, 10.1007/s12648-021-02198-8
8.	Loubna Ouahid, M. A. Abdou, Sachin Kumar, Analytical soliton solutions for cold bosonic atoms (CBA) in a zigzag optical lattice model employing efficient methods, 2022, 36, 0217-9849, 10.1142/S021798492150603X
9.	M. A. Abdou, Loubna Ouahid, Jameelah S. Al Shahrani, Meznah M. Alanazi, Sachin Kumar, New analytical solutions and efficient methodologies for DNA (Double-Chain Model) in mathematical biology, 2022, 36, 0217-9849, 10.1142/S021798492250124X
10.	Loubna Ouahid, Saud Owyed, M.A. Abdou, Nawal A. Alshehri, S.K. Elagan, New optical soliton solutions via generalized Kudryashov’s scheme for Ginzburg–Landau equation in fractal order, 2021, 60, 11100168, 5495, 10.1016/j.aej.2021.04.030
11.	Awatif A. Hendi, Loubna Ouahid, Sachin Kumar, S. Owyed, M. A. Abdou, Dynamical behaviors of various optical soliton solutions for the Fokas–Lenells equation, 2021, 35, 0217-9849, 10.1142/S0217984921505291
12.	Loubna Ouahid, M. A. Abdou, Sachin Kumar, Saud Owyed, S. Saha Ray, A plentiful supply of soliton solutions for DNA Peyrard–Bishop equation by means of a new auxiliary equation strategy, 2021, 35, 0217-9792, 10.1142/S0217979221502659
13.	M. A. Abdou, Loubna Ouahid, Sachin Kumar, Plenteous specific analytical solutions for new extended deoxyribonucleic acid (DNA) model arising in mathematical biology, 2023, 37, 0217-9849, 10.1142/S0217984923501737
14.	Md. Abu Naim Sheikh, Mohammad Abu Taher, Mohammad Mobarak Hossain, Sushika Akter, , Variable coefficient exact solution of Sharma–Tasso–Olver model by enhanced modified simple equation method, 2023, 7, 26668181, 100527, 10.1016/j.padiff.2023.100527
15.	Yingxin Feng, Houping Dai, Xuedan Wei, Numerical solutions to the Sharma–Tasso–Olver equation using Lattice Boltzmann method, 2023, 95, 0271-2091, 1546, 10.1002/fld.5219
16.	U.H.M. Zaman, Mohammad Asif Arefin, M. Ali Akbar, M. Hafiz Uddin, Utilizing the extended tanh-function technique to scrutinize fractional order nonlinear partial differential equations, 2023, 8, 26668181, 100563, 10.1016/j.padiff.2023.100563
17.	M. A. Abdou, L. Ouahid, J. S. Al Shahrani, S. Owyed, Novel analytical techniques for HIV-1 infection of CD4 + T cells on fractional order in mathematical biology, 2023, 97, 0973-1458, 2319, 10.1007/s12648-022-02559-x
18.	U. H. M. Zaman, Mohammad Asif Arefin, M. Ali Akbar, M. Hafiz Uddin, Explore dynamical soliton propagation to the fractional order nonlinear evolution equation in optical fiber systems, 2023, 55, 0306-8919, 10.1007/s11082-023-05474-5
19.	Kalim U. Tariq, Ahmet Bekir, Sana Nisar, The dynamical structures of the Sharma–Tasso–Olver model in doubly dispersive medium, 2023, 177, 09600779, 114290, 10.1016/j.chaos.2023.114290
20.	Man Jia, S.Y. Lou, Physical explanations of infinite symmetries of Sharma-Tasso-Olver equation, 2025, 532, 03759601, 130200, 10.1016/j.physleta.2024.130200
21.	Abdul S. Awan, Sultan Hussain, Differential–anti-differential equations and their solutions, 2023, 1016-2526, 313, 10.52280/pujm.2023.55(7-8)04

Reader Comments

Your name:*

Email:*
© 2020 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

AIMS Mathematics

1.8 3.4

Metrics

Article views(4083) PDF downloads(160) Cited by(21)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

Figures and Tables

Figures(4)

AIMS Mathematics

Explicit solutions to the Sharma-Tasso-Olver equation

Related Papers:

Abstract

1. Introduction

2. Methodology

2.1. Extended F-Expansion method

2.2. New sub-equation method

3. Generalized Kudryashov expansion method

4. Conclusions

Appendix

Acknowledgments

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Catalog

AIMS Mathematics

Explicit solutions to the Sharma-Tasso-Olver equation

Related Papers:

Abstract

1. Introduction

2. Methodology

2.1. Extended F-Expansion method

2.2. New sub-equation method

3. Generalized Kudryashov expansion method

4. Conclusions

Appendix

Acknowledgments

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog