On the density of shapes in three-dimensional affine subdivision

1.   Introduction

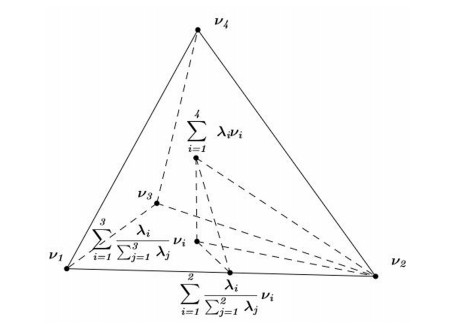
Let $n\geq2$ and $(\lambda_1, \lambda_2, \ldots, \lambda_{n+1})$ be a given $(n+1)$-tuple with all components positive such that $\sum_{j = 1}^{n+1}\lambda_{j} = 1$. Let $\Delta$ be a given Euclidean $n$-simplex with $n+1$ vertices $\nu_1, \nu_2, \cdots, \nu_{n+1}$. The affine subdivision of $\Delta$ with parameter tuple $(\lambda_1, \lambda_2, ..., \lambda_{n+1})$ is a certain collection of $(n+1)!$ smaller $n$-simplices whose union is $\Delta$. It's a kind of $(n+1)!$-interior point subdivision (see ^[11] for the details). Let $\nu$ be the point $\sum_{i = 1}^{n+1}\lambda_{i}\nu_{i}$. For each $(n-2)$-face of $\Delta$, there exits a $(n-1)$-hyperplane decided by the face and $\nu$. The simplex $\Delta$ is divided into $(n+1)!$ smaller $n$-simplices $\Delta_1$, $\Delta_2$, $\cdots$, $\Delta_{(n+1)!}$ by these hyperplanes. A well-known example is the barycentric subdivision when $\lambda_{j} = 1/(n+1)$, for $j\, = \, 1, \cdots, n+1$ (see ^[2,9,10]). The iteration of affine subdivision on a simplex produces a kind of Apollonian networks(see ^[1]). Recently, Liu et al. ^[5,6,7] have studied the linear octagonal-quadrilateral networks, the weighted edge corona networks and the generalized Sierpinski networks. They have obtained rich results.

As shown in the Figure 1, let $\nu_{i1}$ denotes the vertex of $\Delta_i$ coincide with a vertex of $\Delta$ and let $\nu_{i2}$ denotes the vertex of $\Delta_i$ in the interior of a edge of $\Delta$ and so forth. For $k = 1, 2, \cdots, n$, set $\nu_{ik} < \nu_{i(k+1)}$, we obtain a orientation of $\Delta_i$. Taking the same affine subdivision on $\{\Delta_i\}$ and so forth, one obtains an infinite collection $\Lambda$ of simplices. Similar to ^[2], a natural question is whether $\Lambda$ is a dense set of shapes. By shape we mean the equivalence classes of simplices under similarity. Namely, two simplices is said to have the same shape if they are similar.

On barycentric subdivision, the question was raised and positively answered in the two-dimensional case in ^[2]. The three-dimensional case and the four-dimensional case were both solved by Schwartz ^[9,10]. On affine subdivision, Ordin ^[8] raised and gave a positive answer to the queston in the two-dimensional case. Ordin observed that if a 2-simplex has edges $l_1, l_2, l_3$, the triple $(l_1^2, l_2^2, l_3^2)$ is contained in the interior of a cone in $R^3$. Ordin proved his result by the group theory in hyperbolic geometry. For higher dimensions, $(l_1^2, l_2^2, \cdots, l_k^2)$ is bounded by a extremely complicated surfaces, where $l_1, l_2, \cdots, l_k$ are the edges of a simplex. The idea of Ordin seems do not work in higher dimension.

Similar to ^[2,8,9,10], the critical point of solving the question above is making connection with matrices. Let $\mathcal{T}$ be the collection of matrices of the form $T = \pm L/|\det(L)|^{\frac{1}{n}}$, where $L$ is the linear part of an affine map from $\Delta$ to a member of $\Lambda$ and the sign is chosen such that $det(T)$ is a positive number. The affine naturality of affine subdivision forces $\mathcal{T}$ to be a semigroup of $SL_n(\mathbf{R})$. Then to show that $\Lambda$ consists of a dense set of shapes, it suffices to show that $\mathcal{T}$ is a dense set of $SL_n(\mathbf{R})$.

In order to show that $\mathcal{T}$ is dense in $SL_n(\mathbf{R})$, one method is to find some infinite order elliptic elements in $\mathcal{T}$. If the semigroup generated by these elements is a dense set in $SL_n(\mathbf{R})$, then $\mathcal{T}$ is a dense set too. For barycentric subdivision, when $n = 2$, B$\acute{a}$r$\acute{a}$ny et al. ^[2] gave a calculation to show that $\mathcal{T}$ contains some infinite-order elliptic elements. When $n = 3$, it seems that the infinite order elliptic elements are quite rare. Schwartz ^[9] gave a method to find some infinite order elliptic elements by computer searching and proved that infinite process of iterated barycentric subdivision on a tetrahedron produces a dense set of shapes of tetrahedra.

2.   Main result

Following the strategy in Schwartz ^[9], in this paper we will prove the following result for three-dimensional affine subdivision.

Theorem 2.1. Let $(\lambda_1, \lambda_2, \lambda_3, \lambda_4)$ be one of the following tuples

Then the iteration of the corresponding three-dimensional affine subdivision with parameter tuple $(\lambda_1, \lambda_2, \lambda_3, \lambda_4)$ on any fixed tetrahedron produces a dense set of shapes of tetrahedra.

Theorem 2.1 is still valid for $(1/4, 1/4, 1/4, 1/4)$. Note that the corresponding affine subdivision of $(1/4, 1/4, 1/4, 1/4)$ is barycentric subdivision, so Theorem 2.1 is an extension of Theorem $1.1$ in Schwartz ^[9]. To the best of our knowledge, the following problem remains open.

Suppose that $(\lambda_1, \lambda_2, \lambda_3, \lambda_4)$ is a given tuple with all components positive such that $\sum_{i = 1}^{4}\lambda_{i} = 1$. In which case the iterated affine subdivision on a fixed tetrahedron produces a dense set of shape space of tetrahedra?

3.   The proof

Suppose that $(\lambda_1, \lambda_2, ..., \lambda_{n+1})$ is a given tuple with all components positive such that $\sum_{i = 1}^{n+1}\lambda_{i} = 1$ and $\Delta = \nu_1\nu_2...\nu_{n+1}$ is a given $n$-dimension simplex. Let $S_{n+1}$ be the set of permutations of $\{1, 2, ..., n+1\}$. For each element $P_i \in S_{n+1}$, it has a associated simplex $\Delta_i: = \nu_{i1}\nu_{i2}\ldots\nu_{i(n+1)}$, where

for $k = 1, \, \cdots, \, n+1$. Obviously, $\nu_{ik}$ is contained in the interior of a $(k-1)$-dimensional face of $\Delta$. The simplex $\Delta$ is equal to the union of $\Delta_i$ for all related $i$. The process above is called to be the affine subdivision of $\Delta$ with parameter tuple $(\lambda_1, \lambda_2, ..., \lambda_{n+1})$.

In three dimension, without loss of generality, assume that $\Delta$ is the convex hull of the vertices $e_1$, $e_2$, $e_3$ and $e_4$, where $e_1$ is the origin and $\{e_2, e_3, e_4\}$ is the stand basis of $\mathbf{R}^3$. Lexicographically, we order the elements of $S_4$ as follows.

For any given element $P_i \in S_4$, let $A_{P_i}$ be the affine map such that $A_{P_i}(e_k) = \nu_{ik}$ and $L_{P_i}$ be the linear part of $A_{P_i}$. Normalizing $L_{P_i}$, we get

Since the determinant of $T_{P_i}$ may take value $-1$, $T_{P_i}$ is not necessary an element in $\mathcal{T}$ while $T_{P_i}^2$ is exactly an element in $\mathcal{T}$.

Now we try to search some elliptic elements in the set

We present the details for the tuple $(1/6, \ 1/2, \ 1/6, \ 1/6)$ in the below. The calculations for other situations are similar. For simplicity, denote $T_{P_i}T_{P_j}T_{P_k}$ by $F(i, j, k)$. Below are some infinite order elliptic elements we got by a computer.

Lemma 3.1. $S$, $M_1$ and $M_2$ are infinite order elliptic elements of $SL_3(\mathbf{R})$, where

Proof. Calculating $S, M_1$ and $M_2$ (see Section 4 for the details), we get

The eigenvalues of $S$ are $(1+3\sqrt{7}i)/8, (1-3\sqrt{7}i)/8$ and $1$. There exists a real number $\alpha$ such that $1/8 = \cos\pi\alpha$ and we claim that $\alpha$ is a irrational number. Suppose that a rational pair $(x, y)$ satisfies $y = \cos\pi x$. It follows from Conway-Jones ^[3] that $y$ is contained in the set $\{0, -1, 1, -1/2, 1/2\}$. Hence $\alpha$ is an irrational number, which implies $S$ is an infinite order elliptic element. Similarly, $M_1$, $M_2$ are two infinite order elliptic elements as they have eigenvalues $(-7+\sqrt{15}i)/8$ and $(-1+\sqrt{15}i)/4$, respectively.

Let $\langle S\rangle$ denote the group generated by $S$. Since $S$ is an infinite order elliptic element, $\langle S\rangle$ is a closed one-parameter compact subetaoup in $SL_3(\mathbf{R})$. Moreover, $\langle S\rangle$ is equal to the closure of semigroup generated by $S$. Let $\mathfrak{sl}_n(\mathbf{R})$ denotes the set of traceless $n\times n$ matrices. For $\langle S\rangle$, the following result holds.

Lemma 3.2. $\langle S\rangle$ is generated by the matrix

in the sense that $\langle S\rangle = \{\exp(t\mathfrak{s})\ |\ t\ \in\ \mathbf{R}\}$.

Proof. Using the eigenvectors of $S$, we get

This matrix conjugates $S$ to a block diagonal matrix,

According to lemma 3.1, $B$ is an infinite order elliptic element. Let $\langle B\rangle$ be the closure of the semigroup generated by $B$. Then $\langle B\rangle$ is a closed one-parameter compact subetaoup in $SL_2(\mathbf{R})$. It's well-known that $SL_2(\mathbf{R})$ plays the role as an isometrical group on the the hyperbolic plane $H$ by linear fractional transformations. Hence $\langle B\rangle$ is the rotation group about a fixed point $x \in H$. We claim that $\langle B\rangle$ is generated by the matrix

in the sense that $\langle B\rangle = \{\exp(t\mathfrak{b})\ |\ t\ \in\ \mathbf{R}\}$.

It easy to see that $\mathfrak{b}B = B\mathfrak{b}$. For $t\in\mathbf{R}$, let $\beta_t = \exp(t\mathfrak{b})$ and let $B_1$ be a element in $\langle B\rangle$. Then $\beta_t B_1 = B_1 \beta_t$, which implies $\beta_t \in \langle B\rangle$. Therefore,

From the construction above, $\langle S\rangle$ is generated by the matrix

in the sense that $\langle S\rangle = \{\exp(t\mathfrak{s})\ |\ t\ \in\ \mathbf{R}\}$.

Let $G_{ij}$ denote $M_i^j\langle S\rangle M_i^{-j}$ for $i = 1, 2$, $j = 1, 2, 3, 4$. Then for all related $i$, $j$,

Let $G \subset SL_3(\mathbf{R})$ be the closed subetaoup generated by $\{G_{ij} | i = 1, 2, j = 1, 2, 3, 4\}$ and let $\mathfrak{G}$ denote the vector space with a basis $\{\mathfrak{g}_{ij} | i = 1, 2, j = 1, 2, 3, 4\}$. We claim that $G = SL_3(\mathbf{R})$. For Lie algebra vectors $\mathfrak{a}$ and $\mathfrak{b}$, the following formula can be found in ^[4](P. 138) that

Hence $\exp(\mathfrak{G}) \subset G$. To show that $G = SL_3(\mathbf{R})$, it's suffices to show that $\dim(\mathfrak{G}) = 8$. Let $P: \mathfrak{sl}_3(\mathbf{R})\rightarrow \mathbf{R}^8$ be the isomorphism which string out of the coordinates of every element $\mathfrak{g} \in \mathfrak{sl}_3(\mathbf{R})$ except for the lower right coordinate $\mathfrak{g}(3, 3)$. Let $M$ be the $8\times 8$ matrices whose rows composed by $\{P(\mathfrak{g}_{ij})\}$ for all related $i$, $j$. Then

which means that $\{P(\mathfrak{g}_{ij})\}$ is a basis of $\mathbf{R}^8$. It follows that $SL_3(\mathbf{R}) = \exp(\mathfrak{G}) \subset G \subset SL_3(\mathbf{R})$.

3.1.   Proof of Theorem 2.1

Proof. Let $\widetilde{\mathcal{T}}$ denote the closure of $\mathcal{T}$ in $SL_3(\mathbf{R})$. It follows from Lemma 3.1 that $\langle S\rangle \subseteq \widetilde{\mathcal{T}}$ and $M_i^{\pm j} \in \widetilde{\mathcal{T}}$ for all related $i$, $j$. Namely, $G_{ij}$ is contained in $\widetilde{\mathcal{T}}$ too. It implies that $G \subseteq \widetilde{\mathcal{T}}$. According to Lemma 3.2, we have $\widetilde{\mathcal{T}} = SL_3(\mathbf{R})$. Therefore $\mathcal{T}$ is a dense set of $SL_3(\mathbf{R})$. We thus finish the proof of Theorem 2.1 when $(\lambda_1, \lambda_2, \lambda_3, \lambda_4)$ is equal to $(1/6, 1/2, 1/6, 1/6)$. We can use the same method to check other cases in Theorem 2.1. The elliptic elements with infinite order are attached in Table 1.

4.   The Mathematica file

The following program is based on the program of Schwartz ^[9]. Readers can check the calculations above by $\texttt{Mathematica}$ and they can find more details in Wolfram ^[13].

Acknowledgments

This work is supported by NSFC (No.11601141, No.11631010, No.11701165, No.11871202).

Conflict of interest

The authors declare that there are no conflicts of interest regarding the publication of this manuscript.