Quantitative analysis of body metabolism regulation model in the process of weight loss

1.   Introduction

Overweight and obesity is a chronic metabolic disease, which caused by many factors. It is an important factor leading to diabetes mellitus, cardiac and cerebral vessels, and metabolic syndrome (MS), which have become a rapidly growing epidemic worldwide^[1,2]. According to the Body Mass Index (BMI)^[3] standard published by the United Nations World Health Organization (WHO), the number of overweight and obese people in China has approached a quarter of the total population, and obesity not only seriously endangers people's physical and mental health, but places a huge burden on the economy as well as the society ^[4]. Research on weight loss and health can help prevent metabolic diseases, and has been widely employed in other fields such as soldiers' physical reserve and combat effectiveness in the military and prediction of human life limit in natural disasters^[5,6,7].

Many researchers employ clinical experiments and mathematical methods to investigated weight loss. It was found that dieting, exercise, drug and other means are effective approaches to lose weight. Slight obesity can reduce the weight by controlling diet and increasing exercise, while it is necessary for severe obesity to reduce weight by taking medicine^[8,9,10,11]. Different weight loss methods have different weight loss effects. Therefore, considering the need of human energy intake to maintain metabolism, based on the principle of human energy conservation, a series of weight loss mathematical models are established. From a macro perspective, the relationship between energy consumption and weight loss have been studied ^{[12,13,14,15,16,17]}. For example, if the obese lose weight under semi-starvation and complete starvation, the relationship between weight loss and energy demand have been found, and the conclusion has been drawn that people with higher body fat rate would survive longer under complete starvation ^[12,13]. From the established mathematical model of the relation between body weight and caloric intake^[14], it has been known that a direct relationship between body weight and caloric intake has been proposed. Thus, weight loss can be achieved by reducing body fat. Different weight-loss schemes will directly affect the effect of weight-loss. Through model calculation and numerical simulation, the change rule of weight-loss under different schemes are predicted. It has been found that the effects of weight-loss have been achieved by dieting, exercise and other weight-loss methods^[15,16,17]. In recent years, drug weight loss has also been an essential method for people who who diet and exercise less obviously^[18]. Nakai used statistical analysis of clinical data and numerical fits of diet pills to give a relationship between drug dose, changes in excretion fat and weight loss^[19]. From the microcosmic point of view, clinical practice has also studied the impact of the changes of biochemical indicators of human body in the process of weight loss on health. It is found that weight loss not only can improve blood glucose, blood lipid and protein values of three major nutrients and improve the metabolic capacity of the human body, but there are differences in the process time of adjusting the three major nutrients in the way of weight loss^{[20,21,22,23,24,25]}.

Based on the data analysis and biological principles of weight loss through dieting, this paper establishes a mathematical model of metabolic regulation in the process of weight loss. The change trend of three major nutrients in the body under different ways of weight loss is analyzed. The effects of different weight loss schemes on the index values of blood glucose, serum lipid, protein and ketone body are simulated, and optimized the weight loss schemes by comparing and analysing the differences of each index. This paper provides a theoretical basis for guiding healthy weight loss.

This paper is organized as follows. In Section 2, the model of body metabolism regulation in the process of weight loss is established and the change trend of model solution is analyzed qualitatively. Sufficient conditions for the feasibility of three weight loss methods of dieting, dietary exercise and drug are given respectively. In Section 3, some parameters of the model are identified by numerical simulation, and the differences of weight loss methods are analyzed. The conclusion is presented in Section 4.

2.   Metabolic regulation model of body during weight loss

2.1.   Data analysis of three major nutrients change during weight loss

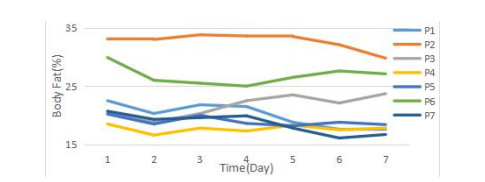
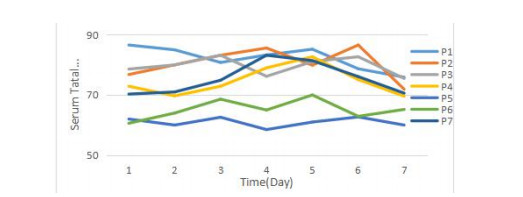
In the process of weight loss, the intake of nutrients and energy consumption have changed, which will lead to change in fasting blood glucose, body fat and protein value and other trace elements^[24,25]. Next, take the experiment of the weight loss by dieting as an example to analyze the changes of three major nutrients metabolic indicators. iCarbonX-Israel conducted a seven-day weight-loss experiment on seven volunteers, collected data on protein, antibody group, metabolic group, biochemical group, and weight, and studied the regulation and health utility of the human weight loss mechanism^[26]. The following part of the data of three major nutrients in the process of dieting are selected to analyze the effects of weight loss on the metabolism of carbohydrate, fat and protein in human body. The index data of carbohydrate, fat and protein metabolism of seven volunteers during the period of fasting are selected, and the changes of blood glucose, body fat rate and total protein are simulated by Excel, as shown in Figures 1–3.

As can be seen from Figure 1, the initial blood glucose values of the seven volunteers were different, but the changes were similar and the overall trend of fluctuations declined. As can be seen from Figure 2, the body fat of the seven volunteers decreased on the first day, and the next day showed an upward or slow downward trend. The overall body fat rate showed a fluctuating downward trend. As can be seen from Figure 3, the total serum protein of seven volunteers fluctuated within a certain range, but most of the volunteers showed a downward trend on the first day and an upward trend on the second day. Some volunteers showed the opposite situation, but the overall trend was slightly downward.

The above figures reflect the three major nutrients metabolic indicators of the volunteers during the dieting period, and the overall changes show a decrease in fluctuations which indicate that the body maintains the balance between the indicators through its own regulation.

2.2.   Metabolic regulation model in the process of weight loss

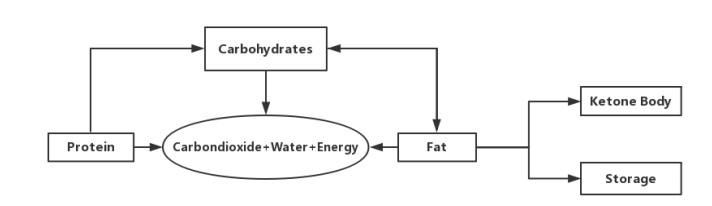
Food is the main source of substances needed to maintain normal metabolic energy. Carbohydrates, fats and proteins in nutrients are the basic substances to maintain human energy. Their conversion rules follow the principle of tricarboxylic acid cycle^[27,28]. When food intake is uneven, the transformation between carbohydrate and fat is a two-way process. When the body absorbs too much carbohydrates, it can be converted into fat and stored in the body. In the process of converse decomposition of fat, a small amount of carbohydrate will be produced, and the derivative ketone body will be produced at the same time. If the ketone body is piled up too much in the human body, it will easy to cause ketoacidosis ^[29]. The conversion of protein and carbohydrate is a one-way process, carbohydrates do not convert to proteins. When carbohydrate metabolism is impaired, protein can be converted to carbohydrate. According to the requirement of biological energy, the three major nutrients and biochemical indicators should be within the standard range when the human body is in a healthy state. Otherwise, the human body will be in a sub-healthy state, and even life-threatening^[29,30]. The metabolic transition diagram of carbohydrate, fat and protein in the human body is shown in Figure 4.

Weight loss methods will affect the transformation of biochemical indicators, in which exercise can help fat consumption, dieting will reduce the absorption of various substances and drugs can inhibit fat absorption. According to the mechanism analysis of the interaction of the three nutrients^[30] in Figure 4, the following biological hypotheses are made in the process of weight loss.

(1) Carbohydrates do not convert to protein, and fat and protein do not convert to each other.

(2) Based on the structure of human body, the three major nutrients have a certain rate of self-production and consumption. When the human body intakes too much carbohydrates, it will increase the storage of fat and consume part of fat and carbohydrate in sports.

(3) Weight loss drugs can inhibit the body's absorption of fat.

(4) The conversion rate and self-production rate of the three major nutrients in the body are limited.

(5) The three major nutrients have normal value range. If the three nutrients beyond the normal range of human body, the human body will present a sub-health state, even life-threatening. The endpoint value of this range is defined as the critical value of human body limit.

(6) When fat is decomposed into carbohydrate, ketone body will be formed. The human body has certain ability to remove ketone body. Because of individual differences, the formation rate and clearance rate of ketone body are different, which are limited. There exists a safety threshold in the ketone body. If the value of the ketone body exceeds the critical value, it will easy to suffer from ketoacidosis.

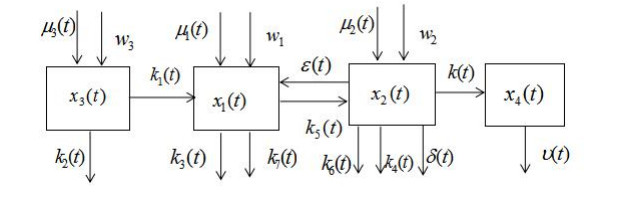
Based on the above hypotheses, we can get the conversion relationship between the three major nutrients and ketone body in the process of weight loss, as shown in Figure 5. The parameters of Figure 5 are shown in Table 1.

Based on the above data analysis and biological hypotheses (1)-(6), a mathematical model for the regulation of three major nutrients and the ketone body during weight loss are established.

where

According to the biological hypotheses of the three major nutrients metabolism in human body (1)-(6), the following basic assumptions are made for model (1) parameters

(H$_1$) $w_i\ (i = 1, 2, 3)$ are all non-negative constants, $k_s(t)\ (s = 1, 2, 3, 4)$ are known continuous non-negative bounded functions. That is to say, there exist positive numbers $\bar{k}_s(t)$, $k_s(t)$ $(s = 1, 2, 3, 4)$ such that $0 < \bar{k}_s(t)\leq k_s(t)\leq k_s$.

(H$_2$) The normal ranges of the three major nutrients $x_j(t)\ (j = 1, 2, 3, 4)$ are

and the initial values of the three major nutrients are satisfied $x_i(0) > a_i$, $0\leq x_4(0) < c$.

(H$_3$) There exist positive numbers $m_r$, $n_r$ $(r = 1, 2)$ such that $m_1\leq k(t)\leq m_2$, $n_1\leq \upsilon(t)\leq n_2$.

Weight loss can achieve the expected goal of healthy weight loss by adjusting diet, exercise and dosage of weight-loss drug. While diet, exercise and dosage of weight-loss drug are limited. That is to say $\mu_i(t)$ $(i = 1, 2, 3)$, $k_h(t)$ $(h = 5, 6, 7)$, $\delta(t)$, $\varepsilon(t)$ affect the law of change of $x_j(t)$ $(j = 1, 2, 3, 4)$, and there exist positive numbers $\varepsilon$, $\delta$, $\bar{\mu}_i$, $\mu_i$, $k_h$ $(i = 1, 2, 3;\ h = 5, 6, 7)$ such that $0\leq \bar{\mu_i} < \mu_i(t)\leq \mu_i$, $0\leq \delta(t)\leq \delta$, $0\leq k_h(t)\leq k_h$ and $0\leq\varepsilon(t)\leq\varepsilon$.

Let $q_i(\xi) < p_i(\xi) < 0$ $(\xi = (\bar{\mu}_i, \mu_i, k_h, \delta, \varepsilon))$ be negative numbers which are determined by the values of $\bar{\mu}_i$, $\mu_i$, $k_h$, $\delta$ and $\varepsilon$. The following research contents is completed under the conditions (H$_1$) - (H$_3$).

Theorem 1. If model (1) satisfies the following conditions

(1) $g_i(t) < 0$ $(i = 1, 2, 3)$, where $g_1(t) = h_1(t)+k_5(t)$, $g_2(t) = h_2(t)+\varepsilon(t)$, $g_3(t) = h_3(t)+k_1(t)$,

(2) $|h_1(t)-h_1(0)|+\rm{max}$ $(|\varepsilon(t), k_1(t)|) < |h_1(0)|$,

(3) $|k_5(t)|+\rm{max}$ $(|h_2(t)-h_2(0)|, |h_3(t)-h_3(0)|) < \rm{min}$ $(|h_2(0)|, |h_3(0)|)$,

then its solution is bounded, and there are two cases as follows.

Case 1 When $0 < \bar{\mu_i}\leq \mu_i(t)\leq \mu_i$, $q_i(\xi)\leq h_i(t)\leq g_i(t)\leq p_i(\xi)$, $-\frac{w_i}{q_i(\xi)}\geq a_i\ (i = 1, 2, 3)$, $-\frac{1}{p_1(\xi)}(w_1+w_2-\frac{k_1w_3}{p_3(\xi)})\leq b_1$, $-\frac{1}{p_2(\xi)}(w_1+w_2-\frac{k_1w_3}{p_3(\xi)})\leq b_2$, $-\frac{w_3}{p_3(\xi)}\leq b_3$ and $\frac{m_2}{n_1}(x_2(0)+\frac{w_2}{q_2(\xi)})\leq c$, then ${\lim_{t \to \infty}\frac{\int^{t}_{0}x_j(s){\rm d}s}{t}\in \Lambda}$ $(j = 1, 2, 3, 4)$.

Case 2 When $0\leq \mu_i(t)\leq \bar{\mu_i}$, $g_i(t)\leq q_i(\xi)$ $(i = 1, 2, 3)$, $-\frac{1}{q_r(\xi)}(\sum\limits_{i = 1}^2w_i-\frac{k_1w_3}{q_3(\xi)}) < a_r$ $(r = 1, 2)$, $-\frac{w_3}{q_3(\xi)} < a_3$ and $\frac{m_1}{n_2}(x_2(0)+\frac{1}{q_2(\xi)}(\sum\limits_{i = 1}^2w_i-\frac{k_1w_3}{q_3(\xi)})) > c$, then ${\lim_{t \to \infty}\frac{\int^{t}_{0}x_j(s){\rm d}s}{t}\not\in \Lambda}$ $(j = 1, 2, 3, 4)$.

Proof. Model (1) is decomposed into the following two sub-models

where

By means of the freeze coefficient method in ^[31], the homogeneous linear equation corresponding to model (3) is frozen as follows

It follows from formula (5) that

We computer

and

as shown in ^[31], the solution of model (1) is stable and bounded.

In order to discuss the changing trend of the solution of model (1), the four equations in model (1) are integrated on both sides.

If the condition of Case 1 holds, the following results are obtained

From $h_3(t)\leq g_3(t)\leq p_3(\xi)$, the third equation of formula (10) can be scaled down to the following formula

Taking the limit of both sides of inequality (11), we have

By condition (H$_1$) and $h_i(t)\leq g_i(t)\leq p_i(\xi)$ $(i = 1, 2)$, the first two equations of formula (10) are added together and then scaled down to get

By substituting inequality (12) into inequality (13) and taking the limit of both sides of inequality (13) yield

Then by $h_3(t) > q_3(\xi)$, the three equations in (10) can be reduced separately to get

Taking the limit of both sides of inequality (15), we have

From formulas (16) and (H$_3$), the fourth equation of formula (10) can be scaled down to get

Taking the limit of both sides of inequality (17), we have

From formula (12), (14), (16) and (18), we get

If the condition of Case 2 holds, the following results are obtained

Take the limit on both sides of the inequality that is obtained by substituting $g_3(t) < q_3(\xi)$ into the third equation of equation (10), we have

By $g_i(t)\leq p_i(\xi)$ $(i = 1, 2)$ and condition (H$_1$), the first two equations of formula (10) are added together and then scaled down to get

By substituting inequality (19) into inequality (20) and taking the limit of both sides of inequality (20) yield

From formula (21) and (H$_3$), the fourth equation of formula (10) can be scaled down to get

Taking the limit of both sides of inequality (22), we have

From formula (19), (21) and (23), we get

Model (1) is used as the basic model to study the changes of three major nutrients and ketone body under weight loss of diet, exercise and drug.

2.3.   Metabolism regulation model of body during weight loss through dieting

If glycogen decomposition is not enough to maintain blood glucose balance, the energy of the body can be provided through the cooperation of fat and protein in the case of dieting and non exercise weight loss ^[30]. Without supplementary energy, the following assumptions can be made for model (1).

(1) The absorption rate of carbohydrates, fats and proteins from food is $\mu_i(t)$ $(i = 1, 2, 3)$.

(2) Fat will not be converted into carbohydrate, that is $k_5(t) = 0$.

(3) The rate of fat and carbohydrate consumption caused by exercise is $k_6(t) = k_7(t) = 0$.

(4) The absorption rate of fat inhibited by drugs is $\delta(t) = 0$.

Based on assumptions (1)-(4) and the modified model (1), we get the following metabolic regulation model during weight loss through dieting.

where

Knowing from assumptions (1)-(4), the parameters of formula (2) can be rewritten as

therefore, the parameters of condition (1) in Theorem 1 are as follows

The above results satisfy condition 1 of Theorem 1, and the Corollary 1 is the following.

Corollary 1. If model (24) satisfies conditions $|k_3(0)-k_3(t)|+\max(|\varepsilon(t)|, |k_1(t)|) < k_3(0)$ and $\max(|\varepsilon(0)+k_4(0)-\varepsilon(t)-k_4(t)|, \ |k_1(0)+k_2(0)-k_1(t)-k_2(t)|) < \max(\varepsilon(0)+k_4(0), \ k_1(0)+k_2(0))$, when $\frac {w_1}{\bar{k}_3} < a_1$, $\frac {w_2}{\bar{k}_4} < a_2$, $\frac {w_3}{\bar{k}_2} < a_3$, $\frac{m_2}{n_1}(x_2(0)+\frac{w_2}{\bar{k}_4}) > c$, then $\lim_{t \to \infty}\frac{\int^{t}_{0}{x_j(s){\rm d}s}}{t}\not\in\Lambda\ (j = 1, 2, 3, 4).$

Proof. According to the given conditions, model (24) conforms to the condition of Theorem 1. Similarly, from the proof of Case 2 of Theorem 1, we can get

therefore

Corollary 1 shows that prolonged dieting can lead to three major nutrients in the body below the normal range. At the same time, with the increase of the demand of transforming fat into carbohydrate, the ketone body produced by fat metabolism will continue to increase to the highest safe critical value, and the human body is more likely to suffer from ketoacidosis.

2.4.   Metabolic regulation model in the process of exercise and dietary weight loss

A certain amount of exercise will promote fat and carbohydrate metabolism when considering the way of exercise and dietary weight loss. In the process of fat decomposition, a small amount of carbohydrate will be produced. Excessive carbohydrate intake translates into fat. When the body's own energy consumption is much greater than the energy intake of the diet, some of the protein will also be converted into carbohydrate, but will not be converted into fat ^[29].

The following assumptions are made for model (1)

(1) Conversion rate of dietary intake of carbohydrate, fat and protein are $\mu_i(t)\neq0$ $(i = 1, 2, 3)$.

(2) The wear rate of carbohydrate consumption and fat consumption caused by exercise are $k_6(t)\neq0$, $k_7(t)\neq0$.

(3) The absorption rate of drugs inhibiting fat in human diet is $\delta(t) = 0$.

Under assumptions (1)-(3), the following metabolic regulation model can be obtained by modifying model (1).

where

As is known by assumptions (1)-(3), the parameters of formula (26) are the same as in the formula (2), except for $\bar{h}_2(t) = \mu_2(t)-\varepsilon(t)-k_4(t)-k_6(t)$. Since there is no drug effect, then $\bar{h}_2(t)\geq h_2(t)$. In this case, the parameter $g_2(t)$ of condition (1) of Theorem 1 becomes $g_2(t) = \bar{h}_2(t)-\varepsilon(t)$, and the other parameters remain unchanged. The parameters $q_i(\xi_1)\leq p_i(\xi_1)$ $(\xi_1 = (\bar{\mu}_i, \mu_i, k_h, \varepsilon))$ are still negative and only depend on the values $\bar{\mu}_i$, $\mu_i$ $(i = 1, 2, 3)$, $k_h\ (h = 5, 6, 7)$ and $\varepsilon$. Thus, the corollary is the following.

Corollary 2. If model (25) satisfies conditions (1)-(2) of Theorem 1, and $\max(|\bar{h}_2(t)-\bar{h}_2(0)|, |h_3(t)-h_3(0)|)+|k_5(t)| < \max(|\bar{h}_2(0)|, |h_3(0)|)$, then the solution of model (25) has the following two cases

Case 1 When $\bar{\mu}_i\leq \mu_i(t)\leq \mu_i$, $-\frac{w_i}{q_i(\xi_1)}\geq a_i\ (i = 1, 2, 3)$, $q_s(\xi_1)\leq h_s(t)\leq g_s(t)\leq p_s(\xi_1)$ $(s = 1, 3)$, $q_2(\xi_1)\leq h_2(t)\leq\bar{g}_2(t)\leq p_2(\xi_1)$, $-\frac{1}{p_r(\xi_1)}(\sum\limits_{i = 1}^{2}w_i-\frac{k_1w_3}{p_3(\xi_1)})\leq b_r$ $(r = 1, 2)$, $-\frac{w_3}{p_3(\xi_1)}\leq b_3$ and $\frac{m_2}{n_1}(x_2(0)+\frac{w_2}{q_2(\xi_1)})\leq c$, then $\lim_{t \to \infty}\frac{1}{t}\int^{t}_{0}{x_j(s){\rm d}s}\in\Lambda$ $(j = 1, 2, 3, 4)$.

Case 2 When $0 < \mu_i(t) < \bar{\mu}$, $g_i(t) < q_i(\xi_1)$$(i = 1, 3)$, $-\frac{1}{q_r(\xi_1)}(\sum\limits_{i = 1}^{2}w_i-\frac{k_1w_3}{q_3(\xi_1)}) < a_r$$(r = 1, 2)$, $-\frac{w_3}{q_3(\xi_1)} < a_3$ and $\frac{m_1}{n_2}(x_2(0)+\frac {1}{q_2(\xi_1)}(\sum\limits_{i = 1}^{2}w_i-\frac{k_1w_3}{q_3(\xi_1)})) > c$, then $\lim_{t \to \infty}\frac{1}{t}\int^{t}_{0}{x_j(s){\rm d}(s)}\not\in\Lambda$ $(j = 1, 2, 3, 4)$.

Proof. According to the given conditions, model (25) satisfies the condition of Theorem 1. Similarly, from the proof of Case 1 of Theorem 1, we get

then $\lim_{t\to\infty}\frac{1}{t}\int^{t}_{0}{x_j(s){\rm d}(s)}\in\Lambda$ $(j = 1, 2, 3, 4)$.

Proceeding as in the proof of Case 2 of Theorem 1, we have

then $\lim_{t\to\infty}\frac{1}{t}\int^{t}_{0}{x_j(s)d(s)}\not\in\Lambda$ $(j = 1, 2, 3, 4)$.

It can be seen from Corollary 2 that healthy weight loss can be achieved when the amount of diet and exercise satisfy Case 1. If the amount of diet and exercise satisfy Case 2, long-term weight loss will lead to the imbalance of the three major nutrients in the human body, so that the three nutrients are lower than the normal range. Therefore, It is necessary to control energy intake and amount of exercise reasonably to achieve the expected weight loss effect.

2.5.   Metabolic regulation model in the process of weight loss drugs

For the people with severe obesity and those who are not sensitive to weight loss of exercise or dieting, they should be treated with drugs. Gastrointestinal lipase inhibitors can effectively inhibit the absorption of fat, reduce the intake of calories, decrease the gluconeogenesis, cut down the conversion rate of fat to carbohydrate, and ultimately achieve the goal of weight loss ^[32,33]. Depending on the human body's consideration of the role of those drugs in the process of non-exercise weight loss, the following modifications and assumptions can be made to model (1).

(1) The absorption rate of fat inhibited by drugs is $\delta(t)\neq0$.

(2) The rate of fat conversion into carbohydrate under the effect of diet pills changed to $\tilde{\varepsilon}(t)$ to satisfy $\tilde{\varepsilon}(t)\leq\tilde{\varepsilon}(t) < \varepsilon$.

(3) Dietary intake parameters $\mu_i\neq0\; (i = 1, 2, 3)$, and the consumption rate of fat and carbohydrate caused by exercise became $k_6(t) = k_7(t) = 0$.

Under assumptions (1)-(3), the following metabolic regulation models can be obtained in the course of weight loss drugs.

where

As is known by assumptions (1)-(3), the parameters of formula (28) are $\tilde{h_1}(t) = \mu_1(t)-k_3(t)-k_5(t)$ and $\tilde{h_2}(t) = \mu_2(t)-k_4(t)-\tilde{\varepsilon}(t)-\delta(t)$, except that $h_3(t)$ is the same as the parameter of formula (2). Since there is no exercise effect, then $\tilde{h}_1(t) \geq h_1(t)$ and $\tilde{h}_2(t) \geq h_2(t)$.

The parameters $g_1(t)$ and $g_2(t)$ of condition (1) of Theorem 1 become $g_1(t) = \tilde{h}_1(t)+k_5(t)$ and $g_2(t) = \tilde{h}_2(t)+\tilde{\varepsilon}(t)$. The parameters $q_i(\xi_2)\leq p_i(\xi_2)$ $(\xi_2 = (\bar{\mu_i}, \mu_i, k_5, \tilde{\varepsilon}, \delta))$ are still negative and only depend on the values of $\bar{\mu_i}$, $\mu_i\; (i = 1, 2, 3)$, $k_5$, $\tilde{\varepsilon}$ and $\delta$. Thus, the corollary is the following.

Corollary 3. If model (27) satisfies conditions (1) of Theorem 1, and $|\tilde{h}_1(t)-\tilde{h}_1(0)|+\max(|\tilde{\varepsilon}(t)|, |k_1(t)|) < |\tilde{h}_1(0)|$, $\max(|\tilde{h}_2(t)-\tilde{h}_2(0)|, |h_3(t)-h_3(0)|)+|k_5(t)| < \min(|\tilde{h}_2(0), h_3(0)|)$, then the solution of model (27) has the following two cases

Case 1 When $\bar{\mu}_i\leq \mu_i(t)\leq \mu_i$, $-\frac{w_i}{q_i(\xi_2)}\geq a_i\ (i = 1, 2, 3)$, $q_r(\xi_2)\leq \tilde{h}_r(t)\leq g_r(t)\leq p_r(\xi_2)$ $(r = 1, 2)$, $q_3(\xi_2)\leq h_3(t)\leq g_3(t)\leq p_3(\xi_2)$, $-\frac{1}{p_r(\xi_2)}(\sum\limits_{i = 1}^{2}w_i-\frac{k_1w_3}{p_3(\xi_2)})\leq b_r$ $(r = 1, 2)$, $-\frac{w_3}{p_3(\xi_2)}\leq b_3$ and $\frac{m_2}{n_1}(x_2(0)+\frac{w_2}{q_2(\xi_2)})\leq c$, then $\lim_{t \to \infty}\frac{1}{t}\int^{t}_{0}{x_j(s){\rm d}}\in\Lambda$ $(j = 1, 2, 3, 4)$.

Case 2 When $0 < \mu_i(t) < \bar{\mu_i}$, $g_i(t) < q_i(\xi_2)$, $-\frac{1}{q_r(\xi_2)}(\sum\limits_{i = 1}^{2}w_i-\frac{k_1w_3}{q_3(\xi_2)}) < a_r$ $(r = 1, 2)$, $-\frac{w_3}{q_3(\xi_2)} < a_3$, $\frac{m_1}{n_2}(x_2(0)+\frac {1}{q_2(\xi_2)}(\sum\limits_{i = 1}^{2}w_i-\frac{k_1w_3}{q_3(\xi_2)})) > c$, then $\lim_{t \to \infty}\frac{1}{t}\int^{t}_{0}{x_j(s){\rm d}s}\not\in\Lambda$ $(j = 1, 2, 3, 4)$.

Proof. According to the given conditions, model (27) satisfies the condition of Theorem 1. Similarly, from the proof of Case 1 of Theorem 1, we get

then $\lim_{t \to \infty}\frac{1}{t}\int^{t}_{0}{x_j(s){\rm d}(s)}\in\Lambda$ $(j = 1, 2, 3, 4)$.

From the proof of Case 2 of Theorem 1, we have

then $\lim_{t\to\infty}\frac{1}{t}\int^{t}_{0}{x_j(s){\rm d}(s)}\not\in\Lambda$ $(j = 1, 2, 3, 4)$.

According to Corollary 3, when model (3) satisfies the condition of Case 1, it can achieve healthy weight loss. Otherwise, Case 2 is established and the three major nutrients will be below the normal range for a limited time. As there is no exercise, the ability of fat consumption is reduced. Since weight loss with drugs is a short-term effect, in the long run, the three major nutrients are in the normal range for a longer period of time than weight loss with exercise. The weight loss effect of the combination of drugs and exercise is more reasonable.

3.   Numerical simulation of the health effects of weight loss

The normal range of human blood glucose is from 60 mg/dL to 80 mg/dL. If it is lower than the lower limit of 45 mg/dL or higher than the upper limit of 120 mg/dL, the risk of late blood glucose symptoms and diabetes mellitus will low. The normal value of total protein content in human serum is 60-80 g/L. If the total protein of human body is lower than 60 g/L, the power of immunity will decrease. According to the proportion of body fat weight to total body weight, the normal body fat of adults ranges from 15% to 25%. The body fat more than the upper limit 25% can be diagnosed as obesity. If the body fat is below the lower limit of 8%, it will cause human dysfunction. When the safety threshold of ketone body in human body is 3 mmol/L, exceeding this standard will lead to ketoacidosis ^[29]. According to the interview with the sixth volunteer who participated in the 7-day fasting and weight loss experiment, when the blood glucose dropped to the range of 45-60 mg/dl, the volunteer did not appear hypoglycemia and other discomfort symptoms. In this section, for the sixth volunteer, the normal ranges of the three major nutrients and ketone body in the human body are selected.

In the process of weight loss, the values of the three major nutrients metabolic indicators can be obtained by real-time blood glucose collection and blood routine. By using the metabolic regulation model in the process of weight loss, we can quantitatively analyze the changes of biochemical indexes of the three major nutrients, predict the impact of different weight loss methods on the health of the body, and guide the correct weight loss methods.

Firstly, based on the actual three days nutrient detection data of seven days fasting and weight loss, some parameter values of model (1) are estimated, and then the effects of different weight loss methods on the three major nutrient changes are analyzed according to the theorem conditions. According to the actual data, the initial values of the three major nutrients are $x_1(0) = 73, \ x_2(0) = 29.2$ and $x_3(0) = 62$ respectively.

3.1.   The effect of weight loss by dieting on health

1. Parameter estimation

The carbohydrate, fat and protein consumed by the body during weight loss through dieting are used to maintain metabolism. When the blood glucose decreases, the conversion rate of fat and protein will gradually increase, which makes the blood glucose present a dynamic equilibrium state. It may be advisable to consider the conversion rate $k_i(t)$ and $\varepsilon(t)$ as time-dependent periodic functions in the following forms

By substituting formula (29) into model (24), we obtain the partial parameter values of model (24), which are estimated by the data for 7 days of carbohydrate, fat and protein metabolism indexes of the 6th volunteer ^[26] by using numerical approximation algorithm ^[34]. Then we get

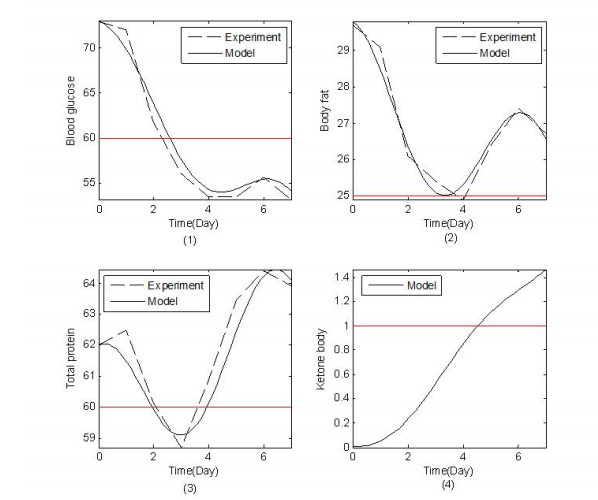
From the literature^[35,36], we choose $k(t) = 0.0034$ and $\upsilon(t) = 0.025$. The theoretical values of blood glucose, body fat, total protein and ketone body can be calculated numerically for model (24) and plotted in the same graph with the actual data collected at 9 o'clock per day. The simulated curves of blood glucose, body fat, total protein and ketone body for 7 days are obtained as shown in Figure 6 (1)-(3). (The solid line represents the model fitting curve, and the dashed line represents the experimental data fitting curve) The simulated curve of theoretical value of ketone body is shown in Figure 6 (4). (The solid line in the figure represents the model fitting curve)

The relative error value of fitting can be calculated from the actual values and the theoretical values of the numerical simulation in Figure 6 (1)-(3) as shown in Table 2.

From Table 2, the overall average error of fitting is 0.01, which shows that the fitting effect is good. Figure 6 (1) shows that blood glucose fluctuate between 50 mg/dL and 60 mg/dL after two days. Figure 6 (2) shows that body fat decreases from 29.7% before weight loss to 26.7% on the 7th day. Figure 6 (3) shows that total protein fluctuate within the normal range. Figure 6 (4) shows that ketone body index rises rapidly after the first day of the fasting and increase to 1.47 mmol/L on the 7th day. During the 7-day weight loss period, the blood glucose and body fat of the volunteers show a downward trend of cyclical fluctuation, but basically keep within the safe range.

2. Numerical simulation of three major nutrients and ketone body

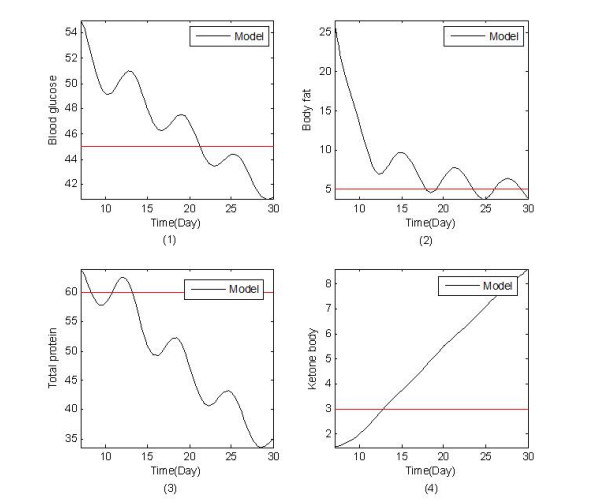
The parameters are selected by formula (30) are substituted into the conclusion of Corollary 1 to verify the validity, and model (24) satisfies

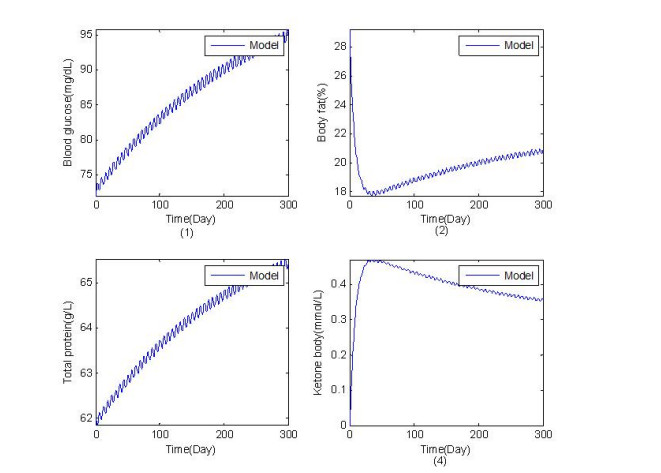
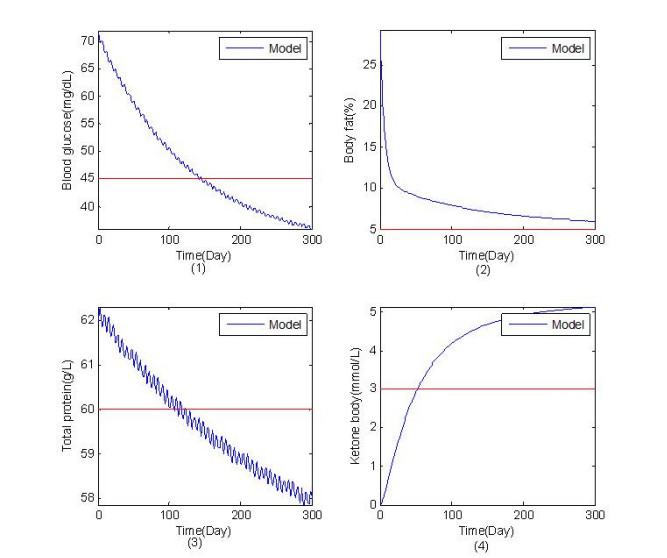
The above results indicate that the three major nutrients and ketone body indexes of the body are not within the safe value range in the long-term weight loss. Explain that the body's three major nutrients and ketone body indicators are not in the safe range after long-term dieting. This result shows that dieting should not be carried out for a long time. The model (24) is used to simulate the predictive curves of blood glucose, total protein, body fat and ketone body for 7 to 30 days as shown in Figure 7. The numerical results are shown in Table 3.

Suggestions for weight loss by dieting: According to the above analysis, the 13th day after losing weight by dieting will be harmful to health, and the behavior of losing weight by fasting should be stopped. Otherwise, on the 23rd day, life may be endangered.

3.2.   The effect of exercise and dietary weight loss on health

Considering the weight loss mode of exercise and diet, $k_j(t)\ (j = 2, 3, 4)$ and $\varepsilon(t)$ in model (25) are still regarded as time-dependent periodic functions. $k_j(t)\ (j = 2, 3, 4)$, $\varepsilon(t)$ and $w_i\ (i = 1, 2, 3)$ selection are the same as the functions in formula (30). When the human body ingests food, the absorption rate of the three major nutrients is gradually increased, and its absorption rate will gradually decrease with the metabolism and energy consumption^[37,38]. The absorption rate $\mu_i(t)\ (i = 1, 2, 3)$ of the three major nutrients can also be regarded as a time-dependent periodic function. The parameters $\mu_i(t)$, $\bar{\mu_i}$, $\mu_i (i = 1, 2, 3)$ and $k_h(t)\ (h = 5, 6, 7)$ for exercise and diet are shown in Table 4.

Two cases of the selected parameters in Table 4 are respectively substituted into model (25), and the conclusion of Corollary 2 is valid. The parameters of two Cases in Table 4 are substituted into the model (25), and the conclusion of Corollary 2 is valid. The numerical simulation of model (25) can be obtained in the following two cases. The numerical simulation of model (25) can be obtained under two Cases.

In Case 1, the solution of model (25) satisfies the following relationship

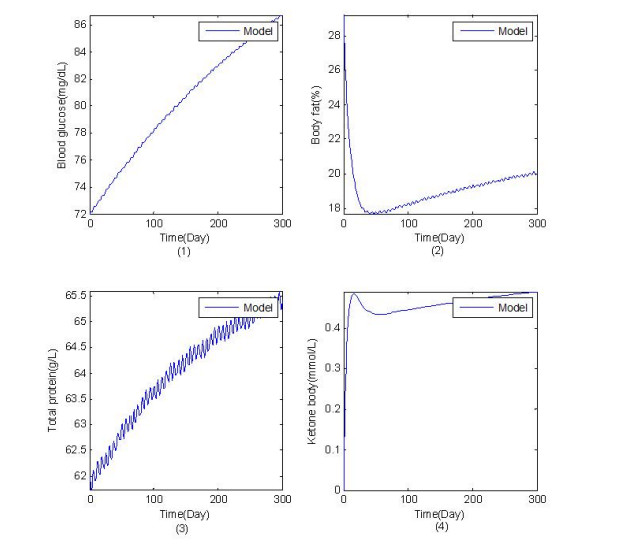
In Case 1, the weight loss can keep the three major nutrients and ketone body indicators within the normal range. The trend curves of the numerical simulation of model (25) in Case 1 are shown in Figure 8 (1)-(4).

Figure 8 (1) shows that blood glucose fluctuated within the range of 80 mg/dL-120 mg/dL after 123 days. Figure 8 (2) shows that body fat is within the range of 18%-20%. The total protein in Figure 8 (3) is always within the range of 60 g/L-80 g/L. Figure 8 (4) shows that the values of ketone body continue to rise within the safe range.

In Case 2, the solution of model (25) satisfies the following relationship

In Case 2, the weight loss ultimately excludes the three major nutrients and ketone body indicators from the safe range. The trend curves of the numerical simulation of model (25) in Case 2 are show in Figure 9 (1)-(4). The changes of three major nutrients and ketone body are described in Table 5.

Exercise and dietary weight loss suggestion: The effect of weight loss can be achieved by reasonably controlling diet and exercise, while the three major nutrients can be controlled within the safe range. If the diet intake too little (too much) and exercise too much (too little), which can not achieve the expected goal of weight loss, it will also damage to your health, and may even endanger your life. This method is better than diet and weight loss.

3.3.   Quantitative analysis of the health impact of the weight-loss with drugs

Considering the way of the weight loss with drugs and without exercise, the parameters $k_j(t)\; (j = 1, 2, 3, 4)$, $k_j(t)$ and $w_i\; (i = 1, 2, 3)$ in model (27) are still regarded as periodic functions related to time, and their selection are the same as the functions in formula (30). According to ^[39], the absorption rate of fat blocked by drugs is $\delta(t) = 0.3\mu_2(t)$, and the rate of fat conversion to blood glucose is $\tilde{\varepsilon}(t) = 0.3\varepsilon(t)$. Two groups of different parameters are selected, as shown in Table 6.

Two Cases of the selected parameters in Table 6 are substituted into model (27) to verify the validity of the conclusion of Corollary 3, and the numerical simulation can be obtained under two cases.

In Case 1, the solution of model (27) satisfies the following relation

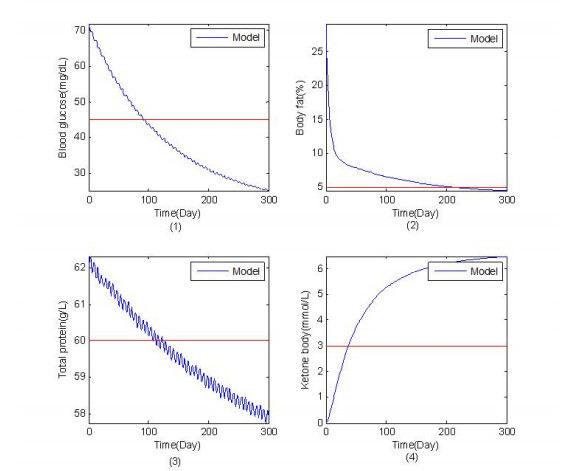
In Case 1, the weight loss can keep the body's three major nutrients and ketone body indicators within the normal range. The trend curves of the numerical simulation of model (27) in Case 1 are shown in Figure 10 (1)-(4).

Figure 10(1) shows that blood glucose fluctuates within the range of 45 mg/dL-120 mg/dL. Figure 10(2) shows that body fat is maintained between 18% and 22%. Figure 10(3) shows that the total protein is always within the range of 60 g/L-80 g/L. Figure 10(4) shows that the values of ketone body continue to rise within the safe range.

In Case 2, the solution of model (27) satisfies the following relationship

In Case 2, the weight loss ultimately makes the three major nutrients and ketone body indicators out of the safe range. The trend curves of the numerical simulation of model (27) in Case 2 are shown in Figure 11 (1)-(4). The changes of three major nutrients and ketone body are described in Table 7.

Weight loss with drugs and without exercise:Under the conditions of Case 1, the three major nutrients and ketone body can be controlled within the safe range. Under the conditions of Case 2, the weight loss with drugs unsafe to lose weight. At the same time, the effect of long-term weight loss is not obvious, especially, the medication may also produce digestive symptoms^[40]. For patients with mild obesity, it is recommended to take exercise weight loss to reduce weight. For those who with severe obesity and who are not sensitive to exercise or dieting weight loss, adjuvant medication can be used.

4.   Conclusion

Based on the biological principles and experimental data of weight loss by dieting, the metabolic regulation models under different weight loss models were established. Based on the normal values of the three major nutrients, the feasible conditions of weight loss by dieting, exercise and drugs were given by using the changing trend of the model solution. By using the numerical simulation method, the rationality of the model, the effectiveness of different weight-loss methods and the impact of different ways of weight reduction on the health of volunteers were analyzed, which provided theoretical reference for guiding weight loss.

The different weight-loss methods are effective. The way of weight loss with proper diet and exercise is better than that without food and drug. Short time dieting can achieve safe weight loss, while long time dieting will affect health, which is dangerous. Drug weight loss can achieve safe weight loss with proper diet, but it has certain side effects, which is not recommended for patients with mild obesity. Therefore, the patients with different obesity should choose the appropriate way to reduce weight according to their own obesity situation.

This model did not consider the metabolism of different age and gender groups in different ways of weight loss and the impact of weight loss on health, which is important in the actual process of weight loss, and the weight loss model will be further studied.

Acknowledgments

This work was supported by the project of Nature Scientific Foundation of Heilongjiang Province (A2016004).

Conflict of interest

The authors declare there is no conflict of interest.