Non-local boundary value problem for a system of fractional partial differential equations of the type I

1.   Introduction

Consider the following system of equations

in the domain $\Omega\! = \!\{(x, y): l_1 < x < l_2, 0 < y < T\},$ where $u(x, y)$ and $f(x, y)$ are unknown and given $n$-vectors, respectively; $A$ and $B$ are the given constant $n\times n$ matrices, $D_{0y}^{\beta}$ is the Riemann – Liouville fracional integro-differentiation operator of order $\beta$ [1,p. 9].

Let us review the papers associated with the investigation of the systems with fractional partial derivatives of order that is not higher than one including the scalar case $n = 1.$ In paper ^[2] for the equation

the solvability of the boundary value problem with the initial conditions $u(0, y) = h_1(y),$ $u(x, 0) = h_2(x)$ is studied in a class of Hölder's continuous functions. The authors obtained a fundamental solution of Eq. (1.2) in the form

where

Note that the function $\psi_{\alpha, \beta} (x, y)$ can be represented (it is shown in ^[3]) as

Paper ^[4] was devoted to study of Hölder's smoothness of solution for the following equation

satisfying the boundary conditions $u(0, y) = u_0(y)$ and $u(x, 0) = u_1(x).$

The uniqueness and existence theorems for a boundary value problem regular solution for the equation

are proved in papers ^[5,6]. The fundamental solution has the form

in the case when $\lambda = 1,$ and, when $\mu = 0$ it has the form

where

is the Wright type function ^[6]. In addition a boundary value problem with negative coefficient $\lambda < 0$ was studied for Eq. (1.4) in the case $\alpha = 1,$ $\mu = 0.$

The Equation (1.4) with variable coefficients $\lambda\equiv \lambda(x)$ and $\mu\equiv\mu(x),$ where $\alpha = 1$ and the function $\lambda(x)$ may have a zero of order $m\geq 0$ at the point $x = 0,$ was investigated in the papers ^[7,8,9]. In that case the fundamental solution

was constructed. In the last expression $\Lambda(x, t) = \int\limits_t^x\lambda(\xi)d\xi,$ $M(x, t) = \int\limits_t^x\mu(\xi)d\xi.$ The existence and uniqueness theorems for a boundary value problem and the Cauchy problem were proved.

We also note the papers ^[10] and ^[11], where the equation

was studied. The fractional derivatives are understood in the sense of Caputo and Riesz in paper ^[10], and in the sense of Caputo, Riemann–Liouville and Riesz in paper ^[11].

For the system

the boundary value problem was solved explicitly in ^[12] when $A$ was an identity matrix, and in ^[13] when $A$ was positive defined matrix. The fundamental solution of system (1.5) was constructed in terms of the introduced Wright function of the matrix argument in paper ^[13]. Article ^[3] used a similar approach to solve the problem with the boundary conditions in the multidimensional case.

Among the works devoted to the study of systems of equations with fractional partial derivatives, we also distinguish papers ^[14,15,16]. In ^[16] A. N. Kochubei described a class of first order systems of equations with constant coefficients containing a fractional derivative with respect to one of the independent variables, for which the Cauchy problem is solvable, and the fundamental solutions of which grow exponentially outside the set $\{|x|y^{-\beta}\leq 1\}.$ Such systems were called fractional hyperbolic systems. System (1.1) also belongs to this class of systems.

Note that, the systems of type (1.1) are differ significantly at the formulations of initial and boundary-value problems, depending on the sign-determinacy or sign-indeterminacy of the eigenvalues of the matrix coefficient in the main part of the system.

In papers ^[12,13,17] boundary value problems in rectangular domains were studied for systems with sign-determined eigenvalues, including systems with partial derivatives, of order strictly lower than one. For such systems the formulation of boundary value problems is similar to the case with a single equation. We call this type of systems the type Ⅰ systems.

In papers ^{[18,19,20,21,22,23]} the Cauchy problem, mixed and non-local problems were investigated for a system of the type Ⅱ, i.e., for the systems, where the matrix coefficient in the main part has an eigenvalues of the different signs.

In this paper, we first solve in explicit form an auxiliary problem for system (1.1) with $B = 0.$ To do this, we use the properties of the Wright function of the matrix argument, which are studied in ^[13]. Next we investigate a non-local boundary value problem for system (1.1) by reducing to the auxiliary problem by using a system of integral equations. We prove the existence and uniqueness theorem. At the end, we give an example of the non-local boundary value problem and construct the graphs of its solution.

2.   Preliminaries

The Riemann-Liouville fractional integro-differentiation operator $D_{ay}^{\nu}$ of order $\nu$ is defined as [1,p. 9]:

for $\nu < 0,$ and for $\nu \geq 0$ the operator $D_{ay}^{\nu}$ can be determined by recursive relation

where $\Gamma(z)$ is the Euler gamma-function.

The symbol $\partial_{0y}^{\nu}$ denotes the Caputo fractional differentiation operator of order $\nu$, [1,p. 11]:

The Wright function ^[24,25] is called an entire function, which is depended from two parameters $\rho$ and $\mu,$ and represented by the series

Here we present the determination and some properties of the Wright function of the matrix argument, which are studied in ^[13].

Let $A$ be a square matrix of order $n.$ In view of the function $\phi(\rho, \mu; z)$ is analytic everywhere in ${\mathbb C},$ following series

is converges for any matrix $A$ given over the field of complex numbers ${\mathbb C},$ and determine the Wright function of the matrix argument.

The following equality holds

here $I$ is an identity matrix of order $n.$

Following differentiation formula holds

Now and further we assume that all of the eigenvalues of the matrix $A$ are positive.

The next fractional integro-differentiation formula holds:

It follows from (2.2) and (2.3) that

The following equality holds

We denote by $|A(x, y)|_*$ the scalar function that takes at each point $(x, y)$ the largest of the values of the moduli of the elements of the matrix $A(x, y) = \|a_{ij}(x, y)\|,$ that is $|A(x, y)|_* = \max\limits_{i, j}|a_{ij}(x, y)|.$ Similarly, for the vector $b(x, y)$ with components $b_i(x, y)$ we denote $|b(x, y)|_* = \max\limits_{i}|b_i(x, y)|.$

Following estimates are hold:

where $\beta\in(0, 1);$ and $\theta\geq 0$ for $\mu\not = 0, -1, -2, ...,$ and $\theta\geq -1$ for $\mu = 0, -1, -2, ...\, ;$ and

where $\beta\in(0, 1),$ $\mu \in {\mathbb R},$ $\sigma < (1-\beta)\left(\lambda\beta^{\beta}\right)^{\frac{1}{1-\beta}},$ $\lambda = \min\limits_{1\leq i\leq p}\{\lambda_i\},$ $\lambda_1, ..., \lambda_p$ are eigenvalues of the matrix $A.$

3.   Auxiliary problem

A regular solution of system (1.1) in the domain $\Omega$ is defined as the vector function $u = u(x, y)$ satisfying system (1.1) at all points $x\in \Omega,$ such that $\frac{\partial u}{\partial x},$ $D_{0y}^{\beta}u \in C(\Omega),$ $y^{1-\beta}u(x, y)\in$ $C(\bar\Omega).$

Before turning to the presentation of the main results, we solve the following auxiliary problem for the case of system (1.1) with $B = 0.$

Problem 1. In the domain $\Omega$ find a solution of the system

with the conditions

where $\varphi(x)$ and $\psi(y)$ are given $n$-vectors.

Theorem 1. Let all the eigenvalues of the matrix $A$ be positive, $\varphi (x)\in C[l_1, l_2],$ $y^{1-\beta}\psi (y)\in C[0, T],$ $y^{1-\beta}f(x, y)\in C(\overline\Omega),$ $f(x, y)$ satisfies the Hölder condition with respect to $y,$ and the matching condition

holds. Then there exists a unique regular in the domain $\Omega,$ solution of Problem 1. Solution can be represented as

where

Remark 1. Without loss of generality, we prove Theorem 1 for the domain $\Omega$ with $l_1 = 0$ and $l_2 = l.$ A more general case reduces to this case by replacing the independent variables $x = \xi + l_1,$ $y = \eta.$

To prove Theorem 1, we need the following assertions.

Lemma 1. Any regular in the domain $\Omega$ solution $u (x, y)$ of Problem 1 can be represented in form (3.5).

Proof. Let $u(x, y)$ be a solution of Problem 1. The function $V(x, y)$ is the solution of the equation

with the conditions

where $I$ is the identity matrix.

Using (2.2), (2.3), (2.1), (2.7) and the relation

it is easy to see that

is the solution of problems (3.6), (3.7).

From (2.2) and (2.3) it follows that

Let $\varepsilon > 0.$ Integration by parts taking into account Eqs. (2.1), (2.7) and (3.7) leads to

From the last two relations we get

Passing to the limit as $\varepsilon \to 0,$ by using (3.1), (3.2), (3.3), (3.6) and analogue of the integration by parts formula in fractional calculus [1,p. 34]

we obtain

Differentiating (3.9) by $x$ and by $y,$ with (3.7) and (3.8), we get (3.5). Lemma 1 is proved.

Lemma 2. Following estimates

are hold, here $C$ is a positive constant.

The validity of Lemma 2 follows from the relations (2.2), (2.3) and (2.6).

Lemma 3. Let all the eigenvalues of the matrix $A$ be positive, $\varphi (x)\in C[0, l],$ $y^{1-\beta}\psi (y)\in C[0, T],$ then the relations

are valid, and limits (3.15) and (3.16) are uniform on any closed subset of $(0; l),$ and limits (3.14) and (3.17) on any closed subset of $(0; T).$

Proof. The validity of relations (3.14) and (3.15) follows from estimates (3.10), (3.11), $|\psi(x)|_*\leq C$ and $|\varphi(y)|_*\leq Cy^{\beta-1}.$

Let us transform the following integral

The limit of the second integral in the right-hand side of (3.18) with $y \rightarrow 0$, due to estimate (3.11) and the boundedness of the function $\psi(x),$ is zero for $x > \varepsilon > 0.$ Denote by $I_1(x, y)$ the first integral in the right-hand side of (3.18), then

Taking advantage of the fact that, by virtue of (2.2),

we obtain that

Passing to the limit at $y \rightarrow 0,$ taking into account formula (2.7), we get

The function $\psi (t)$ is continuous on $[x- \varepsilon, x]$, therefore $\omega(\varepsilon) = \sup | \psi (x-t) - \psi (x) | \rightarrow 0$ with $\varepsilon\rightarrow 0.$ Since $\varepsilon$ can be chosen arbitrary, then the first term in (3.19) is arbitrarily small for any fixed $y,$ that is, tends to zero, with $y \rightarrow 0.$

The second term, by virtue of (3.21), tends to $\psi(x).$ Thus $\lim\limits_{y\rightarrow 0}I_1(x, y) = \psi(x).$ From the latter, together with (3.18) follows (3.16). The relation (3.17) can be proved similarly. Lemma 3 is proved.

Lemma 4. Under the conditions of Theorem 1, function (3.5) is a solution of system (3.1), such that $\frac{\partial}{\partial x}u, D_{0y}^{\beta}u\in C(\Omega).$

Proof. It follows from (3.12), (3.13) that the estimates

are valid for any fixed $y > \varepsilon > 0$ and the estimates

for $x > \varepsilon > 0.$ From these estimates, taking into account relations (2.4), we can see that the first two terms (we denote their sum $u_0(x, y)$) on the right-hand side of (3.5) there are solutions of the homogeneous system

at that $\frac{\partial}{\partial x}u_0, \, D_{0y}^{\beta}u_0\in C(\Omega).$

Denote by $u_f(x, y)$ the third term on the right-hand side of (3.5). Under the condition of Theorem 1, the function $f(x, y)$ satisfies the Hölder condition in the variable $y,$ that is,

here $K$ is positive number. Then

Taking into account estimate (3.12) and condition (3.22), we obtain the estimate for the integrand in the second term of (3.23)

choosing $\theta \in [-1; 0)$ with $q > \beta$ and $\theta \in (-q/\beta; 0)$ with $q \leq \beta,$ it is easy to see that the integral converges uniformly over all $x$ and $y$ for any $q \in (0, 1).$ Transforming the last term of (3.23) with (2.4), we get

From (3.10), (3.11), (3.24) and (3.25) it follows that $\frac{\partial}{\partial x}u_f\in C(\Omega).$

Consider the function $F_{\varepsilon}(x, y) = \int\limits_0^xdt\int\limits_0^{y-\varepsilon}D_{ys}^{\beta-1}G(x-t, y-s)f(t, s)ds.$ From estimate (3.11) we see that $\lim\limits_{\varepsilon\rightarrow 0}F_{\varepsilon}(x, y) = D_{0y}^{\beta-1}u_f(x, y)\in C(\Omega).$ In view of (3.11) and

we get that the derivative

is continuous in $\Omega$ for $\varepsilon\rightarrow 0.$ Therefore

that is

From (2.4), (3.25) and (3.27) we get

Lemma 4 is proved.

3.1.   Proof of Theorem 1

Using estimates (3.10) and $|f(x, y)|\leq Cy^{\beta-1},$ we get

where $u_f(x, y)$ is the third term on the right-hand side of equality (3.5). From (3.28) follow relations

and the inclusion $y^{1-\beta}u_f\in C(\overline{\Omega}).$ Relations (3.14) – (3.17) and (3.29) imply the fulfillment of boundary conditions (3.2) and (3.3).

Denote by $u_{\psi}(x, y)$ and $u_{\varphi}(x, y)$, respectively, the first and second term on the right-hand side of Eq. (3.5). Using estimate (3.10) and the conditions of Theorem 1 on the functions $\psi(x)$ and $\varphi(y),$ we get estimates

From the last two inequalities we get that $y^{1-\beta}(u_{\psi}+u_{\varphi})\in C(\Omega).$

Let us show the validity of the inclusion $y^{1-\beta}(u_{\psi}+u_{\varphi})\in C(\overline{\Omega}).$ For this purpose we represent $u_{\psi}(x, y)$ in the form

In view of (2.2) and (2.1) we obtain

Similarly we get

where $\varphi^*(y) = y^{1-\beta}\varphi(y),$ and

Using (3.30)–(3.33), (2.1), (2.5), (2.7), we get

Relations (3.34) and (3.35) imply that $y^{1-\beta}(u_{\psi}+u_{\varphi})\in C(\overline{\Omega}\setminus \{(0, 0)\}).$

Let $\lim\limits_{x\to 0 \atop y\to 0}xy^{-\beta} = c,$ $0\leq c\leq \infty.$ Then from relation (3.31) we obtain

In view of (3.36) and (3.37) we obtain

This limit does not depend on $c,$ if

that is, under condition (3.4).

The above together with Lemma 4 proves the existence of the solution to problems (3.1), (3.2), (3.3) from the class specified in Theorem 1. The uniqueness of the solution to Problem 1 follows from Lemma 1. Theorem 1 is proved.

4.   Non-local boundary value problem

In this section, we investigate the following non-local boundary value problem in a rectangular domain for system (1.1) of the type Ⅰ.

Problem 2. Find a solution of system (1.1) in the domain $\Omega$ with conditions (3.2) and

where $\varphi(x)$ and $\rho(y)$ are given $n$-vectors, $M$ and $N$ are the given constant $n\times n$ matrix,

Theorem 2. Let all the eigenvalues of the matrix $A$ be positive, $\varphi (x)\in C[l_1, l_2],$ $y^{1-\beta}\rho (y)\in C[0, T],$ $y^{1-\beta}f(x, y)\in C(\overline\Omega),$ $f(x, y)$ satisfies the Hölder condition with respect to $y,$ and the matching condition

holds, matrix $M$ is nonsingular. Then there exists a unique regular in the domain $\Omega,$ solution of Problem 2.

Proof. By virtue of Theorem 1, the solution to Problem 1 for system (1.1) is a solution to the system of the integral equations

where

Due to estimate (3.10) we get the inclusion $y^{1-\beta}F(x, y)\in C(\overline{\Omega}).$

The solution of the system of integral Eqs. (4.3) can be obtained by an iterative method. This solution has the form

where

For iterated kernels, in view of (3.10), the estimate

is valid. Using this estimate, we obtain the convergence of series (4.5) and the estimate for the resolvent

where $C_1 = C |B|_*\Gamma(\varepsilon)\Gamma(\delta),$ and $C$ is a large enough number. Due to the continuity of the function $\phi(\delta, \delta; z),$ the following estimate is valid

Thus, solution (4.4) can be represented as

where

It is easy to show that function (4.4) is the solution to Problem 2. Now let $u(x, y)$ be a regular solution of Problem 1 in the domain $\Omega,$ then equality (4.7) also holds. Using representation (4.7), we express the boundary value:

where $\overline{\Psi}(y) = \Psi(l_2, y),$ $\overline{K}(y-s) = R_1(l_2, y-s).$

Since the matrix $M$ is invertible, condition (4.2) can be rewritten as

Using (4.8), from the last equality we get

where

From (3.10) and (4.5) follow the estimate

and the following inclusions

It follows from (4.10) and the conditions of Theorem 2 on the function $\rho(y),$ that $y^{1-\beta} P(y)\in C[0, T].$

From relations (4.9) and (4.10), it follows that system (4.8) is a system of the Volterra integral equations of the second kind with a weak singularity in the kernel, and has the unique solution $u(l_1, y)$ such that $y^{1-\beta}u(l_1, y)\in C[0, T].$ After the value of $u(l_1, y)$ is found, the solution to Problem 2 can be obtained from representation (4.7).

From Theorem 1 it follows that for the inclusion $y^{1-\beta}u(x, y)\in C(\overline{\Omega})$ the condition

should be met. Taking into account equality (4.9), we rewrite condition (4.11) as

From inclusions (4.10), estimates (3.10), (4.5) and $|u(l_1, y)|_*\leq Cy^{\beta -1}$ we obtain the relations

By virtue of (4.14) and the relation

which follows from (3.16) and Remark 1, we obtain

In view of (4.13) and (4.15), equality (4.12) takes the form

Therefore, condition (4.2) is sufficient for $y^{1-\beta}u(x, y)\in C(\overline{\Omega}).$ Theorem 2 is proved.

Remark 2. The case when all the eigenvalues of the matrix are negative, is reduced to the case with positive eigenvalues by changing the variables $\xi = x-l_1,$ $\eta = y,$ and the function $u(x, y) = u(\xi+l_1, \eta) = w(\xi, \eta).$ Moreover, for the solvability of Problem 2, the matrix $N$ must be nonsingular.

5.   Illustration

As example consider Problem 2 with $n = 2,$ $AB = BA,$ $l_1 = 0,$ $l_2 = 1,$ $T = 1,$ $M = N = I,$ $f(x, y)\equiv 0,$ $\varphi(x)\equiv 0,$ $\rho(y) = \frac{y^{\beta-1}}{\Gamma(\beta)}\left (\begin{array}{c} 1 \\ 2 \end{array}\right),$ i.e., the system

with the conditions

Solution of problem (5.1)–(5.3) satisfies the following relation

where

From (5.4) we get

Substituting (5.5) into (5.4) we obtain following system of integral equations with respect to $u(0, y)$

where

Using the Wright functions convolution formula, we calculate the iterative kernels

and find the following solution of integral Eq. (5.5)

where

Put (5.7) into (5.5) we obtain the solution to problems (5.1)–(5.3) in the form

where

After calculating the integrals, we write equality (5.8) in the form

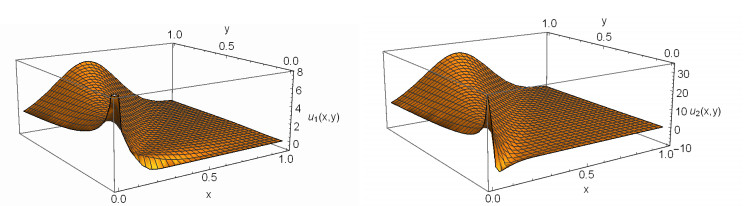
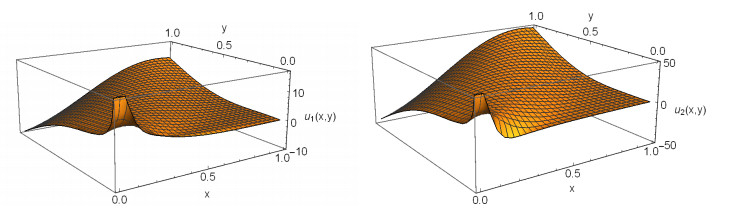
Figures 1 and 2 illustrate the solutions of problems (5.1)–(5.3) in cases $\beta = 0.4$ and $\beta = 0.6.$

6.   Conclusion

We investigated the non-local boundary value Problem 2 for system (1.1). For this, we have written out an explicit solution of auxiliary Problem 1 for system (1.1) with the matrix B = 0 in terms of the matrix Wright function. Then, using the integral equations method, we reduced Problem 2 to Problem 1. Our approach is schematically illustrated by a particular example described in section 5. The system under study is of the type Ⅰ. We previously studied some problems for a system of the type Ⅱ, including Problem 2, which generalizes them. Comparing the results of ^[23] and the present work, we see that the conditions on the matrices M and N, for which Problem 2 is correct, depend on the distribution of the eigenvalues of the matrix A, that is, they are differ for systems of the type Ⅰ and Ⅱ.

Further research will be aimed at expanding the classes of systems and generalizing the described results.

Conflict of interest

The author declares no conflict of interest in this paper.