Modelling and analysis of a delayed viral infection model with follicular dendritic cell

1.   Introduction

Samples cannot always be taken frequently from patients, or detection techniques of the virus may not be accurate, and testing specific hypotheses based on clinical statistic data is a challengeable task, which justifies the key role played by mathematical models in this area. Mathematical models have been proposed to understand the in vivo infection dynamics of viruses. Particularly, theses within-host viral infection models were used to describe the viral infection process, estimate some key parameters, and provide support for research and development of antiviral drugs ^[1,2,3,4]. The classical viral dynamics can be described by the differential equations of three compartments: the uninfected target cells, the infected cells, and the free virus. Sometimes, a fourth compartment corresponding to the immune response was introduced into the model ^[5,6,7,8]. One of the main immune responses is the cytotoxic T-lymphocyte cells (CTLs), which play a crucial role in antiviral defense by attacking virus-infected cells in most virus infections. A basic and simple model with CTL immune response was proposed to explore the interaction between the CTLs and infected cells ^[5]. Then many kinds of models with immune response were further developed to investigate the impact of immune response on the dynamics of virus infection ^[6,7,8] and references therein.

Though antiretroviral therapy can effectively suppress viral replication to a low level, it cannot eradicate the virus permanently. An reliable and possible explanation is that there exists a viral reservoir, which is a possible impediment to virus eradication. Currently, researchers have shown that follicular dendritic cells (FDC) reside in secondary lymphoid organs known as germinal centers and bind HIV-antibody complexes, thus creating an archive of infectious viruses that can perpetuate infection. As such, HIV trapped on FDC is thought to be a significant viral reservoir ^{[9,10,11,12,13]}. Even with antiretroviral therapy, the FDC can still provide a suitable microenvironment for ongoing infection and impair the B cell response ^{[14,15,16,17]}. It is thus important to consider this factor in viral infection models, and some earlier studies of FDC can be found in ^[9,10,11]. For example, Callaway and Perelson ^[11] proposed the following simple model with FDC to understand what the effect FDC could have on the steady state viral load:

where $T(t)$, $I(t)$, $V(t)$, and $W(t)$ are the concentrations of uninfected target cells, infected cells, free viruses, and virus particles bound to FDC at time $t$, respectively. $\lambda$ is a constant production rate of uninfected cells that die at a rate $d$. The uninfected cells are infected by free virus at a rate of $(1-\varepsilon)k T V$, where $\varepsilon$ represents the efficacy of RT inhibitors. The infected cells produce virions at a rate $N\delta I$ and die at a rate $\delta$, where $N$ is the number of virions produced by an infected cell during its life span (burst size). The virions are cleared at a rate of $c_1$. FDC binds free virus at rate $\mu$, bound virus dissociates from FDC at rate $\alpha$, and bound virus is cleared at rate $c_2$.

In addition, it needs to go through a period between initial viral entry into a cell and subsequent viral production, and a maturation time is necessary for the released virions before they become infectious. Moreover, as pointed out by Ciup et al. ^[18], allowing for time delays in the models better predicts viral load data when compared to models without delays. Thus taking time delays into account makes the models more realistic. Then motivated by ^[11], a general viral infection model with two intracellular time delays and CTL immune response takes as follows:

where $Z(t)$ be the concentrations of CTLs at time $t$. The uninfected cells are infected by free virus at a rate of $(1-\varepsilon)k Tf(V)$. The infected cells are cleared by CTLs at a rate of $rIZ$. The CTLs are proliferated at a rate $pIZ$ and decay at a rate $q$. $\tau_1$ represents the time period from being infected to becoming productive infected cells, and $\tau_2$ represents the time necessary for the newly produced virions to become mature. Throughout this paper, we assume that the incidence function $f(V)$ satisfies the following conditions:

Based on condition (1.3), it follows that

The initial conditions for model (1.2) are

where $\tau = \max\{\tau_1, \tau_2\}$ and $(\varphi_1(\theta), \varphi_2(\theta), \varphi_3(\theta), \varphi_4(\theta), \varphi_5(\theta))\in C([-\tau, 0], \mathbb{R}_+^5)$. It is obviously that the model (1.2) includes the model ^[11] as an special case, in which the global dynamics that has not been investigated will be solved in this paper.

The rest of the paper is organized as follows: In Section 2, we derive the basic reproduction number for the viral infection, the basic reproduction number for the immune response, and the existence of equilibria. In Section 3, we prove that the global dynamics of the model are determined by the two threshold parameters. Simulations are carried out to validate the obtained results in Section 4. A summary and a discussion are presented in Section 5.

2.   Preliminary results and thresholds

Some preliminary results will be presented in this part before analyzing the dynamics of model (1.2). According to literature ^[19], it is easy to show that the solutions of model (1.2) are non-negative. Moreover, let $P_1(t) = T+I(t+\tau_1)+\frac{r}{p}Z(t+\tau_1)$, then we have

which implies that $\limsup\limits_{t\rightarrow +\infty}P_1(t)\leq\dfrac{\lambda}{m_1}$, where $m_1 = \min\{d, \delta, q\}$. Then, let $P_2(t) = V(t)+W(t)$, it follows that $P_2'(t)\leq \dfrac{N\delta\lambda}{m_1}-m_2P_2(t),$ which leads to that $\limsup\limits_{t\rightarrow +\infty}P_2(t)\leq\dfrac{N\delta\lambda}{m_1m_2}$ with $m_2 = \min\{c_1, c_2\}$. Therefore, the above analysis leads to the following result.

Lemma 2.1. The solutions $(T(t), I(t), V(t), W(t), Z(t))$ of model (1.2) with initial conditions (1.5) are non-negative and ultimately bounded.

It is easily seen that the model (1.2) possesses an infection-free equilibrium $E_0 = (T_0, 0, 0, 0, 0)$ with $T_0 = \dfrac{\lambda}{d}$. Define the basic reproduction number for viral infection $\mathfrak{R}_0$ of model (1.2) as

When $Z = 0$, an immune-inactivated equilibrium $E_1 = (T_1, I_1, V_1, W_1, 0)$ exists if $T_1, I_1, V_1, W_1 > 0$ satisfy

Since $T_1, V_1 > 0$, it then follows from (2.1) that $V_1\leq\frac{\lambda N(c_2+\alpha)}{c_1\alpha+c_1c_2+c_2\mu} = :\tilde{V}$. In order to show the existence of $E_1$, we define

Together with (1.3) and (1.4), it follows that $F'(V) > 0$. Moreover, for $\mathfrak{R}_0 > 1$ a simple calculation gives

Thus, if $\mathfrak{R}_0 > 1$, there exists $V_1\in(0, \tilde{V})$ such that $F(V_1) = 0$. This proves the existence of $E_1$ when $\mathfrak{R}_0 > 1$.

An immune-activated equilibrium $E_2 = (T_2, I_2, V_2, W_2, Z_2)$ exists if $T_2, I_2, V_2, W_2, Z_2 > 0$ satisfy the following equilibrium equations:

Thus, an immune-activated equilibrium $E_2$ exists if and only if $\mathfrak{R}_1 > 1$, where

Lemma 2.2. $Sign\{T_2-T_1\} = Sign\{I_1-I_2\} = Sign\{V_1-V_2\}$ $= Sign\{W_1-W_2\} = Sign\{\mathfrak{R}_1-1\}.$

Proof. It follows from (2.1) and (2.2) that

Then we have $(d+(1-\varepsilon)kf(V_2))(T_1-T_2) = (1-\varepsilon)kT_1(f(V_2)-f(V_1))$, which implies that $Sign\{T_2-T_1\} = Sign\{V_1-V_2\}$.

Moreover, it follows from (2.1) and (2.2) that $Sign\{V_1-V_2\} = Sign\{W_1-W_2\}$ and

we then have $Sign\{I_1-I_2\} = Sign\{V_1-V_2\} = Sign\{W_1-W_2\}$.

Then

It follows from (1.4) that $Sign\{V_1-V_2\} = Sign\{\dfrac{f(V_2)}{V_2}-\dfrac{f(V_1)}{V_1}\}$, then we have $Sign\{\mathfrak{R}_1-1\} = Sign\{V_1-V_2\} = Sign\{I_1-I_2\}$. This completes the proof. □

3.   Global stability analysis

In this part, the global stability of the equilibria $E_0$, $E_1$, and $E_2$ will be established by constructing Lyapunov functionals, which are motivated by ^{[20,21,22,23]}. Here, we will use the function $\varphi(x) = 1+\ln x-x$, which satisfies $\varphi(x)\leq0$ for $x > 0$ and $\varphi(x) = 0$ if and only if $x = 1$.

Theorem 3.1. If $\mathfrak{R}_0\leq1$, then the infection-free equilibrium of $E_0$ is globally asymptotically stable.

Proof. Let $T(t), I(t), V(t), W(t), Z(t)$ be any arbitrary positive solution of model (1.2) and recall that $T_0 = \dfrac{\lambda}{d}$. Define a Lyapunov functional $G_1(t)$ as

Computing the time derivative of $G_1(t)$ along the solution of model (1.2), which leads to

Clearly, if $\mathfrak{R}_0\leq1$, then $\dfrac{dG_1}{dt}\leq0$, for all $T, I, V, W, Z\leq0$ and $\dfrac{dG_1}{dt} = 0$ is satisfied if and only if $T = T_0, I = V = W = Z = 0$. Thus, the maximal compact invariant set in $\{G_1'(t) = 0\}$ is the singleton $\{E_0\}$. This proves the global stability of $E_0$ by applying the LaSalle invariance principle ^[24]. □

Theorem 3.2. If $\mathfrak{R}_1 < 1 < \mathfrak{R}_0$, then the immune-inactivated equilibrium $E_1$ is globally asymptotically stable.

Proof. Define

For convenience, let $u_{\tau} = u(t-\tau)$. Taking the time derivative of $G_1(t)$ along the solution of model (1.2) and using the equilibrium conditions (2.1) for $E_1$, we have

From Lemma 2.2, we have $I_1-I_2 < 0$. Moreover, $\left(\frac{f(V)}{f(V_1)}-\frac{V}{V_1}\right) \left(1-\frac{f(V_1)}{f(V)}\right)\leq0$ follows from (1.3) and (1.4). Then, we have $\dfrac{dG_2}{dt}\leq0$ and $\dfrac{dG_2}{dt} = 0$ if and only if $T = T_1, I = I_1, V = V_1, W = W_1, Z = Z_1$. Thus, the largest compact invariant set of $\{G_2' = 0\}$ is the singleton $\{E_1\}$. Therefore, the global stability of $E_1$ follows from the LaSalle invariance principle ^[24]. □

Theorem 3.3. If $\mathfrak{R}_1 > 1$, then the immune-activated equilibrium $E_2$ is globally asymptotically stable.

Proof. Define

Taking the time derivative of $G_3(t)$ along the solution of model (1.2) and using the equilibrium conditions (2.1) for $E_2$, we have

Similar to the proof of Theorem 3.2, we have $\dfrac{dG_3}{dt}\leq0$ and $\dfrac{dG_3}{dt} = 0$ if and only if $T = T_2, I = I_2, V = V_2, W = W_2, Z = Z_2$. Thus, the largest compact invariant set of $\{G_2' = 0\}$ is the singleton $\{E_2\}$. Therefore, the global stability of $E_2$ follows from the LaSalle invariance principle ^[24]. □

4.   Numerical simulations

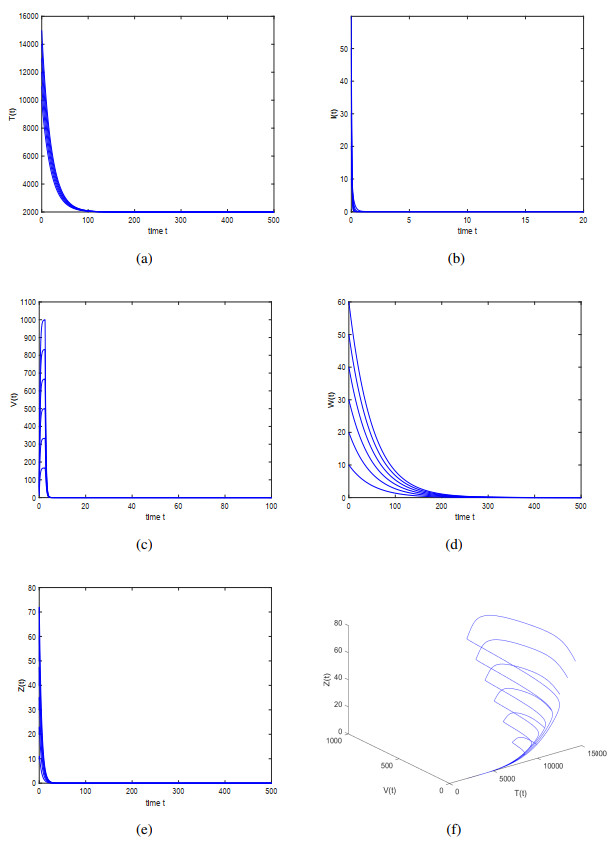
In this part, numerical simulations are carried out to validate the obtained results. Here, we select the function $f(V) = \frac{V}{1+mV}$. Choosing a certain parameter value of the model (1.2), and a simple calculation show that $\mathfrak{R}_0 = 0.016 < 1$, which implies that the infection-free equilibrium $E_0$ is globally asymptotically stable and the infection dies out (see Figure 1). When choosing parameter values, we have $\mathfrak{R}_1 = 0.1894 < 1 < \mathfrak{R}_0 = 15.9999$, then the immune-inactivated equilibrium $E_1$ is globally asymptotically stable, which means the immune response is not enough to inhibit the infection and the virus dominates the infection process, as shown in Figure 2. Moreover, choosing parameter values such that $\mathfrak{R}_1 = 1.8936 > 1$, which implies that the immune-activated equilibrium $E_2$ is globally asymptotically stable, and then immune cells can coexist with viruses within the host, as shown in Figure 3.

5.   Conclusions

In this paper, we studied an improved delayed viral infection model by incorporating nonlinear incidence and CTL immune response into the proposed model ^[11]. Thus, the model investigated here is including some existing literatures. We have shown that the model admits three equilibria $E_0$, $E_1$, and $E_2$. Moreover, two threshold parameters, $\mathfrak{R}_0$ and $\mathfrak{R}_1$ are defined. By constructing corresponding Lyapunov functionals, we have demonstrated that the infection can be inhibited when $\mathfrak{R}_0\leq1$. The infection will persist and the immune response can not be activated when $\mathfrak{R}_1 < 1 < \mathfrak{R}_0$. Both the viruses and immune cells can coexist and reach a steady state provided that $\mathfrak{R}_1 > 1$. The obtained results reveal that both nonlinear incidence and intracellular delay cannot change the stability of the model. Besides, the obtained global dynamics of the model are a theoretical supplement to ^[11], which has not been considered.

Literatures reveals that cell-to-cell transmission is vital to the spread of viruses in vivo ^[25,26,27], and only virus-to-cell infection is taken into consideration in this paper, and whether the cell-to-cell infection can retain the stability or not is an interesting question. Besides, the time for activating the immune response is not included in the model, i.e., the immune time delay. How immune time delays impact the dynamical behavior of the model is also worth studying. We leave these for future work.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

This work was founded by National Natural Science Foundation of China (#11701445, #11971379, #12271431), by Natural Science Basic Research Programm in Shaanxi Province of China (#2022JM-042, #23IP114).

Conflict of interest

The authors declare there is no conflicts of interest.