On forbidden subgraphs of main supergraphs of groups

1.   Introduction

The spleen is the largest lymphoid organ in the human body; its anatomy and physiology fascinate physicians of the early medical era. Despite being a lymphoid organ, the spleen has many other functions unrelated to the lymphoid system and provides an avenue for interaction with all types of blood cells throughout life [1]–[3]. Embryologically, the spleen develops from single or multiple bulges of intraembryonic splenic mesoderm that appears first as a mesenchymal ridge within the dorso-mesogastrium during the fifth week of embryonic life. It took over hemopoiesis from the yolk sac to become an active center by the second trimester and give way to bone marrow as the sole hemopoietic center from the fifth month onward [4],[5].

In the evolutionary trend, the spleen is found in all vertebrates and functions primarily as a blood filter and a sentinel organ for lymphocyte circulation [6]. The spleen location is intra-abdominal, intraperitoneal, sub-diaphragmatic, and encapsulated. The circulation of systemic blood through white pulp and marginal zone (MZ) allows for efficient acquired immune response. Simultaneously, the MZ strategic leucocytes population of macrophages and B1 B cells ensure non-specific immunity [7],[8]. Through phagocytosis, spleen resident innate immune cells provide the human body with proactive defense against non-self [1].

Spleen, even though a non-essential organ in humans, but it has notable roles in the systemic circulation's homeostasis. Deficiencies of these functions become apparent if, for any reason, the individual loses the spleen (asplenia) functionally or anatomically: as a result of disease, surgery, or accident [9]. Individuals who have lost their spleen are prone to a myriad of infection cutting across viral, parasitic, and bacterial pathogens, especially capsulated bacteria [10]–[12].

Opsonized pathogens are mopped up from blood by the spleen and liver, but poorly opsonized microbes like Streptococcus pneumoniae are only removed from circulation by the spleen [11]. Upon splenectomy follows the massive loss of immunoglobulin (Ig)-M memory B cells, loss of splenic clearance of opsonized bacteria due to loss of marginal zone monocytes. Henceforth, a higher prevalence of severe infections within the first year after splenectomy, with half of the cases occurring within the first month [10],[13].

Spleen provides a site for the inception of tumor immune tolerance even though controversial, but some studies support this view as splenectomy was shown to reestablish anti-tumor immunity [14]. Oncogene-induced senescence, a form of cancer signaling, was shown to induce the formation of myeloid-derived suppressor cells (MDSC) in the spleen, bone marrow, and blood in murine studies; after that, MDSC prime an immunosuppressive environment [15]. In animal studies of the pancreatic cancer model after splenectomy, the study animals recorded increase peritoneal metastasis and progressive tumor growth [16],[17].

2.   Spleen microanatomy

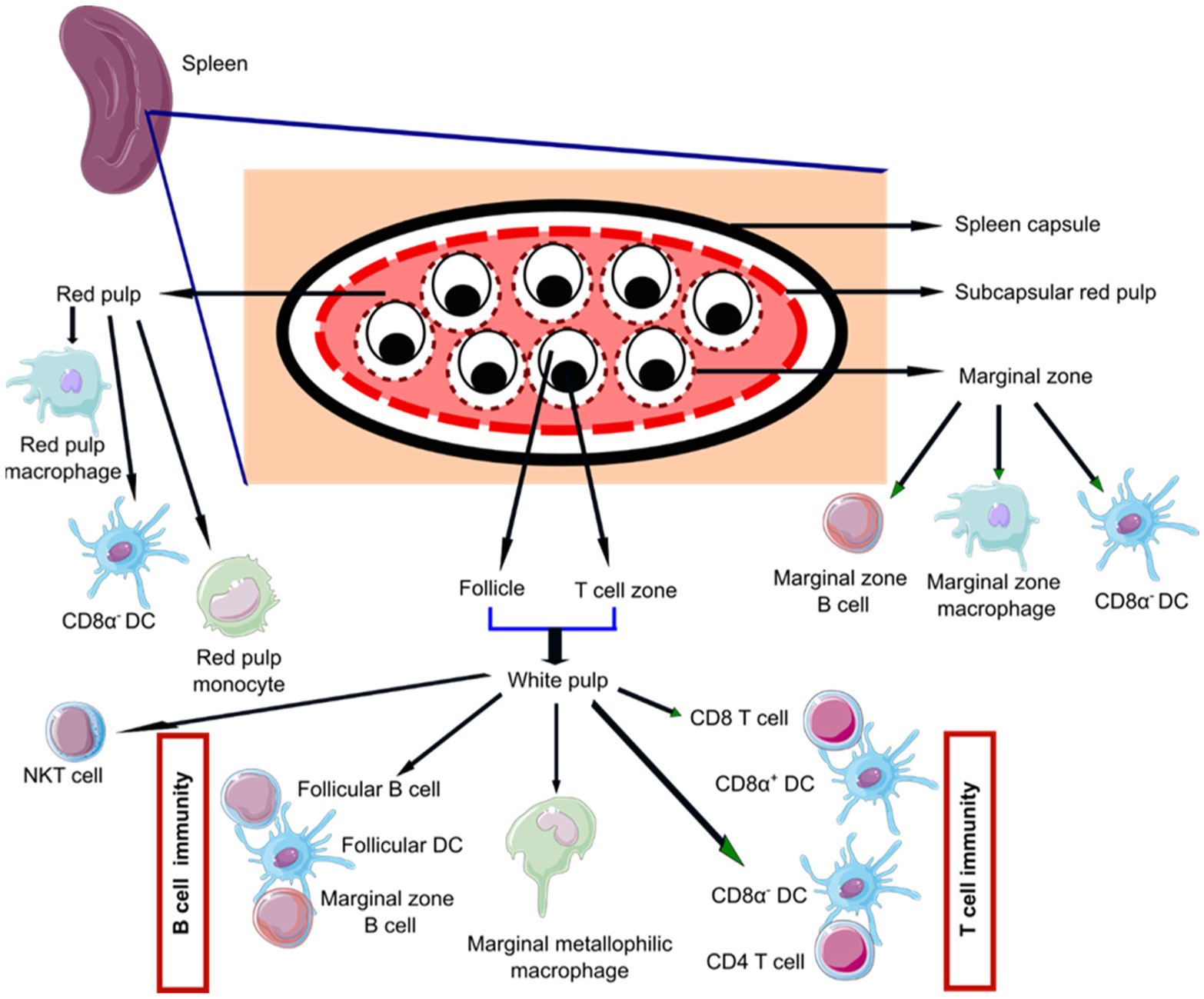
The spleen's histological structure is unique and forms the spleen's framework as a blood filter. The spleen is a sentinel hemodynamic organ for red blood cell homeostasis. The strategic position of different immune cells, as shown in Figure 1 below, the segregation of distinct lymphocyte zones, and efficient blood circulation all favor splenic function. Above also favors innate immune responses to blood-borne antigens and the initiation of the adaptive immune response [18]. The spleen mass comprises one-fourth white pulp and about three-quarters red pulp; both structures develop in the second trimester of intrauterine life [19]–[21].

The massive red pulp is structurally composed of splenic cords and splenic (venous) sinuses [22]. The splenic cords, otherwise called Cords of Billroth, are meshwork found in between sinusoids providing them with a supportive framework; they are composed of reticular cells, reticular fibers, fibroblasts, lymphocytes—mainly CD8⁺ T cells, and abundant macrophages [1],[23]. The reticular cells bear retractile fibers within them functioning like myofibrils, extending and retracting, thereby controlling blood supply into the intervening sinuses. On the other hand, the sinuses are blood vessel channels that possess slit holes between their lined endothelium [1].

Functionally, some scientists consider the MZ as the third compartment of the spleen apart from white pulp and red pulp [24]. The interspersed location of white pulp is similar to a lymph node structure [18]. The white pulp is made up of distinct areas of lymphocytes that are found around the central artery, which branches up from the reticular artery. The area immediately surrounding the central artery is the periarteriolar lymphoid sheaths (PALS), which contain T cells predominantly and is a site for interaction of antigen–bounded DCs with lymphocytes [25].

The B cell areas are scattered adjacent to the PALS as B cell follicles; small arterioles from the central artery supply them—they housed mainly B cells. The follicles are surrounded by the MZ that harbor mainly non-dividing MZ B cells [26],[27]. The MZ B cells do not require T cell help, they respond to bacterial capsular polysaccharide antigens by producing low affinity, mainly IgM antibodies. The MZ contains few T cells and a large population of macrophages. Two distinct populations of MZ B cells were recognized; IgM producing B1 B cells mention above and B2 marginal zone B (MZB) cells that give rise to regulatory B cells [20],[28].

MZ continuously screened blood for opsonized bacteria, other soluble antigens, and immune complexes [29]. Some B cell follicles contain germinal centers, which are hot centers for antigen activation; they contain B cells, T cells, macrophages, and supportive follicular DCs (FDCs) [23]. The B cells here display a high degree of proliferation, hypermutation, and class switching coupled with differentiation of high-affinity B cells to plasma cells and memory B cells [30]. A rim of B cells encircles this hot area termed corona [31]. The follicles occur in areas of central artery branching [27].

The spleen is massively perfused, being a principal blood filter, receives oxygenated blood through the splenic artery. It is the largest branch of a coeliac artery that is long and tortuous, which branches up from the abdominal aorta. Usually, an accessory splenic artery joins the major splenic artery, thereof pierces the spleen through splenic hilus and branches into smaller tributaries, after that penetrates the spleen trabeculae, thereby acquiring the name trabecular arteries [7],[32]. The segmental arteries pass deep into the spleen parenchyma with their size progressively decreasing and then gives off smaller branches called central arteries surrounded by a sheath of lymphoid tissue; the white pulp [33],[34].

Smaller arterioles from the central artery supply the white pulp capillary bed, some curved within the marginal zone supplying its capillaries and ending in the marginal sinus. While some arterioles reenter the white pulp and become follicular capillaries or traverse the MZ and terminate in the red pulp's reticular meshwork. Branches from the central artery that supply the red pulp are termed penicillar arteries. Venous tributaries of corresponding names join to form the splenic vein that empties into the portal vein [7],[27],[31],[33]. The perifollicular zone encircles the MZ, PALS, corona, and follicles [35].

Scientists study mice spleens due to similarities and ease of access. However, disparities do occur. Murine MZ is located between white and red pulp. The human spleen has a different architecture of MZ [36]; somewhat human spleen has a germinal center, mantle zone, and superficial zone in their secondary follicles. Humans have sheathed capillaries (splenic ellipsoids) in their red pulp absent among mice and rats. Substantially, humans possess open circulation systems in the spleen in contrast to murine spleen with both open and close circulation [23]. However, the human spleen's MZ is devoid of marginal sinus and marginal metallophilic macrophages (MMM), and this region cannot be defined by B-cells. Furthermore, humans have CD271⁺⁺ capillary sheath cells also express on FDCs in follicles and fibroblastic reticulum cells (FRCs) in PALSs [34].

Surgical splenectomy is indicated in a spectrum of patients with hematological disorders—Non-Hodgkin's lymphoma, Hodgkin's lymphoma, chronic and acute lymphocytic leukemia, chronic and acute myeloid leukemia, idiopathic thrombocytopenia, hemolytic anemia, refractory anemia, myelofibrosis, hereditary spherocytosis, among others. If thrombocytopenia, symptomatic splenomegaly, or anemia develops, splenectomy is usually often undertaken in the hematological conditions mentioned above. Also, a splenectomy can be undertaken on these patients for diagnosis or treatment modality. However, significant morbidity and mortality are associated with splenectomy [37]. Other indications for splenectomy include splenic cysts, spontaneous splenic rupture, splenic abscesses, splenic haemangioma, splenic infarction, and felty's syndrome [38]. Furthermore, scientist advocates non-surgical less invasive therapeutic modalities for treating splenic lymphomas [39]. Portal hypertension usually complicates surgical splenectomy yielding pulmonary hypertension in the long run [40].

In recent times, surgeons prefer splenotomy for splenic abscesses that complicates metastasis foci, immunodeficiencies, trauma, or infarction; to avoid thromboembolic and infectious complications after splenectomy [41]. The failed spleen physiology becomes evident post-splenectomy (surgical or physiological) when the patients become prone to overwhelming post-splenectomy infection, characterized by impaired: antibody formation, antigen-antibody complex clearance, and deficient phagocytosis. Particularly encapsulated bacteria infection become fulminant [42]. Minimal invasive splenotomy is usually indicated for parasitic and non-parasitic splenic cyst, and the outcome relies on the peripheral location of the cyst and the spleen's blood vessel pattern [43].

3.   Spleen and innate immunity

The MZ housed two distinct populations of macrophages, CD169 (siglec-1, sialoadhesin) metallophilic macrophages and SIGN-R1 expressing marginal zone macrophage. Other MZ inhabitants are MZ B cells, a large temporary population of DCs, and other lymphocytes in transit to a white pulp. Interaction of these leucocytes mentioned above was found to bridge innate immune response and adaptive immunity, thereby forming robust defenses against foreign antigens [24].

Systemic blood flow to the MZ is a slow circulation bearing on MZ's histological structure; this is crucial for efficient phagocytosis of microorganisms compared to fast flow circulation of the red pulp open and close circulations [44],[45]. Splenic macrophages are efficient in clearing effete red blood cells, opsonized bacteria, or parasitized red blood cells harboring protozoans like plasmodia or babesia [46].

In murine studies, splenic NK cells are extensively studied [47]; in humans, NK cells express CD56 and CD16; they are lymphocytes devoid of CD3 and CD 19, which are T and B cell lineage markers, respectively [48]. Splenic NK cells also express NKp46⁺ [49],[50]. The NK cells are members of the innate lymphoid cells (ILCs), produce large interferon-gamma (IFNγ), depend on T-bet, and rely on Tbx21 for development [49]. A large population of splenic resident NK cells inhabits the red pulp, with scanty NK cells dwelling in the germinal centers and follicles. These innate cells are cytotoxic. They recognize their target cells independent of major histocompatibility complex (MHC) molecules, which contrasted with CD8⁺ T cells [51].

The role of human killer immunoglobulin-like receptors (KIRs) on NK cells is to control cytotoxicity on these lymphocytes. In essence, NK cytotoxicity relies on the balance between KIRs inhibitory (3DL1-3, 2DL1-3, and 2DL5) and excitatory (3DS1, 2DS1-2DS5) signals [52], but KIR2DL4 encodes for both [53]. The KIRs are expressed by genes on chromosome 19q13.4 in humans and are richly polymorphic [54]. The MHC I act as a ligand for KIRs [55], while NKG2 receptors are non-polymorphic and conserved, so non-polymorphic MHC I (HLA-E) serve as their ligand to potentiate inhibitory signals [54].

Infections with encapsulated bacteria such as Streptococcus pneumoniae, Neisseria meningitides, and Haemophilus influenzae usually resist the ultimate aim of complement immune response of membrane attack complex [56],[57]. Such pathogens are only responded to in the spleen. Individuals that undergo surgical splenectomy or dysfunctional asplenia tend to suffer the brunt of invasive pneumococcal infections [58]. It is noteworthy that the polysaccharide (pathogen-associated molecular pattern) present on encapsulated bacteria is tightly recognized by DC-specific C-type lectin (DC-SIGN) pathogen recognition receptor on MZ macrophages, an antigen recognition phase for the initiation of receptor-mediated phagocytosis of this pathogen [59].

Studies have shown that different macrophages can engulf Listeria monocytogens, but marginal zone macrophages were among the only immune cells that demonstrated a lethal effect on L. monocytogens. Furthermore, eliminating these phagocytes leads to loss of infection control with L. monocytogens [60]. CD169 on metallophilic macrophages is key to the recognition of both meningococci and Campylobacter jejuni [61].

4.   Spleen and adaptive immunity

As discussed above, the spleen has direct contact with systemic blood and doubles as a richly vascularized organ [62]. Within it are both fast and areas of slow circulation that are continually surveyed by immune cells. Both innate and adaptive immune responses are initiated in the spleen [63], and the whole blood in the body has to transit through the spleen [64]. Most of the spleen molecular and cellular studies are an extrapolation from the murine study, this is due to similarities of this tissue across mammalian species, but the species-specific difference is expected among humans [65].

Spleen is a house for a humoral immune response to T cell-dependent and T cell-independent antigens responses [20]. The PALS T cell population is a mixture of CD4⁺ and CD8⁺ T cells with a preponderance of CD4⁺ T cells. However, this is a reciprocal of what is obtained in the red pulp where CD8⁺ T cells predominate, reflecting the two splenic compartments' functional diversity. But the CD4⁺/CD8⁺ ratio in PALS can be reversed in viral infections [20],[66].

Interdigitating dendritic cells (IDC) in PALS or other DCs in transit after picking a pathogen they recognized using conserved pathogen recognition receptors; the DCs processed the antigen and usually presented it in association to MHC class II molecule to inactivated CD4⁺ T cells providing signal one for their activation [67].

The DCs also express CD80/CD86 and CD40 (B7-1 and B7-7) molecules to bind CD28 and CD40L (CD154) on the corresponding CD4⁺ T cell to provide co-stimulation required for T cell activation. The co-stimulation provides CD4⁺ T cells with the necessary instruction to undergo clonal expansion and cytokine secretion sequel to activated signaling pathways [23],[68]. On the other hand, DCs can also present antigens in association with MHC class I to CD8⁺ T cells within the spleen [31].

An enormous number of B cells that succeeded both positive and negative selection in the bone marrow exit the marrow as transitional 1 (T1) B cells to reach primarily spleen where they attain their full maturation and become transitional 2 (T2) B cells at the B cell follicles. On arrival, the T1 B cells are IgM^highIgD⁻CD21⁻CD23⁻ being immature, but the matured T2 B cells are IgM ^highIgD⁺CD21⁺CD23⁺ [69]. The T1 B cells are restricted to the PALS, while the T2 B cells move easily to the inner B cell follicles [70].

As reviewed by Pabst [35], T and B lymphocytes reaching the spleen are first stationed in MZ, then transit to PALS and proceed to the corona. When T cells migrate through the MZ, they are directed to the B cell zone by CXCL13, whereas CCL19 and CCL21 ligands attract T cells to T cell zones within the spleen white pulp. The same CCL19 and CCL21 are responsible for DC homing to T cell zones [7],[25].

4.1.   Peripheral tolerance induced by the spleen

The human body is equipped with robust immune mechanisms that distinguish foreign and tolerate self, thereby guarding against autoimmunity. The central tolerance is not entirely efficient, so peripheral tolerance is continuously employed as a complementary process. Tolerance is characterized by systemic unresponsiveness to repeated exposure or a high amount of antigens or mucosal contact to antigens-oral tolerance [71]. Immune cells in different splenic compartments like MZ, red pulp, and white pulp are the critical players for the induction of peripheral immune tolerance.

The spleen induces peripheral immune tolerance through several distinctive mechanisms. Buettner and coworkers' murine study has shown that the spleen support skin tolerance through B cells' proliferation, which formed a germinal center in the spleen. In contrast, oral tolerance was spleen independent [72]. In another murine study, the transfusion of ethylene carbodiimide (ECDI)–fixed donor splenocytes with rapamycin prolongs skin allograft survival in the study mice [73].

The spleen contains unique subsets of macrophages known as MZ macrophages (MZMs), marginal metallophilic macrophages (MMM), white pulp macrophages, and red pulp macrophages. The development and differentiation of splenic macrophages are extensively regulated by Irf8, Spic, and Nr1h3 transcription factors [74]. Besides these factors, the differentiation of MZMs in the spleen also depends on nuclear factor LXRα, which may control tissue macrophages [75].

The MZMs induce peripheral tolerance upon interacting with apoptotic bodies entering the spleen from circulation. A mice study by McGaha and colleagues showed that MZMs were the central players for the clearance of apoptotic cells to minimize the immunogenicity to autoantigens, thereby suppressed the immune response to apoptotic cells in the spleen [76]. Metallophillic macrophages (CD169⁺), a subset of MZ macrophages in the spleen, were also critical for immune tolerance. Recent findings showed that functional CD169⁺ macrophages rely on BATF3-dependent DCs to cross-prime CD8⁺ T cells [77],[78].

Systemic challenges with apoptotic cells induced CCL22 on CD169⁺ cells, resulting in FoxP3⁺ Tregs and CD11c⁺ DCs rapidly accumulating and becoming activated in the spleen. The accumulated cells produce anti-inflammatory cytokines: Transforming growth factor-beta (TGF-β) and interleukin (IL)-10, leading to immune tolerance induction [79]. The MZMs, apart from efficiently clearing the apoptotic cells, also selectively engulf the dying cells and cross-present the processed dead cell-associated antigens in the spleen [80],[81].

Furthermore, MZMs express an uptake receptor SIGN-R1, a C-type lectin that captures microbial polysaccharides in the spleen and directly bound the complement C1 subcomponent. Moreover, the C1q leads to innate resistance by regulating the C3 deposit on the bacterial cell wall [82]. Scientists reported that when C1q binds to human apoptotic lymphocytes, it decreases the cleavage of procaspase-1 and IL-1β, thereby inhibits inflammasome activation and suppression of inflammation [83].

Splenic marginal zone Dcs (MZDCs) CD8⁺CD11b⁻ are other vital cells for maintaining peripheral T cells tolerance via clearance of self-antigens embodied in apoptotic cells. In health, phagocytosis of apoptotic cells by DCs and presentation of self-peptides to T cells are the critical steps of peripheral tolerance [18],[84]. In a mice study, splenic MZDCs phagocytosed the apoptotic cells through complement receptors like CR3 and CR4. The MZDCs exhibit decreased messenger ribonucleic acid (mRNA) expression of pro-inflammatory cytokines such as IL-1, IL-6, IL-12, and tissue necrosis factor-alpha (TNF-α) without effect on TGF-β (anti-inflammatory mediators) to maintain peripheral tolerance [85].

Another murine study revealed that splenic CD8α⁺CD103⁺CD207⁺ DCs, a novel subset of DCs in MZ of the spleen, played the dominant phagocytosis of blood-borne apoptotic cells—their transient ablation leads to failure of peripheral tolerance [86]. Antigens arriving in the blood-stream are processed within the spleen by different immune cells that will prime anti-tumor immunity, immune tolerance, or pro-inflammatory immune response based on the antigen's nature as outlined in Figure 2.

The CD8⁺CD205⁺ splenic DCs were also reported in a mice study as specialized cells for the production of TGF-β that permits the peripheral tolerance through induction of Foxp3⁺ Tregs [87]. Spleen also contains a subpopulation of MZB cells, which are novel for tolerance. The MZB cells stimulated by LPS (in TLR4 dependent manner) on bacteria expressed BAFF (B cell-activating factor) and TACI (transmembrane activator, calcium modulator, and cyclophilin ligand interactor). The two interact to upregulate Fas receptor of the same MZB cells to undertake Fas-mediated killing [88].

Surrogate light (SL) chains regulate the tolerogenic properties of MZB cells generally expressed on the surface of pre-B cells at the different developmental stages. Researchers have shown SL's regulatory properties on central and peripheral B-cell tolerance and inhibition of anti-DNA antibody production by MZB cells in a mice study [89]. Another study in mice showed that MZB cells expressed CD1d for efficient generation of Treg cells in CD1d-reactive NKT cells dependent tolerance [90].

A study reported that innate-like regulatory B1a cells (CD43^+veCD19^hiCD5^+veIgM^hiIgD^lo) constitute 80% of spleen B cells that specifically recognize and augment tolerance to apoptotic cells via expression of IL-10 and natural antibodies (Nabs), henceforth prevents autoimmune-mediated inflammation [91]. CD68⁺F4/80⁺ red pulp macrophages are a distinct type of splenic subset whose development in the red pulp of the spleen is essentially controlled by Spi-C, a PU.1 related transcription factor [92].

Scientists reported the tolerogenic function of F4/80⁺ macrophages by inducing antigen-specific efferent CD8⁺ Treg cells in the mice study. In addition to red blood cell recycling and iron homeostasis, the F4/80⁺ macrophages also induce peripheral tolerance [93]. In a mice study, splenic NKT cells expressed chemokines, RANTES in a CD1d dependent manner, which recruited F4/80⁺ antigen-presenting cells (APCs) and CD8⁺ T cells for the generation of CD8⁺ Treg cells [94].

4.2.   Anterior chamber-associated immune deviation (ACAID)

Spleen forms a vital part of the immune system's antigen-specific deviation that is widely studied in murine. An antigen introduced into the eye's anterior chamber in the absence of danger signals is identified, phagocytosed, processed, transported, and presented in the spleen and thymus by the F4/80⁺CD11b⁺ ocular macrophages. These macrophages induced the generation of NKT cells (NK1.1⁺CD4⁻CD8⁻), iNKT, CD8⁺ or CD4⁺ Tregs, γδ Tregs, and MZ regulatory B immunosuppressive cells in the spleen's MZ [95]. Splenic B cells were shown to be essential for the generation of Treg cells in the spleen for the induction of ACAID [96]; they express both MHC I and MHC II [97].

An eye is considered an immune privilege site in the human body. Subretinal injection of antigen resulted in systemic downregulation of pro-inflammatory cytokines in response to the induction antigen [98]. Murine studies have documented corneal allograft rejection after splenectomy; however, scientists reported no corneal graft rejection four years after splenectomy in human subjects [99].

Cunnusamy and colleagues reported two distinct Tregs populations for the induction of ACAID; CD4⁺CD24⁺ Tregs formed in response to corneal allograft to suppress delayed-type hypersensitivity (DTH) and CD8⁺ Tregs that are induced by anterior chamber injection of alloantigens [100]. The V-domain immunoglobulin suppressor of T cell activation (VISTA) is expressed in the cornea, and stromal APC of mice that received a corneal transplant, and anti-VISTA mAbs treatment result in less corneal graft survival with an associated low level of splenic CD8⁺CD103⁺ Tregs [101].

Moreover, AIRE can repress the chromatin accessibility and oppose BRG1—a transcription activator across the genome, thereby precisely regulating peripheral tolerance [102]. The splenocytes are extra-thymic Aire-expressing cells, equipped as professional APCs, and regulate the expression of tissue-specific antigens (TSAs); thus, they play a significant role in self-tolerance [103].

The AIRE⁺ splenic cells include a particular type of bone marrow-derived APCs. As reported by Gardner and colleagues, they express the epithelial cell adhesion molecule (EpCAM). The CD45^low APCs can induce CD4⁺T cell tolerance by functional anergy without involving Treg cells, a vital tolerance mechanism for controlling autoreactive T cells that escape thymic selection [104]. Again, splenic tolerance to apoptotic cells is also influenced by the tryptophan catabolizing enzyme indoleamine 2,3-dioxygenase (IDO) [105].

Ravishankar and coworkers reported in their murine study that systemic challenge with apoptotic cells induced IDO expression selectively in the MZ of the spleen. The IDO expression coordinated with CD169⁺ macrophages limits innate and adaptive immunity to apoptotic self-antigens. Moreover, inhibition of IDO led to inflammatory pathology mediated by systemic autoimmune diseases [105].

5.   Splenectomy and anti-tumor immunity

The spleen is a reservoir of different immune cells, some with anti-tumor immunity like T cells, B cells, NK cells, and macrophages. Weiberg and coworkers showed in a murine study that after in vitro antigen stimulation and oral immunization of donor mice, antigen-specific splenic IgM⁺ B cells could migrate into the intestinal lamina propria of recipient mice. The B cells predominantly switch to IgA⁺ plasma cells, thereby play significant role in the gut immune response; they serve as a reservoir of immune cells that migrate to the site of infection [106]. Researchers have shown that CD169⁺ macrophages in the lymphatics induced potent anti-tumor/anti-pathogen cytotoxic CD8⁺ T cells with concomitant suppression of the CD4⁺ T cell response [107].

Scientists studied the association of splenectomy and the overall risk of cancer in patients and found that splenectomy patients have a higher risk of developing cancer [108]. Conversely, the accumulation of myeloid suppressor cells in the spleen inhibits the spleen's anti-tumor activity during tumor angiogenesis [109]. Anti-tumor properties of the spleen were well studied in splenectomized mice, as discussed in this review. Nevertheless, the effect of splenectomy on the hematogenous metastases of malignant tumors remains controversial.

Recently, a study with a nude-mouse model of human pancreatic cancer demonstrated tumor recurrence detected as multiple peritoneal seeded nodules due to NK cells' decreased activity in a splenectomized group [17]. Another recent study in an orthotopic syngeneic murine pancreatic cancer model showed that splenectomy was associated with agammaessive tumor growth patterns and enhanced peritoneal seeding with low tumor-infiltrating ratios of effector T cells to FoxP3⁺ Treg cells [16].

Spleen NK cells played an essential role in suppressing lung metastasis at the early stage of liver tumors in mice [110]. Higashijima and coworkers showed that splenectomy of study mice significantly decreased the number of Treg and NK cells in the mesenteric lymph node and enhanced liver metastasis [111]. Another study found a sharp decrease in peripheral T cells in asplenic tumor-bearing rats, resulting in enhanced tumor growth [112]. Several studies have shown that splenectomy has a negative effect on the survival of patients with gastric and colonic cancer [113]–[116].

On the other hand, Sonoda and colleagues reported in their mice study that splenectomy decreased the growth rate of metastatic lung lesions as well as serum levels of vascular endothelial growth factor (VEGF) and basic fibroblast growth factor (BFGF) [117]. Another study showed that splenectomy inhibits the early stages of tumorigenesis and reduces the rate of malignant transformation of benign tumors in female rats but did not prevent the progress of carcinogenesis [118].

6.   Conclusions

The spleen is the largest organ of the lymphatic system responsible for the induction of peripheral tolerance through its different immune components like MZMs, MZDCs, MZB cells, red pulp macrophages, and NKT cells. Splenocytes as extra-thymic Aire-expressing cells also induce self-tolerance without involving Treg cells. The spleen plays a vital role in the establishment of ACAID. Moreover, splenic tolerance to apoptotic cells may also induce the IDO enzyme. Splenectomy has a controversial impact on cancer; tumorigenesis, recurrence, and metastases.