Analysis of capital asset pricing model on Deutsche bank energy commodity

1.   Introduction

A commodity is any fixed physical substance that investors buy or sell in the form of future contracts. Commodities are also common assets that people invest in apart from cash, shares, hedge funds among others. Among the commodities we have are energy commodities such as metals, livestock, oil, cotton, etc. Commodities can be described as physical substances produced in large quantities to meet the needs of the people. Crude oil is one of the most volatile and important energy commodities that are traded around the globe. The price of the commodity is determined by the whole market. The market risk is one of the risks that affect a commodity and cannot be diversified. However, a commodity is a type of standardized asset to be invested in.

In portfolio management, the selection of the right and best portfolio that will meet the goal and desire of the investor or organization is very important and cannot be ignored. This gives rise to the checking and analyzing the best method for the selection of the best method and model. Optimization helps to select the best and to make the right decision. Therefore, optimization is of great importance in finance since it plays a great role in decision making. 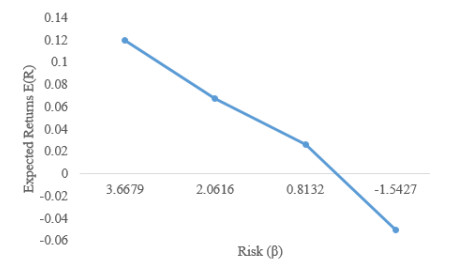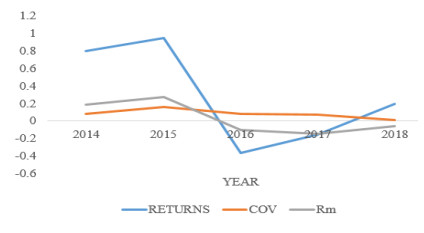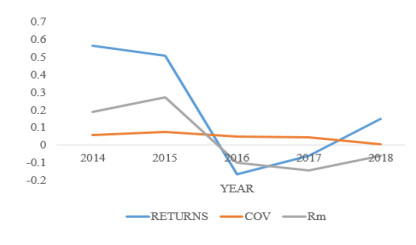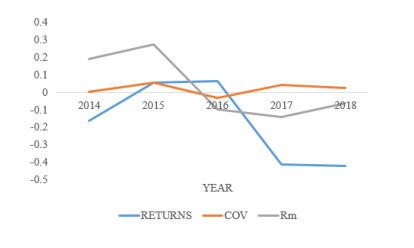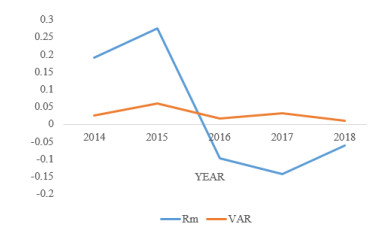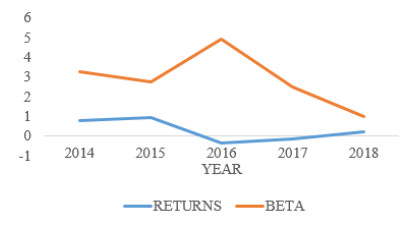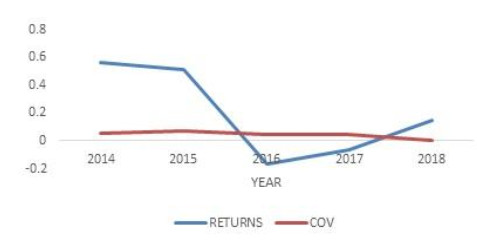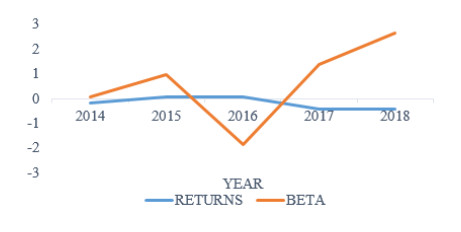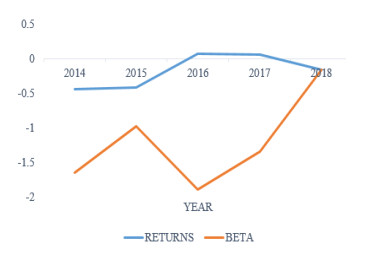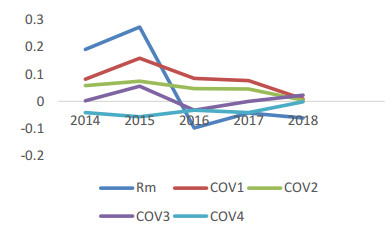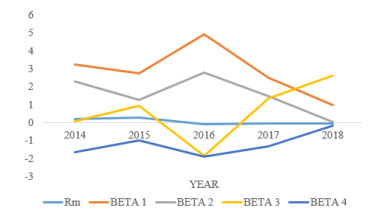Among the various models used in analyzing portfolio is the Capital Asset Pricing Model (CAPM). The CAPM shows the connection that exists between asset’s risk and it’s relating expected returns.

Majorly, People invest to make a profit. They aim to maximize their returns i.e. they are interested in high return while they also consider the risk involved. According to Markowitz (1952), he gave the assumption that investors are risk-averse. This means that they try to avoid risk and when they are presented with assets of the same expected return they prefer an investment with lower risk. Markowitz, who is referred to as the father of modern portfolio theory in his article gave more explanation on diversification. He emphasized that diversification cannot eradicate all the variance. The variance and covariance are the statistical tools used to measure the risk which is also the Beta involved in an investment. Risk in some research work has been classified to be uncertain. Knight (1921), expatiated on the differences between uncertainty and risk. When the randomness facing an organization or an individual can be expressed or defined in terms of probability and values can be assigned to it, the risk is involved. Once a value cannot be assigned to it, uncertainty is involved. Latunde and Bamigbola (2016) introduced an optimal model that is based on the theory of uncertainty, analysed and utilized recommended control policy for sustainability development in Latunde et al. (2020), thus the optimal control model of capital asset management and was refined and characterised as a multifactor model in Latunde (2020). This uncertainty theory associates at least two parameters that are used to measure the state of investments of an individual, Latunde and Bamigbola (2018). The condition of the risk that is involved in asset management is known by these parameters associated with uncertainty. However, the uncertainty capital asset model as applied to a real-life problem in Latunde (2019) where some optimal values were derived. The approach of applying sensitivity analyses to the model uncertainty as post optimal solutions were also utilized in Latunde et al. (2016), Latunde et al. (2019) and Latunde et al. (2020).

The beta can also be said to measure how volatile a stock is compared with the volatility of the market. Stocks with high risk are said to be more volatile than the stock with lower risk. When beta is greater than one, the asset is said to be volatile and it tends to move up and down with the market while beta that is negative reveals that the investment goes down when the market goes up and vice versa. When the beta is of high value, the stock price is more sensitive to news and information. This makes the investment with high beta to move faster than anyone with lesser risk. Also, the return of an asset is of great importance and the mean is used to measure the return of an asset. When a company gets a negative return, it is said that the company has a financial loss. In other words, the company or business loses more money than it brings in. In some cases, negative returns are experienced during the early years of starting the business because of the amount of capital that goes into the business at the initial stage. Negative returns are also gotten in an investment if the risk is high and there is no compensation for it. Whenever the covariance which is one of the statistical tools used is negative the market risk will be negative. Being volatile, there is a tendency that the asset price will move up and down with the market. The thought of not putting all eggs into one basket but that it should be put into different baskets explains what diversification means. Diversification is a theory introduced by Markowitz. It can be explained further that instead of investing in only one asset, one can invest in more than one asset. The collection or combination of these assets is called a portfolio. It can also be understood in Markowitz (1952) where a portfolio’s expected return is of a better value and the risk is reduced to a considerable point rather than the choice of only one asset. Sharpe (1964) made further research on the Markowitz model. He explained using the CAPM, the connection that exists between the expected return and the risk associated. In his model, he explained that not all risks affect the portfolio but the systematic risk does. Unsystematic risk is a kind of risk that pertains to industries or businesses. This type of risk can be diversified i.e. it can be shunned but the Systematic risk can’t be shunned. It is a kind of risk that influences the entire market. The variance of risk-free asset is zero and the expected return of the risk-free asset is the same as the return of the risk-free asset. Konno et al. (2005) also discussed the use of mean-absolute deviation (MAD) to estimate and measure risk. The CAPM is often used in finance as an asset pricing model because of its simple calculation, its ability to predict the risk with its expected return and also it gives a more realistic and useful result. The model considers the systematic risk which other models failed to consider. Since the estimation is very crucial in finance, CAPM is the most common pricing model used. One of the limitations of the CAPM is that the risk-free rate is always measured by considering the short-term government securities which are mostly the treasury bills issued by the government. The Government set these type of securities to finance and provide fundings for some of their projects. The government makes full repayment of the actual amount invested and these securities are also referred to as a conservative investment with low risk because it is issued by the government.

In this work, we study crude oil assets of Deutsche Bank as one of the German banks that specializes in foreign trade and asset management. We employ a mathematical model called the capital asset pricing model to show the relationship between risk and return. With the model, we investigate the relationship and effect of risk on the expected return of investing in four common Deutsche Bank (DB) crude oil assets (DTO-DB Crude Oil Double Short, SZO-DB Crude Oil Short, OLO-DB Crude Oil Short, DBO-Invesco DB Oil Fund). The outcome of the study, with the effect of the asset covariance, is analyzed and described using figures.

2.   Literature review

The capital asset pricing model designed by Sharpe is reviewed herein. Markowitz (1952) initiated the modern portfolio theory which had been widely applied in finance. He considered the expected return as a desirable thing and the risk which is calculated to be the variance is not so desirable. This results in investors looking for ways of minimizing the risk while maximizing the expected return. Markowitz (1956) also introduced a model known as the mean-variance model which is used in minimizing and maximizing the risk and expected return in investment. Many researchers had employed the use of the mean-variance model. Owusu et al. (2016) used the Markowitz model to allocate pension fund in the National Pension Scheme in Ghana.

Sharpe (1964) presented the capital asset pricing model which exists as an extension to Markowitz’s works. He presented the CAPM to be a model that shows and describes the risk of an asset and its effect on the expected return. The model depends on the systematic risk which is also known to be independent. Lintner (1965) also worked on the CAPM and considered the borrowing and lending at a risk-free rate. Sharpe and Lintner in their work considered the difference between the risk-free return and the expected return of the risky asset to be the risk premium. Capital asset pricing model plays the role of describing the linear relationship that is between systematic risk and the expected return of an asset. Also, it is said that Treynor (1961) started the theory of capital asset pricing model without the knowledge of others who introduced CAPM after. According to French (2003), Treynor’s CAPM was developed by employing the idea of experiment space to describe the risk. According to Tobin (1958), he discussed and expatiated on the idea that as investors decide to make any investment in assets that requires risk-free lending and borrowing at a level of risk, it maximizes the return and minimizes the risk. This explanation is known as the Separation theorem. The expected return is the risk-free rate plus a risk premium. Fischer Black (1972) examined and provided the CAPM without using the risk-free borrowing or lending assumption. He suggested the use of a zero beta portfolio. Mayers (1972) introduced the CAPM which is with non-marketable human capital. Miller and Scholes (1972) considered the annual data and used it to study the return-beta with its relationship to stocks on the New York Stock Exchange. The data considered was from the year 1954 to 1963. According to the result, the predictions made by the CAPM is inconsistent. Jensen et al. (1972) studied the New York Stock Exchange (NYSE) using the monthly returns for 34 years. Rather than using individual stocks, ten portfolios were created and their findings were that the predictions made by CAPM were true. Fama and MacBeth (1973) also confirmed the validity of the capital asset pricing model considering the NYSE by extending the years (1926–1968). The Consumption-based capital asset pricing model was introduced by Breeden (1979) as an extension to the work done by Sharpe. Many researchers had made an effort to apply the Capital asset pricing model to show or test its validity. His work shows that the CAPM is inadequate to explain the effect of asset risk in determining the future return which is also known as the expected return. Also, Zhang et al. (2004) applied the conditional and unconditional CAPM in analyzing the pricing of equality of emerging capital markets. Monthly series of the share prices of six countries in Europe was considered for the year 1995–2002.

Michailidis et al. (2006), in his work, considered the weekly stock return of Greece market and also the monthly stock returns of the Nigeria Stock Exchange. It was found that the higher risk related to the higher return and lower risk related to the lower return is not true. Ibrahim et al. (2018) used the CAPM to give the effect of expected returns of the Precious metals and crude oil on the risk. It was observed that the higher the risk, the higher the expected return, and the lower the risk, the lower the expected return. This supports the notion that investors will only invest in any asset knowing fully well that the return will be high.

Merton (1973) introduced the intertemporal capital asset pricing model. The Arbitrage asset pricing model was also introduced as an extension to the study of Sharpe. The research was made to solve the inadequacy of CAPM to capture all the risk involved. The Arbitrage model is a multi-factor model and also function like the capital asset pricing model. It explained that the expected return is dependent on different risk factors, rather than the capital asset pricing model that has its expected return depending on only market risk. Huberman (1982), Chamberlain and Rothschild (1983) and Chen and Ingersol (1983) studied and made a further study on the capital asset pricing model. According to Bollerslev (1986) and Engle (1982), the observation made was that the stock return distribution is time-variant in nature and investor expectations does not act as a constant but it behaves like random variables.

Fama and Macbeth (1973) showed and relates the expected return and the risk where the CAPM was used to under see the connection between the risk and the return of stocks for the New York Stock Exchange. Cochrane (1991) introduced the investment-based CAPM and the Liquidity based CAPM was formed by Acharya and Pedersen (2005). Fama and French (1992) considered the market risk, firm size and book-to-market as a determining factor for the changes in stock returns. Jagannathan and Wang (1996) came up with Conditional CAPM. Fama et al. (2004) explained that the capital asset pricing model is mostly used and applied in estimating the cost and also used to evaluate the outcome of portfolios owned by companies. Liang (2006) formulated the model B CAPM (best-beta CAPM). Choudhary et al. (2010) in his work tested the CAPM in the Indian Stock Market using monthly returns for the period of 1996 to 2009. It was discovered that the higher the beta of stocks and the higher the expected return is not always true. Iqbal (2011) reviewed some of the work done by researchers on the capital asset pricing model. He also said in his conclusion that the CAPM is expected to rule over the capital market as a tool to ascertain the expected returns of risky securities. In 2013, Dzaja et al. (2013) applied the CAPM on the Central and South-East European emerging markets by using the monthly stock returns for the year 2006–2010. Adedokun et al. (2012) applied the capital asset pricing model to the Nigeria Stock Exchange to test if the model will be valid for the Nigeria Stock Exchange. Elbannan (2014) expatiated more on what capital asset pricing model is, and discussed the evaluation, assumptions and the limitation of the CAPM. Celik (2012) also gave a comprehensive theoretical review of the capital asset pricing model. Sukano (2017) employed and used two approaches to estimate the Beta risk of the capital asset pricing model. He used the covariance approach and then used genetic algorithm optimization. The result shows that there is a significant difference in using the two methods to estimate the Beta. Alqisie (2015) studied and tested to know how valid the capital asset pricing model in the Amman Stock Exchange (ASE). The year considered is 2010–2014 and later it was sub-divided. Since CAPM theory explained that the systematic risk of asset changes over time, Mamadou et al. (2018) employed two dynamics and compared the two to choose the best of the two dynamics used. The two dynamics considered are Kalman filter who assumed that Beta follows a random walk and Markov switching model who takes the Beta changes with the regimes. Rossi (2016) also considered the capital asset pricing model, taking into consideration the history, key ideas and the shortcomings of the capital asset pricing model. He discussed the instability of betas over time and the difficulty of getting a risk-free asset. Choudhary et al. (2018) studied the Indian stock market using the capital asset pricing model by selecting the returns from five publics in the Bombay Stock Exchange between 2016–2017. Yang (2019) expatiated and developed an exchange online financial capital asset pricing model. All these are further researches on the capital asset pricing model.

Although the capital asset pricing model is widely used, different researchers explained its inability to explain the movements of asset returns. Some Researchers like Banz challenged the validity of CAPM. Banz (1981) also applied the CAPM on the NYSE from the year 1936 to 1975. He concluded that Beta is not significant but rather the size effect is more significant whether beta is present or not.

Here, the CAPM is analyzed using the real-life data of DB crude oil assets such that the relationship between risk and expected returns are examined.

3.   Methodology

Considering an open market with risky assets where an investor can borrow and lend assets at a risk-free rate. It is also assumed that all investors know some information such as the mean of returns, the covariances and the variances of the stock and other information concerning the stock. A point $({\sigma _m}, E(R))$ is always gotten whenever a rational investor select and put together his assets to form a portfolio $Y = \left({{a_1}, {a_2}, {a_3}, {a_4}..., {a_n}} \right)$. These points are on the capital market line.

where $\sigma$ is the portfolio risk, ${\sigma _m}$ is the market risk and $E(R)$ is the expected return.

According to Sharpe (1965), The capital asset pricing model based on systematic risk which shows the relationship between the risk and expected return is given as:

${\rm{where}}\; i = 1\left(1 \right)N$.

The above model depends on the beta risk (systematic risk), the risk-free rate of return, risk premium and the expected return. The meaning of expected risk premium according to Treynor (1962) is that the expected risk premium is the current value of the risk premium of the portfolio. The risk-free rate is assumed to be the treasury bill rate. The CAPM model is used to analyse the sensitivity of asset to the expected return $\left({E\left({{R_i}} \right)} \right)$ and risk-free rate (${R_f}$).

The expected return on the risky security is the risk-free plus the risk premium. The expected return is a long term assumption of how an investment will behave. The Beta is a kind of measure used for stock’s volatility compared with the market’s volatility as a whole. A risk-free asset has its variance to be zero and the expected return $E({R_f})$ of the risk-free asset is equal to itself i.e. $E({R_f}) = {R_f}$. When beta is -1 the security is said to have a perfect negative correlation with the market. A beta with 1 has its security’s expected return to be equal to the average market return. The asset with high risk or positive risk is more volatile and riskier than the asset with lower risk. Though having a negative number which is a low value of risk does mean that there is no risk involved.

The following parameters are defined:

$E\left({{R_i}} \right) =$ Expected return of the risky asset

${R_f} =$ risk-free rate on the asset i

${\beta _i} =$ Systematic risk which is also referred to as the market risk

$E\left({{R_m}} \right) =$ The expected return on the market.

N is the total number of years that is under consideration.

The behaviour of investors under the CAPM is based on the following assumptions:

● Investors are known to be risk-averse that is they tend to prefer asset with low return than bearing risk. Also, their major concern is to know the mean and variance of their investment return.

● Investors can borrow or lend based on the risk-free rate of interest. The risk-free rate is calculated to be the same for all investors without giving preference to the amount lent or borrowed.

● Markets have no cost for taxes or transaction.

● Assets are fixed and marketable

● All investors are price takers.

● The beta coefficient is the only measure of risk. According to Sharpe, the expected return is only dependent on systematic risk which cannot be diversified.

● All investors have an equal right to access available information like the covariances, the mean rates of stocks.

● Investors made decisions based on risk and return.

${\beta _i}$ is calculated as the ration of covariance of the return on the market return and the variance of the market return. ${\beta _i}$ is used to measure the indivisible risk.

The variance of the market portfolio is calculated by taking the differences between each return and the mean, then the difference is squared to make it positive.

where ${R_i}$ is the return of the asset i and ${R_m}$ is the market return.$\overline {{R_i}}, \overline {{R_m}}$ are the mean of market returns. In the CAPM model, ${\beta _i}\left({E\left({{R_m} - {R_f}} \right)} \right)$ is the risk premium.

The CAPM is designed to determine and show the relations that exist between the systematic risk in the four assets of Deutsche Bank and its expected returns.

4.   Results

4.1.   Returns of Deutsche bank

In this section, data from Deutsche bank crude oil which is obtained from Yahoo Finance Datastream is analysed. Here, we consider the DTO-DB Crude oil Double Short, OLO-DB Crude oil long, SZO-DB Crude oil Short and DBO-Invesco DB oil fund. According to Regnier (2009), oil is classified to be the world’s most strategic commodities that attract people’s attention. The data used is from 2014–2018 due to some inconsistency in the data. The analysis performed here is presented (1)–(8). Table 1 below shows the returns, market returns and risk-free returns on each asset.

Table 1.  Returns, market returns and risk-free returns on assets.

Year	DTO	OLO	SZO	DBO	${R_m}$	${R_f}$
2014	0.8012	0.5670	-0.1634	-0.4398	0.1913	1.4340
2015	0.9458	0.5094	0.0565	-0.4152	0.2741	2.1610
2016	-0.1514	-0.0614	-0.4128	0.0554	-0.0980	2.1680
2017	0.1931	0.1487	-0.4223	-0.1648	-0.1426	2.4538
2018	0.28546	0.2001	-0.1752	-0.1796	-0.0327	2.4433

---

 | Show Table

DownLoad: CSV

Table 2 shows the risk and the expected return. DTO has 3.6679 as its Risk and the expected return to be 0.1199. DTO has its risk and returns to be the highest. This implies that 73% should be invested in risky assets of DTO while 27% can be on the risk-free assets. SZO has its risk to be 2.06 and the expected return to be 0.0674. It can be deduced that 67% of the company’s wealth can be invested in the risky asset of SZO while 33% can be spent on risk-free assets. 16% of the total wealth can be spent on the risky asset should the organization choose to invest in OLO. DBO has the least return and it has its risk to be negative.

Table 2.  Risk and expected returns.

Assets	β	E(R)
DTO	3.6679	0.1199
OLO	2.0616	0.0674
SZO	0.8132	0.0259
DBO	-1.5427	-0.0504

---

 | Show Table

DownLoad: CSV

The negative result gotten in the risk of DBO was as a result of the negative covariance gotten throughout the whole year. This shows that there is no correlation between the asset returns and the market returns. Though the presence of negative does not mean the asset is not risky.

4.2.   Relationship between the asset expected returns and risks

Figure 1 shows the relationship between the expected returns of assets and risks.

Figure 1.  Graph of the expected returns against the risk of the assets.

DownLoad: Full-Size Img PowerPoint

The above graph which is linear shows the risk of each asset and the expected returns. The graph shows the beta values in the x-axis and expected returns in the y-axis. The line drawn on the graph is also known as the security market line (SML) which represents the capital asset pricing model. It shows the various systematic risk of the four assets considered. The risk with the value 3.6679 is seen to be the highest and it has the highest expected returns. From the graph, it is seen that the asset with negative risk also has a negative return. The negative sign implies that the DBO asset investment results in a loss.

Figures 2–5 show the Returns, Market Returns (Rm) and Covariances(COV) of the DTO, SZO, OLO and DBO Crude Oil respectively over the period 2014–2018. DBO-DB Crude Oil has the least returns and all its covariance to be negative all through the year. Suppose a portfolio was to be built, DBO will be a very good asset to include. Covariance can be used to relate two assets together in portfolio management. From Figures 3 and 4, the returns of DTO and SZO falls and rises at the same time. Since the covariances for each year are all positive, the returns of the assets move a similar way with the market returns. In the year 2015, market return rises and the covariance rises. Considering Figure 4, when the covariance is negative, the market return and the asset return moves in a similar way. The covariance was negative in 2016, the direction of the two returns (i.e. market return and the asset return) moves in the opposite direction (i.e., when one is moving to the positive side, the other moves to the negative side). Figure 5 shows that the market returns and the asset return move in the opposite direction since all the covariance is negative.

Figure 2.  Graph of Returns, market returns and covariance of DTO.

DownLoad: Full-Size Img PowerPoint

Figure 3.  Graph of returns, market returns and covariance of SZO.

DownLoad: Full-Size Img PowerPoint

Figure 4.  Graph of market returns and covariance of OLO.

DownLoad: Full-Size Img PowerPoint

Figure 5.  Graph of returns, market returns and covariance of DBO.

DownLoad: Full-Size Img PowerPoint

4.3.   Relationship between the asset returns, market returns and the covariances of assets

The figures in this section show each asset returns, market returns and the covariances of each asset. Since the covariance shows the movement between the asset returns and the market returns. In Figure 2, In the year 2015, it can be seen that the market return increases and was at its peak. The market return and asset return rises also and was at its highest peak that same year. From the year 2015, the return tends to fall and getting to the year 2016 it rises a little bit. The two returns i.e. asset returns and market returns move in the same direction. We can then conclude that asset DTO moves in the same direction as the market return. This can help an investor who is ready to base his decision on previous information to predict what will happen in the future. Since, if the market return increases for a particular year, the return is also increasing. SZO also follows the same way as it is in DTO. The market return moves in the same direction with the asset returns.

It can be seen in Figure 4 that when the covariance was negative in the year 2016, the market return and the asset return for OLO moves in the opposite direction. As the market return was rising in that year, the asset return is falling. In the Year 2014, 2015, 2017 and 2018, the asset returns of OLO moves in the same direction. Also, in Figure 5, the covariance for all the years is negative. Therefore, all the asset returns of DBO and the market return move in different directions.

4.4.   Relationship between market return and its variance

Since variance is used to calculate how a particular number in a set is far apart from the mean. The variance here shows the gap between the market return and its mean. In the year 2015, In Figure 6, the variance is at the highest point. The variance was at the lowest in the year 2014. The variances in the year 2016, 2017 and 2018 show the possibility of negative variance.

Figure 6.  Graph of market returns and variance.

DownLoad: Full-Size Img PowerPoint

4.5.   Relationship between returns and risk of each asset

Figures 7–10 relate the returns and risk of the assets. From Figures 7 and 8, the return was at its lowest value while the risk was at the highest value. Figures 9 and 10 show that the return was at the peak in 2016 while the risk has the most negative value as its risk.

Figure 7.  Graph of returns and risk of DTO.

DownLoad: Full-Size Img PowerPoint

Figure 8.  Graph of returns and risk of SZO.

DownLoad: Full-Size Img PowerPoint

Figure 9.  Graph of returns and risk of OLO.

DownLoad: Full-Size Img PowerPoint

Figure 10.  Graph of returns and risk of DBO.

DownLoad: Full-Size Img PowerPoint

4.6.   Relationship between the market return and covariances of the assets

Figure 11 shows the market return and covariances of the four assets and Figure 12 combines the risk of the four assets and the expected returns. It can be seen here that COV4 which is the covariance of DBO-Invesco DB Oil fund moves in the opposite direction to the covariance of other assets. The negative covariance results to it moving in the opposite direction to the market return.

Figure 11.  Graph of market returns and covariances of the four assets.

DownLoad: Full-Size Img PowerPoint

Figure 12.  Graph of market returns and risk of the four assets.

DownLoad: Full-Size Img PowerPoint

4.7.   Relationship between the market returns and the risk for the four assets

Figure 12 shows and reveals the risk of each asset for each year and the market returns. BETA 1, BETA 2, BETA 3 and BETA 4 represents the market risk for DTO, SZO, OLO and DBO respectively. In the year 2016, DTO and SZO have the highest risk and OLO and DBO have the highest negative value of risk.

5.   Conclusion

In this study, the capital asset pricing model had been used to analyse the market returns and the returns of the four common Deutsche Bank (DB) crude oil assets. In section 4.1, Table 2, the SZO has the least positive risk. Therefore, suppose an investor is risk-averse and want to choose among the four assets, he will choose SZO-DB crude oil short which has the least return and the lowest risk. But, if the investor is a risk taker he will choose to invest in DTO-DB crude oil which has the highest risk and a very high expected return. If the investor chooses to invest in DBO, he should expect a loss i.e. he will not regain the money he deposited in the investment. Also, the capital asset pricing model analysis on these assets shows the validity that the higher the risk, the higher the return.

Conflict of interest

The authors declare no conflicts of interest in this paper.