Study of a complex environmental mixture by electrospray ionization and laser desorption ionization high resolution mass spectrometry: the cigarette smoke aerosol

Vincent Carré; Sébastien Schramm; Frédéric Aubriet; Vincent Carré; Sébastien Schramm; Frédéric Aubriet

doi:10.3934/environsci.2015.3.547

AIMS Environmental Science

2015, Volume 2, Issue 3: 547-564. doi: 10.3934/environsci.2015.3.547

Previous Article Next Article

Research article Special Issues

Study of a complex environmental mixture by electrospray ionization and laser desorption ionization high resolution mass spectrometry: the cigarette smoke aerosol

1.
Laboratoire de Chimie et de Physique - Approche Multi-échelles des Milieux Complexes, Université de Lorraine. Institut Jean Barriol (FR CNRS 2843)/TGE FT-ICR (FR CNRS 3624), 1, Boulevard Arago, F-57070 Metz Technopole Cedex 03, France;
2.
CEA, DAM, DIF, F-91297 Arpajon, France

Received: 07 April 2015 Accepted: 10 June 2015 Published: 11 June 2015

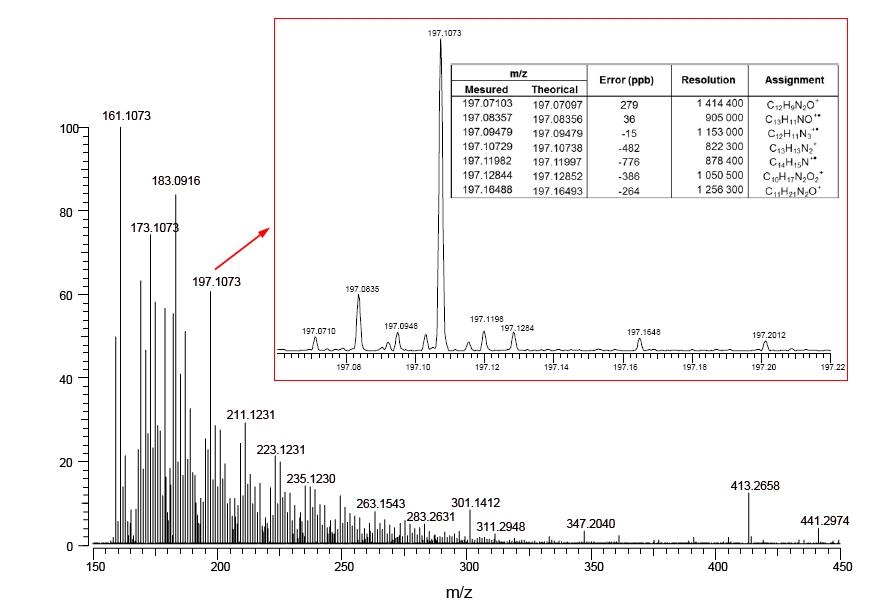
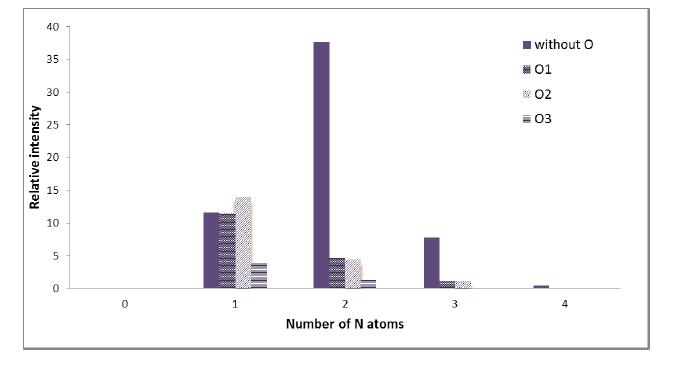
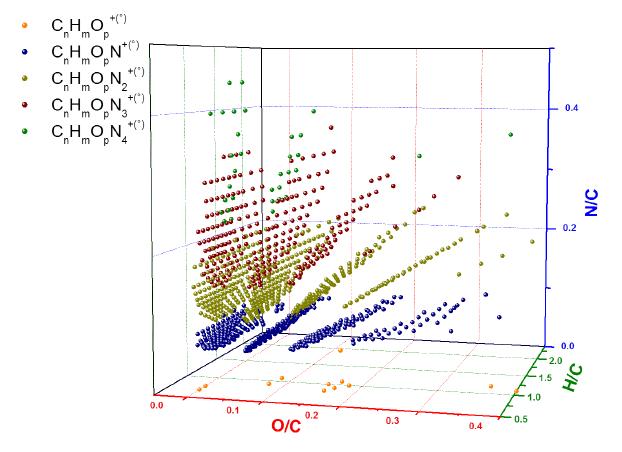
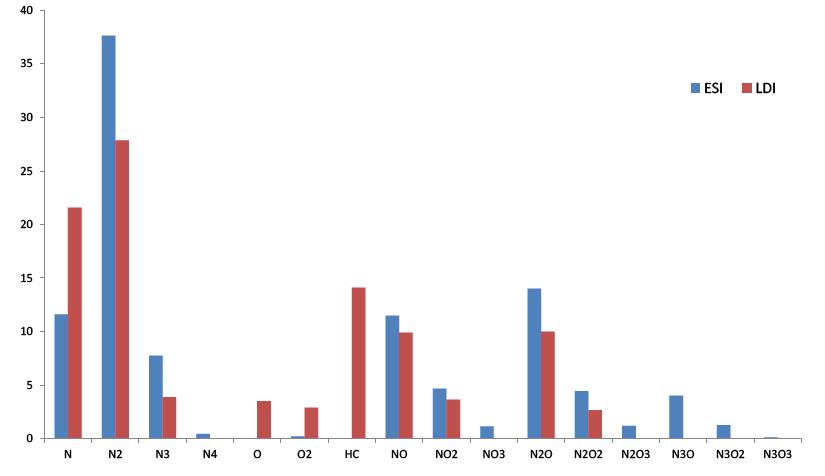
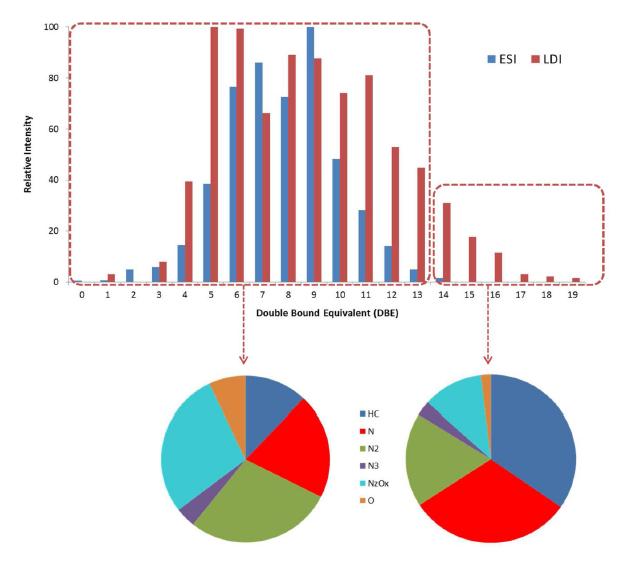
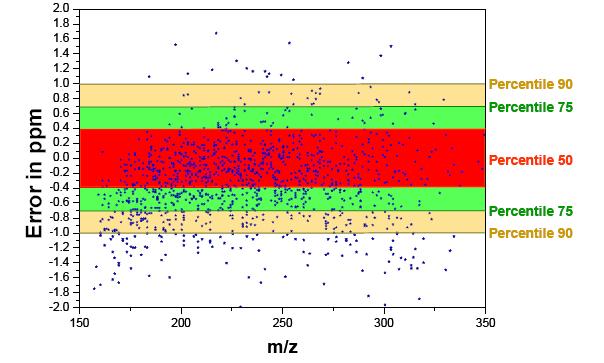
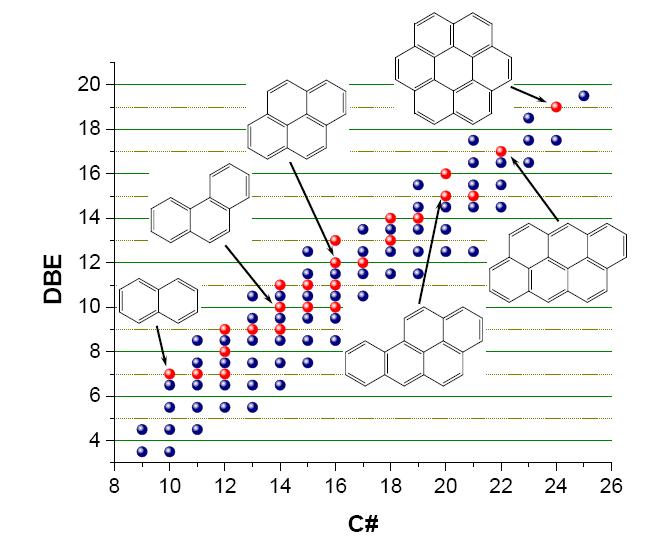
Aerosols from the mainstream cigarette smoke have been analyzed by electrospray ionization (ESI) coupled to Fourier transform ion cyclotron resonance mass spectrometry (FTICRMS). Measurements have been conducted in positive ion mode. The chemical composition of cigarette smoke aerosol is significant because it gives insights of one complex indoor environmental mixture. Almost 1300 chemical compositions relative to nitrogen species were successfully determined through the accurate mass measurement and the good ion production of the used technique. The most abundant class of compounds corresponds to N2 one (hydrocarbons with two nitrogen atoms). For other classes, the van Krevelen diagrams ensured to define that other nitrogen and oxygen-nitrogen compounds adopted similar behavior in terms of unsaturation and alkylation range. The detailed composition of cigarette smoke aerosol provided a typical chemical fingerprint from the biomass pyrolysis with tobacco-specific compounds. We examined also the contribution of laser desorption ionization (LDI) technique coupled to FTICRMS for the acute analysis of cigarette smoke aerosol. While a part of the chemical composition were found similar to ESI results, LDI achieved a broader range of poly-aromatic compounds and highlight new class compounds as pure hydrocarbon and oxygen-containing species. The combination of ESI and LDI with high resolution mass spectrometry clearly increased significantly the coverage of the “whole composition” of environmental aerosols such as cigarette smoke aerosol.

Keywords:

Citation: Vincent Carré, Sébastien Schramm, Frédéric Aubriet. Study of a complex environmental mixture by electrospray ionization and laser desorption ionization high resolution mass spectrometry: the cigarette smoke aerosol[J]. AIMS Environmental Science, 2015, 2(3): 547-564. doi: 10.3934/environsci.2015.3.547

Related Papers:

[1]	Xiaoxue Zhao, Zhuchun Li . Synchronization of a Kuramoto-like model for power grids with frustration. Networks and Heterogeneous Media, 2020, 15(3): 543-553. doi: 10.3934/nhm.2020030
[2]	Tingting Zhu . Synchronization of the generalized Kuramoto model with time delay and frustration. Networks and Heterogeneous Media, 2023, 18(4): 1772-1798. doi: 10.3934/nhm.2023077
[3]	Seung-Yeal Ha, Yongduck Kim, Zhuchun Li . Asymptotic synchronous behavior of Kuramoto type models with frustrations. Networks and Heterogeneous Media, 2014, 9(1): 33-64. doi: 10.3934/nhm.2014.9.33
[4]	Tingting Zhu . Emergence of synchronization in Kuramoto model with frustration under general network topology. Networks and Heterogeneous Media, 2022, 17(2): 255-291. doi: 10.3934/nhm.2022005
[5]	Seung-Yeal Ha, Jaeseung Lee, Zhuchun Li . Emergence of local synchronization in an ensemble of heterogeneous Kuramoto oscillators. Networks and Heterogeneous Media, 2017, 12(1): 1-24. doi: 10.3934/nhm.2017001
[6]	Seung-Yeal Ha, Jeongho Kim, Jinyeong Park, Xiongtao Zhang . Uniform stability and mean-field limit for the augmented Kuramoto model. Networks and Heterogeneous Media, 2018, 13(2): 297-322. doi: 10.3934/nhm.2018013
[7]	Seung-Yeal Ha, Hansol Park, Yinglong Zhang . Nonlinear stability of stationary solutions to the Kuramoto-Sakaguchi equation with frustration. Networks and Heterogeneous Media, 2020, 15(3): 427-461. doi: 10.3934/nhm.2020026
[8]	Seung-Yeal Ha, Se Eun Noh, Jinyeong Park . Practical synchronization of generalized Kuramoto systems with an intrinsic dynamics. Networks and Heterogeneous Media, 2015, 10(4): 787-807. doi: 10.3934/nhm.2015.10.787
[9]	Vladimir Jaćimović, Aladin Crnkić . The General Non-Abelian Kuramoto Model on the 3-sphere. Networks and Heterogeneous Media, 2020, 15(1): 111-124. doi: 10.3934/nhm.2020005
[10]	Young-Pil Choi, Seung-Yeal Ha, Seok-Bae Yun . Global existence and asymptotic behavior of measure valued solutions to the kinetic Kuramoto--Daido model with inertia. Networks and Heterogeneous Media, 2013, 8(4): 943-968. doi: 10.3934/nhm.2013.8.943

Abstract

1. Introduction

Synchronization in complex networks has been a focus of interest for researchers from different disciplines[1,2,4,8,15]. In this paper, we investigate synchronous phenomena in an ensemble of Kuramoto-like oscillators which is regarded as a model for power grid. In [9], a mathematical model for power grid is given by

$\begin{equation} P_{source}^{i} = I\ddot{\theta}_{i}\dot{\theta}_{i}+K_{D}(\dot{\theta}_{i})^2-\sum\limits_{l = 1}^{N}a_{il}\sin(\theta_{l}-\theta_{i}),\quad i = 1,2,\dots,N, \end{equation}$ $

(1)

where $ P_{source}^{i}>0 $ is power source (the energy feeding rate) of the $ i $th node, $ I\ge 0 $ is the moment of inertia, $ K_{D}>0 $ is the ratio between the dissipated energy by the turbine and the square of the angular velocity, and $ a_{il}>0 $ is the maximum transmitted power between the $ i $th and $ l $th nodes. The phase angle $ \theta_{i} $ of $ i $th node is given by $ \theta_i(t) = \Omega t+\tilde\theta_i(t) $ with a standard frequency $ \Omega $ (50 or 60 Hz) and small deviations $ \tilde\theta_i(t) $. Power plants in a big connected network should be synchronized to the same frequency. If loads are too strong and unevenly distributed or if some major fault or a lightening occurs, an oscillator (power plant) may lose synchronization. In that situation the synchronization landscape may change drastically and a blackout may occur. The transient stability of power grids can be regarded as a synchronization problem for nonstationary generator rotor angles aiming to restore synchronism subject to local excitations. Therefore, the region of attraction of synchronized states is a central problem for the transient stability.

By denoting $ \omega_{i} = \frac{P_{source}^{i}}{K_{D}} $ and assuming $ \frac{a_{il}}{K_{D}} = \frac{K}{N} \,(\forall i,l\in\{1,2,\dots,N\}) $ and $ I = 0 $ in (1), Choi et. al derived a Kuramoto-like model for the power grid as follows [5]:

$\begin{equation} (\dot{\theta}_{i})^2 = \omega_{i}+\frac{K}{N}\sum\limits_{l = 1}^{N}\sin(\theta_{l}-\theta_{i}),\quad \dot{\theta}_{i} > 0,\quad i = 1,2,\dots,N. \end{equation}$ $

(2)

Here, the setting $ \dot{\theta}_{i}>0 $ was made in accordance to the observation in system (1) that the grid is operating at a frequency close to the standard frequency with small deviations. In order to ensure that the right-hand side of (2) is positive, it is reasonable to assume that $ \omega_{i}>K,\; i = 1,2,\dots,N $. This model can be used to understand the emergence of synchronization in networks of oscillators. As far as the authors know, there are few analytical results. In [5], the authors considered this model with identical natural frequencies and prove that complete phase synchronization occurs if the initial phases are distributed inside an arc with geodesic length less than $ \pi/2 $.

If $ (\dot{\theta}_{i})^2 $ in (2) is replaced by $ \dot{\theta}_{i} $, the model is the famous Kuramoto model [13]; for its synchronization analysis we refer to [3,6,10], etc. Sakaguchi and Kuramoto [16] proposed a variant of the Kuramoto model to describe richer dynamical phenomena by introducing a phase shift (frustration) in the coupling function, i.e., by replacing $ \sin(\theta_{l}-\theta_{i}) $ with $ \sin(\theta_{l}-\theta_{i}+\alpha) $. They inferred the need of $ \alpha $ from the empirical fact that a pair of oscillators coupled strongly begin to oscillate with a common frequency deviating from the simple average of their natural frequencies. Some physicists also realized via many experiments that the emergence of the phase shift term requires larger coupling strength $ K $ and longer relaxation time to exhibit mutual synchronization compared to the zero phase shift case. This is why we call the phase shift a frustration. The effect of frustration has been intensively studied, for example, [11,12,14]. In power grid model using Kuramoto oscillators, people use the phase shift to depict the energy loss due to the transfer conductance [7]. In this paper, we will incorporate the phase shift term in (2) and study the Kuramoto-like model with frustration $ \alpha\in(-\frac{\pi}{4},\frac{\pi}{4}) $:

$\begin{equation} (\dot{\theta}_{i})^2 = \omega_{i}+\frac{K}{N}\sum\limits_{l = 1}^{N}\sin(\theta_{l}-\theta_{i}+\alpha),\quad \dot{\theta}_{i} > 0,\quad i = 1,2,\dots,N. \end{equation}$ $

(3)

We will find a trapping region such that any nonstationary state located in this region will evolve to a synchronous state.

The contributions of this paper are twofold: First, for identical oscillators without frustration, we show that the initial phase configurations located in the half circle will converge to complete phase and frequency synchronization. This extends the analytical results in [5] in which the initial phase configuration for synchronization needs to be confined in a quarter of circle. Second, we consider the nonidentical oscillators with frustration and present a framework leading to the boundness of the phase diameter and complete frequency synchronization. To the best of our knowledge, this is the first result for the synchronization of (3) with nonidentical oscillators and frustration.

The rest of this paper is organized as follows. In Section 2, we recall the definitions for synchronization and summarize our main results. In Section 3, we give synchronization analysis and prove the main results. Finally, Section 4 is devoted to a concluding summary.

Notations. We use the following simplified notations throughout this paper:

$\begin{align*} &\nu_{i}: = \dot{\theta}_{i},\; i = 1,2,\dots,N,\;{\omega: = (\omega_{1},\omega_{2},\dots,\omega_{N})},\\ &\bar{\omega}: = \max\limits_{1\le i\le N}\omega_{i}, \; \underline{\omega}: = \min\limits_{1\le i\le N}\omega_{i}, \; { D(\omega): = \bar{\omega}-\underline{\omega}},\\ & \theta_{M}: = \max\limits_{1\le i\le N}\theta_{i},\; \theta_{m}: = \min\limits_{1\le i\le N}\theta_{i},\; D(\theta): = \theta_{M}-\theta_{m},\\ & \nu_{M}: = \max\limits_{1\le i\le N}\nu_{i},\; \nu_{m}: = \min\limits_{1\le i\le N}\nu_{i},\; D(\nu): = \nu_{M}-\nu_{m},\\ & {\theta_{M}^\nu\in\{\theta_{j}| \nu_{j} = \nu_{M}\},\; \theta_{m}^\nu\in\{\theta_{j}| \nu_{j} = \nu_{m}\}}. \end{align*}$ $

2. Preliminaries

In this paper, we consider the system

$\begin{align} \begin{aligned} (\dot{\theta}_{i})^2& = \omega_{i}+\frac{K}{N}\sum\limits_{l = 1}^{N}\sin(\theta_{l}-\theta_{i}+\alpha),\quad \dot{\theta}_{i} > 0,\quad \alpha\in(-\frac{\pi}{4},\frac{\pi}{4}),\\ \theta_i(0)& = \theta_i^0,\quad \quad i = 1,2,\dots,N. \end{aligned} \end{align}$ $

(4)

Next we introduce the concepts of complete synchronization and conclude this introductory section with the main result of this paper.

Definition 2.1. Let $ \theta: = (\theta_{1},\theta_{2},\dots,\theta_{N}) $ be a dynamical solution to the system (4). We say

1. it exhibits asymptotically complete phase synchronization if

$\begin{equation*} \lim\limits_{t\to \infty}(\theta_{i}(t)-\theta_{j}(t)) = 0, \; \forall i\ne j. \end{equation*}$ $

2. it exhibits asymptotically complete frequency synchronization if

$\begin{equation*} \lim\limits_{t\to \infty}({\dot \theta}_{i}(t)-{\dot \theta}_{j}(t)) = 0, \; \forall i\ne j. \end{equation*}$ $

For identical oscillators without frustration, we have the following result.

Theorem 2.2. Let $ \theta: = (\theta_{1},\theta_{2},\dots,\theta_{N}) $ be a solution to the coupled system (4) with $ \alpha = 0,\omega_{i} = \omega_0,i = 1,2,\dots,N $ and $ \omega_0>K $. If the initial configuration

$\begin{equation*} \theta^0\in \mathcal{A}: = \{\theta\in[0,2\pi)^N:\; D(\theta) < \pi\}, \end{equation*}$ $

then there exits $ \lambda_1,\lambda_2>0 $ and $ t_{0}>0 $ such that

$\begin{equation} D(\theta(t))\le D(\theta^0)e^{-\lambda_1 t},\;\,\,\,t\ge 0. \end{equation}$ $

(5)

and

$\begin{equation} D(\nu(t))\le D(\nu(t_{0}))e^{-\lambda_2(t-t_{0})},\;\,\,\,t\ge t_{0}. \end{equation}$ $

(6)

Next we introduce the main result for nonidentical oscillators with frustration. For $ \bar{\omega}< \frac{1}{2\sin^2|\alpha|} $, we set

$ K_{c}: = \frac{D({\omega})\sqrt{2\bar{\omega}}}{1-\sqrt{2\bar{\omega}}\sin|\alpha|} > 0. $

For suitable parameters, we denote by $ D_{1}^{\infty} $ and $ D_{*}^{\infty} $ the two angles as follows:

$\begin{align*} &\sin D_{1}^{\infty} = \sin D_{*}^{\infty}: = \frac{\sqrt{\bar{\omega}+K}(D({\omega})+K\sin|\alpha|)}{K\sqrt{\underline{\omega}-K}},\quad 0 < D_{1}^{\infty} < \frac{\pi}{2} < D_{*}^{\infty} < \pi. \end{align*}$ $

Theorem 2.3. Let $ \theta: = (\theta_{1},\theta_{2},\dots,\theta_{N}) $ be a solution to the coupled system (4) with $ K>K_{c} $ and $ [\underline{\omega},\bar{\omega}]\subset \left[K+1,\frac{1}{2\sin^2|\alpha|}\right) $. If

$\begin{equation*} \theta^0\in \mathcal{B}: = \{\theta\in[0,2\pi)^N\;|\; D(\theta) < D_{*}^{\infty}-|\alpha|\}, \end{equation*}$ $

then for any small $ \varepsilon>0 $ with $ D_{1}^{\infty}+\varepsilon<\frac{\pi}{2} $, there exists $ \lambda_{3}>0 $ and $ T>0 $ such that

$\begin{equation} D(\nu(t))\le D(\nu(T))e^{-\lambda_{3}(t-T)},\;t\ge T. \end{equation}$ $

(7)

Remark 1. If the parametric conditions in Theorem 2.3 are fulfilled, the reference angles $ D_{1}^{\infty} $ and $ D_{*}^{\infty} $ are well-defined. Indeed, because $ K>K_{c} $ and $ \bar{\omega}<\frac{1}{2\sin^2 |\alpha|} $, we have

$\begin{equation*} \frac{D({\omega})\sqrt{2\bar{\omega}}}{1-\sqrt{2\bar{\omega}}\sin |\alpha|} < K,\qquad 1-\sqrt{2\bar{\omega}}\sin |\alpha| > 0. \end{equation*}$ $

This implies

$\begin{equation*} \frac{\sqrt{2\bar{\omega}}(D({\omega})+K\sin|\alpha|)}{K} < 1. \end{equation*}$ $

Then, by $ \underline{\omega}\ge K+1 $ and $ K\le \bar{\omega} $ we obtain

$\begin{align*} \sin D_{1}^{\infty} = \sin D_{*}^{\infty}: = \frac{\sqrt{\bar{\omega}+K}(D({\omega})+K\sin|\alpha|)}{K\sqrt{\underline{\omega}-K}}\le \frac{\sqrt{2\bar{\omega}}(D({\omega})+K\sin|\alpha|)}{K} < 1. \end{align*}$ $

Remark 2. In order to make $ 1<K+1<\frac{1}{2\sin^2|\alpha|} $, it is necessary to assume $ \alpha\in \left(-\frac{\pi}{4},\frac{\pi}{4}\right) $. This is the reason for the setting $ \alpha\in \left(-\frac{\pi}{4},\frac{\pi}{4}\right) $.

3. Synchronization analysis

3.1. Synchronization estimates: Identical oscillators without frustration

In this subsection we consider the system (4) with identical natural frequencies and zero frustration:

$\begin{equation} (\dot{\theta}_{i})^2 = \omega_0+\frac{K}{N}\sum\limits_{l = 1}^{N}\sin(\theta_{l}-\theta_{i}),\quad \dot{\theta}_{i} > 0,\quad i = 1,2,\dots,N. \end{equation}$ $

(8)

To obtain the complete synchronization, we need to derive a trapping region. We start with two elementary estimates for the transient frequencies.

Lemma 3.1. Suppose $ \theta: = (\theta_{1},\theta_{2},\dots,\theta_{N}) $ be a solution to the coupled system (8), then for any $ i,j\in\{1,2,\dots,N\}, $ we have

$\begin{equation*} (\dot{\theta}_{i}-\dot{\theta}_{j})(\dot{\theta}_{i}+\dot{\theta}_{j}) = \frac{2K}{N}\sum\limits_{l = 1}^{N}\cos(\theta_{l}-\frac{\theta_{i}+\theta_{j}}{2})\sin\frac{\theta_{j}-\theta_{i}}{2}. \end{equation*}$ $

Proof. It is immediately obtained by (8).

Lemma 3.2. Suppose $ \theta: = (\theta_{1},\theta_{2},\dots,\theta_{N}) $ be a solution to the coupled system (8) and $ \Omega>K $, then we have

$\begin{equation*} \dot{\theta}_{i}\le \sqrt{\omega_0+K}. \end{equation*}$ $

Proof. It follows from (8) and $ \dot{\theta}_{i}>0 $ that we have

$\begin{equation*} (\dot{\theta}_{i})^2 = \omega_0+\frac{K}{N}\sum\limits_{l = 1}^{N}\sin(\theta_{l}-\theta_{i})\le \omega_0 +K. \end{equation*}$ $

Next we give an estimate for trapping region and prove Theorem 2.2. For this aim, we will use the time derivative of $ D(\theta(t)) $ and $ D(\nu(t)) $. Note that $ D(\theta(t)) $ is Lipschitz continuous and differentiable except at times of collision between the extremal phases and their neighboring phases. Therefore, for the collision time $ t $ at which $ D(\theta(t)) $ is not differentiable, we can use the so-called Dini derivative to replace the classic derivative. In this manner, we can proceed the analysis with differential inequality for $ D(\theta(t)) $. For $ D(\nu(t)) $ we can proceed in the similar way. In the following context, we will always use the notation of classic derivative for $ D(\theta(t)) $ and $ D(\nu(t)) $.

Lemma 3.3. Let $ \theta: = (\theta_{1},\theta_{2},\dots,\theta_{N}) $ be a solution to the coupled system (8) and $ \Omega>K $. If the initial configuration $ \theta(0)\in \mathcal{A} $, then $ \theta(t)\in \mathcal{A},\; t\ge 0 $.

Proof. For any $ \theta^0\in \mathcal A $, there exists $ D^\infty\in (0,\pi) $ such that $ D(\theta^0)<D^{\infty} $. Let

$\begin{align*} \mathcal T: = \left\{T\in[0,+\infty)\,\Big|\,D(\theta(t)) < D^{\infty}, \,\, \forall \,t\in [0,T) \right\}. \end{align*}$ $

Since $ D(\theta^0)<D^{\infty} $, and $ D(\theta(t)) $ is a continuous function of $ t $, there exists $ \eta>0 $ such that

$\begin{equation*} D(\theta(t)) < D^{\infty},\;t\in[0,\eta). \end{equation*}$ $

Therefore, the set $ \mathcal T $ is not empty. Let $ T^*: = \sup \mathcal T. $ We claim that

$\begin{equation} T^* = \infty. \end{equation}$ $

(9)

Suppose to the contrary that $ T^*<\infty $. Then from the continuity of $ D(\theta(t)) $, we have

$\begin{align*} D(\theta(t)) < D^{\infty},\;t\in[0,T^*),\quad D(\theta(T^*)) = D^{\infty}. \end{align*}$ $

We use Lemma 3.1 and Lemma 3.2 to obtain

$\begin{align*} \frac{1}{2}\frac{d}{dt}&D(\theta(t))^2 = D(\theta(t))\frac{d}{dt}D(\theta(t)) = \left(\theta_{M}-\theta_{m}\right)\left(\dot{\theta}_{M}-\dot{\theta}_{m}\right) \\ & = \left(\theta_{M}-\theta_{m}\right)\frac{1}{\dot{\theta}_{M}+\dot{\theta}_{m}}\frac{2K}{N}\sum\limits_{l = 1}^{N}\cos\left(\theta_{l}-\frac{\theta_{M}+\theta_{m}}{2}\right)\sin\left(\frac{\theta_{m}-\theta_{M}}{2}\right)\\ &\le \left(\theta_{M}-\theta_{m}\right)\frac{1}{\dot{\theta}_{M}+\dot{\theta}_{m}}\frac{2K}{N}\sum\limits_{l = 1}^{N}\cos\frac{D^{\infty}}{2}\sin\left(\frac{\theta_{m}-\theta_{M}}{2}\right)\\ &\le \left(\theta_{M}-\theta_{m}\right)\frac{1}{\sqrt{\omega_0+K}}\frac{K}{N}\sum\limits_{l = 1}^{N} \cos\frac{D^{\infty}}{2}\sin\left(\frac{\theta_{m}-\theta_{M}}{2}\right)\\ & = -\frac{2K\cos\frac{D^{\infty}}{2}}{\sqrt{\omega_0+K}}\frac{D(\theta)}{2}\sin\frac{D(\theta)}{2}\\ &\le -\frac{K\cos\frac{D^{\infty}}{2}}{\pi\sqrt{\omega_0+K}}D(\theta)^2, \; t\in[0,T^*). \end{align*}$ $

Here we used the relations

$\begin{equation*} -\frac{D^{\infty}}{2} < -\frac{D(\theta)}{2}\le \frac{\theta_{l}-\theta_{M}}{2}\le 0 \le \frac{\theta_{l}-\theta_{m}}{2}\le \frac{D(\theta)}{2} < \frac{D^{\infty}}{2} \end{equation*}$ $

and

$\begin{equation*} x\sin x\ge \frac{2}{\pi}x^2,\; x\in\left[-\frac{\pi}{2},\frac{\pi}{2}\right]. \end{equation*}$ $

Therefore, we have

$\begin{equation} \frac{d}{dt}D(\theta)\le -\frac{K\cos\frac{D^{\infty}}{2}}{\pi\sqrt{\omega_0+K}}D(\theta), \; t\in[0,T^*), \end{equation}$ $

(10)

which implies that

$\begin{align*} D(\theta(T^*))\le D(\theta^0)e^{-\frac{K\cos\frac{D^{\infty}}{2}}{\pi\sqrt{\omega_0+K}}T^*} < D(\theta^0) < D^{\infty}. \end{align*}$ $

This is contradictory to $ D(\theta(T^*)) = D^{\infty}. $ The claim (9) is proved, which yields the desired result.

Now we can give a proof for Theorem 2.2.

Proof of Theorem 2.2.. According to Lemma 3.3, we substitute $ T^* = \infty $ into (10), then (5) is proved with $ \lambda_1 = \frac{K\cos\frac{D^{\infty}}{2}}{\pi\sqrt{\omega_0+K}} $.

On the other hand, by (5) there exist $ t_{0} $ and $ \delta (0<\delta<\frac{\pi}{2}) $ such that $ D(\theta(t))\le \delta $ for $ t\ge t_{0} $. Now we differentiate (8) to find

$\begin{equation*} \dot{\nu}_{i} = \frac{K}{2N\nu_{i}}\sum\limits_{l = 1}^{N}\cos(\theta_{l}-\theta_{i})(\nu_{l}-\nu_{i}). \end{equation*}$ $

Using Lemma 3.2, we now consider the temporal evolution of $ D(\nu(t)) $:

$\begin{align*} \frac{d}{dt}&D(\nu) = \dot{\nu}_{M}-\dot{\nu}_{m}\\ & = \frac{K}{2N\nu_{M}}\sum\limits_{l = 1}^{N}\cos(\theta_{l}-\theta_{M}^{\nu})(\nu_{l}-\nu_{M})-\frac{K}{2N\nu_{m}}\sum\limits_{l = 1}^{N}\cos(\theta_{l}-\theta_{m}^{\nu})(\nu_{l}-\nu_{m})\\ &\le\frac{K\cos\delta}{2N\nu_{M}}\sum\limits_{l = 1}^{N}(\nu_{l}-\nu_{M})-\frac{K\cos\delta}{2N\nu_{m}}\sum\limits_{l = 1}^{N}(\nu_{l}-\nu_{m})\\ &\le \frac{K}{2N}\frac{\cos\delta}{\sqrt{\omega_0+K}}\sum\limits_{l = 1}^{N}(\nu_{l}-\nu_{M})-\frac{K}{2N}\frac{\cos\delta}{\sqrt{\omega_0+K}}\sum\limits_{l = 1}^{N}(\nu_{l}-\nu_{m})\\ & = \frac{K\cos\delta}{2N\sqrt{\omega_0+K}}\sum\limits_{l = 1}^{N}(\nu_{l}-\nu_{M}-\nu_{l}+\nu_{m})\\ & = -\frac{K\cos\delta}{2\sqrt{\omega_0+K}}D(\nu),\;t\ge t_{0}. \end{align*}$ $

This implies that

$\begin{equation*} D(\nu(t))\le D(\nu(t_{0}))e^{-\frac{K\cos\delta}{2\sqrt{\omega_0+K}}(t-t_{0})},\;\,\,\,t\ge t_{0}, \end{equation*}$ $

and proves (6) with $ \lambda_2 = \frac{K\cos\delta}{2\sqrt{\omega_0+K}} $.

Remark 3. Theorem 2.2 shows, as long as the initial phases are confined inside an arc with geodesic length strictly less than $ \pi $, complete phase synchronization occurs exponentially fast. This extends the main result in [5] where the initial phases for synchronization need to be confined in an arc with geodesic length less than $ \pi/2 $.

3.2. Synchronization estimates: Nonidentical oscillators with frustration

In this subsection, we prove the main result for nonidentical oscillators with frustration.

Lemma 3.4. Let $ \theta: = (\theta_{1},\theta_{2},\dots,\theta_{N}) $ be a solution to the coupled system (4), then for any $ i,j\in\{1,2,\dots,N\}, $ we have

$\begin{equation*} (\dot{\theta}_{i}-\dot{\theta}_{j})(\dot{\theta}_{i}+\dot{\theta}_{j})\le D({\omega})+\frac{K}{N}\sum\limits_{l = 1}^{N}\left[\sin(\theta_{l}-\theta_{i}+\alpha)-\sin(\theta_{l}-\theta_{j}+\alpha)\right]. \end{equation*}$ $

Proof. By (4) and for any $ i,j\in\{1,2,\dots,N\} $

$\begin{equation*} (\dot{\theta}_{i}-\dot{\theta}_{j})(\dot{\theta}_{i}+\dot{\theta}_{j}) = (\dot\theta_{i})^2-(\dot\theta_{j})^2, \end{equation*}$ $

the result is immediately obtained.

Lemma 3.5. Let $ \theta: = (\theta_{1},\theta_{2},\dots,\theta_{N}) $ be a solution to the coupled system (4) and $ \underline{\omega}\ge K+1 $, then we have

$\begin{equation*} \dot{\theta}_{i}\in \left[\sqrt{\underline{\omega}-K}, \sqrt{\bar{\omega}+K}\right],\quad \forall i = 1,2,\dots,N. \end{equation*}$ $

Proof. From (4), we have

$\begin{equation*} \underline{\omega}-K\le (\dot{\theta}_{i})^2 \le \bar{\omega}+K,\quad \forall i = 1,2,\dots,N, \end{equation*}$ $

and also because $ \dot{\theta}_{i}>0 $. The following lemma gives a trapping region for nonidentical oscillators.

Lemma 3.6. Let $ \theta: = (\theta_{1},\theta_{2},\dots,\theta_{N}) $ be a solution to the coupled system (4) with $ K>K_{c} $ and $ [\underline{\omega},\bar{\omega}]\subset [K+1,\frac{1}{2\sin^2|\alpha|}) $. If the initial configuration $ \theta^0\in \mathcal{B} $, then $ \theta(t)\in \mathcal{B} $ for $ t\ge 0. $

Proof. We define the set $ \mathcal T $ and its supremum:

$\begin{align*} \mathcal T: = \left\{T\in[0,+\infty)\,\Big|\,D(\theta(t)) < D_{*}^{\infty}-|\alpha|, \,\, \forall \,t\in [0,T) \right\},\quad T^*: = \sup \mathcal T. \end{align*}$ $

Since $ \theta^0\in \mathcal{B} $, and note that $ D(\theta(t)) $ is a continuous function of $ t $, we see that the set $ \mathcal T $ is not empty and $ T^* $ is well-defined. We now claim that

$\begin{align*} T^* = \infty. \end{align*}$ $

Suppose to the contrary that $ T^*<\infty $. Then from the continuity of $ D(\theta(t)) $, we have

$\begin{align*} D(\theta(t)) < D_{*}^{\infty}-|\alpha|,\;t\in[0,T^*),\quad D(\theta(T^*)) = D_{*}^{\infty}-|\alpha|. \end{align*}$ $

We use Lemma 3.4 to obtain

$\begin{align*} \frac{1}{2}\frac{d}{dt}&D(\theta)^2 = D(\theta)\frac{d}{dt}D(\theta) = D(\theta)\left(\dot{\theta}_{M}-\dot{\theta}_{m}\right) \\ \le &D(\theta)\frac{1}{\dot{\theta}_{M}+\dot{\theta}_{m}} \underbrace{\left[D({\omega})+\frac{K}{N}\sum\limits_{l = 1}^{N}\left(\sin(\theta_{l}-\theta_{M}+\alpha)-\sin(\theta_{l}-\theta_{m}+\alpha)\right)\right]}_{I}. \end{align*}$ $

For $ I $, we have

$\begin{align*} I = D({\omega})&+\frac{K\cos\alpha}{N}\sum\limits_{l = 1}^{N}\left[\sin(\theta_{l}-\theta_{M})-\sin(\theta_{l}-\theta_{m})\right]\\ & +\frac{K\sin\alpha}{N}\sum\limits_{l = 1}^{N}\left[\cos(\theta_{l}-\theta_{M})-\cos(\theta_{l}-\theta_{m})\right]. \end{align*}$ $

We now consider two cases according to the sign of $ \alpha $.

(1) $ \alpha\in[0,\frac{\pi}{4}). $ In this case, we have

$\begin{align*} I&\le D({\omega})+\frac{K\cos\alpha\sin D(\theta)}{ND(\theta)}\sum\limits_{l = 1}^{N}\left[(\theta_{l}-\theta_{M})-(\theta_{l}-\theta_{m})\right]\\ &\quad +\frac{K\sin\alpha}{N}\sum\limits_{l = 1}^{N}\left[1-\cos D(\theta)\right]\\ & = D({\omega})-K\left[\sin(D(\theta)+\alpha)-\sin\alpha\right]\\ & = D({\omega})-K\left[\sin(D(\theta)+|\alpha|)-\sin|\alpha|\right]. \end{align*}$ $

(2) $ \alpha\in(-\frac{\pi}{4},0). $ In this case, we have

$\begin{align*} I&\le D({\omega})+\frac{K\cos\alpha\sin D(\theta)}{ND(\theta)}\sum\limits_{l = 1}^{N}\left[(\theta_{l}-\theta_{M})-(\theta_{l}-\theta_{m})\right]\\ &\quad +\frac{K\sin\alpha}{N}\sum\limits_{l = 1}^{N}\left[\cos D(\theta)-1\right]\\ & = D({\omega})-K\left[\sin(D(\theta)-\alpha)+\sin\alpha\right]\\ & = D({\omega})-K\left[\sin(D(\theta)+|\alpha|)-\sin|\alpha|\right]. \end{align*}$ $

Here we used the relations

$\begin{equation*} \frac{\sin(\theta_{l}-\theta_{M})}{\theta_{l}-\theta_{M}}, \,\,\,\frac{\sin(\theta_{l}-\theta_{m})}{\theta_{l}-\theta_{m}}\ge\frac{\sin D(\theta)}{D(\theta)}, \end{equation*}$ $

and

$\begin{equation*} \cos D(\theta)\leq \cos(\theta_{l}-\theta_{M}),\,\cos(\theta_{l}-\theta_{m})\le 1, \quad l = 1,2,\dots,N. \end{equation*}$ $

Since $ D(\theta(t))+|\alpha|<D_{*}^{\infty}<\pi $ for $ t\in[0,T^*), $ we obtain

$\begin{align} I&\le D({\omega})-K\left[\sin(D(\theta)+|\alpha|)-\sin|\alpha|\right] \end{align}$ $

(11)

$\begin{align} &\le D({\omega})+K\sin|\alpha|-K\frac{\sin D_{*}^{\infty}}{D_{*}^{\infty}}(D(\theta)+|\alpha|) . \end{align}$ $

(12)

By (12) and Lemma 3.5 we have

$\begin{align*} &\quad \frac{1}{2}\frac{d}{dt}D(\theta)^2\\&\le D(\theta)\frac{1}{\dot{\theta}_{M}+\dot{\theta}_{m}}\left(D({\omega})+K\sin|\alpha|-K\frac{\sin D_{*}^{\infty}}{D_{*}^{\infty}}(D(\theta)+|\alpha|)\right)\\ & = \frac{D({\omega})+K\sin|\alpha|}{\dot{\theta}_{M}+\dot{\theta}_{m}}D(\theta)-\frac{K\sin D_{*}^{\infty}}{D_{*}^{\infty}(\dot{\theta}_{M}+\dot{\theta}_{m})}D(\theta)(D(\theta)+|\alpha|)\\ &\le \frac{D({\omega})+K\sin|\alpha|}{2\sqrt{\underline{\omega}-K}}D(\theta)-\frac{K\sin D_{*}^{\infty}}{D_{*}^{\infty}2\sqrt{\bar{\omega}+K}}D(\theta)(D(\theta)+|\alpha|),\;\,\, t\in[0,T^*). \end{align*}$ $

Then we obtain

$\begin{align*} \frac{d}{dt}D(\theta)\le \frac{D({\omega})+K\sin|\alpha|}{2\sqrt{\underline{\omega}-K}}-\frac{K\sin D_{*}^{\infty}}{2D_{*}^{\infty}\sqrt{\bar{\omega}+K}}(D(\theta)+|\alpha|),\; t\in[0,T^*), \end{align*}$ $

i.e.,

$\begin{align*} \frac{d}{dt}(D(\theta)+|\alpha|)&\le \frac{D({\omega})+K\sin|\alpha|}{2\sqrt{\underline{\omega}-K}}-\frac{K\sin D_{*}^{\infty}}{2D_{*}^{\infty}\sqrt{\bar{\omega}+K}}(D(\theta)+|\alpha|)\\ & = \frac{K\sin D_{*}^{\infty}}{2\sqrt{\bar{\omega}+K}}-\frac{K\sin D_{*}^{\infty}}{2D_{*}^{\infty}\sqrt{\bar{\omega}+K}}(D(\theta)+|\alpha|),\; t\in[0,T^*). \end{align*}$ $

Here we used the definition of $ \sin D_{*}^{\infty} $. By Gronwall's inequality, we obtain

$\begin{equation*} D(\theta(t))+|\alpha|\le D_{*}^{\infty}+(D(\theta^0)+|\alpha|-D_{*}^{\infty})e^{-\frac{K\sin D_{*}^{\infty}}{2D_{*}^{\infty}\sqrt{\bar{\omega}+K}}t},\; t\in[0,T^*), \end{equation*}$ $

Thus

$\begin{equation*} D(\theta(t))\le (D(\theta^0)+|\alpha|-D_{*}^{\infty})e^{-\frac{K\sin D_{*}^{\infty}}{2D_{*}^{\infty}\sqrt{\bar{\omega}+K}}t}+D_{*}^{\infty}-|\alpha|,\; t\in[0,T^*). \end{equation*}$ $

Let $ t\to T^{*-} $ and we have

$\begin{align*} D(\theta(T^*))\le (D(\theta^0)+|\alpha|-D_{*}^{\infty})e^{-\frac{K\sin D_{*}^{\infty}}{2D_{*}^{\infty}\sqrt{\bar{\omega}+K}}T^*}+D_{*}^{\infty}-|\alpha| < D_{*}^{\infty}-|\alpha|, \end{align*}$ $

which is contradictory to $ D(\theta(T^*)) = D_{*}^{\infty}-|\alpha|. $ Therefore, we have

$\begin{align*} T^* = \infty. \end{align*}$ $

That is,

$\begin{equation*} D(\theta(t))\le D_{*}^{\infty}-|\alpha|,\; \,\,\, \forall\, t\ge 0. \end{equation*}$ $

Lemma 3.7. Let $ \theta: = (\theta_{1},\theta_{2},\dots,\theta_{N}) $ be a solution to the coupled system (4) with $ K>K_{c} $ and $ [\underline{\omega},\bar{\omega}]\subset [K+1,\frac{1}{2\sin^2|\alpha|}) $. If the initial configuration $ \theta(0)\in \mathcal{B} $, then

$\begin{equation*} \frac{d}{dt}D(\theta(t))\le \frac{D({\omega})+K\sin|\alpha|}{2\sqrt{\underline{\omega}-K}}-\frac{K}{2\sqrt{\bar{\omega}+K}}\sin (D(\theta)+|\alpha|),\,\,\,\;t\ge 0. \end{equation*}$ $

Proof. It follows from (11) and Lemma 3.5, Lemma 3.6 and that we have

$\begin{align*} \frac{1}{2}\frac{d}{dt}D(\theta)^2& = D(\theta)\frac{d}{dt}D(\theta)\\ &\le D(\theta)\frac{1}{\dot{\theta}_{M}+\dot{\theta}_{m}}\left[D({\omega})-K\left(\sin(D(\theta)+|\alpha|)-\sin|\alpha|\right)\right]\\ & = \frac{D({\omega})+K\sin|\alpha|}{\dot{\theta}_{M}+\dot{\theta}_{m}}D(\theta)-\frac{K\sin(D(\theta)+|\alpha|)}{{\dot{\theta}_{M}+\dot{\theta}_{m}}}D(\theta)\\ &\le\frac{D({\omega})+K\sin|\alpha|}{2\sqrt{\underline{\omega}-K}}D(\theta)-\frac{K\sin (D(\theta)+|\alpha|)}{2\sqrt{\bar{\omega}+K}}D(\theta),\;\,\,\,t\ge 0. \end{align*}$ $

The proof is completed.

Lemma 3.8. Let $ \theta: = (\theta_{1},\theta_{2},\dots,\theta_{N}) $ be a solution to the coupled system (4) with $ K>K_{c} $ and $ [\underline{\omega},\bar{\omega}]\subset [K+1,\frac{1}{2\sin^2|\alpha|}) $. If the initial configuration $ \theta(0)\in \mathcal{B} $, then for any small $ \varepsilon>0 $ with $ D_{1}^{\infty}+\varepsilon<\frac{\pi}{2} $, there exists some time $ T>0 $ such that

$\begin{equation*} D(\theta(t)) < D_{1}^{\infty}-|\alpha|+\varepsilon,\;\quad t\ge T. \end{equation*}$ $

Proof. Consider the ordinary differential equation:

$\begin{equation} \dot{y} = \frac{D({\omega})+K\sin|\alpha|}{2\sqrt{\underline{\omega}-K}}-\frac{K}{2\sqrt{\bar{\omega}+K}}\sin y,\qquad y(0) = y^0\in [0,D_{*}^{\infty}). \end{equation}$ $

(13)

It is easy to find that $ y_{*} = D_{1}^{\infty} $ is a locally stable equilibrium of (13), while $ y_{**} = D_{*}^{\infty} $ is unstable. Therefore, for any initial data $ y^0 $ with $ 0<y^0<D_{*}^{\infty} $, the trajectory $ y(t) $ monotonically approaches $ y_{*} $. Then, for any $ \varepsilon>0 $ with $ D_{1}^{\infty}+\varepsilon<\frac{\pi}{2} $, there exists some time $ T>0 $ such that

$\begin{equation*} |y(t)-y_{*}| < \varepsilon,\;\quad t\ge T. \end{equation*}$ $

In particular, $ y(t) <y_*+\varepsilon $ for $ t\ge T. $ By Lemma 3.7 and the comparison principle, we have

$\begin{equation*} D(\theta(t))+|\alpha| < D_{1}^{\infty}+\varepsilon,\;\quad t\ge T, \end{equation*}$ $

which is the desired result.

Remark 4. Since

$\begin{align*} \sin D_{1}^{\infty}\ge \frac{D({\omega})}{K}+\sin|\alpha| > \sin|\alpha|, \end{align*}$ $

we have $ D_{1}^{\infty}>|\alpha| $.

Proof of Theorem 2.3. It follows from Lemma 3.8 that for any small $ \varepsilon>0 $, there exists some time $ T>0 $ such that

$\begin{equation*} \sup\limits_{t\ge T} D(\theta(t)) < D_{1}^{\infty}-|\alpha|+\varepsilon < \frac{\pi}{2}. \end{equation*}$ $

We differentiate the equation (4) to find

$\begin{equation*} \dot{\nu}_{i} = \frac{K}{2N\nu_{i}}\sum\limits_{l = 1}^{N}\cos(\theta_{l}-\theta_{i}+\alpha)(\nu_{l}-\nu_{i}),\quad \nu_{i} > 0. \end{equation*}$ $

We now consider the temporal evolution of $ D(\nu(t)) $:

$\begin{align*} \frac{d}{dt}&D(\nu) = \dot{\nu}_{M}-\dot{\nu}_{m}\\ = &\frac{K}{2N\nu_{M}}\sum\limits_{l = 1}^{N}\cos(\theta_{l}-\theta_{M}^\nu+\alpha)(\nu_{l}-\nu_{M})-\frac{K}{2N\nu_{m}}\sum\limits_{l = 1}^{N}\cos(\theta_{l}-\theta_{m}^\nu+\alpha)(\nu_{l}-\nu_{m})\\ \le&\frac{K}{2N\nu_{M}}\sum\limits_{l = 1}^{N}\cos(D_{1}^{\infty}+\varepsilon)(\nu_{l}-\nu_{M})-\frac{K}{2N\nu_{m}}\sum\limits_{l = 1}^{N}\cos(D_{1}^{\infty}+\varepsilon)(\nu_{l}-\nu_{m})\\ \le& \frac{K\cos(D_{1}^{\infty}+\varepsilon)}{2N\sqrt{\bar{\omega}+K}}\sum\limits_{l = 1}^{N}(\nu_{l}-\nu_{M}-\nu_{l}+\nu_{m})\\ = &-\frac{K\cos(D_{1}^{\infty}+\varepsilon)}{2\sqrt{\bar{\omega}+K}}D(\nu),\;\quad t\ge T, \end{align*}$ $

where we used

$\begin{align*} \cos(\theta_{l}-\theta_{M}^\nu+\alpha),\, \cos(\theta_{l}-\theta_{m}^\nu+\alpha)\ge \cos(D_{1}^{\infty}+\varepsilon),\quad \text{and}\quad \nu_{M},\, \nu_{m}\le \sqrt{\bar{\omega}+K}. \end{align*}$ $

Thus we obtain

$\begin{equation*} D(\nu(t))\le D(\nu(T))e^{-\frac{K\cos(D_{1}^{\infty}+\varepsilon)}{2\sqrt{\bar{\omega}+K}}(t-T)},\;t\ge T, \end{equation*}$ $

and proves (7) with $ \lambda_{3} = \frac{K\cos(D_{1}^{\infty}+\varepsilon)}{2\sqrt{\bar{\omega}+K}} $.

4. Conclusions

In this paper, we presented synchronization estimates for the Kuramoto-like model. We show that for identical oscillators with zero frustration, complete phase synchronization occurs exponentially fast if the initial phases are confined inside an arc with geodesic length strictly less than $ \pi $. For nonidentical oscillators with frustration, we present a framework to guarantee the emergence of frequency synchronization.

Acknowledgments

We would like to thank the anonymous referee for his/her comments which helped us to improve this paper.

References

[1]	Stedman RL (1968) Chemical composition of tobacco and tobacco smoke. Chem Rev 68: 153-207 doi: 10.1021/cr60252a002
[2]	Smith CJ, Perfetti TA, Garg R, et al. (2003) IARC carcinogens reported in cigarette mainstream smoke and their calculated log P values. Food Chem Toxicol 41: 807-817
[3]	Dallüge J, van Stee LLP, Xu X, Williams J, et al. (2002) Unravelling the composition of very complex samples by comprehensive gas chromatography coupled to time-of-flight mass spectrometry: Cigarette smoke. J Chromatogr A 974: 169-184 doi: 10.1016/S0021-9673(02)01384-5
[4]	Takanami Y, Chida M, Hasebe H, et al. (2003) Analysis of Cigarette Smoke by an Online Thermal Desorption System and Multidimensional GC-MS. J Chromatogr Sci 41: 317-322 doi: 10.1093/chromsci/41.6.317
[5]	Borgerding M, Klus H (2005) Analysis of complex mixtures -- Cigarette smoke. Exp Toxicol Pathol 57 Suppl 1: 43-73
[6]	Charles SM, Batterman SA, Jia C (2007) Composition and emissions of VOCs in main- and side-stream smoke of research cigarettes. Atmos Environ 41: 5371-5384 doi: 10.1016/j.atmosenv.2007.02.020
[7]	Adam T, Mitschke S, Baker RR (2009) Investigation of tobacco pyrolysis gases and puff-by-puff resolved cigarette smoke by single photon ionisation (SPI)-time-of-flight mass spectrometry (TOFMS). Beitr Zur Tab Tob Res 23: 203-226
[8]	Wang J, Weng J-J, Jia L-Y, et al. (2012) Study on Gas Phase Components in Mainstream Cigarette Smoke by Synchrotron Radiation Photoionization Mass Spectrometry. Chin J Anal Chem 40: 1048-1052 doi: 10.1016/S1872-2040(11)60559-8
[9]	Counts ME, Hsu FS, Laffoon SW, et al. (2004) Mainstream smoke constituent yields and predicting relationships from a worldwide market sample of cigarette brands: ISO smoking conditions. Regul Toxicol Pharmacol 39: 111-134 doi: 10.1016/j.yrtph.2003.12.005
[10]	Counts ME, Morton MJ, Laffoon SW, et al. (2005) Smoke composition and predicting relationships for international commercial cigarettes smoked with three machine-smoking conditions. Regul Toxicol Pharmacol 41: 185-227 doi: 10.1016/j.yrtph.2004.12.002
[11]	Castro D, Slezakova K, Delerue-Matos C, et al. (2011) Polycyclic aromatic hydrocarbons in gas and particulate phases of indoor environments influenced by tobacco smoke: Levels, phase distributions, and health risks. Atmos Environ 45: 1799-1808 doi: 10.1016/j.atmosenv.2011.01.018
[12]	Li M, Dong J-G, Huang Z-X, et al. (2012) Analysis of Cigarette Smoke Aerosol by Single Particle Aerosol Mass Spectrometer. Chin J Anal Chem 40: 936-939 doi: 10.1016/S1872-2040(11)60555-0
[13]	Wright C (2015) Standardized methods for the regulation of cigarette-smoke constituents. Trends Anal Chem 66: 118-127 doi: 10.1016/j.trac.2014.11.011
[14]	Ding YS, Zhang L, Jain RB, et al. (2008) Levels of Tobacco-Specific Nitrosamines and Polycyclic Aromatic Hydrocarbons in Mainstream Smoke from Different Tobacco Varieties. Cancer Epidemiol Biomarkers Prev 17: 3366-3371 doi: 10.1158/1055-9965.EPI-08-0320
[15]	Ding YS, Trommel JS, Yan XJ, et al. (2005) Determination of 14 Polycyclic Aromatic Hydrocarbons in Mainstream Smoke from Domestic Cigarettes. Environ Sci Technol 39: 471-478 doi: 10.1021/es048690k
[16]	Lu X, Cai J, Kong H, et al. (2003) Analysis of Cigarette Smoke Condensates by Comprehensive Two-Dimensional Gas Chromatography/Time-of-Flight Mass Spectrometry I Acidic Fraction. Anal Chem 75: 4441-4451 doi: 10.1021/ac0264224
[17]	Lu X, Zhao M, Kong H, et al. (2004) Characterization of cigarette smoke condensates by comprehensive two-dimensional gas chromatography/time-of-flight mass spectrometry (GC×GC/TOFMS) Part 2: Basic fraction. J Sep Sci 27: 101-109 doi: 10.1002/jssc.200301659
[18]	Brokl M, Bishop L, Wright CG, et al. (2013) Analysis of mainstream tobacco smoke particulate phase using comprehensive two-dimensional gas chromatography time-of-flight mass spectrometry. J Sep Sci 36: 1037-1044 doi: 10.1002/jssc.201200812
[19]	Hughey CA, Rodgers RP, Marshall AG (2002) Resolution of 11000 Compositionally Distinct Components in a Single Electrospray Ionization Fourier Transform Ion Cyclotron Resonance Mass Spectrum of Crude Oil. Anal Chem 74: 4145-4149 doi: 10.1021/ac020146b
[20]	Rodgers RP, Schaub TM, Marshall AG (2005) Petroleomics: MS Returns to Its Roots. Anal Chem 77: 20-A
[21]	Hertkorn N, Ruecker C, Meringer M, et al. (2007) High-precision frequency measurements: indispensable tools at the core of the molecular-level analysis of complex systems. Anal Bioanal Chem 389: 1311-1327 doi: 10.1007/s00216-007-1577-4
[22]	Kujawinski EB (2002) Electrospray Ionization Fourier Transform Ion Cyclotron Resonance Mass Spectrometry (ESI FT-ICR MS): Characterization of Complex Environmental Mixtures. Environ Forensics 3: 207-216 doi: 10.1080/713848382
[23]	Schmitt-Kopplin P, Gelencsér A, Dabek-Zlotorzynska E, et al. (2010) Analysis of the Unresolved Organic Fraction in Atmospheric Aerosols with Ultrahigh-Resolution Mass Spectrometry and Nuclear Magnetic Resonance Spectroscopy: Organosulfates As Photochemical Smog Constituents. Anal Chem 82: 8017-8026 doi: 10.1021/ac101444r
[24]	Gonsior M, Zwartjes M, Cooper WJ, et al. (2011) Molecular characterization of effluent organic matter identified by ultrahigh resolution mass spectrometry. Water Res 45: 2943-2953 doi: 10.1016/j.watres.2011.03.016
[25]	Cottrell BA, Gonsior M, Isabelle LM, et al. (2013) A regional study of the seasonal variation in the molecular composition of rainwater. Atmos Environ 77: 588-597 doi: 10.1016/j.atmosenv.2013.05.027
[26]	Cortés-Francisco N, Harir M, Lucio M, et al. (2014) High-field FT-ICR mass spectrometry and NMR spectroscopy to characterize DOM removal through a nanofiltration pilot plant. Water Res 67: 154-165 doi: 10.1016/j.watres.2014.08.046
[27]	Gonsior M, Schmitt-Kopplin P, Stavklint H, et al. (2014) Changes in Dissolved Organic Matter during the Treatment Processes of a Drinking Water Plant in Sweden and Formation of Previously Unknown Disinfection Byproducts. Environ Sci Technol 48: 12714-12722 doi: 10.1021/es504349p
[28]	Schramm S, Carré V, Scheffler J-L, et al. (2011) Analysis of Mainstream and Sidestream Cigarette Smoke Particulate Matter by Laser Desorption Mass Spectrometry. Anal Chem 83: 133-142 doi: 10.1021/ac1019842
[29]	Schramm S, Carré V, Scheffler J-L, et al. (2014) Active and passive smoking - New insights on the molecular composition of different cigarette smoke aerosols by LDI-FTICRMS. Atmos Environ 92: 411-420 doi: 10.1016/j.atmosenv.2014.04.052
[30]	Schäfer M, Drayß M, Springer A, et al. (2007) Radical Cations in Electrospray Mass Spectrometry: Formation of Open-Shell Species, Examination of the Fragmentation Behaviour in ESI-MSn and Reaction Mechanism Studies by Detection of Transient Radical Cations. Eur J Org Chem 2007: 5162-5174 doi: 10.1002/ejoc.200700199
[31]	Cole DP, Smith EA, Dalluge D, et al. (2013) Molecular characterization of nitrogen-containing species in switchgrass bio-oils at various harvest times. Fuel 111: 718-726 doi: 10.1016/j.fuel.2013.04.064
[32]	Sudasinghe N, Dungan B, Lammers P, et al. (2014) High resolution FT-ICR mass spectral analysis of bio-oil and residual water soluble organics produced by hydrothermal liquefaction of the marine microalga Nannochloropsis salina. Fuel 119: 47-56 doi: 10.1016/j.fuel.2013.11.019
[33]	Baker RR (1987) A review of pyrolysis studies to unravel reaction steps in burning tobacco. J Anal Appl Pyrolysis 11: 555-573 doi: 10.1016/0165-2370(87)85054-4
[34]	Wu Z, Rodgers RP, Marshall AG (2004) Two- and Three-Dimensional van Krevelen Diagrams: A Graphical Analysis Complementary to the Kendrick Mass Plot for Sorting Elemental Compositions of Complex Organic Mixtures Based on Ultrahigh-Resolution Broadband Fourier Transform Ion Cyclotron Resonance Mass Measurements. Anal Chem 76: 2511-2516 doi: 10.1021/ac0355449
[35]	Cho Y, Ahmed A, Islam A, et al. (2015) Developments in FT-ICR MS instrumentation, ionization techniques, and data interpretation methods for petroleomics. Mass Spectrom Rev 34: 248-263 doi: 10.1002/mas.21438
[36]	Baker RR, Bishop LJ (2004) The pyrolysis of tobacco ingredients. J Anal Appl Pyrolysis 71: 223-311 doi: 10.1016/S0165-2370(03)00090-1
[37]	Mullen CA, Boateng AA (2008) Chemical Composition of Bio-oils Produced by Fast Pyrolysis of Two Energy Crops^†. Energy Fuels 22: 2104-2109 doi: 10.1021/ef700776w
[38]	Olcese R, Carré V, Aubriet F, et al. (2013) Selectivity of Bio-oils Catalytic Hydrotreatment Assessed by Petroleomic and GCGC/MS-FID Analysis. Energy Fuels* 27: 2135-2145 doi: 10.1021/ef302145g
[39]	Li S, Olegario RM, Banyasz JL, et al. (2003) Gas chromatography-mass spectrometry analysis of polycyclic aromatic hydrocarbons in single puff of cigarette smoke. J Anal Appl Pyrolysis 66: 155-163 doi: 10.1016/S0165-2370(02)00111-0
[40]	Carré V, Aubriet F, Muller J-F (2005) Analysis of cigarette smoke by laser desorption mass spectrometry. Anal Chim Acta 540: 257-268 doi: 10.1016/j.aca.2005.03.034
[41]	Aubriet F, Carré V (2010) Potential of laser mass spectrometry for the analysis of environmental dust particles—A review. Anal Chim Acta 659: 34-54 doi: 10.1016/j.aca.2009.11.047
[42]	Cho Y, Witt M, Kim YH, et al. (2012) Characterization of Crude Oils at the Molecular Level by Use of Laser Desorption Ionization Fourier-Transform Ion Cyclotron Resonance Mass Spectrometry. Anal Chem 84: 8587-8594 doi: 10.1021/ac301615m
[43]	Cho Y, Jin JM, Witt M, et al. (2013) Comparing Laser Desorption Ionization and Atmospheric Pressure Photoionization Coupled to Fourier Transform Ion Cyclotron Resonance Mass Spectrometry To Characterize Shale Oils at the Molecular Level. Energy Fuels 27: 1830-1837 doi: 10.1021/ef3015662
[44]	Le Brech Y, Delmotte L, Raya J, et al. (2015) High Resolution Solid State 2D NMR Analysis of Biomass and Biochar. Anal Chem 87: 843-847 doi: 10.1021/ac504237c

This article has been cited by:

1.	Sha Xu, Xiaoyue Huang, Hua Zhang, 2024, Synchronization of a Kuramoto-like Model with Time Delay and Phase Shift, 978-9-8875-8158-1, 5299, 10.23919/CCC63176.2024.10662837
2.	Sun-Ho Choi, Hyowon Seo, Inertial power balance system with nonlinear time-derivatives and periodic natural frequencies, 2024, 129, 10075704, 107695, 10.1016/j.cnsns.2023.107695

Reader Comments

Your name:*

Email:*
© 2015 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)