Emergence of local synchronization in an ensemble of heterogeneous Kuramoto oscillators

1.   Introduction

Synchronization of weakly coupled oscillators is a collective behavior often found in complex biological systems such as groups of fireflies, neurons, and cardiac pacemaker cells [1,3,6,36,39,40]. It was first reported in scientific literature by physicist Christiaan Huygens around the middle of the seventh century; however, its rigorous mathematical treatment was conducted by Winfree [39] and Kuramoto [24] only several decades ago. Since then, several extensions of the Kuramoto model have been extensively investigated in several scientific fields such as applied mathematics, control theory, neuroscience, physics and engineering [1,4,10,12,13,14,26,29,30,32]. In this paper, we are primarily interested in the {generalized Kuramoto model with intrinsic dynamics [20,21]. Here, the intrinsic dynamics refer to smooth functions which describe the individual dynamics when the couplings vanish. Note that the classic Kuramoto oscillator has simple intrinsic dynamics governed by its natural frequency so that the uncoupled Kuramoto oscillator's phase has Kronecker dynamics on the unit circle $\mathbb S^1$. A natural question that arises for the dynamic behavior of generalized Kuramoto oscillators is the following: if the intrinsic dynamics are heterogeneous and rather complicated, can some form of synchrony be expected among the oscillators? We can easily find many examples such as the daily cycling of light and darkness that affects human sleep rhythms [37]. External fields can also model the external current applied to a neuron, which describes the collective properties of excitable systems with planar symmetry. For other physical devices such as Josephson junctions, a periodic external force can model an oscillating current across junctions.

The main purpose of this work is to study the emergence of local exponential synchronization of Kuramoto oscillators in networks with heterogeneous intrinsic dynamics. To better understand our goal, consider a mixture of two homogeneous ensembles denoted by ${\mathcal G}_\zeta$ and ${\mathcal G}_\eta$ with $|{\mathcal G}_\zeta| = N_1$ and $|{\mathcal G}_\eta| = N_2$. In the absence of mutual couplings, these two homogeneous groups are governed by the following two types of decoupled dynamics:

The functions $F_1$ and $F_2$ are assumed to be ${\mathcal C}^1$ so that the decoupled dynamics in (1) are well-posed by standard Cauchy-Lipschitz theory. We now consider the situation when the coupling strengths between pairs of oscillators are constant. Let $K_s\geq 0$ and $K_d\geq 0$ denote the intra and inter coupling strengths, respectively. In reality, one can reasonably argue that $K_s \gg K_d$. Then the dynamics of $(\zeta, \eta)$ are governed by the following coupled Kuramoto system:

Note that (1) gives the intrinsic dynamic for each oscillator in (2). The system (2) can be viewed as an interaction of two generalized Kuramoto models with external forcing terms [2,31,33,34,35]; moreover, it can be used to model the sleep-wake cycle. The sleep-wake cycle and circadian rhythms are phase-locked to each other in a 24-hour time period. Many biological experiments have shown that in isolation from a 24-hour periodic environment such as the light-dark cycle, various circadian rhythms, e.g., feeding, body temperature, and neuroendocrine variables, as well as the pattern of sleep and wakefulness, are maintained. However, a certain internal desynchronization phenomenon occurs, i.e., separate rhythmic variables oscillate with different periods. Many mathematical models have been developed to explain these phenomena, notably the generalized Kuramoto model with an external periodic force $F(\zeta, t) = A\sin(\sigma t -\zeta)$. In particular, Sakaguchi [34] showed numerically that forced entrainment is not always achieved, and analytic studies of this feature have also been reported [2,7,31]. Our main interest is not restricted to periodic forcing terms; instead, we consider general forcings without assuming the periodicity of $F_i$ in the first argument. In the presence of heterogeneous forcing terms in (2), the differences $\zeta_i - \eta_j$ between oscillators in different homogeneous groups do not converge asymptotically to a constant value. Thus, complete synchronization of the coupled system in (2) cannot be expected as in [9,10,13,14,19,22]. This is why the new concept of practical synchronization has been introduced in relation to the Kuramoto model [5,20,21,27,28,23] (see Definition 2.1). However, the synchronization can be observed in a local subgroup of oscillators with the same intrinsic dynamics, which is the main topic of this paper.

One of the most notable results of this study is the emergence of exponential local synchronization in each homogeneous group under suitable frameworks. Here let us introduce what the local synchronization means in this paper.

Definition 1.1. Let $\zeta = (\zeta_1, \dots, \zeta_{N_1})$ and $\eta = (\eta_1, \dots, \eta_{N_2})$ be a solution to system (2).The solution $(\zeta, \eta)$ exhibits asymptotic local synchronization (LS) if and only if the following conditions hold:

In Theorems 3.3, 4.3, 5.3, and 5.4, we show that the state-diameter of each homogeneous group converges to zero exponentially fast, although the total ensemble diameter does not converge to zero as $t \to \infty$. In particular, the proof of local synchronization in Theorems 3.3, 4.3, and 5.3 relies on the cohesiveness of phases, while the proof in Theorem 5.4 does not require cohesiveness. Here, the jargon "cohesiveness" means that there exists a length $\gamma\in[0, \pi)$ such that at each time $t$ there exists an arc of length $\gamma$ containing all phases (see [13]). As far as the authors know, most literature on the synchronization of Kuramoto oscillators (see [12]) focus on the "global synchronization" (in other words, complete synchronization). In contrast, our results exhibit exponential "local synchronization" even for all-to-all interactions. We refer to Remark 2.1 for the comparison between our results and the earlier results available in [20,21].

The rest of this paper is organized as follows. In Section 2, we discuss the generalized Kuramoto model with intrinsic dynamics and review earlier results on its practical synchronization. To motivate our analysis, in Sections 3 and 4 we consider the interaction of two homogeneous groups of oscillators with $N_1 = N_2 = 2$ and observe the emergence of exponential local synchronization of each group using numerical simulations. In particular, in Section 3 we consider generalized Kuramoto oscillators on a bipartite network, i.e., $K_s = 0$ and $K_d > 0$. In this setting, oscillators in the same group do not interact directly with each other, but interact through oscillators in the other group. We present a sufficient framework in terms of intrinsic dynamics, inter coupling strength, and admissible initial data in Theorem 3.3. In Section 4, we consider local synchronization of the coupled system in (2) with all-to-all coupling, i.e., $K_s > 0$ and $K_d > 0$. In Theorem 4.3, we present a framework leading to exponential local synchronization. In Section 5, we generalize the results in Section 4 to an ensemble of many (more than 2) groups of homogeneous oscillators. In Section 6, we present several numerical simulations and compare them with the analytical results in the previous sections. Finally, Section 7 is devoted to summarizing our main results and discussing future directions of research.

Notation: Throughout this paper, we will use the following notation. For state vectors $\zeta =(\zeta_1, \dots, \zeta_{N_1})$ and $\eta = (\eta_1, \dots, \eta_{N_2})$, we set

2.   preliminaries

In this section, we first consider the interactions of two homogeneous systems with $N_1 = N_2 = 2$ and then briefly review the Kuramoto model with intrinsic dynamics. Moreover, we discuss the difficulties encountered when analyzing our model mathematically and review previous results.

2.1.   Motivation

Consider the four-oscillator system with $N_1 = 2$ and $N_2 = 2$:

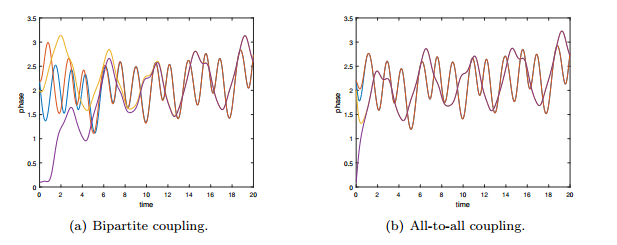
To illustrate the dynamics of (3), we perform two numerical simulations. Figure 1 shows the temporal evolution of the phases of the four oscillators with the following network structures:

Different colors are used to represent the different oscillators. Note that the emergence of local synchronization is observed in each case; however, system (3) does not show asymptotic complete synchronization, even in in Fig. 1(b) if the intra coupling strength $K_s$ drastically increases. Instead, the difference between the two groups is uniformly bounded, which motivates the new concept of practical synchronization. The numerical simulations in Fig. 1 illustrate that asymptotic behavior is independent of different network structures.

2.2.   Previous works and discussions

Let $\zeta_i$ be the phase of the i-th oscillator. Then a generalized system of coupled oscillators with intrinsic dynamics are given by the following equations:

For the zero coupling strength $K = 0$, i.e., when nonlinear coupling is turned off, system (4) becomes decoupled:

When the intrinsic dynamics $F_i$ in (4) are different, in general, the relative phases $\zeta_{ij} = \zeta_i - \zeta_j$ do not have a limit as $t \to \infty$. Thus, system (4) does not exhibit complete synchronization as in [7,13,14]. Therefore, a relaxed concept of synchronization was introduced, namely, practical synchronization.

Definition 2.1.[21] Let $\zeta = (\zeta_1, \dots, \zeta_{N})$ be a solution to system (4).

1. The solution $\zeta = \zeta(t)$ exhibits asymptotic complete synchronization (ACS) if and only if the following condition holds:

2. The solution $\zeta = \zeta(t)$ exhibits asymptotic practical synchronization (APS) if and only if the following condition holds:

The synchronization property of (4) was studied in [21] under the relaxed concept of practical synchronization. When the forcing function $F_i$ is constant, i.e., $F_i(\zeta_i, t)\equiv\Omega_i$, it reduces to the Kuramoto model [24]:

One nice feature of the Kuramoto model in (5) is that it can be rewritten as a gradient flow system (see [38]), i.e., for $\zeta =(\zeta_1, \dots, \zeta_N)$,

where the potential $V$ is given by

Since the potential function $V$ is analytic, the Lojasiewicz inequality holds, which immediately indicates that the boundedness of the phase fluctuations implies the emergence of phase-locking [16,17,18,25].

A natural question that arises is whether this gradient flow approach can be extended to a general model of oscillators with intrinsic dynamics. For (4), a possible candidate for the potential function is

Note that for the non-autonomous system in (4), a natural way of converting the non-autonomous system in (4) into an autonomous system on $\mathbb R^{N+1}$ is by introducing the extra variable $\zeta_{N+1} := t$, i.e., we set

To reformulate the gradient flow, it is required that

This means

which is not true for a family of non-constant analytic functions $\{F_i\}$ because the left hand side depends on $\zeta_i$, while the right hand side is independent of $\zeta_i$. On the other hand, the last coordinate, time $t$, is naturally unbounded; thus, the extended state vector ${\tilde \zeta}$ is not bounded. Therefore, the synchronizability of the system cannot be studied by extending the approach of gradient flow.

However, a rudimentary approach based on the Lyapunov functional approach can be taken for the state-diameter. In [21] the practical synchronization of (4) was studied by the method of energy estimate. The main result for system (4) is summarized as follows: under suitable conditions on the heterogeneous dynamics $\{F_i \}$ and initial data, for sufficient large coupling strength $K$, we have

For more details we refer to ([21,Theorem 4.1]). In [20], the authors considered the Kuramoto model with inertia and heterogeneous intrinsic dynamics:

The following practical synchronization for (6) has been obtained under suitable conditions on the heterogeneous dynamics $\{F_i \}$ and initial data whose size is dependent on the strength of inertia:

Note that the practical synchronization result in [21] focuses on the "global (or complete) synchronization" for the whole ensemble, i.e., asymptotic dynamics of phase diameter for the whole ensemble. However, as can be seen in Figure 1(b), the phase diameter for the whole ensemble does not converge to a constant value as time goes on, in fact, it is only bounded, but fluctuates in time. In contrast, our results in this paper focus on the dynamics of phase diameter for the local (or partial) ensemble consisting of oscillators with the same intrinsic dynamics, thus our paper deal with "local synchronization" for the ensemble. This is the difference between the results in [20,21] and our main results described in Theorem 3.1, Theorem 4.1 and Theorem 5.1. Moreover, our results shows that under suitable conditions on the intrinsic dynamics, coupling strength and initial configuration, the local synchronization occurs exponentially fast. In contrast, for the second-order flocking models in [8], it is known that local flockings occur algebraically slowly even for all-to-all interactions.

Throughout the paper, we frequently use the time derivative of group phase diameter $\frac{dD(t)}{dt}$. Since the diameter $D(t)$ is differentiable almost everywhere, the estimate is presented without arguing the differentiability at the points. Before we close this section, we introduce the following elementary inequality as a lemma.

Lemma 2.2. Let $0 < x < a < \pi$ or $-\pi < a < x < 0$. Then we have

In the following two sections, we consider the following cases for arbitrary finite $N_1$ and $N_2$:

The first case is called bipartite interactions and the latter case is called all-to-all interactions, between two homogeneous groups.

3.   Synchronization analysis Ⅰ: Bipartite network

In this section, we study bipartite interactions so that oscillators do not interact in the same groups directly; instead, they interact indirectly through oscillators in other groups:

In this setting, system (2) can be simplified as follows:

Lemma 3.1.(Cohesiveness) Suppose that the size of the local groups and initial data, intrinsic dynamics, and inter coupling strength satisfy the following conditions:

Then for any solution $(\zeta_i, \eta_i)$ to system (7) with initial configuration $(\zeta_{i0}, \eta_{i0})$,

1. The functional $D^*(t) := D^*(\zeta(t), \eta(t))$ is uniformly bounded by $D_\infty$:

2. The functional $D^*(t)$ satisfies an Adler differential inequality:

Proof.(1) First, we apply the continuity argument. Set

Since $D^*(0) < D_\infty$, by the continuity of $D^*(\cdot)$, it follows that $\mathcal T\neq \emptyset$, and $T_*>0$ is well-defined. In order to prove the first assertion, it suffices to show that $T_*=\infty$. Assume to the contrary that $T_* < \infty$. Then at the time instant $t=T_*,$

We now derive an estimate for the time-derivative of $D^*(t)$ at $t=T_*$ in two cases that depend on the membership of extremal phases in ${\mathcal G}_\zeta \cup {\mathcal G}_\eta$.

$\bullet$ Case 1: The maximal and minimal phases are attained in the same groups, say $\zeta_{M}$ and $\zeta_{m}$, respectively. In this case,

Here, we use the elementary inequality in Lemma 2.2. Similarly, if the extremal phases are attained in $\eta$-group ${\mathcal G}_\eta$, i.e., $\eta_M$ and $\eta_m$, then

$\bullet$ Case 2: The extremal phases are attained in different groups, say $\zeta_M$ and $\eta_m$, respectively. In this case,

where the condition (ⅲ) and the elementary inequality in Lemma 2.2. If the extremal phases are $\eta_M$ and $\zeta_m$, the same argument can be applied. The analysis in Case 1 and Case 2, together with assumptions (ⅰ)-(ⅲ) show that $D^*(t)$ strictly decreases beginning at $t=T_*$. This contradicts the definition of $T_*$; therefore, $T_*=\infty$, and hence, assertion (1) holds.

(2) Since the uniform bound $D^*(t)\leq D_\infty$ has been established, the differential inequality can be derived using the same arguments used in part (1).

Lemma 3.2. Under the same assumptions as Lemma 3.1, there exists $t_0>0$ such that

Here, ${\bar D}_\infty$ is the dual angle of $D_\infty$ defined by the following relation:

In other words, ${\bar D}_\infty=\min\{D_\infty, \pi-D_\infty\}.$

proof. The proof is similar to the proof in Appendix A.1 in [9], thus, it is omitted here.

We are now ready to prove local exponential synchronization in each group.

Theorem 3.3. Suppose that the size of the local groups and initial data, intrinsic dynamics, and inter coupling strength satisfy the following conditions:

Then for any solution $(\zeta_i, \eta_i)$ to system (7), there exists a positive constant $\Lambda_1$ such that

Proof. It follows from Lemma 3.2 that there exists a finite time $t_0$ such that

Now, consider the intra group phase diameter $D(\zeta)$. It follows from the system equation that

On the other hand, note that

Thus, we have

where the relation $D(\zeta(t)) \leq D^*(t)\leq {\bar D}_\infty$ and Lemma 2.2 are applied. Similarly, it follows that

Note that assumption (ⅲ) implies

By combining (8) and (9), the desired estimate is obtained.

4.   Synchronization analysis Ⅱ: All-to-all network

In this section, we consider the case of all-to-all couplings with $K_d > 0$ and $K_s > 0$:

For the case

the coupled system (10) reduces to the generalized Kuramoto model [21]. In the following, we present a framework for exponential local synchronization. We begin with a lemma on the cohesiveness of the total ensemble.

Lemma 4.1. (Cohesiveness) Suppose that $N_1 \geq 2, N_2 \geq 2$. If the sizes of the subgroups, intrinsic dynamics, and coupling strengths satisfy the following conditions:

then for any solution $(\zeta_i, \eta_i)$ to (7), the following assertions hold:

1. The functional $D^*(t)$ is uniformly bounded by $D_\infty$:

2. The functional $D^*(t)$ satisfies the Adler differential inequality:

Proof.(1) Let

Since $D^*(0) < D_\infty$, by the continuity of $D(\cdot),$ it follows that $\mathcal T\neq \emptyset$, and $T_*>0$ is well-defined. In order to prove the first assertion, it suffices to show that $T_*=\infty$. Assume to the contrary that $T_* < \infty$. Then at the time slice $t=T_*$,

We now derive an estimate for the time derivative of $D^*(t)$ at $t=T_*$ in two cases.

$\bullet$ Case 1: The maximal and minimal phases are attained in the same groups, say $\zeta_{M}$ and $\zeta_{m}$, respectively. In this case,

Here, we use the relation $K_s+K_d>2\min\{K_s, K_d\}$ and (ⅲ) to obtain the last inequality. If the extremal phases are attained in another group, i.e., $\eta_M$ and $\eta_m$, then

$\bullet$ Case 2: The extremal phases are attained in different groups, say $\zeta_M$ and $\eta_m$, respectively. In this case,

The analysis in Case 1 and Case 2 contradicts the definition of $T_*$. Therefore, we conclude the desired result.

(2) Since the uniform bound $D^*(t)\leq D_\infty$ has been established, the differential inequality can be derived using the same arguments as part (1).

Lemma 4.2. Similar to Lemma 3.2, there exists $t_0>0$ such that

where $\bar D_\infty < \pi/2$ is the dual angle of $D_\infty$, i.e., $\bar D_\infty=\min\{D_\infty, \, \pi-D_\infty\} < \pi/2$.

Next, we consider the exponential local synchronization. We will derive this by following the arguments in Theorem 3.3.

Theorem 4.3. Suppose that the sizes of the subgroups, intrinsic dynamics, and coupling strengths satisfy the following conditions:

then there exists a positive constant $\Lambda$ such that

Proof. Consider the intra group phase diameter $D(\zeta)$. We have

Note that

This yields

Here, we used the relation $D(\zeta(t)) \leq D^*(t)\leq {\bar D}_\infty$ and the elementary inequality in Lemma 2.2. For $D(\eta)$, we can easily verify that

Therefore, the desired results follow from (11) and (12).

Remark 1. 1. For the special case when

complete synchronization of (10) is addressed in [15].

2. For the Kuramoto system with two distinct constant natural frequencies, i.e., $F_1\equiv\Omega_1$ and $F_2\equiv\Omega_2$, conditions (ⅲ) and (ⅳ) are equivalent to $2\min\{K_s, K_d\}>\frac {D(\Omega)}{\sin D_\infty}$.

5.   Synchronization analysis Ⅲ: Many-cluster case

In this section, we extend the results in Sections 3 and 4 to the case of synchronous dynamics of Kuramoto oscillators with $n$ heterogeneous intrinsic dynamics. Suppose that $n$ homogeneous Kuramoto subsystems are coupled with each other, and let $\zeta^{(s)}_i$ denote the phase of the $i$-th oscillator in the $s$-th subsystem. Then the dynamics of $\zeta^{(s)}_i$ satisfy the following coupled system:

Here, the system consists of $n$ subgroups, and the $l$-th subgroup contains $N_l$ homogeneous oscillators with the same intrinsic dynamics $F_l(\zeta, t)$. The intra coupling strength in each subgroup is identical to $K$, whereas the inter coupling between the oscillators in different subgroups, say $\mathcal G_{s_1}$ and $\mathcal G_{s_2}$, is denoted by $K_{s_1s_2}=K_{s_2s_1}$. Let $D^{(s)}$, $D^{(s_1s_2)}$, and $D^*$ denote the local diameter of subgroup $\mathcal G_s$, the diameter of the union-group $\mathcal G_{s_1} \cup \mathcal G_{s_2}$, and the total diameter of a solution to system (13), respectively:

5.1.   Local synchronization in subensembles

In this subsection, we present a scheme for local synchronization in subgroups.

Lemma 5.1. (Cohesiveness) Suppose that the sizes of the subensembles, intrinsic dynamics, and coupling strengths satisfy the following conditions:

Then for any solution $\zeta = (\zeta^{(1)}_1, \dots, \zeta^{(1)}_{N_1}, \zeta^{(2)}_1, \dots, \zeta^{(2)}_{N_2}, \dots, \zeta^{(n)}_1, \dots, \zeta^{(n)}_{N_n})$ to (13) the following assertions hold:

1. The functional $D^*(\zeta(t))$ is uniformly bounded by $D_\infty$:

2. The functional $D^*(\zeta(t))$ satisfies the Adler differential inequality: for $\mbox{a.e}\ t \in (0, \infty)$,

Proof. (1) Set

We now estimate the time {derivative} of $D^*(\zeta(t))$ at $t=T_*$ in two cases.

$\bullet$ Case A: The maximal and minimal phases are attained in the same groups, say $\zeta^{(1)}_{M}$ and $\zeta^{(1)}_{m}$, respectively. In this case,

$\bullet$ Case B: The extremal phases are attained in different groups, say $\zeta^{(1)}_M$ and $\zeta^{(2)}_m$, respectively. In this case,

The analysis in Case 1 and Case 2 contradicts the definition of $T_*$. Therefore, we are able to conclude the desired result.

(2) Since the uniform bound $D^*(\zeta(t))\leq D_\infty$ has been established, the differential inequality can be derived using the same arguments used in part (1).

Lemma 5.2. By the same argument as Lemma 3.2, there exists $t_0>0$ such that

where $\bar D_\infty < \pi/2$ is the dual angle defined as in Lemma 3.2.

Now, we consider the complete phase synchronization in subgroups.

Theorem 5.3. (Local synchronization) Suppose that the sizes of the subgroups, intrinsic dynamics, and coupling strengths satisfy the following conditions:

Then for each $s\in \mathcal S$, there exists a positive constant $\Lambda_s$ such that

Proof. Consider the local phase diameter $D^{(s)}$. It follows that

Note that

This yields

Here, we used the relation $D^{(s)}(\zeta) \leq D^*(\zeta(t))\leq {\bar D}_\infty$, and Lemma 2.2. Since inequality (14) holds for each $s\in \mathcal S$, the desired results are obtained.

5.2.   Local synchronization without cohesive phases

In this subsection, we study local synchronization without cohesiveness of phases. In this case, we do not confine the initial total diameter $D^*(\zeta_0)$ to $[0, \pi)$. Instead, we only confine the initial local diameter $D^{(s)}(\zeta_0)$ of each group to $[0, \pi).$

Theorem 5.4. Suppose that for $s \in \{1, \dots, n \}$, the sizes of the subgroups, intrinsic dynamics, and coupling strengths satisfy the following conditions:

Then there exists a positive constant $\Lambda_s$ such that

Proof. First, we claim that the local phase diameter $D^{(s)}(\zeta(t))$ is uniformly bounded by $D^{(s)}_\infty$, i.e.,

Proof of claim (15): Set

Since $D^{(s)}(\zeta_0) < D^{(s)}_\infty$, by the continuity of $D^{(s)}(\zeta(\cdot)),$ it follows that $\mathcal T^{(s)}\neq \emptyset$, and $T^{(s)}_*>0$ is well-defined. In order to prove claim (15), it suffices to show that $T^{(s)}_*=\infty$. Assume to the contrary that $T^{(s)}_* < \infty$. Then at the time slice $t=T^{(s)}_*$,

However,

This contradicts the definition of $T_*^{(s)}$; hence, claim (15) is true. This further yields the following differential inequality:

Here, we use the relation $D^{(s)}(t) \leq D^{(s)}_\infty < \pi$ and Lemma 2.2. Then our assumption on $K$ leads to the exponential decay $D^{(s)}(\zeta(t))\to 0$ as $t\to \infty$.

6.   Numerical simulations

In this section, we provide several numerical simulations for the frameworks discussed in the previous sections. For all numerical simulations, we use the fourth-order Runge-Kutta method.

6.1.   Mixture of two homogeneous ensembles

In this subsection, we consider the mixture of two homogeneous Kuramoto ensembles with different intrinsic dynamics under two network structures: a bipartite network and an all-to-all network.

6.1.1.   Bipartite network

In this subsection, we present numerical simulations for a bipartite network. The conditions for the simulations in Fig. 2 are as follows:

and the initial phases are randomly chosen from the interval $[0, \frac{\pi}{2}]$.

In Fig. 2(a), snapshots of the particle distribution at $t=0$ and $t=10$ are shown. The different colors register the particles in the different groups, and we represent the particles at $t=0$ and $t=10$ with $\bullet$ and $\ast$, respectively. It is easy to see that the whole ensemble is divided into two subensembles at $t=10$.

In Fig. 2(b), the local ensemble diameters $D(\zeta)$ and $D(\eta)$ go to zero at least exponentially fast and reach approximately zero near $t = 5$; this is consistent with Theorem 3.3. However, the total diameter $D^*(\zeta, \eta)$, represented by a dashed line, fluctuates in time. This shows the failure of asymptotic complete synchronization for the whole ensemble.

In Fig. 2(c), the decay rate of the local diameters is shown in log scale. Notice that the Lyapunov exponent is close to $-1$, although there is some small fluctuation from the linear function.

In Fig. 2(d), we present a three-dimensional plot of the local diameter $D(\zeta)$ according to each pair $(t, K_d)$. Notice that $D(\zeta)$ tends to zero as the time $t$ increases only if $K_d$ is greater than a certain value, which also can be verified by condition (ⅲ) in Theorem 3.3. Thus, local exponential synchronization is not possible for small $K_d$.

6.1.2.   All-to-all network

In this subsection, we present simulations for an all-to-all network under the same initial configuration as in Subsection 6.1.1. We set $K_s=2$.

In Fig. 3(a), we present snapshots of the particle distribution at $t=0$ and $t=10$ denoted by $\bullet$ and $\ast$, respectively. Both configurations are the same as those of the bipartite network.

In Figs. 3(b) and (c), the local diameters $D(\zeta)$ and $D(\eta)$ go to zero exponentially, which is consistent with the analytical results in Theorem 4.3, whereas the whole ensemble diameter oscillates. Compared to Fig. 2 for a bipartite network, the Lyapunov exponent is $-3$; hence, the local diameters converge to zero much faster. However, the total diameter $D^*(\zeta, \eta)$, represented by a dashed line, still fluctuates, which is similar to the bipartite network case.

In Fig. 3(d), we present a three-dimensional plot of the local diameter $D(\zeta)$ at time $t=10$ according to each pair $(K_s, K_d)$. Notice that $D(\zeta)$ tends to zero faster as the coupling strengths increase.

6.2.   Mixture of three homogeneous ensembles

In this subsection, we perform simulations under the conditions of Theorem 5.4. The conditions for the simulations are as follows:

In Fig. 4(a), particle distributions are displayed at $t=0$ and $t=10$. The different colors register the particles in different groups. Each initial local diameter is set to 2, and there is no restriction on the initial ensemble diameter.

In Figs. 4(b) and (c), we observe the local exponential synchronization.

In Fig. 4(d), we present a three-dimensional plot of the local diameter $D(\zeta)$ according to each pair $(t, K)$ with $K_{12}=K_{23}=K_{31}=0.01$. Notice that $D(\zeta)$ tends to zero as time $t$ increases only if $K$ is greater than a certain value, which is also consistent with condition (ⅲ) in Theorem 5.4.

7.   Conclusion

In this paper, we presented several sufficient conditions for the emergence of local exponential synchronization in a mixture of homogeneous Kuramoto ensembles. Our main tool for studying local synchronization was the Lyapunov functional supplemented by the continuity argument. We also extended the result for binary mixture to multi-mixture case. Restricting the initial configuration was crucial for estimating the diameter of the oscillators, although numerical simulations demonstrated that local exponential synchronization is plausible for generic initial configurations such as the classical Kuramoto model. In terms of local exponential synchronization, we were able to observe practical synchronization in a more general framework than in [21]. We found that it is not only the intra coupling strength, but also the inter coupling strength that make a contribution to the emergence of local exponential synchronization in each group. This is due to the fact that the inter coupling strength plays the role of the indirect coupling strength among the oscillators in a group. As evidenced by the numerical simulations, our local exponential synchronization seems to be true for generic initial configurations. However, as discussed in Section 2.2, when homogeneous ensembles have nontrivial intrinsic dynamics, the resulting coupled system may not be a gradient flow. Even if this is true, uniform boundedness of the ensemble is not generally guaranteed. Thus, the gradient flow arguments cannot be applied to our mixture case, unlike the Kuramoto model. Therefore, the emergence of local exponential synchronization for a generic initial configurations is left for a future work.