Common fixed point results via $\mathcal{A}_{\vartheta}$-$\alpha$-contractions with a pair and two pairs of self-mappings in the frame of an extended quasi $b$-metric space

1.   Introduction

Banach's fixed point theory, also referred to as "the contraction mapping theorem", is one of the most significant sources of existence and uniqueness theorems in many areas of analysis, it ensures the existence of a unique fixed point for self-mappings under appropriate contraction conditions over complete metric spaces.

In recent years some author have succeeded in obtaining many fixed and common fixed point findings for different classes of mappings by weakening their hypothesis or changing the Lipschitz constant to real valued functions such that their values are less than unity or in some other way by extending the fixed and common fixed point results from metric spaces to another spaces such as quasi metric spaces, cone metric spaces, $b$-metric space etc.

Some authors used $A$-contraction to introduce some new results, see ^[3,4,5,6,12]. Nurwahyu et al. ^[15] studied some fixed points for mapping of cyclic form. Recently, Ali et al. ^[7,9,10] applied the dynamic iteration to generate some new findings. Also, Shatanawi et al. ^[21,26] have linked some known results to cone metric spaces. Shatanawi ^[20] also studied some fixed point results in orbitally metric spaces. Some researchers have used $\omega$-distance to obtain new results, see ^[1,2,16,24]. Also, others have obtained results on $b$-metric spaces ^{[11,14,18,22,25]}, extended $b$-metric spaces ^[17,27] and quasi metric spaces, see ^{[19,23,28,30]}. Very recently, Ali et al. ^[8] have obtained new results on generalized $\theta_b$-contractions. Song et al. ^[29] utilized fuzzy sets for having their own results on fuzzy metric spaces.

In the current paper, we introduce the concept of $\mathcal{A}_{\vartheta}$-$\alpha$-contractions. We then take advantage of our new concept to formulate and prove some common fixed point results for self-mappings in the frame of an extended quasi $b$-metric space.

2.   Preliminaries

The purpose of this section is to collect the basic concepts from literature about extended quasi $b$-metric spaces, which we will need in our current work.

Definition 2.1. ^[30] A quasi metric space $(\mathcal{Y}, d)$ consists of a non-empty set $\mathcal{Y}$ and a function $d$: $\mathcal{Y} \times \mathcal{Y} \longrightarrow [0, \infty)$ such that

(1) $d(\mu, \nu) = 0$ if $\mu = \nu$, $\forall \mu, \nu\in \mathcal{Y}$.

(2) $d(\mu, \omega) \leq d(\mu, \nu) + d(\nu, \omega)$, $\forall \mu, \nu, \omega\in \mathcal{Y}$.

A function $d$ that satisfies the above conditions is called a quasi-metric.

Definition 2.2. ^[15] On a nonempty set $\mathcal{Y}\times\mathcal{Y}$, let $\theta:\mathcal{Y} \times \mathcal{Y}\longrightarrow [1, \infty)$ be a function. A function $d_{\theta}$: $\mathcal{Y} \times \mathcal{Y}\longrightarrow [0, \infty)$ is called an extended quasi $b$-metric if for all $\mu, \nu, \omega\in \mathcal{Y}$, we have

(1) $d_{\theta} (\mu, \nu) = 0$ if $\mu = \nu.$

(2) $d_{\theta}(\mu, \omega) \leq\theta(\mu, \omega)\big[d_{\theta}(\mu, \nu) + d_{\theta}(\nu, \omega)\big].$

The pair $(\mathcal{Y}, d_{\theta})$ is referred to as an extended quasi $b$-metric space.

If $d_{\theta}$ satisfying $(1)$ and $(2)$ with $\theta = s\geq 1$, then $(\mathcal{Y}, d_{s})$ is referred to as a quasi $b$-metric space with parameter s.

Example 2.1. ^[15] Consider $\mathcal{Y} = [0, 1]$, define $\theta$: $\mathcal{Y}\times \mathcal{Y} \rightarrow [1, \infty)$ by $\theta(\mu, \nu) = 2^{1-(\mu+\nu)/2}$ and $d_{\theta}$: $\mathcal{Y} \times \mathcal{Y} \rightarrow [0, \infty)$ by $d_{\theta}(\mu, \nu) = |2^{\mu-\nu}-1|$. Then $(\mathcal{Y}, d_{\theta})$ is an extended quasi $b$-metric space.

Definition 2.3. ^[27] Let $(\mu_n)$ be a sequence in the extended quasi $b$-metric space $(\mathcal{Y}, d_{\theta})$. Then, we say that $(\mu_n)$ converges to $\mu\in \mathcal{Y}$ if

Definition 2.4. ^[27] Let $(\mu_n)$ be a sequence in the extended quasi $b$-metric space $(\mathcal{Y}, d_{\theta})$. Then, we say that

(1) $(\mu_n)$ is left-Cauchy if and only if for every $\zeta > 0,$ $\exists N\in \mathbb{N}$ such that $d_{\theta}(\mu_n, \mu_\iota) < \zeta$ for all $n\geq \iota > N$.

(2) $(\mu_n)$ is right-Cauchy if and only if for every $\zeta > 0$, $\exists N\in \mathbb{N}$ such that $d_{\theta}(\mu_\iota, \mu_n) < \zeta$ for all $n\geq \iota > N$.

(3) $(\mu_n)$ is Cauchy if and only if it is left-Cauchy and right-Cauchy.

Definition 2.5. ^[27] Let $(\mathcal{Y}, d_{\theta})$ be an extended quasi $b$-metric space. Then, we say that

(1) $(\mathcal{Y}, d_{\theta})$ is left-complete if and only if each left-Cauchy sequence in $\mathcal{Y}$ converges.

(2) $(\mathcal{Y}, d_{\theta})$ is right-complete if and only if each right-Cauchy sequence in $\mathcal{Y}$ converges.

(3) $(\mathcal{Y}, d_{\theta})$ is complete if and only if each Cauchy sequence in $\mathcal{Y}$ converges.

We adopt ^[13,15] to generate the following definition:

Definition 2.6. Let $(\mathcal{Y}, d_{\theta})$ be an extended quasi $b$-metric space. Two self-mappings $f$ and $\ell$ on $\mathcal{Y}$ are said to be compatible if $\lim_{n\rightarrow \infty} d_{\theta}(f\ell\mu_n, \ell f\mu_n) = 0$ and $\lim_{n\rightarrow \infty} d_{\theta}(\ell f\mu_n, f\ell\mu_n) = 0$ when $(\mu_n)$ is a sequence in $\mathcal{Y}$ such that $\lim_{n\rightarrow \infty} f\mu_n = \lim_{n\rightarrow \infty} \ell\mu_n = \nu$ for some $\nu\in \mathcal{Y}.$

Akram and Siddiqui ^[4] introduced a new class of functions, denoted by $A$, as follows: $\tau\in A$ if $\tau$: $\mathbb{R}^3_+\rightarrow \mathbb{R}_+$ satisfies the following assertions:

${\rm (i)}$ $\tau$ is continuous on $\mathbb{R}_+^3.$

${\rm (ii)}$ $\kappa_1\leq\lambda \eta_1$ for some $\lambda\in[0, 1)$, when $\kappa_1\leq\tau(\kappa_1, \eta_1, \eta_1)$ or $\kappa_1\leq\tau(\eta_1, \kappa_1, \eta_1)$ or $\kappa_1\leq\tau(\eta_1, \eta_1, \kappa_1)$ for $\kappa_1, \eta_1\in \mathbb{R}_+.$

Akram and Siddiqui ^[4] took advantage of class $A$ to introduce a new concept of contractions called $A$-contraction as follows:

Definition 2.7. ^[4] On a metric space $(\mathcal{Y}, d)$, a self-mapping $\ell$ is called $A$-contraction if there exists $\tau\in A$ such that

holds for all for all $\mu, \nu \in \mathcal{Y}$.

Based on the above definitions, we extend the class of contraction into a new class known as $\mathcal{A}_{\vartheta}$, from which we derive some common fixed point theorems, as described in the next section.

3.   Main results

In this section, we introduce a new concept of contractions called $\mathcal{A}_{\vartheta}$-$\alpha$-contraction. We then take advantage of our concept to prove the existence and uniqueness common fixed point for self-mappings in complete extended quasi $b$-metric spaces.

To begin our work, we introduce a new class of functions, denoted by $\mathcal{A}_{\vartheta}$, as follows: $\vartheta$: $\mathbb{R}^4_+\rightarrow \mathbb{R}_+\in \mathcal{A}_{\vartheta}$ if $\vartheta$ satisfies the following conditions:

${\rm (i)}$ $\vartheta$ is continuous.

${\rm (ii)}$ $\vartheta$ is non-decreasing in all of its variables.

${\rm (iii)}$ If $\kappa\leq \vartheta(\eta, \kappa, \eta, \alpha(\kappa+\eta)),$ $\kappa\leq \vartheta(\kappa, \eta, \eta, \alpha(\kappa+\eta)),$ or $\kappa\leq \vartheta(\eta, \eta, \kappa, \alpha(\kappa+\eta))$ for $\kappa, \eta \in \mathbb{R}_+$ and $\alpha\in (0, 1),$ then $\kappa\leq\lambda \eta$ for some $\lambda\in[0, 1)$.

Example 3.1. Define the function $\vartheta$: $\mathbb{R}^4_+\rightarrow \mathbb{R}_+$ by $\vartheta(\kappa, \eta, \grave{\kappa}, \grave{\eta}) = \frac{1}{6}(\kappa+\eta+\grave{\kappa}+\grave{\eta})$. Then $\vartheta\in \mathcal{A}_\vartheta$.

Proof. Note that the function $\vartheta$ is well-defined. Moreover, one can easily see $(i)$ and $(ii)$ are satisfied. To prove $(iii)$, we assume that $\kappa\leq\vartheta(\eta, \kappa, \eta, \alpha(\kappa+\eta))$, $\kappa\leq \vartheta(\kappa, \eta, \eta, \alpha(\kappa+\eta)),$ or $\kappa\leq \vartheta(\eta, \eta, \kappa, \alpha(\kappa+\eta))$ for some $\alpha\in (0, 1)$. Take $\lambda = \frac{2+\alpha}{5-\alpha}$, then $\lambda < \frac{3}{4}$. Moreover, with few calculations, one can prove that $\kappa\leq\lambda\eta$.

We have provided the background needed to initiate a new contraction, called $\mathcal{A}_{\vartheta}$-contraction:

Definition 3.1. Let $\alpha\in (0, 1)$, $(\mathcal{Y}, d_\theta)$ be an extended quasi $b$-metric space and $f, \ell$ be two self-mappings on $\mathcal{Y}$. Then the pair $(f, \ell)$ is said to be $\mathcal{A}_{\vartheta}$-$\alpha$-contraction if there exists $\vartheta\in \mathcal{A}_{\vartheta}$ such that

and

hold for all $\mu, \nu\in\mathcal{Y}$

Now, we will present and prove our main result.

Theorem 3.1. Let $\mathcal{Y}$ be a nonempty set, $\alpha\in (0, 1)$, $(\mathcal{Y}, d_\theta)$ be a complete extended quasi $b$-metric space and $f, \ell$ be two self-mappings on $\mathcal{Y}$. Assume the followings:

(1) $\ell$ is continuous.

(2) $\theta(\kappa, \eta)\leq\frac{1}{\alpha}$ for all $\kappa, \eta\in\mathcal{Y}$.

(3) $d_\theta$ is continuous in its variables.

If $(f, \ell)$ is $\mathcal{A}_\vartheta$-$\alpha$-contraction, then $f$ and $\ell$ have a unique common fixed point provided that $\lambda < \alpha$, where $\lambda$ is the constant satisfies condition (iii) of the definition $\mathcal{A}_\vartheta$.

Proof. Choose $\mu_0\in\mathcal{Y}$. Take $\mu_1 = f\mu_0$ and $\mu_2 = \ell\mu_1$. Then, we construct a sequence $(\mu_n)$ as follows:

Now, we verify that $(\mu_n)$ is left Cauchy. Look at

Using $\mathcal{A}_{\vartheta}$-$\alpha$-contraction condition, we get

Therefore,

Since $\theta$ is bounded by $\frac{1}{\alpha}$, we obtain

Due to the triangular inequality, we obtain

By putting $\kappa = d_{\theta}(\mu_{2n+1}, \mu_{2n})$ and $\eta = d_{\theta}(\mu_{2n}, \mu_{2n-1})$, we obtain $\kappa\leq\vartheta(\eta, \kappa, \eta, \alpha(\kappa+\eta))$. Thus we have $\kappa\leq\lambda \eta$ for some $\lambda\in[0, 1)$. Hence

By induction, we get

Thus

From here one can show that $(\mu_n)$ is left Cauchy. Similarly, we can show that $(\mu_n)$ is right Cauchy. As a result, $(\mu_n)$ is Cauchy. So $(\mu_n)$ converges to $\mu,$ for some $\mu\in \mathcal{Y};$ that is,

and

Claim. $\mu$ is a common fixed point of $f$ and $\ell$. Look at

Since $(f, \ell)$ is $\mathcal{A}_{\vartheta}$-$\alpha$-contraction, then

Since $\theta$ is bounded by $\frac{1}{\alpha}$ and by the triangular inequality, we get

By allowing $n\longrightarrow\infty$ in Inequality (3.3), the continuity of $\vartheta$ and $d_\theta$ in their variables implies that

By taking $\kappa = d_{\theta}(f\mu, \mu)$ and $\eta = 0$, then $d_{\theta}(f\mu, \mu) \leq\lambda0 = 0$ for some $\lambda\in[0, 1)$. As a result, $\mu$ is a fixed point of $f$.

Due to the continuity of $\ell$ and the continuity of $d_\theta$ in its variables, we have

which implies that $\ell\mu = \mu$. So $\mu$ is a fixed point of $\ell$.

Finally, to demonstrate the uniqueness, suppose $\mu^*$ is another common fixed point of $f$ and $\ell$ such that $\mu^*\neq\mu$. So, we have

Since $(f, \ell)$ is $\mathcal{A}_\vartheta$-$\alpha$-contraction, then

By taking $\kappa = d_{\theta}(\mu, \mu^*)$ and $\eta = 0$, then $\kappa\leq\lambda \eta$ for some $\lambda\in[0, 1)$. Therefore

Hence $\mu = \mu^*$, a contradiction. Thus $\mu$ is a unique common fixed point of $f$ and $\ell$.

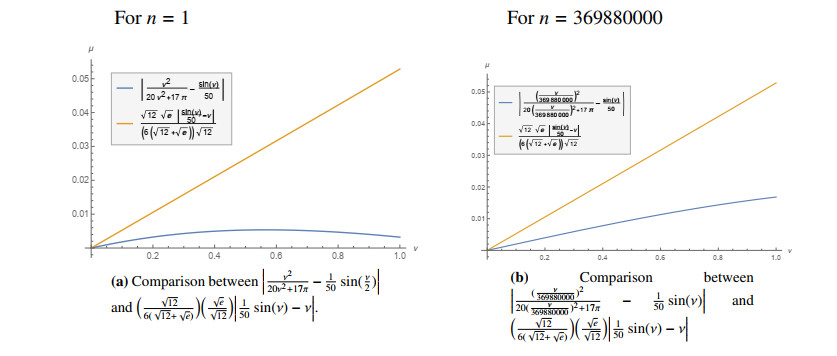
We support our result with the following example, $e$ denotes the Euler's number and $\pi$ denotes the ratio of a circle's circumference to its diameter.

Example 3.2. On $\mathcal{Y} = [0, 1]$, define the mapping $\theta$: $\mathcal{Y}\times \mathcal{Y}\rightarrow [1, \infty)$ via $\theta = 1+|\mu-\nu|$ and define $d_{\theta}$: $\mathcal{Y}\times \mathcal{Y}\rightarrow [0, \infty)$ via $d_{\theta} = |\mu-\nu|$. Then $(\mathcal{Y}, d_{\theta})$ is a complete extended quasi $b$-metric space.

Define the mappings $f, \ell$: $\mathcal{Y}\rightarrow \mathcal{Y}$ by $f(\mu) = \frac{\mu^2}{20\mu^2+17\pi}$ and $\ell(\nu) = \frac{1}{50}\sin(\nu)$. Also, define $\vartheta$: $\mathbb{R}^4_+\rightarrow \mathbb{R}_+$ by

Note that $\vartheta$ is continuous and non-decreasing in all of its variables. Now, assume that

or

Take

with few calculations, we find $\kappa\leq\lambda\eta$ for $\kappa, \eta\in\mathcal{Y}$. Indeed,

Thus,

Note that we can easily figure out:

(1) $\ell$ is continuous.

(2) $\theta(\mu, \nu)\leq\frac{1}{\alpha} = \frac{\sqrt{12}}{\sqrt{e}}$ for all $\mu, \nu\in\mathcal{Y}$.

(3) $d_\theta$ is continuous on its variables.

Given $\mu, \nu\in \mathcal{Y}$, with $\nu > \mu$, let $n\in [1, +\infty)$ such that $\mu = \frac{\nu}{n}$. Then

From Figure 1 (a and b), we deduce the following inequality:

Therefore

Thus,

On a similar manner, we can get

Thus $(f, l)$ is $\mathcal{A}_\vartheta$-$\alpha$-contraction. So all conditions of Theorem 3.1 are satisfied. Hence $f$ and $l$ have a common fixed point. Here, 0 is the unique common fixed of $f$ and $0$.

Corollary 3.1. On the complete quasi $b$-metric space $(\mathcal{Y}, d_s)$, let $f$ and $\ell$ be two self-mappings on $\mathcal{Y}$. Suppose there exist $\alpha \in (0, 1)$ and $\vartheta\in \mathcal{A}_\vartheta$ with

and

hold for all $\mu, \nu\in \mathcal{Y}$. Then $f$ and $\ell$ have a unique common fixed point in $\mathcal{Y}$ provided that $\ell$ is continuous, $s\leq\frac{1}{\alpha}$ and $\lambda < \alpha$, where $\lambda$ is the constant satisfies condition (iii) of the definition $\mathcal{A}_\vartheta$.

Proof. The desired result will be obtained from Theorem (3.1) by defining $\theta:\mathcal{Y}\times\mathcal{Y}\rightarrow [1, +\infty)$ via $\theta(\kappa, \eta) = s$, $s\geq1$.

Corollary 3.2. On the complete extended quasi $b$-metric space $(\mathcal{Y}, d_\theta)$, let $f$ be a continuous self-mapping on $\mathcal{Y}$. Assume there exist $\alpha\in (0, 1)$ and $\vartheta\in\mathcal{A}_{\vartheta}$ such that

and

hold for all $\mu, \nu\in \mathcal{Y}$. Then $f$ has a unique fixed point in $\mathcal{Y}$ provided that $\theta$ is bounded by $\frac{1}{\alpha}$ and $\lambda < \alpha$, where $\lambda$ is the constant satisfies condition (iii) of the definition $\mathcal{A}_\vartheta$.

Proof. The desired result will be obtained from Theorem (3.1) by taking $\ell = f$.

Corollary 3.3. On the complete quasi $b$-metric space $(\mathcal{Y}, d_s)$, let $f$ be a continuous mapping on $\mathcal{Y}$. Suppose there exist $\alpha \in (0, 1)$ and $\vartheta\in \mathcal{A}_\vartheta$ such that

and

hold for all $\mu, \nu\in \mathcal{Y}$.

If $s\leq \frac{1}{\alpha}$, then the mapping $f$ has a unique fixed point in $\mathcal{Y}$ provided that $\lambda < \alpha$, where $\lambda$ is the constant satisfies condition (iii) of the definition $\mathcal{A}_\vartheta$.

Proof. The desired result will be obtained from Corollary (3.1) by taking $f = \ell$. Our second main result for four self-mappings is as follows:

Theorem 3.2. Let $\mathcal{Y}$ be a nonempty set, $\alpha\in(0, 1)$, $(\mathcal{Y}, d_\theta)$ be a complete extended quasi $b$-metric space, and $f, l, g$ and $h$ be four self mappings on $\mathcal{Y}$. Assume the following conditions:

(1) $f(\mathcal{Y})\subseteq h(\mathcal{Y})$ and $g(\mathcal{Y})\subseteq l(\mathcal{Y})$.

(2) $f$ or $l$ is continuous.

(3) $\theta(\kappa, \eta)\leq\frac{1}{\alpha}$ for all $\kappa, \eta\in \mathcal{Y}$.

(4) $d_\theta$ is continuous in its variables.

(5) $(f, l)$ and $(g, h)$ are compatible.

(6) There exists $\vartheta\in\mathcal{A}_\theta$ such that

and

hold for all $\mu, \nu\in \mathcal{Y}$.

Then $f, l, g$ and $h$ have a unique common fixed point in $\mathcal{Y}$ provided that $\lambda < \alpha$, where $\lambda$ is the constant satisfies condition (iii) of the definition $\mathcal{A}_\vartheta$.

Proof. Choose $\mu_0$ in $\mathcal{Y}$. Since $f(\mathcal{Y})\subseteq h(\mathcal{Y})$ and $g(\mathcal{Y})\subseteq l(\mathcal{Y})$, then $\exists \mu_1, \mu_2$ in $\mathcal{Y}$ such that $f\mu_0 = h \mu_1$, $g\mu_1 = l\mu_2$. By continuing this process, we construct a sequence $(\nu_n)$ in $\mathcal{Y}$ as follows:

By Condition ($3.4$), we get

Since $\theta$ is bounded by $\frac{1}{\alpha}$ and due to the triangular inequality of $d_{\theta}$, we get

By putting $\kappa = d_{\theta}(\nu_{2n}, \nu_{2n+1})$ and $\eta = d_{\theta}(\nu_{2n-1}, \nu_{2n})$, we obtain $\kappa\leq\vartheta(\eta, \eta, \kappa, \alpha(\kappa+\eta))$. Hence $\kappa\leq\lambda\eta$ for some $\lambda\in[0, 1)$; that is

Hence, we have

Thus

From here one can show that $(\nu_n)$ is right-Cauchy. On the same way, we can prove that $(\nu_n)$ is left-Cauchy. As a result, $(\nu_n)$ is a Cauchy sequence. So $\exists \varsigma\in \mathcal{Y}$ such that

Claim. $\varsigma$ is a common fixed point for $f, g, h$ and $l$. If $l$ is continuous, then

Since $(f, l)$ is compatible, then $\lim_{n\rightarrow \infty}d_{\theta}(lf\mu_{2n}, fl\mu_{2n}) = 0$. The triangular inequality of $d_{\theta}$ implies

Letting $n\rightarrow \infty$ and recalling the continuity of $d_\theta$, we get

Thus

Now

By letting $n\rightarrow \infty$ in above inequalities, we arrive at $d_{\theta}(l\varsigma, f\varsigma) = 0$. Thus, $\varsigma$ is a coincidence point for $f$ and $l$ in $\mathcal{Y}$. Let $\mu = \varsigma$ and $\nu = \mu_{2n+1}$ in Inequality (3.4), we obtain

By using triangle inequality and keeping in our account that $\theta$ is bounded by $\frac{1}{\alpha}$, we find

By allowing $n\rightarrow \infty$ in above inequality, we obtain

By putting $\kappa = d_{\theta}(l\varsigma, \varsigma)$ and $\eta = 0$, we obtain that $\kappa\leq\vartheta(\kappa, 0, 0, \alpha(\kappa+0))$. Hence $\kappa\leq\lambda \eta$ for some $\lambda\in[0, 1)$; that is

Thus $l\varsigma = \varsigma$ and hence $f\varsigma = \varsigma.$ Since $f(\mathcal{Y})\subseteq h(\mathcal{Y}),$ there exists $p\in \mathcal{Y}$ such that $\varsigma = f\varsigma = hp.$

By putting $\mu = \mu_{2n}$ and $\nu = p$ in Inequality (3.4), we obtain

Through the triangle inequality, given that $\theta$ is bounded by $\frac{1}{\alpha}$, we get

By allowing $n\rightarrow \infty$ and since $hp = \varsigma$, we obtain

By putting $\kappa = d_{\theta}(\varsigma, gp)$ and $\eta = 0$, we get $\kappa\leq\vartheta(0, 0, \kappa, \alpha(\kappa+0))$. So $\kappa\leq\lambda \eta$ for some $\lambda\in[0, 1)$; that is

Therefore $gp = \varsigma$ and hence $gp = hp = \varsigma.$ Now,

Since $\theta$ is bounded by $\frac{1}{\alpha}$ and $(g, h)$ is compatible, we have $d_{\theta}(h\varsigma, g\varsigma) = 0$. Thus, $h\varsigma = g\varsigma.$

By putting $\mu = \mu_{2n}$ and $\nu = \varsigma$ in Inequality (3.4), we obtain

Through the triangle inequality, given that $\theta$ bounded by $\frac{1}{\alpha}$, we get

By allowing $n\rightarrow \infty$ in above inequality, we get

Setting $\kappa = d_{\theta}(\varsigma, g\varsigma)$ and $\eta = 0$, we obtain that $\kappa\leq\vartheta(\kappa, 0, 0, \alpha(\kappa+0))$. Thus $\kappa\leq\lambda \eta$ for some $\lambda\in[0, 1)$; that is

So $g\varsigma = \varsigma$ and hence $g\varsigma = h\varsigma = \varsigma$. Therefore $l\varsigma = f\varsigma = g\varsigma = h\varsigma = \varsigma.$ For the sake of uniqueness, assume that $\varsigma*$ is another common fixed point for $f, g, h$ and $l$ such that $\varsigma*\neq\varsigma$. Then

Thus, we conclude that

So $\varsigma = \varsigma^*$, a contradiction. Thus $f, g, h$ and $l$ have a unique common fixed point.

Now, we support Theorem 3.2 with the following example:

Example 3.3. On the same complete space of Example $(3.2)$. Define the mappings $f, g, l, h:\mathcal{Y}\rightarrow \mathcal{Y}$ by

Define $\vartheta$: $\mathbb{R}^4_+\rightarrow \mathbb{R}_+$ by

It is clear that $\vartheta$ is continuous and non-decreasing in all of its variables. Now, suppose that

or

Then, with a few calculations, we get $\kappa\leq \frac{1}{4}\eta$ for $\kappa, \eta\in\mathcal{Y}$.

Note that we can easily figure out:

(1) $f(\mathcal{Y})\subseteq h(\mathcal{Y})$ and $g(\mathcal{Y})\subseteq l(\mathcal{Y}).$

(2) $f$, $g$, $h$ and $l$ are continuous.

(3) $\theta(\mu, \nu)\leq\frac{1}{\alpha} = \frac{5}{2}$ for all $\mu, \nu\in\mathcal{Y}$.

(4) $d_\theta$ is continuous in its variables.

To show that $(f, l)$ is compatible, let $(\mu_n)$ be a sequence in $\mathcal{Y}$ such that

for some $\nu\in \mathcal{Y}.$ So

Therefore $\mu_n\rightarrow \frac{7}{6}\nu$ and $\sin(\frac{\mu_n}{4})\rightarrow 12\nu.$ Hence $\sin(\frac{\mu_n}{4})\rightarrow \sin(\frac{7}{24}\nu)$ and $\sin(\frac{\mu_n}{4})\rightarrow 12\nu.$

By the uniqueness of limit in Real numbers, we conclude that $\sin(\frac{7}{24}\nu) = 12\nu.$ Thus $\nu = 0$ and so $\mu_n\rightarrow 0$.

So the pair $(f, l)$ is compatible. Similarly, one can show that the pair $(g, h)$ is compatible.

Given $\mu, \nu\in \mathcal{Y}$, with $\nu\geq\mu$, let $n\in [1, +\infty)$ such that $\nu = n\mu$

From Figure 2 (a and b), we deduce that

Therefore

Thus,

On a similar manner, we can get

Therefore, all conditions of Theorem $(3.2)$ have been fulfilled. So the desired result is obtained.

Corollary 3.4. Let $\mathcal{Y}$ be a non-empty set, $(\mathcal{Y}, d_s)$ be a quasi $b$-metric space, $\alpha\in(0, 1)$, and $f, l, h, g$ be four self-mappings on $\mathcal{Y}$. Suppose the following conditions:

(1) $f(\mathcal{Y})\subseteq h(\mathcal{Y})$ and $g(\mathcal{Y})\subseteq l(\mathcal{Y})$.

(2) $f$ or $l$ is continuous.

(3) $s\leq\frac{1}{\alpha}$.

(4) $d_\theta$ is continuous in its variables.

(5) The pairs $(f, l)$ and $(g, h)$ are compatible.

(6) There exists $\vartheta\in \mathcal{A}_{\vartheta}$ such that

and

hold for all $\mu, \nu\in \mathcal{Y}$.

Then $f, l, h$ and $g$ have a unique common fixed point in $\mathcal{Y}$ provided that $\lambda < \alpha$, where $\lambda$ is the constant satisfies condition (iii) of the definition $\mathcal{A}_\vartheta$..

Proof. The desired result will be obtained from Theorem (3.2) by defining $\theta$: $\mathcal{Y}\times\mathcal{Y}\rightarrow [1, +\infty)$ via $\theta(\kappa, \eta) = s$.

4.   Conclusions

In the current paper, we introduced a new concept called $\mathcal{A}_{\vartheta}$-$\alpha$-contraction. We used our new concept to introduce and prove some common fixed point results for several self-mappings under a set of conditions over an extended quasi $b$-metric space. Also, we have provided some examples to show the novelty of our results.

Acknowledgments

The authors would like to thank their affiliations for facilitating the publication of this paper through their support.

Conflict of interest

The authors declare no conflicts of interest.