Numerical investigation of fractional-order wave-like equation

M. Mossa Al-Sawalha; Rasool Shah; Kamsing Nonlaopon; Osama Y. Ababneh; M. Mossa Al-Sawalha; Rasool Shah; Kamsing Nonlaopon; Osama Y. Ababneh

doi:10.3934/math.2023265

AIMS Mathematics

2023, Volume 8, Issue 3: 5281-5302. doi: 10.3934/math.2023265

Previous Article Next Article

Research article

Numerical investigation of fractional-order wave-like equation

1.
Department of Mathematics, College of Science, University of Ha'il, Ha'il 2440, Saudi Arabia
2.
Department of Mathematics, Abdul Wali Khan University, Mardan 23200, Pakistan
3.
Department of Mathematics, Faculty of Science, Khon Kaen University, Khon Kaen 40002, Thailand
4.
Department of Mathematics, Faculty of Science, Zarqa University, Zarqa 13110, Jordan

Received: 03 September 2022 Revised: 28 November 2022 Accepted: 01 December 2022 Published: 14 December 2022
MSC : 32B15, 34A34, 35A22, 35A24, 45A10

The two approaches to solving nonlinear Caputo time-fractional wave-like equations with variable coefficients are examined in this study. The Homotopy perturbation transform method and the Yang transform decomposition method are the names of these two techniques. Three separate numerical examples are provided to demonstrate the effectiveness and precision of the suggested methods. The results were acquired to demonstrate the effectiveness and power of the two approaches, providing estimates with better precision and closed form solutions. The solutions to these kinds of equations can be found using the suggested methods as infinite series, and when these series are in closed form, they provide the exact solution. The suggested techniques have been demonstrated to be effective and efficient in their application. Three numerical examples are used to examine the methods accuracy and effectiveness.

Keywords:

Homotopy perturbation method,
Adomian decomposition method,
Yang transform,
nonlinear time-fractional wave-like equations,
Caputo operator

Citation: M. Mossa Al-Sawalha, Rasool Shah, Kamsing Nonlaopon, Osama Y. Ababneh. Numerical investigation of fractional-order wave-like equation[J]. AIMS Mathematics, 2023, 8(3): 5281-5302. doi: 10.3934/math.2023265

Related Papers:

[1]	Azzh Saad Alshehry, Naila Amir, Naveed Iqbal, Rasool Shah, Kamsing Nonlaopon . On the solution of nonlinear fractional-order shock wave equation via analytical method. AIMS Mathematics, 2022, 7(10): 19325-19343. doi: 10.3934/math.20221061
[2]	M. Mossa Al-Sawalha, Azzh Saad Alshehry, Kamsing Nonlaopon, Rasool Shah, Osama Y. Ababneh . Approximate analytical solution of time-fractional vibration equation via reliable numerical algorithm. AIMS Mathematics, 2022, 7(11): 19739-19757. doi: 10.3934/math.20221082
[3]	Yousef Jawarneh, Humaira Yasmin, M. Mossa Al-Sawalha, Rasool Shah, Asfandyar Khan . Fractional comparative analysis of Camassa-Holm and Degasperis-Procesi equations. AIMS Mathematics, 2023, 8(11): 25845-25862. doi: 10.3934/math.20231318
[4]	Naveed Iqbal, Saleh Alshammari, Thongchai Botmart . Evaluation of regularized long-wave equation via Caputo and Caputo-Fabrizio fractional derivatives. AIMS Mathematics, 2022, 7(11): 20401-20419. doi: 10.3934/math.20221118
[5]	Rasool Shah, Abd-Allah Hyder, Naveed Iqbal, Thongchai Botmart . Fractional view evaluation system of Schrödinger-KdV equation by a comparative analysis. AIMS Mathematics, 2022, 7(11): 19846-19864. doi: 10.3934/math.20221087
[6]	Manal Alqhtani, Khaled M. Saad, Rasool Shah, Thongchai Botmart, Waleed M. Hamanah . Evaluation of fractional-order equal width equations with the exponential-decay kernel. AIMS Mathematics, 2022, 7(9): 17236-17251. doi: 10.3934/math.2022949
[7]	Aslı Alkan, Halil Anaç . The novel numerical solutions for time-fractional Fornberg-Whitham equation by using fractional natural transform decomposition method. AIMS Mathematics, 2024, 9(9): 25333-25359. doi: 10.3934/math.20241237
[8]	Y. Massoun, C. Cesarano, A. K Alomari, A. Said . Numerical study of fractional phi-4 equation. AIMS Mathematics, 2024, 9(4): 8630-8640. doi: 10.3934/math.2024418
[9]	Muhammad Imran Liaqat, Sina Etemad, Shahram Rezapour, Choonkil Park . A novel analytical Aboodh residual power series method for solving linear and nonlinear time-fractional partial differential equations with variable coefficients. AIMS Mathematics, 2022, 7(9): 16917-16948. doi: 10.3934/math.2022929
[10]	Abdul Hamid Ganie, Fatemah Mofarreh, Adnan Khan . A novel analysis of the time-fractional nonlinear dispersive K(m, n, 1) equations using the homotopy perturbation transform method and Yang transform decomposition method. AIMS Mathematics, 2024, 9(1): 1877-1898. doi: 10.3934/math.2024092

Abstract

1. Introduction

Today, fractional calculus (FC) is widely acknowledged as a vital technique for describing real life events ^[1,2,3,4,5]. The presence of fractional formulations is discussed in a range of contexts, despite the fact that scholars view FC as a useful tool in systematic investigation ^[6,7]. In addition to being consistent with the current stage, the dynamical properties of fractional differential equation systems typically provide a sufficient justification for earlier stages ^[8,9]. Due to the novelty of the diversification process, which can result in a modest adjustment leading to significant output variability, the conversion of integer-order Differential equation (DE)-regulated systems to fractional DE-regulated structures should be accurate. Fractional calculus has received a lot of attention, and various applications in the fields of science have been presented ^[1,2,3]. Fractional differential equations are widely used in domains including signal processing, system recognition, reaction diffusion processes, control systems using dynamical systems, random walk models, and neural networks to simulate real-world phenomena ^[13,14]. FC provides us with a useful tool for characterizing memory and hereditary characteristics of various systems.

Differential equations with fractional derivatives offer effective methods for identifying memory and heredity features that classical systems typically ignore. Fractional-order derivative modelling is helpful for studying dynamical systems and accurately describes how real-world systems behave. In applied mathematics and physics, fractional differential equations are frequently employed in interpreting and modeling many realistic issues, including diffusion, fluid mechanics, chemistry, viscoelasticity, damping laws, electrical circuits, mathematical biology, relaxation processes, and so on ^[15,16,17]. Finding the numerical solution to fractional differential equations, linear and nonlinear, is now receiving much more attention from mathematicians. Some of the methods are Homotopy Analysis Method ^[18], Differential Transform Method ^[19], and Adomian Decomposition Method ^[20]. Many researchers have suggested innovative integral transform techniques to discover the analytical solution of linear and nonlinear FDEs. Some of them are Sumudu ^[21], Elzaki ^[22], Laplace ^[23], Mahgoub ^[24] and Natural ^[25]. For solving the nonlinear system of FDEs, the Adomian decomposition method was combined with Sumudu transform method ^[26], with Elzaki transform method ^[27,28], with Laplace transform method ^[29], with Mahgoub transform method ^[30] and with Natural transform method ^[31,32] and so on ^[33,34,35].

This study aims to solve the nonlinear Caputo time-fractional wave-like equation with variable coefficients by combining two effective methods: The Yang transform decomposition method (YTDM), which combines the Yang transform method and the Adomian decomposition method, and the Homotopy perturbation transform method (HPTM), which combines the Yang transform method and the Homotopy perturbation method. Xiao-Jun Yang presented the Yang transform, which can solve various differential equations with constant coefficients. The Adomian decomposition technique (ADM) ^[36,37] is a well-known systematic method for solving deterministic or stochastic operator equations, such as ordinary, partial, integral, and integro-differential equations. The ADM is a sophisticated technique that provides fast algorithms for analytical solutions and numeric simulation in engineering and applied sciences. On the other hand, He ^[38,39] presented the homotopy perturbation approach in 1998. This method sees the solution as the sum of an infinite series, which usually converges quickly to accurate solutions. This approach has been used for a wide range of mathematical problems. The nonlinear Caputo time-fractional wave-like equations with variable coefficients are presented as follows:

$\begin{align} D_{\varsigma}^{\wp}\mathbb{U}& = \sum\limits_{i,j = 1}^{n}B_{1ij}(\chi,\varsigma,\mathbb{U})\frac{\partial^{k+m}}{\partial \textbf{y}_{i}^{k}\partial \textbf{y}_{j}^{m}}B_{2ij}(\mathbb{U}_{\textbf{y}i},\mathbb{U}_{\textbf{y}j})\\ &\quad+\sum\limits_{i = 1}^{n}C_{1i}(\chi,\varsigma,\mathbb{U})\frac{\partial^{p}}{\partial \textbf{y}_{i}^{p}}C_{2i}(\mathbb{U}_{\textbf{y}i})+D(\chi,\varsigma,\mathbb{U})+E(\chi,\varsigma), \end{align}$

(1.1)

with initial values

$\begin{equation} \mathbb{U}(\chi,0) = a_{0}(\chi)\;\;\text{and}\;\;\mathbb{U}_{\varsigma}(\chi,0) = a_{1}(\chi), \end{equation}$

(1.2)

where $D_{\varsigma}^{\wp}$ is (Caputo) fractional operator having order $\wp$ and $1 < \wp\leq2, \mathbb{U} = \mathbb{U}(\chi, \varsigma), \chi = (\textbf{y}_{1}, \textbf{y}_{2}, \dots, \textbf{y}_{n})\in\mathbb{R}^{n}, \; \varsigma\geq0, \; B_{1ij}, \; C_{1i}i, \; j\in{1, 2, \dots, n}$ are nonlinear functions of $\chi, \varsigma$ and $\mathbb{U}, \; B_{2ij}, \; C_{2i}i, \; j\in{1, 2, \dots, n}$ , are nonlinear functions of derivatives of $\mathbb{U}$ with respect to $\textbf{y}_{i}$ and $\textbf{y}_{j}i, \; j\in{1, 2, \dots, n}$ , respectively. In addition, $D, E$ are nonlinear functions and $k, m, p$ are integers.

It should be noted that (1.1) simplifies to the standard wave-like equations with variable coefficients when $\wp = 2$ . Numerous applied sciences, including nonlinear hydrodynamics, mathematical physics, physics, plasma physics, astrophysics, human movement sciences, and engineering biophysics, all highly depend on these kinds of equations. These equations explain the development of microscopic particles moving erratically when submerged in fluids, variations in laser light intensity, and velocity distributions of fluid particles in turbulent flows ^[40].

The rest of the paper is organized as follows: Introduction is included in Section 1. The fundamental definitions of FC, the Yang transform, and its properties are given in Section 2. The notion of HPTM is presented in Section 3, whereas the idea of YTDM is presented in Section 4. Its application to the nonlinear time-fractional wave-like equations is demonstrated in Section 5. We summarise the conclusion in Section 6.

2. Preliminaries

This part clearly explains certain key facts about the fractional derivatives and Yang transform along with their properties.

Definition 2.1. ^[1] The Caputo fractional derivative is given as

$\begin{equation} D_{\varsigma}^{\wp}\mathbb{U}(\textbf{y},\varsigma) = \frac{1}{\Gamma(k-\wp)}\int_{0}^{\varsigma}(\varsigma-\wp)^{k-\wp-1}\mathbb{U}^{(k)}(\textbf{y},\wp)d\wp, \end{equation}$

(2.1)

where $k-1 < \wp\leq k$ and $k\in\mathbb{N}$ .

Definition 2.2. ^[41] Yang transform of a function $\mathbb{U}(\varsigma)$ is given as:

$\begin{equation} Y\{\mathbb{U}(\varsigma)\} = M(u) = \int_0^{\infty}e^{\frac{-\varsigma}{u}}\mathbb{U}(\varsigma)d\varsigma,\quad\varsigma > 0, u\in(-\varsigma_1,\varsigma_2), \end{equation}$

(2.2)

with Yang inverse transform as

$\begin{equation} Y^{-1}\{M(u)\} = \mathbb{U}(\varsigma). \end{equation}$

(2.3)

Definition 2.3. ^[41] Yang transform of a function with derivative of nth order as

$\begin{equation} Y\{\mathbb{U}^{n}(\varsigma)\} = \frac{M(u)}{u^{n}}-\sum\limits_{k = 0}^{n-1}\frac{\mathbb{U}^{k}(0)}{u^{n-k-1}}, \end{equation}$

(2.4)

for all $n\in\mathbb{N}$ .

Definition 2.4. ^[41] Yang transform of the function with derivative having fractional-order as

$\begin{equation} Y\left\{\mathbb{U}^{\wp}(\varsigma)\right\} = \frac{M(u)}{u^{\wp}}-\sum\limits_{k = 0}^{n-1}\frac{\mathbb{U}^{k}(0)}{u^{\wp-(k+1)}},\quad 0 < \wp \leq n. \end{equation}$

(2.5)

3. Construction of Homotopy perturbation transform method for solving FDEs

To illustrate the process of solution of the HPTM, we take FDEs of the form to show the general implementation of the proposed method as stated in ^[42,43]

$\begin{equation} D_{\varsigma}^{\wp}\mathbb{U}(\textbf{y},\varsigma) = \mathcal{P}_1[\textbf{y}]\mathbb{U}(\textbf{y},\varsigma)+\mathcal{Q}_1[\textbf{y}]\mathbb{U}(\textbf{y},\varsigma),\quad 1 < \wp\leq2, \end{equation}$

(3.1)

having initial values

$\begin{equation} \mathbb{U}(\textbf{y},0) = \xi(\textbf{y}),\quad\frac{\partial}{\partial\varsigma}\mathbb{U}(\textbf{y},0) = \zeta(\textbf{y}), \end{equation}$

(3.2)

where $D_{\varsigma}^{\wp} = \frac{\partial^{\wp}}{\partial \varsigma^{\wp}}$ is (Caputo) fractional operator, $\mathcal{P}_1[\textbf{y}],$ $\mathcal{Q}_1[\textbf{y}]$ are operators, linear and nonlinear, respectively.

Proceeds the YT and using (2.5), we have

$\begin{equation*} Y\left\{D_{\varsigma}^{\wp}\mathbb{U}(\textbf{y},\varsigma)\right\} = Y\left\{\mathcal{P}_1[\textbf{y}]\mathbb{U}(\textbf{y},\varsigma)+\mathcal{Q}_1[\textbf{y}]\mathbb{U}(\textbf{y},\varsigma)\right\}, \end{equation*}$

that is

$\begin{equation*} \frac{1}{u^{\wp}}\left\{M(u)- u \mathbb{U}(0)- u^2\mathbb{U}^{'}(0)\right\} = Y\left\{\mathcal{P}_1[\textbf{y}]\mathbb{U}(\textbf{y},\varsigma)+\mathcal{Q}_1[\textbf{y}]\mathbb{U}(\textbf{y},\varsigma)\right\}. \end{equation*}$

After that, we get

$\begin{equation*} M(\mathbb{U}) = u \mathbb{U}(0)+ u^2 \mathbb{U}^{'}(0)+u^{\wp}Y\{\mathcal{P}_1[\textbf{y}]\mathbb{U}(\textbf{y},\varsigma)+\mathcal{Q}_1[\textbf{y}]\mathbb{U}(\textbf{y},\varsigma)\}. \end{equation*}$

Let us take Yang inverse transform on both sides, we have

$\begin{equation} \mathbb{U}(\textbf{y},\varsigma) = \mathbb{U}(0)+ \mathbb{U}^{'}(0)+Y^{-1}\left\{u^{\wp}Y\left\{\mathcal{P}_1[\textbf{y}]\mathbb{U}(\textbf{y},\varsigma)+\mathcal{Q}_1[\textbf{y}]\mathbb{U}(\textbf{y},\varsigma)\right\}\right\}. \end{equation}$

(3.3)

Applying HPM

$\begin{equation} \mathbb{U}(\textbf{y},\varsigma) = \sum\limits_{k = 0}^{\infty}\epsilon^{k}\mathbb{U}_{k}(\textbf{y},\varsigma), \end{equation}$

(3.4)

with homotopy parameter $\epsilon\in[0, 1]$ .

Ultimately, the nonlinear factors are discarded as:

$\begin{equation} \mathcal{Q}_1[\textbf{y}]\mathbb{U}(\textbf{y},\varsigma) = \sum\limits_{k = 0}^{\infty}\epsilon^{k}H_{n}(\mathbb{U}), \end{equation}$

(3.5)

where $H_{k}(\mathbb{U})$ is He's polynomials

$\begin{equation*} \ H_{n}(\mathbb{U}_{0},\mathbb{U}_{1},\ldots,\mathbb{U}_{n}) = \frac{1}{\Gamma(n+1)}D_{\epsilon}^{k}\left[\mathcal{Q}_1\left(\sum\limits_{k = 0}^{\infty}\epsilon^{i}\mathbb{U}_{i}\right)\right]_{\epsilon = 0}, \end{equation*}$

with $D_{\epsilon}^{k} = \frac{\partial^{k}}{\partial \epsilon^{k}}.$

Utilizing (3.4) and (3.5) in (3.3), we have

$\begin{equation} \sum\limits_{k = 0}^{\infty}\epsilon^{k}\mathbb{U}_{k}(\textbf{y},\varsigma) = \mathbb{U}(0)+ \mathbb{U}^{'}(0)+\epsilon\left(Y^{-1}\left\{u^{\wp}Y\left\{\mathcal{P}_1\sum\limits_{k = 0}^{\infty}\epsilon^{k}\mathbb{U}_{k}(\textbf{y},\varsigma)+\sum\limits_{k = 0}^{\infty}\epsilon^{k}H_{k}(\mathbb{U})\right\}\right\}\right). \end{equation}$

(3.6)

Equating the $\epsilon$ coefficients, we get

$\begin{equation} \begin{split} \epsilon^{0}:\mathbb{U}_{0}(\textbf{y},\varsigma)& = \mathbb{U}(0)+ \mathbb{U}^{'}(0),\\ \epsilon^{1}:\mathbb{U}_{1}(\textbf{y},\varsigma)& = Y^{-1}\left\{u^{\wp}Y\{\mathcal{P}_1[\textbf{y}]\mathbb{U}_{0}(\textbf{y},\varsigma)+H_{0}(\mathbb{U})\}\right\},\\ \epsilon^{2}:\mathbb{U}_{2}(\textbf{y},\varsigma)& = Y^{-1}\left\{u^{\wp}Y\{\mathcal{P}_1[\textbf{y}]\mathbb{U}_{1}(\textbf{y},\varsigma)+H_{1}(\mathbb{U})\}\right\},\\ &\;\;\vdots\\ \epsilon^{k}:\mathbb{U}_{k}(\textbf{y},\varsigma)& = Y^{-1}\left\{u^{\wp}Y\{\mathcal{P}_1[\textbf{y}]\mathbb{U}_{k-1}(\textbf{y},\varsigma)+H_{k-1}(\mathbb{U})\}\right\}, \end{split} \end{equation}$

(3.7)

where $k\in\mathbb{N}.$ At last, the analytical solution is approximated by the series,

$\begin{equation} \ \mathbb{U}(\textbf{y},\varsigma) = \lim\limits_{M\rightarrow \infty}\sum\limits_{k = 1}^{M}\mathbb{U}_{k}(\textbf{y},\varsigma). \end{equation}$

(3.8)

4. Construction of Yang transform decomposition method for solving FDEs

To illustrate the process of solution of the YTDM, we take FDEs of the form to show the general implementation of the proposed method as stated in ^[42,43]

$\begin{equation} D_\varsigma^\wp{\mathbb{U}(\textbf{y},\varsigma)} = \mathcal{P}_1(\textbf{y},\varsigma)+\mathcal{Q}_1(\textbf{y},\varsigma),\quad 1 < \wp \leq 2, \end{equation}$

(4.1)

having initial values

$\begin{equation} \mathbb{U}(\textbf{y},0) = \xi(\textbf{y})\;\;\text{and}\;\;\frac{\partial}{\partial\varsigma}\mathbb{U}(\textbf{y},0) = \zeta(\textbf{y}), \end{equation}$

(4.2)

where $D_\varsigma^\wp = \frac{\partial^\wp}{\partial \varsigma^\wp}$ is (Caputo) fractional operator, $\mathcal{P}_1$ , $\mathcal{Q}_1$ are operators, linear and nonlinear, respectively.

Proceeds the YT and using (2.5), we have

$Y\left\{D_\varsigma^\wp{\mathbb{U}(\textbf{y},\varsigma)}\right\} = Y\left\{\mathcal{P}_1(\textbf{y},\varsigma)+\mathcal{Q}_1(\textbf{y},\varsigma)\right\},$

that is

$\begin{equation*} \frac{1}{u^{\wp}}\left\{M(u)- u \mathbb{U}(0)- u^2\mathbb{U}^{'}(0)\right\} = Y\{\mathcal{P}_1(\textbf{y},\varsigma)+\mathcal{Q}_1(\textbf{y},\varsigma)\}. \end{equation*}$

After that, we get

$\begin{equation*} M(\mathbb{U}) = u \mathbb{U}(0)+ u^2 \mathbb{U}^{'}(0)+u^{\wp}Y\left\{\mathcal{P}_1(\textbf{y},\varsigma)+\mathcal{Q}_1(\textbf{y},\varsigma)\right\}. \end{equation*}$

Let us take Yang inverse transform on both sides, we have

$\begin{equation} \mathbb{U}(\textbf{y},\varsigma) = \mathbb{U}(0)+ \mathbb{U}^{'}(0)+Y^{-1}\left\{u^{\wp}Y\left\{\mathcal{P}_1(\textbf{y},\varsigma)+\mathcal{Q}_1(\textbf{y},\varsigma)\right\}\right\}. \end{equation}$

(4.3)

Now, we assume an infinite series solution as

$\begin{equation} \mathbb{U}(\textbf{y},\varsigma) = \sum\limits_{m = 0}^{\infty}\mathbb{U}_m(\textbf{y},\varsigma), \end{equation}$

(4.4)

and the nonlinear terms $\mathcal{Q}_1$ are calculated by operate the formula

$\begin{equation} \mathcal{Q}_1(\textbf{y},\varsigma) = \sum\limits_{m = 0}^{\infty}\mathcal{A}_m, \end{equation}$

(4.5)

where

$\mathcal{A}_m = \frac{1}{m!}\left[\frac{\partial^m}{\partial\ell^m}\left\{\mathcal{Q}_1\left(\sum\limits_{k = 0}^{\infty}\ell^k\textbf{y}_k,\sum\limits_{k = 0}^{\infty}\ell^k\varsigma_k\right)\right\}\right]_{\ell = 0}.$

Utilizing (4.4) and (4.5) into (4.3), we have

$\begin{equation} \sum\limits_{m = 0}^{\infty}\mathbb{U}_m(\textbf{y},\varsigma) = \mathbb{U}(0)+ \mathbb{U}^{'}(0)+Y^{-1}\left\{ u^\wp Y\left\{\mathcal{P}_1\left(\sum\limits_{m = 0}^{\infty}\textbf{y}_m,\sum\limits_{m = 0}^{\infty}\varsigma_m\right)+\sum\limits_{m = 0}^{\infty}\mathcal{A}_m\right\}\right\}. \end{equation}$

(4.6)

By equating both sides, we have

$\begin{equation*} \begin{split} \mathbb{U}_0(\textbf{y},\varsigma)& = \mathbb{U}(0)+ \varsigma\mathbb{U}^{'}(0),\\ \mathbb{U}_1(\textbf{y},\varsigma)& = Y^{-1}\left\{u^\wp Y^+\{\mathcal{P}_1(\textbf{y}_0,\varsigma_0)+\mathcal{A}_0\}\right\}. \end{split} \end{equation*}$

Continuing in this manner to get the general recursive relation,

$\begin{equation} \mathbb{U}_{m+1}(\textbf{y},\varsigma) = Y^{-1}\left\{u^\wp Y^+\{\mathcal{P}_1(\textbf{y}_m,\varsigma_m)+\mathcal{A}_m\}\right\}. \end{equation}$

(4.7)

5. Examples

In this section, we present some Illustrative examples.

Example 1. Consider the 2-dimensional nonlinear time-fractional wave-like equation with variable coefficients:

$\begin{equation} \frac{\partial ^{\wp }{\mathbb{U}}({\textbf{y}},{\textbf{z}},{\varsigma}) }{\partial \varsigma ^{\wp }} = \frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{z}\textbf{z}}\right)-\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\textbf{y}\textbf{z}\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{z}}\right)-\mathbb{U}, \quad \varsigma > 0,1 < \wp \leq2, \end{equation}$

(5.1)

having initial values

$\begin{equation} {\mathbb{U}}({\textbf{y}},{\textbf{z}},0) = e^{{\textbf{y}}{\textbf{z}}}\;\;\text{and}\;\;\frac{\partial}{\partial\varsigma}\mathbb{U}({\textbf{y}},\textbf{z},0) = e^{{\textbf{y}}{\textbf{z}}},\quad({\textbf{y}},{\textbf{z}})\in\mathbb{R}^{2}. \end{equation}$

(5.2)

Proceeds the YT, we have

$\begin{equation*} \ Y\left\{\frac{\partial^{\wp}\mathbb{U}}{\partial \varsigma^{\wp}}\right\} = Y\left\{\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{z}\textbf{z}}\right)-\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\textbf{y}\textbf{z}\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{z}}\right)-\mathbb{U}\right\}, \end{equation*}$

and using (2.5), we have

$\begin{equation*} \ \frac{1}{u^{\wp}}\left\{M(u)- u \mathbb{U}(0)-u^2\mathbb{U}^{'}(0)\right\} = Y\left\{\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{z}\textbf{z}}\right)-\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\textbf{y}\textbf{z}\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{z}}\right)-\mathbb{U}\right\}, \end{equation*}$

that is

$\begin{equation*} \ M(u) = u\mathbb{U}(0)+u^2\mathbb{U}^{'}(0)+u^{\wp}Y\left\{\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{z}\textbf{z}}\right)-\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\textbf{y}\textbf{z}\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{z}}\right)-\mathbb{U}\right\}. \end{equation*}$

Let us take Yang inverse transform on both sides, we have

$\begin{equation*} \mathbb{U}(\textbf{y},{\textbf{z}},\varsigma) = Y^{-1}\{u\mathbb{U}(0)+u^2\mathbb{U}^{'}(0)\}+Y^{-1}\left\{u^\wp Y\left\{\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{z}\textbf{z}}\right)-\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\textbf{y}\textbf{z}\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{z}}\right)-\mathbb{U}\right\}\right\}, \end{equation*}$

that is

$\begin{equation*} \mathbb{U}(\textbf{y},{\textbf{z}},\varsigma) = (e^{{\textbf{y}}{\textbf{z}}}+e^{{\textbf{y}}{\textbf{z}}}\varsigma)+Y^{-1}\left\{u^\wp Y\left\{\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{z}\textbf{z}}\right)-\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\textbf{y}\textbf{z}\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{z}}\right)-\mathbb{U}\right\}\right\}. \end{equation*}$

Applying HPM

$\begin{align*} \sum\limits_{k = 0}^{\infty}\epsilon^{k}\mathbb{U}_{k}(\textbf{y},{\textbf{z}},\varsigma) = (e^{{\textbf{y}}{\textbf{z}}}+e^{{\textbf{y}}{\textbf{z}}}\varsigma)+\epsilon\left(Y^{-1}\left\{u^{\wp}Y\left\{\left(\sum\limits_{k = 0}^{\infty}\epsilon^{k}H^{1}_{k}(\mathbb{U})\right)-\left(\sum\limits_{k = 0}^{\infty}\epsilon^{k}H^{2}_{k}(\mathbb{U})\right)\\+\left(\sum\limits_{k = 0}^{\infty}\epsilon^{k}\mathbb{U}_{k}(\textbf{y},\varsigma)\right)\right\}\right\}\right). \end{align*}$

Ultimately, the nonlinear factors by He's polynomial $H_{k}(\mathbb{U})$ are given as:

$\sum\limits_{k = 0}^{\infty}\epsilon^{k}H^{1}_{k}(\mathbb{U}) = \frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{z}\textbf{z}}\right),$

and

$\sum\limits_{k = 0}^{\infty}\epsilon^{k}H^{2}_{k}(\mathbb{U}) = \frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\textbf{y}\textbf{z}\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{z}}\right).$

Few components are calculated as:

$\begin{align*} H^{1}_{0}(\mathbb{U})& = \mathbb{U}_{0\textbf{y}\textbf{y}}\mathbb{U}_{0\textbf{z}\textbf{z}},\\ H^{1}_{1}(\mathbb{U})& = \mathbb{U}_{0\textbf{y}\textbf{y}}\mathbb{U}_{1\textbf{z}\textbf{z}}+\mathbb{U}_{1\textbf{y}\textbf{y}}\mathbb{U}_{0\textbf{z}\textbf{z}},\\ &\;\;\vdots\\ H^{2}_{0}(\mathbb{U})& = \textbf{y}\textbf{z}\mathbb{U}_{0\textbf{y}}\mathbb{U}_{0\textbf{z}},\\ H^{2}_{1}(\mathbb{U})& = \textbf{y}\textbf{z}\mathbb{U}_{0\textbf{y}}\mathbb{U}_{1\textbf{z}}+\textbf{y}\textbf{z}\mathbb{U}_{1\textbf{y}}\mathbb{U}_{0\textbf{z}}. \end{align*}$

Equating the $\epsilon$ coefficients, we get

$\begin{align*} \epsilon^{0}:\mathbb{U}_{0}(\textbf{y},{\textbf{z}},\varsigma)& = (e^{{\textbf{y}}{\textbf{z}}}+e^{{\textbf{y}}{\textbf{z}}}\varsigma),\\ \epsilon^{1}:\mathbb{U}_{1}(\textbf{y},{\textbf{z}},\varsigma)& = -\left(\frac{\varsigma^{\wp}}{\Gamma(\wp+1)}+\frac{\varsigma^{\wp+1}}{\Gamma(\wp+2)}\right)e^{{\textbf{y}}{\textbf{z}}},\\ \epsilon^{2}:\mathbb{U}_{2}(\textbf{y},{\textbf{z}},\varsigma)& = \left(\frac{\varsigma^{2\wp}}{\Gamma(2\wp+1)}+\frac{\varsigma^{2\wp+1}}{\Gamma(2\wp+2)}\right)e^{{\textbf{y}}{\textbf{z}}},\\ &\;\;\vdots \end{align*}$

At last, the analytical solution is approximated by the series as:

$\mathbb{U}({\textbf{y}},{\textbf{z}},{\varsigma}) = {\mathbb{U}}_{0}({\textbf{y}},{\textbf{z}},{\varsigma})+{\mathbb{U}}_{1}({\textbf{y}},{\textbf{z}},{\varsigma})+{\mathbb{U}}_{2}({\textbf{y}},{\textbf{z}},{\varsigma})+\cdots,$

that is

$\mathbb{U}(\textbf{y},{\textbf{z}},\varsigma) = \left(1+\varsigma-\frac{\varsigma^{\wp}}{\Gamma(\wp+1)}-\frac{\varsigma^{\wp+1}}{\Gamma(\wp+2)}+\frac{\varsigma^{2\wp}}{\Gamma(2\wp+1)}+\frac{\varsigma^{2\wp+1}}{\Gamma(2\wp+2)}+\cdots\right)e^{{\textbf{y}}{\textbf{z}}}.$

Application of the YTDM

Proceeds the YT, we have

$\begin{equation*} Y\left\{\frac{\partial^{\wp}\mathbb{U}}{\partial \varsigma^{\wp}}\right\} = Y\left\{\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{z}\textbf{z}}\right)-\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\textbf{y}\textbf{z}\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{z}}\right)-\mathbb{U}\right\}, \end{equation*}$

and using (2.5), we have

$\begin{equation*} \frac{1}{u^{\wp}}\left\{M(u)- u \mathbb{U}(0)-u^2\mathbb{U}^{'}(0)\right\} = Y\left\{\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{z}\textbf{z}}\right)-\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\textbf{y}\textbf{z}\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{z}}\right)-\mathbb{U}\right\}, \end{equation*}$

equivalently

$\begin{equation*} M(u) = u \mathbb{U}(0)+u^2\mathbb{U}^{'}(0)+u^{\wp}Y\left\{\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{z}\textbf{z}}\right)-\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\textbf{y}\textbf{z}\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{z}}\right)-\mathbb{U}\right\}. \end{equation*}$

Let us take Yang inverse transform on both sides, we have

$\begin{align*} \mathbb{U}(\textbf{y},{\textbf{z}},\varsigma)& = Y^{-1}\left\{u\mathbb{U}(0)+u^2\mathbb{U}^{'}(0)\right\}+Y^{-1}\left\{u^\wp Y\left\{\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{z}\textbf{z}}\right)-\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\textbf{y}\textbf{z}\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{z}}\right)-\mathbb{U}\right\}\right\}\notag\\ & = (e^{{\textbf{y}}{\textbf{z}}}+e^{{\textbf{y}}{\textbf{z}}}\varsigma)+Y^{-1}\left\{u^\wp Y\left\{\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{z}\textbf{z}}\right)-\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\textbf{y}\textbf{z}\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{z}}\right)-\mathbb{U}\right\}\right\}. \end{align*}$

Now, we assume an infinite series solution as:

$\begin{equation*} \mathbb{U}(\textbf{y},{\textbf{z}},\varsigma) = \sum\limits_{m = 0}^{\infty}\mathbb{U}_m(\textbf{y},{\textbf{z}},\varsigma), \end{equation*}$

where

$\frac{\partial^{2} }{\partial\textbf{y}\partial \textbf{z} }\left(\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{z}\textbf{z}}\right) = \sum\limits_{m = 0}^{\infty}\mathcal{A}_m$

and

$\frac{\partial^{2} }{\partial \textbf{y}\partial \textbf{z} }\left(\textbf{y}\textbf{z}\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{z}}\right) = \sum\limits_{m = 0}^{\infty}\mathcal{B}_m$

are the Adomian polynomials that represents the nonlinear terms and

$\begin{align*} \sum\limits_{m = 0}^{\infty}\mathbb{U}_m(\textbf{y},{\textbf{z}},\varsigma)& = \mathbb{U}(\textbf{y},{\textbf{z}},0)+Y^{-1}\left\{u^\wp Y\left\{\sum\limits_{m = 0}^{\infty}\mathcal{A}_m-\sum\limits_{m = 0}^{\infty}\mathcal{B}_m-\mathbb{U}\right\}\right\}\notag\\ & = (e^{{\textbf{y}}{\textbf{z}}}+e^{{\textbf{y}}{\textbf{z}}}\varsigma)+Y^{-1}\left\{u^\wp Y\left\{\sum\limits_{m = 0}^{\infty}\mathcal{A}_m-\sum\limits_{m = 0}^{\infty}\mathcal{B}_m-\mathbb{U}\right\}\right\}. \end{align*}$

The first few nonlinear terms are as follows:

$\begin{align*} \mathcal{A}_0& = \mathbb{U}_{0\textbf{y}\textbf{y}}\mathbb{U}_{0\textbf{z}\textbf{z}},\\ \mathcal{A}_1& = \textbf{y}\textbf{z}\mathbb{U}_{0\textbf{y}}\mathbb{U}_{0\textbf{z}},\\ &\;\;\vdots\\ \mathcal{B}_0& = \mathbb{U}_{0\textbf{y}\textbf{y}}\mathbb{U}_{1\textbf{z}\textbf{z}}+\mathbb{U}_{1\textbf{y}\textbf{y}}\mathbb{U}_{0\textbf{z}\textbf{z}},\\ \mathcal{B}_1& = \textbf{y}\textbf{z}\mathbb{U}_{0\textbf{y}}\mathbb{U}_{1\textbf{z}}-\textbf{y}\textbf{z}\mathbb{U}_{1\textbf{y}}\mathbb{U}_{0\textbf{z}}. \end{align*}$

By equating both sides, we have

$\begin{equation*} \mathbb{U}_0(\textbf{y},{\textbf{z}},\varsigma) = (e^{{\textbf{y}}{\textbf{z}}}+e^{{\textbf{y}}{\textbf{z}}}\varsigma). \end{equation*}$

For $m = 0$ , we have

$\begin{equation*} \mathbb{U}_1(\textbf{y},{\textbf{z}},\varsigma) = -\left(\frac{\varsigma^{\wp}}{\Gamma(\wp+1)}+\frac{\varsigma^{\wp+1}}{\Gamma(\wp+2)}\right)e^{{\textbf{y}}{\textbf{z}}}, \end{equation*}$

and for $m = 1$ , we have

$\begin{equation*} \mathbb{U}_2(\textbf{y},{\textbf{z}},\varsigma) = \left(\frac{\varsigma^{2\wp}}{\Gamma(2\wp+1)}+\frac{\varsigma^{2\wp+1}}{\Gamma(2\wp+2)}\right)e^{{\textbf{y}}{\textbf{z}}}. \end{equation*}$

The YTDM solution is

$\begin{align*} \mathbb{U}(\textbf{y},{\textbf{z}},\varsigma)& = \sum\limits_{m = 0}^{\infty}\mathbb{U}_m(\textbf{y},{\textbf{z}},\varsigma) = \mathbb{U}_0(\textbf{y},{\textbf{z}},\varsigma)+\mathbb{U}_1(\textbf{y},{\textbf{z}},\varsigma)+\mathbb{U}_2(\textbf{y},{\textbf{z}},\varsigma)+\cdots\\ & = \left(1+\varsigma-\frac{\varsigma^{\wp}}{\Gamma(\wp+1)}-\frac{\varsigma^{\wp+1}}{\Gamma(\wp+2)}+\frac{\varsigma^{2\wp}}{\Gamma(2\wp+1)}+\frac{\varsigma^{2\wp+1}}{\Gamma(2\wp+2)}+\cdots\right)e^{{\textbf{y}}{\textbf{z}}}. \end{align*}$

In the special case $\wp = 2$ , we get exact solution as

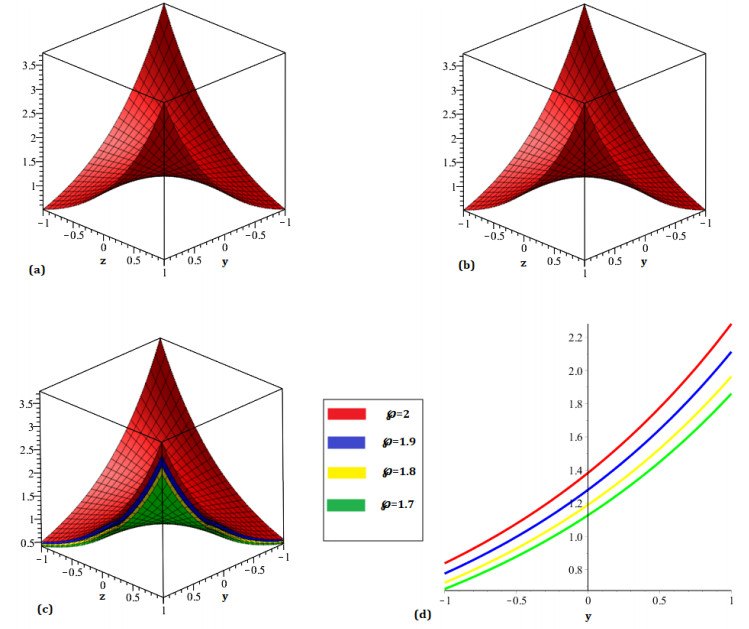
$\begin{equation} \ \mathbb{U}(\textbf{y},{\textbf{z}},\varsigma) = (\cos\varsigma+\sin\varsigma)e^{{\textbf{y}}{\textbf{z}}}. \end{equation}$

(5.3)

The graphs in and show the behavior of the exact and proposed methods solution in Caputo manner at $\wp = 1$ . shows our methods solution at different fractional-orders of $\wp = 2, 1.9, 1.8, 1.7,$ and $-1 \leq\textbf{y}, \textbf{z}\leq1$ for Example 1 and , respectively, at $\varsigma = 0.1$ and $-1 \leq\textbf{y}, \textbf{z}\leq1$ . In Table 1, we computed the absolute errors of the Shehu variational iteration method (SVIM) and suggested methods that confirm that our solution converges quickly compared to SVIM. The graphical representation shows that the exact solution and proposed methods solution are in good agreement.

Figure 1. The nature of

$\mathbb{U}(\textbf{y}, {\textbf{z}}, \varsigma)$ in terms of

$\textbf{y}, \textbf{z}$ and

$\varsigma$ at various values of

$\wp$ for Example 1.

DownLoad: Full-Size Img PowerPoint

Table 1. Comparison of the Shehu variational iteration method (SVIM) and suggested solutions absolute errors at different orders of

$\wp$ for Example 1.

${{\varsigma}}/\textbf{y}, {\textbf{z}}$	$SVIM (\wp=0.5)$	Proposed methods $(\wp=0.5)$	$SVIM (\wp=0.7)$	Proposed methods $(\wp=0.7)$
0.1	3.2196 E-13	2.5111E-14	4.0929E-13	0.01010032E-14
0.3	2.1569 E-09	2.32229E-11	2.7420E-09	0.02040261E-11
0.5	1.3095 E-07	2.0335E-09	1.6647E-07	0.03090871E-09
0.7	1.9680 E-06	1.1448E-07	2.5019E-06	0.04162043E-07
0.9	1.4947 E-05	2.05640176E-06	1.9001E-05	0.05253943E-06

| Show Table

DownLoad: CSV

Example 2. Consider the following nonlinear time-fractional wave-like equation with variable coefficients:

$\begin{equation} \frac{\partial ^{\wp }{\mathbb{U}}({\textbf{y}},{\varsigma}) }{\partial \varsigma ^{\wp }} = \mathbb{U}^{2}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}\textbf{y}}\right)+\mathbb{U}^{2}_{\textbf{y}}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}^{3}_{\textbf{y}\textbf{y}}\right)-18\mathbb{U}^{5}+\mathbb{U} \quad \varsigma > 0,1 < \wp \leq2, \end{equation}$

(5.4)

having initial values

$\begin{equation} {\mathbb{U}}({\textbf{y}},0) = e^{{\textbf{y}}}\;\;\text{and}\;\;\frac{\partial}{\partial\varsigma}\mathbb{U}({\textbf{y}},0) = e^{{\textbf{y}}}. \end{equation}$

(5.5)

Proceeds the YT, we have

$\begin{equation*} \ Y\left\{\frac{\partial^{\wp}\mathbb{U}}{\partial \varsigma^{\wp}}\right\} = Y\left\{\mathbb{U}^{2}\frac{\partial^{2} }{\partial \textbf{y}^{2} }(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}\textbf{y}})+\mathbb{U}^{2}_{\textbf{y}}\frac{\partial^{2} }{\partial \textbf{y}^{2} }(\mathbb{U}^{3}_{\textbf{y}\textbf{y}})-18\mathbb{U}^{5}+\mathbb{U}\right\}, \end{equation*}$

and using (2.5), we have

$\begin{equation*} \ \frac{1}{u^{\wp}}\{M(u)- u \mathbb{U}(0)-u^2\mathbb{U}^{'}(0)\} = Y\left\{\mathbb{U}^{2}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}\textbf{y}}\right)+\mathbb{U}^{2}_{\textbf{y}}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}^{3}_{\textbf{y}\textbf{y}}\right)-18\mathbb{U}^{5}+\mathbb{U}\right\}, \end{equation*}$

that is

$\begin{equation*} M(u) = u\mathbb{U}(0)+u^2\mathbb{U}^{'}(0)+u^{\wp}Y\left\{\mathbb{U}^{2}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}\textbf{y}}\right)+\mathbb{U}^{2}_{\textbf{y}}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}^{3}_{\textbf{y}\textbf{y}}\right)-18\mathbb{U}^{5}+\mathbb{U}\right\}. \end{equation*}$

Let us take Yang inverse transform on both sides, we have

$\begin{align*} \mathbb{U}(\textbf{y},\varsigma)& = Y^{-1}\left\{u\mathbb{U}(0)+u^2\mathbb{U}^{'}(0)\right\}\\ &+Y^{-1}\left\{u^\wp Y\left\{\mathbb{U}^{2}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}\textbf{y}}\right)+\mathbb{U}^{2}_{\textbf{y}}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}^{3}_{\textbf{y}\textbf{y}}\right)-18\mathbb{U}^{5}+\mathbb{U}\right\}\right\}\notag\\ & = (e^{{\textbf{y}}{\textbf{z}}}+e^{{\textbf{y}}{\textbf{z}}}\varsigma)+Y^{-1}\left\{u^\wp Y\left\{\mathbb{U}^{2}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}\textbf{y}}\right)+\mathbb{U}^{2}_{\textbf{y}}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}^{3}_{\textbf{y}\textbf{y}}\right)-18\mathbb{U}^{5}+\mathbb{U}\right\}\right\}. \end{align*}$

By applying HPM, we have

$\begin{align*} \sum\limits_{k = 0}^{\infty}\epsilon^{k}\mathbb{U}_{k}(\textbf{y},\varsigma)& = (e^{{\textbf{y}}}+e^{{\textbf{y}}}\varsigma)+\epsilon\left(Y^{-1}\left\{u^{\wp}Y\left\{\left(\sum\limits_{k = 0}^{\infty}\epsilon^{k}H^{1}_{k}(\mathbb{U})\right)+\left(\sum\limits_{k = 0}^{\infty}\epsilon^{k}H^{2}_{k}(\mathbb{U})\right)\right.\right.\right.\notag\\ &\quad\left.\left.\left.-18\left(\sum\limits_{k = 0}^{\infty}\epsilon^{k}H^{3}_{k}(\mathbb{U})\right)+\left(\sum\limits_{k = 0}^{\infty}\epsilon^{k}\mathbb{U}_{k}(\textbf{y},\varsigma)\right)\right\}\right\}\right). \end{align*}$

Ultimately, the nonlinear factors by He's polynomial $H_{k}(\mathbb{U})$ are given as:

$\begin{equation*} \begin{split} \sum\limits_{k = 0}^{\infty}\epsilon^{k}H^{1}_{k}(\mathbb{U})& = \mathbb{U}^{2}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}\textbf{y}}\right),\\ \sum\limits_{k = 0}^{\infty}\epsilon^{k}H^{2}_{k}(\mathbb{U})& = \mathbb{U}^{2}_{\textbf{y}}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}^{3}_{\textbf{y}\textbf{y}}\right),\\ \sum\limits_{k = 0}^{\infty}\epsilon^{k}H^{3}_{k}(\mathbb{U})& = \mathbb{U}^{5}. \end{split} \end{equation*}$

Few components are calculated as:

$\begin{equation*} \begin{split} H^{1}_{0}(\mathbb{U})& = \mathbb{U}_{0}^{2}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}_{0\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}\textbf{y}}\right),\\ H^{1}_{1}(\mathbb{U})& = 2\mathbb{U}_{0}\mathbb{U}_{1}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}_{0\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}\textbf{y}}\right)+\mathbb{U}_{0}^{2}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}_{1\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}\textbf{y}}+\mathbb{U}_{0\textbf{y}}\mathbb{U}_{1\textbf{y}\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}\textbf{y}}+\mathbb{U}_{0\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}}\mathbb{U}_{1\textbf{y}\textbf{y}\textbf{y}}\right),\\ &\;\;\vdots\\ H^{2}_{0}(\mathbb{U})& = \mathbb{U}^{2}_{0\textbf{y}}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}^{3}_{0\textbf{y}\textbf{y}}\right),\\ H^{2}_{1}(\mathbb{U})& = 2\mathbb{U}_{0\textbf{y}}\mathbb{U}_{1\textbf{y}}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}^{3}_{0\textbf{y}\textbf{y}}\right)+3\mathbb{U}^{2}_{0\textbf{y}}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}^{2}_{0\textbf{y}\textbf{y}}\mathbb{U}_{1\textbf{y}\textbf{y}}\right),\\ &\;\;\vdots\\ H^{3}_{0}(\mathbb{U})& = \mathbb{U}_{0}^{5},\\ H^{3}_{1}(\mathbb{U})& = 5\mathbb{U}_{0}^{4}\mathbb{U}_{1}. \end{split} \end{equation*}$

Equating the $\epsilon$ coefficients, we get

$\begin{align*} \epsilon^{0}:\mathbb{U}_{0}(\textbf{y},\varsigma)& = (e^{{\textbf{y}}}+e^{{\textbf{y}}}\varsigma),\\ \epsilon^{1}:\mathbb{U}_{1}(\textbf{y},\varsigma)& = \left(\frac{\varsigma^{\wp}}{\Gamma(\wp+1)}+\frac{\varsigma^{\wp+1}}{\Gamma(\wp+2)}\right)e^{{\textbf{y}}},\\ \epsilon^{2}:\mathbb{U}_{2}(\textbf{y},\varsigma)& = \left(\frac{\varsigma^{2\wp}}{\Gamma(2\wp+1)}+\frac{\varsigma^{2\wp+1}}{\Gamma(2\wp+2)}\right)e^{{\textbf{y}}},\\ &\;\;\vdots \end{align*}$

At last, the analytical solution is approximated by the series as:

$\begin{align*} \mathbb{U}({\textbf{y}},{\varsigma})& = {\mathbb{U}}_{0}({\textbf{y}},{\varsigma})+{\mathbb{U}}_{1}({\textbf{y}},{\varsigma})+{\mathbb{U}}_{2}({\textbf{y}},{\varsigma})+\cdots\\ & = \left(1+\varsigma+\frac{\varsigma^{\wp}}{\Gamma(\wp+1)}+\frac{\varsigma^{\wp+1}}{\Gamma(\wp+2)}+\frac{\varsigma^{2\wp}}{\Gamma(2\wp+1)}+\frac{\varsigma^{2\wp+1}}{\Gamma(2\wp+2)}+\cdots\right)e^{{\textbf{y}}}. \end{align*}$

Application of the YTDM

Proceeds the YT, we have

$\begin{equation*} Y\left\{\frac{\partial^{\wp}\mathbb{U}}{\partial \varsigma^{\wp}}\right\} = Y\left\{\mathbb{U}^{2}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}\textbf{y}}\right)+\mathbb{U}^{2}_{\textbf{y}}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}^{3}_{\textbf{y}\textbf{y}}\right)-18\mathbb{U}^{5}+\mathbb{U}\right\}, \end{equation*}$

and using (2.5), we have

$\begin{equation*} \frac{1}{u^{\wp}}\left\{M(u)- u \mathbb{U}(0)-u^2\mathbb{U}^{'}(0)\right\} = Y\left\{\mathbb{U}^{2}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}\textbf{y}}\right)+\mathbb{U}^{2}_{\textbf{y}}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}^{3}_{\textbf{y}\textbf{y}}\right)-18\mathbb{U}^{5}+\mathbb{U}\right\}, \end{equation*}$

that is

$\begin{equation*} M(u) = u \mathbb{U}(0)+u^2\mathbb{U}^{'}(0)+u^{\wp}Y\left\{\mathbb{U}^{2}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}\textbf{y}}\right)+\mathbb{U}^{2}_{\textbf{y}}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}^{3}_{\textbf{y}\textbf{y}}\right)-18\mathbb{U}^{5}+\mathbb{U}\right\}. \end{equation*}$

Let us take Yang inverse transform on both sides, we have

$\begin{align*} \mathbb{U}(\textbf{y},{\textbf{z}},\varsigma)& = Y^{-1}\left\{u\mathbb{U}(0)+u^2\mathbb{U}^{'}(0)\right\}\\ &+Y^{-1}\left\{u^\wp Y\left\{\mathbb{U}^{2}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}\textbf{y}}\right)+\mathbb{U}^{2}_{\textbf{y}}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}^{3}_{\textbf{y}\textbf{y}}\right)-18\mathbb{U}^{5}+\mathbb{U}\right\}\right\}\\ & = \left(e^{{\textbf{y}}}+e^{{\textbf{y}}}\varsigma\right)+Y^{-1}\left\{u^\wp Y\left\{\mathbb{U}^{2}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}\textbf{y}}\right)+\mathbb{U}^{2}_{\textbf{y}}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}^{3}_{\textbf{y}\textbf{y}}\right)-18\mathbb{U}^{5}+\mathbb{U}\right\}\right\}. \end{align*}$

Now, we assume an infinite series solution as:

$\begin{equation} \mathbb{U}(\textbf{y},{\textbf{z}},\varsigma) = \sum\limits_{m = 0}^{\infty}\mathbb{U}_m(\textbf{y},{\textbf{z}},\varsigma), \end{equation}$

(5.6)

where

$\begin{align*} \mathbb{U}^{2}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}\textbf{y}}\right)& = \sum\limits_{m = 0}^{\infty}\mathcal{A}_m,\\ \mathbb{U}^{2}_{\textbf{y}}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}^{3}_{\textbf{y}\textbf{y}}\right)& = \sum\limits_{m = 0}^{\infty}\mathcal{B}_m \end{align*}$

and

$\mathbb{U}^{5} = \sum\limits_{m = 0}^{\infty}\mathcal{C}_m$

are the Adomian polynomials that represents the nonlinear terms and

$\begin{align*} \sum\limits_{m = 0}^{\infty}\mathbb{U}_m(\textbf{y},{\textbf{z}},\varsigma)& = \mathbb{U}(\textbf{y},{\textbf{z}},0)+Y^{-1}\left\{u^\wp Y\left\{\sum\limits_{m = 0}^{\infty}\mathcal{A}_m+\sum\limits_{m = 0}^{\infty}\mathcal{B}_m-18\sum\limits_{m = 0}^{\infty}\mathcal{C}_m+\mathbb{U}\right\}\right\}\\ & = (e^{{\textbf{y}}}+e^{{\textbf{y}}}\varsigma)+Y^{-1}\left\{u^\wp Y\left\{\sum\limits_{m = 0}^{\infty}\mathcal{A}_m+\sum\limits_{m = 0}^{\infty}\mathcal{B}_m-18\sum\limits_{m = 0}^{\infty}\mathcal{C}_m+\mathbb{U}\right\}\right\}. \end{align*}$

The first few nonlinear terms are as follows:

$\begin{align*} \mathcal{A}_0& = \mathbb{U}_{0}^{2}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}_{0\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}\textbf{y}}\right),\\ \mathcal{A}_1& = 2\mathbb{U}_{0}\mathbb{U}_{1}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}_{0\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}\textbf{y}}\right)+\mathbb{U}_{0}^{2}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}_{1\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}\textbf{y}}+\mathbb{U}_{0\textbf{y}}\mathbb{U}_{1\textbf{y}\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}\textbf{y}}+\mathbb{U}_{0\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}}\mathbb{U}_{1\textbf{y}\textbf{y}\textbf{y}}\right),\\ &\;\;\vdots\\ \mathcal{B}_0& = \mathbb{U}^{2}_{0\textbf{y}}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}^{3}_{0\textbf{y}\textbf{y}}\right),\\ \mathcal{B}_1& = 2\mathbb{U}_{0\textbf{y}}\mathbb{U}_{1\textbf{y}}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}^{3}_{0\textbf{y}\textbf{y}}\right)+3\mathbb{U}^{2}_{0\textbf{y}}\frac{\partial^{2} }{\partial \textbf{y}^{2} }\left(\mathbb{U}^{2}_{0\textbf{y}\textbf{y}}\mathbb{U}_{1\textbf{y}\textbf{y}}\right),\\ &\;\;\vdots\\ \mathcal{C}_0& = \mathbb{U}_{0}^{5},\\ \mathcal{C}_1& = 5\mathbb{U}_{0}^{4}\mathbb{U}_{1}. \end{align*}$

By equating both sides, we have

$\begin{equation*} \mathbb{U}_0(\textbf{y},\varsigma) = (e^{{\textbf{y}}}+e^{{\textbf{y}}}\varsigma). \end{equation*}$

For $m = 0$ , we have

$\begin{equation*} \mathbb{U}_1(\textbf{y},\varsigma) = \left(\frac{\varsigma^{\wp}}{\Gamma(\wp+1)}+\frac{\varsigma^{\wp+1}}{\Gamma(\wp+2)}\right)e^{{\textbf{y}}}, \end{equation*}$

and for $m = 1$ , we have

$\begin{equation*} \mathbb{U}_2(\textbf{y},\varsigma) = \left(\frac{\varsigma^{2\wp}}{\Gamma(2\wp+1)}+\frac{\varsigma^{2\wp+1}}{\Gamma(2\wp+2)}\right)e^{{\textbf{y}}}. \end{equation*}$

The YTDM solution is

$\begin{align*} \mathbb{U}(\textbf{y},\varsigma) = \sum\limits_{m = 0}^{\infty}\mathbb{U}_m(\textbf{y},\varsigma)& = \mathbb{U}_0(\textbf{y},\varsigma)+\mathbb{U}_1(\textbf{y},\varsigma)+\mathbb{U}_2(\textbf{y},\varsigma)+\cdots\\ & = \left(1+\varsigma+\frac{\varsigma^{\wp}}{\Gamma(\wp+1)}+\frac{\varsigma^{\wp+1}}{\Gamma(\wp+2)}+\frac{\varsigma^{2\wp}}{\Gamma(2\wp+1)}+\frac{\varsigma^{2\wp+1}}{\Gamma(2\wp+2)}+\cdots\right)e^{{\textbf{y}}}. \end{align*}$

In the special case $\wp = 2$ , we get exact solution as:

$\begin{equation} \mathbb{U}(\textbf{y},\varsigma) = e^{{\textbf{y}}+\varsigma}. \end{equation}$

(5.7)

Figure 2. The nature of

$\mathbb{U}(\textbf{y}, \varsigma)$ in terms of

$\textbf{y}$ and

$\varsigma$ at various values of

$\wp$ for Example 2.

DownLoad: Full-Size Img PowerPoint

Example 3. Consider the following nonlinear time-fractional wave-like equation with variable coefficients:

$\begin{equation} \frac{\partial ^{\wp }{\mathbb{U}}({\textbf{y}},{\varsigma}) }{\partial \varsigma ^{\wp }} = {\textbf{y}}^{2}\frac{\partial }{\partial \textbf{y} }\left(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\right)-{\textbf{y}}^{2}\left(\mathbb{U}_{\textbf{y}\textbf{y}}\right)^{2}-\mathbb{U}, \quad \varsigma > 0,1 < \wp \leq2, \end{equation}$

(5.8)

having initial values

$\begin{equation} {\mathbb{U}}({\textbf{y}},0) = 0\;\;\text{and}\;\;\frac{\partial}{\partial\varsigma}\mathbb{U}({\textbf{y}},0) = {\textbf{y}}^{2}. \end{equation}$

(5.9)

Proceeds the YT, we have

$\begin{equation*} \ Y\left\{\frac{\partial^{\wp}\mathbb{U}}{\partial \varsigma^{\wp}}\right\} = Y\left\{{\textbf{y}}^{2}\frac{\partial }{\partial \textbf{y} }(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}})-{\textbf{y}}^{2}(\mathbb{U}_{\textbf{y}\textbf{y}})^{2}-\mathbb{U}\right\}, \end{equation*}$

and using (2.5), we have

$\begin{equation*} \frac{1}{u^{\wp}}\left\{M(u)- u \mathbb{U}(0)-u^2\mathbb{U}^{'}(0)\right\} = Y\left\{{\textbf{y}}^{2}\frac{\partial }{\partial \textbf{y} }\left(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\right)-{\textbf{y}}^{2}\left(\mathbb{U}_{\textbf{y}\textbf{y}}\right)^{2}-\mathbb{U}\right\}, \end{equation*}$

that is

$\begin{equation*} M(u) = u\mathbb{U}(0)+u^2\mathbb{U}^{'}(0)+u^{\wp}Y\left\{{\textbf{y}}^{2}\frac{\partial }{\partial \textbf{y} }\left(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\right)-{\textbf{y}}^{2}\left(\mathbb{U}_{\textbf{y}\textbf{y}}\right)^{2}-\mathbb{U}\right\}. \end{equation*}$

Let us take Yang inverse transform on both sides, we have

$\begin{align*} \mathbb{U}(\textbf{y},\varsigma)& = Y^{-1}\left\{u\mathbb{U}(0)+u^2\mathbb{U}^{'}(0)\right\}+Y^{-1}\left\{u^\wp Y\left\{{\textbf{y}}^{2}\frac{\partial }{\partial \textbf{y} }\left(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\right)-{\textbf{y}}^{2}\left(\mathbb{U}_{\textbf{y}\textbf{y}}\right)^{2}-\mathbb{U}\right\}\right\}\\ & = (e^{{\textbf{y}}}\varsigma)+Y^{-1}\left\{u^\wp Y\left\{{\textbf{y}}^{2}\frac{\partial }{\partial \textbf{y} }\left(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\right)-{\textbf{y}}^{2}\left(\mathbb{U}_{\textbf{y}\textbf{y}}\right)^{2}-\mathbb{U}\right\}\right\}. \end{align*}$

By applying HPM, we obtain

$\begin{equation*} \sum\limits_{k = 0}^{\infty}\epsilon^{k}\mathbb{U}_{k}(\textbf{y},\varsigma) = e^{{\textbf{y}}}\varsigma+\epsilon\left(Y^{-1}\left\{u^{\wp}Y\left\{\left(\sum\limits_{k = 0}^{\infty}\epsilon^{k}H^{1}_{k}(\mathbb{U})\right)-\left(\sum\limits_{k = 0}^{\infty}\epsilon^{k}H^{2}_{k}(\mathbb{U})\right)-\left(\sum\limits_{k = 0}^{\infty}\epsilon^{k}\mathbb{U}_{k}(\textbf{y},\varsigma)\right)\right\}\right\}\right). \end{equation*}$

Ultimately, the nonlinear factors by He's polynomial $H_{k}(\mathbb{U})$ are given as

$\begin{equation*} \begin{split} \sum\limits_{k = 0}^{\infty}\epsilon^{k}H^{1}_{k}(\mathbb{U})& = {\textbf{y}}^{2}\frac{\partial }{\partial \textbf{y} }\left(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\right),\\ \sum\limits_{k = 0}^{\infty}\epsilon^{k}H^{2}_{k}(\mathbb{U})& = {\textbf{y}}^{2}\left(\mathbb{U}_{\textbf{y}\textbf{y}}\right)^{2}. \end{split} \end{equation*}$

Few components are calculated as:

$\begin{align*} H^{1}_{0}(\mathbb{U})& = \mathbb{U}_{0\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}},\\ H^{1}_{1}(\mathbb{U})& = \mathbb{U}_{0\textbf{y}}\mathbb{U}_{1\textbf{y}\textbf{y}}+\mathbb{U}_{1\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}},\\ H^{1}_{2}(\mathbb{U})& = \mathbb{U}_{0\textbf{y}}\mathbb{U}_{2\textbf{y}\textbf{y}}+\mathbb{U}_{1\textbf{y}}\mathbb{U}_{1\textbf{y}\textbf{y}}+\mathbb{U}_{2\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}},\\ &\;\;\vdots\\ H^{2}_{0}(\mathbb{U})& = \mathbb{U}^{2}_{0\textbf{y}\textbf{y}},\\ H^{2}_{1}(\mathbb{U})& = 2\mathbb{U}_{0\textbf{y}\textbf{y}}\mathbb{U}_{1\textbf{y}\textbf{y}},\\ H^{2}_{2}(\mathbb{U})& = 2\mathbb{U}_{0\textbf{y}\textbf{y}}\mathbb{U}_{2\textbf{y}\textbf{y}}+\mathbb{U}^{2}_{1\textbf{y}\textbf{y}}. \end{align*}$

Equating the $\epsilon$ coefficients, we get

$\begin{align*} \epsilon^{0}:\mathbb{U}_{0}(\textbf{y},\varsigma)& = {\textbf{y}}^{2}\varsigma,\\ \epsilon^{1}:\mathbb{U}_{1}(\textbf{y},\varsigma)& = -\frac{\varsigma^{\wp+1}}{\Gamma(\wp+2)}{\textbf{y}}^{2},\\ \epsilon^{2}:\mathbb{U}_{2}(\textbf{y},\varsigma)& = \frac{\varsigma^{2\wp+1}}{\Gamma(2\wp+2)}{\textbf{y}}^{2},\\ \epsilon^{3}:\mathbb{U}_{3}(\textbf{y},\varsigma)& = -\frac{\varsigma^{3\wp+1}}{\Gamma(3\wp+2)}{\textbf{y}}^{2},\\ &\;\;\vdots \end{align*}$

At last, the analytical solution is approximated by the series as:

$\begin{align*} \mathbb{U}({\textbf{y}},{\varsigma})& = {\mathbb{U}}_{0}({\textbf{y}},{\varsigma})+{\mathbb{U}}_{1}({\textbf{y}},{\varsigma})+{\mathbb{U}}_{2}({\textbf{y}},{\varsigma})+\cdots\\ & = {\textbf{y}}^{2}\left(\varsigma-\frac{\varsigma^{\wp+1}}{\Gamma(\wp+2)}+\frac{\varsigma^{2\wp+1}}{\Gamma(2\wp+2)}-\frac{\varsigma^{3\wp+1}}{\Gamma(3\wp+2)}+\cdots\right). \end{align*}$

Application of the YTDM

Proceeds the YT, we have

$\begin{equation*} Y\left\{\frac{\partial^{\wp}\mathbb{U}}{\partial \varsigma^{\wp}}\right\} = Y\left\{{\textbf{y}}^{2}\frac{\partial }{\partial \textbf{y} }\left(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\right)-{\textbf{y}}^{2}\left(\mathbb{U}_{\textbf{y}\textbf{y}}\right)^{2}-\mathbb{U}\right\}, \end{equation*}$

and using the Def.(2.5), we have

that is

$\begin{equation*} M(u) = u \mathbb{U}(0)+u^2\mathbb{U}^{'}(0)+u^{\wp}Y\left\{{\textbf{y}}^{2}\frac{\partial }{\partial \textbf{y} }\left(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\right)-{\textbf{y}}^{2}\left(\mathbb{U}_{\textbf{y}\textbf{y}}\right)^{2}-\mathbb{U}\right\}. \end{equation*}$

Let us take Yang inverse transform on both sides, we have

$\begin{align*} \mathbb{U}(\textbf{y},\varsigma)& = Y^{-1}\left\{u\mathbb{U}(0)+u^2\mathbb{U}^{'}(0)]\right\}+Y^{-1}\left\{u^\wp Y\left\{{\textbf{y}}^{2}\frac{\partial }{\partial \textbf{y} }\left(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}}\right)-{\textbf{y}}^{2}\left(\mathbb{U}_{\textbf{y}\textbf{y}}\right)^{2}-\mathbb{U}\right\}\right\},\\ & = {\textbf{y}}^{2}\varsigma+Y^{-1}\left\{u^\wp Y\left\{{\textbf{y}}^{2}\frac{\partial }{\partial \textbf{y} }(\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}})-{\textbf{y}}^{2}(\mathbb{U}_{\textbf{y}\textbf{y}})^{2}-\mathbb{U}\right\}\right\}. \end{align*}$

Now, we assume an infinite series solution as

$\begin{equation*} \ \mathbb{U}(\textbf{y},\varsigma) = \sum\limits_{m = 0}^{\infty}\mathbb{U}_m(\textbf{y},\varsigma), \end{equation*}$

where

$\mathbb{U}_{\textbf{y}}\mathbb{U}_{\textbf{y}\textbf{y}} = \sum\limits_{m = 0}^{\infty}\mathcal{A}_m$

and

$\left(\mathbb{U}_{\textbf{y}\textbf{y}}\right)^{2} = \sum\limits_{m = 0}^{\infty}\mathcal{B}_m$

are the Adomian polynomials that represents the nonlinear terms and

$\begin{align*} \sum\limits_{m = 0}^{\infty}\mathbb{U}_m(\textbf{y},\varsigma)& = \mathbb{U}(\textbf{y},{\textbf{z}},0)+Y^{-1}\left\{u^\wp Y\left\{\sum\limits_{m = 0}^{\infty}\mathcal{A}_m-\sum\limits_{m = 0}^{\infty}\mathcal{B}_m-\mathbb{U}\right\}\right\}\\ & = {\textbf{y}}^{2}\varsigma+Y^{-1}\left\{u^\wp Y\left\{\sum\limits_{m = 0}^{\infty}\mathcal{A}_m-\sum\limits_{m = 0}^{\infty}\mathcal{B}_m-\mathbb{U}\right\}\right\}. \end{align*}$

The first few nonlinear terms are as follows:

$\begin{align*} \mathcal{A}_0& = \mathbb{U}_{0\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}},\\ \mathcal{A}_1& = \mathbb{U}_{0\textbf{y}}\mathbb{U}_{1\textbf{y}\textbf{y}}+ \mathbb{U}_{1\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}},\\ \mathcal{A}_2& = \mathbb{U}_{0\textbf{y}}\mathbb{U}_{2\textbf{y}\textbf{y}}+ \mathbb{U}_{1\textbf{y}}\mathbb{U}_{1\textbf{y}\textbf{y}}+\mathbb{U}_{2\textbf{y}}\mathbb{U}_{0\textbf{y}\textbf{y}},\\ &\;\;\vdots\\ \mathcal{B}_0& = \mathbb{U}^{2}_{0\textbf{y}\textbf{y}},\\ \mathcal{B}_1& = 2\mathbb{U}_{0\textbf{y}\textbf{y}}\mathbb{U}_{1\textbf{y}\textbf{y}},\\ \mathcal{B}_2& = 2\mathbb{U}_{0\textbf{y}\textbf{y}}\mathbb{U}_{2\textbf{y}\textbf{y}}+\mathbb{U}^{2}_{1\textbf{y}\textbf{y}}. \end{align*}$

By equating both sides, we have

$\begin{equation*} \mathbb{U}_0(\textbf{y},\varsigma) = {\textbf{y}}^{2}\varsigma. \end{equation*}$

For $m = 0$ , we have

$\begin{equation*} \mathbb{U}_1(\textbf{y},\varsigma) = -\frac{\varsigma^{\wp+1}}{\Gamma(\wp+2)}{\textbf{y}}^{2}, \end{equation*}$

for $m = 1$ , we have

$\begin{equation*} \mathbb{U}_2(\textbf{y},\varsigma) = \frac{\varsigma^{2\wp+1}}{\Gamma(2\wp+2)}{\textbf{y}}^{2}, \end{equation*}$

and for $m = 2$ , we have

$\begin{equation*} \mathbb{U}_3(\textbf{y},\varsigma) = -\frac{\varsigma^{3\wp+1}}{\Gamma(3\wp+2)}{\textbf{y}}^{2}. \end{equation*}$

The YTDM solution is

$\begin{align*} \mathbb{U}(\textbf{y},\varsigma)& = \sum\limits_{m = 0}^{\infty}\mathbb{U}_m(\textbf{y},\varsigma) = \mathbb{U}_0(\textbf{y},\varsigma)+\mathbb{U}_1(\textbf{y},\varsigma)+\mathbb{U}_2(\textbf{y},\varsigma)+\mathbb{U}_3(\textbf{y},\varsigma)+\cdots\\ & = {\textbf{y}}^{2}\left(\varsigma-\frac{\varsigma^{\wp+1}}{\Gamma(\wp+2)}+\frac{\varsigma^{2\wp+1}}{\Gamma(2\wp+2)}-\frac{\varsigma^{3\wp+1}}{\Gamma(3\wp+2)}+\cdots\right). \end{align*}$

In the special case $\wp = 2$ , we get exact solution as

$\begin{equation} \mathbb{U}(\textbf{y},\varsigma) = {\textbf{y}}^{2}\sin\varsigma. \end{equation}$

(5.10)

The graphs in and show the behavior of the exact and proposed methods solution in Caputo manner at $\wp = 1$ . shows our methods solution at different fractional-orders of $\wp = 2, 1.9, 1.8, 1.7,$ and $0 \leq\textbf{y}, \varsigma\leq3$ for Example 3 and , respectively, at $\varsigma = 0.5$ and $0 \leq\textbf{y}\leq3$ . The analysis reveals that the fractional-order solutions are strongly convergent to the integer-order solution. The convergence of the solution can be observed in the 2-D and 3-D graphs, respectively.

Figure 3. The nature of

$\mathbb{U}(\textbf{y}, \varsigma)$ in terms of

$\textbf{y}$ and

$\varsigma$ at various values of

$\wp$ for Example 3.

DownLoad: Full-Size Img PowerPoint

6. Conclusions

This article compares two approaches to tackling nonlinear Caputo time-fractional wave-like problems. Three numerical examples are used to examine the accuracy and effectiveness of the suggested method. This research used the Yang transform decomposition method and the Homotopy perturbation transform method to give a new representation of exact solutions for nonlinear time-fractional wave-like equations with variable coefficients. In three numerical cases, the techniques were used. In the numerical cases, our methods provided us with the results as infinite series, and when this series is in closed form, it provides the exact results to the related equations. In comparison to other analytical and numerical techniques, the current methods have proven to be an effective and simple procedure. Furthermore, the proposed methods needed fewer calculations and can thus be used to solve other fractional-order problems.

Conflict of interest

The authors declare no conflicts of interest.

References

[1]	K. S. Miller, B. Ross, An introduction to the fractional calculus and fractional differential equations, New York: Wiley, 1993.
[2]	P. Sunthrayuth, R. Ullah, A. Khan, R. Shah, J. Kafle, I. Mahariq, et al., Numerical analysis of the fractional-order nonlinear system of Volterra integro-differential equations, J. Funct. Space, 2021 (2021). https://doi.org/10.1155/2021/1537958
[3]	J. Cheng, H. Zhang, W. Zhang, H. Zhang, Quasi-projective synchronization for Caputo type fractional-order complex-valued neural networks with mixed delays, Int. J. Control Autom. Syst., 20 (2022), 1723–1734. https://doi.org/10.1007/s12555-021-0392-6 doi: 10.1007/s12555-021-0392-6
[4]	M. Areshi, A. Khan, K. Nonlaopon, Analytical investigation of fractional-order Newell-Whitehead-Segel equations via a novel transform, AIMS Mathematics, 7 (2022), 6936–6958. https://doi.org/10.3934/math.2022385 doi: 10.3934/math.2022385
[5]	S. Alyobi, R. Shah, A. Khan, N. A. Shah, K. Nonlaopon, Fractional analysis of nonlinear boussinesq equation under Atangana-Baleanu-Caputo operator, Symmetry, 14 (2022) 2417. https://doi.org/10.3390/sym14112417
[6]	H. Zhang, Y. Cheng, H. Zhang, W. Zhang, J. Cao, Hybrid control design for Mittag-Leffler projective synchronization on FOQVNNs with multiple mixed delays and impulsive effects, Math. Comput. Simulat., 197 (2022), 341–357. https://doi.org/10.1016/j.matcom.2022.02.022 doi: 10.1016/j.matcom.2022.02.022
[7]	S. Rashid, S. Sultana, Y. Karaca, A. Khalid, Y. M. Chu, Some further extensions considering discrete proportional fractional operators, Fractals, 30 (2022), 2240026. https://doi.org/10.1142/s0218348x22400266 doi: 10.1142/s0218348x22400266
[8]	S. N. Hajiseyedazizi, M. E. Samei, J. Alzabut, Y. M. Chu, On multi-step methods for singular fractional q-integro-differential equations, Open Math., 19 (2021), 1378–1405. https://doi.org/10.1515/math-2021-0093 doi: 10.1515/math-2021-0093
[9]	Y. Cheng, H. Zhang, W. Zhang, H. Zhang, Novel algebraic criteria on global Mittag-Leffler synchronization for FOINNs with the Caputo derivative and delay, J. Appl. Math. Comput., 68 (2022), 3527–3544. https://doi.org/10.1007/s12190-021-01672-0 doi: 10.1007/s12190-021-01672-0
[10]	M. M. Al-Sawalha, K. Nonlaopon, I. Khan, O. Y. Ababneh, Fractional evaluation of Kaup-Kupershmidt equation with the exponential-decay kernel, AIMS Mathematics, 8 (2023), 3730–3746. https://doi.org/10.3934/math.2023186 doi: 10.3934/math.2023186
[11]	M. M. Al-Sawalha, O. Y. Ababneh, K. Nonlaopon, Numerical analysis of fractional-order Whitham-Broer-Kaup equations with non-singular kernel operators, AIMS Mathematics, 8 (2023), 2308–2336. https://doi.org/10.3934/math.2023120 doi: 10.3934/math.2023120
[12]	S. Mukhtar, R. Shah, S. Noor, The numerical investigation of a fractional-order multi-dimensional model of Navier-Stokes equation via novel techniques, Symmetry, 14 (2022), 1102. https://doi.org/10.3390/sym14061102 doi: 10.3390/sym14061102
[13]	L. Liu, L. Zhang, G. Pan, S. Zhang, Robust yaw control of autonomous underwater vehicle based on fractional-order PID controller, Ocean Eng., 257 (2022). https://doi.org/10.1016/j.oceaneng.2022.111493
[14]	H. Y. Jin, Z. A. Wang, Global stabilization of the full attraction-repulsion Keller-Segel system, Discrete Cont. Dyn. A, 40 (2020), 3509–3527. https://doi.org/10.3934/dcds.2020027 doi: 10.3934/dcds.2020027
[15]	P. Liu, J. Shi, Z. A. Wang, Pattern formation of the attraction-repulsion Keller-Segel system, Discrete Cont. Dyn. B, 18 (2013), 2597–2625. https://doi.org/ 10.3934/dcdsb.2013.18.2597 doi: 10.3934/dcdsb.2013.18.2597
[16]	H. Jin, Z. Wang, L. Wu, Global dynamics of a three-species spatial food chain model, J. Differ. Equations, 333 (2022), 144–183. https://doi.org/10.1016/j.jde.2022.06.007 doi: 10.1016/j.jde.2022.06.007
[17]	K. Liu, Z. Yang, W. Wei, B. Gao, D. Xin, C. Sun, et al., Novel detection approach for thermal defects: Study on its feasibility and application to vehicle cables, High Volt., 2022, 1–10. https://doi.org/10.1049/hve2.12258
[18]	D. Das, P. C. Ray, R. K. Bera, Solution of Riccati type nonlinear fractional differential equation by homotopy analysis method, Int. J. Sci. Res. Educ., 2016. https://doi.org/10.18535/ijsre/v4i06.15
[19]	V. S. Erturk, S. Momani, Solving systems of fractional differential equations using differential transform method, J. Comput. Appl. Math., 215 (2008) 142–151. https://doi.org/10.1016/j.cam.2007.03.029
[20]	A. M. Zidan, A. Khan, M. K.Alaoui, W. Weera, Evaluation of time-fractional fishers equations with the help of analytical methods, AIMS Mathematics, 7 (2022), 18746–18766. https://doi.org/10.3934/math.20221031 doi: 10.3934/math.20221031
[21]	F. B. M. Belgacem, V. Gulati, P. Goswami, A. Aljoujiee, A treatment of generalized fractional differential equations: Sumudu transform series expansion solutions and applications, Fract. Dynam., 2022,369. https://doi.org/10.1515/9783110472097-023
[22]	N. Shah, Y. Hamed, K. Abualnaja, J. Chung, A. Khan, A comparative analysis of fractional-order Kaup-Kupershmidt equation within different operators, Symmetry, 14 (2022), 986. https://doi.org/10.3390/sym14050986 doi: 10.3390/sym14050986
[23]	J. B. Yindoula, Exact solution of some linear and nonlinear partial differential equations by Laplace-Adomian method and SBA method, Adv. Differ. Equ. Contr., 2021,141–163. https://doi.org/10.17654/de025020141
[24]	A. E. Puhpam, S. K. Lydia, Mahgoub transform method for solving linear fractional differential equations, Int. J. Math. Trends Technol., 58 (2018), 253–257. https://doi.org/10.14445/22315373/ijmtt-v58p535 doi: 10.14445/22315373/ijmtt-v58p535
[25]	M. M. Al-Sawalha, A. Khan, O. Y. Ababneh, T. Botmart, Fractional view analysis of Kersten-Krasil'shchik coupled KdV-mKdV systems with non-singular kernel derivatives, AIMS Mathematics, 7 (2022), 18334–18359. https://doi.org/10.3934/math.20221010 doi: 10.3934/math.20221010
[26]	H. M. Srivastava, R. Shah, H. Khan, M Arif, Some analytical and numerical investigation of a family of fractional-order Helmholtz equations in two space dimensions, Math. Method Appl. Sci., 43 (2020), 199–212. https://doi.org/10.1002/mma.5846 doi: 10.1002/mma.5846
[27]	K. Nonlaopon, A. M. Alsharif, A. M. Zidan, A. Khan, Y. S. Hamed, R. Shah, Numerical investigation of fractional-order Swift-Hohenberg equations via a Novel transform, Symmetry, 13 (2021), 1263. https://doi.org/10.3390/sym13071263 doi: 10.3390/sym13071263
[28]	N. Shah, E. El-Zahar, A. Akgül, A. Khan, J. Kafle, Analysis of fractional-order regularized long-wave models via a novel transform, J. Funct. Space, 2022 (2022), 2754507. https://doi.org/10.1155/2022/2754507 doi: 10.1155/2022/2754507
[29]	M. Purohit, S. Mushtaq, Applications of Laplace-Adomian decomposition method for solving time-fractional advection dispersion equation, J. Math. Comput. Sci., 10 (2020), 1960–1968. https://doi.org/10.28919/jmcs/4798 doi: 10.28919/jmcs/4798
[30]	S. K. Lydia, Solving a system of nonlinear fractional differential equations using Mahgoub Adomian decomposition method, Bull. Pure Appl. Sci. Math. Stat., 38 (2019), 396–404. https://doi.org/10.5958/2320-3226.2019.00043.2 doi: 10.5958/2320-3226.2019.00043.2
[31]	T. Botmart, R. P. Agarwal, M. Naeem, A. Khan, R. Shah, On the solution of fractional modified Boussinesq and approximate long wave equations with non-singular kernel operators, AIMS Mathematics, 7 (2022), 12483–12513. https://doi.org/10.3934/math.2022693 doi: 10.3934/math.2022693
[32]	M. Kbiri Alaoui, K. Nonlaopon, A. M. Zidan, A. Khan, R. Shah, Analytical investigation of fractional-order cahn-hilliard and gardner equations using two novel techniques, Mathematics, 10 (2022), 1643. https://doi.org/10.3390/math10101643 doi: 10.3390/math10101643
[33]	R. Ye, P. Liu, K. Shi, B. Yan, State damping control: A novel simple method of rotor UAV with high performance, IEEE Access, 8 (2020), 214346–214357. https://doi.org/10.1109/ACCESS.2020.3040779 doi: 10.1109/ACCESS.2020.3040779
[34]	Z. Shao, Q. Zhai, Z. Han, X. Guan, A linear AC unit commitment formulation: An application of data-driven linear power flow model, Int. J. Elec. Power, 145 (2023), 108673. https://doi.org/10.1016/j.ijepes.2022.108673 doi: 10.1016/j.ijepes.2022.108673
[35]	V. N. Kovalnogov, R. V. Fedorov, D. A. Generalov, E. V. Tsvetova, T. E. Simos, C. Tsitouras, On a new family of Runge-Kutta-Nystrom pairs of orders 6(4), Mathematics, 10 (2022), 875. https://doi.org/10.3390/math10060875 doi: 10.3390/math10060875
[36]	G. Adomian, R. Rach, Inversion of nonlinear stochastic operators, J. Math. Anal. Appl., 91 (1983) 39–46. https://doi.org/10.1016/0022-247x(83)90090-2
[37]	K. Nonlaopon, M. Naeem, A. M. Zidan, A. Alsanad, A. Gumaei, Numerical investigation of the time-fractional Whitham-Broer-Kaup equation involving without singular kernel operators, Complexity, 2021 (2021). https://doi.org/10.1155/2021/7979365
[38]	J. H. He, Homotopy perturbation technique, Comput. Method Appl. M., 178 (1999), 257–262. https://doi.org/10.1016/s0045-7825(99)00018-3 doi: 10.1016/s0045-7825(99)00018-3
[39]	J. H. He, A coupling method of a homotopy technique and a perturbation technique for non-linear problems, Int. J. Nonlin. Mech., 35 (2000), 37–43. https://doi.org/10.1016/s0020-7462(98)00085-7 doi: 10.1016/s0020-7462(98)00085-7
[40]	X. B. Yin, S. Kumar, D. Kumar, A modified homotopy analysis method for solution of fractional wave equations, Adv. Mech. Eng., 7 (2015). https://doi.org/10.1177/1687814015620330
[41]	X. J. Yang, D. Baleanu, H. M. Srivastava, Local fractional laplace transform and applications, In: Local fractional integral transforms and their applications, New York: Academic Press, 2016. https://doi.org/10.1016/b978-0-12-804002-7.00004-8
[42]	P. Sunthrayuth, H. A. Alyousef, S. A. El-Tantawy, A. Khan, N. Wyal, Solving fractional-order diffusion equations in a plasma and fluids via a novel transform, J. Funct. Space, 2022 (2022), 1899130. https://doi.org/10.1155/2022/1899130 doi: 10.1155/2022/1899130
[43]	M. K. Alaoui, R. Fayyaz, A. Khan, R. Shah, M. S. Abdo, Analytical investigation of Noyes-Field model for time-fractional Belousov-Zhabotinsky reaction, Complexity, 2021 (2021), 3248376. https://doi.org/10.1155/2021/3248376 doi: 10.1155/2021/3248376

This article has been cited by:

1.	Zareen A. Khan, Sajjad Ahmad, Salman Zeb, Muhammad Yousaf, Yusuf Gurefe, Study of Fractional-Order Boundary Value Problems Using Nondiscretization Numerical Method, 2023, 2023, 2314-4785, 1, 10.1155/2023/5581962
2.	Nikita Saha, Randhir Singh, An efficient new numerical algorithm for solving Emden–Fowler pantograph differential equation using Laguerre polynomials, 2023, 72, 18777503, 102108, 10.1016/j.jocs.2023.102108
3.	Mamta Kapoor, Simran Kour, An analytical approach for Yang transform on fractional-order heat and wave equation, 2024, 99, 0031-8949, 035222, 10.1088/1402-4896/ad24ab

Reader Comments

Your name:*

Email:*
© 2023 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

AIMS Mathematics

1.8 3.4

Metrics

Article views(1489) PDF downloads(128) Cited by(3)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

Figures and Tables

Figures(3) / Tables(1)

AIMS Mathematics

Numerical investigation of fractional-order wave-like equation

Related Papers:

Abstract

1. Introduction

2. Preliminaries

3. Construction of Homotopy perturbation transform method for solving FDEs

4. Construction of Yang transform decomposition method for solving FDEs

5. Examples

6. Conclusions

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Catalog

AIMS Mathematics

Numerical investigation of fractional-order wave-like equation

Related Papers:

Abstract

1. Introduction

2. Preliminaries

3. Construction of Homotopy perturbation transform method for solving FDEs

4. Construction of Yang transform decomposition method for solving FDEs

5. Examples

6. Conclusions

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog