1.
Introduction
In the last few years, fractional calculus has attracted the attention of many researchers due to the non-integer order derivatives and integrals that have extra degrees of freedom. Fractional calculus plays a significant role due to the rapid development of nanotechnology. Several real-life problems are analyzed in more precise ways by taking arbitrary order derivatives and integrals. Fractional calculus allows us to study the characteristic behaviors, memory properties and hereditary properties of several phenomena [1,2]. Leibnitz developed the concept of fractional calculus in a letter to L' Hospital in 1665. Georgesco and Hsieh [3] developed the mathematical models for various dynamics to study the physical and biological problems in fractional order. The study of Fractional differential equations (FDEs) [4,5,6] is rapidly increasing due to its applications in real-world problems including blood flow, signal processing, image processing, economics and chemistry, etc. FDEs an extension of classical differential equations. These are popular due to their wide range of applications in different fields of science and engineering [7,8,9,10]. The mathematical theory and foundations of fractional calculus and FDEs can be found in [11,12,13]. Fractional derivatives, rather than integer-order derivatives, can be used to model a wider range of behaviors. However, FDEs with only one fractional derivative is not always sufficient to describe the physical processes. Therefore more general types of fractional-order models including multi-term equations and multi-dimensional systems, have been analyzed and studied recently by several researchers. The system of FDEs with incommensurate order has gained considerable attention in many fields of science and engineering including financial systems [14,15], circuit simulation [16] and eco-epidemiological model [17,18]. Various authors developed several mathematical models to solve the differential equations with different order of derivative; for example, the differential model with real order [19], generalized RL-fractional fractional order derivative [19] and Caputo fractional order derivative [21]. Luchko Yakubovich [22] discuss the solution of several classes of integro-differential equations. Solution of some fractional model for COVID-19 and fractional blood alcohol model with composite fractional order is extracted in [23,24]. Many authors attaining the attention for solving fractional differential equations system both in crisp and uncertain environment. Bhakta et al. [25] constructed the solution of fractional Hardy-Sobolev equations with nonhomogeneous terms. The solutions for fully nonlinear equations, nonlocal Kirchhoff problems and fractional Schrödinger-Kirchhoff systems with singular and critical nonlinearities have been discussed in [26,27,28]. Bonder et al. [29] extract the solution of optimal and normalized obstacle problem in fractional setting. Fernandez et al. [30] used Laplace, Fourier, and other related transforms to solve multi-term partial FDEs.
Many mathematical models representing real-life phenomena often contain considerable uncertainty due to various circumstances. Fuzzy set theory [31] is a useful tool for describing uncertainty and ambiguity. We use it in disciplines where data involves ambiguity, including environmental science, medicine, economic science, social science and physical science. Fractional calculus has gained attention over the past few years for its numerous applications in science and engineering. Many researchers have sparked research on the theoretical background of the problem containing ambiguity. Fuzzy fractional differentiation has become the most useful tool in scientific and engineering modeling such as modeling of populations, weapon systems, electro-hydraulics and even civil engineering. Several researchers studied fuzzy fractional differential equations (FFDEs) numerically and analytically to visualize uncertainty and ambiguity; for example, fractional difference and differential operators with Mittag-Leffler kernels [32,33], solutions of the systems of first-order and two-point boundary value problems [34], ABC-Fractional Volterra and integrodifferential equations of Fredholm operator [35,36], solutions of advection-diffusion and fredholm time-fractional partial integrodifferential equations [37,38], solution of fractional model [39] in shallow water and the solution of fuzzy differential equation [40].
Chang and Zadeh [41] proposed the concept of fuzzy derivatives. Dubois and Prade [42] extended Chang and Zadeh's ideas to the theory of extension principles. Kaleva [43,44] applied the ideas of Dubois and Prade to solve the fuzzy differential equations and initial-value problems analytically. Seikkala [45] obtained a unique solution to the initial-value problem using gH-differentiation in a fuzzy environment. Numerous researchers used Riemann-Liouville (RL) and Caputo-differential methods to solve fuzzy differential equations and initial-value problems. Hoa et al. [46] developed gH-fractional differentiability with the help of interval number methods. Several local and nonlocal arbitrary fractional terms exist in the literature, including RL, Caputo and Grünwald differentiability. These derivatives are more attractive because of their applications. Indeed, gH-differentiability ensures that without losing the potential to use Caputo-fractional derivatives to determine solutions of FFDEs. Multi-dimensional systems of FDEs have been studied using various analytical and numerical methods. Several researchers have studied the solutions of the systems in case of homogeneous and non-homogeneous with constant coefficients. Akram et al. [47,48,49] discussed the analytical fuzzy solution for solving fuzzy and Pythagorean fuzzy FDEs using Laplace transform technique with Caputo derivative.
In this work, we present an advanced analysis of a two-dimensional system of fuzzy linear fractional differential equations (FLFDEs) with Caputo derivative of two independent fractional orders using the Mittag-Leffler function (MLF) involving triple series. The term MLF is an extension of the exponential function which was first introduced in 1903. Many researchers extended this concept and defined MLF one, two, three or multiple parameters and variables thoroughly in the literature. MLF plays a vital role in the theory of FDEs. Although, many scholars introduced several interesting schemes and approaches for solving FFDEs. This article asserts its originality from the following perspectives.
(i) Determine the solution of a two-dimensional system of fuzzy linear homogeneous fractional differential equations using trivariate Mittag-Leffler kernel with fuzzy constraints and uncertain initial-conditions.
(ii) Two possible solutions the fuzzy system of linear homogeneous fractional differential equations are investigated.
(iii) The concepts of fuzzy fractional derivatives and integrals are extended with trivariate MLFs.
(iv) Develop the inversion formula for derivative-integral in both directions and prove the property of boundedness.
(v) Several important concepts, facts and relationships related to fuzzy fractional calculus are discussed and analyzed.
(vi) Discuss and modify an application A- diffusion processes in fuzzy environments to visualize and support theoretical result.
The rest of the paper is organized as follows: In Section 2, we review the basic concepts and terminologies of fractional calculus and fuzzy fractional calculus. In Sections 3 and 4, we extend fuzzy fractional integral and differential operator in terms of trivariate Mittag-Leffler (TML) function and investigate some properties to establish new results. Section 5 is devoted to solving the two-dimensional system of FLFDEs and interpreting the exact solutions in form of TML function. Section 6 deals with the application of the aforesaid system. Section 7 deals with the concluding remarks and future directions.
2.
Preliminaries
We review some fundamental concepts that are important for this manuscript. Suppose that the fuzzy set h in a non-empty subset Ω of R identified with the rule of membership grade h:Ω⟶k:[0,1]. Firmly, h is convex because
h is upper semi-continuous so that {u∈R|h(u)≥λ} is closed for all λ∈[0,1]; and h is normal because there exist u∈R such that h(u)=1. The support of h is {u∈R|h(u)>0}. First we recall the α-cut representation of triangular fuzzy number is defined as follows:
Definition 2.1. [50] The fuzzy number h is a fuzzy subset of R equipped with normal, convex, upper semi-continuous and bounded support.
Initially, for every α∈k╲{0}, the set [h]α={u∈R|h(u)≥α} with α=0 and [h]0=¯{u∈R|h(u)>0}. Then h∈Fℜ (where Fℜ is the class of all fuzzy number on R) if and only if [h]α∈R and [h]1 is non-empty. In fact, if h∈Fℜ, then [h]α=[h1(α),h2(α)] with h1(α)=min{u:u∈[h]α} and h2(α)=max{u:u∈[h]α}. Here [h]α denotes the α-cut expansion of h. Suppose that h,i∈Fℜ, if there exist j∈Fℜ with h=i+j. Then j will be the H-difference of (h,i). We will compose the standard notation of H-difference is h⊖Hi. Indeed, if H-difference h⊖Hi exists, then [h⊖Hi]α=[h1(α)−i1(α),h2(α)−i2(α)].
Definition 2.2. [50] A parametric fuzzy number h is an ordered pair (h1(α),h2(α)) of the functions h1(α),h2(α);α∈[0,1] and fulfil the following requirements:
(i) h1 is a function of h1(α) which is bounded, monotonically increasing, left continuity on set (0, 1] and right continuity at point 0.
(ii) h2 is a function of h2(α) which is bounded, monotonically decreasing, left continuity on set (0, 1] and right continuity at point 0.
(iii) h2(α)≥h1(α).
Note that, the class of all parametric fuzzy numbers (PFNs) with the operation of addition and scalar multiplication is denoted by Fℜ.
Definition 2.3. The mapping h:E⟶Fℜ is said to be strongly generalized derivative (SGD) [51] at u∈E, if ∃ Duh(u)∈Fℜ such that one of the following conditions satisfied:
(i) For every θ positive, the expressions h(u+θ)⊖h(u) and h(u)⊖h(u−θ) both exists such that
(ii) For every θ positive, the expressions h(u)⊖h(u+θ) and h(u−θ)⊖h(u) both exists such that
Indeed, h is differentiable on E if h is differentiable for every u∈E.
Definition 2.4. [51] For a mapping h:E⟶Fℜ such that the following status can be obtained:
(i) h is called (ı)-differentiable over E, if h is differentiable at status (i) of Definition 2.3, which can be denoted as Dıuh(u).
(ii) h is called (ıı)-differentiable over E, if h is differentiable at status (ii) of Definition 2.3, which can be denoted as Dııuh(u).
Definition 2.5. [51] A mapping h:E⟶Fℜ such that the following condition may achieved:
(i) If h is (ı)-differentiable, then the functions h1(α) and h2(α) (where h1(α) and h2(α) are the crisp differentiable functions over E) are differentiable and
(ii) If h is (ıı)-differentiable, then the functions h1(α) and h2(α) are differentiable and
The Sobolev space of one order on E with the mapping h:E⟶ℜ such that it can be defined as
Definition 2.6. [52,53,54] Let h:E⟶ℜ, h∈S(E). Then the RL fractional (RL-fractional) integral operator of order τ∈C, Re(τ)>0 of h is defined as
In practice, we usually assume a=0 and the real number τ>0.
Definition 2.7. Let h:E⟶ℜ, h∈S(E). Then the RL-fractional derivative of order τ∈C, Re(τ)≥0 of h is defined as
where m is the natural number such that m−1≤Re(τ)<m. In particular, if τ∈(0,1) and a=0 then the definition is
Definition 2.8. Let h:E⟶ℜ, h∈S(E). Then the Caputo fractional derivative of order τ∈C, Re(τ)≥0 of h is defined as
Where m is the natural number such that m−1≤Re(τ)<m. In particular, if τ∈(0,1) and a=0 then the definition is
Fuzzy fractional calculus
In this section, we present some fundamental concepts related to the fuzzy RL and Caputo fuzzy fractional derivative and integral.
Let CFℜ(E) and LFℜ(E) be the space of all continuous and integrable fuzzy-valued functions on E, respectively.
Definition 2.9. Let h:E⟶ℜ, h∈CFℜ(E)∩LFℜ(E). Then the RL-fractional integral [55] for fuzzy-valued function h (in the form of α-cut representation) of order τ∈C, Re(τ)>0 is
where the lower and upper fuzzy-valued functions defined as following
Remark. Now from the onward, we suppose all the m-th differentiable functions are i-th differentiable.
Based on the above definition, the fuzzy RL-fractional derivative is defined as:
Definition 2.10. Let h:E⟶ℜ, h∈CFℜ(E)∩LFℜ(E). If h is τ(ı)-fuzzy fractional differentiable. Then the fuzzy RL-fractional derivative [55] of order τ∈C, Re(τ)≥0 is
If h is τ(ıı)-fuzzy fractional differentiable. Then the fuzzy RL-fractional derivative of order τ∈C, Re(τ)≥0 is
Where, the entire integral is defined in Definition 2.9 and m is the natural number such that m−1≤Re(τ)<m. In particular, if τ∈(0,1) and a=0 then the definition takes the following form for the first and second differentiability
and
Definition 2.11. Let h:E⟶ℜ, h∈CFℜ(E)∩LFℜ(E). If h is τ(ı)-fuzzy fractional differentiable. Then the fuzzy Caputo-fractional derivative [13] of order τ∈C, Re(τ)≥0 is
If h is τ(ıı)-fuzzy fractional differentiable. Then the fuzzy Caputo-fractional derivative of order τ∈C, Re(τ)≥0 is
where, the integral is defined in Definition 2.9 and m is the natural number such that m−1≤Re(τ)<m. In particular, if τ∈(0,1) and a=0 then the definition takes the following form for the first and second differentiability as
and
Definition 2.12. [56] Let h:E⟶Fℜ, h∈CFℜ(E)∩LFℜ(E). Assume that e−quh(u) is improper fuzzy Riemann integrable on [0,∞), then the integral ∫∞0e−quh(u)du is said to be the fuzzy Laplace transform of function h and its symbolic representation
since,
So, Eq (2.13) takes the following form
where, L(h1(u,α))=∫∞0e−qu[h1(u,α)]du, L(h2(u,α))=∫∞0e−qu[h2(u,α)]du and L(h(u)) is the classical notation of Laplace transform of crisp function h(u).
In the section below, we present an analytical approach to solve two dimensional multi-order system of fuzzy fractional differential equations with independent order.
3.
Fuzzy fractional integral with trivariate Mittag-Leffler kernels
The MLF [57] is an extension of the exponential function which was first introduced in 1903. The MLF first define as a one-parameter with a convergent infinite series. The MLF with one, two, three or multiple parameters and variable thoroughly studied in [58,59,60,61,62]. In the fractional calculus, MLF plays a vital role in the theory of FDEs. MLF in classical form is defined as
and with two-parameter is
The MLF [63] in three and four parameter is defined as
and
where, Θ>α,β,γ and λ,μ,ϑ are real number.
We define fuzzy fractional integral in terms of trivariate MLF and related results:
Definition 3.1 Let h:E⟶Fℜ, h∈CFℜ(E)∩LFℜ(E). We define fuzzy fractional integral in terms of trivariate MLF with kernel in univariate form whose convolution can be determined as
where,
and
where ϵ,η1,η2,η3,μ,ν,κ,τ>0 all are real parameters.
Note 3.1. If ϵ=0, then the fuzzy fractional integral (3.4) coincide with fuzzy RL fractional integral
where,
and
The main fact for the above argument is that:
Theorem 3.1. Let h:E⟶Fℜ and h∈CFℜ(E)∩LFℜ(E). Then one can achieve the following status:
i. aIϵ;η1,η2,η3μ,ν,κ,τh(u)∈Fℜ
ii. [aIϵ;η1,η2,η3μ,ν,κ,τh(u)](α)=[aIϵ;η1,η2,η3μ,ν,κ,τh1(u,α),aIϵ;η1,η2,η3μ,ν,κ,τh2(u,α)].
Proof. For condition i: since [h(u)](α)=[h1(u,α),h2(u,α)]. We have
Because, h2(α)(u−s)τ−1−h1(α)(u−s)τ−1≥0. It yields
Thus,
Hence the set [aIϵ;η1,η2,η3μ,ν,κ,τh1(u,α),aIϵ;η1,η2,η3μ,ν,κ,τh2(u,α)] is a compact subset of ℜ and is non-empty at α=1. On the base of [64], we have aIϵ;η1,η2,η3μ,ν,κ,τh(u)∈Fℜ.
Now for the condition ii: we have
Or
This completes the proof. In the following theorem, our target is to prove the fuzzy integral operator is a bounded operator:
Theorem 3.2. Let h:E⟶Fℜ and h∈CFℜ(E)∩LFℜ(E). The fuzzy fractional integral aIϵ;η1,η2,η3μ,ν,κ,τh is bounded operator on h∈CFℜ(E)∩LFℜ(E) such that
where
Proof. Starting with the Definition 3.1, we have
According to the Fubini's theorem, we have
Take
Remember that, K is convergent [60]. So this K is well-defined. Therefore,
where, h∈CFℜ(E)∩LFℜ(E). This completes the proof.
With the help of a series formula, the integral operator (3.1) can be stated in terms of RL-fractional integrals in fuzzy environment as shown in the following theorem:
Theorem 3.3. Let h:E⟶Fℜ, h∈CFℜ(E)∩LFℜ(E). The fuzzy fractional integral aIϵ;η1,η2,η3μ,ν,κ,τh can be expressed as
Proof. Since the trivariate MLF is locally uniformly convergent [60]. We can change summation and order of integration to obtain the desired ressult:
Hence,
This completes the proof.
Theorem 3.4. Let h:E⟶Fℜ, h∈CFℜ(E)∩LFℜ(E). The fuzzy Laplace transform of fuzzy fractional integral is denoted by L(aIϵ;η1,η2,η3μ,ν,κ,τh(u))(q) and is defined as
Proof. We start this proof by taking a=0 in the Definition (3.4) and using convolution theorem [65], we have
Thus
where, L−1 and L are the classical and fuzzy Laplace transform operator, respectively. This completes the proof.
4.
Fuzzy fractional derivative with trivariate Mittag-Leffler kernels
In this section, we introduce the concept of fuzzy fractional derivative in terms of MLF by using strongly generalized differentiability. In addition, several significant concepts, facts and relationships are analyzed and proven. The space of all τ-Caputo crisp differentiable function on E is denoted by Dℜτ(E)
Definition 4.1. Let h:E⟶Fℜ, and h,h′∈CFℜ(E)∩LFℜ(E). Then, the fuzzy RL-fractional derivative corresponding to the operator (3.1) in terms of trivariate MLF at the node point u=0 is symbolized by RL0Dϵ;η1,η2,η3μ,ν,κ,τh(u) and is defined as
when h(u) is τ(ı)-fuzzy RL-fractional derivative and:
when h(u) is τ(ıı)-fuzzy RL-fractional derivative. Where, m∈N and m=⌊ϵ⌋+1 also ϵ,m−ϵ are real number with m−ϵ>0 for the above integral operator.
Definition 4.2. Let h:E⟶Fℜ, and h,h′∈CFℜ(E)∩LFℜ(E). Then, the fuzzy Caputo-fractional derivative corresponding to the operator (3.1) in terms of trivariate MLF at the node point u=0 is symbolized by C0Dϵ;η1,η2,η3μ,ν,κ,τh(u) and is defined as
when h(u) is τ(ı)-fuzzy Caputo fractional derivative and:
when h(u) is τ(ıı)-fuzzy Caputo fractional derivative. Where, m∈N and m=⌊ϵ⌋+1 also ϵ,m−ϵ are real number with m−ϵ>0 for the above integral operator.
Definition 4.3. For C0Dϵ;η1,η2,η3μ,ν,κ,τh(u):E⟶Fℜ, h is said to be τ(ı)-fuzzy fractional differentiable whenever h is (ı)-fuzzy differentiable at status (ⅰ) of Definition 2.5 and is τ(ıı)-fuzzy fractional differentiable whenever h is (ıı)-fuzzy differentiable at status (ⅱ) of Definition 2.5.
Theorem 4.1. Suppose that h:E⟶Fℜ, and h,h′∈CFℜ(E)∩LFℜ(E). Then the following condition holds a=0:
If h is (ı)-fuzzy differentiable, then we have τ(ı)-fuzzy fractional differentiable jointly with h1(u,α),h2(u,α)∈Dℜτ(E) and
If h is (ıı)-fuzzy differentiable, then we have τ(ıı)-fuzzy fractional differentiable jointly with h1(u,α),h2(u,α)∈Dℜτ(E) and
Proof. Remember that Dıuh(u)](α)=[Duh1(u,α),Duh2(u,α)] and Dııuh(u)](α)=[Duh2(u,α),Duh1(u,α)]. By using status (ⅰ) and Definition 4.2, we have
Thus
Now we study the status (ⅱ) and Definition 4.2, we have
Thus
This completes the proof.
Theorem 4.2. Suppose that h:E⟶Fℜ, and h,h′∈CFℜ(E)∩LFℜ(E). Then we have the following achievements:
If h is τ(ı)-fuzzy RL-fractional differentiable, then
If h is τ(ıı)-fuzzy RL-fractional differentiable, then
Similarly, If h is τ(ı)-fuzzy Caputo-fractional differentiable, then
If h is τ(ıı)-fuzzy Caputo-fractional differentiable, then
Proof. In term of h is τ(ı)-fuzzy RL-fractional derivative, we can study the status (ⅰ) as
Suppose if h is τ(ıı)-fuzzy RL-fractional derivative, we can study the status (ⅱ) as
Similarly, if h is τ(ı)-fuzzy Caputo-fractional derivative, we can study the status (ⅰ) as
If h is τ(ıı)-fuzzy Caputo-fractional derivative, we can study the status (ⅱ) as
Hence
This completes the proof.
Theorem 4.3. Suppose that h:E⟶Fℜ, and h,h′∈CFℜ(E)∩LFℜ(E). Then the Definitions 4.1 and 4.2 have the following series formulae:
If h is τ(ı)-fuzzy RL-fractional derivative, then
If h is τ(ıı)-fuzzy RL-fractional derivative, then
Similarly, If h is τ(ı)-fuzzy Caputo-fractional derivative, then
If h is τ(ıı)-fuzzy Caputo-fractional derivative, then
Proof. If h is τ(ı)-fuzzy RL-fractional derivative, then
By using series formula from Theorem 3.3 into the Eqs (4.1) and (4.2), we have:
Therefore,
If h is τ(ıı)-fuzzy RL-fractional derivative, then
By using series formula from Theorem 3.3 into the Eqs (4.1) and (4.2), we have:
Therefore,
We can use similar arguments to prove the Eqs (4.13) and (4.14). This completes the proof.
5.
Two-dimensional system of homogeneous fuzzy fractional differential equations
In this section, first we develop some categorial frame work for the solution of two dimensional multi-order system of fuzzy fractional differential equations with independent order. For this purpose, we prove the following results, which are needed to find the solution of this system. The general 2-dimensional incommensurate coupled system of fuzzy fractional differential equations with Caputo derivatives as:
where the coefficients mij∈ℜ, initial-values mi(0,α)∈Fℜ and order μi∈(0,1) for i,j=1,2.
We observe the solution of the aforementioned system (5.1) in the following results:
Theorem 5.1. If m(u) is τ(ı)-fuzzy Caputo-fractional derivative, then the system (5.1) has a unique solution is of the form
where
and
Proof. We begin to proof this result with the help of Theorem 3.1 in [49]. Applying fuzzy Laplace transform on both sides of the Eq (5.1), we have
5.1. Phase ⅰ:
If m(u) is τ(ı)-fuzzy Caputo-fractional derivative, then Eq (5.2) becomes as
That gives
and
By using initial conditions, we obtain
and
Now first we find the lower fuzzy-valued functions. From the second equation of the expression (5.6), we have
From the first equation of the expression (5.6), we have
By substituting the value of Eq (5.8) in first equation of the expression (5.6), we have
By substituting the value of Eq (5.9) in second equation of the expression (5.6), we have
After arranging, Eq (5.10) gives
and Eq (5.11) gives
where ξ=c12c21−c11c22. Taking inverse fuzzy Laplace transform to the Eqs (5.12) and (5.13), we have
and
By using classical inverse Laplace transform [63] to the Eqs (5.14) and (5.15), we have
and
For upper fuzzy-valued functions, Eq (5.7) becomes as
From the second equation of the expression (5.7), we have
From the first equation of the expression (5.7), we have
By substituting the value of Eq (5.17) in first equation of the expression (5.6), we have
By substituting the value of Eq (5.18) in second equation of the expression (5.6), we have
After arranging, Eq (5.19) gives
and Eq (5.20) gives
where ξ=c12c21−c11c22. Taking inverse fuzzy Laplace transform to the Eqs (5.21) and (5.22), we have
and
By using classical inverse Laplace transform [63] to the Eqs (5.23) and (5.24), we have
and
Hence
Or
5.2. Phase ⅱ:
If \mathfrak{m}(u) is \tau(\imath\imath) -fuzzy Caputo-fractional derivative, then Eq (5.2) becomes as
That gives
and
By using initial conditions, we obtain
and
Solving the above systems (5.28) and (5.29) simultaneously, we have
and
For the sake of simplicity, let \mu_{1} = \mu_{2} = \mu . Taking the inverse Laplace transform, we have
and
By using classical inverse Laplace transform [63] to the above Equations, we have
and
This completes the proof.
6.
Application in diffusion process
Several researchers [67,68,69] introduced the useful techniques to study the physical and biological problems using fractional model. We are going to solve a diffusion problem in fuzzified version which have been studied [66] as a classical version. Consider two neighboring cells completely separated by membrane. We assume that the fluid is flowing from these two cells at a rate in milliliter per minute. The fluid is flow from first cell to the second cell having its volume three times to the first one. Then the fluid is flowing in outside from the second cell having its volume two times to the second one. Let \mathfrak{m}_{1}(u) and \mathfrak{m}_{2}(u) represent the volume of the fluid in the first and second cell, respectively with the time u . Suppose that the first and second cell has fluid \tilde{\jmath} = \big[\underline{\jmath}+(\jmath-\underline{\jmath})\alpha, \overline{\jmath}-(\overline{\jmath}-\jmath)\alpha\big] and \tilde{\ell} = \big[\underline{\ell}+(\ell-\underline{\ell})\alpha, \overline{\ell}-(\overline{\ell}-\ell)\alpha\big] in terms of fuzzy number. Now we try to determine the volume of fluid in term of fuzzy number in each cell at the time u . The linear system of fractional differential equation of the above model can be written as
because the change in \mathfrak{m}_{1}(u) and \mathfrak{m}_{2}(u) is the difference between the amount of fluid which flow in and out to the first and second cell, respectively.
If \mathfrak{m}(u) is \tau(\imath) -fuzzy Caputo-fractional differentiable, then the Theorem 5.1 use as an application to the system (6.1). We have a unique solution is of the form
Where
For instance, we take \tilde{\jmath} = (\underline{\jmath}, \jmath, \overline{\jmath}) = (30, 40, 50) , \tilde{\ell} = (\underline{\ell}, \ell, \overline{\ell}) = (4, 5, 6) and \mu_{1} = \dfrac{1}{2} , \mu_{2} = \dfrac{1}{3} . Then Eq (6.2) becomes as
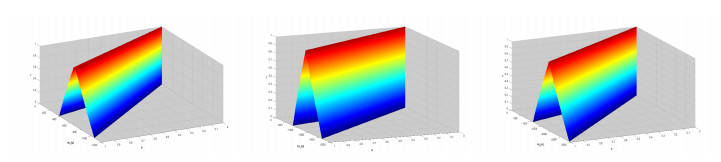
Graphical representation of the solution of expression (6.3), (see Figures 1 and 2).
Sensitive analysis
The crisp solution of this diffusion process is
That is actually equal to the fuzzy fractional solution by taking \alpha = 1 in the expression (6.3).
If we slightly change the crisp initial condition from 40 to 40.1 and 5 to 5.1 . Then the solution will be is of the form
This solution is acceptable and is equal to the fuzzy fractional solution by taking \alpha = 0.99 in the expression (6.3).
This comparative study shows how the fuzzification of this fractional linear system can help the reader to maintain the tolerance of this fractional linear system. This study can be of great importance and its usage in diffusion process as well as in the designing process.
7.
Conclusions
Over the past few decades, fractional calculus has been on the radar of top mathematicians, and over the past period it has become a very useful tool for solving the dynamics of complex systems in various branches of science and engineering. Fuzzy set theory has proven to be a useful tool for describing situations where data is imprecise or ambiguous. Fuzzy sets handle this situation by determining how well an object belongs to a set. In this paper, we have analyzed a two-dimensional system of FLFDE with two independent fractional Caputo derivatives. The solutions of this system are divided into two classes according to strong generalized H-differentiability. Furthermore, we have derived potential solutions for MLF involving three series. We have extended the concept of fuzzy fractional calculus in terms of ternary MLF. Some important concepts, facts, and relationships have derived, and boundedness has been demonstrated. Finally, we have modified and discussed the application of FLFDEs systems to diffusion processes, and have analyzed their graphs to visualize and support theoretical results. In the future, we plan to solve the non-homogeneous two-dimensional system of fuzzy linear fractional differential equations.
Conflicts of interest
The authors declare no conflicts of interest.









 DownLoad:
DownLoad:




