Modeling radicalization of terrorism under the influence of multiple ideologies

1.   Introduction

The spread of a terrorist ideology can have an important impact on the process of individual radicalization. Inspired by the "Islamic State" ideology, a large number of individuals became "jihadists". In 2014, at least 12,000 "foreign fighters" from 81 countries joined the "Islamic State". Some Western youths who are dissatisfied with their circumstances accepted the ideas of the "Islamic State" and embarked on the path of violent extremism. Radicalization is a major issue for national security, so it is necessary to deeply understand its process in order to prevent and stop the radicalization of individuals. It is in this context that this paper mathematically describes radicalization by modeling the spread of extremist ideology, in a manner similar to modeling the spread of infectious diseases.

In recent years, scholars have gradually deepened their research on infectious disease models. These studies involve the nature, transmission dynamics and application of infectious disease models ^[1,2,3,4]. Many mathematical scientists use population dynamics models to simulate the spread of ideology based on the exposure process of tuberculosis. They built a warehouse model, used parameters to simulate the push and instillation of extreme ideological information ^[5].

A similar approach was adopted in ^[6,7,8]. The difference is that scholars introduced the mechanism of super infection in which pathogens competing strains coexist, that is, the process in which individuals previously infected by one pathogen are infected by another ^[9]. Therefore, scholars compare ideological competition to the competition process of pathogens, and propose that the existence of competitive and milder ideologies may prevent the spread of violent extremist ideology. And scholars have analyzed the efficacy of de-radicalization treatments of extremists ^{[7,8,10,11,12,13,14]}.

This paper extends the model established by C. C. McCluskey and M. Santoprete in ^[8]. The difference is that we have considered a more complex ideological dissemination strategy of terrorist organizations.

In epidemiology, in addition to competition mechanisms, there are cooperation mechanisms for overlapping infections. Individuals who have been infected with one pathogen are infected with another pathogen. The two pathogens can coexist and increase the infection of the individual through cooperation. Generally speaking, the existence of a kind of bacteria will create a breeding niche for other pathogenic bacteria, and the host is easy to be colonized by other microorganisms ^[15]. Compared with single microbial infections, overlapping infections are usually associated with an increase in the severity of the infection and a poorer prognosis for patients ^[16,17]. For example, patients with chronic hepatitis B superinfected with hepatitis E virus can aggravate the condition and increase the risk of liver cirrhosis, liver failure and death ^[18].

The ideology and practice of terrorist organizations can be quite complex, and overlapping ideological concerns deserve more attention. Some terrorist organizations may have multiple long-term objectives or programs that they hope to install if they are able to come into power. Such overlaps could be especially true for religious terrorist organizations, which often have both explicitly religious and more directly political objectives. Among the 202 religious terrorist organizations listed in 2006 by the Terrorist Knowledge Base of the National Memorial Association for the Prevention of Terrorism, some of the largest are also included in the classification of nationalist groups, such as Hamas and Hezbollah ^[19]. In order to attract more supporters, these terrorist organizations with multiple objectives often construct multiple ideologies. The "Islamic State" has drawn on the theoretical construction created by earlier movements and parties based in political Islam, and focused on the idea of invoking jihad as holy war to motivate followers. The two core ideologies of the "California" and the violent "jihad" maintain and consolidate their own legitimacy, and through the construction of subsidiary ideologies such as "migration", "loyalty" and "joy and anger for Allah". Identify and maintain global combat attacks ^[20].

We hope to use our model to test some possible strategies for coping with radicalization. First, we need to test the effect of these mutually reinforcing ideologies on individual extremes when terrorist organizations begin to spread multiple ideologies. Therefore, we assume that individuals can transfer from one ideology to another, so we design the model to include two ideologies. The useful parameters in the model to quantify the spreading potential of extremist ideology are the basic reproduction number and the entrance number.

We will prove, if both basic reproduction numbers are less than 1, the two ideologies will be eradicated. If either of the two basic reproduction numbers is greater than 1, coexistence equilibrium may prevail. And if the coexistence equilibrium is asymptotically stable locally, it will make it difficult to eliminate any kind of ideology. Our analysis shows that multiple ideologies may enhance the effectiveness of terrorist organization recruitment strategies, which makes combating violent extremism more difficult.

Finally, we use numerical simulations to test the effectiveness of conventional de-radicalization strategies in the context of cooperative ideology. Unlike the results in the single ideological model, our model has produced new results. The cooperation mechanism provides the ideology with a communication advantage, which makes the ideology more attractive to the crowd. The main lesson we learned from this is that when terrorist organizations adopt multiple ideological dissemination strategies, simply combating or preventing one ideology will not achieve the expected suppression effect, and may even increase the attractiveness of other ideologies of terrorist organizations.

2.   Mathematical model

In order to absorb more individuals, terrorist organizations may spread multiple ideologies. In this section, we want to test the effects of these mutually reinforcing ideologies on the radicalization of individuals. In some epidemiological systems, this phenomenon is called superinfection.

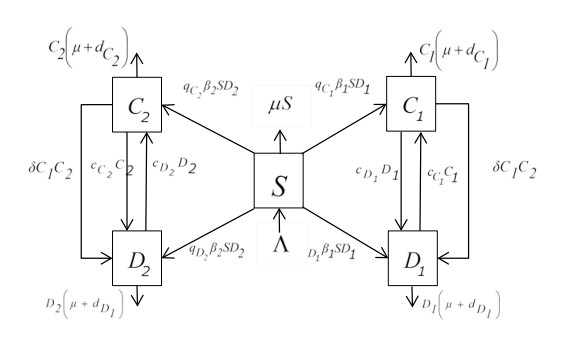
The model assumes that there are two extreme ideologies in the population, and there is a sub-population that is at risk to adopt the ideologies. The sub-population is divided into five compartments: Susceptible --$S$, Sympathizers 1--${C_{\mathit{1}}}$, Defenders 1--${D_{\mathit{1}}}$, Sympathizers 2--${C_{\mathit{2}}}$, Defenders 2--${D_{\mathit{2}}}$.

The individuals in susceptible class are those who have not adopted the extremist ideology. The ones in sympathetic groups initially adopt extreme ideology. The defender group are adopters that engage in violent terrorist acts. They are the most extreme, and have a strong demonstration effect on individuals in other categories and can have a major chance of infecting others. The defenders can recruit and incite susceptible and sympathetic individuals.

New individuals enter the susceptible class in the population at the constant rate $\Lambda$. The average natural mortality constant rate is $\mu$. Sympathizers and defenders have additional removal rates, because they are captured with rate constants ${d_{{C_{\mathit{1}}}}}$, ${d_{{D_{\mathit{1}}}}}$, ${d_{{C_{\mathit{2}}}}}$, ${d_{{D_{\mathit{2}}}}}$, respectively. For subgroups that adopt one ideology, it is assumed that the susceptible and defenders interact according to the laws of group action, and the ratio of recruiting susceptible individuals to adopt the extremist ideology is proportional to the number of interactions that are taking place between the sub-groups. Thus, susceptible individuals are recruited at rate ${\beta _{\mathit{1}}}S{D_{\mathit{1}}}/{\beta _{\mathit{2}}}S{D_{\mathit{2}}}$, with a fraction ${q_{{C_{\mathit{1}}}}}/{q_{{C_{\mathit{2}}}}}$ entering the sympathetic class and a fraction ${q_{{D_{\mathit{1}}}}} = {\mathit{1}} - {q_{{C_{\mathit{1}}}}}/{q_{{D_{\mathit{2}}}}} = {\mathit{1}} - {q_{{C_{\mathit{2}}}}}$ entering the defender class.

Sympathizers can switch to the defender class at a constant rate ${c_{{C_{\mathit{1}}}}}/{c_{{C_{\mathit{2}}}}}$, while defenders enter the sympathetic class at a constant rate ${c_{{D_{\mathit{1}}}}}/{c_{{D_{\mathit{2}}}}}$. The next step is to introduce cross-interaction among sympathetic individuals. As a result of the process of interaction, sympathetic individuals can be influenced by another ideology and become more extreme, the constant rate is $\delta {C_{\mathit{1}}}{C_{\mathit{2}}}$. The flow map is in Figure 1. Physical interpretation of the parameters is in Table 1.

The differential equations are:

Where ${q_{{C_{\mathit{1}}}}} + {q_{{D_{\mathit{1}}}}} = {q_{{C_{\mathit{2}}}}} + {q_{{D_{\mathit{2}}}}} = 1$, ${q_{{C_{\mathit{1}}}}}, {q_{{D_{\mathit{1}}}}}, {q_{{C_{\mathit{2}}}}}, {q_{{D_{\mathit{2}}}}} \in \left[ \mathit{0, 1} \right]$. The assumption for the full population is $N = S + {C_{\mathit{1}}} + {D_{\mathit{1}}} + {C_{\mathit{2}}} + {D_{\mathit{2}}}$.

Proposition 1.1. Under the flow described by Eq (1), the region $\Delta = \left\{ {(S, {C_{\mathit{1}}}, {D_{\mathit{1}}}, {C_{\mathit{2}}}, {D_{\mathit{2}}}) \in \Re _{ \geqslant \mathit{0}}^\mathit{5}:N \leqslant \frac{\Lambda }{\mu }} \right\}$ is positively invariant and the attracting within is $\Re _{ \geqslant \mathit{0}}^\mathit{5}$.

Proof. The direction of the vector field is checked along the boundary of $\Delta$. Along $S = \mathit{0}$, ${S'} = \Lambda > \mathit{0}$ is present, so the vector field points inwards. For $S, {C_{\mathit{1}}}, {D_{\mathit{1}}}, {C_{\mathit{2}}}, {D_{\mathit{2}}} \geqslant \mathit{0}$, along ${C_{\mathit{1}}} = \mathit{0}$, ${C_{\mathit{1}}}' = {q_{{C_{\mathit{1}}}}}{\beta _{\mathit{1}}}S{D_{\mathit{1}}} + {c_{{D_{\mathit{1}}}}}{D_{\mathit{1}}} \geqslant \mathit{0}$ is present. Similarly, with ${D_{\mathit{1}}} = \mathit{0}, {C_{\mathit{2}}} = \mathit{0}, {D_{\mathit{2}}} = \mathit{0}$ respectively, we have ${D_{\mathit{1}}}' = {c_{{C_{\mathit{1}}}}}{C_{\mathit{1}}} + \delta {C_{\mathit{1}}}{C_{\mathit{2}}} \geqslant \mathit{0}$, ${C_{\mathit{2}}}' = {q_{{C_{\mathit{2}}}}}{\beta _{\mathit{2}}}S{D_{\mathit{2}}} + {c_{{D_{\mathit{2}}}}}{D_{\mathit{2}}} \geqslant \mathit{0}$, ${D_{\mathit{2}}}' = {c_{{C_{\mathit{2}}}}}{C_{\mathit{2}}} + \delta {C_{\mathit{1}}}{C_{\mathit{2}}} \geqslant \mathit{0}$, respectively. It follows from [(1), Proposition 1.1] that $\Re _{ \geqslant \mathit{0}}^\mathit{5}$ is positively invariant.

According to the standard comparison theorem, for $t \geqslant \mathit{0}$, it follows that $N(t) \leqslant (N(0) - \frac{\Lambda }{\mu }){e^{ - \mu t}} + \frac{\Lambda }{\mu }$. Thus, if $N(0) \leqslant \frac{\Lambda }{\mu }$, then $N(t) \leqslant \frac{\Lambda }{\mu }$ for all $t \geqslant \mathit{0}$, then the set $\Delta$ is positively invariant and the attracting within is $\Re _{ \geqslant \mathit{0}}^\mathit{5}$.

3.   Equilibrium points and the basic reproduction number

System (1) has three equilibrium points. We define the basic reproduction number to describe the equilibria which can quantify the ideology's transmission potential. In mathematical epidemiology, it is defined as the number of secondary infections caused by infectious individuals in susceptible class.

There is an equilibrium point ${x_{\mathit{0}}} = ({S_{\mathit{0}}}, {\mathit{0}}, {\mathit{0}}, {\mathit{0}}, {\mathit{0}}) = (\frac{\Lambda }{\mu }, {\mathit{0}}, {\mathit{0}}, {\mathit{0}}, {\mathit{0}})$, when ${C_{\mathit{1}}} = {D_{\mathit{1}}} = {C_{\mathit{2}}} = {D_{\mathit{2}}} = \mathit{0}$. If the first ideology is taken as an example, the basic reproduction number ${R_{\mathit{1}}}$ is the spectral radius of the next generation matrix $G$ at ${x_{\mathit{0}}}$. In Eq (1), when only the first ideology exists, the subgroups affected by it includes ${C_{\mathit{1}}}$, ${D_{\mathit{1}}}$. Set ${f_{{C_{\mathit{1}}}}}$, ${f_{{D_{\mathit{1}}}}}$ is the new ratio of individuals using this ideology in ${C_{\mathit{1}}}$, ${D_{\mathit{1}}}$. Let ${v_j} = v_j^ - - v_j^ +$, where $v_j^ +$ is conversion rate for individuals entering the subgroup $j \in \left\{ {{C_{\mathit{1}}}, {D_{\mathit{1}}}} \right\}$, and $v_j^ -$ is conversion rate for individuals moving out the subgroup $j$. The next generation matrix $G = F \cdot {V^{ - \mathit{1}}}$ is:

The next generation matrix $G$ has only one non-zero eigenvalue, and that non-zero eigenvalue is greater than zero, so its spectral radius is the non-zero eigenvalue. When ${S_{\mathit{0}}} = \frac{\Lambda }{\mu }$ is included, then there is the following:

Similarly, the basic reproduction value for the second ideology is:

The following result follows from [21,Theorem 2].

Theorem 2.1. If ${R_{\mathit{1}}} < \mathit{1}$ and ${R_{\mathit{2}}} < \mathit{1}$, then ${x_{\mathit{0}}}$ is locally asymptotically stable. If ${R_{\mathit{1}}} > \mathit{1}$ or ${R_{\mathit{2}}} > \mathit{1}$, then ${x_{\mathit{0}}}$ is unstable.

To calculate other equilibrium points, assume that when ${C_{\mathit{2}}} = {D_{\mathit{2}}} = \mathit{0}$, there is an equilibrium point $x_{\mathit{1}}^* = \left( {S_{_{\mathit{1}}}^*, C_{_{\mathit{1}}}^*, D_{\mathit{1}}^*, 0, 0} \right)$, set $C_{\mathit{1}}' = D_{\mathit{1}}' = \mathit{0}$, treating $S_{\mathit{1}}^*$ as a parameter. This gives the linear system result:

Only when the coefficient matrix is a singular matrix, i.e., when the determinant is 0, $C_{\mathit{1}}^*$, $D_{\mathit{1}}^*$ has non-zero solution.

If the first line of Eq (1) is taken, then

When ${R_{\mathit{1}}} > \mathit{1}$, $D_{\mathit{1}}^* > \mathit{0}$, add columns 2 and 3 in (1) to get:

where

Therefore, $C_{\mathit{1}}^*$ and $D_{\mathit{1}}^*$ have the same positive sign so the following equilibrium point results:

4.   Entrance numbers

There are equilibrium for which the second ideology is absent. The entrance number is the expected number of the ideology's new adopters when the new ideology invades the stable system.

According to ^[4], the effect of the second ideology entering the system at the equilibrium point $x_{\mathit{1}}^*$ (where the first ideology reaches balance) is calculated through the regeneration matrix. The subgroups adopting the second ideology are ${C_{\mathit{2}}}$ and $D{}_{\mathit{2}}$. The proportion of the newly added second ideological component and the conversion rate between the two subgroups are $f$ and $v$.

With the transition matrix $N = F{V^{ - \mathit{1}}}$, results in

where ${H_{\mathit{2}}} = \left( {\mu + {d_{{D_{\mathit{2}}}}}} \right)\left( {\mu + {d_{{C_{\mathit{2}}}}} + {c_{{C_{\mathit{2}}}}} + \delta C_{_{\mathit{1}}}^*} \right) + {c_{{D_{\mathit{2}}}}}\left( {\mu + {d_{{C_{\mathit{2}}}}}} \right)$.

The entrance numbers ${I_{\mathit{2}}}$ is the Spectral radius of $N$ :

When $A, B, O, P$ treated as a function of $\delta$, it can be seen that $A, B, O, P$ are monotonically increasing functions of $\delta$. Because $A, B, O, P$ are greater than zero, $\frac{{\partial {I_{\mathit{2}}}}}{{\partial A}}$, $\frac{{\partial {I_{\mathit{2}}}}}{{\partial B}}$, $\frac{{\partial {I_{\mathit{2}}}}}{{\partial O}}$, $\frac{{\partial {I_{\mathit{2}}}}}{{\partial P}}$ are greater than zero. Then ${I_{\mathit{2}}}$ is a monotonically increasing functions of $\delta$.

Similarly, ${I_{\mathit{1}}}$ is a monotonically increasing functions of $\delta$.

Theorem 3.1. If ${R_{\mathit{1}}} > \mathit{1}$, ${R_{\mathit{2}}} > \mathit{1}$, and ${R_{\mathit{1}}} \ne {R_{\mathit{2}}}$, then ${I_{\mathit{1}}}$ or ${I_{\mathit{2}}}$ must have a value greater than 1. If either of ${I_{\mathit{1}}}$ or ${I_{\mathit{2}}}$ is greater than 1, then the corresponding equilibrium point $x_{\mathit{2}}^*$ or $x_{\mathit{1}}^*$ is unstable.

5.   The coexistence of two ideologies

In this section, discuss how ${R_{\mathit{1}}}$, ${R_{\mathit{2}}}$ affect the qualitative behaviour and properties of the system.

According to Theorem 3.1, whenever the values of ${I_{\mathit{1}}}$ and ${I_{\mathit{2}}}$ are greater than 1 will directly affect the status of the relationship between the ideologies. $\delta$ is set as a bifurcation parameter. ${\delta _{\mathit{1}}}$ and ${\delta _{\mathit{2}}}$ are the bifurcation value of $\delta$ that respectively make ${I_{\mathit{1}}}$ and ${I_{\mathit{2}}}$ equal to 1. Then

Suppose ${R_{\mathit{1}}} > \mathit{1}$, then $x_{\mathit{1}}^*$ exists and ${I_{\mathit{2}}} > \mathit{0}$. A sufficient (but not necessary) condition for ${\delta _{\mathit{2}}}$ to be positive is:

If $\delta = {\delta _{\mathit{2}}} > \mathit{0}$, then ${I_{\mathit{2}}} = 1$, and the Jacobian at $x_{\mathit{1}}^*$ has eigenvalue 0. If $\delta$ increases to ${\delta _{\mathit{2}}}$, then $x_{\mathit{1}}^*$ stability changes. This results in the condition where the only value of $\delta$ that could lead to a coexistence equilibrium and have a bifurcation interaction with $x_{\mathit{1}}^*$.

Suppose ${R_{\mathit{2}}} > \mathit{1}$, then $x_{\mathit{2}}^*$ exists and ${I_{\mathit{1}}} > \mathit{0}$. A sufficient (but not necessary) condition for ${\delta _{\mathit{1}}}$ to be positive is:

If $\delta = {\delta _{\mathit{1}}} > \mathit{0}$, then ${I_{\mathit{1}}} = 1$ and the Jacobian at $x_{\mathit{2}}^*$ has eigenvalue 0. If $\delta$ increases to ${\delta _{\mathit{1}}}$, then $x_{\mathit{2}}^*$ stability changes. This is the only value of $\delta$ that could lead to a coexistence equilibrium that has a bifurcation interaction with $x_{\mathit{2}}^*$.

Situation 1: $1 < {R_{\mathit{1}}} < {R_{\mathit{2}}}$. Then:

$x_{\mathit{1}}^*$ and $x_{\mathit{2}}^*$ are both existing. For $\delta = \mathit{0}$, ${I_{\mathit{2}}} = \frac{{{R_{\mathit{2}}}}}{{{R_{\mathit{1}}}}} > \mathit{1}$ and so $x_{\mathit{1}}^*$ is unstable. ${I_{\mathit{1}}} = \frac{{{R_{\mathit{1}}}}}{{{R_{\mathit{2}}}}} < \mathit{1}$ and so $x_{\mathit{2}}^*$ locally asymptotically stable.

Since ${I_{\mathit{1}}}$ and ${I_{\mathit{2}}}$ are monotonic functions of $\delta$, it follows that ${I_{\mathit{2}}} > \mathit{1}$ for all $\delta$, so that $x_{\mathit{1}}^*$ is unstable, and the second ideology is always accessible at the equilibrium point $x_{\mathit{1}}^*$. ${I_{\mathit{1}}}$ increases with an increase of $\delta$. When $\delta$ increases from a minimum to a certain maximum, ${I_{\mathit{1}}}$ changes from less than 1 to greater than 1. Since ${\delta _{\mathit{1}}}$ may be positive or negative, consider the following:

Case 1A: Suppose that ${\delta _{\mathit{1}}} < \mathit{0}$, then ${I_{\mathit{1}}} > \mathit{1}$ and for all $\delta$ $x_{\mathit{2}}^*$ is unstable, so near $x_{\mathit{2}}^*$ the first ideology can always be available, and there is a coexistence equilibrium and locally asymptotically stable.

Case 1B: Suppose that ${\delta _{\mathit{1}}} > \mathit{0}$, if $0 < \delta < {\delta _{\mathit{1}}}$, then ${I_{\mathit{1}}} < \mathit{1}$ and $x_{\mathit{2}}^*$ is locally asymptotically stable, and the first ideology can not enter near $x_{\mathit{2}}^*$. If $\delta > {\delta _{\mathit{1}}}$, then ${I_{\mathit{1}}} > \mathit{1}$ and $x_{\mathit{2}}^*$ are unstable, and the first ideology can always enter near $x_{\mathit{2}}^*$, and there is a coexistence equilibrium and locally asymptotically stable.

Situation 2: $1 < {R_{\mathit{2}}} < {R_{\mathit{1}}}$. Then:

Where $x_{\mathit{1}}^*$ and $x_{\mathit{2}}^*$ are both existing. For $\delta = \mathit{0}$, ${I_{\mathit{2}}} = \frac{{{R_{\mathit{2}}}}}{{{R_{\mathit{1}}}}} < \mathit{1}$ and thus $x_{\mathit{1}}^*$ is locally asymptotically stable. ${I_{\mathit{1}}} = \frac{{{R_{\mathit{1}}}}}{{{R_{\mathit{2}}}}} > \mathit{1}$ and thus $x_{\mathit{2}}^*$ is unstable.

Since ${I_{\mathit{1}}}$ and ${I_{\mathit{2}}}$ are monotonic functions of $\delta$, it follows that ${I_{\mathit{1}}} > \mathit{1}$ is for all $\delta$, thus $x_{\mathit{2}}^*$ is unstable, and the first ideology is always accessible at the equilibrium point $x_{\mathit{2}}^*$. ${I_{\mathit{2}}}$ increases with an increase of $\delta$. When $\delta$ increases from a minimum to a certain maximum, ${I_{\mathit{2}}}$ changes from less than 1 to greater than 1. Since ${\delta _{\mathit{2}}}$ may be positive or negative, consider the following:

Case 2A: Suppose ${\delta _{\mathit{2}}} < \mathit{0}$, then ${I_{\mathit{2}}} > \mathit{1}$ and $x_{\mathit{1}}^*$ is unstable for all $\delta$, and the second ideology can always enter near $x_{\mathit{1}}^*$, and there is a coexistence equilibrium and locally asymptotically stable.

Case 2B: Suppose ${\delta _{\mathit{2}}} > \mathit{0}$, if $0 < \delta < {\delta _{\mathit{2}}}$, then ${I_{\mathit{2}}} < \mathit{1}$ and $x_{\mathit{1}}^*$ is locally asymptotically stable, and the second ideology can not enter near $x_{\mathit{1}}^*$. If $\delta > {\delta _{\mathit{2}}}$, then ${I_{\mathit{2}}} > \mathit{1}$ and $x_{\mathit{1}}^*$ is unstable, and the second ideology is always able to enter near $x_{\mathit{1}}^*$, and there is a coexistence equilibrium and locally asymptotically stable.

Situation 3: ${R_{\mathit{2}}} < \mathit{1} < {R_{\mathit{1}}}$. Under this assumption we have:

$x_{\mathit{1}}^*$ exists and thus ${I_{\mathit{2}}}$ is defined and is positive. For $\delta = \mathit{0}$, ${I_{\mathit{2}}} = \frac{{{R_{\mathit{2}}}}}{{{R_{\mathit{1}}}}} < \mathit{1}$ and $x_{\mathit{1}}^*$ are locally asymptotically stable. When ${\delta _{\mathit{2}}} > \mathit{0}$ then as $\delta$ increases, when $0 < \delta < {\delta _{\mathit{2}}}$, ${I_{\mathit{2}}} < \mathit{1}$ and $x_{\mathit{1}}^*$ are locally asymptotically stable. When $\delta > {\delta _{\mathit{2}}}$, ${I_{\mathit{2}}} > \mathit{1}$ and $x_{\mathit{1}}^*$ are unstable, and there is a coexistence equilibrium and locally asymptotically stable.

Situation 4: ${R_{\mathit{1}}} < \mathit{1} < {R_{\mathit{2}}}$. Then:

$x_{\mathit{2}}^*$ is existing and thus ${I_{\mathit{1}}}$ is positive. For $\delta = \mathit{0}$, ${I_{\mathit{1}}} = \frac{{{R_{\mathit{1}}}}}{{{R_{\mathit{2}}}}} < \mathit{1}$ and $x_{\mathit{2}}^*$ are locally asymptotically stable. When ${\delta _{\mathit{1}}} > \mathit{0}$ then as $\delta$ increases, then $0 < \delta < {\delta _{\mathit{1}}}$, so ${I_{\mathit{1}}} < \mathit{1}$ and $x_{\mathit{2}}^*$ are locally asymptotically stable. When $\delta > {\delta _{\mathit{1}}}$, ${I_{\mathit{1}}} > \mathit{1}$ and $x_{\mathit{2}}^*$ are unstable, and there is a locally asymptotically stable coexistence equilibrium.

6.   Global stability

Theorem 6.1. If ${R_{\mathit{1}}}, {R_{\mathit{2}}} \leqslant 1$, then ${x_{\mathit{0}}}$ is globally asymptotically stable.

Proof. The Jacobian matrix at ${x_{\mathit{0}}}$ of (1) is:

The characteristic polynomial is

where

Since $0 < {q_{{D_{\mathit{1}}}}} < \mathit{1}$, and when ${R_{\mathit{1}}} \leqslant 1$, then ${Q_\mathit{11}} > \mathit{0}$ and ${Q_\mathit{12}} > \mathit{0}$. Similarly, ${Q_\mathit{21}} > \mathit{0}$ and ${Q_\mathit{22}} > \mathit{0}$. We get 5 eigenvalues, respectively:

If ${R_{\mathit{1}}}, {R_{\mathit{2}}} \leqslant 1$, then ${\lambda _{\mathit{2}}}, {\lambda _{\mathit{3}}}, {\lambda _{\mathit{4}}}, {\lambda _{\mathit{5}}}$ are negative or have negative real parts, so ${x_{\mathit{0}}}$ is globally asymptotically stable.

Theorem 6.2. If ${R_{\mathit{1}}} > \max \left\{ {1, {R_{\mathit{2}}}} \right\}$, suppose that:

$\frac{{D_{\mathit{1}}^*}}{{{D_{\mathit{1}}}}} > \frac{{C_{\mathit{1}}^*}}{{{C_{\mathit{1}}}}}\frac{{{c_{{C_{\mathit{1}}}}}}}{{\mu + {d_{{C_{\mathit{1}}}}} + {c_{{C_{\mathit{1}}}}}}} + \frac{{\mu + {d_{{C_{\mathit{1}}}}}}}{{\mu + {d_{{C_{\mathit{1}}}}} + {c_{{C_{\mathit{1}}}}}}} + \frac{{\mu + {d_{{C_{\mathit{2}}}}}}}{{{c_{{C_{\mathit{2}}}}} + {q_{{D_{\mathit{2}}}}}\left( {\mu + {d_{{C_{\mathit{2}}}}}} \right)}}\frac{{{c_{{C_{\mathit{1}}}}} + {q_{{D_{\mathit{1}}}}}\left( {\mu + {d_{{C_{\mathit{1}}}}}} \right)}}{{\mu + {d_{{C_{\mathit{1}}}}} + {c_{{C_{\mathit{1}}}}}}}$, then $x_{_{\mathit{1}}}^*$ is globally asymptotically stable.

If ${R_{\mathit{2}}} > \max \left\{ {1, {R_{\mathit{1}}}} \right\}$, suppose

$\frac{{D_{\mathit{2}}^*}}{{{D_{\mathit{2}}}}} > \frac{{C_{\mathit{2}}^*}}{{{C_{\mathit{2}}}}}\frac{{{c_{{C_{\mathit{2}}}}}}}{{\mu + {d_{{C_{\mathit{2}}}}} + {c_{{C_{\mathit{2}}}}}}} + \frac{{\mu + {d_{{C_{\mathit{2}}}}}}}{{\mu + {d_{{C_{\mathit{2}}}}} + {c_{{C_{\mathit{2}}}}}}} + \frac{{\mu + {d_{{C_{\mathit{1}}}}}}}{{{c_{{C_{\mathit{1}}}}} + {q_{{D_{\mathit{1}}}}}\left( {\mu + {d_{{C_{\mathit{1}}}}}} \right)}}\frac{{{c_{{C_{\mathit{2}}}}} + {q_{{D_{\mathit{2}}}}}\left( {\mu + {d_{{C_{\mathit{2}}}}}} \right)}}{{\mu + {d_{{C_{\mathit{2}}}}} + {c_{{C_{\mathit{2}}}}}}}$, then $x_{_{\mathit{2}}}^*$ is globally asymptotically stable.

Proof. First it is necessary to analyze the stability of $x_{_{\mathit{2}}}^*$, and refer to ^[4] to construct the Lyapunov function.

where

At $x_{_{\mathit{2}}}^*$, since $E_{\mathit{2}}' = \mathit{0}$, we get ${A_{\mathit{1}}} = \frac{{{c_{{C_{\mathit{2}}}}}}}{{{c_{{C_{\mathit{2}}}}} + {q_{{D_{\mathit{2}}}}}\left( {\mu + {d_{{C_{\mathit{2}}}}}} \right)}}$, ${A_{\mathit{2}}} = \frac{{\mu + {d_{{C_{\mathit{2}}}}} + {c_{{C_{\mathit{2}}}}}}}{{{c_{{C_{\mathit{2}}}}} + {q_{{D_{\mathit{2}}}}}\left( {\mu + {d_{{C_{\mathit{2}}}}}} \right)}}$.

Suppose $\left( {s, {c_{\mathit{2}}}, {d_{\mathit{2}}}} \right) = \left( {\frac{S}{{S_{\mathit{2}}^*}}, \frac{C}{{C_{\mathit{2}}^*}}, \frac{D}{{D_{\mathit{2}}^*}}} \right)$, we get:

where

Thus, if ${R_{\mathit{2}}} > \max \left\{ {1, {R_{\mathit{1}}}} \right\}$ and $\frac{{D_{\mathit{1}}^*}}{{{D_{\mathit{1}}}}} > \frac{{C_{\mathit{1}}^*}}{{{C_{\mathit{1}}}}}\frac{{{c_{{C_{\mathit{1}}}}}}}{{\mu + {d_{{C_{\mathit{1}}}}} + {c_{{C_{\mathit{1}}}}}}} + \frac{{\mu + {d_{{C_{\mathit{1}}}}}}}{{\mu + {d_{{C_{\mathit{1}}}}} + {c_{{C_{\mathit{1}}}}}}} + \frac{{\mu + {d_{{C_{\mathit{2}}}}}}}{{{c_{{C_{\mathit{2}}}}} + {q_{{D_{\mathit{2}}}}}\left( {\mu + {d_{{C_{\mathit{2}}}}}} \right)}}\frac{{{c_{{C_{\mathit{1}}}}} + {q_{{D_{\mathit{1}}}}}\left( {\mu + {d_{{C_{\mathit{1}}}}}} \right)}}{{\mu + {d_{{C_{\mathit{1}}}}} + {c_{{C_{\mathit{1}}}}}}}$, as the arithmetic mean-geometric mean inequality, it follows that ${W'} \leqslant 0$. If and only if $S = S_{\mathit{2}}^*, {D_{\mathit{1}}} = \mathit{0}, {C_{\mathit{1}}} = \mathit{0}$ and ${W'} = \mathit{0}$, then the maximum invariant set where $S$ remains constant at $S_{\mathit{2}}^*$ and ${C_{\mathit{1}}}, {D_{\mathit{1}}}$ remains constant at 0 consists only of the equilibrium $x_{_{\mathit{2}}}^*$, so $x_{_{\mathit{2}}}^*$ is globally asymptotically stable. Similarly, it can be proved that $x_{_{\mathit{1}}}^*$ is globally asymptotically stable.

7.   Numerical simulation

In this section, the mathematical analysis results are verified by numerical simulation.

First, the parameter settings are shown in Table 2 and the basic reproduction numbers ${R_{\mathit{1}}} = \mathit{0.6154} < \mathit{1}$, ${R_{\mathit{2}}} = \mathit{0.5979} < \mathit{1}$ are gotten. The condition of Theorem 2.1 is now satisfied, and ${x_{\mathit{0}}}$ is locally asymptotically stable. Figure 2 shows the local stability of ${x_{\mathit{0}}}$.

7.1.   Cross-interaction of cooperation promotes the survival of ideology

Make ${\beta _{\mathit{1}}} = \mathit{0.000005}$, ${d_{{D_{\mathit{2}}}}} = \mathit{0.003}$, while all other parameter settings remain unchanged, then ${R_{\mathit{1}}} = \mathit{3.0769} > \mathit{1}$, ${R_{\mathit{2}}} = \mathit{0.8558} < \mathit{1}$. The condition of Situation 3 is now satisfied, and when $\delta = \mathit{0}$, $x_{\mathit{1}}^*$ is locally asymptotically stable. Figure 3 shows the local stability of $x_{\mathit{1}}^*$. Increasing the value of $\delta$ results in a coexistence equilibrium as shown in Figure 4.

Make ${\beta _{\mathit{1}}} = \mathit{0.000005}$, ${\beta _{\mathit{2}}} = \mathit{0.000003}$, ${q_{{C_{\mathit{2}}}}} = \mathit{0.86}$, ${q_{{D_{\mathit{2}}}}} = \mathit{0.14}$, and all other parameter settings remain unchanged, we get ${R_{\mathit{1}}} = \mathit{3.0769} > {R_{\mathit{2}}} = \mathit{1.7937} > \mathit{1}$. The condition of Situation 2 is now satisfied, and when $\delta = \mathit{0}$, $x_{\mathit{1}}^*$ is locally asymptotically stable. Increasing the value of $\delta$ results in coexistence equilibrium, as shown in Figure 5.

In the above sets of simulations, the two ideologies have achieved a coexistence equilibrium. Especially in Figure 3 (${R_{\mathit{1}}} = \mathit{3.0769} > \mathit{1}$, ${R_{\mathit{2}}} = \mathit{0.8558} < \mathit{1}$), the stable coexistence of the two ideologies is still achieved. It can be seen that the cooperation mechanism provides more opportunities for the continued existence of the two ideologies.

7.2.   Cooperative cross-interaction weakens the effectiveness of de-radicalization measures

The parameters ${d_{{D_{\mathit{1}}}}}$ and ${d_{{D_{\mathit{2}}}}}$ in the model could simulate the depolarized strike strategy, and ${\beta _{\mathit{1}}}$ and ${\beta _{\mathit{2}}}$ could simulate the depolarized prevention strategy. So based on the parameter setting in Figure 3, and increase ${d_{{D_{\mathit{1}}}}}$, the results are shown in Figure 6. When ${\beta _{\mathit{1}}}$ is decreased, the results are shown in Figure 7.

Based on the parameter setting in Figure 5, increase ${d_{{D_{\mathit{1}}}}}$ and ${d_{{D_{\mathit{2}}}}}$ respectively, leading to the results shown in Figure 8. When ${\beta _{\mathit{1}}}$ and ${\beta _{\mathit{2}}}$ are decreased respectively, the results are shown in Figure 9.

Under the cooperative mechanism, the attractiveness and resistance of ideology have both increased. When one ideology had a strong ability to spread and thus dominated the setting (${R_{\mathit{1}}} = \mathit{3.0769} > \mathit{1}$), the second ideology had a weaker communication ability (${R_{\mathit{2}}} = \mathit{0.8558} < \mathit{1}$), supporters of the second ideology would be attracted under the influence of cooperation. Simply increasing ${d_{{D_{\mathit{1}}}}}$ / ${d_{{D_{\mathit{2}}}}}$ or decreasing ${\beta _{\mathit{1}}}$ / ${\beta _{\mathit{2}}}$ could have a certain inhibitory effect on the dominant ideology. But the weaker ideology attracts more supporters. When both ideologies had strong ability to spread (${R_{\mathit{1}}} = \mathit{3.0769} > \mathit{1}$, ${R_{\mathit{2}}} =\mathit{ 0.8558 }< \mathit{1}$), simply suppressing or preventing one ideology from attracting followers would not serve as an effective inhibitory effect.

8.   Conclusions

This model aimed at the ideological practice strategy of terrorist organizations constructing multiple ideologies in order to attract more supporters, and explored the mechanism of the overlapping infection of multiple ideologies on the individual radicalization. Although our model was simple, it revealed to a certain extent the mechanism of multiple ideological overlap infections in the process of radicalization.

Our model got valuable conclusions: First of all that with the cross-interaction of cooperation, the coexistence equilibrium of the two ideologies persisted universally. When the coexistence equilibrium was locally asymptotically stable, any ideology was difficult to eliminate.

Secondly, under the cooperation mechanism, the probability that either ideology persisted will increase, which meant that the cooperation mechanism provided more opportunities for the continued existence of the two ideological components. As long as the basic reproduction number for either ideology was greater than 1, whether or not the basic reproduction number of the other ideology was greater than 1, it was possible to achieve stable coexistence. This circumstance made the fight against violent extremism more difficult.

In addition, in terms of de-radicalization strategies, the strategy for a single ideology was not suitable for the suppression of multiple ideologies under the cooperative mechanism. The parameters ${d_{{D_{\mathit{1}}}}}$ and ${d_{{D_{\mathit{2}}}}}$ in the model could simulate the depolarized strike strategy, and ${\beta _{\mathit{1}}}$ and ${\beta _{\mathit{2}}}$ could simulate the depolarized prevention strategy. In the model with a single ideological component, increasing ${d_{{D_{\mathit{1}}}}}$ and ${d_{{D_{\mathit{2}}}}}$ and decreasing ${\beta _{\mathit{1}}}$ and ${\beta _{\mathit{2}}}$ could effectively suppress the radicalization process. This model, however, had generated new results. Under the cooperative mechanism, when one ideology had a strong ability to spread and thus dominated the setting (the basic reproduction number was greater than 1), the second ideology had a weaker communication ability (the basic reproduction number was less than 1), supporters of the second ideology would be attracted under the influence of cooperation. Simply increasing the impact of the dominant ideology (increasing ${d_{{D_{\mathit{1}}}}}$ or ${d_{{D_{\mathit{2}}}}}$) or increasing the preventive measures of the dominant ideology (decreasing ${\beta _{\mathit{1}}}$ or ${\beta _{\mathit{2}}}$) could have a certain inhibitory effect on the dominant ideology. For the weaker ideology, however, its attraction for supporters had increased. When both ideologies had strong ability to spread (the basic reproduction numbers for both were greater than 1), simply suppressing or preventing one ideology from attracting followers would not serve as an effective inhibitory effect.

Acknowledgments

Funding source: Beijing Excellent Talents Training Funding Project. The author would like to thank Beijing Municipal Party Committee Organization Department for providing funding of this study.

Conflict of interest

The authors declare no conflict of interest.