The qualitative analysis of solution of the Stokes and Navier-Stokes system in non-smooth domains with weighted Sobolev spaces

1.   Introduction

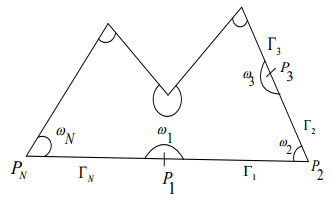
Let $\mathcal{D}\subset\mathbb{R}^{2}$ be a 2-dimensional bounded domain, whose boundary $\partial\mathcal{D}$ comprises the corner points and points at which the type of boundary conditions change. Note that a point $P\in\partial\mathcal{D}$ is said to be a corner point if there exists a neighborhood $\eta(P)$ of $P$ such that $\mathcal{D}\cap\eta(P)$ is diffeomorphic to an angle $\kappa$ intersected with the unit circle. For simplicity, we are considering a bounded plane polygonal domain (see Figure 1) with corner points $(\omega\neq\pi)$ and points $(\omega = \pi)$ where the boundary conditions change. The boundary points where the boundary conditions change are also referred to as corner points or vertices. The obtained results for a polygonal domain can be extended to a 2-dimensional bounded domain, i.e. (Lipschitz continuous) $C^{0, \:1}$ with corner points. We considered one point as a special case of interest of corner points with an angle $\omega = \pi$ on one side of the domain $\mathcal{D}$, where the Neumann boundary condition, the Dirichlet boundary condition, respectively, is prescribed.

For the polygonal domain $\mathcal{D}$ with the vertices $P_{1}, ..., P_{N}$, we introduce the following notations. Let $P_{N+1} = P_{1}$, $\mathcal{J} = \big\{{1}, ..., {N}\big\}$, $\Gamma_{i}\, (i\in \mathcal{J})$ is the open edge connecting the vertices $P_{i+1}$ and $P_{i}$, $\Gamma_{0} = \Gamma_{N}$, and $\omega_{i}\, (i\in \mathcal{J})$ is the interior angle made by $\Gamma_{i-1}, \Gamma_{i}$. Let $\mathcal J_{D} = \big\{i\in \mathcal{J}:$ on $\Gamma_{i}$ the Dirichlet boundary conditions are prescribed$\big\}$ and $\mathcal J_{N} = \big\{i\in \mathcal{J}:$ on $\Gamma_{i}$ the Neumann boundary conditions are prescribed$\big\}$. Let $\mathcal{N}$ denote the set of the boundary points of $\mathcal{D}$, i.e., $\mathcal{N} = \big\{P_{i}\big\}$, $i\in \mathcal{J}$.

We assume that $\mathcal J_{D}$, $\mathcal J_{N}$ are non-empty disjoint sets and $\mathcal{J} = \mathcal J_{D}\cup\mathcal J_{N}$. Moreover, let $\Gamma^{0}$, $\Gamma^{1}$ be given by $\Gamma^{0} = \bigcup_{i\in\mathcal J_{D}}\overline{\Gamma}_{i}$, $\Gamma^{1} = \bigcup_{i\in\mathcal J_{N}}\overline{\Gamma}_{i}$. We have $\Gamma^{0}\cap\Gamma^{1} = \emptyset$ and $\partial\mathcal{D} = \overline{{\Gamma}}^{0}\cup\overline{{\Gamma}}^{1}$.

We consider the Navier-Stokes equations for an incompressible, viscous fluid, i.e.

where $\textbf{u} = ({u}_{1}, {u}_{2})$ is the velocity vector field with the cartesian components ${u}_{1}, {u}_{2}$, $\nu$ is the viscosity parameter of the fluid flow, i.e., ($\nu > 0$), $q$ is the hydrostatic pressure and $\textbf{f}$ is a given volume force density.

If the viscosity coefficient $\nu$ is sufficiently large, then the flow can be described by the following stationary Stokes system on a domain $\mathcal{D}$:

The following mixed boundary conditions are considered on the boundary $\partial\mathcal{D}$:

where $\textbf{n} = ({n}_{1}, {n}_{2})$ is the unit outward normal vector to the boundary and $\mathcal{S}\big[{\bf u}, \, q \big]$ is the hydrostatic stress tensor with the cartesian components

Here, $\delta_{i\, j}$ is the Kronecker symbol. Further note that if the second equation of (1.2) becoming $-{\mbox{div}} \, {\bf u} = g$ for a given function $g$ satisfying the property $\int_{\mathcal{D}} g\, d\mbox{x} = 0$, then a particular regularity of $g$ is required for proving the regularity of the pressure function or for handling the non-zero boundary data. Generally, for incompressible flows the function $g$ is set equal to zero to satisfy the incompressibility condition. For simplicity, we are considering $g$ equal to zero. Therefore, for a smooth boundary, smooth given data and boundary conditions, the system (1.2) has a smooth solution ^[41]. The system (1.2) with the boundary conditions (1.3)-(1.4) is known as the stationary Stokes system with mixed boundary conditions ^[30,36].

The Navier-Stokes equations or even the Stokes equations are solved for Dirichlet boundary conditions ^{[7,10,11,15,17]} but this is not common in some situations like finite channel flow models ^[17,28]. Usually, these boundary conditions are used in the upstream of the channel and on the fixed walls but not downstream of the channel, because the downstream velocity depends on the flow in a channel which is unknown. The situation becomes more intricate when the boundary of the domain has corners or edges and the Neumann boundary conditions are applied on parts of the boundary ^[27,35]. Therefore, the second equation of (1.2) helps to characterize the different types of Neumann boundary conditions with the Green theorem. In numerical methods, the condition (1.4) is used on the downstream boundary ^[13].

Presently, the corner singularity theory has been constructed for compressible viscous Stokes and Navier-Stokes systems for polygonal domains in 2-dimensions and polyhedral domains in 3-dimensions. The mathematical techniques to analyze the singular structure of the solutions near corners, edges, and cusps have been discussed in ^{[12,16,21,22,26,27,37]}. The key point of the corner singularity theory is the decomposition of the solution of the given problem into a regular part and a locally acting singular part which is a linear combination of explicit model singular solutions $s_m$ with unknown coefficients ${c}_{m}$. The special singular functions $s_m$ rely on the geometry of the model problem, the differential operator, and the characteristic boundary conditions.

In the singularity expansion method for the Stokes problem, the spectral problems related to the corner singularities of solutions to elliptic equations were discussed in ^[7,8]. Kweon ^[31] has considered zero Dirichlet boundary conditions to investigate the regularity results of the incompressible Navier-Stokes equations in a non-convex polygonal domain. ^[32,34] have extended these results for a non-convex polyhedral cylinder in $\mathbb{R}^{3}$ with inflow boundary conditions for compressible Navier-Stokes equations. The Helmholtz decomposition to obtain regularity results of the compressible Stokes system in a non-convex polygonal domain with no-slip boundary conditions is used in ^[33]. Recently, Anjam ^[4] has comprehensively discussed the singularities and regularity results of the stationary Stokes and Navier-Stokes equations on polygonal domains with convex or non-convex corners.

This study typically emphasizes analyzing the boundary singularities and regularity results of the stationary Stokes and Navier-Stokes system in two-dimensional non-smooth domains with corner points and points at which the type of boundary conditions change. We use the theory developed by Kondrat$\acute{i}$ev ^[24,25] and further extended by ^[39] for scalar problems in the context of weighted Sobolev spaces. The solvability of the considered boundary value problem is analyzed in the context of these weighted Sobolev spaces and the regularity theorems are formulated. To compute the singular terms for various boundary conditions, the Fourier transform is used to obtain the generalized form of the boundary eigenvalue problem for the stationary Stokes system. The emerging eigenvalues and eigenfunctions produce singular terms, which permits us to evaluate the optimal regularity of the corresponding weak solution of the stationary Stokes system.

The main result for the stationary Stokes system is presented in Theorem 3, and the regularity results that are direct consequences of Theorem 3 are given in Section 4. Moreover, it is proved that the weak solution $({\bf u}, q)$ of the underlying boundary value problem belongs to $W^{2-\gamma, \, 2}\mathcal{(D)}^{2}\times W^{1-\gamma, \, 2}\mathcal{(D)}$, where $\gamma$ is an arbitrarily small positive real number that depends on the apex angle $\omega_{0}$. Additionally, the obtained results for the Stokes system are further extended for the non-linear Navier-Stokes system. It is proved by using the local diffeomorphism theorem that the solution of the Navier-Stokes equations has similar regularity results as the solution of the generalized Stokes problem near the corner points if the norm of the body force is sufficiently small. So far the problem has been ignored with such type of boundary conditions and domain.

The rest of this paper is organized as follows: Section 2 is devoted to present the weak formulation of the Stokes problem and introduce some function spaces. In Section 3, we determine a parametric boundary eigenvalue problem with a complex parameter $\xi$, the stationary Stokes system is being considered for various combinations of Dirichlet, Neumann, and mixed boundary conditions. The main regularity and expansion theorem for the stationary Stokes system is given in Theorem 3. The transcendental equations for various conditions whose zeros are the eigenvalues of the operator $\hat{\mathcal{U}}(\xi)$ are derived. Further, the distribution of eigenvalues and eigenfunctions are discussed. In Section 4, some regularity results for the stationary Stokes system are investigated. The results of Section 4 are further extended for non-linear Navier-Stokes system in Section 5. Section 6 is devoted to conclusions.

2.   Analytical preliminaries

2.1.   The weak formulation of the Stokes problem

In this section, we consider the weak formulation of the stationary Stokes problem (1.2)-(1.4). The variational formulation, solvability, and the uniqueness of the solution are offered. For a weak solution, we have restricted ourself to the homogenous boundary conditions. Denote

where $\mbox{supp}{\bf u} = \big\{{\mbox{x}}\big|\, {\bf u}({\mbox{x}})\neq 0\big\},$ and $\mbox{supp}{\bf u}\, \subset\mathcal{D}$. Let $V^{m, \, p}$ be a closure of $\mathcal{E}(\mathcal{D})$ in the norm of $W^{m, \, p}(\mathcal{D})^2, \, 1\leq p < \infty$ and $m \geq 0$ ($m$ need not be an integer), it is a Banach space with the norm of $W^{m, \, p}(\mathcal{D})^2$. For simplicity, we represent $V^{0, \, 2}$ and $V^{1, \, 2}$, respectively, as $H$ and $V$. Usually, these spaces are used to find the solution of the Navier-Stokes equations with homogeneous Dirichlet boundary conditions, and they are closed subspaces of the spaces $L^{2}(\mathcal{D})^{2}$ and $W^{1, \, 2}(\mathcal{D})^{2}$. They are Hilbert spaces with the scalar products

Let ${\bf f}\in H$. A pair $({\bf u}, q)\in V\times L^{2}(\mathcal{D})$ is called weak solution for the problem (1.2)-(1.4) if it satisfies

where

Equation (2.2) is the weak formulation of the boundary value problem (1.2)-(1.4), which is obtained by multiplying the first equation of (1.2) by a test function ${\bf v}\in V$ and the second equation by $p\in L^{2}(\mathcal{D})$. The pressure $q$ is a scalar function, such that, the pair $({\bf u}, q)$ satisfy the Eqs (1.2)-(1.4) in domain $\mathcal{D}$ in the sense of distributions. The bilinear form $a(., .)$ is elliptic and continuous, whereas the bilinear form $b(., .)$ is continuous and verifies the inf-sup condition (see ^[15,20,41]). It is proved in ^[6,38] that for every ${\bf f}\in H$, there exists a unique weak solution $({\bf u}, q)$ of the boundary value problem (1.2)-(1.4) and for it the following estimate holds:

where $c = c(\mathcal{D})$. Hence, we have to analyze the smoothness of the weak solution $({\bf u}, q)$ and see how it depends on the sizes of the angles $\omega_{i}, \, i = 1, ..., N$.

Remarks 1. If the given data on the right-hand sides of (1.2)-(1.4) are smoother, for example, ${\bf f}\in L^{2}(\mathcal{D})^{2}$, ${\bf h}_{1}\in [H^{\frac{3}{2}}(\Gamma^{0})]^{2}$ and ${\bf h}_{2}\in [H^{\frac{1}{2}}(\Gamma^{1})]^{2}$, if the domain is sufficiently smooth and the boundary conditions do not change their types, then it is proved (see ^[41]) that the weak solution $({\bf u}, q)\in [H^{2}(\mathcal{D})]^{2}\times[H^{1}(\mathcal{D})]$. On the other hand, the same regularity result does not hold, if the domain has corner points or points at which the type of boundary conditions changes (see ^[16,23]). As a matter of fact, in these cases, the regularity can be described by a decomposition of the two-dimensional solution field

into singular and regular parts of the form

Here, the regular part ${\bf u}_{reg}$ belongs to $[H^{2}(\mathcal{D})]^{2}\times[H^{1}(\mathcal{D})]$, the corner points are shown by $k$ with the equivalent polar coordinates $({r_{k}}, \, {\theta_{k}})$, the exponents ${\xi_{j, \, k}}$ are the eigenvalues of a Sturm-Liouville problem, and $\Phi_{j, \, k}$ are the corresponding generalized eigenvector fields.

2.2.   Weighted Sobolev spaces

To investigate the regularity results of the weak solution $({\bf u}, q)$ of the corresponding boundary value problem, firstly, we introduce some function spaces in line with ^[1,14,24,39].

Let $\mathcal{N}$ be the set of the corner points and of points where the type of boundary conditions change (shortly called the singular boundary points), i.e., $\mathcal{N}\subset\partial\mathcal{D}$. Denote

where the $\mbox{supp}\, {v}$ is bounded. Let $\alpha = \big(\alpha_{1}, ..., \alpha_{N}\big)$ be an $N-$tuple of real numbers which satisfying $0 < \alpha_{i} < 1$ for $1 \le i \le N$. Therefore, the weight function is characterized by

where $m$ is an any integer and $r_{i}(x) = \mbox{dist}\, (x, P_{i})$. We denote by ${D}^{\beta}{v}$ be the multi-index notation for higher-order derivatives and in cartesian coordinates is defined as

Let $\mathcal {W}_{\alpha}^{m, \, p}\mathcal{(D)}$ be the weighted Sobolev spaces and is the closure of $C_{\mathcal{N}}^{\infty}\mathcal{(D)}$ equipped with the norm

Analogously to the factor spaces, the trace spaces are also defined as

where $\mathcal {W}_{0, \, \alpha}^{m, \, p}(\mathcal{D})$ is the closure of $C_{0}^{\infty}(\mathcal{D})$ with respect to the norm of $\mathcal {W}_{\alpha}^{m, \, p}(\mathcal{D})$. This approach is classical for domains with conical points. Principally, for $1\leq p < \infty$, we denote $L_{\alpha}^{p}(\mathcal{D}) = \mathcal {W}_{\alpha}^{0, \, p}(\mathcal{D})$.

For negative integers $m$, i.e., $m\in\mathbb{Z}, \, m < 0$, we describe the spaces $\mathcal {W}_{\alpha}^{m, \, p}(\mathcal{D})$ as the closure of the set $C_{\mathcal{N}}^{\infty}(\mathcal{D})$ equipped with the subsequent norm

where $q = \frac{p}{p-1}$ is known as the inverse of $p$. The dual space of $\mathcal {W}_{\alpha}^{m, \, p}(\mathcal{D})$ is given as $\mathcal {W}_{-\alpha}^{-m, \, q}(\mathcal{D})$. Similarly for the trace spaces, defining the space $\mathcal {W}_{\alpha}^{m+\frac{1}{q}, \, p}{(\partial \mathcal{D})}$ for $m < 0, \, m\in\mathbb{Z}$, as the dual space to $\mathcal {W}_{-\alpha}^{-m-\frac{1}{q}, \, q}{(\partial \mathcal{D})}$. Therefore, the consequent continuous imbeddings are considered directly from the definition of the above spaces

For a bounded plane domain $\mathcal{D}$, we have the subsequent continuous imbeddings

and

provided that $p_{2}\geq p_{1}$ and $\alpha_{2}+\frac{2}{p_{2}} < \alpha_{1}+\frac{2}{p_{1}}$.

Let $Q = \big\{(\tau, \theta):-\infty < \tau < \infty, \, 0 < \theta < \omega_{0}\big\}$ denote the infinite strip with positive width $\omega_{0}$. For any real $h > 0$ and for an integer $m\geq0$, the spaces are defined as

where

3.   Singularities of the Stokes problem in an infinite cone

In this section, we will see the occurrence of the singular terms of the solution of the mixed boundary value problem for the stationary Stokes problem near the corners and the structure which they have. Further, the distribution of the eigenvalues and eigenfunctions are given.

3.1.   Localization and the model problem

Assume that $\mathcal{D}$ is a polygonal domain. To show that the weak solution $({\bf u}, q)$ of the underlying boundary value problem is regular, we have to investigate its behaviour near the corner points $P_{i}\, (i\in \mathcal{J})$. Let us consider the point $P_{N}$ as origin and denote $\omega_{N} = \omega_{0}\in (0, 2\pi)$. An appropriate infinite differentiable cut-off function $\chi(\vert \mbox{x} \vert) = \chi(r)$ depending on the distance $r$ from the point $P_{N}$ is defined as

The number $\epsilon$ is so small that $P_{N}$ is the only corner point of the domain $\mathcal{D}$ that lies inside the circle $\{\mbox{x}:\vert \mbox{x} \vert\leq 2\epsilon\}$. We multiply the both sides of (1.2) and (1.3)-(1.4) by the smooth cut-off function $\chi$, then substitute $({\bf v}, \, p) = (\chi{\bf u}, \, \chi q)$ in (1.2) and likewise in (1.3)-(1.4). The derivatives are considered in the distribution sense. Thus, the boundary value problem is set into an infinite cone

and coincides with the original problem near the $P_{N}$. The Stokes system (1.2) becomes

where ${\bf F} = \chi\, {{\bf f}}-2\nu\nabla\chi\cdot\nabla{\bf u}-\nu\, {\bf u}\, \Delta\chi+q\, \nabla\chi$ and $G = {\bf u}\cdot\nabla\chi$. The behavior of $({\bf v}, p)$ near the corner point $P_{N}$ illustrates the regularity of the solution $({\bf u}, q)$ in the neighborhood of the point $P_{N}$. If we suppose that the right-hand side in (1.2) is ${\bf f}\in L^{2}(\mathcal{D})^{2}$, then ${\bf F}\in L^{2}(S)^{2}$ and $G\in H^{1}(S)$. Besides, the following boundary conditions are prescribed on the subsequent edges $\Gamma_{S, \, 0}\, (\theta = 0)$ and $\Gamma_{S, \, \omega_{0}}\, (\theta = \omega_{0})$ of the cone (see Figure 2). Just one condition is considered per edge to differentiate between the mixed boundary conditions. Therefore, the obtained boundary conditions are:

Dirichlet boundary conditions:

where $\chi {\bf h}_{1} = {\bf H}_{1}$.

Neumann boundary conditions:

where $\chi\, {\bf h}_{2}+ \nu\, {\bf n}\big(\nabla\, \chi\cdot{\bf u}+{\bf u}\cdot\chi\big) = {\bf H}_{2}$, and the notation $(\cdot)$ denotes the vector direct product between two vectors.

Mixed boundary conditions:

It is observed that the right-hand sides of the obtained boundary conditions have similar smoothness as the original problem in the domain $\mathcal{D}$. To analyze the regularity results of the boundary value problem (3.1)-(3.4), we rewrite the operators in polar coordinates. Hence, the transformed form is

where $(v_{r}, v_{\theta})$ are the polar components of the velocity vector $\overline{{\bf v}}$, $(F_{r}, F_{\theta})$ are the polar components of $\overline{{\bf F}}$ and are given by

Correspondingly, the boundary conditions (3.2)-(3.4) emerge as

and $\overline{{\bf H}}^{m} = (\overline{{H}}_{r}^{m}, \overline{{H}}_{\theta}^{m})^{T}$, where $m = 1$ for Dirichlet and $m = 2$ for Neumann boundary conditions. They hold in the infinite cone where $\overline{{\bf v}}(r, \theta) = {\bf v}(x_{1}, x_{2}), \, \overline{{p}}(r, \theta) = {p}(x_{1}, x_{2}), \, \overline{{\bf F}}(r, \theta) = {\bf F}(x_{1}, x_{2})$ and $\overline{{G}}(r, \theta) = {G}(x_{1}, x_{2})$.

Now, the variable $\tau$ is introduced by the relation $r = e^{\tau}$. Accordingly, the system (3.5) is set on the infinite strip with width $\omega_{0}$ as

Here, $\bar{S} = \big\{(\tau, \theta):-\infty < \tau < \infty, \, 0 < \theta < \omega_{0}\big\}$ and $\tilde{{\bf v}} = \overline{{{\bf v}}}(e^{\tau}, \theta), \, \tilde{{p}} = e^{\tau}\, \overline{{{p}}}(e^{\tau}, \theta), \, \tilde{{\bf F}} = e^{2\tau}\, \overline{{\bf F}}(e^{\tau}, \theta)$ and $\tilde{G} = e^{\tau}\, \overline{{G}}(e^{\tau}, \theta)$. The Dirichlet, Neumann and mixed boundary conditions also yield the transformed form with the boundary data $\widetilde{{\bf H}}^{l+1} = e^{l\tau}\overline{{\bf H}}^{l+1}(e^{\tau}, \theta), \, l = 0, 1$ as

To obtain the boundary eigenvalue value problem, the complex Fourier transform with respect to $\tau$ is introduced as

and the inverse Fourier transform is

It defines an isomorphic mapping, i.e.,

for $\xi = s+ih$, where $h = \mbox{constant}$, $\mathbb{R}+ih = \Big\{\xi\in\mathbb{C}: \mbox{Im}\, \xi = h\Big\}$. Therefore, the subsequent Parseval identity holds

We have

Moreover, it is noted that if $h_{1} < h_{2}$ and the following properties are satisfied

then $\hat{{\bf v}}(\xi)$ is holomorphic in the strip $h_{1} < \mbox{Im}\, \xi < h_{2}$. Therefore, the relationship between the Fourier transform and the Mellin transform for any $\alpha\in\mathbb{C}$ is given by

Now, by applying (3.13) to (3.9)-(3.8) with respect to $\tau$, the two-point boundary value problem for the unknown functions $(\hat{v}_{\tau}, \hat{v}_{\theta}, \hat{p})$ is obtained. It depends on the complex parameter $\xi$ and holds on the interval $I = (0, \omega_{0})$. The transformed form of the problem (3.9) is given by

For complex parameter $\xi$, we have $\hat{{\bf v}}\in W^{2, \, 2}(I)^{2}$, $\hat{p}\in W^{1, \, 2}(I)$, $\hat{{\bf F}}\in L^{2}(I)^{2}$ and $\hat{G}\in W^{1, \, 2}(I)$. Let $\hat{L}(\xi)$ denote the matrix differential operator analogous to the system (3.19) and maps $W^{2, \, 2}(I)^{2}\times W^{1, \, 2}(I)\rightarrow L^{2}(I)^{2}\times W^{1, \, 2}(I)$. Therefore, one has

where

The operator $\hat{L}(\xi)$ is considered for all parameter $\xi\in\mathbb{C}$ with various combinations of the boundary conditions to analyze the qualitative properties of the solution of the underlying problem near the corner points. Additionally, the Fourier transformed form of the boundary conditions is also expressed as follows:

Additionally, the matrix boundary operators for different kinds of boundary conditions can be written as:

For Dirichlet boundary conditions

For Neumann boundary conditions

For mixed boundary conditions

Analogously, the operator $\hat{B}_{[.\, .]}(\xi)$ is used below to define the general transformed form of the matrix boundary operators for different kinds of boundary conditions

Accordingly, the generalized form of the operator pencil $\hat{\mathcal{U}}(\xi)$ for the two-point boundary value problem can be written as

Thus, the operator $\hat{\mathcal{U}}(\xi)$ maps $W^{2, \, 2}(I)^{2}\, \times W^{1, \, 2}(I)$ into $L^{2}(I)^{2}\times W^{1, \, 2}(I)\times \mathbb{C}^{2}\times \mathbb{C}^{2}$. Note that $\hat{\mathcal{U}}(\xi)$ can be defined for every boundary point in the sense of ^[2,3]. So, $\hat{\mathcal{U}}(\xi)(\theta, \, \xi) = 0$ is used to describe a generalized eigenvalue problem and the solvability of these type of problems is discussed in ^[26]. The operator $\hat{\mathcal{U}}(\xi)$ realizes an isomorphism for all $\xi\in\mathbb{C}$ apart from some isolated points (known as the eigenvalues of $\hat{\mathcal{U}}(\xi)$). So, the resolvent $\mathcal{R}(\xi) = \big[\hat{\mathcal{U}}(\xi)\big]^{-1}$ is an operator-valued, meromorphic function of $\xi$ has poles of finite multiplicity. The eigenvalues of $\hat{\mathcal{U}}(\xi)$ are obtaining with the determinant method, which means that the nontrivial solution of the generalized eigenvalue problem leads a transcendental equation whose zeros are the eigenvalues of $\hat{\mathcal{U}}(\xi)$.

Besides, the properties of the operator $\hat{\mathcal{U}}(\xi)$ in the neighborhood of the corner points can be obtained by the properties of the special operator ${\hat{\mathcal{U}}_0}(\xi)$ which is explicitly given by the principal parts of the matrix differential operator $\hat{L}(\xi)$ and the matrix boundary operators $\hat{B}_{[.\, .]}(\xi)$. Hence, we have

and

Likewise, we can write for mixed boundary conditions. To compute the eigenvalues $\xi_{\mu}$ (generally referred for multiple eigenvalues) and the corresponding eigenfunctions, we proceed as.

Definition 1. A complex number $\xi = \xi_{0}$ is known as the eigenvalue of $\hat{\mathcal{U}}(\xi)$ if there exists a nontrivial solution i.e., $\hat{{u}}(., \xi_{0})\neq 0,$ which is holomorphic at $\xi_{0}$, and $\hat{\mathcal{U}}(\xi_{0})\, \hat{{u}}(\theta, \xi_{0}) = 0$. $\hat{{u}}(\theta, \xi_{0})$ is called an eigenfunction of $\hat{\mathcal{U}}(\xi_{0})$ corresponding to the eigenvalue $\xi_{0}$. The set of fields $\big\{\hat{u}_{0}(\theta, \xi_{0}), \, \hat{u}_{0, 1}(\theta, \xi_{0}), ..., \, \hat{u}_{0, s}(\theta, \xi_{0})\big\}$ with $\hat{u}_{0, 0} = \hat{u}_{0}$ is said to be a Jordan chain corresponding to the eigenvalue $\xi_{0}$, if the equation

is satisfied. The number $s+1$ is called the length of the Jordan chain.

Remarks 2. It is noted ^[24,25,26] that if the complex number $\xi$ is not an eigenvalue of the operator $\hat{\mathcal{U}}(\xi)$, then $\hat{\mathcal{U}}(\xi)$ is an isomorphism between the spaces $W^{2, \, 2}(I)^{2}\times W^{1, \, 2}(I)$ and $L^{2}(I)^{2}\times W^{1, \, 2}(I)\times \mathbb{C}^{2}\times \mathbb{C}^{2}$.

Lemma 1. Let $l_h = \{\xi\in\mathbb{C}: \mathrm{Im}\, \xi = h\}$. If no eigenvalues of $\hat{\mathcal{U}}(\xi)$ lies on the line $l_h$, then the system (3.19) and (3.22)-(3.24) admits a unique solution $(\hat{\bf v}, \hat{p})\in W^{2, 2}(I)^2\times W^{1, 2}(I)$ provided $(\hat{{\bf F}}, \hat{G}, \hat{{\bf H}}^{1}, \hat{{\bf H}}^{2})\in L^{2}(I)^2\times W^{1, 2}(I)\times \mathbb{C}^{2}\times \mathbb{C}^{2}$, and it holds for all $\xi\in l_h$:

with the constant $c$ is independent of $\xi$.

Proof. A similar theorem is proved in [^[19], Theorem 4.9]. So, we omit its proof.

Therefore, the Lemma 1 provides us an opportunity to prove the following theorem of the solvability of the problem (3.1)-(3.4).

Theorem 1. Let ${\bf F}\in{\mathcal {W}_{\alpha}^{0, \, 2}(S)^2}$, $G\in{\mathcal {W}_{\alpha}^{1, \, 2}(S)}$ and ${\bf H_{l+1}}\in{\mathcal {W}_{\alpha}^{2-l-\frac{1}{2}}(\Gamma^l)^2}, \, l = 0, 1$. If the line $\mathrm{Im}\, \xi = h = \alpha-1$ contains no eigenvalue of the operator $\hat{\mathcal{U}}(\xi)$, then the problem (3.1)-(3.4) admits a uniquely determined solution $({\bf v}, \, p)\in {\mathcal {W}_{\alpha}^{2, \, 2}(S)^2}\times {\mathcal {W}_{\alpha}^{1, \, 2}(S)}$ and satisfies the following estimate

where $c > 0$ is independent of ${\bf v}$ and ${\bf F}$.

Proof. We prove this theorem by following the idea of Kondrat$\acute{i}$ev ^[24]. Suppose that the line $\mathrm{Im}\, \xi = h = \alpha-1$ contains no eigenvalue of the operator $\hat{\mathcal{U}}(\xi)$. First of all, we prove that the right-hand sides functions of the system (3.9) are Fourier transform in the sense of (3.15). We know from (3.1) that ${\bf F}\in L^{2}(S)^{2}$, $G\in W^{1, \, 2}(S)$. Further note that for all $\alpha\geq 0$, ${{\bf F}}\in {\mathcal {W}_{\alpha}^{0, \, 2}(S)}^{2}$ and $G\in {\mathcal {W}_{\alpha}^{1, \, 2}(S)}$. Since, ${{\bf F}}\in {\mathcal {W}_{\alpha}^{0, \, 2}(S)}^{2}$, we have

where $h = \alpha-1$ for all $\alpha\geq0$ and it is meaningful in the sense of (3.15). Therefore, the Fourier transform of $\tilde{{\bf F}}(\tau, \theta) = (\tilde{{F}}_{\tau}, \tilde{{F}}_{\theta})$ is meaningful in the half plane $h = \mbox{Im}\, {\xi}\geq-1$ for almost all $\theta\in(0, \, \omega_{0})$.

Analogously, for ${G}\in \mathcal {W}_{\alpha}^{1, \, 2}(S)$, we have

where $h = \alpha-1$ for all $\alpha\geq0$. Therefore, the function $\tilde{G}(\tau, \theta)$ is also Fourier transformable in the half plane $h = \mbox{Im}\, {\xi}\geq-1$ for almost all $\theta\in(0, \omega_{0})$ in the sense of (3.15).

Now, the construction of the singular vector functions can be explained by the observations from ^[25,26,39]. The main question is the inverse Fourier transform of the right-hand sides of (3.19) and (3.22)-(3.24) or simply (3.29) which can be read as follows using the formula (3.14):

Using the Cauchy theorem yields

The first integral and the third integral tend to zero as ${n\rightarrow \infty}$ by ^[24]. The second integral yields ${\bf v}_{j, \, h}(\mbox{x})\in \mathcal {W}_{\alpha}^{2, \, 2}(S)^2$. The calculation of the residue gives the singular terms. If the operator ${\hat{\mathcal{U}}}(\xi)$ contains no eigenvalue on the line $\mathrm{Im}\, {\xi} = \alpha-1, \, \forall\, \alpha\geq0$, then the residue vanishes and the inverse Fourier transform

for $j = 1, 2$ exists. Thus, $\tilde{{\bf v}}_{j, h}(\mbox{x})$ is the uniquely determined solution from $\mathcal {W}_{\alpha}^{2, \, 2}(S)^2$ of the underlying boundary value problem. An analogous result, we obtain for the pressure $\tilde{p}\in \mathcal {W}_{\alpha}^{1, \, 2}(S),$ where $h = \alpha-1$.

Now, using Lemma 1 and (3.16)-(3.17), we can get (3.34).

Lemma 2. ^[29]. Let $\hat{\mathcal{U}}^{-1}(\xi)$ be the inverse operator of $\hat{\mathcal{U}}(\xi)$. $\hat{\mathcal{U}}^{-1}(\xi)$ is a meromorphic operator-valued function with poles which are the eigenvalues of $\hat{\mathcal{U}}(\xi)$. The order $m$ of a pole $\xi_0$ is the largest of the lengths of the Jordan chains corresponding to $\xi_0$. Moreover, the operator $\hat{\mathcal{U}}^{-1}(\xi)$ has the following expansion in the neighborhood of $\xi_0$:

where $q_i:i = 1, ..., m$ are the finite-dimensional operators which do not depend on $\xi$ and $\Gamma(\xi)$ is holomorphic. The operator $q_m$ behaves into the space of eigenfunctions of $\hat{\mathcal{U}}(\xi)$ corresponding to $\xi_0$, while the operators $q_{m-1}, ..., q_1$ behave into the subspaces of the corresponding associate functions.

The next theorem describes the expansion and the regularity of the problem (3.1)-(3.4).

Theorem 2. Let $({\bf v}, p)\in {\mathcal {W}_{\alpha}^{2, \, 2}(S)^2}\times {\mathcal {W}_{\alpha}^{1, \, 2}(S)}$ be a solution of the problem (3.1)-(3.4) for every ${\bf F}\in{\mathcal {W}_{\alpha}^{0, \, 2}(S)^2}\cap{\mathcal {W}_{\alpha_1}^{0, \, 2}(S)^2}$, $G\in{\mathcal {W}_{\alpha}^{1, \, 2}(S)}\cap{\mathcal {W}_{\alpha_1}^{1, \, 2}(S)}$ and ${\bf H_{l+1}}\in{\mathcal {W}_{\alpha}^{2-l-\frac{1}{2}}{(\Gamma^l)}^2}\cap{\mathcal {W}_{\alpha_1}^{2-l-\frac{1}{2}}(\Gamma^l)^2}, \, l = 0, 1$, $\alpha_1 < \alpha$. Assume that no eigenvalue of ${\hat{\mathcal{U}}}(\xi)$ lies on the lines $\mathrm{Im}\, {\xi} = h_1 = \alpha_{1}-1$ and $\mathrm{Im}\, {\xi} = h = \alpha-1$. If $\xi_{1}, \xi_{2}, ..., \xi_{M}$ are the eigenvalues of ${\hat{\mathcal{U}}}(\xi)$ in the strip $\alpha_{1}-1 < \mathrm{Im}\, {\xi} < \alpha-1$, then the solution $({\bf v}, \, p)$ admits the following expansion

where ${\Psi}_{\mu, \, \rho, \, \kappa}(r, \, \theta)$ are the corresponding singular functions given by

with

The regular part $\Big({\bf v}_{reg}(r, \, \theta), {p}_{reg}(r, \, \theta)\Big)\in {\mathcal {W}_{\alpha_1}^{2, \, 2}(S)^2}\times {\mathcal {W}_{\alpha_1}^{1, \, 2}(S)}$ and satisfies the following estimate

Proof. It follows from Theorem 1 that the solution $({\bf v}, p)\in {\mathcal {W}_{\alpha}^{2, \, 2}(S)^2}\times {\mathcal {W}_{\alpha}^{1, \, 2}(S)}$ of the problem (3.1)-(3.4) is uniquely determined and specified by the formula (3.37). The use of Cauchy theorem yields (3.38). It is already stated in Theorem 1 that the first and third integrals in (3.38) are tending to zero as for ${n\rightarrow \infty}$. The second integral produces that ${\bf v}_{reg}(\mbox{x})\in \mathcal {W}_{\alpha_1}^{2, \, 2}(S)^2$, ${p}_{reg}(\mbox{x})\in \mathcal {W}_{\alpha_1}^{1, \, 2}(S)$ is the uniquely determined solution of (3.1)-(3.4) and the estimate (3.41) is valid. This statement follows from Theorem 1.

Now, we should calculate the residue in (3.38). Lemma 2 provide us that $\hat{\mathcal{U}}^{-1}(\xi)$ is a meromorphic operator-valued function with poles which are the eigenvalues of $\hat{\mathcal{U}}(\xi)$, and $\hat{\mathcal{U}}^{-1}(\xi)$ has the expansion in the form of (3.39). Moreover $(\hat{{\bf F}}, \, \hat{G})$ is holomorphic respecting $\xi$ in the strip $\alpha_{1}-1 < \mathrm{Im}\, {\xi} < \alpha-1$. Therefore, we can write

in the neighborhood of $\xi_\mu$, where the coefficients $b_m(\theta)$ are elements of $L^{2}(I)^2\times W^{1, 2}(I)\times \mathbb{C}^{2}\times \mathbb{C}^{2}$. Further, we have

From (3.39), (3.42) and (3.43), it follows that

Therefore, we conclude that

Now, substituting $r = e^{\tau}$, applying Lemma 2 and (^[27], Theorem 1.1.5), we obtain (3.40).

Now, we derive our fundamental regularity and expansion theorem for the mixed boundary value problem for the stationary Stokes system in a two-dimensional bounded domain with corner points. By considering the substitution $\mbox{Re}\, \alpha = -\mbox{Im}\, \xi-2$, it improves the theorems (^[26], Theorem 8.2.1 and Theorem 8.2.2) which are based on the Mellin transform and used for the solvability of the elliptic systems.

Theorem 3. (Regularity and expansion theorem): Let $\alpha_{1}$ and $\alpha_{2}$ be real numbers and satisfying $\alpha_{1}-1 < \alpha_{2} < \alpha_{1}$. Let a pair $({\bf u}, q)\in \mathcal {W}_{\alpha_{1}}^{m, \, 2}(\mathcal{D})^{2}\times \mathcal {W}_{\alpha_{1}}^{m-1, \, 2}(\mathcal{D})$ be a solution of the stationary Stokes system (1.2) with the homogenous Dirichlet, Neumann, and mixed boundary conditions (1.3)-(1.4) and ${\bf f}\in \mathcal {W}_{\alpha_{2}}^{m_{1}-2, \, p}(\mathcal{D})^{2}\cap \mathcal {W}_{\alpha_{1}}^{m-2, \, 2}(\mathcal{D})^{2}$, where $1\leq p < \infty$, $m_{1}\geq m \geq 2$ and $\alpha_{1}\geq\alpha_{2}\geq0$. Then, the following implications hold:

1. If the strip $\alpha_{2}+\frac{2}{p}-m_{1}\leq \mathrm{Im}\, {\xi}\leq \alpha_{1}+1-m$ is free of eigenvalues of the operator $\hat{\mathcal{U}}(\xi)$, then the solution $({\bf u}, \, q)\in \big[\mathcal {W}_{\alpha_{2}}^{m_{1}, \, p}(\mathcal{D})^{2}\times \mathcal {W}_{\alpha_{2}}^{m_{1}-1, \, p}(\mathcal{D})\big]$ and holds the following estimate

2. Let $\xi_{1}, \xi_{2}, ..., \xi_{M}$ are the eigenvalues of the operator $\hat{\mathcal{U}}(\xi)$, and suppose that no eigenvalue lie on the lines $\mathrm{Im}\, {\xi} = \alpha_{2}+\frac{2}{p}-m_{1}$ and $\mathrm{Im}\, {\xi} = \alpha_{1}+1-m$. If the eigenvalues $\xi_{1}, \xi_{2}, ..., \xi_{M}$ are situated in the strip $\alpha_{2}+\frac{2}{p}-m_{1} < \, \mathrm{Im}\, {\xi} < \alpha_{1}+1-m$, then the solution $({\bf u}, q)$ admits the following expansion in the neighborhood $P_{\delta}$ of the corner point $P$:

with $\big({\bf u}_{reg}(r, \, \theta), {q}_{reg}(r, \, \theta)\big)\in \mathcal {W}_{\alpha_{2}}^{m_{1}, \, p}{(P_{\delta})}^{2}\times \mathcal {W}_{\alpha_{2}}^{m_{1}-1, \, p}{(P_{\delta})}$. Here, $M$ is the number of eigenvalues of the operator $\hat{\mathcal{U}}(\xi)$ in the strip, the constants $c_{\mu, \, \rho, \, \kappa}$ depend on the data and the singular functions, $I_{\mu} = \mathrm{dim}\, \mathrm{Ker}\, \hat{\mathcal{U}}(\xi_{\mu})$, $\kappa_{\mu\rho}$ is the length of the Jordan chains of $\hat{\mathcal{U}}(\xi_{\mu})$, and the corresponding singular functions are given by

with

It is noted from (3.46) and (3.47) that the eigenvalues $\xi_{\mu} = 0$ do not yield singularities in the development of the solution in the neighborhood $P_{\delta}$.

It is recognized for elliptic boundary value problems that the eigenvalues of the operator $\hat{\mathcal{U}}(\xi)$ which lies on the strip have a significant role in the regularity results. The assertions 1 and 2 of Theorem 3 represents the regularity and expansion of the solutions of the system (1.2)-(1.4) near the corner points.

Remarks 3. The technique of Mellin transform, the method of special ansatzes, and spherical coordinates are used in ^[26,27] to obtain the generalized form of the boundary eigenvalue problem for the stationary Stokes system with Dirichlet and mixed boundary conditions. The existence of the generalized eigenvalues is discussed in a strip $\mathrm{Re}\, \xi\in(0, \, 1)$. Here, we use the Fourier transform technique to obtain the generalized form of the boundary eigenvalue problem for the stationary Stokes system with mixed boundary conditions. Moreover, the existence of the generalized eigenvalues in a strip $\mathrm{Im}\, \xi\in(-1, \, 0)$ with various combinations of the boundary conditions that depend on the apex angle $\omega_{0}$ are studied.

3.2.   General solutions

Let $(\hat{v}_{\tau}, \hat{v}_{\theta}, \hat{p})$ be denoting the general solution of the system (3.19) by considering the right-hand side functions equal to zero, i.e., $\hat{L}(\xi)(\hat{{\bf v}}, \hat{p}) = 0,$ where $(\hat{v}_{\tau}, \hat{v}_{\theta})$ stands the components of the velocity vector $\hat{{\bf v}}$ and $\hat{p}$ for the pressure function. On the other hand, If the system (3.19) has the non-zero right-hand side functions, then the general solution can be written as

Here, $I_{p}$ denote the particular solution corresponding to the non-zero right-hand side functions and $I_{hom}$ is the homogenous or general solution for zero right-hand sides. Here, our interest is to find the $I_{hom}$ solution.

For simplicity, we substitute $\xi = -iz$ from ^[18] into (3.19), then a system of linear ordinary differential equations is obtained that depends on the complex parameter $z$.

3.2.1.   The fundamental system to the ordinary differential equations

A system of linear ordinary differential equations in $\theta$ with complex parameter $z$ is considered

Furthermore, the system (3.48) provides a linear homogenous fourth-order ordinary differential equation with constant complex coefficients, i.e.,

It is noted from the general theory of ordinary differential equations that (3.49) gives four independent solutions, and the general form of the fundamental solution for $(z\neq 0, \, \pm 1)$ or $(\xi\neq 0, \, \pm i)$ can be written as

Therefore, it is necessary to consider the other cases for various values of $z$ or $\xi$, and the general forms of their fundamental solutions are described as follows:

Case 1. (For $z = 0$; or $\xi = 0$):

Case 2. (For $z = -1$; or $\xi = i$):

Case 3. (For $z = 1$; or $\xi = -i$):

The coefficients ${\bf B} = (B_1, B_2, B_3, B_4)^T$ would be determined according to the types of boundary conditions. In line with the above cases, we can substitute $\xi = -iz$ into Dirichlet, Neumann, and mixed boundary conditions. As well, their transformed forms also depend on the complex parameter $z$.

3.3.   The calculation of the eigenvalues

To evaluate the eigenvalues and corresponding eigenfunctions of the stationary Stokes system, the solution (3.50) with various combinations of the boundary conditions is considered to obtain a system of four linear homogeneous equations with our unknowns $B_{1}, \, B_{2}, \, B_{3}$ and $B_{4}$. The resulting matrix of coefficients of these equations depends on the complex parameter $z$, and a nontrivial solution exists if the determinant of the resulting matrix of coefficients vanishes (see ^[5]). Further, it produces the transcendental equations whose roots are the eigenvalues, namely, $\xi_{\mu}$ wherein (${\mu}$ is used for multiple eigenvalues, i.e., ${\mu} = 1, ..., M$). To compute these results, we proceed as follows.

Dirichlet boundary conditions (DD)

It means that the Dirichlet boundary conditions are given on both sides of the corner point. The determinant method is used from ^[5] to obtain a system of linear homogeneous equations. A non-trivial solution exists if the determinant $\det{D_{DD}(z)}$ of the corresponding system of the matrix of coefficients vanishes. So, the computation leads to the transcendental equation

The roots of (3.54) are the eigenvalues of the operator $\hat{\mathcal{U}}_{DD}(\xi) = \big[\hat{L}(\xi), \{\hat{B}_{[DD]}(\xi)\}\big]$.

Neumann boundary conditions (NN)

It means that the Neumann boundary conditions are given on both sides of the corner point. Therefore, the computation leads to the transcendental equation

The eigenvalues of $\hat{\mathcal{U}}_{NN}(\xi) = \big[\hat{L}(\xi), \, \big\{\hat{B}_{[NN]}(\xi)\big\}\big]$ are the roots of (3.55).

Mixed boundary conditions (DN)

It means that the Dirichlet or Neumann boundary condition is given on one side of the corner point, and the other condition is given on the other side. Similar to the latter cases, the obtained equation for this case is

The roots of (3.56) are the eigenvalues of $\hat{\mathcal{U}}_{DN}(\xi) = \big[\hat{L}(\xi), \, \{\hat{B}_{[DN]}(\xi)\}\big]$.

Remarks 4. Due to symmetry, the same results can be obtained if the versed boundary conditions are used which means that the Neumann condition is at $\theta = 0$ and the Dirichlet condition is at $\theta = \omega_{0}$. Therefore, this case of boundary conditions is not considered here.

Remarks 5. Consequently, the poles of $\mathcal{R}(\xi)$ are the numbers of $-iz_{n}$, where $z_{n}$ are the roots of the Eqs (3.54)-(3.56).

The following theorem describes the distribution of the eigenvalues of the corresponding boundary value problem for Dirichlet, Neumann, and mixed boundary conditions for various cases of the values of $z$.

Theorem 4. Let $\xi = -iz$ be an eigenvalue of the operator $\hat{\mathcal{U}}(\xi)$, and satisfies the following transcendental equations for Dirichlet, Neumann, and mixed boundary conditions. Then

(ⅰ) for the Dirichlet problem (3.48) and (3.22), $z$ satisfies the equation

(ⅱ) for the Neumann problem (3.48) and (3.23), $z$ satisfies the equation

(ⅲ) for the mixed problem (3.48) and (3.24), $z$ satisfies the equation

It is easily examined from (3.29) and (3.57)-(3.59) that the zeros of these equations are symmetric with respect to the origin and the real axis lies in the complex plane. Therefore, the eigenvalues of the operator $\hat{\mathcal{U}}(\xi)$ are positioned in the complex plane symmetrically with respect to the origin and the imaginary axis.

Proof. For Dirichlet boundary conditions The Eq (3.57) is studied in (3.54) with Dirichlet boundary conditions and is satisfied for $z\neq0, \, \pm 1$. Furthermore, the various cases of $z$ are considered with Dirichlet boundary conditions.

For $z = 0$, the general solution is taken from (3.51) with Dirichlet boundary conditions and a system of linear homogeneous equations $\Sigma\, {\bf B} = 0$ is obtained, wherein the symbol $\Sigma$ denotes the matrix of coefficients. It follows from the existence of the non-trivial solution which means that the determinant of the corresponding matrix of coefficients for the linear homogeneous equations is zero. We have

which implies that ${\bf B} = 0$ and zero is not an eigenvalue.

For $z = \pm 1$, the general solutions of the system (3.48) is given in (3.52) and (3.53). By using Dirichlet boundary conditions, a system of linear homogeneous equations is obtained. Moreover, the determinant of the matrix of coefficients is zero and the non-trivial solution exists. However, we are not interested in the null space analogous to the eigenvalues $\xi = \pm i$.

For Neumann boundary conditions The Eq (3.58) is calculated for Neumann boundary conditions in (3.55) and is satisfied for $z\neq0, \, \pm 1$.

For $z = 0$, we consider the general solution given in (3.51) with the Neumann boundary conditions, and a system of linear homogeneous equations is obtained. By the use of the method of the determinant, we get zero determinant of the matrix of coefficients. Further, the rank of the matrix of coefficients is two and $(0, \, 0, \, 0, \, 1)^{T}$ and $(0, \, 0, \, 1, \, 0)^{T}$ are two linearly independent solutions. Thus, the corresponding eigenfunctions are ${\bf e}_{1} = (\cos\theta, -\sin\theta)^{T}$ and ${\bf e}_{2} = (\sin\theta, \cos\theta)^{T}$ which represent the translation in $x$ and $y$ directions.

For $z = 1$, the general solution is given in (3.53). The determinant of the matrix of coefficients for this case is zero and the non-trivial solution exists.

For $z = -1$, the general solution is given in (3.52). The use of the Neumann boundary conditions gives the following determinant $\det(\Sigma) = 2\sin^{2}(\omega_{0})$ of the corresponding matrix of coefficients. Since $\omega_{0}\in(0, \, 2\pi]$, for $\omega_{0} = \pi, \, 2\pi$, a non-trivial solution exists.

For mixed boundary conditions The Eq (3.59) is studied in (3.56) with the mixed boundary conditions and is satisfied for $z\neq0, \, \pm 1$.

For $z = 0$, the general solution is given in (3.51) and the mixed boundary conditions are used to get a system of linear homogenous equations. The determinant of the corresponding system is $\det(\Sigma) = 1 > 0$, and is not an eigenvalue of $\hat{\mathcal{U}}(\xi)$.

For $z = 1$, the general solution is given in (3.53) and the determinant of the matrix of coefficients for this case is $\det(\Sigma) = -\cos 2(\omega_{0})$.

For $z = -1$, the general solution is given in (3.52) and the determinant of the matrix of coefficients is $\det(\Sigma) = -\cos \omega_{0}-\cos 2\omega_{0}+1$.

Consequently, $z = \pm1$, are the eigenvalues of the corresponding problem if the corresponding determinants are equal to zero.

4.   The regularity results

Let $({\bf u}, q)\in W^{1, \, 2}(\mathcal{D})^{2}\times L^{2}(\mathcal{D})$ be the unique weak solution of the stationary Stokes problem. It follows from the theory of Kondrat$\acute{i}$ev ^[24] that the pair $({\bf u}, q)\in \mathcal {W}_{\gamma+1}^{2, \, 2}(\mathcal{D})^{2}\times \mathcal {W}_{\gamma+1}^{1, \, 2}(\mathcal{D})$, where $\gamma$ is a small positive real number. To obtain further qualitative regularity results, the theory proposed by ^[39] is employed and we will analyze that the weak solution $({\bf u}, q)\in \mathcal {W}_{\gamma+1}^{2, \, 2}(\mathcal{D})^{2}\times \mathcal {W}_{\gamma+1}^{1, \, 2}(\mathcal{D})$.

Firstly, the case of $L^{2}$-data is considered for the direct consequences of Theorem 3 and the observations of Section 3. Let $\omega_{0DN}$ denote the maximal angle from the set of all angles of such corner points wherein types of boundary conditions change. Analogously, $\omega_{0DD}$ and $\omega_{0NN}$ are the maximal angle of those corner points which have similar types of boundary conditions, i.e., Dirichlet-Dirichlet and Neumann-Neumann, on both adjacent sides of the corner point. If no such type of points exists or the angles $\omega_{0DD}, \, \omega_{0NN} < \pi$, then strongly we can set $\omega_{0DD}, \, \omega_{0NN} = \pi$ as the minimum value.

The following propositions hold to formulate the regularity results of the weak solution $({\bf u}, q)$ of the stationary Stokes system with various combinations of the boundary conditions.

Lemma 3. Suppose that if the strip $\alpha-1\leq \mathrm{Im}\, {\xi} < \epsilon, \, \alpha > 0,$ is free of the zeros of the equations

and

for any arbitrary small $\epsilon > 0$, then the solution $({\bf u}, q)\in \mathcal {W}_{\alpha}^{2, \, 2}(\mathcal{D})^{2}\times \mathcal {W}_{\alpha}^{1, \, 2}(\mathcal{D})$ and satisfies the following estimate

Proof. To show that no eigenvalue of (4.1) and (4.2) lies in the strip $\alpha-1\leq \mbox{Im}\, {\xi} < \epsilon$ for an angle $\omega_{0}$, where $\alpha$ is a small positive real number. Firstly, we are considering the case of Dirichlet boundary conditions $\hat{\mathcal{U}}_{DD}(\xi)$ for an apex angle $\omega_{0} = \frac{\pi}{2}$ and $\omega_{0} = {\pi}$, respectively, or for an any arbitrary angle $\omega_{0}\in (0, \pi]$. We note that no eigenvalue of (4.1) is found that lie on the line $h = \mbox{Im}\, {\xi} = t$ for $t > -1$. Consequently, $(\tilde{{\bf v}}, \tilde{p})\in[\mathcal {W}_{\alpha}^{2, \, 2}{(S)}]^{2}\times [\mathcal {W}_{\alpha}^{1, \, 2}{(S)}]$ for a small positive real number $\alpha$. In addition, the singularities appear for this case at corners with an apex angle $\omega_{0}$ is greater than $\pi$. (See Figure 3). In all the following graphs, the red lines indicate the pure imaginary values, while the black lines indicate the complex parts of the complex ones.

Analogously, the case of Neumann boundary conditions $\hat{\mathcal{U}}_{NN}(\xi)$ is considered. There is no eigenvalue of (4.2) that lie on the line $h = \mbox{Im}\, {\xi} = t$ for $t > -1$, for an angle $\omega_{0} = \frac{\pi}{2}$ and $\omega_{0} = {\pi}$, respectively, or for any arbitrary angle $\omega_{0}\in (0, \pi]$. It produces singularities when the apex angle $\omega_{0}$ is greater than $\pi$. Hence, it follows that the solution $({\bf u}, q)\in \mathcal {W}_{\alpha}^{2, \, 2}(\mathcal{D})^{2}\times \mathcal {W}_{\alpha}^{1, \, 2}(\mathcal{D})$.

Additionally, we have a bounded domain $\mathcal{D}$ and the corresponding continuous imbeddings (2.9) and (2.12). Let we have

and

Clearly,

So,

Finally, we obtain that the weak solution of the Stokes system is

where $\alpha$ is a small positive real number. Accordingly, the estimate (4.3) can be followed directly from Theorem 3.

Analogously, for the case of mixed boundary conditions, we obtain:

Lemma 4. Suppose that if no eigenvalues of the mixed boundary condition

lie on the line $\mathrm{Im}\, {\xi} = h = \gamma-1$, then the solution $({\bf u}, q)\in W^{2-\gamma, \, 2}(\mathcal{D})^{2}\times W^{1-\gamma, \, 2}(\mathcal{D})$, where $\gamma$ depends on the angle $\omega_{0}$.

Proof. For any arbitrary angle $\omega_{0}\in(0, 2\pi)$, no eigenvalues of the mixed boundary condition (4.4) lie on the line $\mbox{Im}\, {\xi} = h = \gamma-1$ for $h\geq -\frac{1}{4}$. Thus, no eigenvalues of $\hat{\mathcal{U}}_{DN}(\xi)$ that lie in the strip $-\frac{1}{4}\leq \mbox{Im}\, {\xi} < \epsilon$ for $\gamma\geq\frac{3}{4}$, where any arbitrary small $\epsilon > 0$. So, we obtain that the regularity result $({\bf u}, q)\in W^{\frac{5}{4}, \, 2}(\mathcal{D})^{2}\times W^{\frac{1}{4}, \, 2}(\mathcal{D})$. Besides, the singularities appear at a corner with an apex angle greater than $\frac{\pi}{4}$ (See Figure 4).

Respectively, for an angle $\omega_{0} = \frac{\pi}{2}$, we have $h = \mbox{Im}\, {\xi} > -\frac{1}{2}$. So, we obtain that the regularity result $({\bf u}, q)\in W^{2-\gamma, \, 2}(\mathcal{D})^{2}\times W^{1-\gamma, \, 2}(\mathcal{D})$ for $\gamma > \frac{1}{2}$. Accordingly, a similar regularity result is obtained for an angle $\omega_{0} = \pi$.

Finally, we obtain that the regularity result $({\bf u}, q)\in W^{2-\gamma, \, 2}(\mathcal{D})^{2}\times W^{1-\gamma, \, 2}(\mathcal{D})$ for an arbitrary small positive number $\gamma$ that depends on the apex angle $\omega_{0}$.

It is well-known that for every ${\bf f}\in V^{\ast}$, where $V^{\ast}$ is the dual space of $V$, a unique weak solution $({\bf u}, q)\in V\times L^{2}(\mathcal{D})$ of some generalized steady Stokes problem exists.

Therefore, the following lemma describes the regularity results for $L^{p}$-data.

Lemma 5. Suppose that

(ⅰ) for $\hat{\mathcal{U}}_{DD}(\xi)$ and $\hat{\mathcal{U}}_{NN}(\xi)$ boundary conditions, no-eigenvalues lie on the strip $-\mu\leq \mathrm{Im}\, {\xi} < \epsilon$, for $0\leq \mu < 1$, and $\epsilon > 0$ is an arbitrary small positive number,

(ⅱ) for mixed boundary conditions, $\xi_{0}$ is the only eigenvalue of $\hat{\mathcal{U}}_{DN}(\xi)$ that lie within the strip $-\mu\leq \mathrm{Im}\, {\xi} < \epsilon$, for $0\leq \mu < 1$, and additionally, we suppose that this is a simple eigenvalue.

Then for every ${\bf f}\in L^\frac{2}{2-\mu}(\mathcal{D})^{2}$, the weak solution $({\bf u}, q)$ of the stationary Stokes system is contained in $\big[W^{1+\mu, \, 2}(\mathcal{D})\big]^{2}\times \big[W^{\mu, \, 2}(\mathcal{D})\big]$.

Proof. The statement can be followed directly from Theorem 3 by considering $p = \frac{2}{2-\mu}$, $\alpha_{2} = 0$ and $\alpha_{1} = 1+\epsilon$.

Since ${\bf f}\in L^\frac{2}{2-\mu}(\mathcal{D})^{2}\subset V^{\ast}$, the similar result is obtained by applying the same process used in Lemmas 3-4.

5.   The Navier-Stokes equations

We consider the steady Navier-Stokes equations

with the homogenous mixed boundary conditions (1.3)-(1.4). If the given right-hand sides have a sufficiently small norm, then we prove by using the local diffeomorphism theorem that the Navier-Stokes equations have similar regularity results as the solution of the generalized Stokes problem near the corner points. Denote

Additionally, we denote $H$ and $V$ are the closures of $\mathcal{E}(\mathcal{D})$ equipped with the norms of $L^{2}(\mathcal{D})^{2}$ and $W^{1, \, 2}(\mathcal{D})$. Recall that $V$ and $H$ are the Hilbert spaces and their scalar products are given in (2.1).

Definition 2. Let ${\bf f}\in V^{\ast}$. ${\bf u}$ is called the weak solution of the problem (5.1) with the homogenous mixed boundary conditions (1.3)-(1.4), if ${\bf u}\in V$ and satisfies

Further, $b\big({\bf u}, {\bf v}, {\bf w}\big)$ describes the trilinear continuous form for every ${\bf u}, {\bf v}, {\bf w}\in V$ by

Definition 3. Let $H$ represent the closure of $\mathcal{E}(\mathcal{D})$ in the norm $L^{2}(\mathcal{D})^{2}$ and describe the Banach space

Note that, $\mathfrak{M}\hookrightarrow \hookrightarrow L^{\infty}(\mathcal{D})$, $\mathfrak{M}\hookrightarrow \hookrightarrow V$ and $V\hookrightarrow \hookrightarrow H$.

For the solvability of the problem (5.1), the subsequent theorem is considered which is known as the local diffeomorphism theorem (see ^[9]).

Theorem 5. Let $\mathcal{M}$ be a mapping from $\mathcal{X}$ into $\mathcal{Y}$ which belongs to $C^{1}$ in some neighbourhood $\mathrm{W}$ of point $v_{0}\in \mathcal{X}$, where $\mathcal{X}$ and $\mathcal{Y}$ are Banach spaces. If the Fr$\acute{e}$chet derivative $\acute{\mathcal{M}}(v_{0}): X \rightarrow Y$ is continuous, one-to-one and onto $\mathcal{Y}$, then there exists a neighbourhood $\mathrm{U}$ of point $v_{0}$ such that $\mathrm{U}\subset \mathrm{W}$ and a neighbourhood $\mathrm{V}$ of point $\mathcal{M}(v_{0})$ such that $\mathrm{V}\subset \mathcal{Y}$. So, the mapping $\mathcal{M}$ is one-to-one from $\mathrm{W}$ onto $\mathrm{V}$.

Let ${\bf h}\in H$. Then the Lax-Milgram theorem and the Lemmas 3-4 yields that there exists a uniquely determined ${\bf w}\in \mathfrak{M}$, such as

Now, the operator $\mathcal{Q}:\mathfrak{M}\rightarrow H$ is described by

Note that the mapping $\mathcal{Q}$ is one-to-one. Moreover, we define an operator $\mathcal{R}:\mathfrak{M}\rightarrow H$ which is given as

Further, the pertinent problem (5.1) can be considered as the single operator equation $\mathcal{R}({\bf u}) = {\bf f}$.

Let ${\bf u}$ be a fixed point in $\mathfrak{M}$, and $\mathcal{Z}_{\bf u}:\mathfrak{M}\rightarrow H$ be a linear operator which is described as follows

Lemma 6. Let ${\bf u}$ be some arbitrary fixed element in $\mathfrak{M}$. The operator $\mathcal{B}_{\bf u}$ is given by

is the Fr$\acute{e}$chet derivative of $\mathcal{R}$ at the point ${\bf u}$ and $\mathcal{B}_{\bf u}\in C(\mathfrak{M})$.

Proof. Since

and

We get

the smoothness of $\mathcal{B}_{\bf u}\in C(\mathfrak{M})$ is obvious.

Lemma 7. Let ${\bf u} = 0$. Then $\mathcal{B}_{\bf u} = \mathcal{B}_{0} = \mathcal{Q}$ is one-to-one.

The more information to prove that $\mathcal{B}_{\bf u}$ is one-to-one and onto $H$, we refer (^[40], Theorem 5.5F) and ^[6].

Theorem 6. Let $\mathcal{P}_1$ be a continuous one-to-one operator from $\mathcal{X}$ onto $\mathcal{Y}$, and $\mathcal{P}_2$ be a compact linear operator from $\mathcal{X}$ into $\mathcal{Y}$, where $\mathcal{X}$ and $\mathcal{Y}$ are Banach spaces. Therefore, the subsequent statements are equivalent:

1. $\mathcal{P}_1+\mathcal{P}_2$ is one-to-one;

2. $\mathcal{P}_1+\mathcal{P}_2$ is onto $\mathcal{Y}$.

Theorem 7. Let ${\bf f}\in H$, the norm of ${\bf f}$ is sufficiently small. Then a uniquely determined ${\bf u}\in \mathfrak{M}$ exists, such that

Proof. By (5.8), $\mathcal{B}_{\bf u}$ is the sum of operators $\mathcal{Q}$ and $\mathcal{Z}_{\bf u}$. It is noted from the above results that $\mathcal{Q}:\mathfrak{M}\rightarrow H$ is the one-to-one operator and onto $H$, and $\mathcal{Z}_{\bf u}$ is a compact operator. Furthermore, from Lemma 7, $\mathcal{B}_{\bf u}$ is one-to-one. These facts and Theorem 6 produces that $\mathcal{B}_{\bf u}$ is onto $H$. Hence, the Theorem 5 yields a unique solution.

6.   Conclusions

In this article, we have studied the boundary singularities and regularity of the weak solution of the mixed boundary value problem for the stationary Stokes and Navier-Stokes system in a two-dimensional non-smooth domain with corner points and points at which the type of boundary conditions change. The solvability of the considered boundary value problem has been analyzed in the context of the weighted Sobolev spaces with Kondrat$\acute{i}$ev type weights and the regularity theorems are formulated. To compute the singular terms for various boundary conditions, the complex Fourier transform has been used to obtain the generalized form of the boundary eigenvalue problem for the stationary Stokes system. The emerging eigenvalues and eigenfunctions produce singular terms, which permits us to evaluate the optimal regularity of the corresponding weak solution of the stationary Stokes system.

The main regularity and expansion theorem for the stationary Stokes system is presented in Theorem 3. We have discussed the regularity results of the corresponding boundary value problem for the case of $L^2$ and $L^p$-data which are the direct consequences of Theorem 3. It is seen for the case of Dirichlet and Neumann boundary conditions that if the domain $\mathcal{D}$ has reentrant corners $(\omega_i > \pi: i = 1, 2, ...N)$, then the weak solution $({\bf u}, q)$ of the considered problem produces singularities. On the other hand, for the case of mixed conditions, the singularities appear at corners with $(\omega_i > \frac{\pi}{4}: i = 1, 2, ...N)$. Moreover, it is observed that if singularities exist, then splitting the solution into a singular part which defines a linear combination of explicit model singularity functions $s_m$ for the Stokes operator with corresponding unknown coefficients $C_m$ and a regular part that belongs to $H^2\times H^1$. Finally, it is proved that the weak solution $({\bf u}, q)$ of the underlying boundary value problem belongs to $W^{2-\gamma, \, 2}\mathcal{(D)}^{2}\times W^{1-\gamma, \, 2}\mathcal{(D)}$, where $\gamma$ is an arbitrarily small positive real number that depends on the apex angle $\omega_{0}$.

Additionally, we have extended the obtained results for the Stokes system for the non-linear Navier-Stokes system. We have proved this by using the local diffeomorphism theorem that the solution of the Navier-Stokes equations has similar regularity results as the solution of the generalized Stokes problem near the corner points if the given body force has a sufficiently small norm. To prove this, an operator $\mathcal{R}$ relating to the Navier-Stokes equations is defined and has shown that it is Fréchet differentiable at the point ${\bf u} = 0$. Furthermore, the Fréchet derivative of $\mathcal{R}$ at the point ${\bf u}$ is agreed with the Stokes problem.

Presently, the Stokes and Navier-Stokes equations with the Navier-slip boundary conditions and the free-boundary problems in domains with corners have very interesting phenomena. The issues regarding their existence and regularity are considered for smooth domains but theoretical results for the corner singularity decomposition are still not obtained. Therefore, these issues are numerically interesting. In future works, it is important to show the unique existence of the approximations for the regular parts and coefficients, and to derive their error estimates. On the other hand, it is also observed that the non-stationary compressible Stokes and Navier-Stokes equations on polygonal domains could be considered.

Conflict of interest

The author declare that no conflict of interest exist.