Conversion calculation method of multivariate integrals

1.   Introduction

Multivariable calculus ^[1] is a rich and fascinating subject. Multivariate integrals are widely used in various disciplines. For example, in optimization and (multidimensional) optimal control theory, authors ^[2,3] studied the optimization of some multiple or curvilinear integral functionals subject to ODEs, PDEs or isoperimetric constraints. In 2018, Mititelu and Treanţă ^[4] proposed optimality conditions in multiobjective control problems which involve multiple integrals. In the computation method of multivariate integrals, we often convert the double integral into iterated integrals in a Cartesian coordinate system by Fubini's Theorem or a polar coordinate system ^[5]. The triple integral is converted into iterated integrals in a Cartesian coordinate system, or a cylindrical coordinate system, or a spherical coordinate system. In general, a line integral is converted into a definite integral, and a surface integral is converted into a double integral. In addition, Green's formula, Gauss's formula and Stokes's formula could be applied to perform the calculation ^[6].

In the last twenty years, more researchers worked on the conversion relationships for several types of multivariate integrals. For example, the surface integral with respect to area is converted into the line integral with respect to the arc length in ^[7]. In ^[8], Li and Shi proposed a method of computing the surface integral by curvilinear integral in the special case when the projection of the surface on the coordinate plane was a curve. In the current paper, we propose new transformation algorithms for multivariate integrals, which further enhances the computation methods of multivariate integrals.

2.   New methods for calculating double integrals

The main idea is to use the change of variables formula to transform double and triple integrals to iterated integrals which can be done more easily.

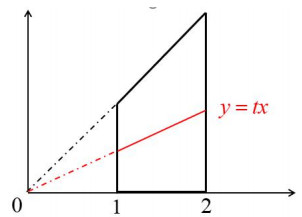
Theorem 1. (1) If the bounded closed region $ D $ is generated by a family of planar smooth curves

with no double point (i.e., a point traced out twice as a closed curve is traversed), and the function $ f(x, y) $ is continuous on the closed region $ D $, then

where the symbol 'ds' represents the arclength differential.

(2) If the bounded closed region $ D $ is generated by a family of planar smooth curves

with no double points, and the function $ f(x, y) $ is continuous on the closed region $ D $, then

Proof: Let transformation $ y = y(x, t) $, which transforms the $ D $ on the $ x O y $ plane to

on the $ t O x $ plane. Notice

According to the integration by substitution, the formula (2.1) holds. On the other hand, since $ \mathrm{d} s = \sqrt{1+y_{x}^{\prime 2}} \mathrm{\; d} x $ on the curve $ L_{t} $,

The formula (2.2) can be obtained from (2.1). In the same way we can get (2.3) and (2.4).

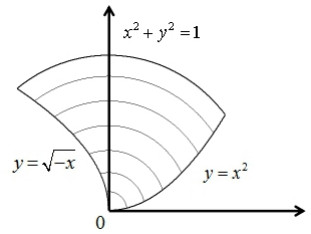
Example 1. Calculate the double integral $ I = \iint\limits_D \sqrt{x^{2}+y^{2}} \mathrm{\; d} x \mathrm{\; d} y $, where the closed region $ D $ is bounded by three curves $ y = \sqrt{1-x^{2}}, x = \sqrt{y} $ and $ y = \sqrt{-x} $.

Method 1. Take a family of concentric circles arcs

where $ 0 \leq t \leq 1 $ as shown in Figure 1. Obviously, the central angle of each arc segment is $ \frac{\pi}{2} $, which results in the length of arc $ L_{t} $ is $ \frac{\pi t}{2} $. It follows from (2.2) that

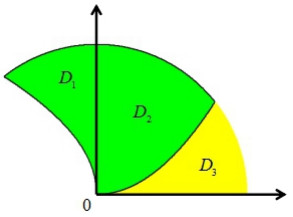
Method 2. Label regions $ D_{1}, D_{2} $ and $ D_{3} $ as shown in Figure 2. Rotate $ D_{1} $ clockwise by $ \frac{\pi}{2} $ to get $ D_{3} $. It is not difficult to find that $ D = D_{1} \cup D_{2} $ and $ D_{2} \cup D_{3}: \quad x^{2}+y^{2} \leq 1, \quad x \geq 0 \quad y \geq 0 $. Make a rotation transformation $ \left\{\begin{array}{l}x = -v, \\ y = u, \end{array}\right. $, then

So,

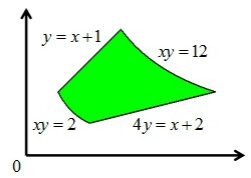
Example 2. Calculate the double integral $ I = \iint\limits_D \frac{1}{x} \mathrm{\; d} x \mathrm{\; d} y $, where the closed region $ D $ is in the first quadrant and is bounded by two curves $ x y = 2, x y = 12 $, and two lines $ y = x+1, y = \frac{1}{4}(x+2) $, as shown in Figure 3.

Since the shape of the integral region D is very complicated (at least it needs to be represented as two sub-regions), as shown in Figure 4, the method of directly converting to the iterated integral in the Cartesian coordinate system or the polar coordinate system is quite cumbersome. Moreover, it is difficult to calculate with the integration by substitution.

The methods developed in Theorem 1 work well for this kind of regions.

Solution: Let's take a family of hyperbolics

as shown in Figure 5.

It follows from (2.1) that

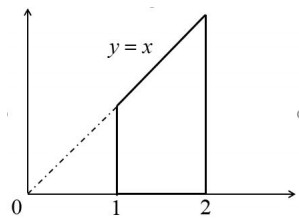
Example 3. Calculate the double integral $ I = \iint\limits_D \frac{\sqrt{x^{2}+y^{2}}}{x} \mathrm{\; d} \sigma $, where the closed region $ D $ is bounded by four lines $ x = 1, x = 2, y = 0 $ and $ y = x $, as shown in Figure 6.

If we adopt the method of converting to the iterated integral in the polar coordinate system, the integral will eventually be transformed into the definite integral $ \int_{0}^{\pi / 4} \sec ^{3} x \mathrm{\; d} x $. And the calculation process is complicated.

Solution: Let's take a family of lines

as shown in Figure 7. It follows from (2.1) that

It can be seen from the above three examples that the calculation method of double integral varies. The calculation method proposed by Theorem 1 is an important component. This method can simplify the calculation process, which has certain theoretical significance for studying the multivariate integrals.

According to the differential method of the implicit function, the following inference is easily obtained by Theorem 1.

Corollary 1. The bounded closed region $ D $ is generated by a family of planar smooth curves

with no double points. If function $ f(x, y) $ is continuous on $ D $, then

In addition, in Theorem 1, if the family of curves take the family of vertical lines, the family of horizontal lines and the family of lines passing through the origin respectively, three common calculation methods of double integral can be obtained.

Corollary 2. (1) The function $ f(x, y) $ is continuous on the bounded closed region

then

(2) The function $ f(x, y) $ is continuous on the bounded closed region

then

(3) The function $ f(x, y) $ is continuous on the bounded closed region $ D $. If $ D $ can be expressed as

with the polar transformation $ x = r \cos \theta, y = r \sin \theta $, then

Proof: Taking the family of vertical lines

we can know from (2.3)

That is, the formula (2.5) is established. Similarly, taking the family of horizontal lines

we can get (2.6) from (2.1).

If we use

where

we can get $ y = x \tan \theta $ and

It follows that from (2.1)

3.   New techniques for calculating triple integrals

Theorem 2. If the bounded closed region $ \Omega $ is generated by a family of smooth surfaces

with no double points, where $ D_{x y}(t) $ is the projection area of the surface $ \Sigma_{t} $ on $ x O y $ coordinate plane and $ z_{t}^{\prime} \neq 0 $, and the function $ f(x, y, z) $ is continuous on the closed region $ \Omega $, then

Proof: Let $ \left\{\begin{array}{l}x = x, \\ y = y, \\ z = z(x, y, t), \end{array}\right. $then the Jacobian determinant

And it transforms $ \Omega $ in $ O-x y z $ to

in $ O-x y t $. By substitution method of triple integral, we can obtain formula (3.1). Since $ \mathrm{d} S = \sqrt{1+z_{x}^{\prime 2}+z_{y}^{\prime 2}} \mathrm{\; d} x \mathrm{\; d} y $ on $ \Sigma_{t} $, we know

Substituting the above formula into (3.1) yields (3.2).

Corollary 3. (1) Let the bounded closed region $ \Omega $ be generated by a family of smooth surfaces

with no double points, where $ D_{y z}(t) $ is the projection area of the surface $ \Sigma_{t} $ on $ yOz $ coordinate plane and $ x_{t}^{\prime} \neq 0 $. If the function $ f(x, y, z) $ is continuous on the closed region $ \Omega $, then

(2) Let the bounded closed region $ \Omega $ be generated by a family of smooth surfaces

with no double points, where $ D_{x z}(t) $ is the projection area of the surface $ \Sigma_{t} $ on $ xOz $ coordinate plane and $ y_{t}^{\prime} \neq 0 $. If the function $ f(x, y, z) $ is continuous on the closed region $ \Omega $, then

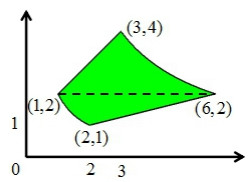
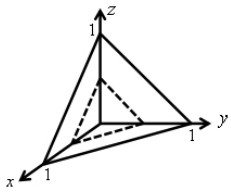
Example 4. Calculate the triple integral $ I = \iiint\limits_{\Omega}(x+y+z) \mathrm{d} x \mathrm{\; d} y \mathrm{\; d} z $, where

as shown in Figure 8.

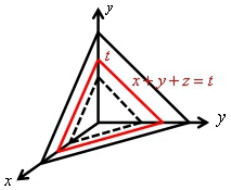
Method 1. As shown in Figure 9, Taking a family of triangle planes

then $ \left|z_{t}^{\prime}\right| = 1, \sqrt{1+z_{x}^{\prime 2}+z_{y}^{\prime 2}} = \sqrt{3} $ hold, and the area of $ \Sigma_{t} $ is $ \frac{\sqrt{3}}{2} t^{2} $. Substituting the above formula into (3.2) gives

According to the additivity of the integral region, this integral can also be calculated as follows.

Method 2. Let

then $ \Omega = \Omega_{1}-\Omega_{2} $. According to the symmetry of the triple integral, we have

Example 5. Calculate the triple integral $ I = \iiint\limits_{\Omega} \sqrt{x^{2}+y^{2}+z^{2}} \mathrm{\; d} x \mathrm{\; d} y \mathrm{\; d} z $, where

Method 1. Taking a family of spheres

then $ \left|z_{t}^{\prime}\right| = \frac{t}{\sqrt{t^{2}-x^{2}-y^{2}}}, \sqrt{1+z_{x}^{\prime 2}+z_{y}^{\prime 2}} = \frac{t}{\sqrt{t^{2}-x^{2}-y^{2}}} $ hold. In addition, the plane $ x+y+z = 0 $ and the plane $ 2z-x-y = 0 $ are perpendicular to each other, so the area of $ \Sigma_{t} $ is $ \pi t^{2} $. Substituting the above results into (3.2) gives

Method 2. Let a orthogonal transformation $ u = \frac{1}{\sqrt{2}}(x-y), v = \frac{1}{\sqrt{3}}(x+y+z), w = \frac{1}{\sqrt{6}}(2 z-x-y). $ And it transforms $ \Omega $ in $ O-x y z $ to

in $ O-uvw $, thus

Using spherical coordinates, we can obtain

Example 6. Calculate the triple integral $ I = \iiint\limits_{\Omega} \sqrt{x^{2}+y^{2}} \mathrm{\; d} x \mathrm{\; d} y \mathrm{\; d} z $, where

Method 1. Taking a family of cylindrical surfaces

then the area of $ \Sigma_{t} $ is $ \frac{1}{2} \pi t^{2} $. Because of $ x = \sqrt{t^{2}-y^{2}} $, $ \frac{\left|x_{t}^{\prime}\right|}{\sqrt{1+x_{y}^{\prime 2}+x_{z}^{\prime 2}}} = 1 $ holds. Substituting the above results into (3.3) gives

Method 2. Taking a family of conic surfaces

then $ z_{t}^{\prime} = \sqrt{x^{2}+y^{2}} $ holds. The projection area on the xoy coordinate plane:

Substituting the above results into (3.1) gives

Similarly, in Theorem 2, if we choose three kinds of special plane families, three common calculation methods of triple integral will be obtained.

Corollary 4. (1) If the function $ f(x, y, z) $ is continuous on the bounded closed region

then

(2) The function $ f(x, y, z) $ is continuous on the bounded closed region $ \Omega $. If $ \Omega $ can be expressed as

with the cylindrical coordinate transformation $ x = r \cos \theta, y = r \sin \theta, z = z $, then

(3) The function $ f(x, y, z) $ is continuous on the bounded closed region $ \Omega $. If $ \Omega $ can be expressed as

with the spherical coordinate transformation $ x = \rho \sin \varphi \cos \theta, y = \rho \sin \varphi \sin \theta, z = \rho \cos \varphi $, then

Proof: (1) In Theorem 2, we take plane family

where $ D_{x y}(t) $ is the projection area of the cross section of $ \Sigma_{z} $ and $ \Omega $ on the $ xOy $ coordinate plane. It is easy to calculate $ z_{t}^{\prime} = 1 $. Substituting this result into (3.1) gives

(2) In Corollary 3, we take plane family

where

is the projection area of the cross section of $ \Sigma_{\theta} $ and $ \Omega $ on the $ rOz $ plane. Obviously, $ y = x \tan \theta $ is true. Thus $ y_{\theta}^{\prime} = \frac{x}{\cos ^{2} \theta} = \frac{r}{\cos \theta} $. Substituting this result into (3.4) gives

Since $ \frac{\partial(x, z)}{\partial(r, z)} = \left|\begin{array}{cc}\cos \theta & 0 \\ 0 & 1\end{array}\right| = \cos \theta $, $ \mathrm{d} x \mathrm{\; d} z = |\cos \theta| \mathrm{d} r \mathrm{\; d} z $ holds. Thus

(3) In Corollary 3, we take plane family

where

is the projection area of the cross section of $ \Sigma_{\theta} $ and $ \Omega $ on the $ \varphi O \rho $ plane. Obviously, $ y = x \tan \theta $ is true. Thus $ y_{\theta}^{\prime} = \frac{x}{\cos ^{2} \theta} = \rho \frac{\sin \varphi}{\cos \theta} $. Substituting this result into (3.4) gives

Since $ \frac{\partial(x, z)}{\partial(\rho, \varphi)} = \left|\begin{array}{cc}\sin \varphi \cos \theta & \cos \varphi \\ \rho \cos \varphi \cos \theta & -\rho \sin \varphi\end{array}\right| = \rho \cos \theta $, $ \mathrm{d} x \mathrm{\; d} z = \rho|\cos \theta| \mathrm{d} \rho \mathrm{d} \varphi $ holds. Thus

4.   Another new scheme for calculating triple integrals

Theorem 3. Let the bounded closed region $ \Omega $ be generated by a family of smooth surfaces

with no double points and $ z_{t}^{\prime} \neq 0 $. Let $ \Gamma_{x t} $ be the curves where the planes, which pass through the point $ (x, 0, 0) $ and are perpendicular to the X-axis, and surface $ \Sigma_{t} $ intersect. If the function $ f(x, y, z) $ is continuous on the closed region $ \Omega $, then

where $ D = \left\{(t, x) \mid a \leq t \leq b, x_{1}(t) \leq x \leq x_{2}(t)\right\} $.

Proof: Let $ x = x, y = y, z = z(x, y, t), $ then the Jacobian determinant

And it transforms $ \Omega $ to

By substitution method of triple integral we can obtain

On the other hand,

then

thus

Example 7. Calculate the triple integral $ I = \iiint\limits_{\Omega} z \mathrm{\; d} v $, where $ \Omega: x^{2}+y^{2}+z^{2} \leq 1, z \geq|y| $.

Method 1. Taking the surface family

For any given $ t \in[0, 1] $, $ x \in[-t, t] $ and

Let $ \Gamma_{x t} $ be the curves where the planes, which pass through the point $ (x, 0, 0) $ and are perpendicular to the X-axis, and surface $ \Sigma_{t} $ intersect. That means

Then $ z_{t}^{\prime} = \frac{t}{\sqrt{t^{2}-x^{2}-y^{2}}} $, thus

Substituting the above formula into (4.1) gives

where $ D = \{(t, x) \mid 0 \leq t \leq 1, -t \leq x \leq t\} $. So,

Method 2. According to the symmetry of the integral, it is easy to see that

where

And the projection area of $ \Omega^{\prime} $ on $ xOy $ plane is

thus

Using the generalized polar transformation, the above integral can be calculated as follows:

Corollary 5. (1) The bounded closed region $ \Omega $ is generated by a family of smooth surfaces

with no double points and $ z_{t}^{\prime} \neq 0 $. Let $ \Gamma_{y t} $ be the curves where the planes, which pass through the point $ (0, y, 0) $ and are perpendicular to the Y-axis, and surface $ \Sigma_{t} $ intersect. If the function $ f(x, y, z) $ is continuous on the closed region $ \Omega $, then

where $ D = \left\{(t, y) \mid a \leq t \leq b, y_{1}(t) \leq y \leq y_{2}(t)\right\} $.

(2) The bounded closed region $ \Omega $ is generated by a family of smooth surfaces

with no double points and $ y_{t}^{\prime} \neq 0 $. Let $ \Gamma_{x t} $ be the curves where the planes, which pass through the point $ (x, 0, 0) $ and are perpendicular to the X-axis, and surface $ \Sigma_{t} $ intersect. If the function $ f(x, y, z) $ is continuous on the closed region $ \Omega $, then

where $ D = \left\{(t, x) \mid a \leq t \leq b, x_{1}(t) \leq x \leq x_{2}(t)\right\} $.

In fact, there are several other cases of Theorem 3, which are not repeated here.

Method 3 of Example 6. Taking a family of cylindrical surfaces

then $ \frac{\left|y_{t}^{\prime}\right|}{\sqrt{1+y_{z}^{\prime 2}}} = \frac{t}{\sqrt{t^{2}-x^{2}}} $ holds. Let $ \Gamma_{x t} $ be $ \left\{\begin{array}{l}x = x, \\ y = \sqrt{t^{2}-x^{2}}, 0 \leq x \leq t \\ z = t, \end{array}\right. $. And the length of the straight-line segment $ \Gamma_{x t} $ is $ t $. Substituting the above results into (4.2) gives

Corollary 6. Let the function $ f(x, y, z) $ be continuous on the bounded closed region

then

where $ D $ is the projection area of $ \Omega $ on the $ xOy $ coordinate plane.

Proof: In the Corollary 5(2), if we take

$ \Gamma_{x t} $ is straight lines $ \Gamma_{x y} $ parallel to the Z-axis. Notice

It follows from (4.2) that

5.   Conclusions

In this paper we establish several formulas for converting the double integral to a line integral with respect to arc length, and the triple integral to a surface integral with respect to area or a line integral with respect to arc length. Some commonly used calculation methods are special cases of our methods. Examples show that the methods presented here are simple and effective in computing certain complex double integrals and triple integrals.

Acknowledgements

This work was supported in part by the National Natural Science Foundation of China under Grant Nos. 12001151. The authors would like to thanks to the experts and scholars who took time out of their busy schedules to review this paper which greatly help improve the clarity and quality of the paper.

Conflict of interest

The authors declare that they have no conflict of interest.