Shifted Legendre polynomials-based single and double integral inequalities with arbitrary approximation order: Application to stability of linear systems with time-varying delays

1.   Introduction

Time-delay systems exist in many practical situations as industry process, biological, ecological groups, telecommunication, economy, mechanical engineering, and so on. A time-delay in a system often induces oscillation and instability, which motivated a huge number of researchers to study the stability analysis with various criteria ^[1,2,3]. Evaluation of system stability with a constant delay has been studied extensively and lots of theoretical tools have been presented like characteristic equation and eigenvalues analysis ^[4,5]. Those methods have been well established currently which can derive effective criteria smoothly with numerical efficiency. However, this type of criteria cannot be applied to a time-varying delay system and some other methodologies have been employed.

Generally, two different methodologies have been employed: the first one is so called input-output method that treats a delay as an uncertain operator, and transforms the original time-varying delay system into a closed loop between a nominal LTI system and a perturbation depending on the delay. The stability criteria of which have been well developed by using conventional robustness tools like Small Gain Theorem ^[6,7], Integral Quadratic Constraint or Quadratic Separation ^[8,9]. The conservativeness is small for a slowly varying delay, but large for a quickly one because it depending on the upper bound on the derivative of the delay. Another technique is based on the proper construction of Lyapunov-Krasovskii functions. The conservativeness of this method comes from two aspects: the choice of functional and the bound on its derivative. It is not easy to find an appropriate Lyapunov-Krasovskii functional (LFK) to obtain less conservative criteria since it contains both the delay and its bounds.

In earlier research, only a single integral term was employed as a part of LFK to analysis and handle the time delay in systems ^[10,11,12]. Up to now, double, triple, even quadruple integral terms has been developed which usually bring more effective stability criteria ^[13,14,15]. And also an augmented and a delay-partitioning LKF method were proposed to reduce the conservativeness, and the difficulty now lies in the bounds of the integrals that appear in the derivative of the functional for a stability condition ^[16,17].

Previously, The Jensen inequality and Wirtinger-based integral inequality were reported as the integral inequality method that yields less conservative stability criteria ^[2,18]. Delay-dependent strategy and delay-independent approach under time-varying delays, uncertainties and disturbance are employed to stability analysis. Delay-dependent strategy has been received many attentions as a result of its less conservatism than delay-independent ^{[19,20,21,22,23,24,25,26,27]}. Later, the first- and second-order reciprocally convex approach were proposed based on a new kind of linear combination of positive functions weighted by the inverses of squared convex parameters emerges when the Jensen inequality was applied to partitioned double integral terms in the derivation of LMI conditions ^[28,29]. And the optimal divided method and the secondary partitioning method were provided for stability criteria in double integral terms in LPF ^[30,31].

Recently, the integral term with higher order approximation has been proposed, such as Wirtinger-based double integral inequality ^[32], free-matrix-based integral inequality ^[33], auxiliary function-based integral inequality ^[34]. These inequalities provided less conservation of stability criteria that those of the Jensen or Wirtinger-based single integral inequities. Especially, a novel integral inequality which called Bessel-Legendre (B-L) inequality has only been applied to the system with constant delays ^{[35,36,37,38]}. And also multiple-integral inequalities were newly developed to give high-order approximation to the original integral, the associated integral terms in LPF are also increased ^[39,40].

In this study, a new single integral inequality is proposed through using shifted Legendre polynomials, and then the double integral inequality is developed with the utilization of Cholesky decomposition. Both single and double integral inequalities are with arbitrary approximation order, which encompasses the well-known Jensen and Wirtinger-based inequalities, auxiliary function-based integral inequalities, and even the B-L inequality. The proposed two inequalities yield improved stability criteria with less conservativeness.

This paper is organized as follows. Section 2 introduces the relevant theories of shifted Legendre polynomials-based single and double integral inequalities, and section 3 and 4 provide application of proposed methods to systems with constant and time-varying delays, including numerical examples.

2.   Shifted Legendre polynomials-based single and double integral inequalities

2.1.   Shifted Legendre polynomials for single integral

The classical shifted Legendre polynomials are a set of functions analogous to the Legendre polynomials, but defined on the interval $[0, 1]$ as follows

where $p_i(s)$ denotes the $i$-order shifted Legendre polynomial, $w_{i, j}$ denotes the $j$th coefficient of $p_i(s)$.

We here call classical shifted Legendre polynomials as the shifted Legendre polynomials for single integral with the following coefficient

where $C_i^j$ denotes the combination which can be written using factorials as

Shifted Legendre polynomials obey the orthogonality relationship, i. e.

where ${\delta _{nm}}$ denotes the Kronecker delta.

Also we can represent shifted Legendre polynomials for single integral in the matrix form as follows

The relationship between ${L_m}(s)$ and ${U_m}(s)$ is obtained

where $W_m$ is the coefficient matrix with the following form

It's obvious that $W_n$ is a lower triangular matrix.

With similar formulation, (2.4) can be rewritten as

2.2.   Shifted Legendre polynomials for double integral

The interest of shifted Legendre polynomials for double integral is that the orthogonality relationship exists if we use double integral instead of single integral.

The double integral of the product of two classical shifted Legendre polynomials can be obtained as follows

which can also be extended using the form of matrix

Considering that $H_m$ is a real-valued symmetric positive semi-definite matrix, we can gain the associated lower triangular matrix using Cholesky decomposition

where

Since ${B_m} > 0$, $H_m$ has the unique Cholesky decomposition. Unfortunately, (2.10) shows that ${L_m}(u)$ is not a proper set of basic functions when the double integral is employed instead of single integral. Thus, we need to find new ones. We introduce the linear combination of $\{ {p_j}(s)\}$ as follows

i.e.

where $D_m$ denotes the transition matrix from $L_m^{}(u)$ to ${\bar L_m}(u)$ with the form

In order to obtain the proper shifted Legendre polynomials for double integral, the following equation should be solved.

where $\{ {d_{ij}}\}$ and $\{ {h_{ij}}\}$ are coefficients to be determined.

Substituting (2.11) into (2.16) yields

By solving a serial of linear equations of (2.17), the matrices $D_m$ and ${\bar H_m}$ are achieved as following

Thus the vector of shifted Legendre polynomials are achieved

where, by (2.6),

2.3.   Shifted Legendre polynomials-based single integral inequality

For continuously vector function $\dot x(\tau):[a{, _{}}b] \to {\textbf{R}^n}$, the associated function $\dot{ \tilde x}(s):[0{, _{}}1] \to {\textbf{R}^n}$ is defined as follows

where $\tau = (b - a)s + a$.

We can develop the relationships between the single integrals of $\dot x(\tau)$ and $\dot {\tilde x}(s)$

The best weighted square approximation can be obtained with minimizing the following cost function

where $R > 0$ denotes a symmetric positive-defined matrix with proper dimensions, $\tilde f(s)$ denotes the approximation function defined as follows

where ${\beta _i} \in {\textbf{R}^n}$ denotes the weight corresponding to the shifted Legendre polynomial ${p_i}(s)$ for single integral.

Substituting (2.25) into (2.24) yields

where ${\omega _i}$ denotes the integral of the product of $\dot {\tilde x}(s)$ and the i-th shifted Legendre polynomial ${p_i}(s)$ for single integral. sym() is defined as the sum of vector/matrix with its own transpose sym$(x) = x+x^{\rm T}$.

i.e.

where ${\widehat W_m}$ denotes the extension matrix associated to ${W_m}$

where $I$ denotes the identity matrix with proper dimensions.

Substituting (2.23) into (2.28) yields

According to the static condition of (2.26), we obtain

The second condition of (2.26)

It means that the optimal $\beta _i^* = (2i + 1){\omega _i}/(b-a)$ leads to the only minimum cost value

Lemma 1 (shifted Legendre polynomials-based single integral inequality): For any symmetric positive-defined constant matrix $R \in {\textbf{R}^{n \times n}}$, $R > 0$, and vector function $\dot x(t):[a{, _{}}b] \to {\textbf{R}^n}$ such that the integrations concerned are well defined, then the following inequality exists

Proof: It can be obtained from (2.33) observably.

Remark 1: The right term of the proposed single integral inequality (2.34) is approximation with arbitrary order to the left term, i.e., when $\dot x(t) = {c_0} + {c_1}t + \cdots + {c_m}{t^m}$, ${c_i} \in {\textbf{R}^n}$, $i = 0{, _{}}1{, _{}} \cdots {, _{}}m$, the left term is exactly equal to the right term.

Proof: The function $\dot x(t) = {c_0} + {c_1}t + \cdots + {c_m}{t^m}$ can be rewritten as

where

$\dot {\tilde x}(s)$ can also be expressed by serial of shifted Legendre polynomials $\{ {p_k}(s)\}$ as follows

where

Thus the left term of (2.34) becomes

This complete the proof.

Remark 2: The integral inequality (2.34) degenerates to Jensen inequality when $m = 0$^[2].

Proof: Substituting $m = 0$ into (2.34) yields

This complete the proof.

Remark 3: The integral inequality (2.34) degenerates to Wirtinger-based inequality when $m = 1$^[18].

Proof: According to (2.30) we have

Substituting (2.41) and (2.42) into (2.34) yields

This complete the proof.

2.4.   Shifted Legendre polynomials-based double integral inequality

For continuously vector function $\dot x(\tau):[a{, _{}}b] \to {\textbf{R}^n}$, and it's associated function $\dot {\tilde x}(s):[0{, _{}}1] \to {\textbf{R}^n}$ defined in (2.22), we can develop the relationships between the double integrals of $\dot x(\tau)$ and $\dot {\tilde x}(s)$ as follows

where

The best weighted square approximation with double integral can be obtained with minimizing the following cost function

where $R > 0$ denotes a positive-defined matrix with proper dimensions, $\tilde g(u)$ denotes the approximation function defined as follows

where ${\beta _i} \in {\textbf{R}^n}$ denotes the weight corresponding to the shifted Legendre polynomial ${\bar p_i}(s)$ for double integral.

Substituting (2.46) into (2.45) yields

where ${\nu _i}$ denotes the integral of the product of $\dot {\tilde x}(s)$ and the i-th shifted Legendre polynomial ${p_i}(s)$ for single integral

i.e.

where ${\widehat{\bar W}_m}$ denotes the extension matrix associated to ${\bar W_m}$

Substituting (2.44) into (2.49) yields

According to the static condition of (2.47), we obtain

The second condition of (2.47)

It means that the optimal $\beta _i^* = \frac{{2i + 2}}{{b - a}}{\nu _i}$ leads to the only minimum cost value

Lemma 2 (shifted Legendre polynomials-based double integral inequality): For any positive-defined constant matrix $R \in {\textbf{R}^{n \times n}}$, $R > 0$, and vector function $\dot x(t):[a{, _{}}b] \to {\textbf{R}^n}$ such that the integrations concerned are well defined, then the following inequality exists

Proof: It can be obtained from (2.54) observably.

Remark 1: The right term of the proposed single integral inequality (2.34) is approximation with arbitrary order to the left term, i.e., when $\dot x(t) = {c_0} + {c_1}t + \cdots + {c_m}{t^m}$, ${c_i} \in {\textbf{R}^n}$, $i = 0{, _{}}1{, _{}} \cdots {, _{}}m$, the left term is exactly equal to the right term.

Proof: The function $\dot x(t) = {c_0} + {c_1}t + \cdots + {c_m}{t^m}$ can be rewritten as

where

$\dot {\tilde x}(s)$ can also be expressed by serial of shifted Legendre polynomials $\{ {\bar p_k}(s)\}$ as follows

where

Thus the left term of (2.34) becomes

This complete the proof.

Remark 2: The integral inequality (2.34) degenerates to auxiliary function-based integral inequality when $m = 1$^[34].

Proof: According to (2.51) we have

Note that ${\nu _0}$ and ${\nu _1}$ are just the coefficients of auxiliary function-based integral inequality. This complete the proof.

3.   Applications to systems with constant delays

3.1.   Systems with constant delays

Let us consider the following linear system with constant delay interval

where $x(t) \in {\textbf{R}^n}$ denotes the state vector of the system with $n$ dimensions, $A$ and $A_h$ are real known constant matrices with appropriate dimensions, the continuously differentiable functions $\varphi (t)$ denote the initial condition, $h \ge 0$ denotes the system's constant delay.

Theorem 1: The system (3.1) is asymptotically stable if there exist matrices $P > 0$, $Q > 0$, $R > 0$ and $S > 0$ such that the following conditions hold ^[41]:

where the notations in (3.2) are intermediate variables that defined properly in previous and in the process of proof, which can be found as $B$ in (3.10), $C$ in (3.12), $e_1, e_2$ in (3.10), $h$ in (3.1), $A_e$ in (3.11), $\Psi$ in (3.13), $\widehat W_m$ in (2.29), $\Omega_m$ in (2.34), $\bar \Psi$ in (3.14), $\widehat{\bar W}_m$ in (3.7), $\bar \Omega_m$ in (3.18).

Proof: We define a set of functions $\{ {y_k}(t)\}$ as follows

The time derivatives of ${y_k}(t)$ can be obtained as follows

And the initial we have

Let $a = t - h$, $b = t$, we can obtain $\{ {\omega _k}\}$ and $\{ {\nu _k}\}$ for shifted Legendre polynomials-based single and double integral inequalities, respectively

We define extra-states $\chi (t)$ and $\xi (t)$ as follows

The extra-states $\chi (t)$ can be expressed by $\xi (t)$

where

where ${e_k} = [{\underbrace {\begin{array}{*{20}{c}} 0 & 0 & 0 \\ \end{array}}_{k - 1}}{I}\underbrace {\begin{array}{*{20}{c}} 0 & 0 & 0 \\ \end{array}}_{m + 2 -k}]$ denotes the k-th row coefficient of $\xi (t)$, $I_n$ and $0_n$ denote the identity and zeros matrix with dimensions $n \times n$, respectively.

And the system (3.1) can be rewritten as

where ${A_e} = A{e_1} + {A_h}{e_2}$.

The time derivative of $\chi (t)$ can be obtained as follows

where

According to (3.6) and (3.8), we have

where

With similar method, we have following according to (3.7) and (3.8)

where $\bar \Psi = \left[{\begin{array}{*{20}{c}} {{0_{nm \times n}}} & {{0_{nm \times n}}} & {{I_{nm}}} \\ \end{array}} \right]$

In order to analysis the stability of the system (3.1), we consider the following Lyapunov-Krasovskii functional (LKF) candidates

Taking the time derivative of $V(t)$ yields

Recalling that (2.34) and (2.55), following inequalities are employed to yield the upper bound of $\dot V(t)$

This complete the proof.

3.2.   Examples

Example 1: We consider the well-known delay dependent stable system (3.1) with following coefficient matrices as given in ^[29]:

Using delay sweeping techniques the maximum allowable delay ${h_{\max }} = 6.1725$ can be obtained. Also many recent papers provide different results using Jensen inequality, Wirtinger-based inequality, and so on. The allowable maximum delays are shown in Table 1. We observe that the upper bounds obtained by our proposed inequalities are significantly better than those in other literatures.

Example 2: We consider the dynamics of machining chatter with following coefficient matrices as firstly studied in ^[36]:

where $K$ denotes a parameter.

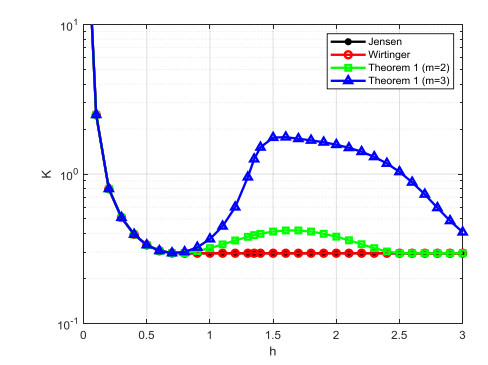
It's obviously that the system is stable with $K$ less than some upper bound. Here we try to the upper bound in various delays. It's shown that Lemma 1 and Lamme 2 yield more stability region than those derived from Jensen and Wirtinger-based Lemma, as illustrated in Figure 1. When the parameter $K \le 0.295$, the system is still stable even the delay is very large, such as $h = 500$.

4.   Applications to systems with time-varying delays

4.1.   Systems with time-varying delays

Let us consider the following system with interval time-varying delay:

where $x(t) \in {\textbf{R}^n}$ denotes the state vector of the system with $n$ dimensions, $A$ and $A_h$ are real known constant matrices with appropriate dimensions, the continuously differentiable functions $h(t)$ and $\varphi (t)$ denote the system's time-varying delay and the initial condition, respectively.

Assumption 1: The delay function $h(t)$ and its differential $\dot h(t)$ both have finite bounds, i.e., there exist scales ${h_2} \ge {h_1} > 0$ and ${\mu _1} \le {\mu _2} \le 1$ such that

Theorem 2: The system (4.1) is asymptotically stable if there exist matrices $P > 0$, $Q_1 > 0$, $Q_2 > 0$, $Q_3 > 0$, $R_1 > 0$, $R_2 > 0$, $R_3 > 0$, and $S_1 > 0$, $S_2 > 0$, $S_3 > 0$ such that the following conditions hold^[41]:

where the notations in (4.2) are intermediate variables that defined properly in previous and in the process of proof, which can be found as $B_2$ in (4.8), $C_2$ in (4.11), $e_1, e_2, e_3, e_4$ in (3.10), $h_1, h_2$ in (4.6), $\mu_2$ in (4.16), $A_e$ in (3.11), $\Psi_1, \Psi_2, \Psi_3$ in (4.14), $\bar \Psi_1, \bar \Psi_2, \bar \Psi_3$ in (4.14), $W_m$ in (2.7), $\widehat W_m$ in (2.29), $\widehat {\bar{W}}_m$ in (3.7), $\Omega_1, \Omega_2, \Omega_3$ in (4.13), $\bar \Omega_m$ in (3.18), $\bar \Omega_1, \bar \Omega_2, \bar \Omega_3$ in (4.13).

Proof: If the delay $h$ is varying with time $t$, then we can develop from (3.3)

If $h = {h_1}$ or $h = {h_2}$ is a constant variable, (3.3) yields

We introduce the following extra-states ${\hat \chi _m}(t)$ and ${\hat \xi _m}(t)$ as follows

The extra-states can be expressed by ${\hat \xi _m}(t)$

where

And the system (3.1) can be rewritten as

where ${A_e} = \left[{\begin{array}{*{20}{c}} A & {{A_h}} & {{0_{n \times (nm + 2n)}}} \end{array}} \right]$

The time derivative of $\hat \chi_m (t)$ can be obtained as follows

where

where

In order to analysis the stability of the system (4.1), we consider the following Lyapunov-Krasovskii functional (LKF) candidates

where

Taking the time derivative of ${V_k}(t)$ yields

where

Thus the sum of ${\dot V_k}(t)$, $k = 1{, _{}}2{, ^{}} \cdots {, _{}}10$ yields

Notice that $\Xi (h{, _{}}\dot h) \le \Xi ({h_2}{, _{}}{\mu _2})$ for all $h \in [{h_1}{, _{}}{h_2}]$ and $\dot h \in [{\mu _1}{, _{}}{\mu _2}]$, we can develop that $\dot V(t) \le {\xi ^T}(t)\Phi \xi (t) < 0$, where

This complete the proof.

4.2.   Examples

Example 1: We also consider the well-known delay dependent stable system (4.1) with following coefficient matrices as given in ^[29]:

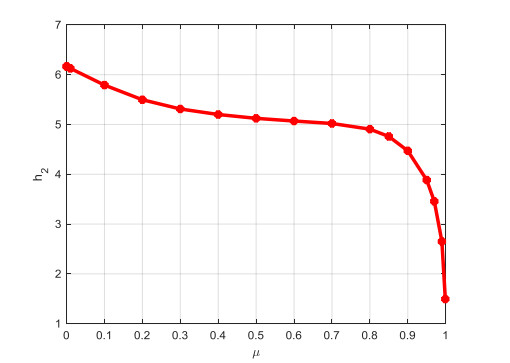
The delay rate bounds ${\mu _1} = - \mu$, ${\mu _2} = \mu$. We herein calculate the allowable upper bound ${h_2}$ for various delay rate $\mu$ via Theorem 2, as illustrate in Figure 2. It's shown that ${h_2}$ deceases continuously with delay rate $\mu$ growing.

The allowable upper bounds ${h_2}$ varying with given $\mu$ are shown in Table 2. We observe that the upper bounds obtained by Theorem 2 are significantly better than others. Theorem 1 provides the least conservative results.

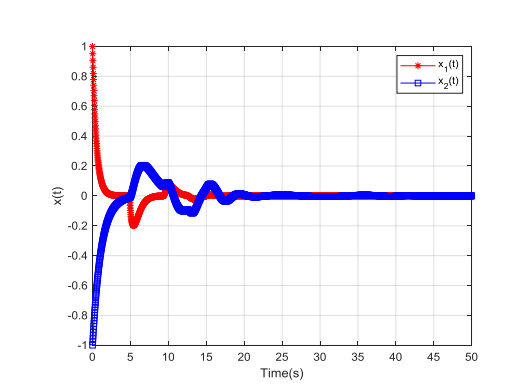
For simulation, let the time-varying delay $h(t) = 3 + 2\cos (0.25t)$, which means that ${h_1} = 1$, ${h_2} = 5$, ${\mu _1} = - 0.5$, and ${\mu _2} = 0.5$. The initial condition of the system is chosen as ${\rm{x}}(0) = {[1{, _{}}-1]^{\mathop{\rm T}\nolimits} }$. The time history of system states is illustrated in Figure 3. As our expectation, both states asymptotically converge to zero despite the previous vibration.

Example 2: Consider the time-varying delay system (4.1) with the following parameters ^[33]:

When the delay is constant ($\mu = 0$), the analytical upper bound can be obtain ${h_{\max }} = \pi$. The improvement of our approach is shown in Table 3. It's verified that the advantage of Theorem 2 is over the results in other literatures.

5.   Conclusions

New single and double integral inequalities with arbitrary approximation order are developed through the use of shifted Legendre polynomials and Cholesky decomposition. These two inequalities encompass several former well-known integral inequities, such as Jensen inequality, Wirtinger-based inequality, auxiliary function-based integral inequalities, and bring new less-conservative stability criteria by employing proper Lyapunov-Krasovskii functionals. Several numerical examples have been provided which show large improvements compared to existing results in both constant and time-varying delay systems.

Acknowledgments

The authors would like to thank the anonymous reviewers for their constructive comments that have greatly improved the quality of this paper.

This work is supported by Shanghai Nature Science Fund under contract No. 19ZR1426800, Shanghai Jiao Tong University Global Strategic Partnership Fund (2019 SJTU-UoT), WF610561702, and Shanghai Jiao Tong University Young Teachers Initiation Programme, AF4130045.

Conflict of interest

All authors declare no conflicts of interest in this paper.