1.
Introduction
The World Health Organization (WHO) declared COVID-19, caused by severe acute respiratory syndrome corona-virus 2 (SARS-CoV-2), a pandemic on March 11, 2020 [1]. Currently, strains of the virus have been reported, suggesting possible multi-point transmission of the SARS-CoV-2 [2],[3]. In addition to the health crisis, the COVID-19 pandemic has disrupted the financial and social systems of most countries and pushed more populations in low- and middle-income countries (LMICs) below the poverty line, thus exacerbating wealth inequality [4],[5]. Sadly, the current pandemic is predicted to impose more morbidity and mortality burdens while simultaneously disrupting an already fragile society and world economy [4]–[6]. As with past outbreaks, the novel nature of the current coronavirus led experts to recommend non-pharmaceutical interventions (NPI) as the most effective public health interventions against COVID-19, as no definitive therapy existed for its treatment [7],[8].
Nigeria reported its first confirmed case on February 27th, 2020, the first reported case in sub-Saharan Africa [9]–[11]. As of March 31st, 2022, of over 11.3 COVID-19 cases (with 251260 deaths) reported in Africa, Nigeria accounts for 255415 cases which are approximately 2.3% of the continent’ COVID-19 burden [12]. The Nigerian Government preemptively announced a series of strict measures, including the early adoption of a lockdown (in early March), followed by an instant nationwide public awareness campaign about COVID–19 prevention and control measures [7]. The lockdown represented a solution to a crisis for which Nigeria was ill-equipped from a public health perspective. Equally, the lockdown was a crucial measure to buy some time to prepare for the surge of COVID-19 cases. On a positive note, the age brackets in Nigeria have been hypothesized as one factor responsible for the low mortality rate [10]. Nigeria has a relatively young population, with 62% of Nigeria's population being younger than 35 years, while only 7% are older than 65 [11]. This age factor could have spared Nigeria high mortality from a disease that poses a greater danger to the elderly.
The announcement of a safe and effective COVID-19 vaccine was arguably the most significant development since the World Health Organization declared COVID-19 a pandemic. Vaccines are safe, cost-friendly, and effective in reducing or eradicating diseases. However, despite the ample scientific evidence supporting vaccines' safety and efficacy, the spread and normalization of myths and anti-vaccine information have greatly threatened Africa's mass vaccination campaign [8]. The reluctance or refusal to vaccinate despite availability of vaccines can be defined as vaccine hesitancy, according to the WHO, is one of the top ten threats to health and well-being in 2019 [13]. Sadly, these threats are heightened by misinformation and the spread of fake news and can reverse progress in tackling vaccine-preventable diseases. Globally, the number of deaths due to vaccine-preventable diseases (VPDs) has significantly reduced in the past few decades. Except for cancer and tuberculosis, the number of deaths from VPDs declined from 4.1 m in 1990 (9% of the total death) to 2–3 million in 2010 (5% of the total death) [14]. A further 1.5 million deaths could be avoided if global coverage of vaccinations is improved [13]. Vaccination controls mortality, morbidity, and complications associated with infectious diseases; thus, expanding robust and routine vaccination programs is one fundamental way to reduce the incidence of infectious diseases such as COVID-19 [8],[15]. Unfortunately, the accelerated pace of vaccine development has further heightened public anxieties [16]. This could also compromise COVID-19 vaccine acceptance in a country that in 2003 witnessed one of the worst ever situations of vaccine hesitancy in Africa during the polio vaccination program [8],[17],[18].
A global survey showed that 65.2% of participants from Nigeria would be willing to get vaccinated against COVID-19 if a vaccine were available [6]. However, this survey was carried out ten months ago, and a lot has changed since then regarding the vast and evolving range of misinformation and conspiracy theories circulating. This misinformation could affect public confidence in the COVID-19 vaccine, resulting in an acute increase in the levels of vaccine hesitancy. Therefore, it is crucial to gauge Nigerians' current levels of willingness to receive a COVID-19 vaccine and determine correlations between vaccine hesitancy and/or acceptance. Hence, this study's objectives were to identify how adults in Nigeria are willing to receive a COVID-19 vaccine and examine whether socio-demographic factors and attitudes toward COVID-19 are associated with their acceptance or refusal of the COVID-19 vaccine. This study also attempted to understand the reasons for COVID-19 vaccine refusals among the general Nigerian population.
2.
Methods
2.1. Study design and data collection
This cross-sectional study was developed using an anonymous online-based questionnaire. Recruitment of respondents was performed using targeted advertising on social media platforms such as WhatsApp, Facebook, LinkedIn, Instagram, and Twitter. Also, to obtain more respondents, our social media networks were urged to share the internet-based questionnaire with their networks, and data were collected using Google Forms. Eligible participants in the online survey were expected to be 18 years of age or older, a Nigerian citizen and resident in Nigeria, read and understand English with access to the internet. The COVID-19 vaccine acceptance rate was evaluated using established questionnaires [6],[19], and three independent reviewers undertook a pretest to assess readability, comprehensibility, and validity. However, the questionnaire utilized in this study was tailored to be short, concise, and easy to understand.
2.2. Analytical measures
A structured questionnaire was developed in the English language to collect data. This questionnaire comprised of 8 questions under four main themes, as follows: (1) demographics, which surveyed participants' socio-demographic information; (2) attitudes toward COVID-19; (3) willingness to accept a COVID-19 vaccine, and (4) reason for not accepting a COVID-19 vaccine. The questionnaire was pre-validated by three independent reviewers, and a pre-test study was conducted with four individuals. Demographics such as personal details, including age, gender, level of education, and average monthly income, were collected. The overall attitude towards COVID-19 was measured using a one-item question, “Do you think COVID-19 is real?”—with a Yes or No option. Among the variables assessed was the willingness of respondents to receive a COVID-19 vaccine when it is widely available in Nigeria. To be more specific, participants were asked: “Will you accept the COVID-19 vaccine if it is generally available in Nigeria?”, and the three potential answers were “Yes”, “No” and “Don't know.” Afterwards, No and Don't Know responses were considered No to dichotomize the acceptance variable during statistical analysis. To gain insight into the reason for vaccine refusal and hesitancy, participants who answered No or Don't know to the question about willingness to accept a COVID-19 vaccine were asked two more questions (i) “Why won't you accept COVID-19 vaccine?”, and the six potential answers were “religious reasons”, “lack of trust in the government”, “I will contract COVID-19 if I take the vaccine”, “I don't the vaccine is safe”, “no much information about the vaccine”, or “other: which allowed the participants to input their reason for COVID-19 vaccine refusal or hesitancy”; and (ii) “what will encourage you to accept a COVID-19 vaccine?”, and the potential answers were “I will accept COVID-19 vaccine if more people take it”, “I will accept COVID-19 vaccine if more information about the vaccine is given”, or “Nothing will make me accept COVID-19 vaccine.”
2.3. Statistical analysis
Descriptive statistics were used to outline the demographic characteristics of the sample population. Univariable analyses of the participants' demographic characteristics and willingness of respondents to accept COVID-19 in Nigeria were carried out using the chi-square tests. Furthermore, we conducted univariable logistic regression to compute odds ratios (OR) and the 95% confidence interval (CI) and corresponding P-values for the association between each socio-demographic characteristic and vaccine acceptance. 2-tailed statistical tests were used in this study, with a P-value of <0.05 considered statistical significance. Statistical analyses were conducted using SAS JMP Statistical Discovery™ Software version 14.3 (SAS Institute, Cary, North Carolina, USA).
2.4. Ethical consideration
This study followed all the ethical protocols involved in conducting studies involving human participants recommended by the National Health Research Ethics Committee of Nigeria, Federal Minister of Health. The ethical approval consent for this study was obtained from Abia State Teaching University Hospital Ethics and Research Committee with approval number/ID ABSUTH/MAC/117/VOL.11/74. Participants were aware of the purpose of the study and provided informed consent before accessing the questionnaire. Respondents were also provided with information that stated their participation was voluntary, and confidentiality and anonymity were also ensured.
3.
Results
3.1. Socio-demographic characteristics of participants
The respondents' characteristics and a summary of the survey questions are listed in Table 1. Of the total 617 respondents recruited in the survey, 56.8% were between 25 and 54 years old. Of all participants, 49% were male, 51% were female, 30.7% of all participants earned more than $134 per month. In comparison, most of our respondents had received a university degree (62%), followed by 17.3% who are still undergraduates.
3.2. Health belief
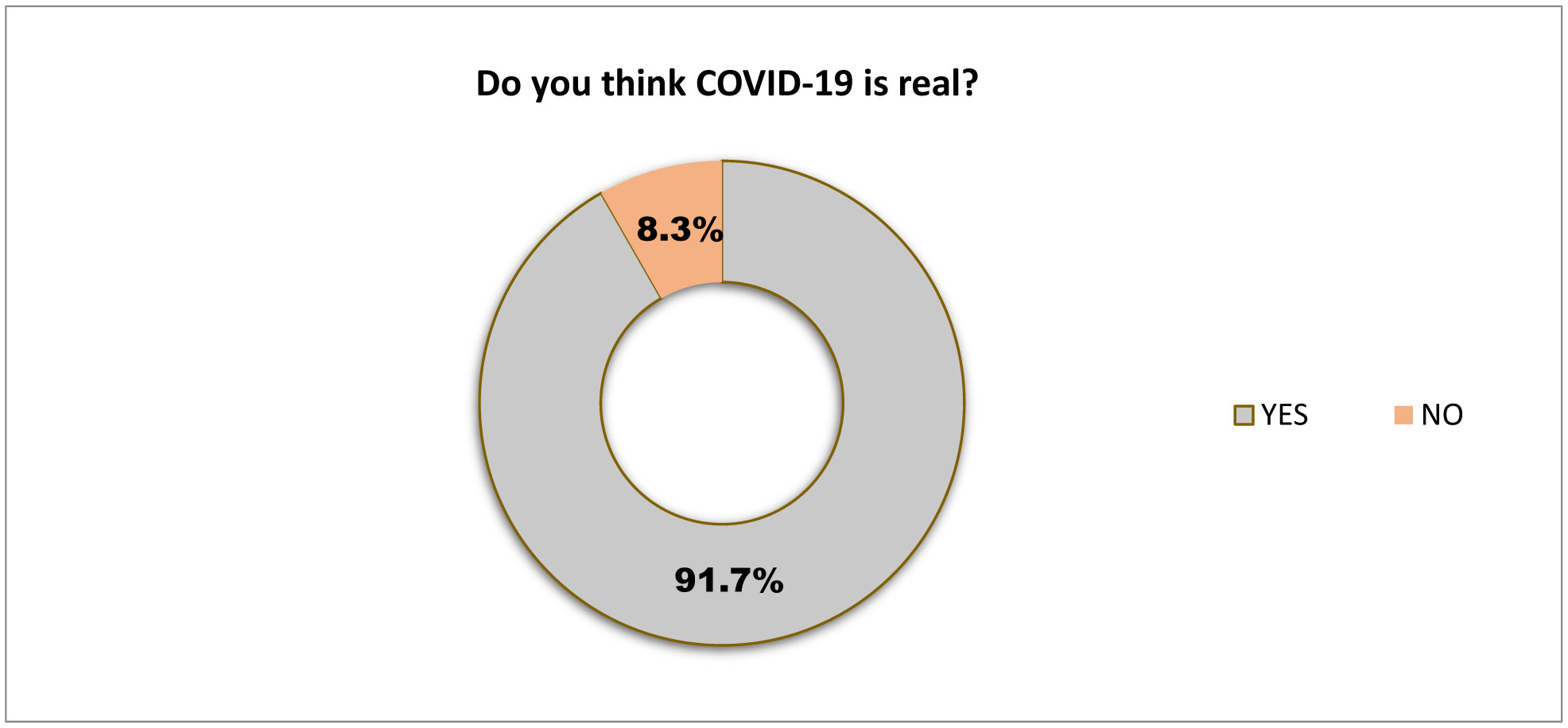
As shown in Figure 1 significant proportion of the participants, 91.7% (n = 567), believed COVID-19 was not a hoax. However, surprisingly, a few participants, 8.3% (n = 51), agreed that there was no COVID-19 in Nigeria. Furthermore, 46.2% (n = 160) of the participants reported that they would only receive the COVID-19 vaccine if given adequate information about the vaccine, 35.3% (n = 122) reported that nothing would persuade them to accept the COVID-19 vaccine, and 18.5% (n = 64) reported that they would only take the vaccine if taken by many in public.
3.3. Factors associated with acceptance and non-acceptance of the COVID-19 vaccine
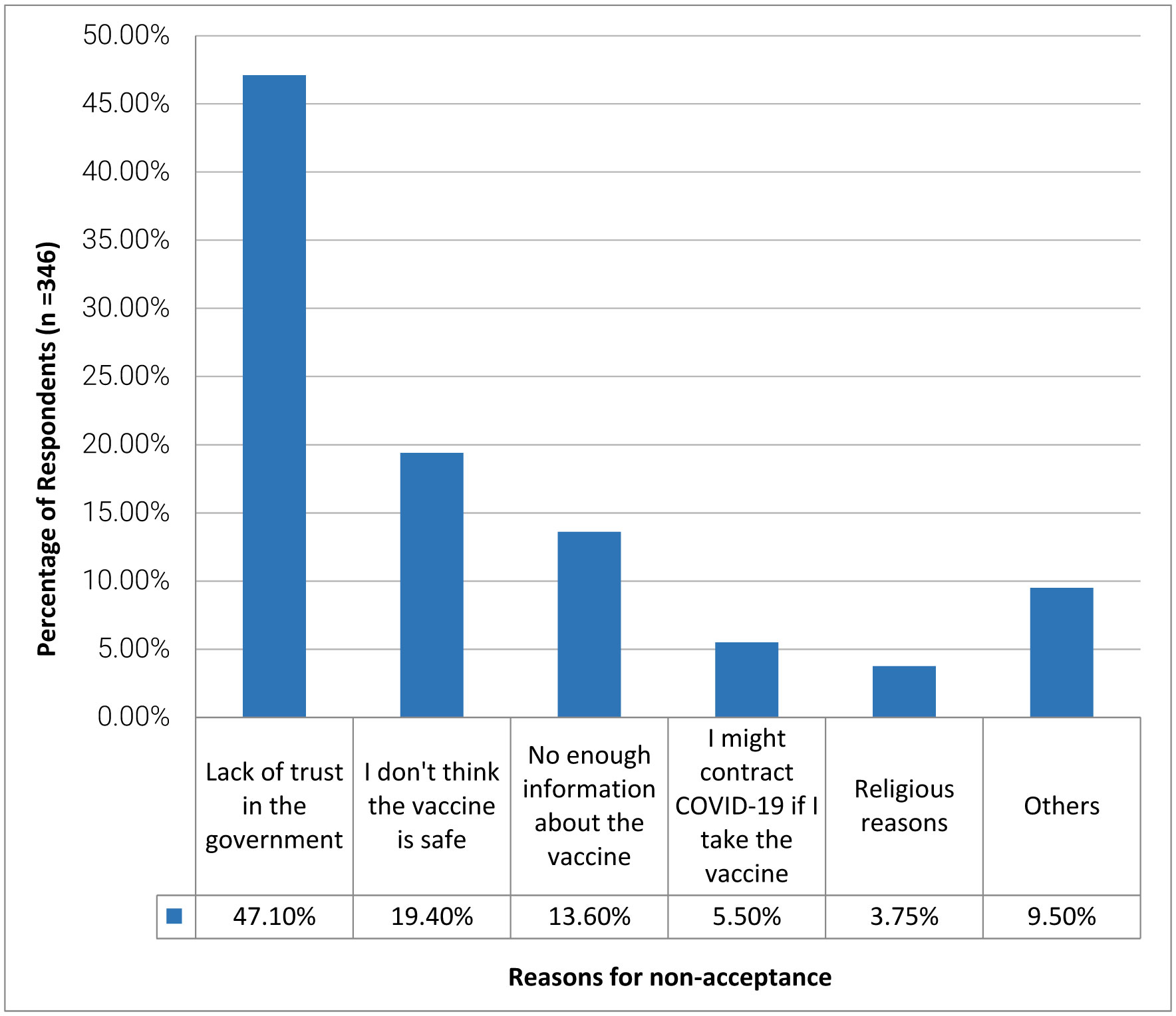
Factors associated with the willingness of respondents to accept COVID-19 in Nigeria are presented in Table 2. In total, 44% (n = 272) of respondents reported their willingness to get vaccinated against COVID-19, while 33.3% (n = 206) confirmed that they are unwilling to receive a COVID-19 vaccine and 22.7% (n = 140) reported that they are unsure. The major reason for the non-acceptance of the COVID-19 vaccine among the respondents is a lack of trust in the government 47.1% (n = 163), followed by the belief that the vaccine is not safe 19.4% (n = 67), and not enough information about the vaccine 13.6% (n = 49). Other reasons for COVID-19 vaccine refusal were fear of contracting COVID-19 through the vaccine 5.5% (n = 21) and religious reasons 3.75% (n = 13) as shown in Figure 2. Our results revealed that gender (χ2 = 8.95, P-value = 0.011), educational level (χ2 = 30.62, P-value < 0.001) and age (χ2 = 14.68, P-value = 0.023) are statistically associatied with with willingness to accept COVID-19 vaccine. We also observed that males were significantly associated with lower odds of accepting vaccines than females (OR, 0.64; 95% CI, 0.46 –0.88). However, income level, educational status, and age were not significantly associated with willingness to accept the COVID-19 vaccine (Table 2).
4.
Discussion
Like any other vaccination program, the success of the current COVID-19 vaccination program depends on the level of acceptance. A low rate of vaccination coverage due to non-acceptance directly correlates with not achieving herd immunity and exposure to the most vulnerable population. As a result, it is critical to understand the people's intention to accept the COVID-19 vaccine, thus, giving time to design and carry out targeted public health interventions and awareness campaigns to inform the public about the importance of vaccination. After all, as demonstrated in this study, 46.2% (n = 160) of the participants reported that they would only receive the COVID-19 vaccine if given adequate information about the vaccine. This suggests that hesitancy towards COVID-19 vaccine can be swayed towards acceptance if presented with the correct information. In this context, the aim of this nationwide cross-sectional survey conducted in Nigeria was to evaluate the vaccination intents of the general adult population and factors that might affect acceptance, refusal, and hesitancy. Previous studies [6],[20] in the area of COVID-19 vaccine acceptance and hesitancy have been carried out. However, the fact that vaccine decisions are multifactorial [8],[21], and can alter over time makes this study relevant. Its results are expected to influence public engagement measures taken by public health officials.
Findings from our study revealed that a significant proportion of individuals, 56% were unwilling or unsure about receiving a COVID-19 vaccine, and only 44% stated that they would get vaccinated. Based on the estimates of the reproduction number (R0) [22],[23] or through vaccination, this percentage of people willing to get vaccinated is not strong enough to achieve herd immunity. Comparing the acceptance rate of our study with that of similar studies conducted in China (97%), Brazil (88%), Australia (88%), India (87%), South Africa (64%), Russia (54%), Poland (56%), Hungary (56%), and France (59%), according to a survey by World Economic Forum's Ipsos involving nearly 20000 adults [24], and or other European countries (ranged from 62 to 80%) [25], it seems like Nigerians are more hesitant towards COVID-19 vaccine compared to other study populations. Furthermore, studies in Indonesia [26] and Malaysia [27] reported 93.3% and 94.3% acceptance rates, respectively. Our “respondents” willingness rate is higher than reported in the Democratic Republic of Congo [28]. Contrarily, the two previous studies focused on Nigerian populations reported willingness rates of 74% [20] and 65.22% [6]. These contrasting findings further suggest that vaccination decision is an evolving phenomenon that changes over time, and if rates of acceptance remain low, the COVID-19 pandemic will continue to stifle public health systems and the country's economy. As an antidote, the Nigerian public health officials need to design and implement an awareness campaign urgently to help boost acceptance rates.
Higher levels of education and low monthly income were also associated with high vaccine acceptance rates. These findings align with studies in the UK [29] and Australia [30]. Among demographically defined groups, the willingness rate was least among those with lower education, while a study in Greece [31] demonstrated the opposite. Therefore, while its imperative to design effective vaccine communication strategies, it is crucial to carefully consider the general literacy level of the subpopulations [21], as well as identify traditionally and locally trusted means of communication [8] that go beyond simply voicing that vaccines are safe and effective. Interestingly, as drivers of vaccine hesitancy rely greatly on both belief systems and personal experiences [31], it will be more effective to boost vaccine literacy and acceptance by directly addressing community-specific concerns or misconceptions, socio-cultural issues, religious or philosophical beliefs that breed distrust in vaccination programs. Although researchers have identified behavioural insights, culturally appropriate and evidence-based communications are promising interventions for building confidence and reducing vaccine hesitancy in different contexts [32],[33]. Other promising tools have been developed primarily for high-income countries [34]. However, these tools will require careful adjustment to make them suitable for use in African nations. Therefore, it is paramount to adapt and validate such existing vaccine hesitancy measurement tools or develop new tools capable of monitoring COVID-19 vaccine hesitancy across African countries, enhancing the comparability of research findings, and facilitating a more robust evidence-based intervention.
Generally, women in this study were less likely to accept vaccines than men; however, this association was not strong. This finding could help the Nigerian government, health professionals, policymakers, and non-governmental organizations to target interventions around COVID-19 vaccination programs effectively. Furthermore, about 56% of our study population showed unwillingness in accepting the COVID-19 vaccine. Comparatively, these results were significantly greater than what was reported in Russia (47%), Poland (45%), Hungary (44%), France (41%), South Africa (36%), Sweden (33%), United States (33%), Germany (33%), Italy (33%), Brazil (12%), Australia (12%), India (13%), Malaysia (15%), UK (15%), Saudi Arabia (16%), South Korea (16%), Peru (21%), China (3%), and Canada (24%) according to a recent survey by World Economic Forum's Ipsos involving nearly 20000 adults on whether they will take COVID-19 vaccine or not when it is available [24].
Our findings revealed that trust is not only an intrinsic component of a vaccination program but also a changeable factor in the successful uptake of a COVID-19 vaccine. Furthermore, our findings show that lack of trust in the government is strongly associated with vaccine refusal and can contribute to non-compliance of the public with recommended protocols. From our observation, the top three reasons for the non-acceptance of the COVID-19 vaccine are lack of trust in the government, the belief that the vaccine is not safe, and concerns there is not enough information about the vaccine. Lessons from past infectious disease outbreaks have pointed out that one of the key contributors to disease control is trusted information and guidance [35]. However, its multifactorial, complex, and context-dependent characteristics would take more than building trust to adequately address vaccine hesitancy. Hence, it is equally important to address vaccine hesitancy simultaneously at federal, state, and local levels.
As this study has shown, it is paramount that the government and public health officials adopt clear, consistent, and competent communication to build public confidence in the COVID-19 vaccination program. This includes, but is not limited to, using a simple linguistic approach to explaining how vaccines work and how they are developed, including regulatory approval based on safety and efficacy. In a similar vein, effective campaigns should be designed to carefully explain the COVID-19 vaccine's level of effectiveness, the required period for protection if double doses are required, and the significance of comprehensive coverage in achieving herd immunity. Above all, credible and culturally informed health communication would be influential in swaying the minds of the fence-sitters towards COVID-19 vaccine acceptance.
5.
Limitations
In interpreting the results of this current study, it is essential to consider some limitations. Firstly, our study used an online survey, involving only those who can read or write English and have access to the internet which may have resulted in sampling bias; hence, the results may not be an accurate representation of the wider population. One of the core disadvantages of online-based surveys is the generality and validity of results, which mandates a careful analysis and interpretation of the research findings [36],[37]. Our survey results show that participants between the ages of 24–54 are over-represented, while older adults aged 65 and above are under-represented. Secondly, the responses from this nationwide survey were focused on self-report. As a result, it may likely be subject to self-reporting bias and a likelihood of respondents choosing socially desirable responses. Further investigation using other methodologically appropriate tools is needed to conclusively assess vaccination willingness in different behavioural and sociocultural contexts.
6.
Conclusions
Contrary to previous studies conducted before the COVID-19 vaccine, this present study has shown that individual vaccination intention can change over time, particularly when a vaccine becomes available. Summarily in March 2021, more than half of the adult population surveyed reported being hesitant towards the Covid-19 vaccine. Women, younger people aged 18–24, and less educated individuals could be potential target groups for strategic interventions aiming to sway the public toward COVID-19 vaccination acceptance. These findings are expected to provide valuable insights to government agencies, public health officials, and health care workers to address the impact of vaccine hesitancy. Furthermore, this observation highlights the need to implement immediate awareness-raising measures to communicate and engage with the public toward COVID-19 vaccine acceptance.









 DownLoad:
DownLoad: