Evaluation of a radial basis function node refinement algorithm applied to bioheat transfer modeling

Rafael Pinheiro Amantéa; Robspierre de Carvalho; Luiz Otávio Barbosa; Rafael Pinheiro Amantéa; Robspierre de Carvalho; Luiz Otávio Barbosa

doi:10.3934/bioeng.2021005

AIMS Bioengineering

2021, Volume 8, Issue 1: 36-51. doi: 10.3934/bioeng.2021005

Previous Article Next Article

Research article

Evaluation of a radial basis function node refinement algorithm applied to bioheat transfer modeling

Department of Engineering and Management of Process and Systems, IETEC – Institute of Technological Education, Belo Horizonte, Minas Gerais, 30140-138, Brazil.

Received: 30 October 2020 Accepted: 17 December 2020 Published: 23 December 2020

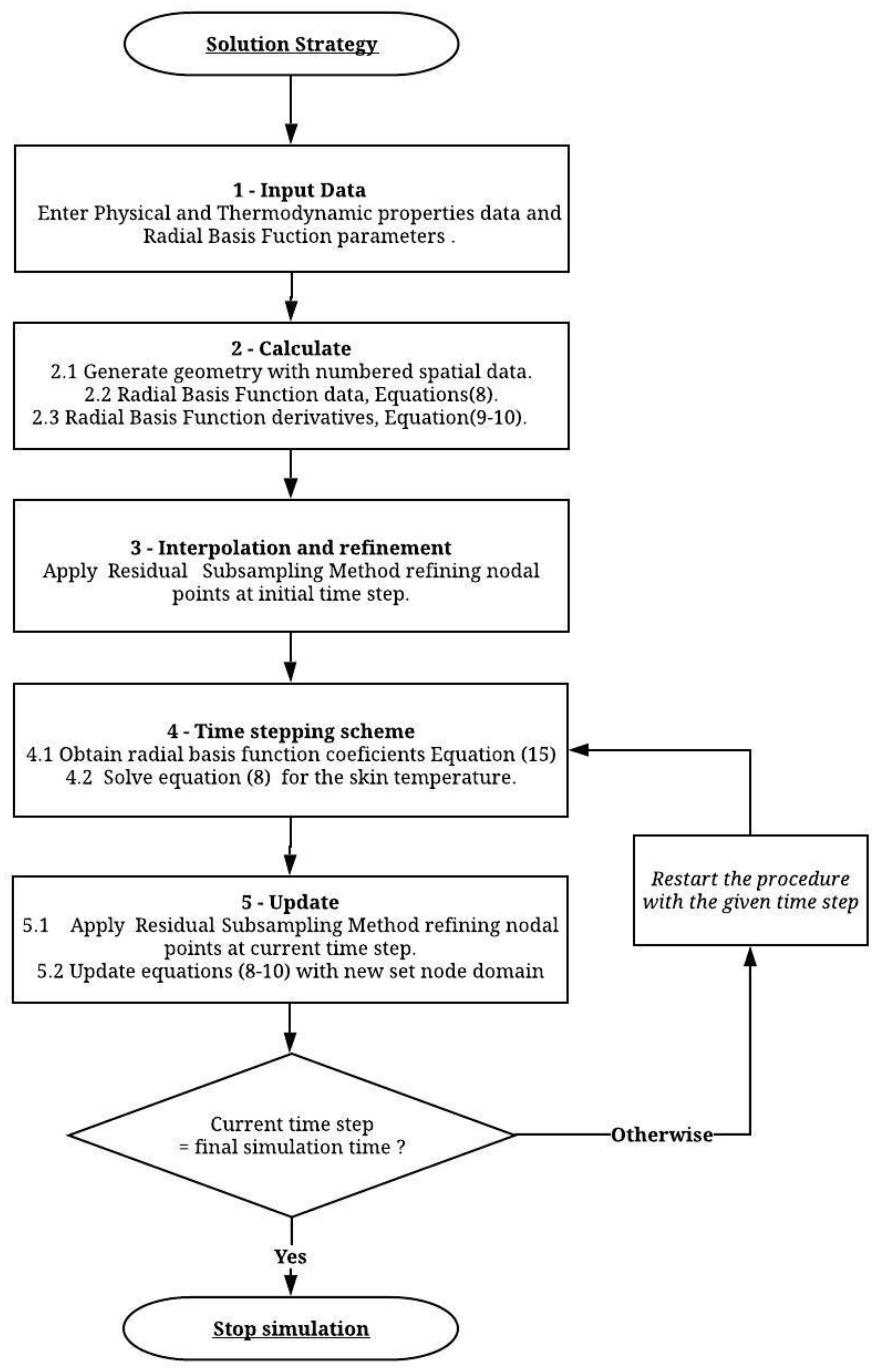
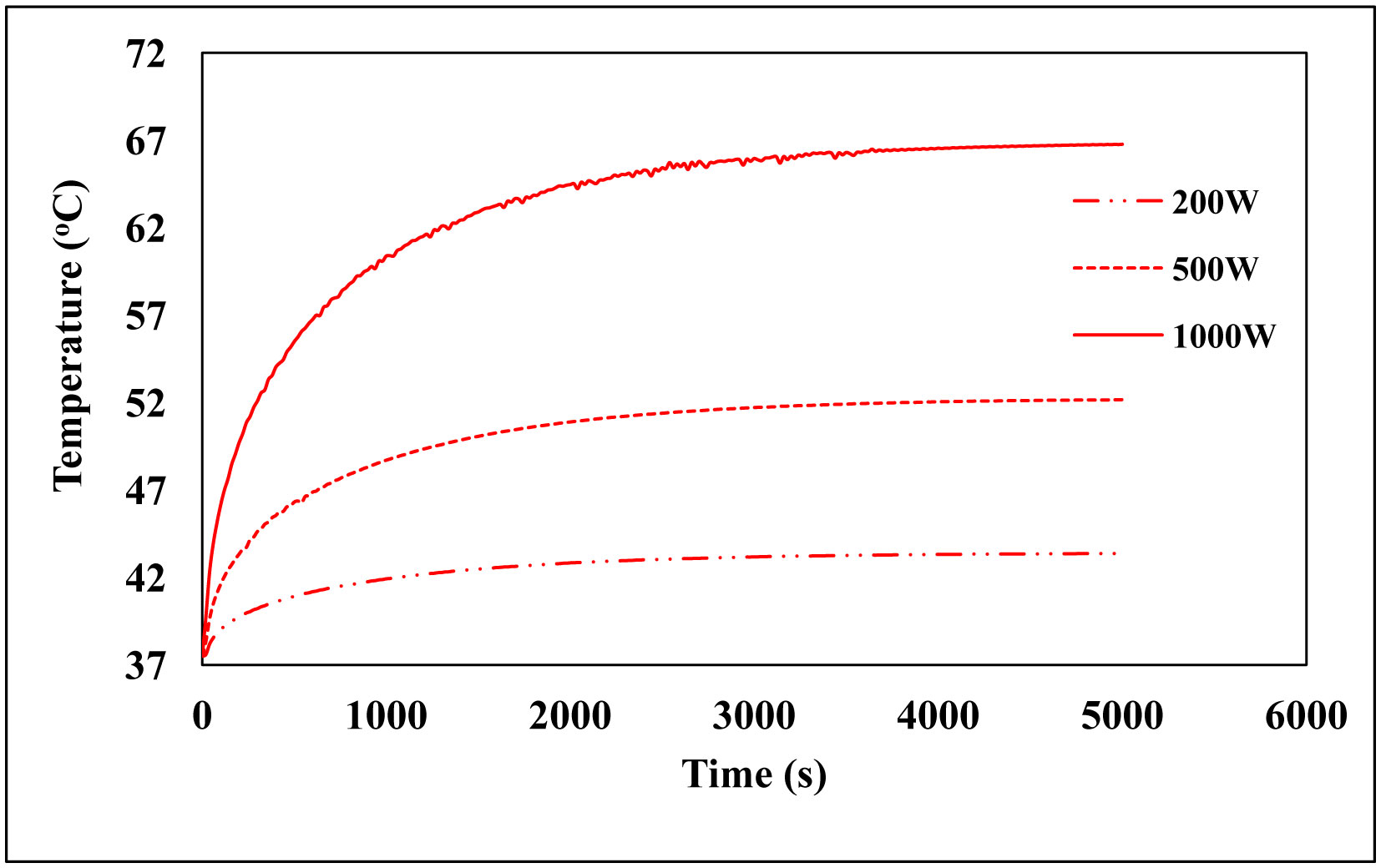
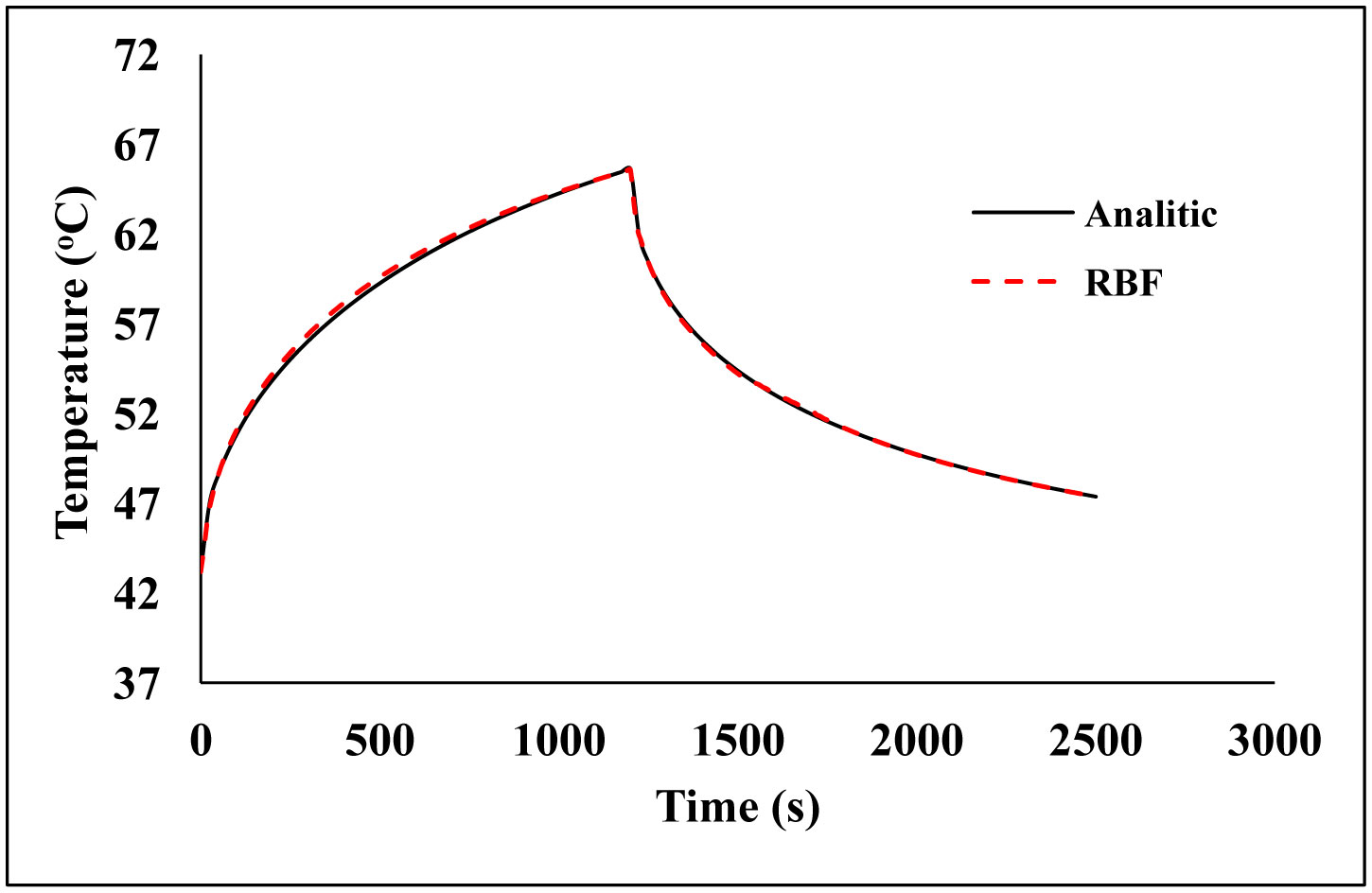
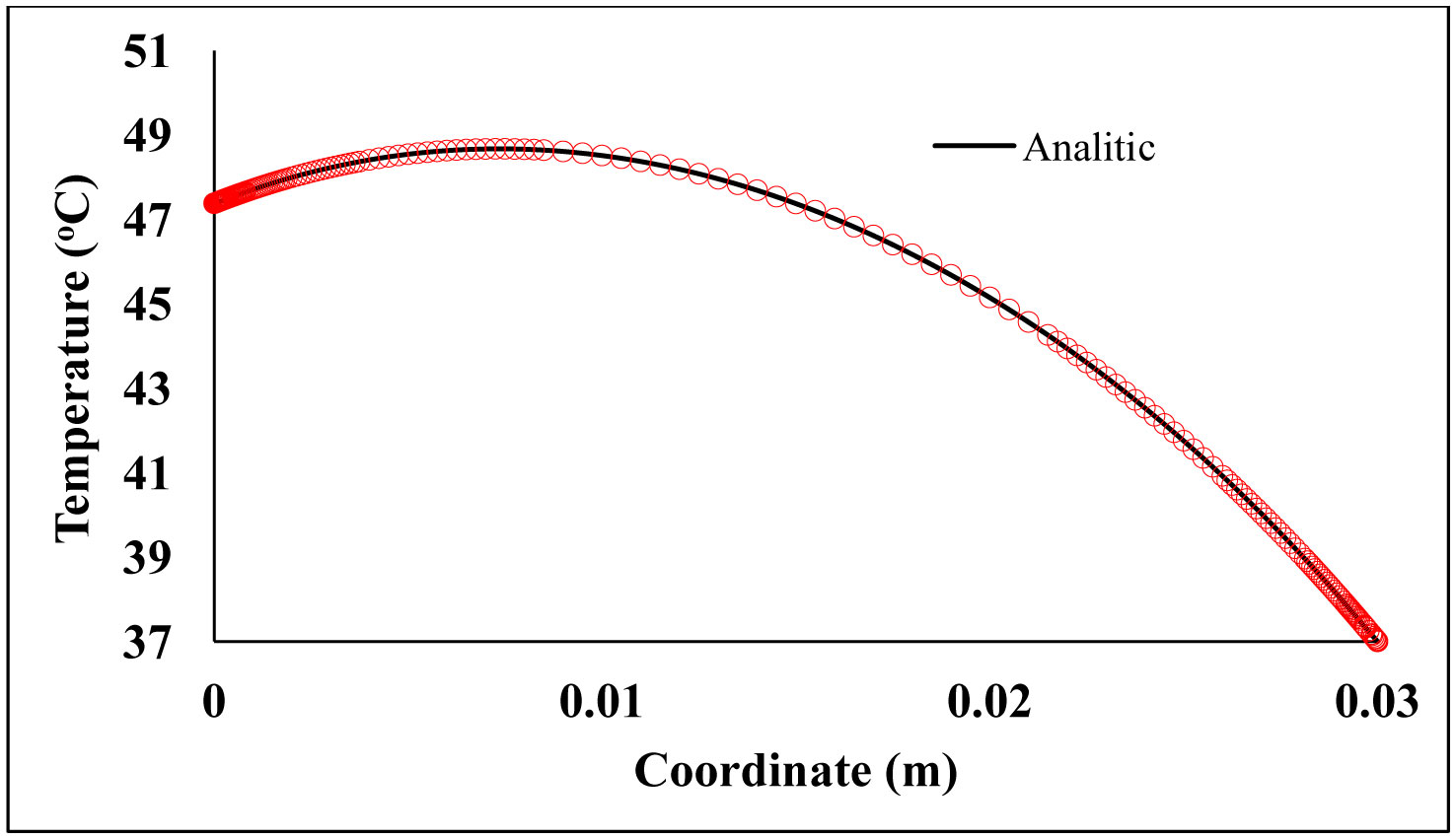
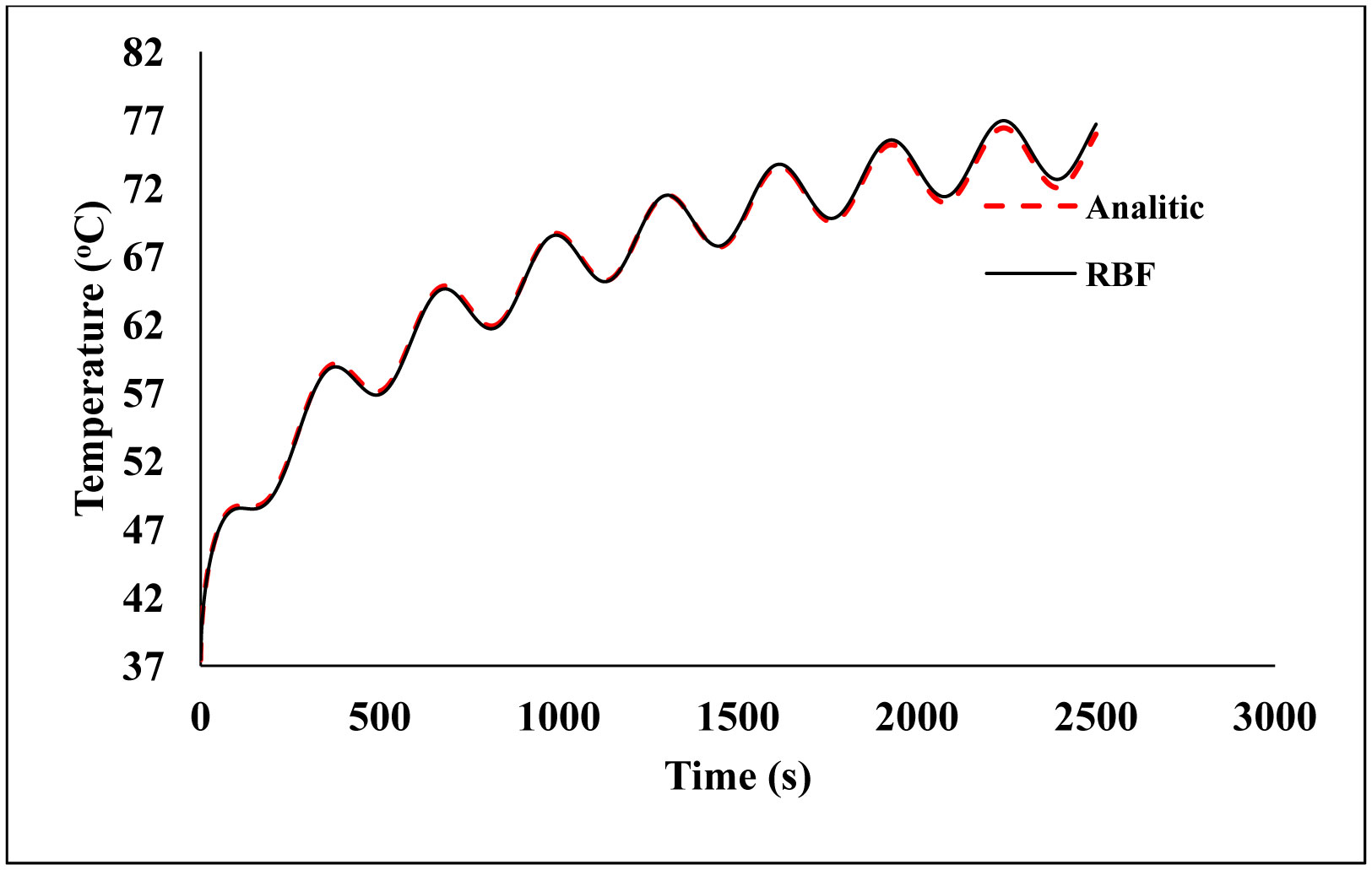
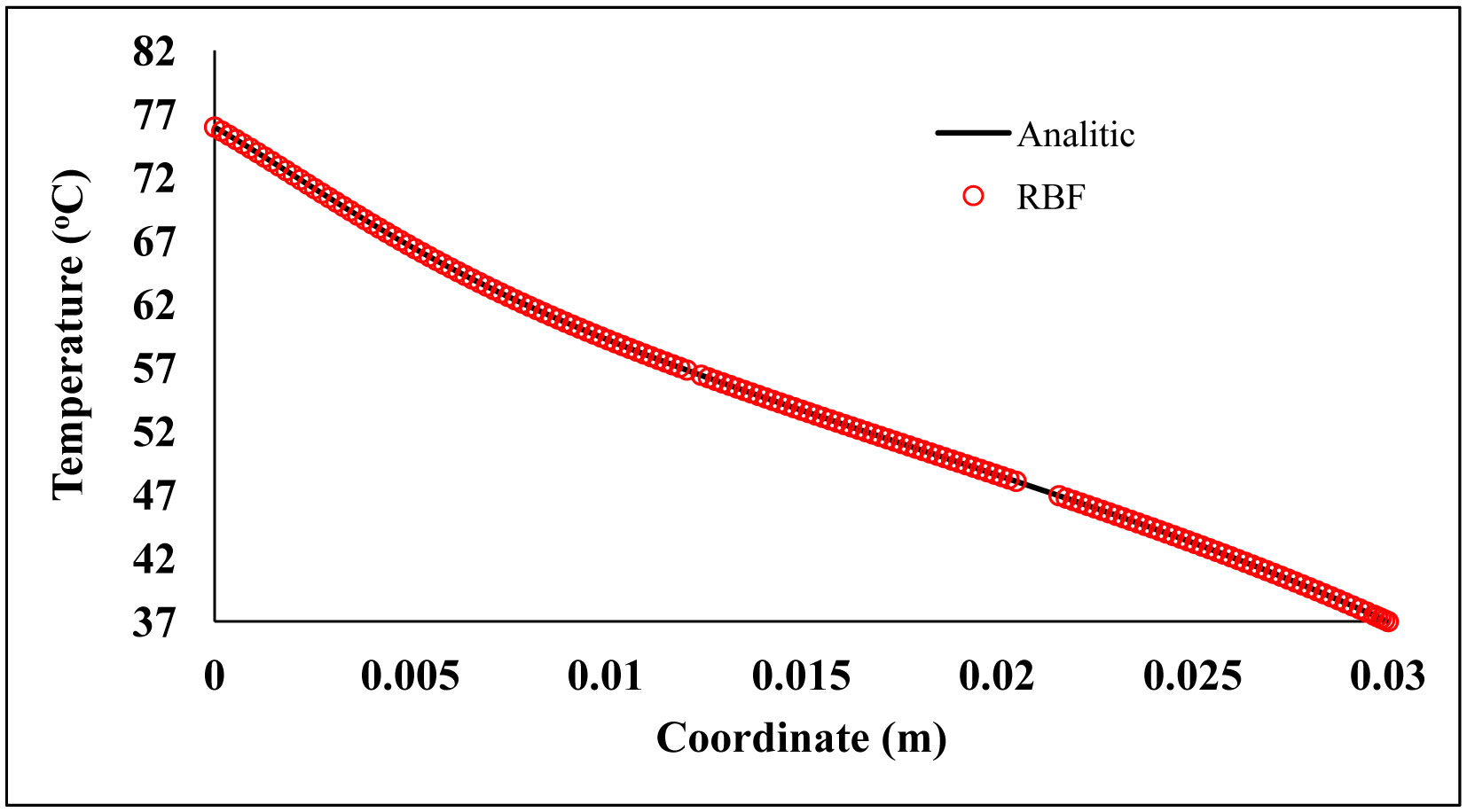
Many bioheat transfer problems involve linear/non-linear equations with non-linear or time-dependent boundary conditions. For heat transfer problems, the presence of time and space-dependent functions under Neumann and Mixed type boundary conditions characterize trivial applications in bioengineering, such as thermotherapies, laser surgeries, and burn studies. This greatly increases the complexity of the numerical solution in several problems, requiring fast and accurate numerical solutions. This paper has a main objective evaluate an adaptive mesh refinement radial basis function method strategy for the classical Penne's bioheat transfer modeling. Our numerical results had errors of ~0.1% compared to analytical solutions. Thus, the proposed methodology is accurate and has a low computational cost. For step function heating, two RBF shape parameters were applied, again achieving excellent results. The distributions of the nodes in the solution domain show that the primary source of error in the numerical solutions came from the boundary conditions. This finding should arouse the interest of engineers and scientists in the development of new strategies for problems involving boundary conditions with periodic functions.

Keywords:

Citation: Rafael Pinheiro Amantéa, Robspierre de Carvalho, Luiz Otávio Barbosa. Evaluation of a radial basis function node refinement algorithm applied to bioheat transfer modeling[J]. AIMS Bioengineering, 2021, 8(1): 36-51. doi: 10.3934/bioeng.2021005

Related Papers:

[1]	Shahida Bashir, Rabia Mazhar, Bander Almutairi, Nauman Riaz Chaudhry . A novel approach to study ternary semihypergroups in terms of prime soft hyperideals. AIMS Mathematics, 2023, 8(9): 20269-20282. doi: 10.3934/math.20231033
[2]	Shahida Bashir, Ahmad N. Al-Kenani, Maria Arif, Rabia Mazhar . A new method to evaluate regular ternary semigroups in multi-polar fuzzy environment. AIMS Mathematics, 2022, 7(7): 12241-12263. doi: 10.3934/math.2022680
[3]	Moquddsa Zahra, Dina Abuzaid, Ghulam Farid, Kamsing Nonlaopon . On Hadamard inequalities for refined convex functions via strictly monotone functions. AIMS Mathematics, 2022, 7(11): 20043-20057. doi: 10.3934/math.20221096
[4]	Adnan, Waseem Abbas, Sayed M. Eldin, Mutasem Z. Bani-Fwaz . Numerical investigation of non-transient comparative heat transport mechanism in ternary nanofluid under various physical constraints. AIMS Mathematics, 2023, 8(7): 15932-15949. doi: 10.3934/math.2023813
[5]	Tao Yan, Ghulam Farid, Sidra Bibi, Kamsing Nonlaopon . On Caputo fractional derivative inequalities by using strongly $ (\alpha, h-m) $-convexity. AIMS Mathematics, 2022, 7(6): 10165-10179. doi: 10.3934/math.2022565
[6]	Tareq Saeed, Muhammad Adil Khan, Hidayat Ullah . Refinements of Jensen's inequality and applications. AIMS Mathematics, 2022, 7(4): 5328-5346. doi: 10.3934/math.2022297
[7]	Yang Pan, Yan Liu . New classes of few-weight ternary codes from simplicial complexes. AIMS Mathematics, 2022, 7(3): 4315-4325. doi: 10.3934/math.2022239
[8]	M. Zakarya, Ghada AlNemer, A. I. Saied, H. M. Rezk . Novel generalized inequalities involving a general Hardy operator with multiple variables and general kernels on time scales. AIMS Mathematics, 2024, 9(8): 21414-21432. doi: 10.3934/math.20241040
[9]	Ling Zhu, Zhengjie Sun . Refinements of Huygens- and Wilker- type inequalities. AIMS Mathematics, 2020, 5(4): 2967-2978. doi: 10.3934/math.2020191
[10]	Muhammad Adil Khan, Josip Pečarić, Yu-Ming Chu . Refinements of Jensen’s and McShane’s inequalities with applications. AIMS Mathematics, 2020, 5(5): 4931-4945. doi: 10.3934/math.2020315

Abstract

Nomenclature

Symbol	Description	Unit
θ_c	Coarse node parameter	-
θ_r	Refine node parameter	-
ω_b	Blood perfusion	m³/s/m³
Ø	Radial basis function	-
Δt	Numerical time step	s
c	Specific heat of tissue	J/(kg·K)
c_b	Specific heat of blood	J/(kg·K)
h₀	Heat convection coeficient	W/(m²·K)
k	Thermal conductivity of tissue	W/(m·K)
L	Distance between skin surface and body core	m
Q_m	Metabolic rate of tissue	W/m³
Q_r	Spatial heating	W/m³
r	Euclidean distance	-
t	Time	s
T	Tissue temperature	°C
T₀	Steady state temperature	°C
T_a	Arterial temperature	°C
T_c	Blood temperature	°C
T_f	Fluid temperature	°C
x	Spatial coordinate	m
α	Thermal diffusivity	m²/s
ϵ	Multiquadric shape parameter	-
λ	Radial basis function interpolator	-
ρ	Density of tissue	Kg/m³
ρ_b	Density of blood	Kg/m³

1. Introduction

The refinement schemes can be viewed as a class of iterative algorithms. The main feature of these schemes is that they use initially the rough sketch of any curve called polygon to finally produce smooth sketch also called refined sketch. Initially, a refinement scheme was introduced by de Rahm ^[3] in 1956. Later on, Chaikin ^[1] introduced the scheme for curve generation in 1974. Deslauriers and Dubuc presented the interpolating schemes for curve modeling in 1989. This triggered off the modern era for the investigation of the refinement schemes. These refinement schemes have been used for curve and surface modeling for a long time by designers, engineers, computer scientists and mathematicians. The details of these applications are presented by Farin ^[7].

Initially, the binary refinement schemes were introduced. These schemes have two rules to refine each edge of the polygon. These rules are same for each edge of the polygon except the initial and final edges. These rules are classified as even and odd rules. These schemes take initial sketch as an input and return the refined sketch as an output. In the next step, these schemes take previously refined sketch as an input and produce more refined sketch. Repeated application of this procedure give the smooth curve/shape. Nowadays, there are many generalizations of the binary schemes. The class of ternary refinement schemes is one of the generalization of the class of binary schemes. In this class of the schemes, three rules are used instead of two rules to refine each edge of the polygon.

The analysis of the schemes is the crucial issue. Initially, Dyn et al. ^[4], introduced the divided difference (DD) technique to analyze the schemes in 1987. Later on, Dyn et al. ^[5], extended this technique for the analysis of the class of binary schemes in 1991. The study of the limiting curves produced by the binary refinement schemes was also presented by Micchelli and Prautzch ^[11] in 1989. Sabin ^[15] in 2010, gave further insight in this technique. The further extension of Dyn et al.'s work was done by Mustafa and Zahid ^[12] in 2013. The cross-differences of directional DD techniques was introduced by Qu ^[14] in 1991. The further generalization of the DD technique has not been done so for.

Another technique for the smoothness analysis of the schemes was introduced by Dyn ^[6] in 2002. This technique is known as Laurent polynomial technique. This technique was used by Hassan and Dodgson ^[8], Hassan et al. ^[9], Mustafa et al. ^[13]. Khan and Mustafa ^[10], Siddiqi and Rehan ^[16], Zheng et al. ^[17] and many others to analyze their binary and ternary refinement schemes. Nowadays many authors are also using this technique. However, this technique also has some limitations and need improvement. In this technique, first a sequence of coefficients used in the refinement rules of the schemes is converted into the polynomial. Secondly, the polynomial has been factorized. In this technique, multiplication, division, factorization of the polynomials are involved. Further, the computation of inequalities and the comparison of the terms are also involved. The technique, we are going to introduce is the generalization of DD technique. In this technique, the computation of inequalities and the comparison of three terms are involved. There are three simple algebraic expressions in each inequality. Further these algebraic expressions contain only the coefficients used in the refinement rules of the schemes.

The rest of this paper is structured as follows. In Section 2, a general ternary refinement scheme and its DD schemes are introduced. Their convergence is also presented in this section. In Section 3, the deviation between successive levels of polygons produced by the ternary and its DD refinement schemes are presented. The inequalities for the analysis of ternary schemes are presented in Section 4. Applications of these inequalities are also presented in this section. Summary of the work and comparison are presented in Sections 5 and 6 respectively.

2. Ternary and its DD refinement schemes

We first introduce the ternary and its first, second and third order divided differences (DD) refinement schemes. Then we present their convergence in this section.

2.1. Ternary refinement scheme (TRS)

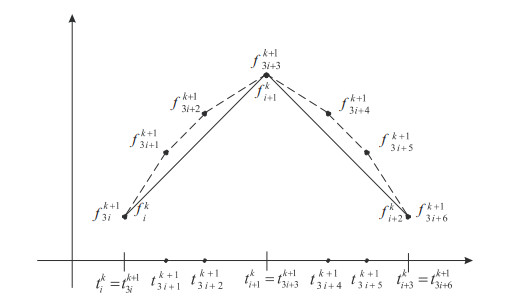
Let $f^{k} = \{\left (i/3^k, f^{k}_{i} \right) \in \mathbb{R}^{N}, \ i \in \mathbb{Z}, \ N \geq 2, \ k \geq 0 \}$ , be a polygon at $k$ th refinement level then the $(k+1)$ th polygon $f^{k+1}$ , can be obtained by the following three refinement rules

$\begin{equation} \left\{\begin{array}{l} f^{k+1}_{3i} = \sum\limits_{j = 0}^{m}\rho_{j}f^{k}_{i+j}, \\ f^{k+1}_{3i+1} = \sum\limits_{j = 0}^{m}\varrho_{j}f^{k}_{i+j}, \\ f^{k+1}_{3i+2} = \sum\limits_{j = 0}^{m}\sigma_{j}f^{k}_{i+j}. \end{array}\right. \end{equation}$

(2.1)

whereas $m > 0$ while if $t^{k}_{i} = i/3^{k}$ then $\left (t^{k+1}_{3i} = t^{k}_{i}, \ f^{k+1}_{3i} \right)$ , $\left (t^{k+1}_{3i+1} = \frac{1}{3}(2t^{k}_{i}+t^{k}_{i+1}), \ f^{k+1}_{3i+1} \right)$

and $\left (t^{k+1}_{3i+2} = \frac{1}{3}(t^{k}_{i}+2t^{k}_{i+1}), \ f^{k+1}_{3i+2} \right)$ are the points of the polygon $f^{k+1}$ .

The above rules are the affine combinations of $f^{k}_{i}$ 's so

$\begin{equation} \begin{array}{l} \sum\limits_{\nu = 0}^{m}\rho_{\nu} = \sum\limits_{\nu = 0}^{m}\varrho_{\nu} = \sum\limits_{\nu = 0}^{m}\sigma_{\nu} = 1. \end{array} \end{equation}$

(2.2)

The one step of the refinement procedure is shown in Figure 1. The repeated application of these rules is known as ternary refinement scheme.

Figure 1. The part of polygons at

$k$ th and

$(k+1)$ th levels are shown by solid and dash lines respectively.

DownLoad: Full-Size Img PowerPoint

2.2. First to third order DD refinement schemes

The first, second and third order divided difference (DD) ternary refinement schemes (TRS) are presented in this section.

Lemma 2.1. Let $f^{k} = \{\left (i/3^k, f^{k}_{i} \right) \}$ be a polygon of TRS at $k$ th refinement level and $d^{[1] k}_{j} = 3^{k}(f^{k}_{j+1}-f^{k}_{j})$ be the first divided difference then the first order DD TRS is defined as

$\begin{equation} \left\{\begin{array}{l} d^{[1] (k+1)}_{3i} = 3\sum\limits_{\mu = 0}^{m-1}\left\{\sum\limits_{\nu = 0}^{\mu}(\rho_{\mu-\nu}-\varrho_{\mu-\nu}) d^{[1] k}_{i+\mu}\right\}, \\ \\ d^{[1] (k+1)}_{3i+1} = 3\sum\limits_{\mu = 0}^{m-1}\left\{\sum\limits_{\nu = 0}^{\mu}(\varrho_{\mu-\nu}-\sigma_{\mu-\nu}) d^{[1] k}_{i+\mu}\right\}, \\ \\ d^{[1] (k+1)}_{3i+2} = 3\sum\limits_{\mu = 0}^{m}\left\{\sum\limits_{\nu = 0}^{\mu}(\sigma_{\mu-\nu}-\rho_{\mu-\nu-1}) d^{[1] k}_{i+\mu}\right\}. \end{array}\right. \end{equation}$

(2.3)

Proof. Since the first order DD is defined as

$\begin{equation} d^{[1] k}_{\nu} = 3^{k}(f^{k}_{\nu+1}-f^{k}_{\nu}), \end{equation}$

(2.4)

therefore by (2.1), we get

$\begin{eqnarray} d^{[1] (k+1)}_{3i}& = &3^{k+1}\left\{\left(\varrho_{0}-\rho_{0}\right)f^{k}_{i}+\left(\varrho_{1}-\rho_{1}\right)f^{k}_{i+1} +\left(\varrho_{2}-\rho_{2}\right)f^{k}_{i+2} +\ldots\right.\\&&\left. +\left(\varrho_{m-1}-\rho_{m-1}\right)f^{k}_{i+m-1}+\left(\varrho_{m}-\rho_{m}\right)f^{k}_{i+m}\right\}. \end{eqnarray}$

(2.5)

Now consider the linear combination

$\begin{eqnarray} d^{[1] (k+1)}_{3i} = y_{0} d^{[1] k}_{i}+y_{1} d^{[1] k}_{i+1}+y_{2} d^{[1] k}_{i+2}+\ldots+y_{m-1} d^{[1] k}_{i+(m-1)}. \end{eqnarray}$

(2.6)

The goal is to find the unknown $y_{0}, \cdots, y_{m-1}$ . It can be written as

$\begin{eqnarray} d^{[1] (k+1)}_{3i}& = &3^{k}\left\{-y_{0}f^{k}_{i}+(y_{0}-y_{1})f^{k}_{i+1}+(y_{1}-y_{2})f^{k}_{i+2} +\ldots+(y_{m-3}-y_{m-2})f^{k}_{m-2} \right.\\&&\left.+(y_{m-2}-y_{m-1})f^{k}_{i+(m-1)}+y_{m-1}f^{k}_{i+m}\right\}. \end{eqnarray}$

(2.7)

Comparing (2.5) and (2.7), we get

$\begin{eqnarray*} y_{0}& = &3(\rho_{0}-\varrho_{0}), \\ y_{1}& = &3(\rho_{0}-\varrho_{0})+3(\rho_{1}-\varrho_{1}), \\ y_{2}& = &3(\rho_{0}-\varrho_{0})+3(\rho_{1}-\varrho_{1})+3(\rho_{2}-\varrho_{2}), \\ \vdots \\ y_{m-1}& = &3(\rho_{0}-\varrho_{0})+3(\rho_{1}-\varrho_{1})+\dots+3(\rho_{m-1}-\varrho_{m-1}). \end{eqnarray*}$

Substituting in (2.6), we get

$\begin{eqnarray*} d^{[1] (k+1)}_{3i}& = &3\left[\left(\rho_{0}-\varrho_{0}\right) d^{[1] k}_{i}+\left\{\left(\rho_{0}-\varrho_{0}\right) +\left(\rho_{1}-\varrho_{1}\right)\right\} d^{[1] k}_{i+1}+\left\{\left(\rho_{0}-\varrho_{0}\right)+\left(\rho_{1}- \right.\right.\right.\\&&\left.\left.\left.\varrho_{1}\right) +\left(\rho_{2}-\varrho_{2}\right)\right\} d^{[1] k}_{i+2}+\ldots+\left\{\left(\rho_{0}-\varrho_{0}\right) +\left(\rho_{1}-\varrho_{1}\right)+\dots\right.\right.\\&&\left.\left. +\left(\rho_{m-1}-\varrho_{m-1}\right)\right\} d^{[1] k}_{i+\left(m-1\right)}\right]. \end{eqnarray*}$

This implies

$\begin{eqnarray*} d^{[1] (k+1)}_{3i} = 3\sum\limits_{\mu = 0}^{m-1}\left\{\sum\limits_{\nu = 0}^{\mu}(\rho_{\mu-\nu}-\varrho_{\mu-\nu}) d^{[1] k}_{i+\mu}\right\}. \end{eqnarray*}$

Similarly, we get

$\begin{equation*} d^{[1] (k+1)}_{3i+1} = 3\sum\limits_{\mu = 0}^{m-1}\left\{\sum\limits_{\nu = 0}^{\mu}(\varrho_{\mu-\nu}-\sigma_{\mu-\nu}) d^{[1] k}_{i+\mu}\right\}, \end{equation*}$

and

$\begin{equation*} d^{[1] (k+1)}_{3i+2} = 3\sum\limits_{\mu = 0}^{m}\left\{\sum\limits_{\nu = 0}^{\mu}(\sigma_{\mu-\nu}-\rho_{\mu-\nu-1}) d^{[1] k}_{i+\mu}\right\}. \end{equation*}$

This completes the proof. By adopting the similar procedure, we get the following results.

Lemma 2.2. Let $f^{k} = \{\left (i/3^k, f^{k}_{i} \right)\}$ be a polygon of TRS at $k$ th refinement level and

$\begin{eqnarray*} d^{[2] k}_{j} = 3^{2k}(2!)^{-1}\left(f^{k}_{j-1}-2f^{k}_{j}+f^{k}_{j+1}\right) \end{eqnarray*}$

be the second order divided difference then the second order DD TRS is defined as

$\begin{equation} \left\{\begin{array}{l} d^{[2] (k+1)}_{3i} = 3^{2}\sum\limits_{\mu = 0}^{m-1}\left[\sum\limits_{\nu = 0}^{\mu}\left\{(\nu+1)\sigma_{\mu-\nu}+\nu\varrho_{\mu-\nu}-2\nu\rho_{\mu-\nu}\right\} d^{[2] k}_{i+\mu}\right], \\ \\ d^{[2] (k+1)}_{3i+1} = 3^{2}\sum\limits_{\mu = 0}^{m-2}\left[\sum\limits_{\nu = 0}^{\mu}\left\{(\nu+1)(\rho_{\mu-\nu}+\sigma_{\mu-\nu}-2\varrho_{\mu-\nu})\right\} d^{[2] k}_{i+\mu+1}\right], \\ \\ d^{[2] (k+1)}_{3i+2} = 3^{2}\sum\limits_{\mu = 0}^{m-1}\left[\sum\limits_{\nu = 0}^{\mu}\left\{\nu\rho_{\mu-\nu}+(\nu+1)\varrho_{\mu-\nu}-(2\nu+2) \sigma_{\mu-\nu}\right\} d^{[2] k}_{i+\mu+1}\right]. \end{array}\right. \end{equation}$

(2.8)

Lemma 2.3. Let $f^{k} = \{\left (i/3^k, f^{k}_{i} \right)\}$ be a polygon of TRS at $k$ th refinement level and

$\begin{eqnarray*} d^{[3] k)}_{j} = 3^{3k}\left(3!\right)^{-1}\left(-f^{k}_{j-1}+3f^{k}_{j}-3f^{k}_{j+1}+f^{k}_{j+2}\right) \end{eqnarray*}$

be the third order divided difference then the third order DD TRS is defined as

$\begin{equation} \left\{\begin{array}{l} d^{[3] (k+1)}_{3i} = 3^{3}\sum\limits_{\mu = 0}^{m-2}\left[\sum\limits_{\nu = 0}^{\mu}\left\{(\nu+1)\sigma_{\mu-\nu}- \frac{3\nu(\nu+1)}{2}\left(\rho_{\mu-\nu}-\varrho_{\mu-\nu}\right)\right\} d^{[3] k}_{i+\mu}\right], \\ \\ d^{[3] (k+1)}_{3i+1} = 3^{3}\sum\limits_{\mu = 0}^{m-2}\left[\sum\limits_{\nu = 0}^{\mu}\left\{(\nu+1)\rho_{\mu-\nu}- \frac{3(\nu+1)(\nu+2)}{2}(\varrho_{\mu-\nu}-\sigma_{\mu-\nu})\right\} d^{[3] k}_{i+\mu+1}\right], \\ \\ d^{[3] (k+1)}_{3i+2} = 3^{3}\sum\limits_{\mu = 0}^{m-2}\left[\sum\limits_{\nu = 0}^{\mu}\left\{\frac{3\nu(\nu+1)}{2}\rho_{\mu-\nu}+(\nu+1)\varrho_{\mu-\nu}- \frac{3(\nu+1)(\nu+2)}{2}\sigma_{\mu-\nu}\right\} d^{[3] k}_{i+\mu+1}\right]. \end{array}\right. \end{equation}$

(2.9)

2.3. The convergence of ternary and its DD refinement schemes

The convergence of first, second and third order divided difference (DD) ternary refinement schemes (TRS) is presented in this section. Throughout the paper, $\pi{[0, n]}$ denotes the set of continuous functions on the closed and bounded interval $[0, n]$ .

Lemma 2.4. Let $f^{k} = \left\{\left (i/3^k, f^{k}_{i} \right)\right\}$ be a polygon of TRS at $k$ th refinement level and $d^{[1] k} = \left\{\left (i/3^k, d^{[1] k}_{i} \right) \right\}$ be a polygon of first order DD scheme. If $lim_{k\rightarrow\infty} d^{[1] k} = d^{[1] } \in \pi{[0, n]}$ , and $f$ is the limiting curve/shape produced by TRS then $d^{[1] } = f'$ .

Proof. Bernstein polynomial for $x \in [0, n]$ with data values ${f_{i}^{k}}$ is

$\begin{eqnarray*} &&B_{k}(x) = \sum\limits_{i = 0}^{s}\left(\begin{array}{c}s\\i\end{array}\right)\left(\frac{x}{n} \right)^{i}\left(1-\frac{x}{n}\right)^{s-i}f_{i}^{k}. \end{eqnarray*}$

Its first derivative is

$\begin{eqnarray} B^{'}_{k}(x)& = &\sum\limits_{i = 0}^{s}\left(\begin{array}{c}s\\i\end{array}\right)\frac{i}{n}\left(\frac{x}{n}\right)^{i-1} \left(1-\frac{x}{n}\right)^{s-i} f_{i}^{k}\\&& +\sum\limits_{i = 0}^{s}\left(\begin{array} {c}s\\i\end{array}\right)\left(\frac{x}{n}\right)^{i}(s-i)\left(\frac{-1}{n}\right)\left(1-\frac{x}{n} \right)^{s-i-1}f_{i}^{k}. \end{eqnarray}$

(2.10)

This implies

$\begin{eqnarray*} B^{'}_{k}(x) & = &\frac{s}{n}\sum\limits_{i = 0}^{s-1}\left(\begin{array}{c}{s-1}\\i\end{array}\right)\left(\frac{x}{n}\right)^i \left(1-\frac{x}{n}\right)^{s-i-1}\left(f_{i+1}^k-f_{i}^k\right). \end{eqnarray*}$

For $s = 3^kn$ , we get

$\begin{eqnarray*} B^{'}_{k}(x)& = &\sum\limits_{i = 0}^{s-1}\left(\begin{array}{c}{s-1}\\i\end{array}\right)\left(\frac{x}{n}\right)^i \left(1-\frac{x}{n}\right)^{s-i-1}3^k\left(f_{i+1}^k-f_{i}^k\right). \end{eqnarray*}$

This again implies

$\begin{eqnarray*} B^{'}_{k}(x) & = &\sum\limits_{i = 0}^{s-1}\left(\begin{array}{c}{s-1}\\i\end{array}\right)\left(\frac{x}{n}\right)^i \left(1-\frac{x}{n}\right)^{s-i-1}d^{[1]k}_i. \end{eqnarray*}$

Since the Bernstien polynomials are uniformly convergent therefore $lim_{k\rightarrow\infty}B_k = f$ and $lim_{k\rightarrow\infty}B'_k = d^{[1] }$ on $[0, n]$ . So $f' = d^{[1] } \in \pi{[0, n]}$ . Hence the proof.

Lemma 2.5. Let $f^{k} = \left\{\left (i/3^k, f^{k}_{i} \right)\right\}$ and $d^{[2] k} = \left\{\left (i/3^k, d^{[2] k}_{i} \right) \right\}$ be the polygons of TRS and its second order DD schemes respectively. If $lim_{k\rightarrow\infty} d^{[2] k} = d^{[2] } \in \pi{[0, n]}$ , and $f$ is the limiting curve produced by TRS then $d^{[2] } = f''$ .

Proof. Take the derivative of (2.10)

$\begin{eqnarray} B^{''}_{k}(x)& = &\frac{1}{n^2}\left\{s(s-1)\sum\limits_{i = 2}^{s}\frac{(s-2)!}{(i-2)!(s-i)!}\ \left(\frac{x}{n}\right)^{i-2} \left(1-\frac{x}{n}\right)^{s-i}f_{i}^{k}\right. \\&& \left. -2s(s-1)\sum\limits_{i = 1}^{s-1}\frac{(s-2)!}{(i-1)!(s-i-1)!}\ \left(\frac{x}{n}\right)^{i-1} \left(1-\frac{x}{n}\right)^{s-i-1}f_{i}^{k}\right.\\&& \ +\left.s(s-1)\sum\limits_{i = 0}^{s-2}\frac{(s-2)!}{i!(s-i-2)!}\left(\frac{x}{n}\right)^{i} \left(1-\frac{x}{n}\right)^{s-i-2}f_{i}^{k} \right\}. \end{eqnarray}$

(2.11)

This implies

$\begin{eqnarray*} B^{''}_{k}(x)& = &\frac{s(s-1)}{n^2}\left\{\sum\limits_{i = 0}^{s-2}\frac{(s-2)!}{(i)!(s-i-2)!}\ \left(\frac{x}{n}\right)^{i} \left(1-\frac{x}{n}\right)^{s-i-2}\times\right.\\&&\left.\left(f_{i+2}^{k}-2f_{i+1}+f_{i}^{k}\right) \right\}. \end{eqnarray*}$

Since $s = 3^{k}n$ therefore $\frac{s(s-1)}{n^2} = 3^{2k}2^{-1}$ , so we get

$\begin{eqnarray*} B^{''}_{k}(x)& = &3^{2k}2^{-1}\left\{\sum\limits_{i = 0}^{s-2}\frac{(s-2)!}{(i)!(s-i-2)!}\ \left(\frac{x}{n}\right)^{i} \left(1-\frac{x}{n}\right)^{s-i-2}\times\right.\\&&\left.\left(f_{i+2}^{k}-2f_{i+1}+f_{i}^{k}\right) \right\}. \end{eqnarray*}$

This implies

$\begin{eqnarray*} B^{''}_{k}(x)& = &\sum\limits_{i = 0}^{s-2}\left\{\frac{(s-2)!}{(i)!(s-i-2)!}\ \left(\frac{x}{n}\right)^{i} \left(1-\frac{x}{n}\right)^{s-i-2}\right\}d^{[2]k}_{i+1}, \end{eqnarray*}$

where $d^{[2] k}_{i+1}$ = $3^{2k}(2!)^{-1}(f_{i+2}^{k}-2f_{i+1}^{k}+f_{i}^{k}).$

Again the uniform convergence of the Bernstien polynomials implies $lim_{k\rightarrow\infty}B^{''}_k = d^{[2] }$ and $lim_{k\rightarrow\infty}B_k = f$ . Therefore when $k\rightarrow\infty$ then $d^{[2] }$ converges uniformly to $f^{''}$ on $[0, n]$ . This concludes $d^{[2] } = f^{''} \in \pi{[0, n]}$ . Hence the proof.

Lemma 2.6. Let $f^{k} = \{\left (i/3^k, f^{k}_{i} \right)\}$ and $d^{[3] k)} = \left\{\left (i/3^k, d^{[3] k}_{i} \right) \right\}$ be the polygons of TRS and its third order DD schemes respectively. If $lim_{k\rightarrow\infty} d^{[3] k} = d^{[3] } \in \pi{[0, n]}$ then $d^{[3] } = f'''$ .

Proof. Now take the derivative of (2.11), then

$\begin{eqnarray*} B^{'''}_{k}(x)& = &\frac{1}{n^3}\sum\limits_{i = 3}^{s}\frac{s(s-1)(s-2)(s-3)!}{(i-3)!(s-i)!} \left(\frac{x}{n}\right)^{i-3} \left(1-\frac{x}{n}\right)^{s-i}f_{i}^{k}\\&& -\frac{3}{n^3}\sum\limits_{i = 2}^{s-1}\frac{s(s-1)(s-2)(s-3)!}{(i-2)!(s-i-1)!} \left(\frac{x}{n}\right)^{i-2} \left(1-\frac{x}{n}\right)^{s-i-1}f_{i}^{k}\\&& +\frac{3}{n^3}\sum\limits_{i = 1}^{s-2}\frac{s(s-1)(s-2)(s-3)!}{(i-1)!(s-i-2)!} \left(\frac{x}{n}\right)^{i-1} \left(1-\frac{x}{n}\right)^{s-i-2}f_{i}^{k}\\&& -\frac{1}{n^3}\sum\limits_{i = 0}^{s-3}\frac{s(s-1)(s-2)(s-3)!}{i!(s-i-3)!} \left(\frac{x}{n}\right)^{i} \left(1-\frac{x}{n}\right)^{s-i-3}f_{i}^{k}. \end{eqnarray*}$

Since, $s = 3^{k}n$ , then

$\begin{eqnarray*} B^{'''}_{k}(x) & = & 3^{3k}(3!)^{-1}\sum\limits_{i = 0}^{s-3}\left(\begin{array}{c}{s-3}\\i\end{array}\right) \left(\frac{x}{n}\right)^{i} \left(1-\frac{x}{n}\right)^{s-i-3}\times\\&&\left(-f_{i}^{k}+3f_{i+1}^{k}-3f_{i+2}^{k}+f_{i+3}^{k}\right). \end{eqnarray*}$

This implies

$\begin{eqnarray*} B^{'''}_{k}(x) & = & \sum\limits_{i = 0}^{s-3}\left(\begin{array}{c}{s-3}\\i\end{array}\right) \left(\frac{x}{n}\right)^{i} \left(1-\frac{x}{n}\right)^{s-i-3}d^{[3]k}_{i+1}, \end{eqnarray*}$

where

$\begin{eqnarray*} d^{[3]k}_{i+1} = 3^{3k}(3!)^{-1}\left(-f_{i}^{k}+3f_{i+1}^{k}-3f_{i+2}^{k}+f_{i+3}^{k}\right). \end{eqnarray*}$

This implies $B^{'''}_k\rightarrow d^{[3] }$ and $B_k\rightarrow f.$ This implies that for $k\rightarrow\infty$ the $d^{[3] }$ converges uniformly to $f^{'''}$ on $[0, n]$ . This concludes that $d^{[3] } = f^{'''} \in \pi{[0, n]}$ . Hence the proof.

3. The deviation of ternary and its DD refinement schemes

We first introduce the inequalities to compute the deviation between two consecutive points at the same refinement level then we introduce the inequalities to compute the deviation between successive levels of polygons produced by ternary and its DD refinement schemes.

Lemma 3.1. If $f^{0} = \{\left (i/3^0, f^{0}_{i} \right)\}$ is the initial polygon and $f^{k+1} = \{\left (i/3^{k+1}, f^{k+1}_{i} \right)\}$ is the polygon obtained by TRS at $(k+1)$ th refinement level. If $\varpi < 1$ then the deviation between two consecutive points at $(k+1)$ th level is

$\begin{equation} \max\limits_{i}\left\|f^{k+1}_{i+1}-f^{k+1}_{i}\right\| \leq (\varpi)^{k+1}\max\limits_{i}\left\|f^{0}_{i+1}-f^{0}_{i}\right\|, \end{equation}$

(3.1)

where

$\begin{equation} \varpi = \max\left\{\varpi_1, \varpi_2, \varpi_3\right\} \lt 1, \\ \end{equation}$

(3.2)

$\begin{equation} \left\{\begin{array}{l} \varpi_{1} = \sum\limits_{\mu = 0}^{m-1}\left|\sum\limits_{\nu = 0}^{\mu}\left(\rho_{\mu-\nu}-\varrho_{\mu-\nu}\right)\right|, \\ \\ \varpi_{2} = \sum\limits_{\mu = 0}^{m-1}\left|\sum\limits_{\nu = 0}^{\mu}(\varrho_{\mu-\nu}-\sigma_{\mu-\nu})\right|, \\ \\ \varpi_{3} = \sum\limits_{\mu = 0}^{m}\left|\sum\limits_{\nu = 0}^{\mu}(\sigma_{\mu-\nu}-\rho_{\mu-\nu-1})\right|. \end{array}\right. \end{equation}$

(3.3)

Proof. From (2.1), we get

$\begin{eqnarray*} &&f^{k+1}_{3i+1}-f^{k+1}_{3i} = \sum\limits_{\nu = 0}^{m}\varrho_{\nu}f^{k}_{i+\nu}-\sum\limits_{\nu = 0}^{m}\rho_{\nu}f^{k}_{i+\nu} = \sum\limits_{\nu = 0}^{m}(\varrho_{\nu}-\rho_{\nu})f^{k}_{i+\nu}. \end{eqnarray*}$

This further implies

$\begin{eqnarray*} f^{k+1}_{3i+1}-f^{k+1}_{3i} & = &\left[(\rho_{0}-\varrho_{0})\left(f^{k}_{i+1}-f^{k}_{i}\right)+\left\{(\rho_{0}-\varrho_{0})+(\rho_{1}-\varrho_{1})\right\} \left(f^{k}_{i+2}-f^{k}_{i+1}\right)\right.\\ &&\left.+\left\{(\rho_{0}-\varrho_{0})+(\rho_{1}-\varrho_{1})+(\rho_{2}-\varrho_{2})\right\}\left(f^{k}_{i+3}-f^{k}_{i+2}\right)+\ldots\right.\\ &&\left.+\left\{(\rho_{0}-\varrho_{0})+(\rho_{1}-\varrho_{1})+\ldots+(\rho_{m-1}-\varrho_{m-1})\right\} \left(f^{k}_{i+m}-f^{k}_{i+m-1}\right)\right.\\ &&\left. +\left\{(\varrho_{0}-a_{0})+(\varrho_{1}-\rho_{1})+\ldots+(\varrho_{m}-\rho_{m})\right\}f^{k}_{i+m}\right]. \end{eqnarray*}$

This leads to

$\begin{eqnarray*} f^{k+1}_{3i+1}-f^{k+1}_{3i} = \sum\limits_{\mu = 0}^{m-1}\left\{\sum\limits_{\nu = 0}^{\mu}\left(\rho_{\mu-\nu}-\varrho_{\mu-\nu}\right)\right\}\left(f^{k}_{i+\mu+1}-f^{k}_{i+\mu}\right) +\sum\limits_{\nu = 0}^{m}\left(\varrho_{\nu}-\rho_{\nu}\right)f^{k}_{i+m}. \end{eqnarray*}$

Since by (2.2), $\sum\limits_{\nu = 0}^m\left(\varrho_{\nu}-\rho_{\nu}\right) = 0$ , then

$\begin{eqnarray*} f^{k+1}_{3i+1}-f^{k+1}_{3i} = \sum\limits_{\mu = 0}^{m-1}\left\{\sum\limits_{\nu = 0}^{\mu}(\rho_{\mu-\nu}-\varrho_{\mu-\nu})\left(f^{k}_{i+\mu+1}-f^{k}_{i+\mu}\right)\right\}. \end{eqnarray*}$

By taking maximum norm, we get

$\begin{eqnarray} \left\|f^{k+1}_{3i+1}-f^{k+1}_{3i}\right\|&\leq& \varpi_{1}\max\limits_{i}\left\|f^{k}_{i+1}-f^{k}_{i}\right\|, \end{eqnarray}$

(3.4)

where

$\begin{eqnarray*} \varpi_{1}& = &\sum\limits_{\mu = 0}^{m-1}\left|\sum\limits_{\nu = 0}^{\mu}\left(\rho_{\mu-\nu}-\varrho_{\mu-\nu}\right)\right|. \end{eqnarray*}$

Using (2.1) and similar procedure as above, we get

$\begin{eqnarray} \left\|f^{k+1}_{3i+2}-f^{k+1}_{3i+1}\right\|&\leq& \varpi_{2}\max\limits_{i}\left\|f^{k}_{i+1}-f^{k}_{i}\right\|, \end{eqnarray}$

(3.5)

where

$\begin{eqnarray*} \varpi_{2} = \sum\limits_{\mu = 0}^{m-1}\left|\sum\limits_{\nu = 0}^{\mu}(\varrho_{\mu-\nu}-\sigma_{\mu-\nu})\right|. \end{eqnarray*}$

and

$\begin{eqnarray} \left\|f^{k+1}_{3i+3}-f^{k+1}_{3i+2}\right\|\leq \varpi_{3}\max\limits_{i}\left\|f^{k}_{i+1}-f^{k}_{i}\right\|, \end{eqnarray}$

(3.6)

where

$\begin{eqnarray*} \varpi_{3} = \sum\limits_{\mu = 0}^{m}\left|\sum\limits_{\nu = 0}^{\mu}(\sigma_{\mu-\nu}-\rho_{\mu-\nu-1})\right|. \end{eqnarray*}$

Combining (3.4), (3.5) and (3.6), it proceeds as

$\begin{equation*} \max \limits_{i}\left\|f_{i+1}^{k+1}-f_{i}^{k+1}\right\| \leq \varpi\max \limits_{i}\left\|f_{i+1}^{k}-f_{i}^{k}\right\|, \end{equation*}$

where

$\begin{equation*} \varpi = \max\left\{\varpi_1, \varpi_2, \varpi_3\right\}. \end{equation*}$

This concludes

$\begin{equation*} \max\limits_{i}\left\|f^{k+1}_{i+1}-f^{k+1}_{i}\right\| \leq (\varpi)^{k+1}\max\limits_{i}\left\|f^{0}_{i+1}-f^{0}_{i}\right\|. \end{equation*}$

This completes the proof.

Theorem 3.2. If $f^{0} = \{\left (i/3^0, f^{0}_{i} \right)\}$ is the initial polygon and $f^{k+1} = \{\left (i/3^{k+1}, f^{k+1}_{i} \right)\}$ is the polygon obtained by TRS at $(k+1)$ th refinement level. If $\varpi^* < 1$ then the deviation between two successive polygons at $k$ th and $(k+1)$ th levels is

$\begin{equation} \left\|f^{k+1}-f^k\right\|_\infty \leq \varpi^*\max \limits_{i}\left\|f_{i+1}^{k}-f_{i}^k\right\|, \end{equation}$

(3.7)

where

$\begin{equation} \varpi^* = \max\left\{\varpi_4, \varpi_5, \varpi_6\right\}, \end{equation}$

(3.8)

$\begin{equation} \left\{\begin{array}{l} \varpi_{4} = \sum\limits_{\mu = 0}^{m-1}\left|1-\sum\limits_{\nu = 0}^{\mu}\rho_{\mu-\nu}\right|, \\ \\ \varpi_5 = \left|\frac{2}{3}-\varrho_0\right|+\sum\limits_{\mu = 1}^{m-1}\left|1-\sum\limits_{\nu = 0}^{\mu}\varrho_{\mu-\nu}\right|, \\ \\ \varpi_6 = \left|\frac{1}{3}-\sigma_0\right|+\sum\limits_{\mu = 1}^{m-1}\left|1-\sum\limits_{\nu = 0}^{\mu}\sigma_{\mu-\nu}\right|. \end{array}\right. \end{equation}$

(3.9)

Proof. Since the maximum deviation between $f^{k+1}$ and $f^{k}$ occurs at the diadic values $t^{k+1}_{3i} = t^{k}_{i}$ , $t^{k+1}_{3i+1} = \frac{1}{3}(2t^{k}_{i}+t^{k}_{i+1})$ and $t^{k+1}_{3i+2} = \frac{1}{3}(t^{k}_{i}+2t^{k}_{i+1})$ respectively, therefore

$\begin{eqnarray} \left\|f^{k+1}-f^{k} \right\|_{\infty} &\leq& \mbox{max}\{ Z^{1}_{k}, Z^{2}_{k}, Z^{3}_{k}\}, \end{eqnarray}$

(3.10)

where

$\begin{equation} \left\{\begin{array}{l} Z^{1}_{k} = \max\limits_{i}\left \|f^{k+1}_{3i}-f^{k}_{i}\right \|, \\ Z^{2}_{k} = \max\limits_{i}\left \|f^{k+1}_{3i+1}-\frac{1}{3}\left(2f^{k}_{i}+f^{k}_{i+1}\right)\right \|, \\ Z^{3}_{k} = \max\limits_{i}\left \|f^{k+1}_{3i+2}-\frac{1}{3}\left(f^{k}_{i}+2f^{k}_{i+1}\right)\right \|. \end{array}\right. \end{equation}$

(3.11)

From (2.1), we obtain

$\begin{eqnarray*} f^{k+1}_{3i}-f^{k}_{i}& = &\sum\limits_{\nu = 0}^{m}\rho_{\nu}f^{k}_{i+\nu}-f^{k}_{i}. \end{eqnarray*}$

Since by (2.1), $\sum\limits_{\nu = 0}^{m}\rho_{\nu}-1 = 0$ , then

$\begin{eqnarray*} f^{k+1}_{3i}-f^{k}_{i} = \sum\limits_{\mu = 0}^{m-1}\left(1-\sum\limits_{\nu = 0}^{\mu}\rho_{\mu-\nu}\right)\left(f^{k}_{i+\mu+1}-f^{k}_{i+\mu}\right). \end{eqnarray*}$

Taking norm, we get

$\begin{eqnarray} \left\|f^{k+1}_{3i}-f^{k}_{i}\right\|\leq \varpi_{4}\max\limits_{i}\left\|f^{k}_{i+1}-f^{k}_{i}\right\|, \end{eqnarray}$

(3.12)

where

$\begin{eqnarray*} \varpi_{4} = \sum\limits_{\mu = 0}^{m-1}\left|1-\sum\limits_{\nu = 0}^{\mu}\rho_{\mu-\nu}\right|. \end{eqnarray*}$

Using (2.1) and similar procedure as above, we get

$\begin{eqnarray} \left\|f_{3i+1}^{k+1}-\left(\frac{2}{3}f_i^k+\frac{1}{3}f_{i+1}^k\right)\right\| &\leq& \varpi_5\max \limits_{i}\left\|f_{i+1}^k-f_{i}^k\right\|, \end{eqnarray}$

(3.13)

where

$\begin{equation*} \varpi_5 = \left|\frac{2}{3}-\varrho_0\right|+\sum\limits_{\mu = 1}^{m-1}\left|1-\sum\limits_{\nu = 0}^{\mu}\varrho_{\mu-\nu}\right|. \end{equation*}$

$\begin{eqnarray} \left\|f_{3i+2}^{k+1}-\left(\frac{1}{3}f_i^k+\frac{2}{3}f_{i+1}^k\right)\right\| &\leq& \varpi_6\max \limits_{i}\left\|f_{i+1}^k-f_{i}^k\right\|, \end{eqnarray}$

(3.14)

where

$\begin{equation*} \varpi_6 = \left|\frac{1}{3}-\sigma_0\right|+\sum\limits_{\mu = 1}^{m-1}\left|1-\sum\limits_{\nu = 0}^{\mu}\sigma_{\mu-\nu}\right|. \end{equation*}$

From (3.10)-(3.14), we get

$\begin{equation*} \left\|f^{k+1}-f^k\right\|_\infty \leq \varpi^*\max \limits_{i}\left|f_{i+1}^{k}-f_{i}^k\right|, \end{equation*}$

where

$\begin{equation*} \varpi^* = \max\left\{\varpi_4, \varpi_5, \varpi_6\right\}. \end{equation*}$

Hence the proof.

Lemma 3.3. If $d^{[1] 0} = \{\left (i/3^0, d^{[1] 0}_{i} \right)\}$ is the initial polygon and $d^{[1] (k+1)} =$

$\left\{\left (i/3^{k+1}, d^{[1] (k+1)}_{i} \right) \right\}$ is the polygon obtained by first order DD TRS at $(k+1)$ th refinement level. If $\varpi^{**} < 1$ and

$\begin{equation} \left\{\begin{array}{l} c_{1} = \sum\limits_{\nu = 0}^{m-1}(m-\nu)(2\varrho_{\nu}-\sigma_{\nu}-\rho_{\nu}) = 0, \\ c_{2} = \sum\limits_{\nu = 0}^{m}\left\{(2\nu+1)\sigma_{m-\nu}-(m-\nu)(\rho_{\nu}+\varrho_{\nu})\right\} = 0, \\ c_{3} = \sum\limits_{\nu = 0}^{m}\{2\nu\rho_{m-\nu}-\nu\varrho_{m-\nu}-(\nu+1)\sigma_{m-\nu}\} = 0, \end{array}\right. \end{equation}$

(3.15)

then the deviation between two consecutive points at $(k+1)$ th level is

$\begin{equation} \max\limits_{i}\left\| d^{[1] (k+1)}_{i+1}- d^{[1] (k+1)}_{i}\right\| \leq (\varpi^{**})^{k+1}\max\limits_{i}\left\| d^{[1]0}_{i+1}- d^{[1]0}_{i}\right\|, \end{equation}$

(3.16)

where

$\begin{equation} \varpi^{**} = \max\left\{\varpi^{*}_1, \varpi^{*}_2, \varpi^{*}_3\right\}, \end{equation}$

(3.17)

$\begin{equation} \left\{\begin{array}{l} \varpi^{*}_{1} = 3\sum\limits_{\mu = 0}^{m-2}\left|\sum\limits_{\nu = 0}^{\mu}(\nu+1)(\rho_{\mu-\nu}-2\varrho_{\mu-\nu}+\sigma_{\mu-\nu}) \right|, \\ \\ \varpi^{*}_{2} = 3\sum\limits_{\mu = 0}^{m-1}\left|\sum\limits_{\nu = 0}^{\mu}\left\{(\nu+1)\varrho_{\mu-\nu}-(2\nu+2)\sigma_{\mu-\nu}+\nu\rho_{\mu-\nu}\right\}\right|, \\ \\ \varpi^{*}_{3} = 3\sum\limits_{\mu = 0}^{m-1}\left|\sum\limits_{\nu = 0}^{\mu}\{(\nu+1)\sigma_{\mu-\nu}-2\nu\rho_{\mu-\nu}+\nu\varrho_{\mu-\nu}\}\right|. \end{array}\right. \end{equation}$

(3.18)

Proof. Using (2.3) and proceeding similarly as in Lemma 3.1, we get

If, $c_{1} = \sum\limits_{\nu = 0}^{m-1}(m-\nu)(2\varrho_{\nu}-\sigma_{\nu}-\rho_{\nu}) = 0,$ then

$\begin{eqnarray} \begin{array}{l} \left\| d^{[1] (k+1)}_{3i+1}- d^{[1] (k+1)}_{3i}\right\|\leq \varpi^{*}_{1}\max\limits_{i}\left\| d^{[1] k}_{i+1}- d^{[1] k}_{i}\right\|, \end{array} \end{eqnarray}$

(3.19)

where

$\begin{eqnarray*} \varpi^{*}_{1}& = &3\sum\limits_{\mu = 0}^{m-2}\left|\sum\limits_{\nu = 0}^{\mu}(\nu+1)(\rho_{\mu-\nu}-2\varrho_{\mu-\nu}+\sigma_{\mu-\nu})\right|.\\ \end{eqnarray*}$

Using (2.3) and similar procedure as above, we get

$c_{2} = \sum\limits_{\nu = 0}^{m}\left\{(2\nu+1)\sigma_{m-\nu}-(m-\nu)(\rho_{\nu}+\varrho_{\nu})\right\} = 0,$

$\begin{eqnarray} \begin{array}{l} \left\| d^{[1] (k+1)}_{3i+2}- d^{[1] (k+1)}_{3i+1}\right\|\leq \varpi^{*}_{2}\max\limits_{i}\left\| d^{[1] k}_{i+1}- d^{[1] k}_{i}\right\|. \end{array} \end{eqnarray}$

(3.20)

where

$\begin{eqnarray*} \varpi^{*}_{2}& = &3\sum\limits_{\mu = 0}^{m-1}\left|\sum\limits_{\nu = 0}^{\mu}\left\{(\nu+1)\varrho_{\mu-\nu}-(2\nu+2)\sigma_{\mu-\nu}+\nu\rho_{\mu-\nu}\right\}\right|.\\ \end{eqnarray*}$

And

$c_{3} = \sum\limits_{\nu = 0}^{m}\left\{2\nu\rho_{m-\nu}-\nu\varrho_{m-\nu}-(\nu+1)\sigma_{m-\nu}\right\} = 0,$

$\begin{equation} \left\| d^{[1] (k+1)}_{3i+3}- d^{[1] (k+1)}_{3i+2}\right\|\leq \varpi^{*}_{3}\max\limits_{i}\left\| d^{[1] k}_{i+1}- d^{[1] k}_{i}\right\|, \end{equation}$

(3.21)

where

$\begin{equation*} \varpi^{*}_{3} = 3\sum\limits_{\mu = 0}^{m-1}\left|\sum\limits_{\nu = 0}^{\mu} \left\{(\nu+1)\sigma_{\mu-\nu}-2\nu\rho_{\mu-\nu}+\nu\varrho_{\mu-\nu}\right\}\right|.\\ \end{equation*}$

Combining Eqs (3.19), (3.20) and (3.21), we get

$\begin{equation*} \max\limits_{i}\left\| d^{[1] (k+1)}_{i+1}-d^{[1] (k+1)}_{i}\right\|\leq \varpi^{**}\max\limits_{i}\left\| d^{[1] k}_{i+1}-d^{[1] k}_{i}\right\|, \end{equation*}$

whereas

$\begin{equation*} \varpi^{**} = \max\left\{\varpi^{*}_{1}, \varpi^{*}_{2}, \varpi^{*}_{3}\right\}. \end{equation*}$

This implies

$\begin{equation*} \max\limits_{i}\left\| d^{[1] (k+1)}_{i+1}-d^{[1] (k+1)}_{i}\right\|\leq(\varpi^{**})^{k+1}\max\limits_{i}\left\| d^{[1]0}_{i+1}-d^{[1]0}_{i}\right\|. \end{equation*}$

This completes the proof.

Theorem 3.4. If $d^{[1] 0} = \left\{\left (i/3^0, d^{[1] 0}_{i} \right) \right\}$ is the initial polygon and $d^{[1] (k+1)} =$

$\left\{\left (i/3^{k+1}, d^{[1] (k+1)}_{i} \right) \right\}$ is the polygon obtained by first order DD TRS at $(k+1)$ th refinement level. If $\varpi^{***} < 1$ and

$\begin{equation} \left\{\begin{array}{l} c_{4} = -\frac{1}{3}+\sum\limits_{\nu = 1}^{m}\nu(\rho_{m-\nu}-\varrho_{m-\nu}) = 0, \\ c_{5} = -\frac{1}{3}+\sum\limits_{\nu = 1}^{m}\nu(\varrho_{m-\nu}-\sigma_{m-\nu}) = 0, \\ c_{6} = -\frac{1}{3}+\sum\limits_{\nu = 0}^{m}\{(\nu+1)\sigma_{m-\nu}-\nu\rho_{m-\nu}\} = 0, \end{array}\right. \end{equation}$

(3.22)

then the deviation between two successive polygons at $k$ th and $(k+1)$ th levels is

$\begin{equation} \left\| d^{[1] (k+1)}- d^{[1]k}\right\|_\infty \leq \varpi^{***}\max \limits_{i}\left\| d^{[1]k}_{i+1}- d^{[1]k}_{i}\right\|, \end{equation}$

(3.23)

where

$\begin{equation} \varpi^{***} = \max\left\{\varpi^{*}_4, \varpi^{*}_5, \varpi^{*}_6\right\}, \end{equation}$

(3.24)

$\begin{equation} \left\{\begin{array}{l} \varpi^{*}_{4} = 3\sum\limits_{\mu = 0}^{m-2}\left|\frac{1}{3}+\sum\limits_{\nu = 0}^{\mu}\left\{\left(\nu+1\right)\left(\varrho_{\mu-\nu} -\rho_{\mu-\nu}\right)\right\}\right|, \\ \varpi^{*}_5 = 3\left|\frac{2}{3^2}-\varrho_{0}+\sigma_{0}\right| +3\sum\limits_{\mu = 1}^{m-2}\left|\frac{1}{3} +\sum\limits_{\nu = 0}^{\mu}\{(\nu+1)(\sigma_{\mu-\nu}-\varrho_{\mu-\nu})\}\right|, \\ \varpi^{*}_6 = 3\left| \frac{1}{3^2}-\sigma_{0}\right|+3\sum\limits_{\mu = 1}^{m-1}\left|\frac{1}{3}+\sum\limits_{\nu = 0}^{\mu}(\nu\rho_{\mu-\nu}-(\nu+1)\sigma_{\mu-\nu}) \right|. \end{array}\right. \end{equation}$

(3.25)

Proof. Since the maximum deviation between $d^{[1] (k+1)}$ and $d^{[1] k}$ occurs at the diadic values $t^{k+1}_{3i} = t^{k}_{i}$ , $t^{k+1}_{3i+1} = \frac{1}{3}(2t^{k}_{i}+t^{k}_{i+1})$ and $t^{k+1}_{3i+2} = \frac{1}{3}(t^{k}_{i}+2t^{k}_{i+1})$ respectively, therefore

$\begin{eqnarray} \left\| d^{[1] (k+1)}- d^{[1] k} \right\|_{\infty} &\leq& \mbox{max}\{ Z^{4}_{k}, Z^{5}_{k}, Z^{6}_{k}\}, \end{eqnarray}$

(3.26)

where

$\begin{equation} \left\{\begin{array}{l} Z^{4}_{k} = \max\limits_{i}\left \| d^{[1] (k+1)}_{3i}- d^{[1] k}_{i}\right \|, \\ Z^{5}_{k} = \max\limits_{i}\left \| d^{[1] (k+1)}_{3i+1}-\frac{1}{3}(2 d^{[1] k}_{i}+ d^{[1] k}_{i+1})\right \|, \\ Z^{6}_{k} = \max\limits_{i}\left \| d^{[1] (k+1)}_{3i+2}-\frac{1}{3}( d^{[1] k}_{i}+2 d^{[1] k}_{i+1})\right \|. \end{array}\right. \end{equation}$

(3.27)

By (2.3), we get

$\begin{equation*} d^{[1] (k+1)}_{3i}- d^{[1] k}_{i} = 3\sum\limits_{\mu = 0}^{m-1}\left\{\sum\limits_{\nu = 0}^{\mu}(\rho_{\mu-\nu}-\varrho_{\mu-\nu}) d^{[1]k}_{i+\mu}\right\}- d^{[1] k}_{i}. \end{equation*}$

This leads to

$\begin{eqnarray*} d^{[1] (k+1)}_{3i}-d^{[1] k}_{i}& = &3\left[\sum\limits_{\mu = 0}^{m-2}\left\{\frac{1}{3} +\sum\limits_{\nu = 0}^{\mu}(\nu+1)(\varrho_{\mu-\nu}-\rho_{\mu-\nu})( d^{[1] k}_{i+\mu+1}- d^{[1] k}_{i+\mu+1})\right\}\right.\\&& \left.\left.+\left\{-\frac{1}{3}+\sum\limits_{\nu = 1}^{m}\nu(\rho_{m-\nu}-\varrho_{m-\nu})\right\} d^{[1] k}_{i+m-1}\right.\right]. \end{eqnarray*}$

If, $c_{4} = -\frac{1}{3}+\sum\limits_{\nu = 1}^{m}\nu(\rho_{m-\nu}-\varrho_{m-\nu}) = 0,$ taking norm we get

$\begin{equation} \left\| d^{[1] (k+1)}_{3i}-d^{[1] k}_{i}\right\|\leq \varpi^{*}_{4}\max\limits_{i}\left\|d^{[1] k}_{i+1}- d^{[1] k}_{i}\right\|, \end{equation}$

(3.28)

where,

$\begin{equation*} \varpi^{*}_{4} = 3\sum\limits_{\mu = 0}^{m-2}\left|\frac{1}{3}+ \sum\limits_{\nu = 0}^{\mu}\left\{(\nu+1)(\varrho_{\mu-\nu}-\rho_{\mu-\nu})\right\}\right|.\\ \end{equation*}$

Using (2.3) and similar procedure as above, we get

$c_{5} = -\frac{1}{3}+\sum\limits_{\nu = 1}^{m}\nu(\varrho_{m-\nu}-\sigma_{m-\nu}) = 0,$

$\begin{eqnarray} \left\| d^{[1](k+1)}_{3i+1}-\left(\frac{2}{3} d^{[1]k}_{i}+\frac{1}{3} d^{[1]k}_{i+1}\right)\right\|&\leq& \varpi^{*}_{5}\max\limits_{i}\left\| d^{[1]k}_{i+1}-d^{[1]k}_{i}\right\|, \end{eqnarray}$

(3.29)

where

$\begin{eqnarray*} \varpi^{*}_{5} = 3\left|\frac{2}{3^2}-\varrho_{0}+\sigma_{0}\right| +3\sum\limits_{\mu = 1}^{m-2}\left|\frac{1}{3} +\sum\limits_{\nu = 0}^{\mu}\{(\nu+1)(\sigma_{\mu-\nu}-\varrho_{\mu-\nu})\}\right|. \end{eqnarray*}$

And

$c_{6} = -\frac{1}{3}+\sum\limits_{\nu = 0}^{m}\{(\nu+1)\sigma_{m-\nu}-\nu\rho_{m-\nu}\} = 0,$

$\begin{eqnarray} \max\limits_{i}\left\|\hat{d}_{3i+2}^{k+1}-\left(\frac{1}{3}\hat{d}_{i}^{k}+\frac{2}{3}\hat{d}_{i+1}^{k}\right)\right\|&\leq& \varpi^{*}_{6} \max\limits_{i}\left\| d^{[1]k}_{i+1}-d^{[1]k}_{i}\right\|, \end{eqnarray}$

(3.30)

where

$\begin{eqnarray*} \varpi^{*}_{6} = 3\left| \frac{1}{3^2}-\sigma_{0}\right|+3\sum\limits_{\mu = 1}^{m-1}\left|\frac{1}{3}+\sum\limits_{\nu = 0}^{\mu}(\nu\rho_{\mu-\nu}-(\nu+1)\sigma_{\mu-\nu}) \right|.\\ \end{eqnarray*}$

From (3.26)-(3.30)

$\begin{eqnarray*} \left\| d^{[1] (k+1)}-d^{[1] k}\right\|_{\infty}\leq \varpi^{***}\max\limits_{i}\left\| d^{[1]k}_{i+1}- d^{[1]k}_{i}\right\|, \end{eqnarray*}$

whereas

$\begin{equation*} \varpi^{***} = \max\left\{\varpi^{*}_4, \varpi^{*}_5, \varpi^{*}_6\right\}. \end{equation*}$

Hence the proof.

Lemma 3.5. If $d^{[2] 0} = \left\{\left (i/3^0, d^{[2] 0}_{i} \right) \right\}$ is the initial polygon and $d^{[2] (k+1)} =$

$\left \{\left (i/3^{k+1}, d^{[2] (k+1)}_{i} \right)\right\}$ is the polygon obtained by second order DD TRS at $(k+1)$ th refinement level. If $\vartheta < 1$ and

$\begin{equation} \left\{\begin{array}{l} \chi_{1} = \sum\limits_{\nu = 0}^{m-1}\left\{\frac{3\nu(\nu+1)}{2}(\rho_{m-\nu-1}-\varrho_{m-\nu-1})-(\nu+1)\sigma_{m-\nu-1}\right\} = 0, \\ \chi_{2} = \sum\limits_{\nu = 0}^{m-1}\left\{\frac{(\nu+1)(3\nu+2)}{2}\varrho_{m-1-\nu}-\frac{(\nu+1)(3\nu+4)}{2}\sigma_{m-1-\nu}\right\} = 0, \\ \chi_{3} = \sum\limits_{\nu = 0}^{m-1}\left\{\frac{3(\nu+1)(\nu+2)}{2}\sigma_{m-1-\nu}-(\nu+1)\varrho_{m-1-\nu} -\frac{3\nu(\nu+1)}{2}\rho_{m-1-\nu}\right\} = 0, \end{array}\right. \end{equation}$

(3.31)

then the deviation between two consecutive points at $(k+1)$ th level is

$\begin{equation} \max\limits_{i}\left\| d^{[2] (k+1)}_{i+1}- d^{[2] (k+1)}_{i}\right\| \leq (\vartheta)^{k+1}\max\limits_{i}\left\| d^{[2]0}_{i+1}- d^{[2]0}_{i}\right\|, \end{equation}$

(3.32)

where

$\begin{equation} \vartheta = \max\{\vartheta_1, \vartheta_2, \vartheta_3\}, \end{equation}$

(3.33)

$\begin{equation} \left\{\begin{array}{l} \vartheta_{1} = 3^{2}\sum\limits_{\mu = 0}^{m-2}\left|\sum\limits_{\nu = 0}^{\mu}\left\{(\nu+1)\sigma_{\mu-\nu} +\frac{3\nu(\nu+1)}{2}(\varrho_{\mu-\nu}-\rho_{\mu-\nu})\right\}\right|, \\ \\ \vartheta_{2} = 3^{2}\sum\limits_{\mu = 0}^{m-2}\left| \sum\limits_{\nu = 0}^{\mu}\left\{(\nu+1)\rho_{\mu-\nu}+\frac{3(\nu+1)(\nu+2)}{2}(\sigma_{\mu-\nu}-\varrho_{\mu-\nu})\right\} \right|, \\ \\ \vartheta_{3} = 3^{2}\sum\limits_{\mu = 0}^{m-2}\left| \sum\limits_{\nu = 0}^{\mu}\left\{\frac{3\nu(\nu+1)}{2}\rho_{\mu-\nu}+(\nu+1)\varrho_{\mu-\nu}-\frac{3(\nu+1) (\nu+2)}{2}\sigma_{\mu-\nu}\right\}\right|. \end{array}\right. \end{equation}$

(3.34)

Theorem 3.6. If $d^{[2] 0} = \left\{\left (i/3^0, d^{[2] 0}_{i} \right)\right\}$ is the initial polygon and $d^{[2] (k+1)} =$

$\left\{\left (i/3^{k+1}, d^{[2] (k+1)}_{i} \right)\right\}$ is the polygon obtained by second order DD TRS at $(k+1)$ th refinement level. If $\vartheta^{*} < 1$ and

$\begin{equation} \left\{\begin{array}{l} \chi_{4} = -\frac{1}{3^2}+\sum\limits_{\nu = 0}^{m-1}\left[\frac{(\nu+1)}{2}\left\{\nu\varrho_{m-1-\nu}+(\nu+2)\sigma_{m-1-\nu} -2\nu\rho_{m-1-\nu}\right\}\right] = 0, \\ \chi_{5} = -\frac{1}{3^2}+\sum\limits_{\nu = 1}^{m-1}\left[\frac{\nu(\nu+1)}{2}\left\{\rho_{m-\nu-1}+\sigma_{m-\nu-1}-2\varrho_{m-\nu-1}\right\}\right] = 0, \\ \chi_{6} = -\frac{1}{3^2}+\sum\limits_{\nu = 1}^{m}\left\{\frac{\nu(\nu+1)}{2}\varrho_{m-\nu}-\nu(\nu+1)\sigma_{m-\nu}+\frac{\nu(\nu-1)}{2}\rho_{m-\nu}\right\} = 0, \end{array}\right. \end{equation}$

(3.35)

then the deviation between two successive polygons at $k$ th and $(k+1)$ th levels is

$\begin{equation} \left\| d^{[2] (k+1)}-d^{[2] k}\right\|_\infty \leq \vartheta^{*}\max \limits_{i}\left\| d^{[2]k}_{i+1}- d^{[2]k}_{i}\right\|, \end{equation}$

(3.36)

where

$\begin{equation} \vartheta^{*} = \max\left\{\vartheta_4, \vartheta_5, \vartheta_6\right\}, \end{equation}$

(3.37)

$\begin{equation} \left\{\begin{array}{l} \vartheta_{4} = 3^{2}\sum\limits_{\mu = 0}^{m-2}\left|\frac{1}{3^2}+\sum\limits_{\nu = 0}^{\mu}\left\{\nu(\nu+1)\rho_{\mu-\nu}- \frac{\nu(\nu+1)}{2}\varrho_{\mu-\nu}-\frac{(\nu+1)(\nu+2)}{2}\sigma_{\mu-\nu}\right\}\right|, \\ \vartheta_5 = 3^{2}\left|\frac{2}{3^{3}}\right|+3^{2}\sum\limits_{\mu = 1}^{m-2}\left|\frac{1}{9}+\sum\limits_{\nu = 1}^{\mu}\left\{ \frac{\nu(\nu+1)}{2}(2\varrho_{\mu-\nu}-\rho_{\mu-\nu}-\sigma_{\mu-\nu})\right\}\right|, \\ \vartheta_6 = 3^{2}\left|\frac{1}{3^3}\right| +3^{2}\sum\limits_{\mu = 1}^{m-1}\left|\frac{1}{3^2}+\sum\limits_{\nu = 1}^{\mu}\left\{\nu(\nu+1)\sigma_{\mu-\nu} -\frac{\nu(\nu+1)}{2}\varrho_{\mu-\nu}-\frac{\nu(\nu-1)}{2}\rho_{\mu-\nu}\right\}\right|. \end{array}\right. \end{equation}$

(3.38)

Lemma 3.7. If $d^{[3] 0} = \left\{\left (i/3^0, d^{[3] 0}_{i} \right)\right\}$ is the initial polygon and $d^{[3] (k+1)} =$

$\left\{\left (i/3^{k+1}, d^{[3] (k+1)}_{i} \right)\right\}$ is the polygon obtained by third order DD TRS at $(k+1)$ th refinement level. If $\vartheta^{**} < 1$ and

$\begin{equation} \left\{\begin{array}{l} \chi_{7} = \sum\limits_{\nu = 0}^{m-2}\left[\frac{(\nu+1)(\nu+2)}{2}\left\{(\nu+1)\rho_{m-\nu-2}- (2\nu+3)\varrho_{m-\nu-2}+(\nu+2)\sigma_{m-\nu-2}\right\}\right] = 0, \\ \chi_{8} = \sum\limits_{\nu = 0}^{m-2}\left[(\nu+1)(\nu+2)\left\{\frac{(\nu-1)}{2}\rho_{m-\nu-2}+ \frac{(\nu+4)}{2}\varrho_{m-\nu-2}-(\nu+3)\sigma_{m-\nu-2}\right\}\right] = 0, \\ \chi_{9} = \sum\limits_{\nu = 0}^{m-2}\left[(\nu+1)(\nu+2)\left\{-\nu\rho_{m-\nu-2}+ \frac{(\nu-1)}{2}\varrho_{m-\nu-2}+\frac{(\nu+4)}{2}\sigma_{m-\nu-2}\right\}\right] = 0, \end{array}\right. \end{equation}$

(3.39)

then the deviation between two consecutive points at $(k+1)$ th level is

$\begin{equation} \max\limits_{i}\left\| d^{[3] (k+1)}_{i+1}- d^{[3] (k+1)}_{i}\right\| \leq (\vartheta^{**})^{k+1}\max\limits_{i}\left\| d^{[3]0}_{i+1}- d^{[3]0}_{i}\right\|, \end{equation}$

(3.40)

where

$\begin{equation} \vartheta^{**} = \max\{\vartheta_7, \vartheta_8, \vartheta_9\}, \end{equation}$

(3.41)

$\begin{equation} \left\{\begin{array}{l} \vartheta_{7} = 3^{3}\sum\limits_{\mu = 0}^{m-2}\left|\sum\limits_{\nu = 0}^{\mu}\left\{\nu(\nu+1)(\nu+2)\varrho_{\mu-\nu} -\frac{\nu(\nu+1)(\nu+3)}{2}\rho_{\mu-\nu} -\frac{(\nu-1)(\nu+1)(\nu+2)}{2}\sigma_{\mu-\nu}\right\}\right|, \\ \\ \vartheta_{8} = 3^{3}\sum\limits_{\mu = 0}^{m-3}\left|\sum\limits_{\nu = 0}^{\mu}\left\{\frac{-(\nu-1)(\nu+1)(\nu+2)}{2}\rho_{\mu-\nu}- \frac{(\nu+1)(\nu+2)(\nu+4)}{2}\varrho_{\mu-\nu} +(\nu+1)(\nu+2)(\nu+3)\sigma_{\mu-\nu}\right\}\right|, \\ \\ \vartheta_{9} = 3^{3}\sum\limits_{\mu = 0}^{m-3}\left|\sum\limits_{\nu = 0}^{\mu}\left\{\nu(\nu+1)(\nu+2)\rho_{\mu-\nu}- \frac{(\nu-1)(\nu+1)(\nu+2)}{2}\varrho_{\mu-\nu} -\frac{(\nu+1)(\nu+2)(\nu+4)}{2}\sigma_{\mu-\nu}\right\}\right|. \end{array}\right. \end{equation}$

(3.42)

Theorem 3.8. If $d^{[3] 0} = \left\{\left (i/3^0, d^{[3] 0}_{i} \right)\right\}$ is the initial polygon and $d^{[3] (k+1)} = \left\{\left (i/3^{k+1}, d^{[3] (k+1)}_{i} \right)\right\}$ is the polygon obtained by third order DD TRS at $(k+1)$ th refinement level. If $\vartheta^{***} < 1$ and

$\begin{equation} \left\{\begin{array}{l} \chi_{10} = -\frac{1}{3^3}+\sum\limits_{\nu = 0}^{m-2}\left[\frac{(\nu+1)(\nu+2)}{2}\left\{\sigma_{m-2-\nu} +\nu\varrho_{m-2-\nu}-\nu\rho_{m-2-\nu}\right\}\right] = 0, \\ \chi_{11} = -\frac{1}{3^3}+ \sum\limits_{\nu = 0}^{m-2}\left[\frac{(\nu+1)(\nu+2)}{2}\left\{\rho_{m-\nu-2}-(\nu+3)\varrho_{m-\nu-2}+ (\nu+3)\sigma_{m-\nu-2}\right\}\right] = 0, \\ \chi_{12} = -\frac{1}{3^3}+\sum\limits_{\nu = 0}^{m-2} \left[\frac{(\nu+1)(\nu+2)}{2}\left\{\varrho_{m-\nu-2}-(\nu+3)\sigma_{m-\nu-2}+\nu\rho_{m-\nu-2}\right\}\right] = 0, \end{array}\right. \end{equation}$

(3.43)

then the deviation between two successive polygons at $k$ th and $(k+1)$ th levels is

$\begin{equation} \left\| d^{[3] (k+1)}- d^{[3]k}\right\|_\infty \leq \vartheta^{***}\max \limits_{i}\left\| d^{[3]k}_{i+1}- d^{[3]k}_{i}\right\|, \end{equation}$

(3.44)

where

$\begin{equation} \vartheta^{***} = \max\left\{\vartheta_{10}, \vartheta_{11}, \vartheta_{12}\right\}, \end{equation}$

(3.45)

$\begin{equation} \left\{\begin{array}{l} \vartheta_{10} = 3^{3}\sum\limits_{\mu = 0}^{m-3} \left|\frac{1}{3^3}+\sum\limits_{\nu = 0}^{\mu}\left\{\frac{(\nu+1)(\nu+2)}{2}(\nu\rho_{\mu-\nu}- \nu\varrho_{\mu-\nu}-\sigma_{\mu-\nu})\right\}\right|, \\ \vartheta_{11} = 3^{3}\left|\frac{2}{3^4}\right|+3^{3}\sum\limits_{\mu = 1}^{m-2}\left|\frac{1}{3^3} +\sum\limits_{\nu = 1}^{\mu}\left\{\frac{(\nu+1)}{2}\{-\nu\rho_{\mu-\nu}+(\nu+2)\varrho_{\mu-\nu}-(\nu+2)\sigma_{\mu-\nu}\}\right\}\right|, \\ \vartheta_{12} = 3^{3}\left|\frac{1}{3^4}\right| +3^{3}\sum\limits_{\mu = 1}^{m-2}\left|\left\{\frac{1}{3^3}+\sum\limits_{\nu = 1}^{\mu}\frac{\nu(\nu+1)}{2}\left\{(\nu+2)\sigma_{\mu-\nu} -\varrho_{\mu-\nu}-(\nu-1)\rho_{\mu-\nu}\right\}\right\}\right|.\\ \end{array}\right. \end{equation}$

(3.46)

4. The analysis of the ternary refinement schemes

Now we present the analysis of the ternary refinement schemes. A function is called $C^m$ -continuous if its $m$ th order derivative is continuous. The common class of continuous function is $C = C^0$ . It is natural to think of a $C^m$ function as being a little bit rough, but the graph of a $C^3$ function looks smooth. So we discuss the analysis of the schemes up to $C^3$ -continuity. The results can be extended similarly. Let { $\pi^{m}{[0, n]}, \ m = 0, 1, 2, 3\}$ denotes the set of $C^m$ -continuous functions on the closed and bounded interval $[0, n]$ .

Theorem 4.1. If $f^{0} = \{\left (i/3^0, f^{0}_{i} \right)\}$ is the initial polygon and $f^{k+1} = \{\left (i/3^{k+1}, f^{k+1}_{i} \right)\}$ is the polygon obtained by TRS at $(k+1)$ th refinement level. If $\varpi, \ \varpi^* < 1$ then $\lim_{k\rightarrow\infty}f^{k} = f \in \pi{[0, n]} = \pi^{0}{[0, n]}$ .

Proof. The (3.7), gives

$\begin{equation*} \left\|f^{k+1}-f^{k}\right\|_\infty \leq \varpi^*\max \limits_{i}\left\|f_{i+1}^{k}-f_{i}^k\right\|, \end{equation*}$

and (3.1), gives

$\begin{equation*} \left\|f^{k+1}-f^k\right\|_\infty \leq \varpi^*(\varpi)^{k}\max\limits_{i}\left\|f^{0}_{i+1}-f^{0}_{i}\right\|, \end{equation*}$

Since $\varpi, \ \varpi^* < 1$ therefore $\{f^{k}\}_{k = 0}^{\infty}$ is a Cauchy sequence on closed and bounded interval $[0, n]$ . Hence it is convergent. That is

$\lim\limits_{k\rightarrow\infty}f^{k} = f \in \pi{[0, n]} = \pi^{0}{[0, n]}.$

Hence the proof.

Lemma 4.2. If $d^{[1] 0} = \left\{\left (i/3^0, d^{[1] 0}_{i} \right)\right\}$ is the initial polygon and $d^{[1] (k+1)} =$

$\left\{\left (i/3^{k+1}, d^{[1] (k+1)}_{i} \right)\right\}$ is the polygon obtained by first order DD TRS at $(k+1)$ th refinement level. If $\varpi^{**}, \ \varpi^{***} < 1$ and $c_{1}, c_{2}, c_{3}, c_{4}, c_{5}, c_{6} = 0$ , then $\lim_{k\rightarrow\infty} d^{[1] k} = d^{[1] } \in \pi{[0, n]} = \pi^{0}{[0, n]}$ .

Proof. By (3.23), we have

$\begin{equation*} \left\| d^{[1] (k+1)}-d^{[1] k}\right\|_\infty \leq \varpi^{***}\max \limits_{i}\left\| d^{[1] k}_{i+1}- d^{[1] k}_{i}\right\|, \end{equation*}$

Using (3.16), we have

$\begin{equation*} \left\| d^{[1] (k+1)}- d^{[1] k}\right\|_\infty \leq \varpi^{***}(\varpi^{**})^{k}\max\limits_{i}\left\|{d^{[1] 0}}_{i+1}-d^{[1] 0}_{i}\right\|, \end{equation*}$

Since $\varpi^{**}, \ \varpi^{**} < 1$ , therefore $\{ d^{[1] k}\}_{k = 0}^{\infty}$ defines a Cauchy sequence on $[0, n]$ and

$\lim\limits_{k\rightarrow\infty} d^{[1] k} = d^{[1]}\in \pi{[0, n]} = \pi^{0}{[0, n]}.$

This completes the proof.

Theorem 4.3. Let $f \in \pi^{0}{[0, n]}$ be the limiting curve produced by the ternary refinement scheme. If $\varpi^{**}, \ \varpi^{***} < 1$ and $c_{1}, c_{2}, c_{3}, c_{4}, c_{5}, c_{6} = 0$ then $f\in \pi^{1}{[0, n]}$ .

Proof. Lemma 4.2 leads to $\lim_{k\rightarrow\infty} d^{[1] k} = d^{[1] }\in \pi^{0}{[0, n]}$ . Lemma 2.4 gives $d^{[1] } = f'$ . Therefore, we have the result, $f\in \pi^{1}{[0, n]}$ .

Lemma 4.4. If $d^{[2] 0} = \left\{\left (i/3^0, d^{[2] 0}_{i} \right)\right\}$ is the initial polygon and $d^{[2] (k+1)} =$

$\left\{\left (i/3^{k+1}, d^{[2] (k+1)}_{i} \right)\right\}$ is the polygon obtained by second order DD TRS at $(k+1)$ th refinement level. If $\vartheta, \ \vartheta^{*} < 1$ and $\chi_{1}, \chi_{2}, \chi_{3}, \chi_{4}, \chi_{5}, \chi_{6} = 0$ then $\lim_{k\rightarrow\infty} d^{[2] k} = d^{[2] } \in \pi^{0}{[0, n]}$ .

Proof. By (3.36), we have

$\begin{equation*} \left\| d^{[2] (k+1)}- d^{[2] k}\right\|_\infty \leq \vartheta^{*}\max \limits_{i}\left\| d^{[2] k}_{i+1}- d^{[2] k}_{i}\right\|, \end{equation*}$

Using (3.32), we have

$\begin{equation*} \left\| d^{[2] (k+1)}- d^{[2] k}\right\|_\infty \leq \vartheta^{*}(\vartheta)^{k}\max\limits_{i}\left\| d^{[2] 0}_{i+1}-d^{[2] 0}_{i}\right\|, \end{equation*}$

Since $\vartheta, \ \vartheta^{*} < 1$ therefore $\{d^{[2] k}\}_{k = 0}^{\infty}$ is a Cauchy convergent sequence. So

$\lim\limits_{k\rightarrow\infty} d^{[2] k} = d^{[2]} \in \pi^{0}{[0, n]}.$

Hence the proof.

Theorem 4.5. Let $f \in \pi^{0}{[0, n]}$ be the limiting curve produced by the ternary refinement scheme. If $\vartheta, \ \vartheta^{*} < 1$ and $\varphi_{1}, \varphi_{2}, \varphi_{3}, \varphi_{4}, \varphi_{5}, \varphi_{6} = 0$ then $f\in \pi^{2}{[0, n]}$ .

Proof. Lemma 4.4 gives $\lim_{k\rightarrow\infty} d^{[2] k} = d^{[2] }\in \pi^{0}{[0, n]}$ . Lemma 2.5 leads to $d^{[2] } = f''$ . This implies $f\in \pi^{2}{[0, n]}$ .

Lemma 4.6. If $d^{[3] 0} = \left\{\left (i/3^0, d^{[3] 0}_{i} \right)\right\}$ is the initial polygon and $d^{[3] (k+1)} =$

$\left\{\left (i/3^{k+1}, d^{[3] (k+1)}_{i} \right)\right\}$ is the polygon obtained by third order DD TRS at $(k+1)$ th refinement level. If $\vartheta^{**}, \ \vartheta^{***} < 1$ and $\varphi_{7}, \varphi_{8}, \varphi_{9}, \varphi_{10}, \varphi_{11}, \varphi_{12} = 0$ then $\lim_{k\rightarrow\infty} d^{[3] k)} = d^{[3] } \in \pi^{0}{[0, n]}$ .

Proof. By (3.44), we have

$\begin{equation*} \left\| d^{[3] (k+1)}- d^{[3] k}\right\|_\infty \leq \vartheta^{***}\max \limits_{i}\left\| d^{[3] k}_{i+1}-d^{[3] k}_{i}\right\|, \end{equation*}$

Using (3.40), we have

$\begin{equation*} \left\| d^{[3] (k+1)}- d^{[3] k}\right\|_\infty \leq \vartheta^{***}(\vartheta^{**})^{k}\max\limits_{i}\left\| d^{[3] 0}_{i+1}- d^{[3] 0}_{i}\right\|, \end{equation*}$

Since $\vartheta^{**}, \ \vartheta^{***} < 1$ therefore $\{ d^{[3] k}\}_{k = 0}^{\infty}$ is a Cauchy convergent sequence. Thus

$\lim\limits_{k\rightarrow\infty} d^{[3] k} = d^{[3] }\in \pi^{0}{[0, n]}.$

Hence the proof.

Theorem 4.7. Let $f \in \pi^{0}{[0, n]}$ be the limiting curve produced by the ternary refinement scheme. If $\vartheta, \ \vartheta^{*} < 1$ and $\varphi_{7}, \varphi_{8}, \varphi_{9}, \varphi_{10}, \varphi_{11}, \varphi_{12} = 0$ then $f\in \pi^{3}{[0, n]}$ .

Proof. Lemma 4.6, implies that $\lim_{k\rightarrow\infty} d^{[3] k)} = d^{[3] } \in \pi^{0}{[0, n]}$ while Lemma 2.6 leads to $d^{[3] } = f'''$ . This implies $f\in \pi^{3}{[0, n]}$ .

Application and authenticity of the results

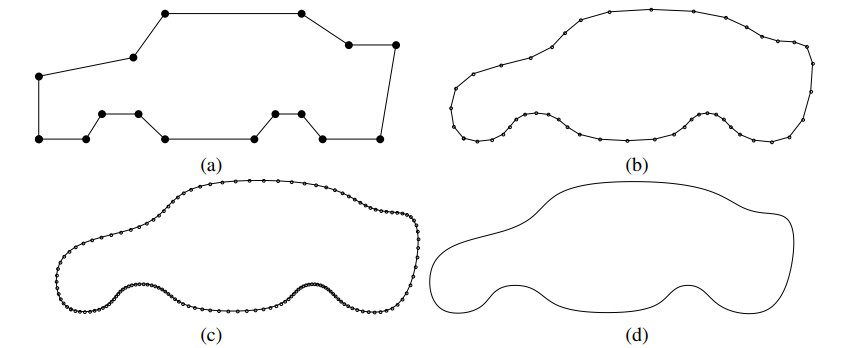
We discuss the analysis of the ternary refinement schemes introduced by ^{[8,9,10,16,17]}. We conclude that the results obtained by our methods are always equivalent to the one returned by the Laurent polynomial method. We add the Figure 2 for the interest of general readers by the direction of anonymous reviewer of this paper. The Figure 2(d) depicts the smoothness of the limiting curve produced by the ternary scheme. The Figure 2(a), (b) and (c) represent the initial, first and second refinement level of the ternary scheme.

Figure 2. Applications of ternary refinement scheme ^[17]. Here, the value of

$\mu$ is -

$\frac{1}{54}$ .

DownLoad: Full-Size Img PowerPoint

Example 4.1. If the curve is produced by the following 4-point ternary refinement scheme ^[17]

$\begin{equation*} \left\{\begin{array}{l} \label{4.6} f^{k+1}_{3i} = \rho_{0}f^{k}_{i-1}+\rho_{1}f^{k}_{i}+\rho_{2}f^{k}_{i+1}+\rho_{3}f^{k}_{i+2}, \\ f^{k+1}_{3i+1} = \varrho_{0}f^{k}_{i-1}+\varrho_{1}f^{k}_{i}+\varrho_{2}f^{k}_{i+2}+\varrho_{3}f^{k}_{i+3}, \\ f^{k+1}_{3i+2} = \sigma_{0}f^{k}_{i-1}+\sigma_{1}f^{k}_{i}+\sigma_{2}f^{k}_{i+2}+\sigma_{3}f^{k}_{i+3}, \end{array}\right. \end{equation*}$

where

$\begin{equation*} \left\{\begin{array}{l} \rho_{0} = \left( \frac{5}{81}+\frac{5}{3}\mu \right), \qquad \ \rho_{1} = \left( \frac{19}{27}-3\mu \right), \qquad \ \rho_{2} = \left( \frac{13}{54}+\mu \right), \qquad \ \rho_{3} = \left( \frac{-1}{162}+\frac{1}{3}\mu\right), \\ \varrho_{0} = \mu, \qquad \qquad \quad \ \ \varrho_{1} = (\frac{1}{2}- \mu), \qquad \quad \ \varrho_{2} = (\frac{1}{2}-\mu), \qquad \quad \ \varrho_{3} = \mu, \\ \sigma_{0} = (\frac{-1}{162}+\frac{1}{3}\mu), \quad \ \ \ \sigma_{1} = (\frac{13}{54}+\mu), \quad \quad \ \ \ \sigma_{2} = (\frac{19}{27}-3\mu), \quad \quad \ \sigma_3 = (\frac{5}{81}+\frac{5}{3}\mu), \end{array}\right. \end{equation*}$

then by Laurent polynomial method the scheme produces $C^{3}$ -continuous curve for $\mu\in(-\frac{1}{27}, \frac{2}{27})$ . By Theorems 4.1, 4.3, 4.5 and 4.7, we also get the same results.

Example 4.2. If the curve is produced by the following 4-point ternary refinement scheme ^[9]

$\begin{equation*} \left\{\begin{array}{l} \label{4.1} f^{k+1}_{3i} = \rho_{1}f^{k}_{i}, \\ f^{k+1}_{3i+1} = \varrho_{0}f^{k}_{i-1}+\varrho_{1}f^{k}_{i}+\varrho_{2}f^{k}_{i+2}+\varrho_{3}f^{k}_{i+3}, \\ f^{k+1}_{3i+2} = \sigma_{0}f^{k}_{i-1}+\sigma_{1}f^{k}_{i}+\sigma_{2}f^{k}_{i+2}+\sigma_{3}f^{k}_{i+3}, \end{array}\right. \end{equation*}$

where

$\begin{eqnarray*} \left\{\begin{array}{l} \rho_{0} = 0, \quad \qquad\qquad \rho_{1} = 1, \quad \qquad \qquad\rho_{2} = 0, \quad \qquad \qquad \rho_{3} = 0, \\ \varrho_{0} = \frac{-1}{18}-\frac{1}{6}\mu, \qquad \ \varrho_{1} = \frac{13}{18}+\frac{1}{2} \mu, \qquad \ \ \varrho_{2} = \frac{7}{18}-\frac{1}{2}\mu, \qquad \ \ \varrho_{3} = \frac{-1}{18}+\frac{1}{6} \mu , \\ \sigma_{0} = \frac{-1}{18}+\frac{1}{6} \mu, \qquad \sigma_{1} = \frac{7}{18}-\frac{1}{2}\mu, \qquad \ \sigma_{2} = \frac{13}{18}+\frac{1}{2}\mu, \qquad \ \sigma_3 = \frac{-1}{18}-\frac{1}{6} \mu, \end{array}\right. \end{eqnarray*}$

then by Laurent polynomial method the scheme produces $C^{2}$ -continuous curve over the interval $\mu\in(\frac{1}{15}, \frac{1}{9})$ . By Theorems 4.1, 4.3, and 4.5, we also get the same results.

Example 4.3. If the curve is produced by the following 3-point ternary refinement scheme ^[8]

$\begin{eqnarray*} \left\{\begin{array}{l} f^{k+1}_{3i} = \rho_{0}f^{k}_{i-1}+\rho_{1}f^{k}_{i}+\rho_{2}f^{k}_{i+1}, \\ f^{k+1}_{3i+1} = \varrho_{1}f^{k}_{i}, \\ f^{k+1}_{3i+2} = \sigma_{0}f^{k}_{i-1}+\sigma_{1}f^{k}_{i}+\sigma_{2}f^{k}_{i+1}, \end{array}\right. \end{eqnarray*}$

where

$\begin{eqnarray*} \left\{\begin{array}{l} \rho_{0} = \left(\rho+\frac{1}{3}\right), \qquad \ \ \rho_{1} = \left(\frac{2}{3}-2\rho\right), \qquad \rho_{2} = \rho, \\ \varrho_{0} = 0, \qquad \qquad \quad \varrho_{1} = 1, \qquad \qquad \quad \ \varrho_{2} = 0, \\ \sigma_{0} = \rho, \qquad \qquad \ \ \ \sigma_{1} = \left(\frac{2}{3}-2\rho\right), \qquad \sigma_{2} = \left(\rho+\frac{1}{3}\right), \end{array}\right. \end{eqnarray*}$

then by both methods the scheme produces $C^{1}$ -continuous curve for $w\in\left(-\frac{1}{9}, 0\right)$ .

Example 4.4. If the curve is produced by the following 6-point ternary refinement scheme ^[10]

$\begin{eqnarray} \left\{\begin{array}{l} f^{k+1}_{3i} = \rho_{2}f^{k}_{i}, \\ f^{k+1}_{3i+1} = \varrho_{0}f^{k}_{i-2} +\varrho_{1}f^{k}_{i-1}+\varrho_{2}f^{k}_{i} +\varrho_{3}f^{k}_{i+1}+\varrho_{4}f^{k}_{i+2}+\varrho_{5} f^{k}_{i+3}, \\ f^{k+1}_{3i+2} = \sigma_{0} f^{k}_{i-2}+\sigma_{1}f^{k}_{i-1}+\sigma_{2}f^{k}_{i} +\sigma_{3}f^{k}_{i+1} +\sigma_{4}f^{k}_{i+2} +\sigma_{5}f^{k}_{i+3}, \end{array}\right. \end{eqnarray}$

(4.1)

where

$\begin{equation*} \left\{\begin{array}{l} \rho_{0} = 0, \qquad\qquad\qquad\qquad \rho_{1} = 0, \qquad\qquad\qquad\qquad \rho_{2} = 1, \\ \rho_{3} = 0, \qquad\qquad\qquad\qquad \rho_{4} = 0, \qquad\qquad\qquad\qquad \rho_{5} = 0, \\ \varrho_{0} = \left(-\frac{11}{81}+13\omega\right), \quad\quad\quad \ \varrho_{1} = \left(\frac{13}{27}-51\omega\right), \quad\quad\qquad \varrho_{2} = \left(-\frac{2}{27}+74\omega\right), \quad\quad\quad\\ \varrho_{3} = \left(\frac{74}{81}-46\omega\right), \quad\quad\qquad \varrho_{4} = \left(-\frac{5}{27}+9\omega\right), \quad\quad\quad\ \ \ \varrho_{5} = \omega, \quad\quad\quad\\ \sigma_{0} = \omega, \quad\quad\quad\quad\quad\qquad\ \ \sigma_{1} = \left(-\frac{5}{27}+9\omega\right), \quad\quad\quad\ \ \ \sigma_{2} = \left(\frac{74}{81}-46\omega\right), \quad\quad\quad\\ \sigma_{3} = \left(-\frac{2}{27}+74\omega\right), \quad\quad\quad \sigma_{4} = \left(\frac{13}{27}-51\omega\right), \quad\quad\quad\ \ \ \sigma_{5} = \left(-\frac{11}{81}+13\omega\right), \quad\quad\quad \end{array}\right. \end{equation*}$

then by both methods the scheme produces $C^{2}$ -continuous curve over the interval $w\in\left(\frac{14}{1215}, \frac{23}{1944}\right)$ .

Example 4.5. If the curve is produced by the following 3-point ternary refinement scheme ^[16]

$\begin{equation} \left\{\begin{array}{l} f^{k+1}_{3i} = \rho_{0}f^{k}_{i-1}+\rho_{1}f^{k}_{i}+\rho_{2}f^{k}_{i+1}, \\ f^{k+1}_{3i+1} = \varrho_{0}f^{k}_{i-1}+\varrho_{1}f^{k}_{i}+\varrho_{2}f^{k}_{i+2}, \\ f^{k+1}_{3i+2} = \sigma_{0}f^{k}_{i-1}+\sigma_{1}f^{k}_{i}+\sigma_{2}f^{k}_{i+2}, \end{array}\right. \end{equation}$

(4.2)

where

$\begin{equation*} \left\{\begin{array}{l} \rho_{0} = \left( \frac{25}{72}+\mu \right), \rho_{1} = \left( \frac{23}{36}-2\mu \right), \rho_{2} = \left( \frac{1}{72}+\mu \right), \\ \varrho_{0} = \left(\frac{1}{8}+\mu\right), \ \varrho_{1} = \left(\frac{3}{4}- 2\mu\right), \ \varrho_{2} = \left(\frac{1}{8}+\mu\right), \\ \sigma_{0} = \left(\frac{1}{72}+\mu \right), \sigma_{1} = \left( \frac{23}{36}-2\mu \right), \ \sigma_{2} = \left( \frac{25}{72}+\mu \right), \end{array}\right. \end{equation*}$

then by both methods the scheme produces $C^{2}$ -continuous curve for $\mu\in\left(-\frac{1}{72}, \frac{7}{72}\right)$ .

5. Summary of the work

Here we present the brief summary of the work done so for in this paper.

● If $\max\left\{\varpi_1, \varpi_2, \varpi_3\right\} < 1$ where $\varpi_1, \varpi_2, \varpi_3$ are defined in (3.3) then TRS will generate $C^0$ continuous curve.

● If $c_{1}$ , $c_{2}$ , $c_{3}$ , $c_{4}$ , $c_{5}$ , $c_{6}$ defined in (3.15) and (3.22) are equal to zero and $\max\left\{\varpi^{*}_1, \varpi^{*}_2, \varpi^{*}_3\right\} < 1$ , where $\varpi^{*}_1, \varpi^{*}_2, \varpi^{*}_3$ are defined in (3.18) then TRS will generate $C^1$ continuous curve.

● If $\chi_{1}$ , $\chi_{2}$ , $\chi_{3}$ , $\chi_{4}$ , $\chi_{5}$ , $\chi_{6}$ defined in (3.31) and (3.35) are equal to zero and $\max\{\vartheta_1, \vartheta_2, \vartheta_3\} < 1$ , where $\vartheta_1, \vartheta_2, \vartheta_3$ are defined in (3.34) then TRS will generate $C^2$ continuous curve.

● If $\chi_{7}$ , $\chi_{8}$ , $\chi_{9}$ $\chi_{10}$ , $\chi_{11}$ , $\chi_{12}$ defined in (3.39) and (3.43) are equal to zero and $\max\{\vartheta_7, \vartheta_8, \vartheta_9\} < 1$ , where $\vartheta_7, \vartheta_8, \vartheta_9$ are defined in (3.42) then TRS will generate $C^3$ continuous curve.

6. Comparison and advantages

There are two major techniques to analyze the refinement schemes. These are called Laurent polynomial and divided difference (DD) techniques.

● Our technique is the generalization of the DD technique for ternary refinement schemes.

● We have presented the explicit form of the general inequalities for the analysis of the ternary refinement schemes. These inequalities contain simple algebraic expressions. In these inequalities the polynomial factorization, division and summation are not involved. Simple arithmetic operations such as subtraction and multiplication are involved to evaluate the inequalities.

● While in Laurent polynomial technique, the polynomial factorization, division and summation are involved to evaluate the inequalities. In this technique, the explicit form of the general inequalities are also not available.

● So it is obvious that the computational complexity of our techniques is less than the complexity of Laurent polynomial technique.

7. Conclusions

In this paper, we have introduced an alternative technique to analyze a class of ternary refinement schemes. A comparative study of the proposed technique with other existing technique has been presented to prove the effectiveness of the proposed technique. It has been observed that the alternative technique has less computational cost comparative to the existing Laurent polynomial technique.

Acknowledgment

The second author is grate full to HEC Pakistan for awarding the fellowship under the Indigenous Ph.D. Scholarship Scheme. The author Yu-Ming Chu is supported by National Natural Science Foundation of China (Grant Nos. 61673169, 11971142).

Contributions of the authors

Conceptualization, Ghulam Mustafa and Dumitru Baleanu; Formal analysis, Dumitru Baleanu and Yu-Ming Chu; Methodology, Ghulam Mustafa and Syeda Tehmina Ejaz; Supervision, Ghulam Mustafa; Writing original draft, Syeda Tehmina Ejaz and Ghulam Mustafa; Writing, review and editing, Syeda Tehmina Ejaz and Yu-Ming Chu.

Conflicts of interest

The authors declare no conflict of interest.

References

[1]	Pennes HH (1948) Analysis of tissue and arterial blood temperature in the resting human forearm. J Appl Phisiol 1: 93-122.
[2]	Dillenseger JL, Esneault S (2010) Fast FFT-based bioheat transfer equation computation. Comput Biol Med 40: 119-123.
[3]	Zhao JJ, Zhang J, Kang N, et al. (2005) A two level finite difference scheme for one dimensional Pennes' bioheat equation. Appl Math Comput 171: 320-331.
[4]	Huang HW, Horng TL (2015) Bioheat transfer and thermal heating for tumor treatment. Heat Transfer and Fluid Flow in Biological Processes Amsterdam: Elsevier, 1-42.
[5]	Bedin L, Bazán FSV (2014) On the 2D bioheat equation with convective boundary conditions and its numerical realization via a highly accurate approach. Appl Math Comput 236: 422-436.
[6]	Karaa S, Zhang J, Yang F (2005) A numerical study of a 3D bioheat transfer problem with different spatial heating. Math Comput Simulat 68: 375-388.
[7]	Askarizadeh H, Ahmadikia H (2015) Analytical study on the transient heating of a two-dimensional skin tissue using parabolic and hyperbolic bioheat transfer equations. Appl Math Model 39: 3704-3720.
[8]	Deng ZS, Liu J (2002) Analytical study on bioheat transfer problems with spatial or transient heating on skin surface or inside biological bodies. J Biomech Eng 124: 638-649.
[9]	Dutta J, Kundu B (2017) A revised approach for an exact analytical solution for thermal response in biological tissues significant in therapeutic treatments. J Therm Biol 66: 33-48.
[10]	Bojdi ZK, Hemmat AA (2017) Wavelet collocation methods for solving the Pennes bioheat transfer equation. Optik 130: 345-355.
[11]	Kansa EJ (1990) Multiquadrics—A scattered data approximation scheme with applications to computational fluid-dynamics—II solutions to parabolic, hyperbolic and elliptic partial differential equations. Comput Math Appl 19: 147-161.
[12]	Beatson RK, Levesley J, Mouat CT (2011) Better bases for radial basis function interpolation problems. J Comput Appl Math 236: 434-446.
[13]	Zerroukat M, Power H, Chen CS (1998) A numerical method for heat transfer problems using collocation and radial basis functions. Int J Numer Meth Eng 42: 1263-1278.
[14]	Cao L, Qin QH, Zhao N (2010) An RBF-MFS model for analysing thermal behaviour of skin tissues. Int J Heat Mass Tran 53: 1298-1307.
[15]	Jamil M, Ng EYK (2013) Evaluation of meshless radial basis collocation method (RBCM) for heterogeneous conduction and simulation of temperature inside the biological tissues. Int J Therm Sci 68: 42-52.
[16]	Hon YC, Mao XZ (1998) An efficient numerical scheme for Burgers' equation. Appl Math Comput 95: 37-50.
[17]	Sarra SA (2005) Adaptive radial basis function methods for time dependent partial differential equations. Appl Numer Math 54: 79-94.
[18]	Driscoll TA, Heryudono ARH (2007) Adaptive residual subsampling methods for radial basis function interpolation and collocation problems. Comput Math Appl 53: 927-939.
[19]	Zhang J, Chauhan S (2019) Real-time computation of bio-heat transfer in the fast explicit dynamics finite element algorithm (FED-FEM) framework. Numer Heat Transfer, Part B 75: 217-238.
[20]	Zhang J, Chauhan S (2019) Neural network methodology for real-time modelling of bio-heat transfer during thermo-therapeutic applications. Artif Intell Med 101: 101728.
[21]	Fahmy MA (2019) Boundary element modeling and simulation of biothermomechanical behavior in anisotropic laser-induced tissue hyperthermia. Eng Anal Bound Elem 101: 156-164.
[22]	Fahmy MA (2020) A new convolution variational boundary element technique for design sensitivity analysis and topology optimization of anisotropic thermo-poroelastic structures. Arab J Basic Appl Scis 27: 1-12.
[23]	Verma R, Kumar S (2020) Computational study on constant and sinusoidal heating of skin tissue using radial basis functions. Comput Biol Med 121: 103808.
[24]	Hoffman JD, Hoffman JD, Frankel S (2001) Numerical Methods for Engineers and Scientists CRC Press.
[25]	Kengne E, Mellal I, Hamouda MB, et al. (2014) A mathematical model to solve bio-heat transfer problems through a bio-heat transfer equation with quadratic temperature-dependent blood perfusion under a constant spatial heating on skin surface. J Biomed Sci Eng 7: 721-730.
[26]	Zhang ZW, Wang H, Qin QH (2015) Meshless method with operator splitting technique for transient nonlinear bioheat transfer in two-dimensional skin tissues. Int J Mol Sci 16: 2001-2019.
[27]	Woolfe G, MacDonald AD (1944) The evaluation of the analgesic action of pethidine hydrochloride (Demerol). J Pharmacol Exp Ther 80: 300-307.
[28]	Gholami M, Saboory E, Mehraban S, et al. (2015) Time dependent antinociceptive effects of morphine and tramadol in the hot plate test: Using different methods of drug administration in female rats. Iran J Pharm Res 14: 303-311.
[29]	Da Silva S, França AS, Pinatti M (2011) One-dimensional simulation of heat transfer in the canine knee joint during therapeutic heating and cooling. Braz J Biom Eng 27: 163-174.
[30]	Tita B, Abdel-Haq H, Vitalone A, et al. (2001) Analgesic properties of Epilobium angustifolium, evaluated by the hot plate test and the writhing test. Farmaco 56: 341-343.
[31]	Narasimhan A, Jha KK (2012) Bio-heat transfer simulation of retinal laser irradiation. Int J Numer Meth Biomed Eng 28: 547-559.
[32]	Ezzat MA, El-Bary AA, Al-Sowayan NS (2016) Tissue responses to fractional transient heating with sinusoidal heat flux condition on skin surface. Anim Sci J 87: 1304-1311.
[33]	Shih TC, Kou HS, Liauh CT, et al. (2002) Thermal models of bioheat transfer equations in living tissue and thermal dose equivalence due to hyperthermia. Biomed Eng: Appl, Basis, Commun 14: 86-96.
[34]	Zhang J, Chauhan S (2020) Fast computation of soft tissue thermal response under deformation based on fast explicit dynamics finite element algorithm for surgical simulation. Comput Meth Prog Bio 187: 105244.
[35]	Zhang J, Lay RJ, Roberts SK, et al. (2020) Towards real-time finite-strain anisotropic thermo-visco-elastodynamic analysis of soft tissues for thermal ablative therapy. Comput Meth Prog Bio 198: 105789.

This article has been cited by:

Humaira Mustanira Tariq, Rabia Hameed, Ghulam Mustafa, A Study on the Class of Non-Symmetric 3-Point Relaxed Quaternary Subdivision Schemes, 2022, 10, 2169-3536, 132164, 10.1109/ACCESS.2022.3230281

Reader Comments

Your name:*

Email:*
© 2021 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)