1.
Introduction
The sterile insect technique (SIT) is presently one of the most ecological methods for controlling insect pests responsible for disease transmission or crop destruction worldwide. This technique consists in releasing sterile males into the insect pest population [1,2,3]. This approach aims at reducing fertility and, consequently, reducing the target insect population after a few generations. Classical SIT has been modeled and studied theoretically in a large number of papers to derive results to study the success of these strategies using discrete, continuous, or hybrid modeling approaches (for instance, the recent papers[4,5,6,7,8,9,10]).
Despite this extensive research, little has been done concerning the stabilization of the target population near extinction after the decay caused by the massive initial SIT intervention, and there are still major difficulties due to the complexity of the dependency on climate, landscape, and many other parameters which would be difficult to be integrated into the mathematical models studied. Not being able to consider all these parameters in our mathematical models and knowing that these external factors strongly impact the evolution of the density of the target population, we focus our studies on releases that now depend on the target population density measurements since, as we will see below, this makes our control more robust. Indeed, several monitoring tools can provide information on the size of the wild population throughout the year. So, a control that considers this information to adapt the size of the releases is possible and useful. This was already the case of [9,11] in which a state feedback control law gives significant robustness qualities to the mathematical model of SIT. Although this approach provides evidence in terms of robustness because the control is directly adjusted according to the density of the population, its application requires to continuously measure the different states of the model. In practice, traps allow data to be collected to analyze the control's impact and technology is being developed that may allow us to obtain continuous data in the near future.
However, specific categories of data are still problematic or very expensive to obtain. For example, during an SIT intervention, it is difficult to measure the density of young females that have not yet been fecundated or of females that were fecundated by wild males. In this work we use another control theory tool, which consists of constructing a state estimator for a dynamical system and using this estimator to apply feedback control. A state observer or state estimator is a system that provides an estimate of the natural state using some partial measurements of the real system. In our case, using traps, wild males as well as sterile males, can be measured. Using the observer system technique, we have built a system that allows us to estimate all other states.
The problem of observer design for linear systems was established and solved by [12] and [13]. While Kalman's observer [12] was highly successful for linear systems, extending it to nonlinear systems took a lot of work. In several cases, the observer can be obtained from the extended Kalman filter by a particular choice of the matrix gain using linear matrix inequalities (LMIs). The development of the observer in this paper was motivated by its application to the SIT model. A model of this process can be written as
where y∈Rm is the output, x∈Rn is the state vector, and u∈Rp is the input. The output matrix B(y) is such that the coefficients b(y)ij are bounded for all i,j.
Our paper has three parts. In the first part, thanks to the backstepping approach, we build a feedback control law that stabilizes the zero population state for the SIT model for the mosquito population, which considers only the compartments of young females and fertilized females presented in [14]. In the second part we construct a state estimator for the SIT model. Finally, in the third part we show that the application of this feedback, depending on the measured states and the ones estimated thanks to the state estimator, globally stabilizes the system.
2.
Mosquito population dynamics
The mosquito life cycle has several phases. The aquatic stage comprises eggs, larvae, and pupa, followed by the adult stage, where we consider both wild males and females. After emergence from the pupa, a female mosquito needs to mate and then to get a blood meal before it can start laying eggs. Then, every 4−5 days, it will take a blood meal and lay 100−150 eggs at different places (10−15 per place). For the mathematical description, we will consider the following compartments [14].
● E the density of population in aquatic stage,
● Y the density of young females, not yet laying eggs,
● F the density of fertilized and egg-laying females,
● M the density of males,
● Ms the density of sterile males,
● U the density of females that mate with sterile males.
The Y compartment represents the stage of the young females before the start of their gonotropic cycle, i.e., before they mate and take their first blood meal. It generally lasts for 3 to 4 days. The sterile insect technique introduces male mosquitoes to compete with wild males. We denote by Ms the density of sterile mosquitoes and by U the density of females that have mated with them. We assume that a female mating mosquito has probability MM+Ms to mate with a wild male and probability MsM+Ms to mate with a sterile one. Hence, the transfer rate η from the compartment Y splits into transfer rate of η1MM+Ms to compartment F and a transfer rate of η2MsM+Ms to compartment U of females that will be laying sterile (non-hatching) eggs. The mathematical model is the system of ordinary differential equations presented in [15]
The parameter δY is the mortality rate for young females (they can die without mating for diverse reasons like predators or other hostile environmental conditions). Male mosquitoes can mate for most of their lives. A female mosquito needs a successful mating to be able to reproduce for the rest of her life, βE>0 is the oviposition rate; δE,δM,δF,δY,δs>0 are the death rates, respectively, for eggs, wild adult males, fertilized females, young females, and sterile males; νE>0 is the hatching rate for eggs; ν∈(0,1), the probability that a pupa gives rise to a female, and (1−ν) is, therefore, the probability of giving rise to a male. K>0 is the environmental capacity for eggs. It can be interpreted as the maximum density of eggs that females can lay in breeding sites. Since here the larval and pupal compartments are not present, we consider that E represents all the aquatic compartments, in which case, this term K represents a logistic law's carrying capacity for the aquatic phase, which also includes the effects of competition between larvae. The control function u represents the number of mosquitoes released during the SIT intervention. It is interesting to follow the evolution of the state U because female mosquitoes, once fertilized by sterile males, will continue their gonotrophic cycle normally and, therefore, can still transmit disease. We will assume in this work that
In [14,15], equilibria and their stability property were studied for the system without control.
Its basic offspring number is R0=η1βEννEδF(νE+δE)(η1+δY). For the rest of our work, we assume that
3.
Global stabilization by a feedback law
We assume that wild males are more likely to fertilize young females because they are born in the same egg-laying site. We define
Other authors, such as in [14], have already studied the stability of this type of model. The difference in our approach lies in the kind of control used initially for global stabilization. Indeed, in most of the prior studies, the controls u studied were independent of system states. Some previous works have considered certain simple applications of feedback control to SIT (see, for instance, [9,16,17]). In a previous paper [11], we used the backstepping method to build a feedback control system that simplifies the SIT model, which is presented in [4], assuming that all females are immediately fertilized. Here, we consider the system
Let N:=[0,+∞)6 and X:=(E,M,Y,F,U,Ms)T. When applying a feedback law u:N→[0,+∞), the closed-loop system is the system
where H is the righthand side of Eqs (3.2)–(3.7). The construction method remains the same as in our previous paper [11]. In this work, we also consider solutions in the Filippov sense of our discontinuous closed-loop system (see, for instance, [18,19,20,21,22,23]). Let us define x:=(E,M,Y,F,U)T. We must rewrite the target system (3.2)–(3.6) in the following form to apply the backstepping method (see, for instance, [35]HY__HY, Theorem 12.24, page 334]):
where f:R6→R5 represents the righthand side of (3.2)–(3.6). We then consider the control system ˙x=f(x,Ms) with the state being x∈D:=[0,+∞)5 and the control being Ms∈[0,+∞). We assume that Ms is of the form Ms=θM for a constant θ>0. Then, we define and study the closed-loop system
Its offspring number is
Note that if R(θ)≤1, 0∈R5 is the only equilibrium point of the system in D. Our next proposition shows that the feedback law Ms=θM stabilizes our control system ˙x=f(x,Ms) if R(θ)<1.
Proposition 3.1. Assume that
Then, 00 is globally exponentially stable in D for system (3.10). The exponential convergence rate is bounded from above by the positive constant c defined by relation (3.16).
Proof. We apply Lyapunov's second theorem. To do so, we define V:[0,+∞)5→R+, x↦V(x),
where σ>0 is a constant that we will choose later.
As (3.12) holds, V is of class C1, V(x)>V((0,0,0,0,0)T)=0,∀x∈[0,+∞)5∖{(0,0,0,0,0)T}, V(x)→+∞ when ‖x‖→+∞ with x∈D and
By choosing
we get
Using (3.12) once more, we get
with
This concludes the proof of Proposition 3.1. □
Remark 3.1. When the Allee effect is included in the model (for instance, [4,Eq (2.5),Page 25]), the control Ms=θM can still be used, and the proof of the stability result can still be done using the same Lyapunov function (3.13).
We define
and for α>0, the map G:N:=[0,+∞)6→R, (xT,Ms)T↦G((xT,Ms)T) by
Finally, let us define the feedback law u:N→[0,+∞), (xT,Ms)T↦u((xT,Ms)T), by
The global stability result is the following.
Theorem 3.1. Assume that (3.12) holds. Then, 00∈N is globally exponentially stable in N for system (3.2)–(3.6) with the feedback law (3.21). The exponential convergence rate is bounded by the positive constant cp defined by
Lemma 3.1. Assume that (2.13) and (3.12) hold, then ϕ>0.
Proof.
Let us define ϕ1:=(2+R(θ))η1βEννE−3R(θ)ΔηδF(νE+δE)δF(νE+δE)(1−R(θ))(1+θ). We get from the relation (2.13) that η1βEννE>δF(νE+δE)(η1+δY). So,
From relation (3.12), we get βEη1ννEδF(νE+δE)<Δη+(1+θ)(η2+δY). Thus,
□
Proof of Theorem 3.1. Let α>0 and define W:N→R by
We have
From now on, and until the end of this proof, we assume that (xT,Ms)T is in N, and until (3.43) below, we further assume that
One has
Since
We take u as given by (3.21). Therefore, in the case where
which, together with (3.31), leads to
Otherwise, i.e., if (3.32) does not hold,
so, by (3.21),
We consider two cases. If θM>Ms using (3.31), (3.34), and (3.35),
with
Otherwise, if θM≤Ms, using once more (3.31) and (3.35),
From Lemma 3.1, we deduce that ϕ>0, and as (xT,Ms)T∈N, one has ϕY(θM+Ms)2α(M+Ms)+θ(1−ν)νEE≥0.
Equation (3.39) becomes
The inequality (2.7) gives δs≥δM, and one has
(3.42) together with θM−Ms≤0 implies that
with
Let us now deal with the case where (3.30) is not satisfied. Note that, for every τ≥0, M(τ)+Ms(τ)>0 implies that M(t)+Ms(t)>0 for all t≥τ. Thus, if M(0)+Ms(0)=0, there exists ts∈[0,+∞] such that M(t)+Ms(t)=0 if, and only if, t∈[0,ts]∖{+∞}. Let us study only the case ts∈(0,+∞) (the case ts=0 is obvious and the case ts=+∞ is a corollary of our study of the case ts∈(0,+∞)). Let us first point out that, for every (M,Ms)T∈[0,+∞)2 such that M+Ms>0, one has
So,
Let t↦X(t)=(E(t),M(t),Y(t),F(t),U(t),Ms(t))T be a solution (in the Filippov sense) of the closed-loop system (3.2)–(3.6) such that, for some ts∈(0,+∞),
Note that (3.46) implies that
From (3.45), (3.47), and the definition of a Filippov solution, one has on (0,ts)
with
From (3.47) and the second line of (3.48), one has
From the first line of (3.48) and (3.50), we get
Let us first consider the case where Y(0)=0. Then, from the third line of (3.48) and (3.50), one has
To summarize, from (3.47), the fifth line of (3.48), (3.50), (3.51), and (3.52),
which, with (3.13), (3.16), and (3.25) gives
Let us finally consider the case where Y(0)>0. Then, from the third line of (3.48),
which, together with the fourth line of (3.48) and (3.51), implies
To summarize, from (3.47), the third and the fifth line of (3.48), (3.50), (3.51) and (3.56),
which, with (3.13), (3.14), (3.16), and (3.25) gives
where
To end the proof, we have to prove that Q>0. Using the relation (3.11) and (3.12), we have
Recall that Δη=η1−η2. One has
From the relation (2.13), η1βEννE>δF(νE+δE)(η1+δY).
We get
where
This proves Theorem 3.1 and gives the global exponential stability. From (3.37), (3.44), and (3.61), we obtain an estimate on the exponential decay rate
□
3.1. Numerical simulations
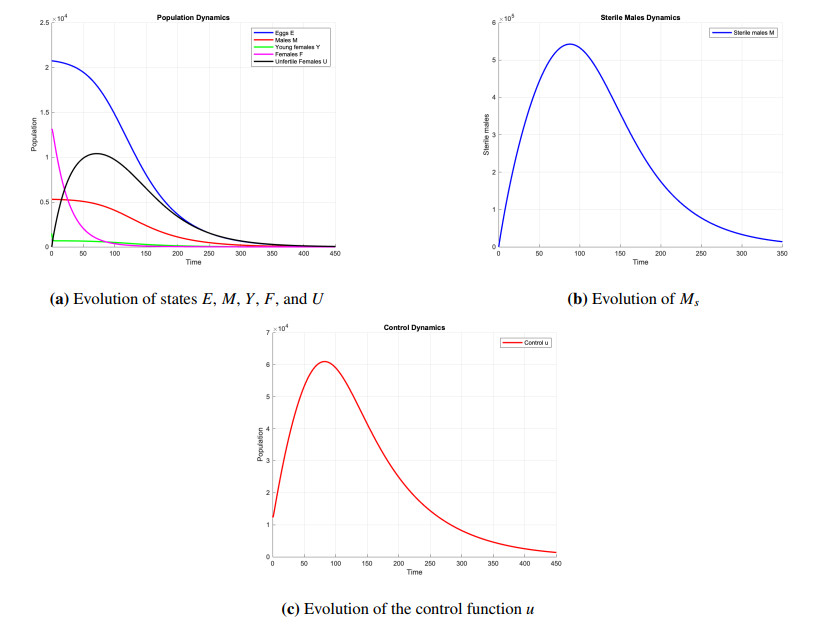
Note that η1 represents the natural fertility rate in the mosquito population. Wild males have a shorter maturity time in their life cycle than females. Thus, the fertilization phase is essentially around the hatching site. Sterile males are artificially released into the intervention region. We denote by p with (0≤p≤1) the proportion of sterile males that are released. Also, the effective fertilization during the mating could be diminished due to the sterilization, which leads us to assume that the effective mating rate of sterile insects is given by qη1 with 0≤q≤1. Putting together these assumptions, we get that the probability for a young female to mate with sterile males is η2MM+Ms with η2=pqη1. For the numerical simulation, we take η1=1 and η2=0.7. The numerical simulations of the dynamics when applying the feedback (3.21) is given in Figure 1. The parameters we use are given in the following table.
With the parameters given in Table 1, condition (3.12) is θ>102,06. We fix K=21000 and we consider the persistence equilibrium z0=(E0,M0,Y0,F0,U0,M0s) as initial condition. That gives E0=20700,M0=5300,Y0=1500,F0=13000, and U0=M0s=0. We take θ=290 and α=90.
Remark 3.2. Note that the feedback satisfies
The advantage of applying feedback control is that when the density of the target population decreases, the control also decreases.
Remark 3.3. It is important to note that the backstepping feedback control (3.21) does not depend on the environmental capacity K, which is also an interesting feature for the field applications. In the case K=+∞, the Eq (2.8) becomes
and we prove by the same process that the same feedback law (3.21) ensures the exponential stability of the SIT system (3.64), (3.3)–(3.7) with the same lower bound of the exponential convergence rate.
Our stabilization result is the following one.
Theorem 3.2. Assume that (3.12) holds and K=+∞. Then, 00∈N is globally exponentially stable in N for system (3.64), (3.3)–(3.7) with the feedback law (3.21). The exponential convergence rate is bounded by cp>0 and defined in (3.62).
Remark 3.4. Let us assume that the heterogeneity of the intervention zone strongly impacts the mating of female mosquitoes with sterile males more than we would have estimated. Suppose the estimated mating rate for the control (3.21) is ηe2=0.7, and let the mating rate be ηr2=0.4 for the dynamics. Keeping the other parameters and the same initial condition, we obtain the following figure. This parameter considerably impacts the convergence time of the states of the system. Note that with e=3×10−1 of error difference, we still have convergence. Estimation errors of the order of e=10−2 will have a negligible impact on the convergence time. This is because the backstepping control also depends on the states of the system. Thus, the states make a correction that can compensate for a certain margin of error. Unlike control, which only depends on the parameters, estimation errors have no correction from the dynamics. Therefore, this can be fatal to the success of the intervention. In practice, many external factors impact the life cycle of mosquitoes. These factors modify parameters such as birth, hatching, and fertilization rates. These factors are, for example, rainfall and the topography of the region. An SIT model that can integrate these factors is challenging to study (see [24]). The success of an SIT intervention depends strongly on the robustness of the control strategy. The results of our previous test that are reported in Figure 2 show us the advantage feedback control can provide in terms of robustness.
4.
Observer design for SIT model
The application of feedback control requires measuring states such as eggs E and young females Y of the intervention zone over time. In practice, it is always important to estimate the density of adult mosquitoes to intervene in an area. This data is collected using mosquito traps distributed throughout the region. Despite various technological advances to improve these traps, it should be noted that some data is still easier to be measured than others. Measuring mosquito density in the aquatic phase E is difficult, especially in a heterogeneous area. It is also challenging to measure young females Y because females come in three categories, and we need to distinguish between unfertilized and fertilized females. Males are more easily measured because they are distinguishable. It can be also easy to distinguish wild males from laboratory males by marking processes applied to laboratory males. In this part of our paper, we will assume that the density of wild males and that of sterile males can be measured continuously. Our objective is to estimate the other densities. Observer design for nonlinear dynamic systems is a technique used in control theory to estimate the states of a system when only partial or indirect measurements are available. The difficulties in dealing with observer problems for general nonlinear systems is the proof of global convergence of the estimation error. Much literature exists on state observers and filters for nonlinear systems as they play crucial roles in control theory. To simplify the nonlinearity F(1−EK) of the SIT model, in this section we consider the simplified SIT model for environmental capacity K=+∞. On the one hand, the reason for studying such a model is that the simplified model can be considered relevant from a biological point of view within a large intervention domain or in areas where environmental capacity is difficult to estimate. On the other hand, based on the result presented in Theorem 3.2, the proposed feedback law (3.21) still stabilizes the simplified model around zero with the same convergence rate. We consider the following output control system:
where the states are X=(E,M,Y,F,U,Ms)T∈N, the control is u∈[0,+∞), and the output is y=(M,Ms)T∈R2+.
In particular, in this model we are confronted with a difficulty in which most observer construction theories are invalid because of the singularity at the origin. To go around this difficulty, we will use the fact that the main nonlinearity term MM+Ms is bounded and essentially the most accessible data to measure. This leads us to develop an observer for this type of system.
4.1. Observer design for a class of nonlinear systems
The usual observers for linear systems are the Luenberger observer and the Kalman observer. Observer design for a nonlinear system is a complex problem in control theory and has received much attention from many authors yielding a large literature of methods. Among than, the most famous are the change of coordinates to transform the nonlinear system into a linear system [25,26,27,28,29] and a second approach consists in using the extended Kalman filter (EKF) [30,31,32,33]. The state observer is called an exponential state observer if the observer error converges exponentially to zero. In this section we provide an explicit construction of a global observer for the following system.
where x(t)∈Rn is the state vector, u(t)∈Rp is the input vector, and y(t)∈Rm is the output vector. A∈Rn×n and C∈Rm×n are the appropriate matrices. The matrice B(y(t)) is in the form
We assume that for all y(t)∈Rm, the coefficients bij are bounded for all i=1,⋯,n and j=1,⋯,n, and denote
Then, the parameter vector b(t) remains in a bounded convex domain Sn,n of which 2(n2) vertices are defined by:
A state observer corresponding to (4.9) is given as follows:
where ˆx(t) denotes the estimate of the state x(t). The dynamics of the observer error e(t):=ˆx(t)−x(t) are ˙e(t)=(A−LC)e(t)+B(y(t))e(t)=(A+B(y(t))−LC)e(t). We define
The dynamics of the observer error becomes
The observation problem consists in finding a gain L such that (4.14) converges exponentially toward zero. We use the following results in [34].
Theorem 4.1. The observer error converges exponentially toward zero if there exist matrices P=PT>0 and R of appropriate dimensions such that following LMIs are feasible:
for some constant ξ>0. When these LMIs are feasible, the observer gain L is given by L=P−1RT.
Proof. We follow [34] and consider the following quadratic Lyapunov function:
where P is the matrix in Theorem 4.1. We have ˙V(e)(t)=e(t)TF(b(t))e(t), where F(b(t))=(A(b(t))−LC)TP+P(A(b(t))−LC). For e(t)≠0, the condition V(e(t))>0 is satisfied because P>0, and the condition ˙V(e(t))<0 is satisfied if we have
Since the matrix function F is affine in b(t), using a convexity argument we deduce that ∀t≥0
if the following condition is satisfied F(η)<−ξI, ∀η∈Vn,n. Thus, if (4.15) holds, this inequality is also satisfied. □
4.2. Application to the SIT model
We rewrite the output SIT models (4.1)–(4.7) as
where X=(E,M,Y,F,U,Ms)T,
As, N is an invariant set, one has 0≤y1y1+y2≤1. Solving the corresponding equation of (4.15) with ξ=1 in MATLAB, we get
With the parameters given in Table 1, the result for a simulation run with x0=(400,100,150,120,120,50)T, ˆx0=(120,70,70,50,60,0)T, and u=500000 is plotted in Figure 3. The asymptotic behavior of the different estimates ˆE,ˆF,ˆY, and ˆU (dashed) illustrates the exponential convergence of the estimation error shown in Theorem 4.1.
5.
Dynamic output feedback
The feedback control (3.21) depends on the states E, M, Y, and Ms. From the measurement of states M and Ms, an observer system has been built in the previous section. This state observer is used to estimate both eggs E and young females Y. In this section, we show that u(ˆX,y) stabilizes the dynamics at the origin. We consider the coupled system
with
where S:R4×R2+→R, (ˆX,y)T↦S(ˆX,y) is defined by
The main result of this section is the following theorem.
Theorem 5.1. Assume that (3.12) holds. Then, 00∈E=N×R6 is globally exponentially stable in E for system (5.1) with the feedback law (5.2). The convergence rate is bounded by the positive constant ce defined by
Proof. Let λ>0, and we define H:E→R by
with e=ˆX−X.
In this proof, from now on we assume that (X,ˆX)T is in E. Until (5.16) is included, we also assume that
One has
Replacing the term ˆu(ˆX,y) by ˆu(ˆX,y)−ˆu(X,y)+ˆu(X,y), we get
Lemma 5.1. There exists C>0 such that, for all (X,ˆX)∈E and for all y∈R2+,
Note that ˙V(e)≤−ξ‖e‖2P. Thanks to this lemma, there exists C′>0 independent of y such that
Note that there exists a constant β>0 such that ‖e‖≤β‖e‖P. So,
Hence, for λ=4C′β/ξ, and using the relation (3.37) and (3.44),
We conclude that there exists a constant
such that
Let us now deal with the case where (3.30) is not satisfied. As we explained previously in the proof of the Theorem 3.1, it is sufficient to study only the case ts∈(0,+∞). Let t↦(E(t),M(t),Y(t),F(t),U(t),Ms(t),ˆE(t),ˆM(t),ˆY(t),ˆF(t),ˆU(t),ˆMs(t))T be a solution (in the Filippov sense) of the closed-loop system (5.1) such that, for some ts∈(0,+∞),
Note that (5.17) implies that
From (3.45), (3.47), and the definition of a Filippov solution, one has on (0,ts),
with
From (5.18) and the second line of (5.19), one has
From the first line of (5.19) and (5.22), we get
In the case where Y(0)=0, from the third line of (5.19) and (5.22), one has
To summarize, from (5.18), the fifth line of (5.19), (5.22), (5.23), and (5.24),
which, with (3.13), (3.16), and (3.25) gives
In the case where Y(0)>0, from the third line of (5.19),
which, together with the fourth line of (5.19) and (5.23) implies
Referring to this case already studied in the proof of Theorem 3.1, we get
Since Ms(t)=0∀t∈[0,ts], max(0,ˆYg1+ˆEg2)=0. For all κ(t)∈[0,1], in these two cases, the dynamics of the observation error remains
and one has
We conclude that there exists a constant
such that
This proves Theorem 5.1 and gives the global exponential stability with the exponential decay rate ce given by relation (5.4). □
5.1. Numerical simulations
We apply the backstepping control u function of the measured states y and the estimated states ˆE and ˆY given by the relation (5.2) with the following initial condition x0=(20000,5000,1500,12000,500) and ˆx0=(2000,500,150,1200,0).
The response of system (5.1) to the backstepping control (5.2) is illustrated in the Figure 4. The asymptotic convergence in large t of the different estimated ˆE,ˆF,ˆY, and ˆU (dashed) to their corresponding state variables E,F,Y, and U, respectively, illustrates the exponential convergence of the estimation error stated in Theorem 4.1. The convergence of the states and their estimates, toward zero proves the efficiency of the control (5.2) as shown in the Theorem 5.1. Figure 5 shows that the applied control function decreases when the density of the target population decreases.
6.
Conclusions
In this work, we have built a feedback control law to stabilize the SIT model presented in [14,15] at extinction. Control by state feedback is a type of control rarely proposed in the literature for the overall stabilization of the SIT model. The feedback control (3.21) developed in this work has many advantages, including robustness to changing parameters. We have shown in Remark 3.4 that despite the margin of error that can be made in the estimation of the parameters, this feedback control still makes the system converge to extinction. Moreover, it does not depend on environmental capacity and this control law ensures exponential stability with the same convergence rate for the SIT system even in the high environmental capacity limit (see Theorem 3.2). Remark 3.2 shows that when the density of the target population decreases, the control also decreases
In Section 4 of our work, we built an observer for the SIT model where, using the measurement of male mosquitoes, our state estimator gives us an estimate of the other states of the system. This aspect is rarely studied for this type of dynamics. An accurate estimate of the mosquito population enables resources to be allocated more efficiently. If the intervention is effective in some areas but not in others, resources can be reallocated to maximize impact. On the other hand, the data collected during the SIT intervention provides essential information on the impact of the control in the conditions of the intervention area. This will enable informed decisions on future control strategies to be adopted according to conditions in the intervention zone by adding complementary methods or by adapting existing approaches.
One of the applications we made was to show in Section 5 that by using the data estimated via our observer to adjust the feedback control, we globally stabilize the system upon extinction. Figure 4 shows that the difficulty of estimating eggs and young females during an intervention can be compensated by the application of the observer system. Data collected on the mosquito population is also used in epidemic prevention programs. They help to adapt public health programs for better control of mosquito-borne diseases.
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Acknowledgements
The author wishes to thank Luis Almeida and Jean-Michel Coron for having drawn his attention to this problem and for the many enlightening discussions during this work.
Conflict of interest
The authors declare there is no conflict of interest.









 DownLoad:
DownLoad:







