Global dynamics of an impulsive vector-borne disease model with time delays

1.   Introduction

Vector-borne disease involves a vector (an organism) that transmits infectious pathogens from the infected host to the uninfected host. For example, the malaria parasites are transmitted through the bite of infected female mosquitoes, spreading the disease from mosquitoes to humans and back to mosquitoes ^[2]. West Nile virus (WNv) is another vector-borne disease caused by Flavivirus and transmitted primarily by mosquitoes (Culex species) to vertebrate hosts, such as humans, birds and horses ^[3]. Those vector-borne diseases have caused a significant threat to public health as well as wildlife worldwide.

Mathematical models give insights to predict the spread of vector-borne diseases, and to test control strategies. Impulsive (delayed) differential systems are widely used in population biology and epidemiology to study the various factors: birth pulses ^[4,5], effectiveness of vaccination ^[6,7,8,9,10], and elimination of vector insects ^[11,12], see also ^[13,14] for stochastic effects. To investigate the control strategies against vector-borne diseases, Gourley et al. ^[11] used two time-delayed models with impulses to evaluate the effectiveness of age-structured culling strategies. Yang et al. ^[10] studied the effect of impulsive controls in multiple patch models with age-structure. Xu and Xiao ^[9] considered the impacts of periodic impulsive culling of mosquitoes for control of WNv transmission. Based on ^[9], the incubation period of mosquitoes was furthered incorporated in ^[15].

To describe the disease transmission between vector and host as well as the strategy of periodically culling the vector, we use a set of impulsive delayed differential equations to incorporate the extrinsic incubation period (EIP) of vector and host. By the theory recently developed in ^[1], the basic reproduction ratio $\mathcal{R}_{0}$ of the model is first established. The global dynamics of the model are further investigated in terms of $\mathcal{R}_0$. The uniform persistence theory based on dynamical systems is used extensively in population biology and epidemiology models, see for instance ^{[16,17,18,19]} and references therein. However, it seems like this dynamical system approach is rarely adapted to time-delayed impulsive systems to study the threshold dynamics. There are two possible issues: first, it is not an easy task to find an appropriate phase space for time-delayed impulsive systems so that the evolution operators are well-defined, which was recently solved by ^[1] for a large class of time-delayed impulsive systems; second, the solutions of the time-delayed impulsive systems may not be continuous in $t$, and hence, such systems might not generate continuous periodic semiflows (see ^[20]), which brings trouble for direct application of the uniform persistence theory in ^[21] to investigate the sharp permanence of infectious compartments when $\mathcal{R}_0 > 1$. Meanwhile, the analytic method developed in ^[1,6,7] for the uniform persistence of a time-delayed impulsive system is quite useful for a form like Susceptible-Exposed-Infected-Removed (SEIR), but it would not be easy to apply to the vector-borne model (including multiple infected compartments). We refer to ^[9,15] for attempts in this direction. Combing the ideas in ^[1] and ^[21], we establish the sharp persistence of infectious compartments, by using the uniform persistence theory of discrete-time semiflows on some appropriate phase space. It is worth pointing out that our current approach would be easily applied to the models where the period of model coefficients is the same as that of fixed impulsive moments.

The rest of this paper is organized as follows: In Section 2, we formulate a time-delayed impulsive differential model including several factors: EIP and the strategy of periodically culling the vector, EIP and vertical transmission of host. In Section 3, we first introduce the basic reproduction number $\mathcal{R}_0$ for the model and then study the threshold dynamics in terms of $\mathcal{R}_0$. In Section 4, we use numerical simulations to test the differences with and without culling, and further explore the impacts of parameters such as time delays and vertical transmission rate on $\mathcal{R}_0$. A brief discussion then concludes the paper.

2.   Model formulation

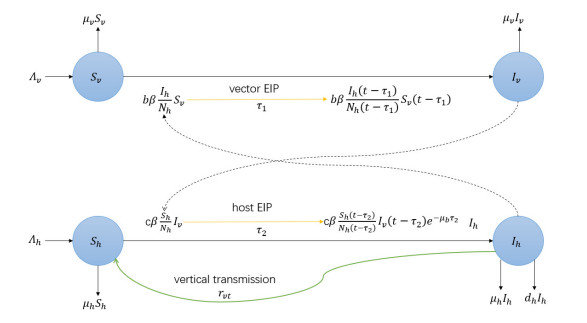
Inspired by the WNv infection process in ^[9,15,18], we consider an impulsive delay differential equation model to describe the periodic culling of the vector with two differnt EIPs. Let $S_{v}(t)$, $I_{v}(t)$, $S_{h}(t)$, $E_{h}(t)$ and $I_{h}(t)$ be the the total numbers of the susceptible adult vector, infected adult vector, susceptible host, exposed host and infected host at time $t$, respectively. The total number of the adult vector is given by $N_{h}(t) = S_{h}(t)+E_{h}(t)+I_{h}(t)$. Let $\Lambda_{v}$ and $\Lambda_{h}$ be the recruitment rate of the vector and (susceptible) host, respectively, $\mu_v$ and $\mu_h$ be the natural death rate of vector and host, and $d_h$ be the disease-induced death rate of the host. Similar to ^[7], we suppose that the birth rate of the vector equals its natural death rate. Vertical transmission of the virus in the host population is incorporated by a fraction $r_{vt}\in(0, 1)$. For the virus transmission, we assume that the susceptible host becomes infectious given contact with the infected vector, and the susceptible vector can receive the infection by biting a virus-carrying host. By similar arguments to those in ^[2,17,19], we simply assume that the numbers of newly occurred infectious host and newly occurred infected vector per unit time at time $t$ are given by

respectively, where $\beta$ is the average biting rate of the vector, $b$ and $c$ are the transmission probabilities of the virus from vector to host and from host to vector, respectively. However, the newly infected vector and infected host need to survive the EIP to become infectious. We denote the finite constants $\tau_1$ and $\tau_2$ to represent the length of the EIP in the vector and host, respectively. The probability that the vector and host survive the EIP is $e^{-\mu_{v}\tau_{1}}$ and $e^{-\mu_{h}\tau_{2}}$, then of those vectors and hosts infected $\tau_{1}$ and $\tau_{2}$ unit times ago, only the proportions

are infectious at time $t$. Throughout this paper, we suppose that culling occurs at certain particular times, and denote $T$ to be the period of culling. We also assume that spraying reduces both the susceptible and infected vector, $p\in\left[0, +\infty\right)$ is the culling intensity of those killed, where $p = 0$ means that there is no culling.

The transmission of cross-infection between vector and host is shown in Figure 1. Consequently, our model takes the form:

All the parameters in model (2.1) are positive. In view of the biological meaning of $\tau_{2}$, we impose the following compatibility condition:

To investigate the long time behavior of system (2.1) from the point view of dynamical systems, we first introduce a few notations related to the phase space (see also ^[1,22]). Given two constants $a < b$, let

where $\phi(t^+) = \lim\limits_{s\to t^+}\phi(s)$ and $\phi(t^-) = \lim\limits_{s\to t^-}\phi(s)$. Then for any $r > 0$, $PC([-r, 0], \mathbb{R}^m)$ is a Banach space with the norm $\|\cdot\|_{r}$ given by $\|\phi\|_{r} = \sup\limits_{-r\le\theta\le0}\vert\phi(\theta)\vert$. Set $\hat{\tau} = \max\left\{ \tau_{1}, \tau_{2}\right\} > 0$, $\widetilde{J} = [-\hat{\tau}, 0)\cap\{t_{n} = nT:n\in\mathbb{Z}\}$(possibly empty) and $J = [-\hat{\tau}, 0)\backslash\widetilde{J}$. Denote

and $PC_{J}^{+}: = \{ \phi\in PC_{J}:\phi(t)\geq 0, \forall t\in[-\hat\tau, 0]\}$. It then follows that $PC_{J}$ is a Banach space endowed with the norm $\|\cdot\|_{\hat\tau}$, and $PC^+_{J}$ is a closed cone of $PC_{J}$, which induces a partial ordering on $PC_{J}$. Clearly, $PC_{J}\subset PC([-\hat\tau, 0], \mathbb{R})$. For any $u = (u_1, u_2, ..., u_5)\in PC([-\hat\tau, \eta), \mathbb{R}^5)$ with $\eta > 0$, define $u_t\in PC([-\hat\tau, 0], \mathbb{R}^5)$ by

Define

and

for any $\epsilon\in\left(0, \frac{\Lambda_{h}}{\mu_{h}+d_{h}}\right).$ Now we are ready to state the well-posed result on the solutions of system (2.1).

Theorem 2.1. For any $\phi\in D_{\epsilon}$, system (2.1) exists a unique non-negative solution $u\left(t, \phi \right)$ on $\left[0, +\infty\right)$ with the initial value $u_{0} = \phi$, $u_{nT}\in D_{\epsilon}$ for any $n\in \mathbb{N}$. Moreover, $u_{t+T}(\phi) = u_t(u_T(\phi))$ for all $t\ge0$ and $\Phi: = u_T$ admits a global attractor in $D_\epsilon$.

Proof. For convenience, we denote

For any $t\ge 0, t\not = nT, n\in \mathbb{N}, \quad \phi\in D_\epsilon$, define

where

It is easy to check that $f(t, \phi)$ satisfies Lipschitz condition for $\phi$ on any compact subset of $\mathbb{R}_+\times X$. It follows from [22, Theorems 3.1 and 3.3] that system (2.1) admits a unique solution $u\left(t, \phi \right)$ satisfying $\sum_{i = 3}^{5}u_i(t, \phi) > \epsilon$ on the maximal existence interval $\left[0, t_{\phi} \right)$ with $u_0 = \phi\in D_{\epsilon}$ and $t_\phi\in(0, \infty)$.

Next we prove the solution of system (2.1) is non-negative on $[0, t_\phi)$. Given $\phi\in D_{\epsilon}$ and $t\in[0, T)$, we see that $f_i(t, \phi): = f_i(\phi)\ge0$ provided $i\in\{1, 2, 3, 5\}$ and $\phi_i(0) = 0$. It follows from [23, Theorem 5.2.1] and its proof that for $u_i(t, \phi)\ge0, i\in\{1, 2, 3, 5\},$ $\forall t\in[0, T)\cap[0, t_\phi).$ If $T < t_\phi$, then by the formulas of system (2.1) at the impulsive points, we have $u_i(t, \phi)\ge0, i\in\{1, 2, 3, 5\}, t\in[0, T]$. It then follows from the method of steps that $u_i(t, \phi)\ge0, i\in\{1, 2, 3, 5\}, t\in[0, t_\phi)$. Meanwhile, by the uniqueness of solutions of system (2.1) and the compatibility condition (2.2), we obtain that

Therefore, $u_4(t, \phi)\ge0$ for $t\in[0, t_\phi)$.

Notice that the total host population ($N_{h}(t): = \sum_{i = 3}^{5}u_i(t)$) satisfies

which implies $N_{h}(t)$ is an upper solution of

Denote $x(t; N_h(0))$ as the unique solution of (2.6) with $x(0) = N_h(0)$, then $N_{h}(t)\geq x(t; N_h(0))$ for any $t \in\left[0, t_\phi \right)$. This further yields that $N_{h}(t)\geq x(t; N_h(0))\geq\epsilon$ for any $t \in\left[0, t_\phi \right)$ provided $N_h(0)\geq\epsilon$ with $\epsilon\in\left(0, \frac{\Lambda_{h}}{\mu_{h}+d_h}\right)$. Furthermore,

which implies that if $\epsilon\leq\sum_{i = 3}^{5}\phi_i(0)\leq\frac{\Lambda_{h}}{\min\{\mu_h, d_h\}}$, then $\epsilon\leq\sum_{i = 3}^{5}u_i(t)\leq\frac{\Lambda_{h}}{\min\{\mu_h, d_h\}}$ for any $t \in\left[0, t_\phi \right)$.

Since $\epsilon\leq N_{h}(t)$ and $u_5(t)\le N_h(t)$ for any $t\in[0, t_\phi)$, by comparison, we have

which yields that,

Therefore, the solutions of system (2.1) with initial data in $D_{\epsilon}$ exist globally on $[0, \infty)$ and are ultimately bounded. By similar argument to that in [1, Lemma 4] (see also [24, Page 192]), we infer that for each $n > \frac{\hat\tau}{T}$, $u_{nT}$ is compact. It then follows from [21, Theorem 1.1.3] that $U_T: D_\epsilon\to D_\epsilon$ admits a global attractor. Moreover, since if $u_t(\phi)$ is a solution of (2.1), then $u_{t+T}(\phi)$ is also a solution (2.1). By the uniqueness of the solution, we then have $u_{t+T}(\phi) = u_t(u_T(\phi))$.

3.   Threshold dynamics in terms of $\mathcal{R}_0$

In this section, we first introduce the basic reproduction number $\mathcal{R}_0$ for system (2.1) and then investigate its threshold dynamics in terms of $\mathcal{R}_0$.

3.1.   Basic reproduction number

The basic reproduction number $\mathcal{R}_0$ is defined as the expected number of secondary infections produced by a typical infectious individual in a completely susceptible population. We will use the theory developed in ^[1] to give the definition of $\mathcal{R}_0$. In order to obtain the disease-free periodic solution of system (2.1), we recall a lemma (see [6, Lemma 1] for detail).

Lemma 3.1. Consider the following impulsive differential equation:

where $a, b > 0$, $\theta\in(0, 1)$. Then system (3.1) admits a unique positive periodic solution $\hat{u}_{e}(t): = \frac{a}{b}+ \left(u^*-\frac{a}{b}\right)e^{-b(t-nT)}$, $nT < t < (n+1)T$, which is globally asymptotically stable. Here $u^* = \frac{a(1-\theta)(1-e^{-bT})}{b(1-(1-\theta)e^{-bT})}$.

Letting $I_{v} = I_{h} = 0$ in system (2.1), we then get the following disease-free system:

By Lemma 3.1, system (3.2) exists a unique positive $T$-periodic solution $\hat u(t): = (\hat{S}_{v}(t), \hat{S}_{h})$, with $\hat{S}_{h} = \frac{\Lambda_h}{\mu_{h}}$ and

which is globally asymptotically stable.

Let $E = PC([-\hat{\tau}, 0], \mathbb{R}^2)$, $E^+ = \{\phi\in E:\phi(t)\geq 0, t\in[-\hat\tau, 0]\},$ and

with the norm $\| v \|_{X_T} = \sup\limits_{t\in [0, T]} \vert v(t)\vert$. It then follows that $X_T$ is a Banach space. Linearizing system (2.1) at its disease-free periodic solution $E_{0}(t) = (\hat{S}_{v}(t), 0, \hat{S}_{h}(t), 0, 0)$, we obtain the following linear system for the infectious compartments:

Define $F(t):E\rightarrow \mathbb{R}^2$, where

Set $Y(t, s), t\geq s$ as the Cauchy matrix [24, Section 1.2] of

that is,

where each $t_k$ denotes the impulsive point on $\left[s, t\right)$ and $V = \left(\begin{array}{ccc} \mu_{v} & 0\\ 0 & \mu_{h}+d_h \end{array} \right)$. It is easy to check that $F(t)$ and $Y(t, s)$ satisfy the following properties: (a) for each $t\in\mathbb{R}$, $F(t)$ is a positive operator; (b) the matrix $-V$ is cooperative and $r(Y(T, 0)) < 1$, where $r(Y(T, 0)$ is the spectral radius of $Y(T, 0)$.

Consequently, the linear impulse periodic differential system (3.5) could be rewritten as:

where $P = \text{ diag }(\frac{1}{1+p}, 1)$.

After the above settings, we can apply the theory and method in ^[1] to define basic the reproduction number for system (2.1). Let $v(t)$ be the initial distribution of infected individuals with period $T$. For $t\geq s$, then $F(t-s)v_{t-s}$ is the distribution of newly infected individuals at time $t-s$. It is produced by infected individuals introduced during the time interval $\left[t-s-\hat{\tau}, t-s\right]$, which means it is the distribution of newly infected at time $t-s$ and still infected at time $t$. Define the linear operator $L$ on $X_T$:

We define the basic reproduction number as

where $r(L)$ is the spectral radius of the linear operator $L$. Denote

For any $\phi\in E_J$, let $w(t, \phi) = (w_1(t, \phi), w_2(t, \phi))$ be the unique solution of (3.4) with the initial value $w_0 = \phi$, and

Let $Q(t):E_J\rightarrow E = PC([-\hat{\tau}, 0], \mathbb{R}^2)$ be the solution map of (3.4), then

In view of the definition of $PC_{J}$, we infer that $Q: = Q(T)$ is the operator from $E_J$ to $E_J$ (in other words, they have the same number of discontinuous points), and set $r(Q)$ to be the spectral radius of $Q$ on $E_J$. By [1, Theorem 1], we have the following observation:

Lemma 3.2. $\mathcal{R}_0-1$ has the same sign as $r(Q)-1$.

Let

where $PC_{\tau_i}$ is understood as $PC_J$ with $\hat\tau$ replaced by $\tau_i$, and then $(Z, Z^+)$ is an ordered Banach space. For any $\psi\in Z^+$, system (3.4) admits a unique non-negative solution $z(t, \psi)$ with the intial value $z_0 = \psi$. For all $t\geq0$, we have $z_t(\psi) = (z_{1t}(\psi), Z_{2t}(\psi))$, for all $\theta_{i}\in\left[-\tau_{i}, 0\right]$, and then $Z_{it}(\psi)(\theta_i) = z_i(t+\theta_i, \psi)$, $i = 1, 2.$ For any given $t\geq0$, let $\hat{Q}(t)$ be the solution map of system (3.4) on $Z$, that is,

We remark that $z_{1t}\in PC([-\tau_2, 0], \mathbb{R})$ and $z_{2t}\in PC_{\tau_1}$ for all $t\ge0$ as $z_2(t, \psi)$ is continuous for all $t\ge0$ even if $\psi$ is piecewise continuous. Therefore, $\hat{Q}(T)$ is a map from $Z$ to $Z$. Next, we will illustrate that $\hat{Q}(t)$ is eventually strongly positive on $Z^+$.

Lemma 3.3. $\hat{Q}(t)\varphi\gg0$ for any $t > 3\hat\tau$ provided $\varphi = (\varphi_1, \varphi_2)\in Z^+\backslash\left\lbrace 0\right\rbrace$.

Proof. For convenience, denote

By the similar argument to that in Theorem 2.1, we obtain that $z_i(t)\ge0$ for any $t\ge0$, $i = 1, 2$.

In the case that $\varphi_1 > 0$. We argue that there exists $\hat{t}\in\left[0, \hat{\tau}\right]$ such that $z_2(\hat{t}) > 0$. Assume, by contradiction, that $z_2(t) = 0$ for all $t\in[0, \hat{\tau}]$, that is, $z'_2(t) = 0$ for all $t\in[0, \hat{\tau}]$. Then we deduce from the second equation of system (3.4) that:

This yields $\varphi_1(t)\equiv0$ for any $t\in[-\tau_2, 0]$, which is a contradiction. Moreover, from the second equation of system (3.4) and $z_1(t)\ge0$ for all $t\ge-\tau_2$, we obtain

Then by the integral form of the first equation of system (3.4), we have

where

Since $\hat{t}\in[0, \hat{\tau}]$, it follows that $z_1(t) > 0$ for all $t > 2\hat\tau$. The result as follows.

In the case that $\varphi_2 > 0$. We have

provided $t > \hat \tau$. Then by the integral form of the second equation of system (3.4), we have

whenever $t\ge 2\hat\tau.$ Therefore, $\hat{Q}(t)\varphi\gg0$ for $t > 3\hat{\tau}$.

Denote $r(\hat{Q})$ as the spectral radius of $\hat{Q}: = \hat{Q}(T)$. One might perform the same argument as in [16, Lemma 3.8] to obtain $r(Q) = r(\hat{Q})$. Moreover, we have the following observation:

Lemma 3.4. Let $\mu = \frac{\ln r(Q)}{T}$. Then there exists a positive T-periodic function $\tilde{v}(t) = (\tilde{v}_1(t), \tilde{v}_2(t))$ such that $e^{\mu t}\hat v(t)$ is a solution of system (3.4) with the feasible domain either $Z^+$ or $E^{+}_{J}$ for any $t\geq0$.

Proof. Resembling the arguments in [1, Lemma 4], we infer that for each $t > \hat{\tau}$, $Q (t)$ and $\hat{Q}(t)$ are compact on $E_J$ and $Z$, respectively.

In the case that the feasible domain is $Z^+$, fix an integer $n_0 > 0$ such that $n_0T > 3\hat{\tau}$. It follows that $\hat{Q}^{n_0} = \hat{Q}(n_0T)$ is compact and strongly positively on $Z$. By [25, Lemma 3.1], we obtain that there is a strongly positive eigenvector $\varphi^* = (\varphi_1^*, \varphi_2^*)$ such that $\hat{Q}(\varphi^*) = r(\hat{Q})\varphi^*$. Let $v(t, \varphi^*)$ be the solution of system (3.4) with the initial value

Since $\varphi^*\gg 0$, it is easy to see that $v_t(\varphi^*)\gg 0$ for any $t\ge0$. Let $\hat{v}(t) = e^{-\mu t}v(t, \varphi^*)$. Then $\hat{v}(t)\gg 0$ satisfies

For $\theta_2\in[-\tau_1, 0]$ and $\theta_1\in[-\tau_2, 0]$, we have

This implies $\hat{v}_i(\theta_i) = \hat{v}_i(T+\theta_i)$, $i = 1, 2.$ Therefore, $\hat v(t)$ is a $T$-periodic solution satisfying $\tilde{v}_i(\theta_i) = e^{-\mu\theta_i}\varphi^*_i(\theta_i)$, $i = 1, 2.$

In the case that the feasible domain is $E^+_J$, since $e^{\mu t}\hat{v}(t)$ given in the above is a function for $t\in\mathbb{R}$. We denote $\hat{\phi} = (\hat{\phi_1}, \hat{\phi_2})$ as:

By the uniqueness of solutions, we have that $w(t, \hat{\varphi}): = e^{\mu t}\hat{v}(t)$ satisfies (3.4) for all $t\geq 0$ with the initial value $w_0 = \hat\phi\in E^+_J$.

3.2.   Threshold dynamics

We are now in a position to prove a threshold-type result of system (2.1) in terms of $\mathcal{R}_0$.

Theorem 3.5. If $\mathcal{R}_0 < 1$ and $\mu_h\ge d_h$, then the disease-free periodic solution $E_{0}(t) = (\hat{S}_{v}(t), 0,$ $\hat{S}_{h}(t), 0, 0)$ is globally attractive for system (2.1) with respect to $D_{\epsilon}$.

Proof. By the first and sixth equations of system (2.1), we have

Consider the following auxiliary system

According to Lemma 3.1 and the comparison theorem on impulsive differential equations, we have $S_{v}(t, a)\leq x(t, a)$ with the same initial condition $S_v(0) = x(0) = a > 0$, $\forall t\geq 0$ and $|x(t)-\hat{S}_v(t)|\to 0$ as $t\rightarrow \infty$ with $\hat S_v(t)$ given in (3.3). Then for any $\delta\in(0, \frac{\Lambda_{h}}{\mu_{h}})$, there exists $\hat{t}_1 > 0$ such that

When $\mu_h\ge d_h$, in view of system (2.1), we have

It then follows that for the above $\delta$, there exists $\hat{t}_2 > \hat{t}_1$ such that

Thus, we have

We consider the following impulsive differential equations with parameter $\delta$:

From Lemma 3.2, we know that $\mathcal{R}_0 < 1$ if and only if $r(Q) < 1$. Let $Q_\delta$ be the Poincaré map of system (3.9). Observe that $\lim\limits_{\delta\rightarrow0^+} r(Q_\delta) = r(Q) < 1$ (as $r(Q_\delta)$ is non-decreasing and upper semi-continuous in $\delta\ge0$ but close to $0$ (see ^[26,27] for example), we can fix a small positive number $\delta$ such that $r(Q_{\delta}) < 1$. By Lemma 3.4, there is a positive $T$-periodic function $\hat{v}^{\delta}(t) = (\hat{v}_1^{\delta}(t), \hat{v}_2^{\delta}(t))$ such that $e^{\mu_{\delta}t}\hat{v}^{\delta}(t)$ is a positive solution of system (3.9), where $\mu_{\delta} = \frac{\ln r(Q_{\delta})}{T} < 0$. Choose a positive constant $K_{\delta}$ such that:

Applying the comparison principle for cooperative impulsive delay differential systems [28, Lemma 2.2], we obtain that

Letting $t\to\infty$ in (3.10), we have $(I_v(t), I_h(t))\rightarrow (0, 0)$. It then follows from the fourth equation or (2.4) that $E_h(t)\to 0$ as $t\to\infty$. In view of the theories of asymptotically periodic semiflows and internally chain transitive sets ^[21], we further deduce from the third equation of system (2.1) that

Then for any given $\delta_1\in(0, \delta)$, there exists $\hat{t}_3 > \hat{t}_2+\hat{\tau}$ such that

and hence,

For this inequality, we consider the following impulsive differential equation:

By Lemma 3.1, system (3.11) admits a globally stable $T$-periodic solution, denoted as $\hat{S}_v^{\delta_1}(t)$, which depends continuously on $\delta_1$. It follows that for the above $\delta$, there exists a $\hat{t}_4 > \hat{t}_3$ such that

This, together with (3.8), yields for any $\delta_1\in(0, \delta)$

Letting $\delta_1\rightarrow0^+$, we obtain for any small $\delta > 0$, that there holds

which implies that $\lim\limits_{t\rightarrow \infty}(S_{v}(t)-\hat{S}_v(t)) = 0$.

The result follows. In the remainder of this section, we investigate the uniform persistence of system (2.1).

Theorem 3.6. Assume that $\left(S_v(t, \phi), I_v(t, \phi), S_h(t, \phi), E_h(t, \phi), I_h(t, \phi) \right)$ is the unique solution of system (2.1) through $\phi\in X_0: = \left\{\left(\phi_1, \phi_2, \phi_3, \phi_4, \phi_5\right) \in D_{\epsilon}:\phi_2(0) > 0, \phi_5(0) > 0\right\}$. If $\mathcal{R}_0 > 1$, then there exists $\rho^* > 0$ such that $\liminf_{t\rightarrow \infty} I_i(t, \phi)\geq\rho^*, i = v, h$.

Proof. Let $\partial X_0: = \{\phi\in D_\epsilon: \phi_2(0) = 0 \text{ or } \phi_5(0) = 0\}$. For any $\phi\in X_0$, it is easy to check that

that is, $\Phi^n(X_0)\subset X_0$ for any $n\in\mathbb N$ with $\Phi: = u_T$ given in Theorem 2.1 having a global attractor in $D_\epsilon$. Define

let $\omega(\psi)$ be the omega limit set of the forward orbit $\Gamma = \{\Phi^n\psi:n\in \mathbb{N}\}$, and

Claim 1: For any $\psi\in M_{\partial}$, $\omega(\psi) = \mathcal{M}$, which is locally stable in $M_\partial$.

For any given $\psi\in M_\partial$, it follows from the definition of $M_\partial$ that for each $n\in \mathbb{N}$, there holds $I_v(nT, \psi) = 0$ or $I_h(nT, \psi) = 0$. Consequently, we infer that either $I_v(t, \psi)\equiv 0$ or $I_h(t, \psi)\equiv0$ for any $t\ge0$ (as if there exists $t_0\ge0$ such that $I_{i_0}(t_0, \psi)\not = 0$ for some $i_0\in\{v, h\}$, then $I_{i_0}(t, \psi) > 0$ for all $t\ge t_0$).

In the case that $I_v(t, \psi)\equiv0$ for each $t\ge0$, it follows from the second equation of system (2.1) that $I_h(t-\tau_1)S_v(t-\tau_1) = 0$ for any $t\ge0$. By the first and sixth equations of (2.1), we have

This implies there exists $\bar t_1 > 0$, such that $S_v(t) > 0$ for all $t > \bar t_1+\tau_1$, and hence, $I_h(t) = 0$ and $E_h(t) = 0$ for all $t\ge0$. Since disease-free system (3.2) admits a globally stable $T$ periodic solution $(\hat S(\cdot), \hat S_h)$, we obtain that $\omega(\psi) = \mathcal{M}$.

In the case that $I_h(t, \psi)\equiv0$ for each $t\ge0$, it follows from the fifth equation of system (2.1) that $S_h(t-\tau_2)I_v(t-\tau_2) = 0$ for any $t\ge0$. By the third equation of (2.1), we have $S'_h(t)\ge \Lambda_{h}-(\mu_v+c\beta)S_h(t), t > 0.$ This implies there exists $\bar t_2 > 0$, such that $S_h(t) > 0$ for all $t > \bar t_2+\tau_2$, and hence, $I_v(t) = 0$ and $E_h(t) = 0$ for all $t\ge0$. For the same reason, we obtain that $\omega(\psi) = \mathcal{M}$. Therefore, Claim 1 is valid.

For $\sigma\in\left(0, \min\{\min_{t\in(0, T] }\hat{S}_v(t), \hat{S}_h\}\right)$, let $Q_\sigma(t)$ be the solution map of the following system and $Q_\sigma = Q_\sigma(T)$ be the associated Poincaré map

For $\sigma$ close to $0^+$, by the comparison principle, it follows that the spectral radius $r(Q_\sigma)$ is non-increasing in $\sigma$, which implies $\lim\limits_{\sigma\to0^+}r(Q_\sigma)$ exists and $\lim\limits_{\sigma\to0^+}r(Q_\sigma)\le r(Q)$. Note that $r(Q_\sigma)$ is upper semi-continuous in $\sigma\ge0$ (see ^[26,27]), which further yields $\limsup_{\sigma\to 0^+}r(Q_\sigma)\ge r(Q)$, and hence, $\lim\limits_{\sigma\rightarrow0^+}r(Q_\sigma) = r(Q) > 1$. Thus, we can fix a sufficiently small number $\sigma > 0$ such that $r(Q_{\sigma_0}) > 1$. By Lemma 3.4, there exists a positive $T$-periodic function $\hat{v}^\sigma = (\hat{v}^\sigma_1, \hat{v}^\sigma_2)$ such that $e^{\mu_\sigma t}\hat{v}^\sigma(t)$ is a positive solution of system (3.14), where $\mu_\sigma = \frac{\ln r(Q_\sigma)}{T} > 0$.

By the continuous dependence of the solution (see ^[20]) on the initial value, we have for the above chosen $\sigma > 0$, and there exists $\sigma^*\in(0, \sigma)$ such that $\|u_t(\phi)- u_t(\mathcal{M})\| < \sigma$ for any $t\in[0, T]$ provided $\|\phi-\mathcal{M}\| < \sigma^*$. Next we prove the following claim:

Claim 2: For all $\phi\in X_0$, there holds $\limsup\limits_{n\to\infty}\|\Phi^n\phi-\mathcal{M}\|\ge\sigma^*.$ Assume, by contradiction, that $\limsup\limits_{n\to\infty}\|\Phi^n\hat\phi-\mathcal{M}\| < \sigma^*$ for some $\hat\phi\in X_0$. Then there exists $n_1\ge1$ such that $\|\Phi^n\hat\phi-\mathcal{M}\| < \sigma^*$ for any $n\ge n_1$. For any $t\ge n_1 T$, letting $t = n T+t'$ with $n = [\frac{t}{T}]$ and $t'\in[0, T)$, by Theorem 2.1, we have

It then follows that for any $t > n_1T-\hat\tau$, $0 < I_i(t) < \sigma, i = v, h$, $S_v(t) > \hat S_v(t)-\sigma$, $\hat{S}_h-\sigma < S_h(t)\le N_h(t) < \hat S_h+3\sigma$. And hence, $\frac{S_v(t-\tau_1)}{N_h(t-\tau_1)}\ge \frac{\hat S_v(t-\tau_1)-\sigma}{\hat S_h+3\sigma} > 0$ and $\frac{S_h(t-\tau_2)}{N_h(t-\tau_2)}\ge\frac{\hat{S}_h-\sigma}{\hat S_h+3\sigma} > 0$ for any $t > n_1T$. By the comparison principle for cooperative systems, it follows that $I_v(t, \hat\phi)$ and $I_h(t, \hat\phi)$ in system (2.1) satisfy

Recall that $Ke^{\mu_\sigma t} \hat v^\sigma(t)$ is a positive solution of (3.14) for any $K > 0$. Choose $K_\sigma > 0$ such that

Then the comparison theorem for cooperative impulsive delay differential systems implies that

Since $\mu_\sigma > 0$, we get $I_v(t, \hat\phi), I_h(t, \hat\phi)\rightarrow \infty$ as $t\rightarrow \infty$, which leads to a contradiction.

The above claims indicate that $\mathcal{M}$ cannot form a cycle for $\Phi$ in $D_\epsilon$ and $W^s(\mathcal{M})\cap X_0 = \emptyset$, where $W^s(\mathcal{M})$ is the stable set of $\mathcal{M}$ for $\Phi$. Now we define a continuous distance function $p: D_\epsilon\to \mathbb{R}_+$ by $p(\phi) = \min\{\phi_2(0), \phi_5(0)\}$. In view of Claim 1, we have $W^s(\mathcal{M})\cap p^{-1}(0, \infty) = \emptyset$. Now by [21, Lemma 1.2.1 and Theorem 1.3.2] and Theorem 2.1, it then follows that $\Phi$ is uniformly persistent with respect to $(X_0, \partial X_0, p)$, that is, there exists $\rho_1 > 0$ such that

Consequently, there exists $n_2\ge 1$, such that

Now for any $t\ge n_2 T$, letting $t = n T+\bar t$ with $n = [\frac{t}{T}]$ and $\bar t\in[0, T)$, we obtain from the equation of $I_v$ that

Therefore,

Similarly, we see from $I_h(t)\ge-(\mu_h+d_h)I_h(t), t\ge nT$ that

Now set $\rho^* = \min\left\{\frac{\rho_1 e^{-\mu_v T}}{2(1+p)}, \frac{\rho_1e^{-(\mu_h+d_h)T}}{2}\right\} > 0$, we see that $\liminf_{t\to\infty} I_i(t)\ge\rho^*, i = v, h.$

4.   Numerical simulations

In this section, we perform illustrative numerical simulations to verify theoretical results and explore the influences of key model parameters on the disease transmission. Note that the following simulations were based on the WNv disease transmission process studied in ^[9,15], where vector and host are mosquito and bird, respectively, and some of parameters were chosen only for the test of parameter sensitivity on the basic reproduction number.

Below, we take day as the time unit. Following the work in ^[9], we choose the baseline parameters as $\Lambda_{v} = 100, \Lambda_{h} = 2.1, \mu_{v} = 0.05, \mu_{h} = 0.001, \beta = 0.16, c = 0.6, d_h = 0.005$. Since the EIP of vector (mosquito) is about 8–12 days, and the EIP of the host (bird) is about 3–5 days, we set $\tau_{1} = 8, \tau_{2} = 3$ unless stated otherwise.

Similar to ^[15], choose $T = 10, p = 0.6$ while $\mathcal{R}_0 = 1.1490$. Figure 2(a) shows that the number of infected vectors tends to the periodical oscillation when culling occurs, whereas it tends to a equilibrium without culling. In addition, we see from Figure 2(a) that culling significantly reduces the amount of infections of the vector compared to the case of no culling. Choose $T = 10, p = 2$ while $\mathcal{R}_0 = 0.7142$. As shown in the Figure 3(b), the number of infected hosts that are not culled will continue to increase, and after the action of culling, the number of infected hosts will decrease, and the disease will eventually die out.

Next, we use PRCCs (partial rank correlation coefficients) to obtain the sensitivity analysis of $\mathcal{R}_0$. We take $\Lambda_{v}, \Lambda_{h}, \mu_{v}, \mu_{h}, \beta, b, c, p, d_h$ and $r_{vt}$ as the input variables and the value of $\mathcal{R}_0$ as the output variable. Figure 4 illustrates that parameters $\Lambda_{h}, \mu_{v}, \mu_{h}, p$ and $d_h$ are negatively correlated with $\mathcal{R}_0$ and the others are positively correlated. We also see that $\mathcal{R}_0$ is more sensitive to $\mu_{h}, \beta, b, c$ and $p$. Thus, the corresponding control measures should be taken for these sensitive parameters. For instance, to reduce mosquito-bird contact and further control the disease spread, we could burn repellent plants in the bird habitat or at their water sources.

In Figure 5, we investigate the joint effects of $\beta, p$ on $\mathcal{R}_0$. It shows that when the biting rate $\beta$ is large, it requires very strong culling effect. In Figure 6 we also choose different $r_{vt} = 0.01, r = 0.05, r = 0.1$, while $\beta = 0.05$, and get $\mathcal{R}_0$ increasing in $r_{vt}$ (compare (a)–(c)). Both Figures 5 and 6 indicate that when all parameters are equal, $\mathcal{R}_0$ is decreasing in $p$. All the results are consistent with the conclusion in sensitivity analysis.

Finally, we examine the impact of the EIP on $\mathcal{R}_0$. We let $\tau_{1}$ vary from $0$ to $10$ and $\tau_{2}$ vary from $0$ to $5$. Figure 7 describes the dependence of $\mathcal{R}_0$ on $\tau_{1}$ and $\tau_{2}$ for three different frequencies of culling (i.e. $\frac{1}{T}$). As we see from this figure, $\mathcal{R}_0$ is decreasing with respect to $\tau_{1}$ as well as $\tau_{2}$ and it increases as $T$ increases for fixed $\tau_{2}$ and $\tau_{1}$. This suggests that culling during the EIP and prolonging the EIP would be beneficial for disease control, while infrequent culling might be counterproductive.

5.   Discussion and conclusions

In this paper, we have formulated an impulsive vector-borne disease model with time delays to investigate the joint effects of the EIP and impulsive intervention. The basic reproduction number $\mathcal{R}_0$ is first derived by the theory in ^[1], which serves as a threshold value to determine the extinction and uniform persistence of the disease. Unlike most existing works ^[1,6,9,15], we utilize the dynamical system approach to show the sharp uniform persistence as $\mathcal{R}_0 > 1$. As is well known, the uniform persistence theory often gives rise to the existence of a positive periodic solution (see [21, Theorem 1.3.10]). As a complement, we could also show the existence of a positive periodic solution in ^[1,9,15] via our strategy. However, we emphasize that since our feasible domain $D_\epsilon$ in (2.3) is non-convex, we could not directly utilize it to verify the existence of the positive periodic solution, and we will leave the general existence and its stability for further investigation.

In addition, there are several possible extensions of this work. For example, EIP is often sensitive to temperature, which would lead to study of the time-varying delay (especially time-periodic delays as in ^[16]), whose periods might be different from the fixed impulsive moments, and so it requires a more careful and delicate deviation of the theory in ^[1,21]. At the moment, we are working on a time-periodic impulsive model with constant delays, where the impulsive moments are different from the real-time periods. In a different work, one could elaborate our model by incorporating the stochastic effects, and perform the stability analysis of the model system using some recent results in ^[29,30,31].

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

We are grateful to anonymous reviewers for their careful reading and valuable suggestions which led to an improvement of our original manuscript. This research was supported by the NSF of China (Nos. 12001205 and 12371169).

Conflict of interest

The authors declare there is no conflict of interest.