1.
Introduction
Business firms have a typical life cycle; they legally come into being, have an initial start-up phase, grow and enjoy stabilization and maturity. However, in today’s volatile, uncertain, complex and ambiguous as well as at a dizzying pace changing business environment, the life cycle is subject to frequent and even consecutive negative phases. Managed improperly, bankruptcy is the ultimate end of such downturns.
To illustrate, averagely 56,590 companies were established and 13,103 were liquidated each year between 2010 and 2017 in Turkey corresponding to a percentage of 23.2% including corporations, limited liability companies and general partnerships (Central Bank of the Republic of Turkey (CBRT) Electronic Data Delivery System (EVDS), 2018c). This is to imply that each year thousands of companies were set up and started their operations. On the contrary, a large number of companies ceased their operations in the same period. Although it may not be definitely inferred that approximately one-quarter of newly established companies are quitting their business, it is obvious to say that a considerably high number of companies regularly stop their operations.
The reasons why companies suffer from insufficient performance, fail and quit their business have been of major interest to researchers. One reviewing the literature in the field of distress and performance prediction models is likely to encounter voluminous studies incorporating accounting-based and market-based firm data. In contrast, those touching upon external conditions and agammaegate indicators in relation to firm performance are comparatively less evident.
Accordingly, the aim of this study is to analyze the impact of financial fragility on firm performance through panel data regression models. Integrating data from 4193 observations of 492 diverse listed companies on Borsa Istanbul with coverage from 2005 to 2017, the study will focus on selected firm performance constructs with respect to a variety of macroeconomic indicators.
In this context, the study will emphasize the research questions on how the impact of financial fragility on firm performance can be measured and what macroeconomic indicators are to be primarily followed up on by company management in terms of financial performance.
The rest of this paper is organized as follows. Section 2 focuses on the literature review on the concepts of financial fragility, firm performance and their relationship with each other. Section 3 describes the data set and methodology to assess the relationship between financial fragility and firm performance. Section 4 presents the empirical results. Section 5 is the conclusion of the study.
2.
Literature review
2.1. Financial fragility and firm performance
In general, the common characteristic of all economic units such as the households and firms at the micro-level and governments and policy-makers at the macro level is to strive for a sound and a healthy functioning economy. In reality, a sound and a healthy functioning economy is foremost characterized with monetary and financial stability in which monetary stability implies the absence of inflation or deflation; hence, stability in the overall price level, and financial stability is relevant to the proper functioning of financial markets and institutions (Crockett, 1997). In stable financial markets, financial asset prices do not fluctuate extensively and the solvency of financial institutions is secured (Saccomanni, 2008). In addition, as Montiel (2011) concludes with empirical evidence from the findings of recent research, macroeconomic stability fosters economic growth; hence, it is of key importance from the viewpoint of all economic and financial players.
Besides the basic definitions illustrated above, describing and gauging financial stability is a tough endeavor due to the complexity of the financial system as well as the cobweb-like structure of diverse relations among financial and macroeconomic units (Gadanecz and Jayaram, 2009). With this respect, the endeavors aimed at expressing financial stability on quantitative terms have been a focal point for researchers from academic, public and private areas in which a concise summary of financial stability relevant variables by sectors in the literature is depicted in Table 1 below (Gadanecz and Jayaram, 2009). This table portrays the various dimensions of financial stability and its complex nature in terms of micro and macroeconomic actors as well as the production, consumption, service and financial sectors including a country’s transactions with the rest of the world.
The financial system acts as an intermediary between those in excess of funds and fund seekers literally known as creditors/lenders and debtors/borrowers. In this context, it is incumbent on the financial system to make an efficient allocation of funds between these two parties (Klemkosky, 2013). Any possible malfunctions in the system increase the likelihood of an unwanted adverse state in the economy as a whole.
The periods where stability is distorted can be expressed as times of instability, disorder, stress or distress. Such periods are characterized and defined by particular instances. To illustrate, Hakkio and Keeton (2009) describe the situation in which the ordinary operations of the financial system are disturbed as financial stress. The authors further argue that stress in this context pertains to elevated ambiguity in asset values, raised unpredictability in the actions of financial actors, reduction in the availability of information to all relevant parties, increase in risk-averseness and increase in the preference for liquidity. Davis (1995) describes financial instability by referring to systematic risk and disorder clarifying that instability is an instance characterized by upheavals and volatilities in the financial markets that are likely to result in financial failure. Allen and Wood (2006) define financial instability as an incident where numerous economic actors regardless of being at the micro or macro level such as the “households, companies, or (individual) governments experience financial crises which are not warranted by their previous behavior, and where these crises collectively have seriously adverse macro-economic effects”. Taking this definition as a starting point, the authors define financial stability as an instance far from financial instability in which the actions of economic actors are not affected by the likelihood of such an unfavorable situation. Montiel (2011) defines macroeconomic instability as an event in which macroeconomic parameters are no longer foreseen or forecasted clearly and highlights volatility and unsustainability as the main reasons for instability. As for volatility, the author explains that as macroeconomic circumstances alter quickly, predictability becomes thus more and more difficult. From the point of unsustainability, Montiel explains three fundamental reasons; the debt-paying ability of governments, imbalances in the real exchange rates and financial-sector fragility.
Instability and fragility are two terms that may be often used interchangeably. For instance, Bernanke and Gertler (1990) define financial instability or fragility as an instance in which economic actors willing to invest in a project are short of sufficient funds or net wealth, causing them to borrow at a comparatively high rate which implies inefficiency for the economy as a whole. In this definition, it can be said that fragility and instability are evaluated in the context of indebtedness, investment finance and risk-taking behavior. As for risk-taking, Demirgüç-Kunt and Detragiache (1998) relate the risk-taking attitude of financial institutions to financial liberalization and deregulation, which in turn augments the possibility of instabilities.
When the relevant literature on financial fragility is reviewed, we are most likely to be confronted with a number of definitions. As for a start, Davis (1995) defines financial fragility as a condition characterized by “heightened vulnerability to default in a wide variety of circumstances”. In this sense, fragility is associated with elevated susceptibility to fail to meet financial obligations. Minsky (1995) alleges that financial fragility can be primarily traced back to the single microeconomic unit due to the fact that the debt of these individual units constitutes in turn the assets of financial institutions. Minsky concludes that the debt-paying ability of microeconomic units basically determines the degree of financial fragility in the given economy. In this sense, it can be said that Minsky relates financial fragility with indebtedness and ability to service debt. Based on the Minskyan view, the instability is an intrinsic characteristic of the economic system in that in the positive periods of the business cycle, economic actors are more inclined to take additional risks, especially in the form of augmented borrowing. However, in the subsequent downturn period, such vulnerable debtors end up with bankruptcy (Sinapi, 2014). Calomiris (1995) explains that models that examine the relationship to what extent financial institutions, contracting forms and government financial policies have an impact on macroeconomic volatility are occasionally coined as financial fragility models. According to the author, these models determine the causes for the disorders in the financial markets which may emanate either from the financial activities such as financial contracts, the undertakings of intermediaries or even the relevant actions of governments concerning the finance sector or from the production sector. Taylor (1995) puts forward that the understanding of financial fragility evolved in the course of time. The author explains that this term used to express market volatility; however, this association lost its significance as instruments for hedging evolved which in turn increased the endurance against volatility. Goodhart et al. (2006) interpret that fragility occurs when a considerable number of economic actors no longer have the ability to service their debt along with declining profits of lenders, especially the banks. This definition is again relevant to extreme borrowing and riskiness. Montiel (2011) focuses on the fragility in terms of the financial sector which is characterized by low net worth, high-risk exposure and insufficient regulation. Together with fiscal insolvency and exchange rate misalignment, the author considers financial fragility as one of the main reasons for an unsustainable macroeconomic environment, especially in view of emerging and developing countries. Taking the country’s financial system into consideration, Montiel (2011) further defines fragility “as vulnerability to a loss of capital in response to even mild shocks”.
In the light of the explanations above, financial fragility can be viewed as the ability of the nation’s economy to tolerate indigenous and exogenous shocks. It can be thought of as a measure gauging to what degree the economy is susceptible to and resilient against crises. Starting from the 1990s onwards, the measurement of fragility has been a major focus of interest among academicians. Largely, studies of fragility measurement primarily focused on diverse variables or indicators to represent the fragility phenomena as well as various empirical methods to construct composite measures or indexes. On the other hand, it is observed that the first endeavors of fragility measurement were pertaining to the banking sector. Nevertheless, towards the end of the 2000s, fragility related indexes considering primarily macroeconomic indicators were proposed. The supplementary Appendix A to this paper summarizes the development of the literature on fragility measurement in the historical context where the Tables 1 and 2 portray previous studies in terms of the subject and aim of the study, the data scope referring to the countries and years, the empirical methods applied with specific coverage of the banking sector as well as the macroeconomic circumstances, respectively.
Firm performance can be thought of as the degree to what extent the firm is successful or unsuccessful. In this sense, firm success can be evaluated by the attainment of particular pre-determined objectives. These objectives constitute the success or therefore the performance criteria of a firm. Moreover, the realization of business objectives has a time component in line with the going concern principle; operating and remaining in the market continuously. The assessment of whether a firm is performing well implying whether everything is well on track with the preset objectives obviously necessitates a method for measurement. From a quantitative point of view, firm performance can be measured by accounting-based or market-based models (Pozzoli and Paolone, 2017).
Table 2 is a concise comparison of both. As this table suggests, the evaluation of whether a firm is successful or not can be made through the firm-specific financial statement data and market indicators.
The questions that may arise at this point are whether all firm-related parties have common expectations from the firm and there are generally accepted performance criteria for firms. Chief Executive Officers (CEOs) of companies deal with several internal and external parties which are mainly the shareholders, especially when the company is listed (its shares of stocks are traded on stock exchange markets), board of directors, stock exchange analysts, investors, creditors and banks and other stakeholders, each of whom is characterized with differing expectations and criteria for success assessment (Leon, 2016). On the other hand, there is no agreement on generally accepted or uniform firm performance indicators in the literature. Diverse stakeholder groups as well as shareholders are likely to have different expectations from the for-profit company in terms of firm performance; for this reason, performance or success evaluation criteria are to be rather determined within the relevant context (Carton and Hofer, 2007). Therefore, organizational performance has a complex nature in that it has a variety of dimensions and these dimensions are further represented by a high number of indicators. To illustrate, Carton and Hofer (2007) reviewed 1,045 different articles published in five highly esteemed journals from 1996 to 2001. The authors found out that out of the examined articles, 138 of them empirically analyzed organizational performance as the dependent variable, which were represented by 88 diverse indicators.
These indicators were further grouped into nine diverse performance segments based on previous researches of Helfert (1994), Higgins (1995), Brealey et al. (2001) and Penman (2001). The corporate performance dimensions and their brief explanations are summarized in Table 3 below.
The findings of Carton and Hofer’s study revealed that:
● The profitability dimension covered 28% of the total variables in 70% of the total number of articles.
● The operational dimension with 21% of the variables in 18% of total articles.
● The marked-based dimension with 15% of the variables in 17% of total articles.
● The growth dimension with 14% of the variables in 27% of total articles.
● The rest five dimensions (efficiency, liquidity, size, survival and other) included 22% of the variables in total with 26% article coverage.
Referring to previous studies1, Carton and Hofer (2007) conclude that firm performance is a many-sided phenomenon that can be mainly divided into three components, which are the financial performance, operational performance and stakeholder performance, respectively.
1 Drucker (1954), Steers (1975), Cameron (1980), Chakravarthy (1986), Venkatraman and Ramanujam(1986, 1987), Kaplan and Norton (1992), Murphy et al. (1996).
The term firm performance used in this paper implies financial performance. Financial performance can be defined as the degree representing “the change of the financial state of an organization or the financial outcomes that result from management decisions and the execution of those decisions by members of the organization” (Carton and Hofer, 2007). In this sense, of the given performance dimensions in Table 3 above, it can be argued that financial performance is relevant to the profitability, market-based, growth, efficiency, liquidity, size and survival dimensions of overall corporate performance, on the grounds that the components of these dimensions have an impact on the financials of the organization.
Despite the fact that financial performance is a function of a variety of determinants, practically the first and foremost indicator can be stated as profitability. In other words, the success of a company is mainly evaluated based on the extent to which it generates profits, generally for large enterprises and partly for public companies (Kaymaz et al., 2015). Mazumdar (2013) explains that there are two mainstream views concerning the determinants of firm profitability. Represented by Bain(1951, 1956), the first view considers the industry-specific attributes as the primary determinant of firm profitability. In contrast, the second view, put forward by the Chicago School of Economics, emphasizes firm-specific characteristics referring to the efficiency of the firm as the primary factor for firm profitability. Referring to the related former studies2, Mazumdar illuminates that both firm and industry-specific parameters jointly determine firm profitability.
2 Scott and Pascoe (1986), Kessides(1986, 1987), Cubbin and Geroski (1987), McGahan (1999), Amel and Froeb (1991), Rumelt et al. (1991).
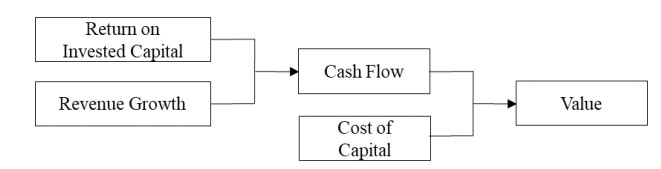
From a value-based perspective, the reason why firms come into existence is to create value for its shareholders; therefore, the assessment of whether a firm is successful or not is measured considering to what extent the wealth of the shareholders changes in a favorable way (Lu, 2009). On the other hand, a key aspect of creating value is the long-term sustainability of generated cash flows. Long-term value creation includes the consideration of the interests of not just the shareholders but the various stakeholders of the company as a whole (Koller et al., 2015). As for how value is created, Koller et al. (2015) argue that firms basically create additional value as long as their return on capital exceeds the cost capital which the authors elaborate through the flow chart depicted below in Figure 1. As illustrated in this figure, the authors explain that owners make a cash investment in the company with the expectation that they receive more cash in the future. In this sense, additional value is expressed as the difference between future cash flows and the amount of capital investment made. In addition, future cash flows may emanate from revenue growth and return on invested capital (Koller et al., 2015).
On the other hand, the earnings of shareholders are twofold; firstly, the increase in the share price which are the capital gains and secondly, the dividend payments, respectively (Koller et al., 2015). In other words, shareholder value is maximized by appreciating share prices and dividend payments. A further remark to be noted here is that the decision whether to make dividend payments depends on the year-end profitability condition of the firm. For this reason, profitability expressed in the form of earnings after taxes can be considered as a parameter for shareholder earnings and their value as well. To sum up, the change or increase in the share price can be regarded as a fundamental financial performance indicator concerning especially public companies along with profitability.
In the course of their operations, firms may experience successful and unsuccessful periods. No doubt, this is an ordinary process as long as the firm operates. Besides, negative or downturn periods are described in a variety of ways depending on their severity. Ratner et al. (2009) describe four distinctive phases that are typical to observe in the life cycle of companies; “the start-up or development phase, the growth phase, the maturity or stabilization phase and the disruption or decline phase”, respectively. Similarly, Pozzoli and Paolone (2017) emphasize that business enterprises have a life cycle and explain the order of consecutive negative phases as “decline, crises, financial distress and bankruptcy”. Altman and Hotchkiss (2006) explain that firms that do not prove to be successful can be classified by four ways, which are failure, insolvency, default and bankruptcy, respectively. Hence, they can be thought of as the incidents that are relevant to corporate distress. Financially distressed firms may indeed avoid going bankrupt by taking the appropriate countermeasures (Ratner et al., 2009). Agostini (2018) points out that financially distressed firms are on the verge of either recovery or failure implying bankruptcy and explains that once distress is recognized, the firm may enter into the recovery path through corrective measures. The author adds that if possible distress situations are detected earlier, the interested parties will be in such an advantageous position to act accordingly to mitigate any negative consequences that are likely to occur. Accordingly, financial distress prediction models can be used as management tools to monitor the financial improvement of a company, especially during times of distress and reorganization (Altman and Hotchkiss, 2006). In the light of the aforementioned explanations, the prediction of bankruptcy can be thought of a risk assessment whether the firm is in an unfavorable phase in its life cycle and if so, prediction modeling may help to understand how severe the situation is and it may guide for taking action plans and restructuring.
The Z-Score approach of Altman is the first multivariate statistical approach to assess the risk of firm failure. It is a measure showing the “financial likelihood of organizational survival” (Carton and Hofer, 2007). Altman criticizes the use of single ratios to evaluate bankruptcy risk due to their comparatively lower statistical significance and evaluates ratio analysis as a comparatively rudimentary method (Altman, 1968). In his model, Altman indeed utilizes a multiple discriminant statistical methodology based on empirical data of selected firms operating in the real sector (Altman, 1968).
The model is illustrated with the equation as follows (Altman, 1968):
where
X1: = Working Capital/Total Assets
X2: = Retained Earnings/Total Assets
X3: = Earnings before Interest and Taxes/Total Assets
X4: = Market Value Equity/Book Value of Total Debt
X5: = Sales/Total Assets
Z = Overall Index
As a compound measure of five diverse ratios, the overall index value is made up of the components reflecting the ability of the company to utilize its assets to meet its short-term obligations (X1), accumulate earnings (X2), generate profits or returns (X3) and turnover (X5) and as well as to finance its growth (X4). In the context of organizational performance dimensions, besides being a survival dimension itself, it can be asserted that the Z-Score encompasses profitability, market-based, liquidity and growth dimensions as well.
According to the calculated Z-Score value, the model classifies firms in three groups, which are the non-bankrupt class (Z > 2.99), the bankrupt class (Z < 1.81) and the gray area (1.81 < Z < 2.99), respectively (Altman, 1968).
When first introduced, it was empirically found out that the model estimated bankruptcy with 95% accuracy with regard to classification in the bankrupt and non-bankrupt classes (Altman, 1968). In the following years from 1969 to 1999, Altman further tested his static model three times and concluded that the initially developed model was still accurate and relevant nearly forty years following its introduction (Altman and Hotchkiss, 2006).
As mentioned above, the Z-Score model made use of the data set relevant to the listed companies operating in the production sector. In 1983, the model was further modified for the companies not traded in the stock exchange in which X4 included the book value of equity instead of the market value, which can be portrayed as follows (Altman, 1983):
where X1: = Working Capital/Total Assets
X2: = Retained Earnings/Total Assets
X3: = Earnings before Interest and Taxes/Total Assets
X4: = Book Value Equity/Total Equity
X5: = Sales/Total Assets
Z' = Overall Index for Private Firms
Similar to the initial model, the private firm Z-Score model classifies the firms again in three groups which are the non-bankrupt class (Z' > 2.90), the bankrupt class (Z' < 1.23) and the gray area (1.23 < Z' < 2.90) now with altered threshold values (Altman, 1983).
The Z-Score approach developed by Altman is a widely accepted model for bankruptcy risk evaluation, which is accessible on Bloomberg across the globe; besides, similar discriminant models are in use in diverse countries (Zhang et al., 2010).
To sum up, company financial performance especially for listed companies can be mainly evaluated based on three main performance dimensions, which are the profitability, market-based and probability of survival performance dimensions, respectively. The selected dimensions refer to the very fundamental objectives of a for-profit organization, which are profit maximization, maximizing the market value, operating continuously and hence, remaining in the business.
2.2. The relationship between financial fragility and firm performance
The relationship between financial fragility and firm performance can be said to be rather an empirical issue without a general theoretical basis in which even cornerstone studies are primarily based on empirical research. Previous studies mainly concentrated on analyzing the relationship between macroeconomic indicators and stock prices as well as between macroeconomic indicators and company profitability.
Studies aimed at investigating the relationship between macroeconomic indicators and stock prices (Bailey and Chung, 1995; Bartov et al., 1995; Durukan, 1999; Karamustafa and Karakaya, 2004; Kyereboah-Coleman and Agyire-Tettey, 2008; Adjasi, 2009; Alam and Uddin, 2009) were mainly based on correlation analyses between exchange rates and stock prices where macro-level indicators were additionally represented by interest rates, money supply and commodity prices as well.
On the other hand, studies that focused on the relationship between macroeconomic indicators and company profitability (Shapiro, 1975; Dumas, 1978; Gao, 2000; Baum et al., 2001; Naceur, 2003; Yurtoglu, 2004; Pasiouras and Kosmidou, 2007; Aydeniz, 2009; Tuncay and Cengiz, 2017) generally employed interest rates, exchange rates, inflation and growth rates. Apart from analyzing as permanent and transitory components, profitability was mainly represented by total return on assets.
3.
Method, data and regression models
The method encompasses analyzing the relationship between financial fragility and firm performance with linear regression models, in which financial fragility will be represented by a selected set of macroeconomic indicators as independent variables and firm performance by the Altman Z-Score, EBT and share price of BIST companies as dependent variables, respectively. In other words, having the firm performance indicators as the dependent variables, three diverse linear regression models will be generated.
Gujarati (2011) explains that linear regression models, in general, make use of three different sorts of data, which can be defined as follows:
● Time Series Data include repetitive time-bounded instances, hence they can be thought of as variables taking values at particular periods such as on daily, weekly, monthly, etc. basis.
● Cross-Sectional Data are the variables observed at a given time, hence they do not possess a variable time component.
● Panel Data carry the characteristics of both time series and cross-sectional data, therefore they can be regarded as the mixture of the former two data types defined above.
Therefore, an empirical study making use of data collected for “same units repeatedly over time is called a panel” (Andreß et al., 2013).
In the light of the definitions above, the empirical data subject to the analysis in this study can be classified as panel data in that the data set possesses both cross-sectional (BIST companies) and times series (annual time dimension, years from 2005 to 2017) characteristics. For this reason, the data at hand combine both the time-based and spatial measurements at the same time (Biørn, 2017).
In an effort to attain the aim of this paper, we will employ two diverse data sets. Firstly, the review of literature on fragility measurement allowed for the conclusion that financial fragility is represented with nine diverse ratios and indexes for Turkey which are listed as follows:
● The real exchange rate of Turkish Lira (TRY).
● BIST (Istanbul Stock Exchange Corporation, Borsa Istanbul)100 index.
● The ratio of short-term foreign debt (STFD) to long-term foreign debt (LTFD).
● The ratio of short-term foreign debt (STFD) to international reserves.
● The ratio of current account deficit to gross domestic product (GDP).
● The ratio of budget deficit to gross domestic product (GDP).
● The ratio of net public debt to gross domestic product (GDP).
● The ratio of exports to imports.
● The ratio of private sector foreign debt (PSFD) to gross domestic product (GDP).
The real exchange rate refers to the value of a local currency (TRY) in terms of foreign currency. Hence, an increase in the real exchange rate of TRY implies the appreciation of TRY against the foreign currency basket. BIST 100 is the key stock index for equities traded in Turkey which is aimed at measuring the performance of selected 100 stocks traded in BIST. The ratio of STFD to LTFD can be thought of as a measure to what extent an economy is able to finance or re-finance its short-term foreign debt with respect to that of the long term. International reserves can be regarded as a source for servicing foreign debt. Ratios having the GDP as the denominator, in general, can be thought of as a measure of a given variable in terms of national income. The current account is the difference between exports of goods/services and imports of goods/services additionally encompassing the net unilateral transfers of income. Budget deficit, in broader meaning the budget deficit of central governments, is the unfavorable difference between government revenues and expenditures. Public debt is the total value of all budget deficits and surpluses of governments accumulated in the previous periods. The ratio of the value of exports to imports for a country can be thought of as a measure for the country’s trade balance, which implies to what extent a country is able to cover its imports with its exports. Private Sector Gross Foreign Debt shows the external indebtedness of the private sector.
This data set is derived from three main sources which are the Central Bank of the Republic of Turkey, General Directorate of Budget and Fiscal Control and the Ministry of Treasury of the Republic of Turkey covering a thirteen-year-time-period from 2005 to 2017. Appendix B supplementary to this paper presents a detailed overview of the data source for each relevant indicator. Besides, the subsequent tables in this appendix portray the base data of the macroeconomic variables used in this study.
The macro-level variables to represent financial fragility is largely an extension of the study of Çakmak (2013). In his research, Çakmak used eight macroeconomic indicators which were the ratio of the current account balance to gross national product (GNP), the real exchange rate index, exports to imports ratio, the ratio of STFD to reserves, consolidated budget balance to GDP ratio, annual increase rate of BIST 100 Index, the ratio of STFD to LTFD and net public debt stock to GDP ratio and incorporated these variables into a financial fragility index covering a twenty-three-year-time period from 1989 to 2011. In our analysis an additional indicator, namely the Gross PSFD/GDP used in the index due to the fact that the composition of foreign indebtedness shifted considerably from public to private sector in recent years in Turkey. As for another difference,
Çakmak used the GNP as the denominator in the current account ratio instead of the GDP, which the author applied in the budget and public debt ratios. In our study, the GDP was the denominator in each relevant case.
Secondly, in the light of the literature review on firm performance, selected parameters are the earnings before tax (EBT) for profitability; the share price for the market-based profitability dimension; and the Altman Z-Score for probability of survival. The reason why EBT can be regarded as a proxy for profitability is that it encompasses the operating as well as the non-operating performance of a firm. Since firms may have different tax bases even in the same country (due to the possibility of deduction of previous years’ losses, research and development incentives, export incentives, other tax regulation-based deductions etc.) the net result before taxes can be picked up to present better comparability among firms. The share price can be selected as a proxy for the market-based firm performance dimension due to the fact that it is a measure for firm value and its appreciation indicates an increase in the shareholder value as well. The Altman Z-Score implies the level of financial distress representing the risk of bankruptcy. This indicator presents the degree to which a firm is close to failure, hence offering the possibility of an overall performance and risk assessment.
This second data set on the other hand consists of the financial statements of listed companies, which are derived from the Borsa Istanbul Historic and Reference Data Platform (Datastore) for the years from 2005 to 2008 and Public Disclosure Platform (PDP) for the years from 2009 to 2017. The financial statements include the balance sheet and the income statement of a given company. Whereas the total number of accessible observations in the given thirteen-year period was 5,325, the number of observations integrated in the data set was 4,193. There are two main reasons why several observations were excluded from the analysis. Firstly, in an effort to provide comparability, the BIST companies operating in particular sectors were removed from the analysis due to the fact that the structure of their balance sheets and income statements differ from that of the rest. The excluded sectors are mainly related to the companies operating in the finance-related business; banks, insurance, factoring, asset management, rent certificates and other finance companies. Secondly, companies with a special fiscal period other than the calendar year were also excluded from the data set due to consistency reasons. On the other hand, the Altman Z-Score values of the BIST companies that are subject to analysis are calculated based on the initial Z-Score model of Altman, which is relevant to listed companies in the manufacturing sector. The model is depicted as follows:
Compared to the original model (Altman, 1968), the first four coefficients are rephrased and the latter is rounded to “1.0” for convenience reasons in which the cutoff points of 1.81 and 2.99 are kept unchanged (Altman, 2000). In addition, while calculating the X4, the book value of equity is used which is principally the difference between total assets and total liabilities instead of the market value of equity due to simplicity and data accessibility reasons.
The data set at hand can be classified as an unbalanced short panel, since each single BIST firm does not have an observed value in all given years (unbalanced), and the number of cross-sectional instances exceeds the number of observation years (short) (Gujarati, 2011). The reason why the data set is an unbalanced panel is that new firms may have been listed on BIST or may have ceased operations, gone bankrupt or failed to fulfill the requirements of related governmental authorities, hence become unlisted.
The linear regression approach to be employed in this paper can be indeed better defined as a panel data regression model in which the regression model will have two dimensions, which are specific to cross-sectional units such as the firm and time-based units such as the calendar year, respectively.
Biørn (2017) illuminates that the intercepts and coefficients in the panel data regression model will vary between the given cross-sectional as well as time-driven units. The author defines these variations as individual-specific and/or time-specific heterogeneity and points out that these heterogeneities can be represented with either unknown fixed parameters or random variables with specific properties (Biørn, 2017).
Accordingly, panel data regression models can be divided into two main types, which are the fixed effects models and random effects models, respectively. The basic difference between a fixed- effects and a random-effects model is that in fixed-effects models, each single cross-sectional unit has its own intercept value; whereas in random-effects models, these values are arbitrary selections from a bigger population of cross-sectional units (Gujarati, 2011).
Pesaran (2015) formulates the general panel data regression model as follows:
In this equation, the author clarifies that i refers to the cross-sectional units such as the firm, t stands for the time component such as the calendar year, xit is the independent variable observed for the ith individual unit for the given tth time unit, uit is the error term, β represents unknown parameters and finally αi is the time-invariant unit-specific effect (Pesaran, 2015).
In addition, the fixed effects model can be formulated as follows (Verbeek, 2017):
In fixed-effects models, “the unobserved effects are allowed to be arbitrarily correlated with the explanatory variables in each time period” (Wooldridge, 2016). Here, each single cross-sectional unit has its individual intercept value (Gujarati, 2011).
Secondly, below is the mathematical expression of the random effects model (Verbeek, 2017):
In random-effects models, “the unobserved effect is assumed to be uncorrelated with the explanatory variables in each time period” (Wooldridge, 2016). In these models, intercept values are arbitrary selections from larger cross-sectional units (Gujarati, 2011).
When the aforementioned two equations for fixed effects and random effects models are compared, it can be stated that the αi representing “the effects of omitted individual-specific variables” denotes fixed measurements over time in fixed-effects models; in contrast the αi stands for random measurements similar to uit in random-effects models (Hsiao, 2014).
In panel data regression models, a decision is to be made whether to construct the model with fixed or random effects, hence with fixed or random αi values (Hsiao, 2014). In this context, the choice between the application of a fixed and a random-effects model can be made with the Hausman test. Basically, the Hausman test evaluates whether the fixed effects and random effects estimators significantly differ from each other (Verbeek, 2017).
The Hausman test can be formulated as follows (Biørn, 2017):
H0:λ=0 (Random effects are acceptable)
H1:λ≠0 (Fixed effects are acceptable)
Based on the hypotheses expressed above, the rejection of the Hausman test implies that the panel data regression model is to be constructed based on the fixed effects model (Wooldridge, 2016).
The panel regression models to be employed in the assessment of the impact of financial fragility on firm performance are listed below. In addition, Table 4 following the models depicts the full description of each variable included in the equations.
As it may be inferred from the equations presented above, the independent variables in the three models are common, which are the selected nine different annual macroeconomic indicators. Having identical independent variables, each model includes the Altman Z-Scores, EBT values and share prices of BIST companies as dependent variables. In other words, identical nine macroeconomic indicators are regressed onto Altman Z-Scores, EBT values and share prices of BIST companies for the period from 2005 to 2017.
Whereas the dependent variables include both firm-specific (cross-sectional) and time series (years) components, the independent variables can be thought of as time-series data, since annual macroeconomic indicators are identical for each firm in the given years.
4.
Empirical results
The presentation of the empirical results in this section will start with the illustration of the descriptive statistics and the correlation matrix of the variables. Secondly, the choice between the fixed-effects or random-effects models will be explained. As for the third step, the results of the panel regressions based on the selected model type will be elaborated.
The descriptive statistics of the variables used in the models are presented in Table 5 on the next page. In addition to the descriptive statistics, Table 6 illustrates the correlation matrix of the variables. A closer examination of the correlation values between the independent variables reveals that the following variables are strongly correlated with each other, where the correlation coefficient is either greater than +0.80 or less than −0.80:
● RealER and PFD_GDP (−0.83)
● ST_LT and ST_Res (+0.89)
● ST_Res and PD_GDP (−0.85)
● CA_GDP and Ex_Im (−0.89)
● PD_GDP and PFD_GDP (−0.88)
As formerly explained in detail, panel data regression models can be fixed-effects or random-effects models depending on the treatment of omitted individual-specific variables. Since the independent variables in the models are composed of annual macroeconomic indicators, which are identical across all firms in each given year, the intuitive expectation for the model type can be said to be the fixed effects model. In order to verify this expectation, Hausman tests were run for each model. The common outcome of the Hausman tests was that the cross-section test variance was invalid which set the Hausman statistic to zero. This was interpreted in the way that the estimate of the random effects variance was zero leading to the conclusion that no random effects were visible. As for an additional verification step, the redundant fixed effects were evaluated by the likelihood ratio. When the tests were run, the probability value was below 0.05 for each model. Based on the outcomes of the Hausman and redundant fixed effects tests, the decision was to go on with the fixed effects models in the panel regression analysis.
While forming the panel regression models, variables were added and removed based on the findings of the correlation values presented in Table 6. Testing diverse models with the addition and removal of correlated independent variables did not lead to significant differences; for this reason, the decision was to include all dependent variables in the final models.
Preceding to the examination of the panel regression results, we will firstly focus on the expected direction of the relationship between the independent and dependent variables in the panel regression models. Accordingly, Table 7 illustrates the expected direction of the association between financial fragility and its proxy variable as well as its implication with firm performance.
As depicted in Table 7, an increase in the real exchange rate (appreciation of TRY) is assumed to have a positive relationship with financial fragility. In other words, the overvaluation of TRY is associated with a macroeconomic imbalance, hence increasing financial fragility. The debt-related ratios with the national income or reserves in the denominator which are the STFD to international reserves, net public debt to GDP and PSFD (Gross) to GDP are supposed to have a positive association with financial fragility, since increasing debt stocks are considered as a macroeconomic risk. The same relationship is assumed to exist between STFD/LTFD and fragility, since it can be concluded that the closer the due date is, the higher the risk of default is. Similarly, it is presumed that the current account and budget deficits in terms of GDP are positively related to financial fragility. Again, increasing deficits are regarded as increased riskiness for the economy. Moreover, increases in the value of the BIST 100 index and exports to imports ratio are assumed to have a negative association with financial fragility. Higher BIST 100 index values imply an increase in the value of the listed companies in Turkey, which may be coupled with increasing corporate profits, hence reduced fragility. In addition, a rise in the exports to imports ratio indicates an improvement in the trade balance, which is supposed to decrease fragility. On the other hand, it will be convenient to assume a negative relationship between financial fragility and firm performance; namely the Altman Z-Score, EBT and share price. To conclude, the coefficients of the change in the BIST 100 index and the ratio of exports to imports are expected to have a positive sign in the equations; in contrast, the rest is expected to have a negative sign.
Table 8 summarizes the results of the first panel regression model, in which the Altman Z-Score of BIST companies according to year-end financial statements is the dependent variable. The results suggest that of the selected variables, the coefficients of the real exchange rate, the change in the BIST 100 index, the ratio of STFD to international reserves, the ratio of current account deficit to GDP, the ratio of net public debt to GDP and the ratio of exports to imports are significant at the 0.1 level. In addition, the coefficients of the change in the BIST 100 index and the ratio of STFD to LTFD are positive; whereas that of the rest is negative. When the coefficient signs are compared with the expectations set out in Table 7 presented previously, it can be concluded that all coefficient signs excluding the ratio of STFD to LTFD and the ratio of exports to imports are in line with what is expected. As the R-squared suggests, the independent variables explain 58.6% of the variability in Altman Z-Score. The Durbin-Watson statistic shows no signs of autocorrelation and the model as a whole is significant at the 0.01 level.
In an effort to analyze further, the model was then modified by replacing the real exchange rate value of the current year with that of the previous year. In other words, the panel regression model covered in this case the period from 2006 to 2017, in which the real exchange rate values had a one-year time lag as they started from 2005 and ended in 2016 instead of 2017. The lagging variable of the real exchange rate is denoted by RealER(−1) in the model. The findings are depicted in Table 9 where the inclusion of the real exchange rate with one-year time lag reduced the total number of observations from 4,193 to 3,701, since the observations for one year were sacrificed in the data set. On the other hand, the comparison of the two models reveals that the R-squared rose from 58.6% to 60.9% with a 2.3% improvement. As for the change in the significance of regressors, the ratio of STFD to international reserves, the ratio of current account deficit to GDP and the ratio of exports to imports are significant in both models at the 0.1 level. Conversely, the coefficients of the real exchange rate, the change in the BIST 100 index and the ratio of net public debt to GDP became insignificant. Moreover, the ratio of budget deficit to GDP and the ratio of STFD to LTFD turned into significant in the modified model. The ratio of private-sector gross foreign debt to GDP remains insignificant in both models at the 0.1 level. Another finding of the comparison is that the coefficient of private-sector gross foreign debt to GDP ratio changed from (−) to (+) in the modified model, which in this case contradicts with the expectation.
The findings of the second panel regression model are revealed in Table 10. In this model, the year-end EBT values of the BIST companies are the dependent variables. According to Table 10, the coefficients of the change in the BIST 100 index, the ratio of STFD to international reserves, the ratio of budget deficit to GDP and the ratio of private-sector gross foreign debt to GDP are significant at the 0.1 level. Moreover, all coefficients of the independent variables except for the ratio of budget deficit to GDP are positive. With this respect, it can be stated that only the change in the BIST 100 index, the ratio of budget deficit to GDP as well as the ratio of exports to imports meet the expectations as for the direction of association with the EBT. The R-squared portrays that the independent variables explain 74.5% of the variability in EBT. The Durbin-Watson statistic shows no signs of autocorrelation and the model as a whole is significant at the 0.01 level.
The model was further modified by replacing the RealER with RealER(−1) in which the outcome is presented in Table 11 below.
When the models presented in Tables 10 and 11 are compared, it can be derived that the modification of RealER(−1) led to the change of R-squared from 74.5% to 76.7% corresponding to an improvement of 2.2%. Leaving the ratio of private-sector gross foreign debt to GDP aside, the rest of the regressors became insignificant at the 0.1 level in the transformed model.
On the other hand, the aforementioned transformation had a major impact on the signs of the coefficients of the independent variables in that the coefficient signs of the real exchange rate, the ratio of STFD to international reserves, the ratio of budget deficit to GDP as well as the ratio of exports to imports changed the other way around. With this change, the real exchange rate and the ratio of STFD to international reserves seem to move in the expected direction with EBT; whereas the ratios of exports to imports and budget deficit to GDP not.
What follows next is Table 12 in which the outcomes of the third panel regression model are disclosed. In this model, the logarithm of the year-end share price of the BIST companies is the dependent variable.
According to Table 12, the coefficients of the change in the BIST 100 index, the ratio of STFD to LTFD, the ratio of STFD to international reserves and the ratio of budget deficit to GDP are significant at the 0.1 level. As for the signs of the coefficients of the independent variables, the real exchange rate, the change in the BIST 100 index and the ratio of STFD to international reserves are positive and the rest is negative. The coefficient signs lead to the conclusion that the real exchange rate, the ratio of STFD to international reserves and the ratio of exports to imports do not correspond to the expected direction of relationship with the share price. The R-squared shows that the independent variables explain 83.9% of the variability in the share price. The Durbin-Watson statistic displays no signs of autocorrelation and the model as a whole is significant at the 0.01 level.
Table 13 shows the results with the RealER(−1) replacement in the third model. As summarized in this figure, the switch of REALER with REALER(−1) again improved the significance of the total model from 83.9% to 85.8% by 1.9%. An examination of the change in the significance of regressors reveals that the real exchange rate, the ratio of current account deficit to GDP, the ratio of exports to imports and the ratio of private-sector gross foreign debt to GDP became significant at 0.1 level with the RealER(−1) modification. Conversely, the ratio of budget deficit to GDP turned out to be insignificant with the modification. The ratio of net public debt to GDP is still insignificant in both cases. Additionally, the replacement of REALER(−1) caused the signs of the real exchange rate and the ratio of net public debt to GDP to change the other way around. With this change, the real exchange rate is now associated with the share price in the expected direction; in contrast the ratio of net public debt to GDP contradicts the expected direction of the association.
In an effort to enhance the robustness of the models, panel cross-sectional dependence tests were conducted. In this context, four diverse cross-section dependence tests were performed, which were Breusch-Pagan LM, Pesaran scaled LM, Bias-corrected scaled LM and Pesaran CD, respectively. Test results suggested no signs that the disturbances in the panel data models were cross-sectionally dependent.
The empirical results derived from the six different panel regression equations can be basically interpreted in terms of the significance of the regressors as well as the signs of the coefficients. Tables 14 and 15 make a comparison of the models based on these two criteria; the tables portray whether the regressors are significant at the 0.1 level (represented by “Yes” or “No”) and whether the signs of the coefficients meet the expected direction of association (denoted as “Exp.” or “Unexp.” meaning expected or unexpected) as outlined in Table 7
As Table 14 disseminates, the real exchange rate, the change in the BIST 100 index, the ratios of STFD to international reserves, current account deficit to GDP and net public debt to GDP are significant independent variables in the first model in which their association with the Altman Z-Score is in the expected direction. In the second model, the change in the BIST 100 index and the ratio of budget deficit to GDP are the two regressors which are both significant and associated expectedly with the EBT. As for the third model, there are three independent variables that are both significant and moving in the expected direction with the share price which is the change in the BIST 100 index, the ratio of STFD to LTFD and the ratio of budget deficit to GDP, respectively.
Table 15 makes the same comparison this time with the models in which the regressor RealER is substituted with the RealER(−1). When the real exchange rate is included with a one-year time lag, the ratios of STFD to international reserves, current account deficit to GDP and budget deficit to GDP are the significant independent variables observed to be in the expected way of association with the Altman Z-Score. Secondly, it can be concluded that the modification is irrelevant for the second model in that no regressor in the modified second model is significant and showing the expected direction of association with the EBT. With regard to the third panel regression model, the number of the variables realizing both of the criteria rises from three to five with the addition of the real exchange rate, the ratio of current account deficit to GDP and the ratio of private-sector gross foreign debt to GDP, where the ratio of budget deficit to GDP is no more eligible in contrast to the unmodified model.
5.
Conclusion
Literally speaking, this paper constructed a bridge between financial fragility and firm performance. The focal point was to provide insights into the question to what extent the financial performance of business enterprises is affected by macroeconomic conditions. Answers to this question were sought by utilizing annual financial reporting figures of 492 diverse companies listed on Borsa Istanbul as well as nine macroeconomic indicators in Turkey for a thirteen-year period from 2005 to 2017 in Turkey. The empirical analysis included the use of panel data regression models formulated separately for diverse firm performance indicators, namely the Altman Z-Score, EBT and share price, respectively. Accordingly, macroeconomic indicators were regressed on three different firm performance variables. Empirical findings primarily suggested that a significant relationship existed between financial fragility and firm performance based on selected representative parameters. In this context, the real exchange rate, the change in the BIST 100 index, the ratios of STFD to international reserves, current account deficit to GDP and net public debt to GDP were regarded as the key determinants of Altman Z-Score. In addition, the change in the BIST 100 index and the budget deficit to GDP ratio were proposed to be the most significant factors for EBT. On the other hand, the change in the BIST 100 index, the ratio of STFD to LTFD as well as the budget deficit to GDP ratio were posited to be the most influential elements for share price development.
Several conclusions can be drawn in the light of the findings of the empirical analysis. Firstly, it can be asserted the real exchange rate can be regarded as an influential indicator for firm performance in terms of share price development for the coming period. In other words, a decrease in the real exchange rate meaning depreciation of TRY in terms of foreign currencies in real terms is likely to signal for an increase in share prices for the coming year. One explanation that can be proposed is that depreciated or undervalued TRY may also mean undervalued share prices in the stock exchange market which may attract the attention of especially foreign investors leading to an increase in share prices due to increased demand. On the other hand, from the investors’ point of view, the fluctuations in the real exchange rate in one period can signal for associated changes in the share prices in the subsequent period. By following up on the fluctuations in the real exchange rate, equity investors may make their investment decisions.
Secondly, being eligible for each of the three dependent variables based on the given two criteria which are the significance of the regressors and the signs of the coefficients, the change in the BIST 100 index can be concluded as an influential indicator for firm performance. An improvement in the BIST 100 index is therefore highly likely to affect the Altman Z-Score, EBT and share price of firms in a positive way. Yet another influential indicator is the ratio of budget deficit to GDP, which fulfills the evaluation criteria for the EBT and share price. In this sense, a deteriorating budget deficit to GDP ratio is likely to signal for worsening firm performance in terms of EBT and share price. In this sense, the BIST 100 index and the budget deficit to GDP ratio can be regarded as the two key macro variables having a significant impact on company financial performance. In addition to the internal key performance indicators based on own company data, company managers may focus on the BIST 100 index and the budget deficit to GDP ratio as the primary two external indicators to assess company performance. On the other hand, actions undertaken by macroeconomic policymakers to reduce budget deficits are likely to have a positive impact on the financial performance of companies.
As for a third implication, it can be posited that the riskiness of a firm to go bankrupt represented by the Altman Z-Score can be estimated by investigating especially the development of five main macroeconomic indicators which are the real exchange rate, the change in the BIST 100 index, the ratios of STFD to international reserves, current account deficit to GDP and net public debt to GDP, respectively. Out of numerous macro-level indicators, managers may investigate these five indicators to assess the bankruptcy risk of their companies along with their own company financials.
Fourthly, the change in the BIST 100 index and the budget deficit to GDP ratio are found to be the most significant parameters that can be monitored to estimate the EBT development of firms. Similarly, company managers may enrich their profitability related internal performance indicators with these two external macro-level variables additionally.
What can be mentioned as the last conclusion is that the share price of companies is likely to be most affected by the current developments in the change in the BIST 100 index, STFD to LTFD ratio as well as the budget deficit to GDP ratio. These three external indicators can be thought of as early warning parameters that may signal for fluctuations in the share price of the companies which may be monitored as a small set of macro-level indicators.
To conclude, being aware of which macroeconomic indicator has the most significant impact on profitability, bankruptcy risk or share price value, company managers may determine a set of economic variables to be primarily followed-up and take relevant measures according to their development. On the macroeconomics side, policymakers may formulate to the point actions to flourish company growth and performance.
There are several restrictions that should be taken into consideration while reviewing the findings of the empirical analysis. Firstly, in addition to the macroeconomic environment, many factors at the firm level may affect firm performance such as firm innovation capabilities, CEO duality, etc. Since the paper primarily focused on macroeconomic factors, micro-level variables were not included in the study. Secondly, the data set of BIST companies excluded those that were mainly operating in the finance sector due to the fact that their financial statements were constructed in such a way that firm performance-related indicators could not be extracted properly. Thirdly, the company data set contained a different number of companies each year leading to an unbalanced panel, since the number of companies being listed or unlisted altered in different years. Lastly, the proposed models included a pre-selected set of parameters that represented firm performance and financial fragility. In other words, the choice on the variables as for proxy factors for firm performance and financial fragility is likely to affect the significance of the relationship between these two.
The restrictions stated above can be on the other hand regarded as implications for future research. Future research in this area may be primarily directed at incorporating micro-level factors at the firm level as for control variables. Moreover, employing an extended set of variables that may represent financial fragility and firm performance is yet another area for future research. Such an effort may provide additional insights for understanding the impact of macroeconomic policies on the future of business enterprises. Furthermore, the impact of macroeconomic conditions on firms may be evaluated firm by firm or a group of firms in the same industry or belonging to the same group. These future endeavors may guide company managers concerning strategy formulation for future business success.
Acknowledgments
This manuscript benefited from the valuable comments of two anonymous reviewers.
Conflict of interest
The author declares no conflicts of interest in this paper.









 DownLoad:
DownLoad: