
Chikungunya is a vector-borne viral disease transmitted by Aedes aegypti and Aedes albopictus mosquitoes. It does not have any specific treatment, and there is no vaccine. Recent epidemiological data have indicated that a relapse of the infection can occur within three months of the initial infection; however, until now, mathematical models for the spread of the disease have not considered this factor. We propose a mathematical model for the transmission of the Chikungunya virus that considers relapse. We calculated the basic reproductive number (R0) of the disease by using the next-generation operator method. We proved the existence of a forward bifurcation. We determined the existence and the global stability of the equilibrium points by using the Lyapunov function method. We fitted the model to data from an outbreak in 2015 in Acapulco, Mexico to estimate the model parameters and R0 with the Bayesian approach via a Hamiltonian Monte Carlo method. In the local sensitivity analysis, we found that the fraction of infected individuals who become asymptomatic has a strong impact on the basic reproductive number and makes some control measures insufficient. The impact of the fraction of infected individuals who become asymptomatic should be considered in Chikungunya control strategies.
Citation: María Guadalupe Vázquez-Peña, Cruz Vargas-De-León, Jorge Fernando Camacho-Pérez, Jorge Velázquez-Castro. Analysis and Bayesian estimation of a model for Chikungunya dynamics with relapse: An outbreak in Acapulco, Mexico[J]. Mathematical Biosciences and Engineering, 2023, 20(10): 18123-18145. doi: 10.3934/mbe.2023805
| [1] | Yves Dumont, Frederic Chiroleu . Vector control for the Chikungunya disease. Mathematical Biosciences and Engineering, 2010, 7(2): 313-345. doi: 10.3934/mbe.2010.7.313 |
| [2] | Fernando Saldaña, Hugo Flores-Arguedas, José Ariel Camacho-Gutiérrez, Ignacio Barradas . Modeling the transmission dynamics and the impact of the control interventions for the COVID-19 epidemic outbreak. Mathematical Biosciences and Engineering, 2020, 17(4): 4165-4183. doi: 10.3934/mbe.2020231 |
| [3] | Yonghua Zhuang, Kristen Wade, Laura M. Saba, Katerina Kechris . Development of a tissue augmented Bayesian model for expression quantitative trait loci analysis. Mathematical Biosciences and Engineering, 2020, 17(1): 122-143. doi: 10.3934/mbe.2020007 |
| [4] | Fabio Sanchez, Luis A. Barboza, Paola Vásquez . Parameter estimates of the 2016-2017 Zika outbreak in Costa Rica: An Approximate Bayesian Computation (ABC) approach. Mathematical Biosciences and Engineering, 2019, 16(4): 2738-2755. doi: 10.3934/mbe.2019136 |
| [5] | Phongchai Jittamai, Natdanai Chanlawong, Wanyok Atisattapong, Wanwarat Anlamlert, Natthiya Buensanteai . Reproduction number and sensitivity analysis of cassava mosaic disease spread for policy design. Mathematical Biosciences and Engineering, 2021, 18(5): 5069-5093. doi: 10.3934/mbe.2021258 |
| [6] | Zimeng Lv, Xinyu Liu, Yuting Ding . Dynamic behavior analysis of an $ SVIR $ epidemic model with two time delays associated with the COVID-19 booster vaccination time. Mathematical Biosciences and Engineering, 2023, 20(4): 6030-6061. doi: 10.3934/mbe.2023261 |
| [7] | Yuncheng He, Zhen Liu, Zhi Li, Jiurong Wu, Jiyang Fu . Modal identification of a high-rise building subjected to a landfall typhoon via both deterministic and Bayesian methods. Mathematical Biosciences and Engineering, 2019, 16(6): 7155-7176. doi: 10.3934/mbe.2019359 |
| [8] | Salihu Sabiu Musa, Shi Zhao, Hei-Shen Chan, Zhen Jin, Daihai He . A mathematical model to study the 2014–2015 large-scale dengue epidemics in Kaohsiung and Tainan cities in Taiwan, China. Mathematical Biosciences and Engineering, 2019, 16(5): 3841-3863. doi: 10.3934/mbe.2019190 |
| [9] | Xiaochen Sheng, Weili Xiong . Soft sensor design based on phase partition ensemble of LSSVR models for nonlinear batch processes. Mathematical Biosciences and Engineering, 2020, 17(2): 1901-1921. doi: 10.3934/mbe.2020100 |
| [10] | Hamdy M. Youssef, Najat A. Alghamdi, Magdy A. Ezzat, Alaa A. El-Bary, Ahmed M. Shawky . A new dynamical modeling SEIR with global analysis applied to the real data of spreading COVID-19 in Saudi Arabia. Mathematical Biosciences and Engineering, 2020, 17(6): 7018-7044. doi: 10.3934/mbe.2020362 |
Chikungunya is a vector-borne viral disease transmitted by Aedes aegypti and Aedes albopictus mosquitoes. It does not have any specific treatment, and there is no vaccine. Recent epidemiological data have indicated that a relapse of the infection can occur within three months of the initial infection; however, until now, mathematical models for the spread of the disease have not considered this factor. We propose a mathematical model for the transmission of the Chikungunya virus that considers relapse. We calculated the basic reproductive number (R0) of the disease by using the next-generation operator method. We proved the existence of a forward bifurcation. We determined the existence and the global stability of the equilibrium points by using the Lyapunov function method. We fitted the model to data from an outbreak in 2015 in Acapulco, Mexico to estimate the model parameters and R0 with the Bayesian approach via a Hamiltonian Monte Carlo method. In the local sensitivity analysis, we found that the fraction of infected individuals who become asymptomatic has a strong impact on the basic reproductive number and makes some control measures insufficient. The impact of the fraction of infected individuals who become asymptomatic should be considered in Chikungunya control strategies.
Chikungunya fever is a viral disease caused by an arboviral alphavirus transmitted to humans by Aedes mosquitoes, mainly Aedes aegypti and Aedes albopictus mosquitoes. These vectors are widely distributed in the Americas. In 1952, this virus was first isolated and described in humans in Tanzania; it was identified in the Americas in 2013, and in Mexico one year later [1].
Once an infected mosquito bites a person, the disease has an incubation period between 3 and 7 days. The symptoms are severe joint pain (arthralgia) and high fever (above 39℃); they also include occasional nausea, myalgia (muscle pain), vomiting and rash [2]. This joint pain is usually debilitating, affecting the quality of life of the patient, but the persistence of the symptoms has not been thoroughly studied.
In 2008, Dumont et al. proposed a host-vector model that considers the aquatic phase of the mosquito for Chikungunya disease; they also studied the local and global stability of the equilibrium points, fitted the model to epidemiological data from four cities in France and estimated the basic reproductive numbers of the outbreak [3]. Subsequently, Dumont et al developed a model that incorporates a combination of the early use of massive fumigation and mechanical control (such as the destruction of breeding sites) and concluded that it can be effective in stopping or containing the spread of Chikungunya infections with minimal environmental impact [4]. In 2012, Ruiz-Moreno et al. developed a climate-based stochastic model of mosquito population dynamics with an epidemiological model to identify temporal windows that carry epidemic risk they found that, in places with a marked seasonal variation in temperature, there was also an epidemic risk season that coincided with the period of the year in which mosquito populations survive and grow [5]. In 2019, González-Parra et al. analyzed an SEIRC-SEI model with intrinsic and extrinsic incubation periods for the dynamics of the transmission of Chikungunya that considers a constant human population and vectors. They studied the global stability of the disease-free and endemic equilibriums by using the second method of Lyapunov and they used bootstrapping and Markov chain Monte Carlo techniques to estimate some model parameters and the basic reproductive number for an outbreak in Colombia [6]. Abboubakar et al. included density-dependent rates and some control mechanisms in existing Chikungunya models. Their model presents a backward bifurcation, and the least squares method was used to estimate the basic reproductive number of an outbreak that occurred in Chad and Cameroon [7].
The phenomenon of relapse has been reported for Chikungunya infections [8,9,10,11,12]. Relapse is defined as the reappearance of arthralgia due to virus' persistence in the cells of the musculoskeletal tissue after a symptom-free period of at least one week [8], or after one month [12]. A cohort study based on data from a laboratory-based surveillance system in France was performed; the initial infection was confirmed via an antibody or polymerase chain reaction (PCR) testing. In this study, relapses of arthralgia were reported in 72% of patients; the mean number of relapses was four and the mean time between two relapses was 8 weeks [8]. On the other hand, a cross-sectional study of Acapulco in southern Mexico in December 2015 was performed; 66% of the population (3531 out of 5870 people) self-reported that they had been infected; 31.1% of those who suffered from Chikungunya (1098 out of 3531 people) reported at least one relapse at least 1 month after they recovered from the disease, 13% reported exactly one relapse, 12% reported two relapses, 4% reported three relapses and just 2% reported more than four relapses [12].
The relapse phenomenon has not been incorporated into the host-vector models for Chikungunya infections in the mathematical epidemiology literature. For this reason, the objectives of our study were to incorporate relapses into the standard model for Chikungunya, and to study the effect of the relapse rate on Chikungunya outbreaks. Finally, using the data collected in [12] that consisted of self-reported Chikungunya cases per month in Acapulco, Mexico, we have estimated several model parameters and the basic reproductive number of the infection.
In the present paper we address a Chikungunya model with relapse. We have made the following contributions:
● We extended the standard host-vector model of Chikungunya to incorporate the relapse phenomenon.
● The global stability of the disease-free equilibrium and the endemic equilibrium has been analyzed using the method of Lyapunov functions.
● We performed Bayesian estimates of the model parameters and the basic reproductive number of a Chikungunya outbreak in Acapulco, Mexico.
● Local sensitivity analysis was performed to measure the relative change of basic reproductive number for each parameter.
This paper is organized as follows: In Section 2, we will propose a Chikungunya model with relapse. In Section 3, we will compute the equilibria and basic reproductive number, and we will analyze the bifurcation that occurs at the disease-free equilibrium point as well as the global stability of the disease-free and endemic equilibrium points. In Section 4, we will fit the model to data from an outbreak in 2015 in Acapulco, Mexico to estimate model parameters and the basic reproductive number (R0) with the Bayesian approach by using the Hamiltonian Monte Carlo method. In Section 5, we will present the results of a local sensitivity analysis to describe the impact of the parameters on the R0 value. Finally, Section 6 contains the concluding remarks.
Let Nh and Nv be the total number of humans (hosts) and the total number of mosquitoes (vectors), respectively. Both populations are divided into mutually exclusive compartments that are dependent on each individual's epidemiological state. Humans are classified into four compartments:
1. Susceptible humans (Sh): We assume that all humans are born susceptible to the virus; then, the population in this compartment increases according to a constant rate μh and decreases due to natural death at the same rate. Additionally, the size of the susceptible population decreases when a susceptible human becomes an infected human after an infected mosquito bite (βhbNhShIv); thus, the number of new infections depends on the number of susceptible humans who are bitten by a mosquito per time unit b and the probability of an effective transmission from a vector to a human βh. Consequently, the dynamics of the compartment of susceptible humans are given by the following equation:
| S′h=μhNh−βhbNhShIv−μhSh. |
2. Infected humans (Ih): The number of infected humans increases when a mosquito bite effectively transmits the virus to a susceptible human according to the mechanism described above, and also when symptoms return after a symptom-free period, that is, the relapse period 1/δh. The number of individuals in this compartment decreases when the symptomatology disappears (recovery rate γ) and due to natural death (μh). The number of infected humans is determined by the following equation:
| I′h=βhbNhShIv−(μh+γ)Ih+δhAh. |
3. Asymptomatic humans (Ah): A fraction p of infected individuals are assumed to undergo an asymptomatic period since a significant percentage of patients have relapsed after the initial infection. The number of asymptomatic humans increases at a rate pγ and decreases due to natural death (μh) and when symptoms occur again at a rate δh. The reappearance of symptoms is due to the persistence of the virus in musculoskeletal tissue cells after a symptom-free period (1/δh). This mechanism is suggested in [12]. As a result, the dynamics of the asymptomatic humans are described by
| A′h=pγIh−(μh+δh)Ah. |
4. Recovered humans (Rh): A fraction (1−p) of infected individuals are assumed to undergo a full recovery with permanent immunity. The number of recovered humans increases at a rate (1−p)γ and decreases due to natural death (μh). This translates into the following equation:
| R′h=(1−p)γIh−μhRh. |
Mosquitoes are classified into two compartments:
1. Susceptible vectors (Sv): Because vertical transmission has not been reported, we assume that all mosquitoes are born without the virus. Additionally, we assume that the birth and death rates are the same (μv), and that consequently, the vector population is constant (Sv+Iv=Nv). As a susceptible mosquito can become infected only if it comes into contact with an individual who has the virus, it is supposed to become infected after biting an infected or asymptomatic human, because hosts in the second compartment still have a viral load in the cells of the musculoskeletal tissue. Therefore, analogous to transmission in humans, the rate of infection in vectors depends on the bites b and the transmission probability from humans to vectors βv; under the hypothesis that there is less transmission by the asymptomatic humans than by infected humans, we introduce the non-negative parameter κ to moderate the fraction of transmission from asymptomatic humans to vectors. These assumptions give rise to the following equation:
| S′v=μvNv−βvbNhSvIh−κβvbNhSvAh−μvSv. |
2. Infected vectors (Iv): The number of infected mosquitoes increases according to the transmission mechanism described above and it decreases when infected mosquitoes die, since a mosquito never recovers and its life expectancy is not modified. Therefore, the number of infected mosquitoes is subject to the following equation:
| I′v=βvbNhSvIh+κβvbNhSvAh−μvIv. |
In summary, the dynamics between the hosts and vectors that determine the spread of Chikungunya (see Figure 1), including relapse, translate into the following system of ordinary differential equations:
| S′h=μhNh−βhbNhShIv−μhSh,I′h=βhbNhShIv−(μh+γ)Ih+δhAh,A′h=pγIh−(μh+δh)Ah,R′h=(1−p)γIh−μhRh,S′v=μvNv−βvbNhSvIh−κβvbNhSvAh−μvSv,I′v=βvbNhSvIh+κβvbNhSvAh−μvIv. | (2.1) |
Here, both total populations are considered constant, so Nh=Sh+Ih+Ah+Rh y Nv=Sv+Iv. Under these conditions, system (2.1) can be written as follows:
| S′h=μhNh−βhbNhShIv−μhSh,I′h=βhbNhShIv−(μh+γ)Ih+δhAh,A′h=pγIh−(μh+δh)Ah,I′v=βvbNh(Nv−Iv)(Ih+κAh)−μvIv. | (2.2) |
We analyze the model given in (2.2) in the following epidemiologically feasible region:
| Δ={(Sh,Ih,Ah,Iv)∈R4+:Sh+Ih+Ah≤Nh,Iv≤Nv}. |
System (2.2) contains two epidemiologically feasible equilibrium points in the non-negative orthant R4+ according to direct calculation: the disease-free equilibrium P0=(Nh,0,0,0) and a unique endemic equilibrium P∗=(S∗h,I∗h,A∗h,I∗v), with
| S∗h=Nh(NhN−1vμhˆR0+βhb)ˆR0(NhN−1vμh+βhb),I∗h=(μhNh(μh+δh)βhb(μh+γ)(μh+δh)−δhpγ)(ˆR0−1ˆR0(NhN−1vμh+βhb)),A∗h=(μhNhβhbpγ(μh+γ)(μh+δh)−δhpγ)(ˆR0−1ˆR0(NhN−1vμh+βhb)),I∗v=μhNh(ˆR0−1)NhN−1vμhˆR0+βhb, | (3.1) |
where
| ˆR0=βhβvb2Nv(κpγ+μh+δh)Nhμv[(μh+γ)(μh+δh)−δhpγ]. | (3.2) |
For the computation of the basic reproductive number, we use the next-generation operator method introduced in [13]. The notation is as follows: F is a non-negative matrix of the new transmission terms, and V is an M-matrix of the transition terms for individuals between compartments. For system (2.2), the matrices F and V are, respectively,
| F=(00βhb000βvbNvNhκβvbNvNh0) and V=(μh+γ−δh0−pγμh+δh000μv). |
It follows that the basic reproductive number, denoted by R0=ρ(FV−1), where ρ is the spectral radius, is given by
| R0=√ˆR0. | (3.3) |
In the following subsections, without loss of generality, we present the bifurcation and global stability results in terms of the threshold quantity ˆR0.
It is easy to prove that the disease-free equilibrium P0 is non-hyperbolic when ˆR0=1 since one of its eigenvalues vanishes; consequently, in this case, a bifurcation could occur. This subsection shows that this is indeed the case. For this purpose, Theorem 4.1 of [14] will be used.
First, the abbreviated set of equations in (2.2) is rewritten in the following form:
| dx1dt=μhNh−βhbNhx1x4−μhx1,dx2dt=βhbNhx1x4−(μh+γ)x2+δhx3,dx3dt=pγx2−(μh+δh)x3,dx4dt=βvbNh(Nv−x4)(x2+κx3)−μvx4, | (3.4) |
to which the following new variables have been introduced:
| x1≡Sh, x2≡Ih, x3≡Ah, x4≡Iv. |
If we assume that βv=ξβh and ϕ≡βhb is the bifurcation parameter, system (3.4) can take the following form:
| dx1dt=μhNh−ϕNhx1x4−μhx1≡f1,dx2dt=ϕNhx1x4−α1x2+δhx3≡f2,dx3dt=pγx2−α2x3≡f3,dx4dt=ξϕNh(Nv−x4)(x2+κx3)−μvx4≡f4, | (3.5) |
where we have made the following identifications:
| α1≡μh+γ and α2≡μh+δh, | (3.6) |
and the components of the vector field of (3.5) have been denoted by fi, with i=1,...,4. When ˆR0=1, we write ϕ=ϕ∗, which, according to (3.2), satisfies the following relationship:
| 1=ξ(ϕ∗)2Nv(κpγ+μh+δh)Nhμv[(μh+γ)(μh+δh)−δhpγ]. | (3.7) |
The Jacobian matrix of system (3.5), evaluated at the equilibrium point P0 when ϕ=ϕ∗, is given as
| J(P0)=(−μh00−ϕ∗0−α1δhϕ∗0pγ−α200ξϕ∗NvNhκξϕ∗NvNh−μv). | (3.8) |
It can easily be shown that this matrix has a zero eigenvalue.
According to the procedure described in [14], the eigenvector corresponding to this zero eigenvalue is
| w=1α1+α2+ξ(ϕ∗)2Nv(κpγ+α2−μv)Nhμ2v(−ϕ∗μhϕ∗α2α1α2−δhpγpγϕ∗α1α2−δhpγ1), | (3.9) |
while the left eigenvector corresponding to the same eigenvalue of the transpose of the matrix in (3.8) is
| v=α1α2−δhpγϕ∗(011pγ[α1−ξ(ϕ∗)2NvμvNh]ϕ∗μv). | (3.10) |
Both vectors satisfy the condition that w⋅v=1. The nonzero second partial derivatives of the components fi are given by
| ∂2f2(P0)∂x1∂x4=∂2f2(P0)∂x4∂x1=ϕNh, ∂2f4(P0)∂x2∂x4=∂2f4(P0)∂x4∂x2=−ξϕNh, ∂2f4(P0)∂x3∂x4=∂2f4(P0)∂x4∂x3=−κξϕNh | (3.11) |
and
| ∂2f2(P0)∂x4∂ϕ=1, ∂2f4(P0)∂x2∂ϕ=ξNvNh, ∂2f4(P0)∂x3∂ϕ=κξNvNh. | (3.12) |
Thus, the quantities a and b as indicated in Theorem 4.1 of [14] and written in terms of the previous derivatives, are, respectively,
| a=2v2w1w4ϕNh−2v4w4(w2+κw3)ξϕNh | (3.13) |
and
| b=v2w4+v4(w2+κw3)ξNvNh, | (3.14) |
where w1, w2, w3 and w4 are the first, second, third and fourth components of the eigenvector in (3.9); meanwhile, v2 and v4 are the first and fourth components of the left eigenvector given in (3.10).
It can be shown that, according to the Routh-Hurwitz stability criterion, to guarantee the local asymptotic stability of P0 when ˆR0<1, the following relations must be satisfied:
| α1α2−δhpγ>0 | (3.15) |
and
| α1+α2−ˆR0(α1α2−δhpγ)κpγ+α2>0. | (3.16) |
Based on relations (3.7), (3.15) and (3.16), it can be shown that w1<0,w2>0,w3>0 and w4>0, while v1>0,v2>0 and v4>0. Thus, according to (3.13) and (3.14), we have that a<0 and b>0. Therefore, since a<0 and b>0, part iv of Theorem 4.1 in [14] must hold. Alternatively, this result can be formulated as follows.
Theorem 3.1. The disease-free equilibrium point P0=(Nh,0,0,0), when ˆR0=1, presents a forward bifurcation.
As a consequence of this result, when ˆR0>1, there is a family of asymptotically stable infected equilibrium points, which we will denote as P∗=(S∗h,I∗h,A∗h,I∗v), constituting the upper branch of these types of bifurcations.
We obtained some conditions on the global stability of the disease-free equilibrium of system (2.2) by using the method of Lyapunov functions. The usual process to construct a Lyapunov function for a disease-free equilibrium in epidemic or intra-host viral infection models is to introduce the Volterra-type function to the susceptible compartment and linear functions to the other compartments, and to determine the constants to guarantee the negativity of the derivative of the Lyapunov function along trajectories.
Theorem 3.2. If ˆR0≤1, then the disease-free equilibrium P0=(Nh,0,0,0) of system (2.2) is globally asymptotically stable in Δ.
Proof. We construct the following Lyapunov function for system (2.2):
| V(Sh,Ih,Ah,Iv)=(Sh−Nh−NhlnShNh)+Ih+1μh+δh(δh+βhβvb2NhμvκNv)Ah+βhbμvIv. | (3.17) |
The function V(t) is defined, continuous and positive definite for all Sh,Ih,Ah,Iv≥0. Additionally, the global minimum V(Sh,Ih,Ah,Iv)=0 occurs at P0=(Nh,0,0,0), and, therefore, V is a Lyapunov function. By calculating its derivative along the solution of (2.2), we obtain
| ddtV(Sh,Ih,Ah,Av)=(1−NhSh)dShdt+dIhdt+1μh+δh(δh+βhβvb2NhμvκNv)dAhdt+βhbμvdIvdt=(Sh−NhSh)(μhNh−βhbNhShIv−μhSh)+(βhbNhShIv−(μh+γ)Ih+δhAh)+1μh+δh(δh+βhβvb2NhμvκNv)(pγIh−(μh+δh)Ah)+βhbμv(βvbNh(Nv−Iv)(Ih+κAh)−μvIv)=−μh(Sh−Nh)2Sh+[pγμh+δh(δh+βhβvb2NhμvκNv)+βhβvb2Nhμv−(μh+γ)]Ih−βhβvb2Nhμv(Ih+κAh)Iv,=−μh(Sh−Nh)2Sh−[(μh+γ)(μh+δh)−δhpγμh+δh](1−ˆR0)Ih−βhβvb2Nhμv(Ih+κAh)Iv. |
If ˆR0≤1, then dV/dt≤0. Note that dV/dt=0 if and only if Sh=Nh, Ih=0 and Iv=0 or if ˆR0=1, Sh=Nh and Iv=0. Therefore, the largest compact invariant set in {(Sh,Ih,Ah,Iv):dV/dt=0} is the singleton {P0}. By the classical LaSalle invariance principle (Theorem 5.3 of [15]), P0 is globally asymptotically stable in Δ if ˆR0≤1.
We consider the global asymptotic stability of a unique endemic equilibrium P∗ by using linear combinations of Volterra-type functions. The usual process to construct a Lyapunov function for a positive equilibrium in epidemic [16,17,18,19] or intra-host viral infection [20] models is to propose the use of the Volterra-type function, and to determine the constants to guarantee the negativity of the derivative of the Lyapunov function along trajectories.
Theorem 3.3. If ˆR0>1, then the endemic equilibrium P∗=(S∗h,I∗h,A∗h,I∗v) of system (2.2) is globally asymptotically stable in int(Δ).
Proof. Define L:int(R4+)→R+:
| L(Sh,Ih,Ah,Iv)=(I∗h+κA∗h)(Sh−S∗h−S∗hlnShS∗h)+(I∗h+κA∗h)(Ih−I∗h−I∗hlnIhI∗h)+1pγI∗h(δhA∗h(I∗h+κA∗h)+κβhbNhS∗hI∗vA∗h)(Ah−A∗h−A∗hlnAhA∗h)+βhS∗hI∗vβv(Nv−I∗v)(Iv−I∗v−I∗vlnIvI∗v). |
The function L(t) is defined, continuous and positive definite for all Sh,Ih,Ah,Iv≥0. Additionally, the global minimum L(Sh,Ih,Ah,Iv)=0 occurs at P∗=(S∗h,I∗h,A∗h,I∗v), and, therefore, L is a Lyapunov function. We calculate the time derivative of L(t):
| dL(t)dt=(I∗h+κA∗h)(1−S∗hSh)[μhNh−βhbNhShIv−μhSh]+(I∗h+κA∗h)(1−I∗hIh)[βhbNhShIv−(μh+γ)Ih+δhAh]+1pγI∗h(δhA∗h(I∗h+κA∗h)+κβhbNhS∗hI∗vA∗h)(1−A∗hAh)[pγIh−(μh+δh)Ah]+βhS∗hI∗vβv(Nv−I∗v)(1−I∗vIv)(βvbNh(Nv−I∗v)I∗hIhI∗h+κβvbNh(Nv−I∗v)A∗hAhA∗h−μvI∗vIvI∗v)+βhS∗hI∗vβv(Nv−I∗v)βvbNhI∗v(2−I∗vIv−IvI∗v)(Ih+κAh). |
The coordinates S∗h, I∗h, A∗h and I∗v of the endemic equilibrium (3.1) satisfy the following equations:
| μhNh=βhbNhS∗hI∗v+μhS∗h,μh+γ=βhbNhS∗hI∗vI∗h+δhA∗hI∗h,μh+δh=pγI∗hA∗h,μvI∗v=βvbNh(Nv−I∗v)(I∗h+κA∗h). | (3.18) |
Using the identities given in (3.18), we have
| dL(t)dt=(I∗h+κA∗h)[μhS∗h(2−S∗hSh−ShS∗h)+βhbNhS∗hI∗v(1−S∗hSh−ShIvS∗hI∗v+IvI∗v)]+(I∗h+κA∗h)[βhbNhS∗hI∗v(1+ShIvS∗hI∗v−IhI∗h−I∗hIhShIvS∗hI∗v)+δhA∗h(1+AhA∗h−IhI∗h−AhA∗hI∗hIh)]+1pγI∗h(δhA∗h(I∗h+κA∗h)+κβhbNhS∗hI∗vA∗h)[pγI∗h(1+IhI∗h−AhA∗h−A∗hAhIhI∗h)]+βhS∗hI∗vβv(Nv−I∗v)[βvbNh(Nv−I∗v)I∗h(1−I∗vIhIvI∗h+IhI∗h−IvI∗v)+κβvbNh(Nv−I∗v)A∗h(1−I∗vAhIvA∗h+AhA∗h−IvI∗v)]+βhS∗hI∗vβv(Nv−I∗v)βvbNhI∗v(2−I∗vIv−IvI∗v)(Ih+κAh). |
After several calculations, we have
| dL(t)dt=(I∗h+κA∗h)μhS∗h(2−S∗hSh−ShS∗h)+(I∗h+κA∗h)βhbNhS∗hI∗v(2−S∗hSh−IhI∗h−I∗hShIvIhS∗hI∗v+IvI∗v)+δhA∗h(I∗h+κA∗h)(1+AhA∗h−IhI∗h−AhA∗hI∗hIh)+(δhA∗h(I∗h+κA∗h)+κβhbNhS∗hI∗vA∗h)(1+IhI∗h−AhA∗h−A∗hAhIhI∗h)+bβhS∗hI∗vI∗hNh(1−I∗vIhIvI∗h+IhI∗h−IvI∗v)+κbβhS∗hI∗vA∗hNh(1−I∗vAhIvA∗h+AhA∗h−IvI∗v)+bβhS∗h(I∗v)2(Nv−I∗v)Nh(2−I∗vIv−IvI∗v)(Ih+κAh). |
Therefore,
| dL(t)dt=μhS∗h(I∗h+κA∗h)(2−S∗hSh−ShS∗h)+bβhS∗h(I∗v)2(Nv−I∗v)Nh(2−I∗vIv−IvI∗v)(Ih+κAh)+bβhS∗hI∗vI∗hNh(3−S∗hSh−I∗vIhIvI∗h−I∗hShIvIhS∗hI∗v)+δhA∗h(I∗h+κA∗h)(2−A∗hIhAhI∗h−AhI∗hA∗hIh)+κbβhS∗hI∗vA∗hNh(4−S∗hSh−I∗vAhIvA∗h−A∗hIhAhI∗h−I∗hShIvIhS∗hI∗v). |
The arithmetic mean is greater than the geometric mean, i.e., the terms (2−S∗hSh−ShS∗h), (2−I∗vIv−IvI∗v), (3−S∗hSh−I∗vIhIvI∗h−I∗hShIvIhS∗hI∗v), (2−A∗hIhAhI∗h−AhI∗hA∗hIh) and (4−S∗hSh−I∗vAhIvA∗h−A∗hIhAhI∗h−I∗hShIvIhS∗hI∗v) are negative. Consequently, dL/dt≤0 for any coordinate values (Sh,Ih,Ah,Iv) and dL/dt=0 if and only if Sh=S∗h, Ih=I∗h, Ah=A∗h and Iv=I∗v. Therefore, the largest compact invariant set in {(Sh,Ih,Ah,Iv):dL/dt=0} is the singleton {P∗}. If ˆR0>1, by the classical LaSalle invariance principle (Theorem 5.3 of [15]), the endemic equilibrium P∗ is globally asymptotically stable in int(Δ).
We consider the model
| yi=Xθ(ti)+ε(ti),i=1,…,n, | (4.1) |
where yi is the i-th observation of the data that represents the number of infected humans, θ=(βh,βv,b,μv,γ,p,δh,κ,Nv) is the unknown vector of parameters of the model in (2.2) to estimate, Xθ is the numerical solution of the system (2.2) for the state variable Ih according to the Runge–Kutta fourth-order method and ε(ti) is the random error. The Bayesian statistical model is used in situations in which the dynamics of the expected value E(yi)=Xθ(ti) are described by the host-vector model and there is an error around it, i.e, yi−E(yi)=ε(ti). The errors in each measurement are assumed to be independent even if the expected values E(yi) follow the host-vector model.
We estimate θ by using Bayesian inference, which allowed us to add previous information from the literature to the data to generate the distribution for each parameter in θ. According to Bayes' theorem,
| P(θ|y)∝P(y|θ)P(θ), |
where P(θ) is the prior distribution, P(y|θ) is the likelihood function and P(θ|y) is the posterior distribution. We assume that ε(ti)∼N(0,σ2) and yi∼N(Xθ(ti),σ2). The likelihood function is given by
| P(y|θ)=n∏i=11√2πσ2exp[−12σ2(yi−Xθ(ti))2]. |
To choose the prior distributions, we conducted a literature review of the parameters βh, βv, b, μv and γ reported in host-vector models for Chikungunya [3,4,5,6,7], the relapse period 1/δh from the epidemiological literature [8,12] and the parameters of the town (Acapulco, Mexico) where the epidemic outbreak occurred (μh, Nh and Nv) [12,21]. The parameter values are given in the third column of Table 1. We used uniform densities for most of the parameters, whose support includes the values collected from the literature. We only use beta informative prior distributions for the transmission probability parameters. The prior distributions are given in the fourth column of Table 1. Cole [22] showed that the lack of identifiability results in a strong dependence on the prior information, and that, if you use informative prior information, the estimates will be close to the true parameter values.
| Parameter | Description | Reference | Mean value or range of values | Prior distribution |
| βh | Transmission probability: from vector to human | [7] | 0.9999 [0.6, 1] | Beta(5,2) |
| [4] | 0.375 | |||
| [3] | [0.5, 0.8] | |||
| [5] | 0.67 [0.26, 1] | |||
| βv | Transmission probability: from human to vector | [7] | 0.6 [0.6, 1] | Beta(5,2) |
| [4] | 0.375 | |||
| [3] | 0.37 | |||
| b | Number of bites | [7] | 2.4676 [1, 3] | U(0,4) |
| [4] | 1 | |||
| [3] | 0.5 or 1 | |||
| μv | Birth and death rates of mosquitoes [month−1] | [4] | 2.72 | U(2,4.5) |
| [3] | 4.28 | |||
| [6] | 2.143 | |||
| γ | Recovery rate [month−1] | [7] | [3.7, 4.5] | U(2.5,7.5) |
| [6] | [2, 6] | |||
| p | Fraction of infected becoming asymptomatic | Supposed | U(0,1) | |
| δh | Relapse rate [month−1] | [8] | 0.5 | U(0,1) |
| [12] | 0.66 | |||
| κ | Transmission fraction: from asymptomatic humans to vectors | Supposed | Beta(2,5) | |
| Nv | Total number of vectors | Supposed | 2 to 4.5 times more than the number of humans | U(11740,26415) |
| μh | Birth and death rates of humans [month−1] | [21] | 175×12≈0.0011 | Fixed value |
| Nh | Total number of humans | [12] | 5870 | Fixed value |
To sample from the posterior distributions of nine parameters, we use the No-U-Turn-Sampler algorithm, which is a Hamiltonian Monte Carlo method. The reader is referred to [23] for a description of the method. Three independent Hamiltonian Monte Carlo chains were initialized with a random initial value and run with 20,000 iterations. For each parameter, we calculated the potential scale reduction factor, commonly known as Rhat [24]. Rhat values less than or equal to 1.2 indicate convergence to a stationary distribution. Furthermore, we verified that the trace plots showed good mixing. The libraries DifferentialEquations [25] and turing [26] of the Julia software [27] were used.
The Bayesian point estimators were the means of the posterior distributions corresponding to a minimization of the expected squared error loss function. The 95% credible interval (CrI) was calculated by using the 2.5th and 97.5th percentiles.
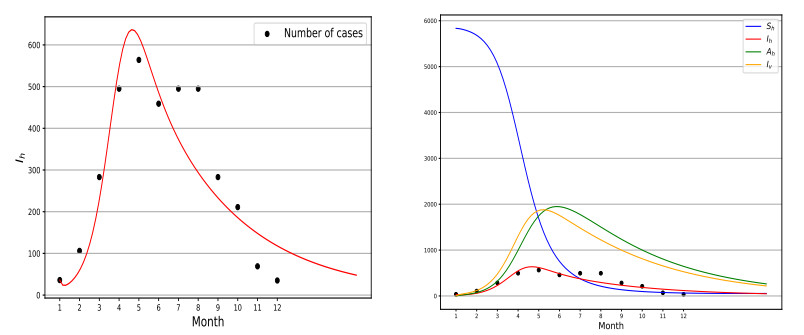
In Table 2, we report the point and interval estimations of parameters and the basic reproductive number. We will discuss the convergence criteria of the Markov chain Monte Carlo method in the Appendix. The estimated fraction of infected individuals who become asymptomatic, p, was approximately 0.6536, and with a probability of 0.95, this value is between 0.3916 and 0.8324. The estimated fraction of transmission from asymptomatic humans to vectors was approximately 0.2558, and with a probability of 0.95, this value is between 0.0385 and 0.6030. The estimated relapse rate δh was 0.7506 (95% CrI: 0.2914–0.9906). The estimated basic reproductive number R0 was 2.80 (95% CrI: 2.65–4.50). In Figure 2, we show the fit of the model to the number of humans infected with Chikungunya and numerical simulations of the susceptible human, infected human, asymptomatic human and infected vector compartments as performed by using the Bayesian mean estimation of the parameters.
| Parameter | Mean | Median | 95% Credible interval | Rhat |
| βh | 0.7379 | 0.7531 | (0.4377, 0.9574) | 1.0001 |
| βv | 0.7570 | 0.7737 | (0.4633, 0.9638) | 1.0002 |
| b | 3.2158 | 3.2528 | (2.2392, 3.9579) | 1.0002 |
| μv | 3.3510 | 3.3914 | (2.0874, 4.4459) | 1.0000 |
| γ | 4.5821 | 4.4888 | (2.6747, 7.0150) | 1.0002 |
| p | 0.6536 | 0.6710 | (0.3916, 0.8324) | 1.0001 |
| δh | 0.7506 | 0.7910 | (0.2914, 0.9906) | 1.0000 |
| κ | 0.2558 | 0.2321 | (0.0385, 0.6030) | 1.0001 |
| Nv | 19440.1813 | 19580.1146 | (12254.6677, 26040.5418) | 1.0001 |
| R0 | 2.8057 | 1.8199 | (2.6528, 4.5063) | * |
We performed a sensitivity analysis to measure the dependency of a variable on the parameters and thus identify the parameters that have the highest impact. The sensitivity index approximates the fractional change in a variable X that results from a unit fractional change in a parameter α when the other parameters are kept constant, and it is given by Eα=αX∂X∂α [28]. If Eα<0, the relationship between the parameter α and X is inversely proportional. Otherwise, if Eα is positive, the relationship is directly proportional.
Using the basic reproductive number R0, as determined by using (3.3), we calculated the sensitivity index for each parameter:
| Eμh=−12μh[(μh+δh)2+κpγ(2μh+δh+γ)+δhpγ](κpγ+μh+δh)[(μh+γ)(μh+δh)−δhpγ], |
| Ep=12pγ(μh+δh)[(μh+γ)κ+δh](κpγ+μh+δh)[(μh+γ)(μh+δh)−δhpγ], |
| Eγ=12γ(μh+δh)[μh(pκ−1)+δh(p−1)](κpγ+μh+δh)[(μh+γ)(μh+δh)−δhpγ], |
| Eδh=12δhpγ[−κ(μh+γ(1−p))+μh](κpγ+μh+δh)[(μh+γ)(μh+δh)−δhpγ], |
| Eκ=12κpγκpγ+μh+δh, |
| Eb=1, |
| ENv=Eβh=Eβv=12, |
| ENh=Eμv=−12. |
We have used the fixed values of μh and Nh from Table 1 and the Bayesian mean estimation of the model parameters from Table 2 to create Figure 3. The sensitivity indices shown in this figure indicate that the parameters βh, βv, b, p, κ and Nh have direct relationships with R0. This implies that decreasing the values of these parameters will reduce R0. On the contrary, the parameters μv, γ, δh, μv and Nh have inverse relationships with R0. This implies that increasing the values of these parameters will decrease R0. From Figure 3, we observe that the fraction of infected individuals who become asymptomatic, p, has the greatest (direct or inverse) impact on R0, followed by the number of bites b, the transmission probability (βh and βv), the total number of vectors Nv and the death rate of mosquitoes μv.
Among the new parameters incorporated into the Chikungunya model, we note that the relapse rate δh and the fraction of transmission from asymptomatic humans to vectors κ have a weak impact on R0. We interpret the negative relationship between δh and R0 as increasing the relapse rate, which decreases R0, that is to say, by decreasing the relapse period (1/δh), R0 is reduced. On the other hand, the fraction of infected individuals who become asymptomatic, p, has a strong impact on R0.
Mathematical models for Chikungunya have incorporated the aquatic phase of the mosquito [3,29], control mechanics [4,7] and the incorporation of intrinsic and extrinsic incubation periods [6,29]. The phenomenon of relapses in Chikungunya virus infections has been reported in many studies [8,9,10,11,12], but it has not been considered in mathematical models. In this paper, we proposed the first model that incorporates the recurrence of symptoms into the dynamics of Chikungunya.
First, we carried out a qualitative analysis of the Chikungunya model with relapses. The system has the classic equilibrium points, namely, disease-free and endemic equilibrium points. We calculated the basic reproductive number (R0=√ˆR0) for the disease by using the next-generation operator method. We proved that the disease-free equilibrium point P0, when ˆR0=1, presents a forward bifurcation. We analyzed the global stability of the equilibrium points by using the Lyapunov function method. We proved that the disease-free equilibrium is globally asymptotically stable when ˆR0≤1, and that the endemic equilibrium is globally asymptotically stable when ˆR0>1 in the interior of the feasible region.
Second, a Chikungunya outbreak occurred in Acapulco, Mexico, and we used the epidemiological data of self-reported cases from this outbreak obtained in [12] to fit the state variable Ih of the model. We used the Bayesian approach to estimate nine model parameters and two fixed parameter values. For this outbreak, we estimated that the fraction of infected individuals who become asymptomatic, p, is between 0.3916 and 0.8324. These estimates of the infected people who experience relapses with rheumatoid symptoms are consistent with those reported in the literature which ranges from approximately 30% [12] to 72% [8]. The relapse period (1/δh) is estimated to be between 1.00 and 3.43. Infected asymptomatic humans play a relatively small role in the transmission of the infection; the fraction of transmission from asymptomatic humans to vectors ranged from 0.0385 to 0.6030. We estimated that the basic reproductive number was equal to 2.80 (95% CrI: 2.65–4.50); this value plays an important role as a bifurcation value and in epidemiological interpretation. Recently, Haider et al. [30] estimated, using a frequentist meta-analysis, the basic reproductive number for Chikungunya based on various studies that have estimated this value; they obtained a value of 3.4 (95% confidence interval: 2.4–4.2). Our Bayesian estimate of R0 is very similar to that reported in [30].
Third, we performed a local sensitivity analysis of the basic reproductive number by using the Bayesian mean estimation of the model parameters. Among the new parameters incorporated into the Chikungunya model, we found that the fraction of infected individuals who become asymptomatic, p, has a strong impact on R0. The fraction p and the relapse period 1/δh could be reduced with antiviral therapies, and, consequently, R0 could be decreased. Conversely, the relapse rate δh has a weak impact on R0, but it is not insignificant.
Fourth, to assess the efficiency of insecticide control in the presence and absence of relapses, we varied the mosquito death rate and examined its impact on R0. The results for these two scenarios are shown in Figure 4. For the case in which the fraction p is considered, we note that we need a higher mortality rate to ensure that the disease is controlled, with an R0 value below unity. When relapses are not considered in the modeling, it intuitively follows that less control is needed over the mosquito population. This demonstrates the importance of incorporating relapse into Chikungunya models for the estimation of the dynamics of the disease and the study of control measures and protocols.
Finally, this work has some limitations. The occurrence of Chikungunya cases was self-reported through a designed questionnaire, and the cases were not confirmed by PCR or serological tests. Our model considers that the relapse rate is constant, but the epidemiological literature reports variability in the relapse periods that should be considered in future work.
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
This work was partially supported by the CONAHCYT-Ciencia de Frontera project "Ecuaciones de evolución, sus estados estacionarios y su comportamiento asintótico con aplicaciones en Física y Biología" (project no. 684340). Vázquez-Peña is indebted to CONAHCYT for the scholarship (project no. 684340) that allowed him to carry out his thesis to obtain a Bachelor's degree in Applied Mathematics at the Benemérita Universidad Autónoma de Puebla.
The authors declare that they have no conflicts of interest regarding the publication of this manuscript.
In this Appendix, the convergence criteria of the host-vector model for Chikungunya are discussed. The chains of model parameters show good mixing, as shown in Figure 5. Table 2 shows the results of the computation of the Rhat values, thus indicating that both convergence results are consistent.
| [1] | R. C. Rivera-Ávila, Fiebre chikungunya en México: Caso confirmado y apuntes para la respuesta epidemiológica, (Spanish) [Chikungunya fever in Mexico: confirmed case and notes on the epidemiologic response], Salud Pública de México, 56 (2014), 402–404. |
| [2] |
S. Yactayo, J. E. Staples, V. Millot, L. Cibrelus, P. Ramon-Pardo, Epidemiology of Chikungunya in the Americas, J. Infect. Diseases, 214 (2016), S441–S445. https://doi.org/10.1093/infdis/jiw390 doi: 10.1093/infdis/jiw390

|
| [3] |
Y. Dumont, F. Chiroleu, C. Domerg, On a temporal model for the Chikungunya disease: modeling, theory and numerics, Math. Biosci., 213 (2008), 80–91. https://doi.org/10.1016/j.mbs.2008.02.008 doi: 10.1016/j.mbs.2008.02.008

|
| [4] |
Y. Dumont, F. Chiroleu, Vector control for the Chikungunya disease, Math. Biosci. Eng., 7 (2010), 313–345. https://doi.org/10.3934/mbe.2010.7.313 doi: 10.3934/mbe.2010.7.313

|
| [5] |
D. Ruiz-Moreno, I. S. Vargas, K. E. Olson, L. C. Harrington, Modeling dynamic introduction of Chikungunya virus in the United States, PLoS Negl. Trop. Diseases, 6 (2012), e1918. https://doi.org/10.1371/journal.pntd.0001918 doi: 10.1371/journal.pntd.0001918

|
| [6] |
G. C. González-Parra, D. F. Aranda, B. Chen-Charpentier, M. Díaz-Rodríguez, J. E. Castellanos, Mathematical modeling and characterization of the spread of Chikungunya in Colombia, Math. Comput. Appl., 24 (2019), 6. https://doi.org/10.3390/mca24010006 doi: 10.3390/mca24010006

|
| [7] |
H. Abboubakar, A. K. Guidzavaï, J. Yangla, I. Damakoa, R. Mouangue, Mathematical modeling and projections of a vector-borne disease with optimal control strategies: A case study of the Chikungunya in Chad, Chaos Solit. Fract., 150 (2021), 111197. https://doi.org/10.1016/j.chaos.2021.111197 doi: 10.1016/j.chaos.2021.111197

|
| [8] |
E. Couturier, F. Guillemin, M. Mura, L. León, J.M. Virion, M.J. Letort, et al., Impaired quality of life after Chikungunya virus infection: a 2-year follow-up study, Rheumatology, 51 (2012), 1315–1322. https://doi.org/10.1093/rheumatology/kes015 doi: 10.1093/rheumatology/kes015

|
| [9] |
F. Simon, P. Parola, M. Grandadam, S. Fourcade, M. Oliver, P. Brouqui, et al., Chikungunya infection: An emerging rheumatism among travelers returned from Indian Ocean islands: report of 47 cases, Medicine, 86 (2007), 123–137. https://doi.org/10.1097/md/0b013e31806010a5 doi: 10.1097/md/0b013e31806010a5

|
| [10] |
F. Staikowsky, K. Le Roux, I. Schuffenecker, P. Laurent, P. Grivard, A. Develay, et al., Retrospective survey of Chikungunya disease in Reunion Island hospital staff, Epidemiol. Infect., 136 (2008), 196–206. https://doi.org/10.1017/S0950268807008424 doi: 10.1017/S0950268807008424

|
| [11] |
G. Borgherini, P. Poubeau, A. Jossaume, A. Gouix, L. Cotte, A. Michault, et al., Persistent arthralgia associated with Chikungunya virus: A study of 88 adult patients on Reunion Island, Clin. Infect. Diseases, 47 (2008), 469–475. https://doi.org/10.1086/590003 doi: 10.1086/590003

|
| [12] |
I. X. de la Cruz-Castro, E. Nava-Aguilera, A. Morales-Pérez, Á. F. Betanzos-Reyes, M. Flores-Moreno, L. Morales-Nava, et al., Factors associated with Chikungunya relapse in Acapulco, Mexico: A cross-sectional study, Vector-Borne Zoonotic Diseases, 20 (2020), 782–787. http://doi.org/10.1089/vbz.2020.2615 doi: 10.1089/vbz.2020.2615

|
| [13] |
P. van den Driessche, J. Watmough, Reproduction numbers and sub-threshold endemic equilibria for compartmental models of disease transmission, Math. Biosci., 180 (2002), 29–48. https://doi.org/10.1016/S0025-5564(02)00108-6 doi: 10.1016/S0025-5564(02)00108-6

|
| [14] |
C. Castillo-Chávez, B. Song, Dynamical models of tuberculosis and their applications, Math. Biosci. Eng., 1 (2004), 361–404. https://doi.org/10.3934/mbe.2004.1.361 doi: 10.3934/mbe.2004.1.361

|
| [15] | J. La Salle, S. Lefschetz, Stability by Liapunov's Direct Method with Applications, Academic Press, (1961). |
| [16] |
A. Korobeinikov, Lyapunov functions and global properties for SEIR and SEIS epidemic models, Math. Med. Biol., 21 (2004), 75–83. https://doi.org/10.1093/imammb/21.2.75 doi: 10.1093/imammb/21.2.75

|
| [17] | C. Vargas-De-León, J.A. Castro-Hernández, Local and global stability of host-vector disease models, Foro-Red-Mat: Revista Electrónica de Contenido Matemático, 25 (2008), 1–9. |
| [18] |
C. Vargas-De-León, Global analysis of a delayed vector-bias model for malaria transmission with incubation period in mosquitoes, Math. Biosci. Eng., 9 (2012), 165–174. https://doi.org/10.3934/mbe.2012.9.165 doi: 10.3934/mbe.2012.9.165

|
| [19] |
A. Li-Martín, R. Reyes-Carreto, C. Vargas-De-León, Dynamics of a dengue disease transmission model with two-stage structure in the human population, Math. Biosci. Eng., 20 (2023), 955–974. https://doi.org/10.3934/mbe.2023044 doi: 10.3934/mbe.2023044

|
| [20] |
A. Korobeinikov, Global properties of basic virus dynamics models, Bull. Math. Biol., 66 (2004), 879–883. https://doi.org/10.1016/j.bulm.2004.02.001 doi: 10.1016/j.bulm.2004.02.001

|
| [21] | Consejo Nacional de Población, Indicadores Demográficos de México de 1950 a 2050 (Spanish) [National Population Council, Demographic Indicators of Mexico from 1950 to 2050], 2022. |
| [22] | D. Cole, Parameter redundancy and identifiability, CRC Press, Taylor & Francis Group, (2020). |
| [23] |
M. D. Hoffman, A. Gelman, The No-U-Turn sampler: adaptively setting path lengths in Hamiltonian Monte Carlo, J. Machine Learn. Res., 15 (2014), 1593–1623. https://doi.org/10.48550/arXiv.1111.4246 doi: 10.48550/arXiv.1111.4246

|
| [24] |
A. Gelman, D.B. Rubin, Inference from iterative simulation using multiple sequences, Statistical Science, 7 (1992), 457–472. https://doi.org/10.1214/ss/1177011136 doi: 10.1214/ss/1177011136

|
| [25] |
C. Rackauckas, Q. Nie, DifferentialEquations.jl–a performant and feature-rich ecosystem for solving differential equations in Julia, J. Open Res. Software, 5 (2017), 1–5. https://doi.org/10.5334/jors.151 doi: 10.5334/jors.151

|
| [26] | H. Ge, K. Xu, Z. Ghahramani, Turing: A language for flexible probabilistic inference, in International Conference on Artificial Intelligence and Statistics, 84 (2018), 1682–1690. https://proceedings.mlr.press/v84/ge18b.html |
| [27] |
J. Bezanson, A. Edelman, S. Karpinski, V. B. Shah, Julia: A fresh approach to numerical computing, SIAM Rev., 59 (2017), 65–98. https://doi.org/10.1137/141000671 doi: 10.1137/141000671

|
| [28] | A.L. Lloyd, Sensitivity of model-based epidemiological parameter estimation to model assumptions, in Mathematical and Statistical Estimation Approaches in Epidemiology, Springer, (2009), 123–141. https://doi.org/10.1007/978-90-481-2313-1_6 |
| [29] | J. Yangla, H. Abboubakar, E. Dangbe, R. Yankoulo, A. A. A. Ari, I. Damakoa, et al., Fractional dynamics of a Chikungunya transmission model Sci. African, (2023), e01812. https://doi.org/10.1016/j.sciaf.2023.e01812 |
| [30] |
N. Haider, F. Vairo, G. Ippolito, A. Zumla, R. A. Kock, Basic reproduction number of Chikungunya virus transmitted by Aedes mosquitoes, Emerg. Infect. Diseases, 26 (2020), 2429–2431. https://doi.org/10.3201/eid2610.190957 doi: 10.3201/eid2610.190957

|
| 1. | Maria Guadalupe Vazquez-Peña, Cruz Vargas-De-León, Jorge Velázquez-Castro, Global stability for a mosquito-borne disease model with continuous-time age structure in the susceptible and relapsed host classes, 2024, 21, 1551-0018, 7582, 10.3934/mbe.2024333 | |
| 2. | U. J. Giménez-Mujica, J. Velázquez-Castro, A. Anzo-Hernández, T. P. Herrera-Ramírez, I. Barradas, Estimating the final size of vector-borne epidemics in metapopulation networks: methodologies and comparisons, 2025, 31, 1405-213X, 10.1007/s40590-025-00712-0 |
| Parameter | Description | Reference | Mean value or range of values | Prior distribution |
| βh | Transmission probability: from vector to human | [7] | 0.9999 [0.6, 1] | Beta(5,2) |
| [4] | 0.375 | |||
| [3] | [0.5, 0.8] | |||
| [5] | 0.67 [0.26, 1] | |||
| βv | Transmission probability: from human to vector | [7] | 0.6 [0.6, 1] | Beta(5,2) |
| [4] | 0.375 | |||
| [3] | 0.37 | |||
| b | Number of bites | [7] | 2.4676 [1, 3] | U(0,4) |
| [4] | 1 | |||
| [3] | 0.5 or 1 | |||
| μv | Birth and death rates of mosquitoes [month−1] | [4] | 2.72 | U(2,4.5) |
| [3] | 4.28 | |||
| [6] | 2.143 | |||
| γ | Recovery rate [month−1] | [7] | [3.7, 4.5] | U(2.5,7.5) |
| [6] | [2, 6] | |||
| p | Fraction of infected becoming asymptomatic | Supposed | U(0,1) | |
| δh | Relapse rate [month−1] | [8] | 0.5 | U(0,1) |
| [12] | 0.66 | |||
| κ | Transmission fraction: from asymptomatic humans to vectors | Supposed | Beta(2,5) | |
| Nv | Total number of vectors | Supposed | 2 to 4.5 times more than the number of humans | U(11740,26415) |
| μh | Birth and death rates of humans [month−1] | [21] | 175×12≈0.0011 | Fixed value |
| Nh | Total number of humans | [12] | 5870 | Fixed value |
| Parameter | Mean | Median | 95% Credible interval | Rhat |
| βh | 0.7379 | 0.7531 | (0.4377, 0.9574) | 1.0001 |
| βv | 0.7570 | 0.7737 | (0.4633, 0.9638) | 1.0002 |
| b | 3.2158 | 3.2528 | (2.2392, 3.9579) | 1.0002 |
| μv | 3.3510 | 3.3914 | (2.0874, 4.4459) | 1.0000 |
| γ | 4.5821 | 4.4888 | (2.6747, 7.0150) | 1.0002 |
| p | 0.6536 | 0.6710 | (0.3916, 0.8324) | 1.0001 |
| δh | 0.7506 | 0.7910 | (0.2914, 0.9906) | 1.0000 |
| κ | 0.2558 | 0.2321 | (0.0385, 0.6030) | 1.0001 |
| Nv | 19440.1813 | 19580.1146 | (12254.6677, 26040.5418) | 1.0001 |
| R0 | 2.8057 | 1.8199 | (2.6528, 4.5063) | * |
| Parameter | Description | Reference | Mean value or range of values | Prior distribution |
| βh | Transmission probability: from vector to human | [7] | 0.9999 [0.6, 1] | Beta(5,2) |
| [4] | 0.375 | |||
| [3] | [0.5, 0.8] | |||
| [5] | 0.67 [0.26, 1] | |||
| βv | Transmission probability: from human to vector | [7] | 0.6 [0.6, 1] | Beta(5,2) |
| [4] | 0.375 | |||
| [3] | 0.37 | |||
| b | Number of bites | [7] | 2.4676 [1, 3] | U(0,4) |
| [4] | 1 | |||
| [3] | 0.5 or 1 | |||
| μv | Birth and death rates of mosquitoes [month−1] | [4] | 2.72 | U(2,4.5) |
| [3] | 4.28 | |||
| [6] | 2.143 | |||
| γ | Recovery rate [month−1] | [7] | [3.7, 4.5] | U(2.5,7.5) |
| [6] | [2, 6] | |||
| p | Fraction of infected becoming asymptomatic | Supposed | U(0,1) | |
| δh | Relapse rate [month−1] | [8] | 0.5 | U(0,1) |
| [12] | 0.66 | |||
| κ | Transmission fraction: from asymptomatic humans to vectors | Supposed | Beta(2,5) | |
| Nv | Total number of vectors | Supposed | 2 to 4.5 times more than the number of humans | U(11740,26415) |
| μh | Birth and death rates of humans [month−1] | [21] | 175×12≈0.0011 | Fixed value |
| Nh | Total number of humans | [12] | 5870 | Fixed value |
| Parameter | Mean | Median | 95% Credible interval | Rhat |
| βh | 0.7379 | 0.7531 | (0.4377, 0.9574) | 1.0001 |
| βv | 0.7570 | 0.7737 | (0.4633, 0.9638) | 1.0002 |
| b | 3.2158 | 3.2528 | (2.2392, 3.9579) | 1.0002 |
| μv | 3.3510 | 3.3914 | (2.0874, 4.4459) | 1.0000 |
| γ | 4.5821 | 4.4888 | (2.6747, 7.0150) | 1.0002 |
| p | 0.6536 | 0.6710 | (0.3916, 0.8324) | 1.0001 |
| δh | 0.7506 | 0.7910 | (0.2914, 0.9906) | 1.0000 |
| κ | 0.2558 | 0.2321 | (0.0385, 0.6030) | 1.0001 |
| Nv | 19440.1813 | 19580.1146 | (12254.6677, 26040.5418) | 1.0001 |
| R0 | 2.8057 | 1.8199 | (2.6528, 4.5063) | * |