In this paper we develop a compartmental epidemic model to study the transmission dynamics of the COVID-19 epidemic outbreak, with Mexico as a practical example. In particular, we evaluate the theoretical impact of plausible control interventions such as home quarantine, social distancing, cautious behavior and other self-imposed measures. We also investigate the impact of environmental cleaning and disinfection, and government-imposed isolation of infected individuals. We use a Bayesian approach and officially published data to estimate some of the model parameters, including the basic reproduction number. Our findings suggest that social distancing and quarantine are the winning strategies to reduce the impact of the outbreak. Environmental cleaning can also be relevant, but its cost and effort required to bring the maximum of the outbreak under control indicate that its cost-efficacy is low.
Citation: Fernando Saldaña, Hugo Flores-Arguedas, José Ariel Camacho-Gutiérrez, Ignacio Barradas. Modeling the transmission dynamics and the impact of the control interventions for the COVID-19 epidemic outbreak[J]. Mathematical Biosciences and Engineering, 2020, 17(4): 4165-4183. doi: 10.3934/mbe.2020231
| [1] | Akira Nishimura, Ryuki Toyoda, Daichi Tatematsu, Masafumi Hirota, Akira Koshio, Fumio Kokai, Eric Hu . Optimum reductants ratio for CO2 reduction by overlapped Cu/TiO2. AIMS Materials Science, 2019, 6(2): 214-233. doi: 10.3934/matersci.2019.2.214 |
| [2] | Nhung Thi-Tuyet Hoang, Anh Thi-Kim Tran, Nguyen Van Suc, The-Vinh Nguyen . Antibacterial activities of gel-derived Ag-TiO2-SiO2 nanomaterials under different light irradiation. AIMS Materials Science, 2016, 3(2): 339-348. doi: 10.3934/matersci.2016.2.339 |
| [3] | Ya-Ting Tsu, Yu-Wen Chen . Preparation of gold-containing binary metal clusters by co-deposition-precipitation method and for hydrogenation of chloronitrobenzene. AIMS Materials Science, 2017, 4(3): 738-754. doi: 10.3934/matersci.2017.3.738 |
| [4] | Ririn Cahyanti, Sumari Sumari, Fauziatul Fajaroh, Muhammad Roy Asrori, Yana Fajar Prakasa . Fe-TiO2/zeolite H-A photocatalyst for degradation of waste dye (methylene blue) under UV irradiation. AIMS Materials Science, 2023, 10(1): 40-54. doi: 10.3934/matersci.2023003 |
| [5] | Ahmed Z. Abdullah, Adawiya J. Haider, Allaa A. Jabbar . Pure TiO2/PSi and TiO2@Ag/PSi structures as controllable sensor for toxic gases. AIMS Materials Science, 2022, 9(4): 522-533. doi: 10.3934/matersci.2022031 |
| [6] | Alfa Akustia Widati, Nuryono Nuryono, Indriana Kartini . Water-repellent glass coated with SiO2–TiO2–methyltrimethoxysilane through sol–gel coating. AIMS Materials Science, 2019, 6(1): 10-24. doi: 10.3934/matersci.2019.1.10 |
| [7] | Johana Gamez, Luis Reyes-Osorio, Oscar Zapata, Roberto Cabriales, Luis Lopez, Miguel Delgado-Pamanes . Study of protective hard coatings of SiO2-TiO2 on aluminum substrates. AIMS Materials Science, 2024, 11(2): 200-215. doi: 10.3934/matersci.2024011 |
| [8] | Douglas M. Franz, Mak Djulbegovic, Tony Pham, Brian Space . Theoretical study of the effect of halogen substitution in molecular porous materials for CO2 and C2H2 sorption. AIMS Materials Science, 2018, 5(2): 226-245. doi: 10.3934/matersci.2018.2.226 |
| [9] | Muhammad Yakob, Hamdani Umar, Puji Wahyuningsih, Rachmad Almi Putra . Characterization of microstructural and optical CoFe2O4/SiO2 ferrite nanocomposite for photodegradation of methylene blue. AIMS Materials Science, 2019, 6(1): 45-51. doi: 10.3934/matersci.2019.1.45 |
| [10] | Frank Tietz . Phase relations of NASICON materials and compilation of the quaternary phase diagram Na2O-P2O5-SiO2-ZrO2. AIMS Materials Science, 2017, 4(6): 1305-1318. doi: 10.3934/matersci.2017.6.1305 |
In this paper we develop a compartmental epidemic model to study the transmission dynamics of the COVID-19 epidemic outbreak, with Mexico as a practical example. In particular, we evaluate the theoretical impact of plausible control interventions such as home quarantine, social distancing, cautious behavior and other self-imposed measures. We also investigate the impact of environmental cleaning and disinfection, and government-imposed isolation of infected individuals. We use a Bayesian approach and officially published data to estimate some of the model parameters, including the basic reproduction number. Our findings suggest that social distancing and quarantine are the winning strategies to reduce the impact of the outbreak. Environmental cleaning can also be relevant, but its cost and effort required to bring the maximum of the outbreak under control indicate that its cost-efficacy is low.
Due to the increase in the global mean concentration of CO2 up to 407.8 ppmV as at February in 2019 [1], new CO2 utilization technology to reduce the amount of CO2 is required.
This study focuses on CO2 reduction and conversion into fuel by photocatalysis. TiO2 is the most common photocatalyt since it is convenient, inexpensive and has strong durability for chemicals and corrosion [2]. TiO2 is popular photocatalyst that can reduce CO2 into CO, CH4, CH3OH, and H2 etc. with ultraviolet (UV) light [3,4,5].
Unfortunately, pure TiO2 can only be activated under UV light illumination, it is not very effective under sunlight illumination as UV light accounts for only approximately 4% in the solar spectrum. In addition, the rate of electron/hole pair recombination is faster than the rate of chemical interaction between the adsorbents during redox reactions when using TiO2 [6].
According to recent research [3], many trials have been conducted for TiO2 to promote the performance of absorbing a photon with visible light illumination as well as to reduce the electron-hole recombination rate. For example, the previous studies investigated doping precious metal such as Pt [7], Ag [8], Au [9], Cu [10,11], composite material formed by GaP and TiO2 [12], complex assembly CdS/TiO2 to utilize two photocatalysts that have different band gaps [13], carbon-based AgBr nanocomposites TiO2 [14], CuInS2 sensitized TiO2 hybrid nanofibers [15] and preparation procedure of TiO2 using two alcohols (ethanol and isopropyl alcohol) and supercritical CO2 [16] to promote the performance of TiO2. Though the CO2 reduction performance was improved by these trials, the concentrations of the products were still low, which was ranging from 1 to 150 μmol/g-cat [7,8,9,10,11,12,13,14,15,16].
Among various metals have been used for doping, Pt is reportedly a good dopant for TiO2 [17,18,19]. However, Pd is considered as a favorite candidate [20,21,22], as Pd can extend the absorption band to 400–800 nm [23,24], which covers the whole visible light range. Pd/TiO2 performs a higher reduction performance compared to pure TiO2, especially, to produce hydrocarbon [23,24,25].
According to the literature survey, H2O or H2 was normally used as the reductant for CO2 reduction over Pd/TiO2 [20,21,22,23,24,25,26]. In studies of CO2 reducing with H2O [20,21,22,23,24,25], the mixture ratio of CO2 and H2O was fixed, but the ratio was not controlled and unclear. According to the report on CO2 reduction with H2 [26], the moral ratio of CO2:H2 was fixed at 1:4, but the impact of the ratio on CO2 reduction performance of Pd/TiO2 was not investigated. Though it is thought that the mixture ratio of CO2 and reductants influences the CO2 reduction performance of Pd/TiO2, there was no study investigating it. In addition, the effect of combination of H2O and H2 as reductant on CO2 reduction over Pd/TiO2 was not investigated yet.
To promote the CO2 reduction performance, the optimum reductant providing the proton (H+) should be clarified. According to the previous studies [27,28,29,30], the reaction mechanism to reduce CO2 with H2O can be summarized as shown below:
< Photocatalytic reaction >
| TiO2+hv→h++e− | (1) |
< Oxidization >
| 2H2O+4h+→4H++O2 | (2) |
< Reduction >
| CO2+2H++2e−→CO+H2O | (3) |
| CO2+8H++8e−→CH4+2H2O | (4) |
The reaction mechanism to reduce CO2 with H2 can be summarized as shown below [31].
< Photocatalytic reaction >
| TiO2+hv→h++e− | (5) |
< Oxidization >
| H2→2H++2e− | (6) |
< Reduction >
| CO2+e−→⋅CO−2 | (7) |
| ⋅CO−2+H++e−→HCOO− | (8) |
| HCOO−+H+→CO+H2O | (9) |
| H++e−→⋅H | (10) |
| CO2+8e−+8⋅H→CH4+2H2O | (11) |
Though a few studies using pure TiO2 under CO2/H2/H2O condition were reported [31,32,33], the effect of ratio of CO2, H2 and H2O as well as the effect of Pd doping with TiO2 on CO2 reduction characteristics was not investigated previously.
Consequently, the purpose of this study was to clarify the effect of moral ratio of CO2 and reductants of H2 and H2O on CO2 reduction characteristics of Pd/TiO2. Additionally, the present study also aims to clarify the optimum combination of reductants.
In the present paper, TiO2 film is coated on netlike glass fiber (SILIGLASS U, Nihonmuki Co.) by sol-gel and dip-coating process. Glass fiber whose diameter is about 10 μm is weaved as a net, resulting in the diameter of collected fiber of approximately 1 mm. As to the specification of each fiber, the porous diameter is approximately 1 nm and the specific surface area is approximately 400 m2/g. The composition of netlike glass fiber is SiO2 of 96 wt%. The aperture area is approximately 2 mm × 2 mm. Due to the porous structure of the netlike glass fiber, TiO2 film can be captured on netlike glass fiber easily in the step of preparation by sol-gel and dip-coating procedure. Additionally, it was believed that CO2 would be more easily absorbed by the prepared photocatalyst since the fiber has the porous characteristics.
After the coating of TiO2, this study loads nanosized Pd particles on TiO2 by pulse arc plasma method applying high voltage. The pulse number can control the quantity of Pd loaded on TiO2. We set the pulse number at 100 [34].
Since the netlike glass fiber is transparent, the light can pass through the netlike glass fiber. The present study has also investigated if two Pd/TiO2 coated netlike glass fiber discs were put one on top of the other (with certain distance, i.e., overlapping), what impact/improvement would be on the CO2 reduction performance. The following merits were expected by overlapping: (1) effective light utilization, (2) increment of usable photocatalyst to reduce CO2.
In this paper, the characterization of Pd/TiO2 was conducted by Scanning Electron Microscope (SEM), Electron Probe Micro Analyzer (EPMA), Scanning Transmission Electron Microscope (STEM), Energy Dispersive X-ray Spectrometer (EDS) and Electron Energy Loss Spectrum (EELS) analysis before CO2 reduction experiment. This study investigated the performance of CO2 reduction with H2 and H2O with illumination of Xe lamp including or excluding UV light. The combination of CO2/H2/H2O was changed for 1:0.5:0.5, 1:0.5:1, 1:1:0.5, 1:1:1, 1:2:2 based on moral ratio to clarify the optimum combination of CO2/H2/H2O for CO2 reduction with Pd/TiO2. In addition, the effect of overlapping two layers of Pd/TiO2 coated netlike glass fiber on CO2 reduction performance was investigated.
This study prepared TiO2 film using sol-gel and dip-coating procedure [34,35,36]. At first, [(CH3)2CHO]4Ti (95 wt% purification, produced by Nacalai Tesque Co.) of 0.3 mol, anhydrous C2H5OH (99.5 wt% purification, produced by Nacalai Tesque Co.) of 2.4 mol, distilled water of 0.3 mol, and HCl (35 wt% purification, produced by Nacalai Tesque Co.) of 0.07 mol were mixed to make TiO2 sol solution. As the basis to coat TiO2 film, this study cut off the sheet of netlike glass fiber as disc shape whose diameter and thickness were 50 mm and 1 mm, respectively. After that, this study immersed the disc shaped netlike glass fiber into TiO2 sol solution at 1.5 mm/s and lifted at 0.22 mm/s. In the next step, we dried it out and heated up controlling firing temperature (FT) and firing duration time (FD), where this study set FT and FD at 623 K and 180 s, respectively. Therefore, TiO2 film was coated on netlike glass disc. Pulse arc plasma method was selected to load Pd on TiO2 film. This study applied the pulse arc plasma gun device (ARL-300, produced by ULVAC, Inc.) with Pd electrode having diameter of 10 mm. This study set TiO2 film coated on netlike glass fiber in the vacuumed chamber of the pulse arc plasma gun device. After that, Pd electrode emitted nanosized Pd particles under the condition of the voltage of 200 V. Pd particles can be evaporated by the pulse arc plasma gun over the target area whose diameter is 100 mm under the condition of the distance between Pd electrode and the target of 160 mm. This study set the difference between Pd electrode and TiO2 film at 150 mm, resulting that we can evaporate Pd particle over TiO2 film uniformly. This study controlled the quantity of loaded Pd by pulse number. This study set the pulse number at 100. According to STEM analysis, the thickness of loaded Pd after 100 pulsation is approximately 60 nm. It is confirmed the Pd/TiO2 film prepared in this way could not be removed from the netlike glass fiber by rubbing.
This study evaluated the structural and crystal characteristics of Pd/TiO2 film by SEM (JXA-8530F, produced by JEOL Ltd.), EPMA (JXA-8530F, produced by JEOL Ltd.), STEM (JEM-ARM200F, produced by JEOL Ltd.), EDS (JEM-ARM200F, produced by JEOL Ltd.) and EELS (JEM-ARM2007 Cold, produced by JEOL Ltd.). Electron is used for analysis by these measuring instruments. Therefore, the sample must conduct electron. However, the netlike glass disc cannot conduct electron. Therefore, this study coated carbon on Pd/TiO2 whose thickness was approximately 15 nm by the dedicated device (JEC-1600, produced by JEOL Ltd.) before analysis.
The electron was emitted on the sample by the electron probe applying the acceleration voltage of 15 kV and the current of 3.0 × 10-8 A to analyze the surface structure of sample by SEM. Simultaneously, EPMA detects the characteristic X-ray. After that, we measured the concentration of chemical element referring to the relation between the characteristic X-ray energy and the atomic number. The space resolution for SEM and EPMA is 10 μm. We can understand the coating state of prepared photocatalyst as well as measure the quantity of doped metal within TiO2 film by EPMA analysis.
The electron probe emits electrons to the sample at the acceleration voltage of 200 kV, when the inner structure of the sample is analyzed by STEM. The size, thickness and structure of loaded Pu were evaluated. The X-ray characteristics of the sample is detected by EDS at the same time, so the concentration distribution of chemical element toward thickness direction of the sample is known. In the present paper, the concentration distribution of Ti, Pd and Si were analyzed.
EELS is used to detect elements as well as to determine oxidation states of transition metals. The EELS characterization was determined by JEM-ARM200F equipped with GIF Quantum having 2048 ch. The dispersion of 0.5 eV/ch for the full width at half maximum of the zero loss peak was achieved in the study.
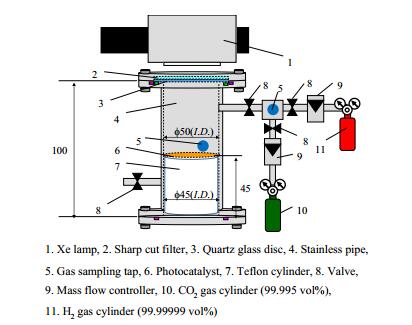
Figure 1 shows that experimental set-up of the reactor composing of stainless tube (height of 100 mm and inside diameter of 50 mm), Pd/TiO2 film coated on netlike glass disc (diameter of 50 mm and thickness of 1 mm) located on the teflon cylinder (height of 50 mm and diameter of 50 mm), a quartz glass disc (diameter of 84 mm and thickness of 10 mm), a sharp cut filter cutting off the light whose wavelength is below 400 nm (SCF – 49.5C-42L, produced by SIGMA KOKI CO. LTD.), a 150 W Xe lamp (L2175, produced by Hamamatsu Photonics K. K.), mass flow controller, gas cylinder of CO2 and H2.
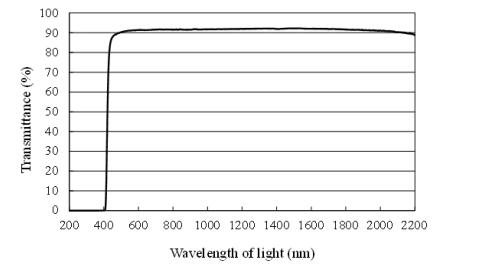
The volume of reactor to charge CO2 is 1.25 × 10-4 m3. The light of Xe lamp which is located inside the stainless tube illuminates Pd/TiO2 film coated on the netlike glass disc through the sharp cut filter and the quartz glass disc that are at the top of the stainless tube. The wavelength of light illuminating by Xe lamp is distributed from 185 nm to 2000 nm. Since a sharp cut filter can remove UV components of the light from the Xe lamp, the wavelength from Xe lamp is distributed from 401 to 2000 nm with the filter. Figure 2 displays the performance of the sharp cut filter to cut off the wavelength of light, which can prove to remove the light whose wavelength is below 400 nm. The average light intensities of Xe lamp without and with the sharp cut filter are 62.4 mW/cm2 and 40.6 mW/cm2, respectively.
CO2 gas and H2 gas whose purity were 99.995 vol% and 99.99999 vol%, respectively were controlled by mass flow controller and mixed in the buffer chamber for the CO2 reduction experiment. After that, they were introduced into the pre-vacuumed reactor using a vacuum pump. We confirmed the mixing ratio of CO2 and H2 by TCD gas chromatogragh (Micro GC CP4900, produced by GL Science) before introducing into the reactor. After confirming the mixing ratio of CO2 and H2, we injected the distilled water into the reactor via gas sampling tap and started to illuminate Xe lamp together. The injected water was changed into a vapor by the heat of Xe lamp completely. The moral ratio of CO2/H2/H2O was set at 1:0.5:0.5, 1:0.5:1, 1:1:0.5, 1:1:1, 1:2:2. The temperature in reactor rose up to 343 K within one hour and was kept at about 343 K during the experiment due to the heat of Xe lamp.
In CO2 reduction experiment, we sampled the gas in the reactor every 24 hours. We analyzed the gas samples using FID gas chromatograph (GC353B, produced by GL Science) and methanizer (MT221, produced by GD Science). FID gas chromatograph and methanizer can analyze in the minimum range of 1 ppmV.
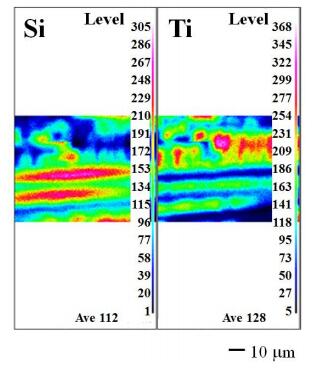
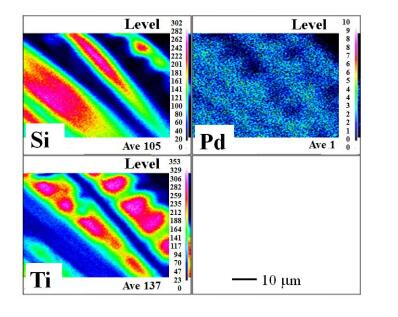
Figures 3 and 4 show EPMA results of TiO2 and Pd/TiO2 film coated on netlike glass disc, respectively. We used 1500 times magnification SEM image for EPMA. In these figures, the different colors identify the concentrations of each element in observation area. For example, light colors such as white, pink, and red display the quantity of element is large. On the other hand, dark colors such as black and blue display the quantity of element is small.
According to Figures 3 and 4, it is obvious that TiO2 film was coated on netlike glass fiber. Since the thermal conductivity is different between Ti and SiO2, which are 19.4 W/(m·K) and 1.82 W/(m·K), respectively at 600 K [37], the temperature distribution of TiO2 solution adhered on the netlike glass disc was not uniform during firing process. It can be considered that crack was formed on the TiO2 film by the thermal expansion and shrinkage around netlike glass fiber.
In addition, we can observe the uniform loading of nanosized Pd particles on TiO2 according to Figure 4.
The observation area of diameter of 300 μm which is the center of netlike glass disc is analyzed by EPMA to evaluate the quantity of loaded Pd within TiO2 film quantitatively. We estimate the ratio of Pd to Ti by averaging the data obtained. As a result, the weight percentages of Pd and Ti in Pd/TiO2 film are 0.44 wt% and 99.56 wt%, respectively.
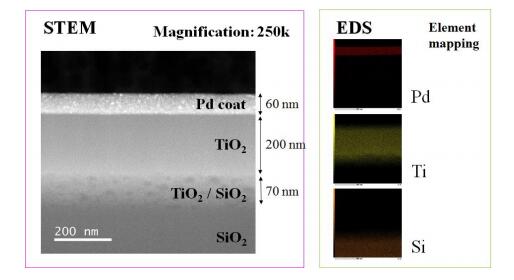
Figure 5 shows STEM and EDS results of Pd/TiO2 film coated on netlike glass disc. 250000 times magnification STEM image was used in the EDS. It is observed that Pd is coated on TiO2 film according to STEM image, which is confirmed from EDS image. It is also observed that the layers of Pd and Ti are separated. The thickness of the Pd coated is approximately 60 nm. Since the observation area is small compared to EPMA image shown in Figure 4, nano-sized Pd particle is loaded on TiO2 dispersedly.
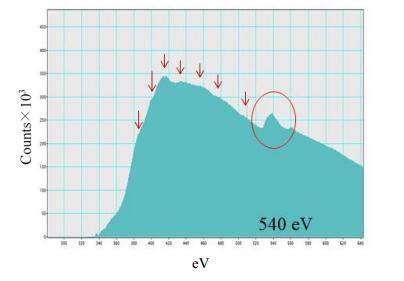
Figure 6 shows EELS spectra of Pd in Pd/TiO2 film in which peaks at around 540 eV can be observed. Compared to the spectra peaks of Pd nanowire, Pd metal and PdO in [38], the EELS spectra of Pd metal matches that in Figure 6. Therefore, it is believed that Pd in Pd/TiO2 prepared in this study exists as Pd metal. Since the photoreduction performance of Pd/TiO2 was higher than that of PdO/TiO2 [25,39], the desirable Pd/TiO2 without oxidization was proved to be prepared in this study.
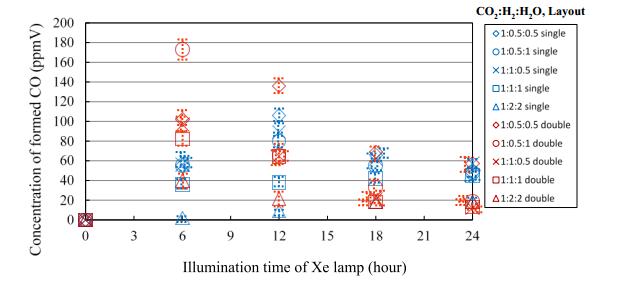
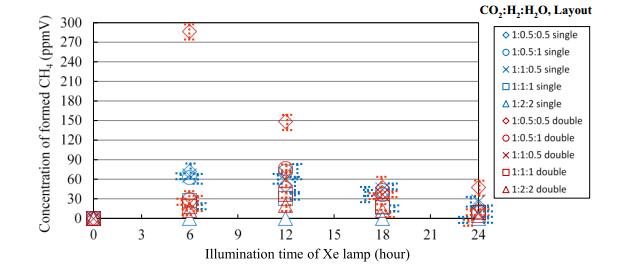
Figures 7 and 8 show the change in concentrations of formed CO and CH4 with single Pd/TiO2 film coated on netlike glass disc and overlapped Pd/TiO2 films with the time under the condition of Xe lamp illumination with UV light, respectively. The error bars in three experiments are also shown in Figures 7 and 8 to demonstrate the reproducibility of prepared Pd/TiO2, respectively. The amount of Pd/TiO2 for single film and two films are 0.05 g and 0.18 g, respectively. In this experiment, a blank test conducting the same experiment without Xe lamp illumination had been carried out as a reference, resulting that no fuel was detected as expected. Tables 1 and 2 list maximum concentration of formed CO and CH4 under the condition shown in Figures 7 and 8, respectively.
| 1:0.5:0.5 | 1:0.5:1 | 1:1:0.5 | 1:1:1 | 1:2:2 | |
| Single | 106 ppmV | 80 ppmV | 89 ppmV | 45 ppmV | 18 ppmV |
| Double | 136 ppmV | 173 ppmV | 97 ppmV | 82 ppmV | 40 ppmV |
DownLoad:
CSV
| 1:0.5:0.5 | 1:0.5:1 | 1:1:0.5 | 1:1:1 | 1:2:2 | |
| Single | 72 ppmV | 72 ppmV | 68 ppmV | 31 ppmV | 0 ppmV |
| Double | 287 ppmV | 77 ppmV | 59 ppmV | 36 ppmV | 20 ppmV |
DownLoad:
CSV
Figures 7 and 8 reveal that the concentrations of CO and CH4 for overlapped Pd/TiO2 discs are higher than those for single Pd/TiO2 disc under every moral ratio of CO2/H2/H2O. The reasons are believed to be that: (ⅰ) The amount of photocatalyst used for photocatalysis reaction is increased. (ⅱ) The electron transfer between two Pd/TiO2 films promotes the activity of photocatalysis reaction. (ⅲ) The lower positioned Pd/TiO2 disc utilizes the light passing through the top disc [36].
According to Figure 7 and Table 1, the CO2 reduction performance to produce CO is the highest at the moral ratio of CO2/H2/H2O = 1:0.5:1 with overlapped Pd/TiO2 coated netlike glass discs, of which the concentration of CO is 173 ppmV. On the other hand, according to Figure 8 and Table 2, the CO2 reduction performance to produce CH4 is the highest for the moral ratio of CO2/H2/H2O = 1:0.5:0.5 with overlapped Pd/TiO2 films coated netlike glass discs, of which the concentration of CH4 is 287 ppmV. Since the reaction scheme of CO2/H2/H2O is not fully understood, this study refers to the reaction mechanism of CO2 with H2O or H2 as shown by Eqs 1–11. It is known from the reaction mechanism that the theoretical moral ratio of CO2 with H2O or H2 to produce CO is 1:1. On the other hand, the theoretical moral ratio of with H2O or H2 to produce CH4 is 1:4. The moral ratio of CO2/H2/H2O = 1:0.5:0.5 can be identified with the moral ratio of CO2/total reductants = 1:1. According to Eqs 1–11, CO is produced first compared to CH4. In addition, it is believed that some CO might be converted into CH4 according to Eq 5. Producing CH4 needs four times H+ and electrons as many as producing CO needs. Since Pd has high reduction performance [23,24,25], it is thought that the optimum moral ratio of CO2/total reductants to produce CH4 in this study was smaller than the theoretical moral ratio required.
The optimum moral ratio to produce CO found in this study is the moral ratio of CO2/H2/H2O = 1:0.5:1 according to Figure 7 and Table 1. In addition, the second best moral ratio to produce CO is the moral ratio of CO2/H2/H2O = 1:0.5:0.5, which is the optimum moral ratio to produce CH4 according to Figure 8 and Table 2. As described above, it is believed that some CO might be converted into CH4. It is thought that more CO converts into CH4 at the moral ratio of CO2/H2/H2O = 1:0.5:0.5. Therefore, the moral ratio of CO2/H2/H2O = 1:0.5:1, which is close to the moral ratio of CO2/H2/H2O = 1:0.5:0.5, becomes the optimum moral ratio to produce CO. Additionally, the moral ratio of CO2/H2/H2O = 1:0.5:1 is superior to that of CO2/H2/H2O = 1:1:0.5 from the viewpoint of CO production though the moral ratio of CO2/total reductants is the same. Since H2 has higher reduction performance than H2O [40], the moral ratio of CO2/H2/H2O = 1:0.5:1, that would have sufficient H2 to promote the reduction reaction even less H2, would be more suitable for CO2 conversion into CO over Pd/TiO2.
Table 3 lists the selectivity of products under the condition shown in Figures 7 and 8. The selectivity is calculated using the maximum concentration of formed CO and CH4. It is revealed that the highest selectivity of CH4 production is obtained for the moral ratio of CO2/H2/H2O = 1:0.5:0.5 with overlapped Pd/TiO2 films coated netlike glass discs. Therefore, it can be concluded that the optimum moral ratio of CO2/H2/H2O is 1:0.5:0.5.
| 1:0.5:0.5 | 1:0.5:1 | 1:1:0.5 | 1:1:1 | 1:2:2 | |
| Single (CO) | 59.6 % | 52.6 % | 56.7 % | 59.2 % | 100 % |
| Single (CH4) | 40.4 % | 47.4 % | 43.3 % | 40.8 % | 0 % |
| Double (CO) | 32.2 % | 69.2 % | 62.2 % | 69.5 % | 66.7 % |
| Double (CH4) | 67.8 % | 30.8 % | 37.8 % | 30.5 % | 33.3 % |
DownLoad:
CSV
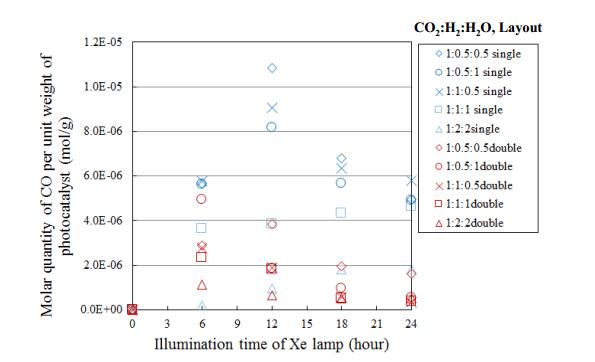
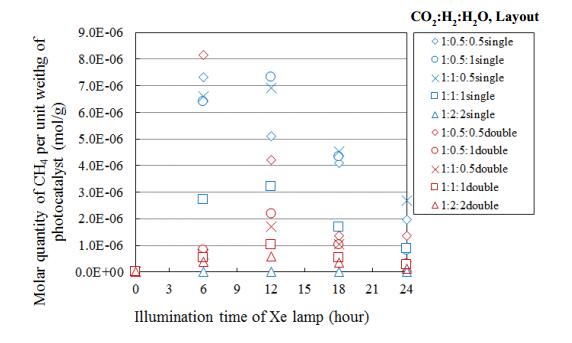
Figures 9 and 10 show the moral quantities of CO and CH4 per weight of photocatalyst with the time under the condition of the Xe lamp illumination with UV light, respectively. The moral quantities of CO and CH4 obtained with single Pd/TiO2 film and that with overlapped Pd/TiO2 are shown in Figures 9 and 10. The quantity of Pd/TiO2 for single disc and two overlapped discs are 0.05 g and 0.18 g, respectively. Since the data of formed CO and CH4 shown in Figures 9 and 10 are the same data as shown in Figures 7 and 8, respectively, the error bars are omitted in Figures 9 and 10. This study adopts the photocatalytic activity evaluation using moral quantities of product per weight of photocatalyst since this evaluation is popular in recent photocatalyst studies [41,42,43,44,45,46]. Before this experiment, a blank test conducting the same experiment without Xe lamp illumination had been carried out as a reference, in which no fuel was detected as expected.
According to Figures 9 and 10, the moral quantities of CO and CH4 per weight of photocatalyst in two Pd/TiO2 discs case overlapped are lower than those for single Pd/TiO2 disc case except for the moral ratio of CO2/H2/H2O = 1:0.5:0.5. The reason why the moral quantities of CO and CH4 per weight of photocatalyst in overlapped case are lower than those in single case is considered to be: (ⅰ) Some parts of the Pd/TiO2 film on the lower positioned disc can't receive the light. (ⅱ) If the produced fuel keeps within space between two discs, this prevents CO2, H2 and H2O from contacting the surface of photocatalyst. As a result, the photoreduction cannot progress well [36]. However, in the case of the moral ratio of CO2/H2/H2O is 1:0.5:0.5, the moral quantity of CH4 per weight of photocatalyst for overlapped case which is 8.14 μmol/g is approximately 1.1 times larger than that for single Pd/TiO2 disc. On the other hand, the moral quantity of CO per weight of photocatalyst for single Pd/TiO2 disc which is 10.8 μmol/g is approximately 2.8 times as large as that for overlapped case. Since the high reduction performance is necessary to produce CH4 via CO as mentioned above, the high CO2 reduction performance is obtained for the moral ratio of CO2/H2/H2O = 1:0.5:0.5 with two overlapped Pd/TiO2 discs.
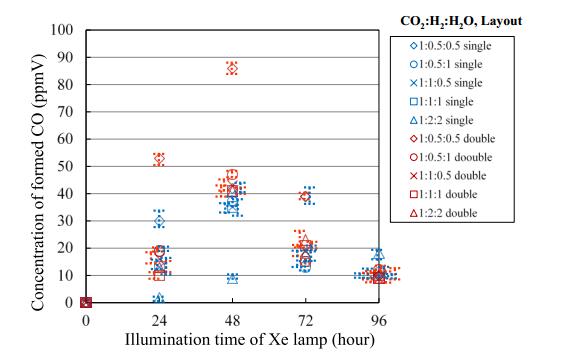
Figure 11 shows the change in concentrations of formed CO with the time under the condition of Xe lamp illumination without UV light. CO is only detected as a fuel produced from this experiment. The error bars in three experiments are also shown in Figure 10 to demonstrate the reproducibility of prepared Pd/TiO2. Before this experiment, a blank test conducting the same experiment without Xe lamp illumination had been carried out as a reference, in which no fuel was detected as expected. In addition, TiO2 did not display the CO2 reduction performance under the illumination condition of Xe lamp without UV light. The CO2 reduction performance has been realized by Pd doping under the illumination condition of Xe lamp without UV light. Table 4 lists maximum concentration of formed CO under the condition shown in Figure 11.
| 1:0.5:0.5 | 1:0.5:1 | 1:1:0.5 | 1:1:1 | 1:2:2 | |
| Single | 42 ppmV | 37 ppmV | 35 ppmV | 35 ppmV | 18 ppmV |
| Double | 86 ppmV | 47 ppmV | 43 ppmV | 41 ppmV | 41 ppmV |
DownLoad:
CSV
Figure 11 reveals that the concentrations of CO with two overlapped Pd/TiO2 discs are higher than those with single Pd/TiO2 disc under every moral ratio of CO2/H2/H2O. The same reason of this result as UV light illumination case can be considered. In addition, the CO2 reduction performance is the highest for the moral ratio of CO2/H2/H2O = 1:0.5:0.5 in this case, where the concentration of CO is 86 ppmV as shown in Table 4. Therefore, it can be concluded that the optimum moral ratio of CO2/H2/H2O is 1:0.5:0.5. It is considered that the same reaction mechanism as mentioned in the UV light illumination case is conducted. The CO2 reduction performance of Pd/TiO2 under the condition of Xe lamp illumination without UV light is lower than that under the condition of Xe lamp illumination with UV light. Therefore, it is revealed that Pd/TiO2 obtains the main photoenergy from UV light.
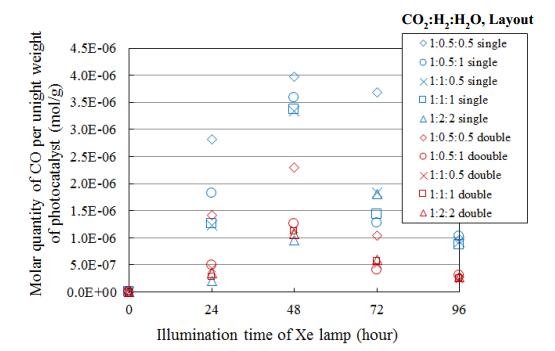
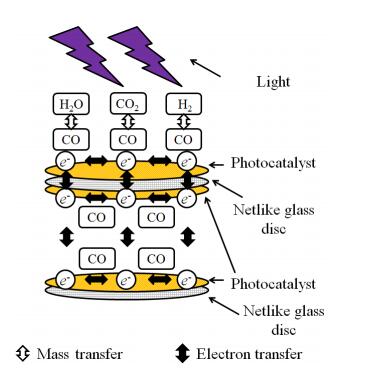
Figure 12 shows the moral quantity of CO per weight of photocatalyst with the time under the condition of Xe lamp illumination without UV light. Since the data of formed CO shown in Figure 12 are the same data as shown in Figures 11, the error bars are omitted in Figure 12. Similarly, before this experiment, a blank test conducting the same experiment without Xe lamp illumination had also been carried out as a reference, in which no fuel was detected as expected. Since TiO2 did not display the CO2 reduction performance under the illumination condition of Xe lamp without UV light. The CO2 reduction performance has been realized by Pd doping under the illumination condition of Xe lamp without UV light. It is seen that the moral quantities of CO per weight of photocatalyst with overlapped discs are lower than those with the single Pd/TiO2 disc under every moral ratio of CO2/H2/H2O. The highest moral quantity of CO per weight of photocatalyst in overlapped case is obtained under the moral ratio of CO2/H2/H2O is 1:0.5:0.5, which is 3.97 μmol/g. This is 59% of moral quantity of CO per weight of photocatalyst in single disc case. The same reasons explained in the case of illumination with UV light are thought to cause this result as well. Figure 13 displays the image of mass and electron transfer between overlapped photocatalyst discs to explain that produced fuel of CO would block the reactants of CO2, H2 and H2O to contact the surface of photocatalyst. This figure illustrates the reason for lower performance of two overlapped discs case.
In this study, the highest moral quantity of CH4 per weight of photocatalyst is 8.14 μmol/g in overlapped discs case under the condition of Xe lamp illumination with UV light. The previous studies reported the moral quantities of CH4 per weight of photocatalyst in the case of CO2/H2O with Pd/TiO2 were 25, 4.8 and 1.9 μmol/g [23,24,25]. The other study reported that the moral quantities of CH4 per weight of photocatalyst in the case of CO2/H2 with Pd/TiO2 was 356 μmol/g [26].
However, the highest moral quantity of CO per weight of photocatalyst is 10.8 μmol/g in single disc case under the condition of Xe lamp illumination with UV light in this study. The previous studies reported that the moral quantities of CO per weight of photocatalyst in the case of CO2/H2O with Pd/TiO2 were 0.12 and 0.13 mmol/g [14,15]. The other study reported that the moral quantity of CO per weight of photocatalyst in the case of CO2/H2 with Pd/TiO2 was 45 μmol/g [26].
This study also investigates the quantum efficiency which is well known as a factor to evaluate the photocatalytic activity and efficiency [47]. The quantum efficiency is calculated by the following equation [48]:
| η=(N output /N input )×100 | (12) |
| N input =(I×t×λ×A re )/(h×c) | (13) |
| N ouput =NCOMCONA+NCH4MCH4NA | (14) |
where η is the quantum efficiency (%), Ninput is the number of photon absorbed by photocatalyst (-), Noutput is the number of photon used for photocatalytic reaction (-), I is the light intensity of UV light (W/cm2), t is the UV light illumination time (s), λ is the wave length limit of light which photocatalyst absorbs for photocatalytic reaction (m), Are is the reaction surface area of photocatalyst which is assumed to be equal to one surface area of netlike glass disc (cm2), h is Plank's constant ( = 6.626 × 10-34) (J·s), c is light speed ( = 2.998 × 108) (m/s), NCO is the electron number which is necessary for forming CO of a molecular ( = 2) (-), MCO is the moral number of formed CO (mol), NA is Avogadro's number ( = 6.022 × 1023) (1/mol), NCH4 is the electron number which is necessary for forming CH4 of a molecular (-), MCH4 is the moral number of formed CH4 ( = 8) (mol). In this study, I averaged during all experiments under the condition of Xe lamp illumination with UV light and without UV light were 62.4 mW/cm2 and 40.6 mW/cm2, respectively. t under the condition of Xe lamp illumination with UV light and without UV light were 86400 s ( = 24 hours) and 345600 s ( = 96 hours).
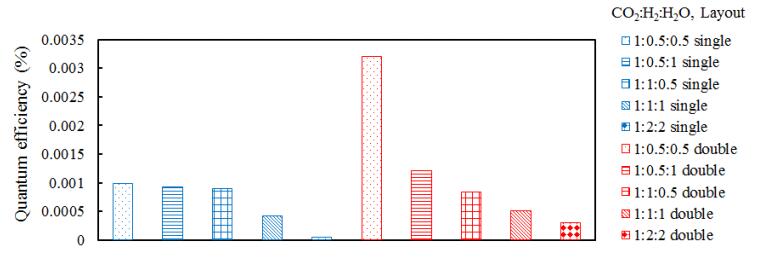
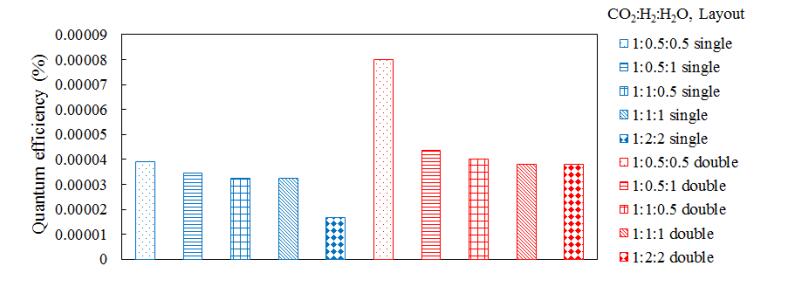
Figures 14 and 15 show the quantum efficiency among different moral ratios of CO2/H2/H2O under the condition of Xe lamp illumination with and without UV light, respectively. They revealed that the highest quantum efficiency is obtained at the moral ratio of CO2/H2/H2O = 1:0.5:0.5 with two overlapped Pd/TiO2 discs under the condition of Xe lamp illumination not only with UV light but also without UV light, which follows the results shown in Figures 8 and 11. The highest quantum efficiency under the condition of Xe lamp illumination with UV light is 3.21 × 10-3%, while that under the condition of Xe lamp illumination without UV light is 7.99 × 10-5%.
According to the comparison with the previous studies in the case of CO2/H2O or CO2/H2 mentioned above, the CO and CH4 production performance achieved in this study is higher than that reported in the case of CO2/H2O [23,24,25], while it is lower than that reported in the case of CO2/H2 [26]. Though it is thought that the doped Pd can provide the free electron not only to prevent the recombination of electron and hole produced but also to improve the light absorption effect, it was not confirmed that the CO2 reduction performance was improved significantly in this study.
This study suggests that different metals should be doped on the higher and the lower positioned photocatalysts discs to promote the CO2 reduction performance more. The co-doped TiO2 such as PbS–Cu/TiO2, Cu–Fe/TiO2, Cu–Ce/TiO2, Cu–Mn/TiO2 and Cu–CdS/TiO2 was reported to promote the CO2 reduction performance of TiO2 with H2O [4,40]. Then, the promotion of CO2 reduction performance by different metal doping is expected when the combination of CO2/H2/H2O is considered. Furthermore, the dopant such as Fe absorbing the shorter wavelength light than Pd [49] can be suggested to be used at the higher located layer. The wavelength of light extends after penetrating the higher located photocatalyst [50]. Therefore, the overlapping of the higher positioned Fe/TiO2 which absorbs the shorter wavelength light and the lower positioned Pd/TiO2 which absorbs the longer wavelength light may be an effective way for utilization of wide range light. This proposal is almost the same as the idea of hybridizing two photocatalysts which have different band gaps [10,51,52,53].
This study obtained the following conclusions based on the investigation:
The nanosized Pd particles could be loaded on TiO2 uniformly by pulse arc plasma method. Pd in Pd/TiO2 prepared by this method exists in the form of Pd metal.
With UV light, the concentrations of CO and CH4 with two overlapped Pd/TiO2 discs are higher than those with single Pd/TiO2 disc under every moral ratio of CO2/H2/H2O. The CO2 reduction performance for producing CO is the highest at the moral ratio of CO2/H2/H2O = 1:0.5:1 with two overlapped Pd/TiO2 films coated on netlike glass discs, where the concentration of CO is 173 ppmV. The CO2 reduction performance to produce CH4 is the highest at the moral ratio of CO2/H2/H2O = 1:0.5:0.5 with two overlapped Pd/TiO2 films coated on netlike glass discs, where the concentration of CH4 is 287 ppmV. In addition, the moral quantities of CO and CH4 per weight of photocatalyst in two overlapped Pd/TiO2 discs case are lower than those for single Pd/TiO2 disc case except at the moral ratio of CO2/H2/H2O = 1:0.5:0.5. When the moral ratio of CO2/H2/H2O is 1:0.5:0.5, the moral quantity of CH4 per weight of photocatalyst with two overlapped Pd/TiO2 discs which is 8.14 μmol/g is about 1.1 times larger than that with single Pd/TiO2 disc. It can be concluded that the optimum moral ratio of CO2/H2/H2O is 1:0.5:0.5.
Without UV light, the concentrations of CO with two overlapped Pd/TiO2 discs are also higher than those with single Pd/TiO2 disc under every moral ratio of CO2/H2/H2O. The CO2 reduction performance is the highest at the moral ratio of CO2/H2/H2O = 1:0.5:0.5, where the concentration of CO is 86 ppmV. Therefore, it can be concluded that the optimum moral ratio of CO2/H2/H2O is 1:0.5:0.5. In addition, the moral quantities of CO per weight of photocatalyst in two discs case are lower than those in single Pd/TiO2 disc case under every moral ratio of CO2/H2/H2O. The highest moral quantity of CO per weight of photocatalyst in two discs overlapped case is obtained under the moral ratio of CO2/H2/H2O is 1:0.5:0.5, which is 3.97 μmol/g. This is 59% of moral quantity of CO per weight of photocatalyst in single disc case.
The authors appreciate for not only JSPS KAKENHI Grant Number 16K06970 but also joint research program of the Institute of Materials and Systems for Sustainability, Nagoya University for the economic assistance.
The authors declare that this paper has no conflict of interest for the publication.
| [1] | H. A. Rothan, S. N. Byrareddy, The epidemiology and pathogenesis of coronavirus disease (COVID-19) outbreak, J. Autoimmun., (2020), 102433. |
| [2] | World Health Organization, Coronavirus disease 2019 (COVID-19): situation report-51, 2020. Available from: https://www.who.int/docs/default-source/coronaviruse/situationreports/20200311-sitrep-51-COVID-19.pdf. |
| [3] | World Health Organization, Assessment of risk factors for coronavirus disease 2019 (COVID-19) in health workers: protocol for a case-control study, 26 May 2020. Available from: https://www.who.int/publications/i/item/assessment-of-risk-factors-for-coronavirus-disease-2019-(COVID-19)-in-health-workers-protocol-for-a-case-control-study |
| [4] | A. Teslya, T. M. Pham, N. E. Godijk, M. E. Kretzschmar, M. C. Bootsma, G. Rozhnova, Impact of self-imposed prevention measures and short-term government intervention on mitigating and delaying a COVID-19 epidemic, medRxiv, (2020), 2020.03.12.20034827. |
| [5] | F. Brauer, Mathematical epidemiology: Past, present, and future, Infect. Dis. Model., 2 (2017), 113-127. |
| [6] | J. Jia, J. Ding, S. Liu, G. Liao, J. Li, B. Duan, et al., Modeling the control of COVID-19: Impact of policy interventions and meteorological factors, arXiv, (2020), 2003.02985. |
| [7] | S. S. Nadim, I. Ghosh, J. Chattopadhyay, Short-term predictions and prevention strategies for COVID-2019: A model based study, arXiv, (2020), 2003.08150. |
| [8] | B. Tang, X. Wang, Q. Li, N. L. Bragazzi, S. Tang, Y. Xiao, et al., Estimation of the transmission risk of the 2019-ncov and its implication for public health interventions, J. Clin. Med., 9 (2020), 462. |
| [9] |
C. Yang, J. Wang, A mathematical model for the novel coronavirus epidemic in Wuhan, China, Math. Biosci. Eng., 17 (2020), 2708-2724. doi: 10.3934/mbe.2020148
|
| [10] | Y. Liu, A. A. Gayle, A. Wilder-Smith, J. Rocklöv, The reproductive number of COVID-19 is higher compared to SARS coronavirus, J. Travel. Med., 27 (2020), taaa021. |
| [11] |
G. Kampf, D. Todt, S. Pfaender, E. Steinmann, Persistence of coronaviruses on inanimate surfaces and their inactivation with biocidal agents, J. Hosp. Infect., 104 (2020), 246-251. doi: 10.1016/j.jhin.2020.01.022
|
| [12] | H. W. Hethcote, The mathematics of infectious diseases, SIAM Rev. Soc. Ind. Appl. Math., 42 (2000), 599-653. |
| [13] | O. Diekmann, J. A. P. Heesterbeek, J. A. Metz, On the definition and the computation of the basic reproduction ratio r 0 in models for infectious diseases in heterogeneous populations, J. Math. Biol., 28 (1990), 365-382. |
| [14] |
P. Van den Driessche, J. Watmough, Reproduction numbers and sub-threshold endemic equilibria for compartmental models of disease transmission, Math. Biosci., 180 (2002), 29-48. doi: 10.1016/S0025-5564(02)00108-6
|
| [15] | J. A. Backer, D. Klinkenberg, J. Wallinga, Incubation period of 2019 novel coronavirus (2019-ncov) infections among travellers from Wuhan, China, 20-28 january 2020, Euro. Surveil., 25 (2020), 2000062. |
| [16] | Secretary of Health, Aviso epidemiológico: casos de infección respiratoria asociados a nuevo-coronavirus-2019-ncov, 2020. Available from: https://www.gob.mx/salud/documentos/avisoepidemiologico-casos-de-infeccion-respiratoria-asociados-a-nuevo-coronavirus-2019-ncov. |
| [17] |
J. A. Christen, C. Fox, A general purpose sampling algorithm for continuous distributions (the t-walk), Bayesian Anal., 5 (2010), 263-281. doi: 10.1214/10-BA603
|
| [18] | El Financiero, Al 10% de los casos sospechosos de COVID-19 con síntomas leves se les aplica prueba: Imss, 2020. Available from: https://www.elfinanciero.com.mx/nacional/al-10-de-loscasos-sospechosos-de-covid-19-con-sintomas-leves-se-les-aplica-prueba-imss. |
| [19] |
E. Shim, A. Tariq, W. Choi, Y. Lee, G. Chowell, Transmission potential and severity of COVID-19 in South Korea, Int. J. Infect. Dis., 93 (2020), 339-344. doi: 10.1016/j.ijid.2020.03.031
|
| [20] | A. Kuzdeuov, D. Baimukashev, A. Karabay, B. Ibragimov, A. Mirzakhmetov, M. Nurpeiissov, et al., A network-based stochastic epidemic simulator: Controlling COVID-19 with region-specific policies, medRxiv, (2020), 2020.05.02.20089136v1. |
| [21] | J. Herman, W. Usher, SALib: An open-source python library for sensitivity analysis, J. Open Res. Softw., 2 (2017), 97. |
| 1. | Akira Nishimura, Tadaaki Inoue, Yoshito Sakakibara, Masafumi Hirota, Akira Koshio, Eric Hu, Impact of Pd Loading on CO2 Reduction Performance over Pd/TiO2 with H2 and H2O, 2020, 25, 1420-3049, 1468, 10.3390/molecules25061468 | |
| 2. | Akira Nishimura, Ryouga Shimada, Yoshito Sakakibara, Akira Koshio, Eric Hu, Comparison of CO2 Reduction Performance with NH3 and H2O between Cu/TiO2 and Pd/TiO2, 2021, 26, 1420-3049, 2904, 10.3390/molecules26102904 | |
| 3. | Akira Nishimura, Yoshito Sakakibara, Akira Koshio, Eric Hu, The Impact of Amount of Cu on CO2 Reduction Performance of Cu/TiO2 with NH3 and H2O, 2021, 11, 2073-4344, 610, 10.3390/catal11050610 |
Figures(10) / Tables(1)
Fernando Saldaña, Hugo Flores-Arguedas, José Ariel Camacho-Gutiérrez, Ignacio Barradas. Modeling the transmission dynamics and the impact of the control interventions for the COVID-19 epidemic outbreak[J]. Mathematical Biosciences and Engineering, 2020, 17(4): 4165-4183. doi: 10.3934/mbe.2020231
| 1:0.5:0.5 | 1:0.5:1 | 1:1:0.5 | 1:1:1 | 1:2:2 | |
| Single | 106 ppmV | 80 ppmV | 89 ppmV | 45 ppmV | 18 ppmV |
| Double | 136 ppmV | 173 ppmV | 97 ppmV | 82 ppmV | 40 ppmV |
DownLoad:
CSV
| 1:0.5:0.5 | 1:0.5:1 | 1:1:0.5 | 1:1:1 | 1:2:2 | |
| Single | 72 ppmV | 72 ppmV | 68 ppmV | 31 ppmV | 0 ppmV |
| Double | 287 ppmV | 77 ppmV | 59 ppmV | 36 ppmV | 20 ppmV |
DownLoad:
CSV
| 1:0.5:0.5 | 1:0.5:1 | 1:1:0.5 | 1:1:1 | 1:2:2 | |
| Single (CO) | 59.6 % | 52.6 % | 56.7 % | 59.2 % | 100 % |
| Single (CH4) | 40.4 % | 47.4 % | 43.3 % | 40.8 % | 0 % |
| Double (CO) | 32.2 % | 69.2 % | 62.2 % | 69.5 % | 66.7 % |
| Double (CH4) | 67.8 % | 30.8 % | 37.8 % | 30.5 % | 33.3 % |
DownLoad:
CSV
| 1:0.5:0.5 | 1:0.5:1 | 1:1:0.5 | 1:1:1 | 1:2:2 | |
| Single | 42 ppmV | 37 ppmV | 35 ppmV | 35 ppmV | 18 ppmV |
| Double | 86 ppmV | 47 ppmV | 43 ppmV | 41 ppmV | 41 ppmV |
DownLoad:
CSV
| 1:0.5:0.5 | 1:0.5:1 | 1:1:0.5 | 1:1:1 | 1:2:2 | |
| Single | 106 ppmV | 80 ppmV | 89 ppmV | 45 ppmV | 18 ppmV |
| Double | 136 ppmV | 173 ppmV | 97 ppmV | 82 ppmV | 40 ppmV |
| 1:0.5:0.5 | 1:0.5:1 | 1:1:0.5 | 1:1:1 | 1:2:2 | |
| Single | 72 ppmV | 72 ppmV | 68 ppmV | 31 ppmV | 0 ppmV |
| Double | 287 ppmV | 77 ppmV | 59 ppmV | 36 ppmV | 20 ppmV |
| 1:0.5:0.5 | 1:0.5:1 | 1:1:0.5 | 1:1:1 | 1:2:2 | |
| Single (CO) | 59.6 % | 52.6 % | 56.7 % | 59.2 % | 100 % |
| Single (CH4) | 40.4 % | 47.4 % | 43.3 % | 40.8 % | 0 % |
| Double (CO) | 32.2 % | 69.2 % | 62.2 % | 69.5 % | 66.7 % |
| Double (CH4) | 67.8 % | 30.8 % | 37.8 % | 30.5 % | 33.3 % |
| 1:0.5:0.5 | 1:0.5:1 | 1:1:0.5 | 1:1:1 | 1:2:2 | |
| Single | 42 ppmV | 37 ppmV | 35 ppmV | 35 ppmV | 18 ppmV |
| Double | 86 ppmV | 47 ppmV | 43 ppmV | 41 ppmV | 41 ppmV |
