Double-integrator consensus for a switching network without dwell time

1.   Introduction

The multi-agent system(MAS) consensus problem has attracted great interest in recent years ^{[1,2,3,4,5,6,7,8]}. An MAS is composed of a group of agents, which interacts with each other to complete the complex task that a single agent cannot complete. The control method of MASs roughly fall into two categories, one is centralized control and the other is distributed control. In distributed control, each agent independently analyzes the information obtained from its neighbors ^[5,9].

The interaction assumptions influence the convergence of the dynamics. The most common assumption of the communication is the static communication assumption which requires that the connection among agents to be constant ^[3]. Since the network connection may be broken or switched due to the failure of the connection or cyberattacks ^[10], some loose assumptions are discussed in literature, such as the assumptions of a periodic connection and uniform joint connection ^[11,12]. The uniformly jointly connected assumption allows the agents to be completely disconnected and the dwell-time constraint is usually needed simultaneously.

The control in a switching network will create a switched closed-loop system which is usually associated with higher complexity ^[13]. Analysis tools such as the generalized LaSalle invariance principle ^[14], the small-gain theorem ^[15], non-smooth analysis ^[16], LaSalle's invariance principle ^[17] and the generalized Barbalat's lemma ^[12] have been developed to handle the stability of the closed-loop system.

The dwell-time assumption requires that the connection among the agents remain unchanged for a period of time; it makes the Lyapunov function technique an effective method to manage stability problems ^[18,19,20]. However, in the real world, the communication connection often changes instantaneously. The change is characterized by frequent occurrence, disorder and randomness which is inconsistent with the dwell time assumption.

Given the numerous applications in engineering such as manipulator motion, spacecraft rotation and rotary crane motion, double-integrator dynamics is of the most fundamental topics in control theory ^{[21,22,23,24,25,26]}. For instance, in ^[23], a networked permanent-magnet synchronous motor speed-regulation system is written as a double-integrator system. Previous work has demonstrated that the agents with double integrator dynamics are meaningful in both theory and application ^[3]. In ^[27], the double integrator consensus problem is solved for a strongly connected network. In ^[28], under the static network assumption, the consensus is solved by applying a distributed fixed-time protocol. In ^[17], an extension of LaSalle's invariance principle is proposed and used to solve the consensus problem of MASs with double-integrator dynamics for a jointly connected network with a dwell time. When there is no dwell time, since the general stability analysis techniques presented in ^[17,27,28] are no longer valid, the process of stability analysis is challenging, and the controller may be invalid for the double integrator consensus. In ^[29], the consensus with a short dwell time is studied through the use of the multiple Lyapunov function technique. A question naturally arises as to whether the controller design is still valid for the switching network if the dwell-time assumption is broken. New methods should be adopted to investigate the control problem.

Motivated by this situation, to solve the leader-follower double integrator consensus problem under the conditions of a relatively loose connection and to cut the connection cost, we consider switching networks without dwell time in this paper. The novelty of this work is that we adopt a new network assumption in the consensus problem that can adapt to a relatively loose connection and lower connection cost. The connection can be switched infinitely frequently in a finite time interval. Additionally, the control problem can be solved without a dwell time which is often adopted in current works. The main contributions of this paper are listed as follows.

First, we extend the study on the consensus problem to generalized jointly connected switching networks. For comparison with the results of a static network ^[28] or switching network with dwell time ^[17,29], we remove the dwell time assumption. Allowing the instantaneous change of communication connection makes the stability analysis much more challenging. We prove that the controller can be robust against the switching network.

Second, when considering the fast switching communication network topology, the common Lyapunov function techniques based on the system trajectory such as that in ^[17] are invalid. No strict negative Lyapunov function such as that in ^[27] or the use of multiple Lyapunov functions such as in ^[29] can be found. To overcome the difficulty in analyzing the stability of closed-loop systems, a virtual output method and the newly developed generalized Krasovskii-LaSalle theorem are applied by using a limiting zeroing-output solution to describe the stable states set.

Finally, we compare our result with the stability result of ^[30] which was derived with dwell time in a switching network; the advantage of our result is that stability is improved to uniformly globally exponential stability when subjected to the fast switching signals.

The rest of this paper is organized as follows. In section 2, we recall some concepts and introduce the consensus problem. In section 3, we prove the stability of the closed-loop system. A numerical example is proposed in section 4. Finally, we present the conclusions and discuss the future work.

Notations $\mathbb{R}^p$ stands for $p$ dimensional Euclidean space and $\mathbb{R}^{p\times q}$ denotes the set of all $p\times q$ matrices with real entries. $\Vert u \Vert$ is the Euclidean norm of a vector $u$. $I_n$ is the $n \times n$ identity matrix and $\otimes$ denotes the Kronecker product operator of two matrices. A scalar continuous strictly increasing function $f$ is said to be a class $K_{\infty}$ function if it satisfies $f:[0, +\infty)\rightarrow [0, +\infty)$ and $f(0) = 0$, $f(x)\rightarrow +\infty$ as $x\rightarrow +\infty$.

2.   Preliminaries and problem formulation

We review some concepts for switched systems. See ^[31] for more details. Consider the following switched linear system

where ${x} \in \mathbb{R}^p$ is the state and ${y}\in \mathbb{R}^q$ is the output.

Denote the switching signal as $\sigma:\; \mathbb{R}_{\geq 0}\rightarrow \Lambda$ where $\Lambda$ is a finite index set. For all $\lambda \in \Lambda$, $\mathbf{A}_{\lambda }, \tilde{\mathbf{A}}_{\lambda }\in \mathbb{R}^{p\times p}$ and $\mathbf{C}_{\lambda }\in \mathbb{R}^{q\times p}$.

Definition 1. If there is a continuous function $\rho: [0, \infty) \mapsto [0, \infty)$ with $\rho(0) = 0$ satisfying $\Vert \tilde{\mathbf{A}}_{\lambda}{x} \Vert \leq \rho\left(\Vert \mathbf{C}_{\lambda}{x}\Vert\right)$ for any $\lambda \in \Lambda$, then System (2.1) is said to be in the output-injection form.

Denote the set of all forward complete solution pairs $({x}, \sigma)$ as $\Phi(\Theta)$ where $\sigma \in \Theta$ and $\Theta$ is the set of all possible switching signals :

for $x\in \mathbb{X}$.

Definition 2. If there exists $\{t_n\} \subseteq [0, \infty)$ with $t_n \geq 2n$ and $\{({w}_n, \sigma_n)\}\subseteq \Phi(\Theta)$ such that 1) $\{{w}_n(\cdot+t_n):\; [-n, n] \mapsto \mathbb{R}^p \}$ converges uniformly to $\bar{{w}}$ on every compact subset of $\mathbb{R}$ and 2) $\lim_{n\rightarrow \infty} \textbf{C}_{\sigma_n(t+t_n)} \bar{{{w}}}(t) = 0$ for almost all $t\in \mathbb{R}$, then $\bar{{{w}}}(t)$ is said to be a limiting zeroing-output solution of (2.1) w.r.t. $\Phi(\Theta)$.

The limiting zeroing-output solution satisfies the following system: for all $t_n \geq 2n$,

Definition 3. If every bounded limiting zeroing-output solution $\bar{{w}}: \mathbb{R} \mapsto \mathbb{R}^p$ of system (2.1) satisfies $\inf _{t \in \mathbb{R}}\Vert\bar{{w}}(t)\Vert = 0,$ then System (2.1) is said to be weakly zero-state detectable w.r.t. $\Theta$.

The stability concepts of System (2.1) are listed as follows:

Definition 4. The origin of System (2.1) is said to be uniformly globally stable w.r.t. $\Phi(\Theta)$ if there is a class $K_{\infty}$ function $\vartheta$ such that, for any $(x, \sigma) \in \Phi(\Theta)$ and any $0 < s < t$, $\Vert x(t)\Vert \leq \vartheta(\Vert x(s)\Vert).$

Definition 5. The origin of System (2.1) is said to be uniformly globally exponentially stable (UGES) w.r.t. $\Phi(\Theta)$ if there are $a > 0$ and $b > 0$ for any $(x, \sigma)\in \Phi(\Theta)$ and any $0 < s < t$, the following inequality holds,

In this paper, the uniformly globally stable, UGES and limiting zeroing-output solutions are all with respect to $\Phi(\Theta)$ and the terminology of weakly zero-state detectable is with respect to $\Theta$. For convenience and simplicity, we omit ''w.r.t.'' in the remainder of this paper. The generalized Krasovskii-LaSalle theorem ^[31] is as follows.

Lemma 1. ^[31] Consider $\sigma \in \Theta$ and System (2.1) in the output injection form. If there is a continuous function $\mu:\; [0, \infty) \mapsto [0, \infty)$ satisfying the following conditions, then the origin of System (2.1) is UGES:

(a) The origin is uniformly globally stable;

(b) $\int_{s}^{+\infty} \Vert {\textbf{C}}_{\sigma(\tau)}{{x}}(\tau) \Vert ^2 \mathrm{d} \tau \leq \mu(\Vert{{x}}(s)\Vert)$ for all $s \geq 0$;

(c) System (2.1) is weakly zero-state detectable.

We also need the following lemma in the analysis.

Lemma 2. ^[14] Consider three functions $\phi_{i}:[s, +\infty) \rightarrow \mathbb{R}^+$, $i = 1, 2, 3$, for some $s \in \mathbb{R}^+$.Suppose that $\phi_{1}$ and $\phi_{2}$ are both continuous, and that $\phi_{3}$ is Lebesgue integrable. If

for all $t \in[s, +\infty)$, then,

and

hold, where $\phi_{4}(s) \triangleq \int_{s}^{+\infty} \phi_{3}(\tau) \mathrm{d} \tau$ and $\phi_{5}(s) \triangleq \phi_{4}(s) \exp \left(\phi_{4}(s)\right)$.

In this paper, we consider an MAS consisting of $N$ agents and a leader system. Let $\bar{\mathcal{V}} = \{0, 1, \dots, N\}$ and $\mathcal{V} = \{1, \dots, N\}$. The node $0$ represents the leader system and the node $i$ represents the $i$-th agent. Each agent $i$ has the following double-integrator dynamics:

where $x_{i} = [x_{1i}, x_{2i}]^{\top} \in \mathbb{R}^{2}$ represents the states of agent $i$ and and $u_{i} \in \mathbb{R}$ represents the control input.

The leader follower consensus problem is to find suitable control $u_{i}$ for each agent $i\in \mathcal{V}$ to follow the leader system with the following dynamics:

where $v = [v_1, v_2]^\top$ is the state and archives $\lim _{t \rightarrow \infty}\left(x_{ji}(t)-v_{j}(t)\right) = 0$ for all $j = 1, 2$ and $i \in \mathcal{V}$.

Remark 1. The dynamics of the agents and the leader are all of double integrator type. So the consensus problem is stated as double-integrator consensus. The breadth application of this model is very wide, and it is a basic model used in mechanical engineering, electronics and other fields. Here, the consensus problem is solved with a typical controller when the communications among the agents can be broken at any time or switched at any frequency.

For any switching signal $\sigma\in \Sigma$ and any $\tau > 0$, over a time interval $[t_1, t_2)$, the $\tau$-joint graphs of $\bar{\mathcal{G}}_{\sigma(t)}$ and ${\mathcal{G}}_{\sigma(t)}$ are defined as $\bar{\mathcal{G}}^{\tau}_{\sigma}([t_1, t_2)) = (\bar{\mathcal {V}}, \bigcup_{\zeta \in \sigma_{\tau} [t_1, t_2)} \bar{\mathcal {E}}_{\zeta})$ and ${\mathcal{G}}^{\tau}_{\sigma}([t_1, t_2)) = ({\mathcal {V}}, \bigcup_{\zeta \in \sigma_{\tau} [t_1, t_2)} {\mathcal {E}}_{\zeta}),$ respectively, where $\sigma_{\tau} [t_1, t_2) = \{\zeta\in \Lambda:\int_{t_1}^{t_2}\delta^{\zeta}(t) \mathrm{d} t\geq \tau \}$ with $\delta^{\zeta}(\tau) = 1$ if $\sigma(\tau) = \zeta$ and $\delta^{\zeta}(\tau) = 0$ if $\sigma(\tau)\neq\zeta$.

Let $\mathcal{A}_{\lambda} = [a_{ij}^{\lambda}]_{i, j = 1}^{N}\in\mathbb{R}^{N\times N}$ and $\bar{\mathcal{A}}_{\lambda} = [a_{ij}^{\lambda}]_{i, j = 0}^{N}\in\mathbb{R}^{(N+1)\times (N+1)}$ be weighted adjacency matrices of $\mathcal{G}_{\lambda}$ and $\bar{\mathcal{G}}_{\lambda}$, respectively. Let $\mathcal{L}_{\lambda}\in\mathbb{R}^{N\times N}$ be the associated Laplacian of $\mathcal{G}_{\lambda}$ and denote $\mathcal{H}_{\lambda} = \mathcal{L}_{\lambda}+\hbox{diag}[a_{10}^{\lambda}, \dots, a_{N0}^{\lambda}]$. We assume that for all $\lambda\in\Lambda$, the graph ${\mathcal{G}}_\lambda$ is undirected. Then, $\mathcal{L}_{\lambda}$ and $\mathcal{H}_{\lambda}$ are both symmetric.

We consider the consensus problem under the following generalized uniformly jointly connected (GUJC) condition without any dwell-time constraints.

Assumption 1. ^[31]There is a constant pair $(\tau, T)$, $T\geq \tau > 0$, for all $\sigma\in \Theta$ and any $t \geq 0$; then, the $\tau$-joint graph $\bar{\mathcal{G}}^{\tau}_{\sigma}([t, t+T))$ contains a spanning tree with the node $0$ as the root.

To make a comparison between the network assumptions, the uniformly jointly connected (UJC) condition is stated as follows.

Assumption 2. ^[12]There is a sequence $\{0 = t_0 < t_1 < t_2 < \dots\}\subset \mathcal{R}^+$ with $t_{i+1}-t_i < \upsilon$ for some $\upsilon > 0$, then the joint graph $({\mathcal{V}, \bigcup_{t\in [t_i, t_{i+1})}})$ contains a spanning tree with the node $0$ as the root.

The following dwell time assumption is usually adopted when the switching topology satisfies the UJC condition.

Assumption 3. ^[32]There exists a constant $\tau > 0$ and a strictly increasing sequence $\{0 = t_0 < t_1 < t_2 < \dots\}\subset \mathcal{R}^+$, such that $t_{i+1}-t_{i}\geq \tau$ the switching signal $\sigma(t_{i+1})\neq \sigma(t_{i})$ and $\sigma(s) = \sigma(t_{i})$ for every $t_{i}\leq s \leq t_{i+1}$.

Remark 2. Assumption 1 is a strictly more weakly connected condition than the UJC condition combined with dwell time. In particular, it does not need to meet the dwell time constraint, which requires the connection graph to include a fixed time interval. Since it uses the characteristic function in $\sigma_{\tau} [t_1, t_2)$, the network can be switched at any frequency within the a zero measure time subset of $[t_1, t_2)$. For example, if a network switches at the rate of any rational number within a time interval $[t_1, t_2)$, the switching network cannot be modeled by applying the UJC condition with the dwell-time assumption.

3.   Main results

In this paper, we assume that every agent $i$ knows the information of its neighbors $j\in \mathcal{N}(i)$ in the graph. We adopt the following control law for system $i \in \mathcal{V}$

where $\eta_{i} = [\eta_{1i}, \eta_{2i}]^{\top}\in \mathbb{R}^2$, $k_1 > 0$ and $k_2 > 0$ are to be determined later, $E = \left[ {\begin{array}{*{20}{c}} 0 & 1\\ 0 & 0 \end{array}} \right]$, $\eta_0 = v$ and $\mu$ is an arbitrary positive constant.

Remark 3 Controller (3.1) comes from Controller (4) in ^[30] on distributed dynamic state feedback. $\eta_{i}$ is a dynamic distributed observer of the state of $i$-th system. Only the neighbors of the leader system can use the state information of the leader system. Thus, we set $\eta_0 = v$. We will prove that the control law is strongly robust against various unreliable communication networks that switch arbitrarily fast.

Let $e_{li} = x_{li}-v_{l}$ and $\tilde{e}_{li} = \eta_{li}-v_{l}, l = 1, 2$. Moreover, let $e = [e_{11}, e_{21}, e_{12}, e_{22}\cdots e_{N1}, e_{N2}]^\top$, and $\tilde{e} = [\tilde{e}_{11}, \tilde{e}_{21}, \tilde{e}_{12}, \tilde{e}_{22}\cdots \tilde{e}_{N1}, \tilde{e}_{N2}]^\top$. One can see that $\lim _{t \rightarrow \infty}e(t) = 0$ if and only if $\lim _{t \rightarrow \infty}\left(x_{ji}(t)-v_{j}(t)\right) = 0$ for all $j = 1, 2$ and $i \in \mathcal{V}$. Now the compact form of the closed loop switched system can be written as

where $M_1 = \left[ {\begin{array}{*{20}{c}} 0 & 1\\ -k_1 & -k_2 \end{array}} \right]$ and $M_2 = \left[ {\begin{array}{*{20}{c}} 0 & 0\\ k_1&k_2 \end{array}} \right].$ The main result of this paper is that the leader-follower consensus can be achieved by the distributed controller given by (3.1). The main theorem is given as follows.

Theorem 1. Under Assumption 1, the origin of the closed-loop system described by (3.2) is UGES. The consensus problemis solvable by applying the distributed switching controller given by (3.1).

Proof.

First, we prove that the origin of Subsystem (3.2a) is UGES.

We use the following coordinate transform.

Let $\zeta(t) = (I_N \otimes e^{-Et})\tilde{e}(t)$. Then,

Moreover, the coordinate transformation does not change the origin. We now turn to prove that the origin of (3.3) is UGES. We define a virtual output $Y = \sqrt{ \mathcal{H}_{\sigma }\otimes I_2}\zeta$ for System (3.3). Then, let

and select $\rho(x) = x^2$; then, System (3.3) can be put into the output-injection form presented in (2.1).

Let $V = \zeta^\top\zeta$. Then, $V$ is positive definite. And we have

which means that the origin of System (3.3) is uniformly globally stable. Condition (a) in Lemma 1 is then satisfied. Let $\phi_1(t) = V(t)$, $\phi_2(t) = \sqrt{ \mathcal{H}_{\sigma(t) }\otimes I_2}\zeta(t)$ and $\phi_3(t) = 0$. Then, by Lemma 2, we have that

for any $s\geq 0$. Thus, Condition (b) in Lemma 1 is then satisfied.

Next, we assume that $\bar{ \zeta}:\mathbb{R} \rightarrow \mathbb{R}^{2N}$ is any bounded limiting zeroing-output solution of System (3.3); then, there exist $\left\{t_{n}\right\} \subseteq \mathbb{R} ^{+}$ and $\left\{\lambda_{n}\right\} \subseteq \Theta$ with $t_{n} \geq 2 n$ such that

for almost all $t$ in $\mathbb{R}$ and

Thus, by (3.8), we have that $\bar{\zeta} = \bar{\zeta}(0).$ Moreover, by (3.7), we obtain

for almost all $t$ in $\mathbb{R}$. Thus, we have

for almost all $t$ in $\mathbb{R}$. Thus, there is $c_{\zeta}\in \mathbb{R}^{2N}$ such that $\bar{\zeta}(0) = c_{\zeta}$ and $\lim _{n \rightarrow \infty} (\mathcal{H}_{\sigma(t+t_n) }\otimes I_2)c_{\zeta} = 0.$

We turn to prove that $c_{\zeta} = 0.$ Under Assumption 1, there is a spanning tree of the $\tau$-joint graph $\bar{\mathcal{G}}^{\tau}_{\sigma}([t, t+T))$ with the node $0$ as the root. From [31, Lemma 3], there exists $\epsilon_1 > 0$ such that for any unit vector $u \in \mathbb{R}^{N}$ and all $\sigma \in \Theta$, the inequality $u^{\top}\left[\int_{t}^{t+T}(\mathcal{H}_{\sigma(\tau)} \otimes I_2) \mathrm{d} \tau\right] u \geq \epsilon_1$ for all $t \geq 0$. If $c_{\zeta}\neq 0$, we obtain that

reaching a contradiction. Thus, System (3.3) is weakly zero-state detectable. Condition (c) in Lemma 1 is satisfied. By Theorem 1, we have proved that the origin of system (3.3) is UGES, thus, the origin of Subsystem (3.2a) is UGES.

Second, we prove that the origin of the whole system given by (3.2) is UGES.

Select $k_1$ and $k_2$ such that $M_1$ is a Hurwitz matrix, there is a symmetric positive definite matrix $P$, such that $M_3 = M_1^{\top}P+PM_1 < 0$. Define an output as $Y_1 = [(\sqrt{-I_N \otimes M_3}e)^\top, \tilde{e}^\top]^\top.$ Then, let

and

Since

we have

for some $\alpha > 0$. In other words, System (3.2) can be put into the output-injection form given by (2.1). Let $V_1 = e^\top (I_N \otimes P) e$. Then,

Since in the first step, we have proved that the origin of Subsystem (3.2a) is UGES, there is $a > 0$, $b > 0$ such that $\Vert \tilde{e} \Vert < a e^{-b(t-s)}\Vert \tilde{e}(s) \Vert$ for any $t > s > 0$. Thus, by using the fact that $\Vert AB\Vert\leq \Vert A\Vert \Vert B\Vert$ for any proper matrices $A$ and $B$ and $2x\leq 1+x^2$ for any $x \in \mathbb{R}$, we have

Let $\beta(t) = 1/2\Vert I_N \otimes (M_2P+PM_2^\top)\Vert a e^{-b(t-s)}\Vert \tilde{e}(s) \Vert$; then, we have $\int_{s}^{+\infty}\beta(\tau) < \infty.$ Therefore,

Let $\phi_1(t) = V_1(t)$, $\phi_2(t) = e^\top(t)[I_N \otimes (-M_3)]e(t)$ and $\phi_3(t) = \beta(t)$. By Lemma 2, there is a proper $a_1 > 0$ and $b_1 > 0$, we have that $V_1\leq e^{a_1\beta(s)}(1+b_1\Vert e(s)\Vert^2)$, $\Vert e(t)\Vert\leq e^{1/2a_1\beta(s)}(1+b_1\Vert e(s)\Vert)$ and then

which means that the origin of System (3.2) is uniformly globally stable. Condition (a) in Lemma 1 is then satisfied.

Applying Lemma 2 also gives

Thus, Condition (b) in Lemma 1 is satisfied.

Next, we assume that $[\bar{e}^\top, \bar{\tilde{e}}^\top]^\top:\mathbb{R} \rightarrow \mathbb{R}^{4N}$ is any bounded limiting zeroing-output solution of System (3.2); then, there exist $\left\{t_{n}\right\} \subseteq \mathbb{R} ^{+}$and $\left\{\lambda_{n}\right\} \subseteq \Theta$ with $t_{n} \geq 2 n$ such that

for almost all $t$ in $\mathbb{R}$ and

By (3.23), we have

By (3.22), since $\sqrt{-I_N \otimes M_3}$ is invertible, we have

for almost all $t$ in $\mathbb{R}$.

Thus, (3.24) and (3.25) imply that

which means that System (3.2) is weakly zero-state detectable. Thus, Condition (c) in Lemma 1 is then satisfied. Then, by Theorem 1, the origin of System (3.2) is UGES.

Remark 4. We have proved that the origin of System (3.3) is UGES. It means that $\lim _{t \rightarrow \infty}e(t) = 0$ holds exponentially and globally. Thus, by the definition of $e(t)$, the consensus problem is solved by Controller (3.1). Controller (3.1) is a typical controller with distributed dynamic state feedback. We improve the stability result to exponentially stable. The control law is proved to be strongly robust against various unreliable communication network switch arbitrarily fast.

Remark 5. When $\Lambda$ is a singleton, Assumption 1 reduces to the static graph case. When $\bar{\mathcal{G}}_{\lambda}$ is UJC with dwell time, the problem in ^[17] can be viewed as a special case of our main results. To show the stability of a closed-loop system with dwell time, it is possible to use the information of system trajectory to find a common joint Lyapunov function ^[17,33] or a strict Lyapunov function and adopt the classical Lyapunov analysis method. In the proof of Theorem 1, $V$ is not a strict Lyapunov function for Subsystem (3.2a) and $V_1$ is not likely to qualify as a Lyapunov function for System (3.2) since we do not know whether $\dot{V}_1\leq 0$ holds. This situation makes the stability analysis much more challenging. If there is no dwell time, only the UJC network topology is assumed; then, the very fast switching in the network will make the trajectory incomputable and the techniques that rely on the trajectory cannot be used. Hence, the MAS will lose controllability in the fast switching moments. Thus, it is a difficult task to check the stability of the closed-loop system. However, a two-step recursive stability analysis method can be applied to overcome the technical difficulty with the cascaded structure of System (3.2).

4.   Simulation

An example of nonholonomic mobile robots is provided to illustrate the effectiveness of the theoretical results. Unlike the static network results such as those in ^[34], the network here is switching.

In ^[30], a nonholonomic mobile robots control problem is written as follows:

where $(x_i, y_i)$ is the position and $\theta_i$ is orientation of the robot center. Additionally, $\nu_i$ and $\omega_i$ respectively represent the linear and angular velocities. $m_i$ is the mass. $J_i$ is the mass moment of inertia. $\tilde{u}_i = \left[f_i, \tau_i\right]^T$ is the control input applied to the robot, where $f_i$ is the force and $\tau_i$ is the torque.

The output of System (4.1) is defined as

which represents the robot hand position off of the wheel axis of the $i$ th mobile robot by a constant distance $d_i$. As in ^[30], we use the following standard input transformation:

where $\bar{u}_i = \left[\bar{u}_{x i}, \bar{u}_{y i}\right]^T$ is the new input; we can obtain a simple linear input-output relation as follows:

where $h_i = [x_{h i}, y_{h i}]^\top\in \mathbb{R}^2$ is the the robot hand position off of the wheel axis of the $i$-th mobile robot and $\bar{u}_i = [\bar{u}_{x i}, \bar{u}_{y i}]^\top\in \mathbb{R}^2$ is the control scheme. System (4.4) is a multiple double integrators system and can be written in the form as follows:

where $[w_{x i}, w_{y i}]$ is the speed vector of the $i$-th robot. We want to find control input $\bar{u}_i$ to achieve $\lim _{t \rightarrow \infty}\left(h_i(t)-h_{d }\right) = 0$ and $\lim _{t \rightarrow \infty} \dot{h}_i(t) = \dot{h}_d(t)$ for $i = 1, \ldots, N$, where $h_d(t)$ is the desired motion for all robots. We can view $h_d(t)$ as the dynamics of a leader mobile robot.

Now the nonholonomic mobile robots consensus can be studied by applying (2.7) and (2.8) and Controller (3.1). We consider $N = 7$ and

which means that the dynamics of the leader mobile robot can be expressed as a line. We set $\mu = 1$, $k_1 = 1$ and $k_2 = 1$ for the controller. The controller for the $i$-th mobile robot is

where $\eta_{xi} = [\eta_{x1i}, \eta_{x2i}]^\top\in \mathbb{R}^{2}$ and $\eta_{yi} = [\eta_{y1i}, \eta_{y2i}]^\top\in \mathbb{R}^{2}$. Denote ${\bf 1}_{11} = [1, \dots, 1]^{\top}\in \mathbb{R}^{11}$. Define the consensus error as $e_i = [e_{1i}, e_{2i}]^{\top} = h_i-h_d$. The initial states are set as

Moreover, let $m_i = J_i = d_i = 1$ for all robots and $hx_1 = hy_1 = 1$ and $hx_2 = hy_2 = 0.1$ for the leader mobile robot. We also set

Case 1: UJC network with dwell time

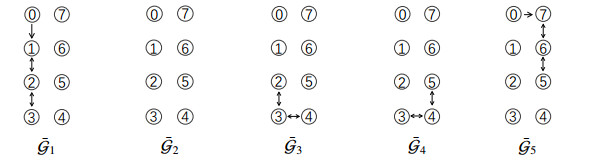
The following UJC switching signals $\sigma_1(t)$ is considered. The switching graphs $\bar{\mathcal{G}}_{\lambda}$, with $\lambda \in \{1, 2, 3, 4, 5\}$, are shown in Figure. 1. The node $0$ is associated with the desired destination and the other nodes represent the mobile robots. The two-way arrow means that there is a communication connection between the two mobile robots. We adopt the following switching signal:

with $k = 0, 1, 2, \dots$ and $T^{\prime} > 0$. Then, the switching signal $\sigma(t)$ is UJC with a dwell-time of $\tau = T^{\prime}/5$. We set $T^{\prime} = 1$. The connections among the mobile robots and the leader mobile robot are shown in Figure 1. It is worth mentioning that the mobile robots and the leader mobile robot in $\bar{\mathcal{G}}_2$ are not connected to each other. The corresponding edge set is empty.

As shown in Figure 2, we can see that the errors $e_i$ tend to zero. Figure 3 shows that states of the mobile robots tend to consensus with the leader mobile robot and that the consensus problem in the UJC case is solved by Controller (3.1).

Case 2: GUJC network

The following signal is also adopted.

with $k = 0, 1, 2, \dots$, $a_k = k$, $l = 0, 1, \dots, k$ and $T^{\prime} > 0$. The switching graphs $\bar{\mathcal{G}}_{\lambda}$ are the same as in the UJC case. Assumption 1 holds with $\tau = T'/10$ and $T = T^{\prime}$. We also set $T^{\prime} = 1$ in this case.

We can see that the errors tend to zero in Figure 4. Figure 5 shows that states of the mobile robots tend to consensus with the leader mobile robot and that the consensus problem in the GUJC case is also solved by Controller (3.1). Unlike with the results in ^[17,29], the switching network does not have any dwell time and since the time between two switching instants approaches zero, the switching frequency tends to infinity.

5.   Conclusions

This study yielded a double integrator MAS consensus control problem for generalized jointly connected switching networks without dwell time by making use of the generalized Krasovskii-LaSalle theorem combined with the virtual output method. The generalized Krasovskii-LaSalle theorem only requires the weak Lyapunov function to guarantee the uniform global stability. We use the limiting zeroing-output solution to describe the stable set. Thus, exponential stability of the switched closed-loop system can be attained even the dwell time is avoided. We have proven that the controller is still valid under the conditions of a fast switching network. A nonholonomic mobile robot control problem has been solved with the presented double integrator consensus controller. In the future, we may consider some other more practical issues, such as control based on sampling or event-triggered control.

Conflict of interest

The author declares no conflict of interest regarding the publication of this paper.