Revan Sombor indices: Analytical and statistical study

1.   Introduction

Type 2 diabetes mellitus is one of the most prevalent, multifactorial chronic diseases with a high rate of disability and mortality, which badly demands lifelong cost-efficient personalised management ^[1,2]. Blood glucose control is essential to postpone disease progression and alleviate symptoms ^[3,4].

RT-CGM (Real-time continuous glucose monitor), a portable subcutaneous interstitial glucose detector, enables long-term glucose control for its benefits in less puncture and more holistic pictures of blood glucose ^[5]. However, this CGM system limits its leverage due to its gap in glucose prediction ^[6], which can be filled by glucose trajectory prediction, such as deep learning tools. In this way, people can take action beforehand to eliminate imminent events, such as hyperglycaemic crisis and hypoglycaemic coma ^[7,8].

LSTM-RNN (long short-term memory - recursive neural network), the deep learning model fitting long sequence data such as continuous glucose ^[9], has the potential competence to provide a more accurate glucose trajectory prediction ^[10]. Previously, Sadegh Mirshekarian et al. presented an RNN approach with LSTM units to learn a physiological model of blood glucose ^[11]. And Mario Munoz-Organero also proposed, implemented, validated and compared a new hybrid deep learning model to mimic the metabolic behaviour of physiological blood glucose methods ^[12]. These studies indicated that deep learning contained the power to predict health-related parameters. Besides, the differential equations for carbohydrate and insulin absorption in physiological models were also modelled using LSTM cells. Rabby et al. proposed a novel approach to predict blood glucose levels with a stacked LSTM based on a deep RNN model considering sensor fault ^[13]. Based on these studies, we believe that LSTM-RNN would improve glucose prediction in diabetic patients with a stronger prediction power.

Although the precise prediction of glucose among patients suffering from type 2 diabetes is necessary and many researchers have verified the accuracy of deep learning models ^[14], only limited studies have tested the detailed effects of these potentially burden-free, deep-learning-involved care in the community ^[15]. Besides, only a few studies paid attention to the ethnoracial disparity, not to mention the inter-personal variation of glucose management with the deep-learning models ^[16]. Furthermore, scarce studies cared for the mental conditions of patients who utilise these deep learning-based health management models. Hence, we would like to explore whether this personally developed deep-learning model supported glucose management would improve the health of type 2 diabetes, both physically and mentally.

In this study, we would apply a personalized glucose prediction model to test the merits of the deep learning-assisted personalised health management pattern, aimed to support the management strategies for type 2 diabetic patients in the community. Firstly, we represented the development of individual deep learning models for blood glucose trajectory prediction. Then we exhibited a participant receiving personalised diabetes self-management in a real-world scenario who finally showed well-controlled blood glucose data despite increased stress.

2.   Materials and methods

2.1.   Participant

A 42-year man (the Han Chinese; living at home) with a history of type 2 diabetes for four years expressed interest in our management strategy in a review at Tianjin Medical University Metabolic Diseases Hospital. His body mass index (BMI) was 26.23 kg/m². With fasting blood glucose (FBG) of 8.2 mmol/L and glycosylated haemoglobin (HbA1c) of 9.2 × 10^-2 mmol/mol (8.4%), he was treated with Metformin 0.5 g three times a day. There was no history of diabetic retinopathy, neuropathy, carcinoma or any other comorbidities except a history of mild depression for four years. He was a non-smoker, and he seldom drank alcohol. His diabetes management aimed to control fasting glucose from 4.4 mmol/L to 7.0 mmol/L. With a clear consciousness and independent ability in daily life, the person agreed to participate and signed the consent for engagement and publication. In addition, the medical jargon was explained in the supplement file.

2.2.   The intelligence-aided personalised health management for diabetic patients

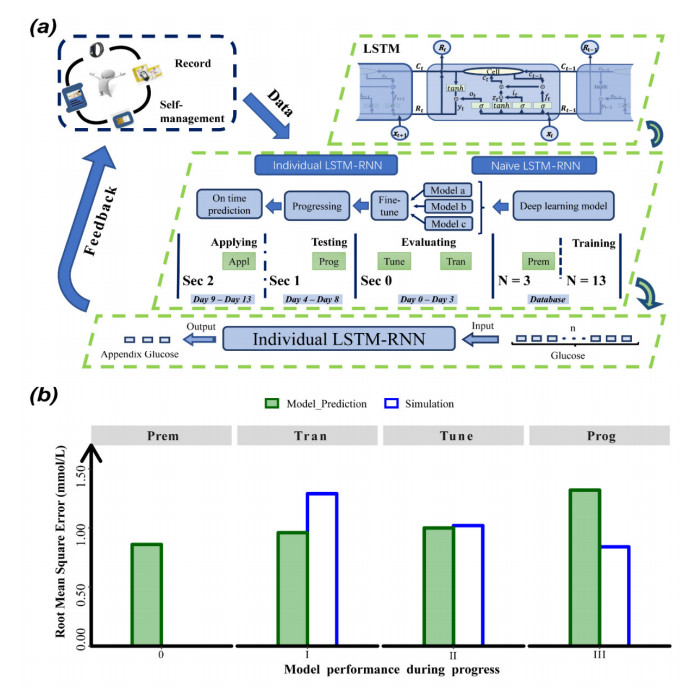
In this paradigm (Figure 1(a)), the person was armed with RT-CGM (FreeStyle® Libre, Abbott Diabetes Care Ltd.) and instructed to submit relevant data each day (for adjustment, Sec 0, day0–day3). The predicted trajectory by the personalised deep learning model, which was transferred from general glucose models, was not returned (for monitoring, Sec 1, day4–day8) until the ninth day, following the model application (Sec 2, day9–day13) and regular follow-up.

Figure 1.  Overview of deep learning customised self-management. (a) The architecture of individual LSTM-RNN (Long short-term memory recurrent neural network) models ^[9] facilitated self-management. The participant recorded and submitted real-world data such as food intake, physical activity, and continuous glucose, followed by glucose prediction and feedback by individual models for on-time application. Individual LSTM-RNN models were transferred (Tran) from naïve models, the top three models in ten replications with the best performance. Then, they were fine-tuned (Tune) and progressed (Prog) by personal CGM (continuous glucose monitor) data. The naïve models processed entire glucose sequence (length n) in steps, in which networks predicted appendix 2-hour glucose through 30-min triplicate recurrences by optimised weights through memory data (c_t-1) and input data (x_t), filtered by gates. Each prediction was made on the top model in Prog and Appl sections and tested in triplicate. (b) Model performance during individual model progression. RMSE (Root mean standard error) was compared between real-time CGM data and predicted glucose data (Model_Prediction, in which Glucose data were predicted in 30-min recurrence, green), and simulated application of appendix 2-hour glucose data generated through 30-min recurrence (Model_Application, blue).

DownLoad: Full-Size Img PowerPoint

2.3.   The development of individual LSTM-RNN deep learning prediction model

The individual LSTM-RNN model was transferred from naïve LSTM-RNN models (Prem) and developed from personal RT-CGM data. Naïve LSTM-RNN models were created and evaluated with 16 cases of CGM data (17,182 sets of glucose data) from the CGM system, whose interval was about 15 minutes. We chose three top models (Model a-c in Figure 1(a)) from ten replications of naïve models in case of the disparate features between cases in the naïve model development and the person applied the personalised prediction model. While in the following progressing and prediction sections, only the top model was employed to acquire personalised super-parameters, even though we tripled each forecast to assess the stability of the models. In these prediction models, the independent variables were a sequence of glucose obtained from CGM data, and the dependent variables were the glucose trajectory in the ensuing two hours. The core of the prediction models was iterated by Adam gradient optimization ^[17,18] and assessed by RMSE ^[19].

Personalised models were transferred (Tran) and fine-tuned (Tune) from naïve models by RT-CGM data in the adjustment section (Sec 0). And the individual LSTM-RNN models were progressed (Prog) during the monitoring section (Sec 1). Then the appendix 2-hour prediction was applied (Appl) on time for the 3rd section by three individual LSTM-RNN models.

2.4.   Efficacy of the intelligence-aided health management paradigm

Since blood glucose is commonly acknowledged in the short-term management of diabetic patients, some glucose-related parameters were chosen, including average glucose, daily hyperglycaemic time, daily time of high glucose and high blood glucose index ^[10,20]. Besides, fasting glucose, glycosylated haemoglobin, and BMI were used to contrast the lasting efficacy of this interference. Moreover, we also inspected psychological disturbance by the PAID (the Problem Area in Diabetes) scale, a reliable psychometric examiner for diabetes-related emotional distress ^[21]. Further description of the parameters used above was in the supplement file.

2.5.   Statistics

RMSE (Root Mean Square Error) was calculated to evaluate the model accuracy. The student's T test was applied for continuous data with Gaussian distribution (mean ± SEM), and the chi-square test was used for those non-Gaussian distribution data. As for the individual application results, data were reported directly for scarce cases.

3.   Results

3.1.   Model construction

The fine-tuned models had an average RMSE of 0.99 mmol/L for glucose prediction. And the simulation of on-time application was followed with an average RMSE of 0.98 mmol/L. The progressed models had a lower average RMSE (0.75 mmol/L) in on-time simulation.

3.2.   Model application

Compared with before application (Sec 1), the daily average glucose of this participant was decreased with the incorporation of a personalised self-management strategy in daily glucose level (Sec 2) (8.68 mmol/L ± 0.24 mmol/L to 8.02 mmol/L ± 0.11 mmol/L, mean ± SEM, P < 0.05), especially at night monitoring during 18:00 to 6:00 the next day (8.99 mmol/L ± 0.34 mmol/L to 7.65 mmol/L ± 0.13 mmol/L, mean ± SEM, P < 0.01). The measurements are exhibited in Figure 2(a, b). Mean of daily difference tended to be lower (from 0.15 mmol/L ± 0.66 mmol/L to 0.05 mmol/L ± 0.35 mmol/L). Time in the target range (3.9 mmol/L to 10.0 mmol/L) increased from 19.40 hours to 21.70 hours per day, along with less high glucose (Alert, CGM in a range of 10.0 mmol/L to 13.9 mmol/L, 1.70 hours per day; Clinically significant 0.55 hour per day). The hyperglycaemic risk was alleviated for high blood glucose index (HBGI) has dropped from 12.99 × 10² to 9.17 × 10², with a mild decrease in glucose variation (standard deviation, from 1.81 mmol/L to 1.59 mmol/L). The measured results are shown in Figure 2(c–e).

Figure 2.  Efficacy of deep learning aided self-management. (a–e) Data obtained from the real-time CGM (continuous glucose monitoring) system were compared between the 2nd section (Sec 1, day4–day8) and the 3rd section (Sec 2, day9–day13). (a, b) Average blood glucose. Average blood glucose dropped significantly daily and at night (18 o'clock to 6 o'clock the next day). (c) Average hyperglycaemic volume, high glucose multiplied by time (hours), representing clinical risk, alert (CGM data > 10.0 mmol/L, CGM data < 13.9 mmol/L) and clinically significant (CGM data ≥ 13.9 mmol/L) ^[10]. (d) High glucose time, the time (hours) of glucose higher than specific levels. (e) High blood glucose index, reflecting the risk of hyperglycaemia ^[22]. (f–h) Changes between baseline and 3-month follow-up. (f) Glycated haemoglobin. (g) Fasting blood glucose. (h) Body mass index. Asterisk mark (^* and ^**) represents significance (Wilcox's test) P < 0.05 and P < 0.01 separately.

DownLoad: Full-Size Img PowerPoint

Furthermore, the follow-up questionnaire in 3 months displayed a dropped BMI (24.69 kg/m²), less energy intake, more physical exercise, and better sleep. Besides, the participant had a lower FBG (6.7 mmol/L) and HbA1c 7.9 × 10^-2 mol/mol, with a regimen of Trajenta 5.0 mg in the morning and Metformin 1.0 g at night. The measurements are shown in Figure 2(f–h) and Table 1. More diabetes-associated distress was revealed by PAID scale, with a total increase of 6.25%, especially in the diet facet (sense of dietary deprivation), attributing to more than half of the rise. Others were related to social support (less safety) and emotion, like loneliness and depression (Table 2).

Table 1.  The participant's physical status between the baseline and 3-month follow-up.

Physical status	Baseline	Follow-up
BMI (kg/m2)	26.23	24.69
FBG (mmol/L)	8.2	6.7
HbA1c (mol/mol)	9.2 × 10-2	7.9 × 10-2

---

 | Show Table

DownLoad: CSV

Table 2.  Diabetes-associated stress between the baseline and 3-month follow-up.

PAID (%)	Baseline	Follow-up
Emotional	26.25	27.50
Therapeutic	12.50	12.50
Dietary	18.75	22.50
Social supporting	15.00	16.25
total	72.50	78.75
Note: Diabetes-associated distress data assessed by PAID scale (Problem Areas In Diabetes) were compared between baseline and follow-up in three months [21].

---

 | Show Table

DownLoad: CSV

4.   Discussion

Efficient and economical life-long glucose control is vital for people with diabetes in the community. This case depicts the construction of personalised deep learning models for on-time application. It suggests that deep learning customised glucose prediction may be a potential remedy for the CGM system in glucose forecast for life-long health management. And the application of the predicted system also requires the proper support from health care providers.

Personalised deep learning models are a potential supplement to CGM with kind of accuracy (Figure 2(b, c)). The individual may avoid abnormal hyperglycaemia glucose actively by more physical activity and less food intake, learning how to control his glucose appropriately. Moreover, the significant decrease of glucose (Figure 2(a–e)) and the mild change of glucose variation crossing two segments, along with the average daily RMSE (1.59 mmol/L) in the application segment, also support the applicability of deep learning in glucose prediction as insertion of the CGM system. However, the specific role of deep learning customised diabetes control still needs more cases engaged.

Deep learning customised glucose prediction can be more accurate with more data and advanced artificial intelligence techniques. Customised models built from 16 instances of CGM data and personal historical CGM data had a limited performance in this case. Considering the data reliance on prediction models, we believe that with more training data, multiple information involved in the era of big data and cutting-edge artificial intelligence techniques ^[23,24,25], the accuracy of deep prediction models will be higher, accomplishing individual healthcare step-by-step ^[26].

Deep learning customised glucose prediction with a CGM system may be a feasible personalised self-management strategy in the community. The participant has experienced a promising change in glucose after two sessions, which was sustained and with a lower BMI in 3 months, together with more physical activity and less food intake (Figure 2(f–h)). Besides, due to the limited accessibility of caregivers, the deep learning prediction provides optional guidance on how to act before the occurrence of abnormal glucose and to alleviate burdens in labour and economics.

Despite the promising results of this strategy mentioned above, attention must be paid to aspects such as stress. The participant suffered more stress both during the monitoring fortnight and 3-month follow-up. Researches indicate that the intervention efforts of diabetes stress were helpful in the comprehensive diabetes care, contributing to behaviour changes ^[27], elevated diabetes-associated stress might barricade the responsibility to beneficial intervention ^[28], and may increase depression burden ^[29]. The prolonged impact on glucose and behaviours, together with the condition of his mental health and stress, requires support from peers and healthcare providers ^[30,31]. Moreover, this strategy also demands a regular follow-up. Increasing the size of the participant samples, will help reinforce the certainty of the results., the one tailored for the personalised management of diabetes. Therefore, we would incorporate more patients to further research. We believe that more participants involved in more strictly designed clinical trials integrated with deep learning prediction would improve the outcome of patients with diabetes and march on the development of health management.

5.   Conclusions

In summary, deep learning customised glucose prediction may be accessible to personalised health care in the long-term management of type 2 diabetes, for example, by aiding in the CGM system. This would be beneficial for people suffering from this chronic disease, since a promising outcome (i.e., a decrease of glucose into a safer range, as shown above) might also occur on them using this care pattern, though more cases should be involved to test the validity of this caring pattern and more clinical settings should also be tested. Furthermore, the diabetes stress should be emphasized too, which seems to require a periodical care from the healthcare providers and the family members of patients.

Acknowledgments

We thank the support from the China Postdoctoral International Exchange Program Academic Exchange Project, Science and Technology Program of Tianjin (18ZXZNSY00280) and the Tianjin Medical University college student Innovation training program.

Conflict of interest

The participant has no conflicts of interest to disclose. Written informed consent was obtained from the participant for collecting his real-world data and publication. The funders did not participate in the designing, data gathering and analysing, publicising, or preparing of the manuscript. Abbott Diabetes Care supported the CGM data, discounted continuous glucose monitoring system (device and sensor), and equipment guidance and real-time communication.

Supplementary

S1.   Medical jargon and metrics

Except for general clinical glucose metrics like fasting glucose and glycosylated haemoglobin, body mass index was also applied to contrast the lasting efficacy of the intelligence-assisted health management.

Furthermore, continuous glucose parameters were included to evaluate the short-dated efficacy of glucose management, such as average glucose, daily hyperglycaemic time, daily time of high glucose and high blood glucose index.

Average glucose: the arithmetic average of glucose.

SD, standard deviation, evaluating the glucose variation.

Daily time of high glucose: the total time of glucose above range each day.

daily hyperglycaemic time: glucose multiplied by the time of glucose above range each day. The glucose above range were sectioned by clinical risk, alert (CGM data > 10.0 mmol/l, CGM data < 13.9 mmol/l) and clinically significant (CGM data ≥ 13.9 mmol/l).

High blood glucose index: a metric reflecting the risk of hyperglycaemia, calculated by functions below.

$HBGI = \frac{\sum 22.77\hspace{0.17em} \cdot \hspace{0.17em}{{f}_{\left({x}_{i}\right)}}^{2}}{n}$, ${f}_{\left({x}_{i}\right)} = {\mathrm{ln}\left({x}_{i}\right)}^{1.084}–5.381, {\;if\;f}_{\left({x}_{i}\right)}\ge 0$ for glucose readings x₁, …, x_n measured in mg/dl.

MODD, the mean of daily differences, evaluating intraday variability from all 24h intervals².

S2.   Deep learning model construction

Deep learning has leapt into the public view since the triumph of AlphaGo and got unceasing victories from diabetic retinopathy identification, medical events or outcomes prediction, and health care opmisation. The recurrent neural network (RNN), designed to sequence data, is powerful for long sequences after the incorporation of Long Short-Term Memory (LSTM), the one proposed to solve the "long-term dependencies" problem. We then described the LSTM-RNN models to predict glucose trajectories for diabetes management.

S2.1.   The CGM data set

Continuous glucose data by CGM (continuous glucose monitor) system (FreeStyle® Libre, Abbott Diabetes Care Ltd.) from December 2014 to September 2017 were collected from16 cases (up to 15 days per case). These data were arranged as daily glucose from 0 to 24 O'clock, at an interval of about 15 mins. Missing values were imputed by the likelihood StructTS method on the R 3.4 platform.

S2.2.   Participant data

Real-time monitoring data of this participant were obtained from CGM system (FreeStyle® Libre, Abbott Diabetes Care Ltd.) in September 2017. Fortnight average glucose from CGM was 8.9 mmol/L (160 mg/dL), with an estimated haemoglobin of 7.2% (55 mmol/mol). The distribution of glucose was 0 in Very Low, 0 in Low Alert, 77.0% in Target Range, 18.2% High Alert, and 4.8% in Very High4. About 23% of the glucose points were above the normal range. The utilisation rate of the CGM system was 99%, with an average of 53 times scans a day. No insulin was used during the two-weeks monitoring, which was assured by the assignment.

S2.3.   Naïve LSTM-RNN models

Model architecture referred to previous works. Input and output layers were 1-dimensional glucose data, and prediction of appendix 2-hour glucose by recurrence was iterated by Adam gradient optimization. The formula of naïve LSTM-RNN models was composed here where input gate, forget gate, and output gate were sigmoid function, while those of input and output block were hyperbolic tangent functions. The CGM data set was divided into two groups for the construction of naïve models (Prem), in which 14,878 (13 cases) in 17,182 data were put in the training set in python 3.6.

Block input: ${z}_{t} = tanh\left({W}_{z} \cdot \left[{R}_{t-1}, {x}_{t}\right]+{b}_{z}\right)$

Input gate: ${i}_{t} = \sigma \left({W}_{i} \cdot \left[{R}_{t-1}, {x}_{t}\right]+{b}_{i}\right)$

Forget gate: ${f}_{t} = \sigma \left({W}_{f} \cdot \left[{R}_{t-1}, {x}_{t}\right]+{b}_{f}\right)$

Cell: ${c}_{t} = {z}_{t} \cdot {i}_{t}+{c}_{t-1} \cdot {f}_{t}$

Output gate: ${o}_{t} = \sigma \left({W}_{o} \cdot \left[{R}_{t-1}, {x}_{t}\right]+{b}_{o}\right)$

Block output: ${y}_{t} = tanh\left({c}_{t}\right) \cdot {o}_{t}$

${{R}_{t} = y}_{t}$

S2.4.   Progression of the individual LSTM-RNN model

Transferring model (Tran): Ⅰ, load three best models; train three days, test 1 day, chose superparameters with the best RMSE.

Fine-tuned model (Tune): Ⅱ, super-parameters fine-tuned based on those output from Ⅰ, and each selected 3 top models with ten replications; train three days, test 1 day, chose superparameters with the best RMSE.

Progressing model (Prog): Ⅲ, super-parameters fine-tuned based on the top model from Ⅱ, though each prediction was tested in triplicate to assess the accuracy. Each one was re-built for three times; train seven days, test 1day (all past personalised data).

On-time application (Appl): Ⅴ, super-parameters fine-tuned based on the top models from Ⅲ, though each prediction was tested in triplicate to assess the accuracy; train N-1 days (all past personalised data), test for 2-hour trajectory.