Rapid exponential stabilization of Lotka-McKendrick's equation via event-triggered impulsive control

1.   Introduction

It is well known that continuous control fails to solve many stabilization tasks for nonlinear control systems ^[1]. The first result concerning the use of discontinuous controllers that stabilize any asymptotically controllable systems is given in ^[2], where the author assumes that the system is analytic and completely controllable. Recently, thanks to the use of new technologies, the powerful event-triggered impulsive control method (ETIC) has provided impressive results and has successfully controlled many complex systems. In the last two decades, considerable attention was paid to the use of event-triggered impulsive control to control ordinary differential equations.

The ETIC method transmits data packages and updates control inputs only when the predefined criterion is satisfied ^{[3,4,5,6,7,8]}. In ^[9], the stabilization of nonlinear systems was solved by using the ETIC method and the hybrid system tools. The ETIC method was also used to stabilize networked systems ^[10,11] and multi-agent systems (MASs) ^[12,13]. A Survey of Trends and Techniques is given ^[14] as well as in ^[15], recently.

The success of the ETIC method with ordinary differential equations has encouraged their generalization to partial differential equations. In ^[16], the ETIC method was used for designing a predictive control for spatially distributed processes with low order dynamics and for a limited number of output measurements modeled by nonlinear parabolic PDEs. This check is carried out using a state observer in order to monitor the estimation error of the model at each sampling instant. In ^[17], boundary control for 1-dimensional linear hyperbolic systems of conservation laws was investigated. In ^[18], the stabilization problem of boundary controlled hyperbolic partial differential equations was achieved. Later, an event-triggered boundary control based on the emulation of backstepping boundary control is given in ^[19]. More recently, an event-triggered boundary control to stabilize a PDE reaction-diffusion system with a Dirichlet boundary condition was proposed in ^[20].

Inspired by the event-triggered impulsive control method developed for finite-dimensional systems ^[6,8] an extension to the linear Lotka Mckendrick's equation (which is infinite dimensional) is introduced in this paper. The control problem of Lotka Mckendrick's equation has been largely studied in the literature of various kinds. Firstly, in the linear case in which diffusion is neglected, some null controllability results concerning the age-dependent population dynamics model, were first obtained by Barbu et al.^[21]. The authors proved that the system is controllable, provided that the control is supported in an age interval not containing zero. Recently, Hegoburu et al. ^[22] proved that the restriction of ^[21] is unnecessary, as long as the individuals do not reproduce at the age close to zero. More recently, ^[23] demonstrated that null controllability can be achieved by controls supported in any sub-interval of the age domain, provided we take control before individuals start reproducing.

Secondly, in the linear case where spatial diffusion is taken into account, Ainseba ^[24] proved the null controllability using a control that acts in a spatial sub-domain and for all ages except for a small age interval near zero. The case where the control operated in a spatial sub-domain and for all ages and for initial data near the target trajectory was investigated by Ainseba and Anita ^[24], and Kavian and Traore ^[25]. In ^[26] Traore has demonstrated the null controllability for such model with non-linear distributions of newborns and where the control is localized in a variable and active space for all ages (except for small ages). More recently, Maity, Tucsnak and Zuazua, in ^[27], have shown that the same result can be obtained by a control localized in the variable space as well as with respect to age. The controllability of non-linear controlled population dynamics without diffusion was discussed in ^[28]. Using a comparison principle for age-structured population dynamics, null controllability was obtained while preserving the non-negativity of the state trajectory. While in ^[29], the approximate controllability was proved via the unique continuation property of the adjoint system for the non-linear controlled population dynamics case with diffusion.

In this paper, we design an event-triggered impulsive control that rapidly exponentially stabilizes the system under consideration. The main contribution of our work is three-folds :i) the generalization of the ETIC method initially adopted for finite dimension systems in order to stabilize systems of infinite dimensions, ii) the rapid nature of the exponential stabilization, and iii) the removal of the non-verifiable convergence condition given by ^[6].

The paper is organized as follows. In the second section, we formulate our problem and we define the control task to be solved. In the third section, we present our event-triggered control strategy and we prove the main result. In the third section, we illustrate this result numerically.

2.   Problem statement

Let's consider the Lotka-McKendrick equation:

where $p(t, a)$ denotes the distribution of individuals of age $a >$0 at time $t >$0, $a_M$ designates the life expectancy of an individual, $\beta(a) >$0 represents the natural fertility rate and $\mu(a) >$0 signifies the natural mortality rate of individuals of age $a$. Equation (2.2) is viewed as a boundary condition and Eq (2.3) is some initial condition. In the following, we assume that the following standards conditions are fulfilled:

H1. $\beta \in L^\infty (0, a_M), \beta \geq 0$ for almost every $a \in (0, a_M)$.

H2. $\mu \in L^1 (0, a^*)$, for every $a^* \in (0, a_M), \mu \geq 0$ for almost every $a \in (0, a_M)$.

H3. ${ \int_{0}^{a_M} \mu (a) da = + \infty }$.

Conditions (H2)–(H3) are considered in the following papers ^[30,31] and ^[27], to cite a few. A discussion about the meaning of such conditions can be found in ^[32].

For Eqs (2.1)–(2.3), we assume that we can act on the state $p(t, a)$ only at some sampling sequence of times $0 = t_0 < t_1 < \cdots < t_k < t_{k+1} < \cdots$, with $t_k$ tends to $+\infty$ as $t$ towards to $+\infty$, where the sequence $(t_k)_{k\geq 0}$ is chosen in such a way to achieve our control objective which is the stabilization around a given referential distribution $p_r(a)$.

To the best of our knowledge, this is the first work using the ETIC method to control the Lotka-McKendrick equation.

In this paper, we will construct a sequence $(t_k^+, p(t_k^+, a))$ to control system Eqs (2.1)–(2.3), taking inspiration from the event-triggered strategy given recently in ^[6] for finite dimensional ordinary differential equations. This is the first attempt to generalize this strategy for infinite dimensional systems. We point out that the ETIC method is new in the stabilization of infinite dimensional systems and many recent results are established ^[18,19,20]. We shall generalize this strategy to adopt it to a system in infinite dimension, under a more rational and easy to verify hypothesis, and such that the solution of the following system:

Converge exponentially to a given referential population distribution $p_r(a)$, where $i_k\in Q$, $Q$ is a finite discrete set and $p_e(t_k, a)$ is the error between the current state $p(t_k, a)$ at time $t_k$ and the reference trajectory $p_r(a)$:

We can guarantee that the Eqs (2.4)–(2.7) is well posed if the Eqs (2.1)–(2.3) is well posed for all initial conditions $p_0\in L^1\left(0, a_{M}\right)$ and the process continues as long as the solution exists and as long as they do not cause an infinite number to occur out of discrete transitions in a finite time interval. The phenomenon of an infinite number of discrete transitions in a finite time interval is known as the "Zeno behavior". The existence of solutions of the linear Eqs (2.1)–(2.3) has been implicitly proved by several authors and goes back to the work of McKendrick ^[33], Lotka ^[34], Gurtin and MacCamy ^[30] and more recently Kappel and Zhang ^[31]. A more complete and detailed study of this equation is given in ^[32,35], where a more general case was considered and the boundary condition (2.2) becomes:

In the following we give a precise definition of the notion of rapidly exponential stabilization of a reference trajectory by means of data-driven control.

Definition 2.1. The referential population $p_r(a)$ is rapidly exponentially stable if for all decay rate $\alpha$, there exist a sequence $(t_k^+, p(t_k^+, .))$ and a positive constant $c$, such that for all initial condition $p_0\in L^1(0, a_M)$, the solution $p$ of Eqs (2.4)–(2.7) satisfies :

Remark 1. In the above definition, the word "rapidly" in the definition of "rapid exponential stability" is due to the fact that the decay rate $\alpha$ in this case is predetermined, and therefore we can choose it as large as we want. As well as, we have considered the $L^1(0, a_M)$-norm since the nature state space for the Eqs (2.1)–(2.3) is $L^1\left(0, a_{M}\right)$. In fact, the $L^1\left(0, a_{M}\right)$ norm of a non negative age distribution is the corresponding total population,

For this reason, we adopt this norm in order to measure the convergence of the total population towards the reference population.

3.   Main result

In this section, we establish our main result concerning the design of data-driven control strategy that rapidly stabilizes our Eqs (2.4)–(2.7) in the sense of the above definition.

Let $\sigma$ and $\Delta$ be two positive real numbers such that $\sigma > 1$. Let $Q = \{1, 2, 3\}$ and $\lambda_1, \lambda_2, \lambda_3$ are such that

From $t = 0$, we put $t_0^+ = 0$ and $p_e(t_{0}^+, a) = p_0(a)-p_r(a)$, the construction of the sequence $(t_{k}, p(t_{k}^+, a))$ for $k\geq 1$ is done in a recursive way according to the occurrence of the three following events:

The following Theorem is the main result of our work.

Theorem 3.1. There exist $\sigma > 1$, $\Delta > 0$, $\lambda_1, \lambda_2, \lambda_3$ satisfying (3.1), such that Eqs (2.4)–(2.7) with the above switching strategy is rapidly exponentially stable.

Proof. First of all, let us note that the system cannot have a Zeno behavior. In fact, it is well known that a sufficient condition for that is the existence of some positive constant $\delta > 0$ such that for all $k\geq 0, \, t_{k+1}-t_k\geq \delta$. To do this, let $\delta = \min\{\Delta, \ln(\sigma)\}$. From the construction of the events $E_1$ and $E_2$, we have $t_{k+1}-t_k = \Delta\geq \delta$, while in event $E_3$ we have $t_{k+1} = \min \{ t\in]t_k, t_k + \Delta], \Vert p_e(t)\Vert_1 \geq \sigma \Vert p_e(t_k^+)\Vert_1\}$ and thus $\Vert p_e(t)\Vert_1 = \sigma \Vert p_e(t_k^+)\Vert_1$ for $t = t_{k+1}$ while $\Vert p_e(t)\Vert_1 < \sigma \Vert p_e(t_k^+)\Vert$ for all $t\in (t_k, t_{k+1})$. In particular it follows that $t_{k+1} \neq t_{k}$ and then there exists $\delta > 0$ such that $t_{k+1}-t_{k} = \delta$, It therefore ensues that the Zeno behaviour cannot occur.

Now, let's prove the rapid exponential stability of the Eqs (2.4)–(2.7). Let

In view of Eq (3.1), we have $\sigma^* > 1$. In the following, we will show that for all $\sigma\in(1, \sigma^*)$ the referential trajectory $p_r$ is exponentially stable with a decay rate

Let $t\in (t_k, t_{k+1}]$. Three cases are possible.

First case : If $t_{k+1}$ results from an occurrence of event $E_1$. Then, by the definition of $t_{k+1}$, we have $\Vert p_e(t_{k+1})\Vert_1 \leq \Vert p_e(t_{k}^+)\Vert_1$ and $\Vert p_e(t)\Vert_1 < \Vert p_e(t_k^+)\Vert_1$ for all $t \in [t_k, t_{k+1})$. Then, we obtain in this case,

Second case : If $t_{k+1}$ results from an occurrence of event $E_2$. Then, from the definition of $t_{k+1}$, we have $\Vert p_e(t_{k+1})\Vert_1 \leq \sigma \Vert p_e(t_{k}^+)\Vert_1$ and $\Vert p_e(t)\Vert_1 < \sigma \Vert p_e(t_k^+)\Vert_1$ for all $t \in [t_k, t_{k+1})$. Then, we obtain in this case

Third case : If $t_{k+1}$ results from an occurrence of event $E_3$. Then, by continuity, we have $\Vert p_e(t_{k+1})\Vert_1 = \sigma \Vert p_e(t_{k}^+)\Vert_1$ and $\Vert p_e(t)\Vert_1 < \sigma \Vert p_e(t_k^+)\Vert_1$ for all $t \in [t_k, t_{k+1})$. Then, we obtain in this case

Let $N_i^k$ be the number of occurrences of event $E_i$, $i = 1, 2, 3$ in the interval $(0, t_k]$. Then, combining (3.3), (3.4) and (3.5), it follows that for all $k\geq 0$ and $t\in (t_k, t_{k+1}]$ we have:

where $C = \frac{1}{1+\lambda_1}.$ We have used the fact that if $t\in (t_k, t_{k+1})$, then $t\leq (k+1)\Delta$ and therefore $k\geq t/\Delta-1$. Thus, Eqs (2.4)–(2.7) with the switching strategy defined above is exponentially stable with the prescribed decay rate $\alpha$ defined in (3.2). Noting that if $\lambda_1$ (which can be chosen arbitrarily in $(-1, 0)$) is very close to $-1$, then the decay rate $\alpha$ can be as large as one wants. Then, the rapid exponential stabilization for the Eqs (2.4)–(2.7) is proved.

Remark 2. Note that the fact that the impulsive control acts on the whole Omega domain does not diminish the interest of our work for several reasons. In addition to the reasons mentioned at the end of the introduction of this paper, it often happens that in practice, one cannot control a system neither from the boundary nor on a sub-domain, and one is thus obliged to apply a control on the whole domain. For example, in the case of an epidemic (as in the case of Covid-19), many strict measures imposed such as social distancing and the wearing of masks failed to control the propagation of the pandemic, and many countries were forced to resort to a complete lockdown despite its disastrous effects on the economy. The hope of controlling the spread of the epidemic has become dependent on the vaccination of the whole population ^[36]. Finally, our control strategy can be applied to other types of equations, such as those derived from brain activity. Although the localization in the brain of simple functions is well known, brain observation techniques such as (MEG), (EEG) and (fMRI) allow us to study the dynamics of the human brain. But unfortunately, for more complex functions (language, memory, attention, ...), neuroscientists have not reached the level of the spatio-temporal resolution necessary to specify the localizations corresponding to brain activities by these techniques ^[37,38]. Therefore a control on the whole domain is necessary ^[39].

Remark 3. The main issue to investigate is how to generalize the event-triggered impulsive control method for the more general class of semi-linear evolution equation

with initial condition $u(0) = u_0\in D(A)$, where $A$ is the generator of $C_0$-semigroup and $f$ satisfies the hypothesis of Theorem 1.5 in ^[40].

Remark 4. It is important to mention that in the original ETIC method introduced by ^[6], the condition imposed on the system that guaranteed the global uniform exponential stabilization cannot be applied to obtain a rapid exponential stabilization. Indeed, the condition is expressed according to the number of occurrences of events $E_1, E_2$ and $E_3$, which are future incidents and therefore they are not yet produced at the beginning of the process. This makes the validation of the convergence condition impossible. Furthermore, the convergence rate in the cited paper is bounded as mentioned in Remark 3.1 in ^[6]. However, in our work, the convergence is not formulated as a function of the numbers of these incidents. In addition, our decay rate is unbounded.

4.   Numerical application

To illustrate the applicability of our result, we consider Lotka-Mckendrick's equation in a general case where the functions birth rate and death rate are functions and not constants: the death rate function is $\mu (a) = \frac{1 }{ a_M - a }$ and the birth rate function is $\beta (a) = \mathrm{1}_{[3,8]}(a) \tanh (a).$ To compute the numerical solution, we adopt the discretization of Eqs (1)–(3) considered by ^[41] with age and time steps of length $h = 0.0078125$. Moreover, we take as initial condition $p_0(t, a) = 100 exp(-a-t)$ and as referential solution the solution $p_r = a(a_M-a)$ and we assume that the maximum age of an individual is $a_M = 10$ and $T = 10$.

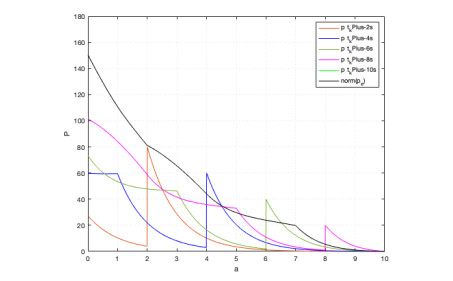
The state trajectories of Eqs (2.1)–(2.3) with initial condition $p_0(t, a) = 100 exp(-a-t)$ and without control are depicted in Figure 1.

Now, to stabilize the Eqs (2.1)–(2.3), we apply our strategy for event-triggered impulsive control given in in Section $3$ with the following values of parameters $\Delta = 0.39, \, \sigma = 1.2, \, \lambda_{1} = -0.9, \, \lambda_{2} = -0.3,$ and $\lambda_{1} = -0.1$.

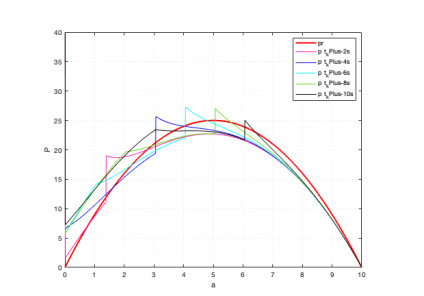
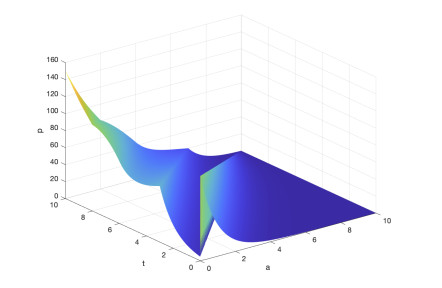
The convergence of trajectories of the error dynamic $p_e = p-p_r$ is depicted in Figure 2 in 3D and in Figure 3, which shows that the error dynamics is exponentially stable under designed ETIC. Finally the Figure 4 shows the exponential convergence of the solution $p$ of system to the referential population $p_r = a(a_M-a)$.

5.   Conclusions

In this paper, an event triggered impulsive control to stabilize the Lotka-McKendrick equation has been designed. The rapid exponential stabilization is achieved and a numerical illustration to validate the result is given. This work leaves some open questions for future works. The event-based stabilization approaches may be applied to a semilinear evolution equation. Another interesting point is to apply this control strategy for boundary control or sub-domain control with a finite number of actuation points particularly for some systems in particular for Lotka-McKendrick equation.

Acknowledgments

The authors would like to thank the anonymous reviewers for their comments on earlier versions of this article.

Conflict of interest

The authors declare no conflict of interest.