Mathematical analysis of an age-structured HIV-1 infection model with CTL immune response

1.   Introduction

Human immunodeficiency virus (HIV) is a pathogen that infects T-helper cells of the immune system and can cause Acquired Immune Deficiency Syndrome (AIDS) ^[1]. These are white blood cells that move around the body, detecting faults and anomalies in cells as well as infections. When HIV targets and infiltrates these cells, it reduces the body's ability to combat other diseases. This increases the risk and impact of opportunistic infections and cancers. In past decades, many works have been developed for HIV-1 infection using simple differential equation models (see, for example, ^{[2,3,4,5,6,7,8]}). Let $x, y$ and $v$ denote the concentrations of uninfected target cells (i.e. cells susceptible to HIV-1 infection), productively infected cells, and free virions, respectively. A basic mathematical model describing HIV-1 infection dynamics has been studied in ^[4,8]:

where uninfected cells are produced at a rate $\Lambda$ and die at rate $dx$ per target cell, and become infected at rate $\beta xv$, where $\beta$ is the rate constant describing the infection process; infected cells are produced at rate $\beta xv$ and die at rate $ay$; free virions are produced from infected cells at rate $ky$ and are removed at rate $uv$.

We note that in system (1.1), the death rate and virus production rate of infected cells are both assumed to be constant. In reality, as argued by Nelson et al. ^[9], the production of new virus particles(virions) by an infected cell does not occur at a constant rate, but rather ramps up as viral proteins and unspliced viral RNA accumulate within the cytoplasm of an infected cell. In ^[9], in order to describe this phenomenon, by considering the variations in the death rate of productively infected $T$ cells and the productions rate of viral particles as a function of the length of time a $T$ cells has been infected, Nelson et al. developed and analyzed the following age-structured within-host HIV-1 infection model:

with boundary condition $T^*(0, t) = \beta T(t)V(t)$. In system (1.2), $T^*(a, t)$ denotes the density of infected T cells of infection age $a$ (i.e. the time that has elapsed since an HIV virion has penetrated the cell) at time $t$, $\mu(a)$ is the age-dependent per capita death rate of infected cells, $p(a)$ is the viral production rate of an infected cell with age $a$. In ^[9], Nelson et al. discussed the local stability of the nontrivial equilibrium solution and provided a general stability condition for models with age structure. In ^[10], by constructing suitable Lyapunov functions, Huang et al. established the global dynamical properties for Nelson's age-structured model without (or with) drug treatment. In ^[11], Rong et al. considered two models with age-of-infection and combination therapies involving reverse transcriptase, protease, and entry/fusion inhibitors. In ^[12], considering the infection rate of microparasitic infections is an increasing function of the parasite dose, Xu et al. further investigated a within-host HIV-1 infection model with saturation incidence and age-since-infection structure for infected cells. Recently, great attention has been paid by many researchers to age-structured model of HIV infection due to their greater flexibility in exploring fundamental issues of viral production and death, and allow coupling of biological processes happening on different time scales (see, for example, ^{[10,11,12,13,14,15,16,17]}).

It is worth noting that the effect of immune response is ignored in the models above. However, in most virus infections, cytotoxic T lymphocytes (CTLs) play a critical role in cell-mediated immunity by regulating the functions of other immune cells (such as the B cells and macrophages) and attacking diseased cells and tumors ^[18]. For HIV-1 infection, the main clinical indicators of that HIV-1 positive patient are in the follow up both the viral load and the CD4$^+$T cells count in blood plasma, therapy is started, make a portion to the immune cells to be toxic thereby introducing toxicity in the immune system of the individual^[19]. In ^[20], Cao et al. have shown that CTL immune response is often associated with better virus control and slower disease progression during the early stage of HIV infection. In ^[4], in order to discuss the effect of the population dynamics of viral infection with CTL immune response, Nowak et al. proposed the basic HIV-1 infection model with immune response. At present, there are few works on global dynamics in the age-structured within-host HIV-1 infection model with CTL immune response ^[21,22].

Motivated by the works of Nelson et al. ^[9], Nowak et al.^[4] and Regoes et al. ^[23], in the present paper, we are concerned with the effects of age-structured, CTL immune response and saturation incidence on the dynamics of HIV-1 infection. To this end, we consider the following HIV-1 infection model:

with boundary condition

and initial condition

where $\mathscr{X} = \mathbb{R}^+\times L_{+}^1(0, \infty)\times \mathbb{R}^+ \times \mathbb{R}^+$, $L_+^1(0, \infty)$ is the set of all integrable functions from $(0, \infty)$ into $\mathbb{R}^+ = [0, \infty)$. In system (1.3), $x(t)$ represents the concentration of uninfected target T cells at time $t$, $y(a, t)$ denotes the density of infected T cells of infection age $a$ at time $t$, $v(t)$ denotes the concentration of infectious free virion at time $t$, and $z(t)$ denotes the concentration of CTLs at time $t$. The definitions of all parameters in system (1.3) are listed in Table 1.

In the sequel, we further make the following assumptions.

(H1) $c, k, p, \mu \in L_{+}^\infty(0, \infty)$ with essential upper bounds $\bar{c}, \bar{k}, \bar{p}$ and $\bar{\mu}$, respectively.

(H2) There are positive constants $\mu_0, \mu_1, \bar{b}$ and satisfying $\mu_0\leq d$, $\bar{b} = b-\bar{c}\max\left\{\frac{\Lambda}{\mu_0}, \|X_0\|_{\mathscr{X}}\right\}$ and $\mu_1 = \min\left\{\mu_0, u, \bar{b}\right\}$. $\mu(a)$ is a bounded function on $\mathbb{R}^+$ satisfying $\mu(a)\geq \mu_0$ for $a\geq 0$.

(H3) For any $a > 0$, there exist $a_k, a_c > a$ such that $k(a)$ is positive in a neighbourhood of $a_k$ and $c(a)$ is positive in a neighbourhood of $a_c$.

Using the theory of age-structured dynamical systems introduced in ^[24,25], one can show that system (1.3) has a unique solution $(x(t), y(\cdot, t), v(t), z(t))$ satisfying the boundary condition (1.4) and the initial condition (1.5). Moreover, it is easy to show that all solutions of system (1.3) with the boundary condition (1.4) and the initial condition (1.5) are defined on $[0, +\infty)$ and remain positive for all $t\geq 0$. Furthermore, $\mathscr{X}$ is positively invariant and system (1.3) exhibits a continuous semi-flow $\Phi: \mathbb{R}^+\times \mathscr{X} \rightarrow \mathscr{X}$, namely,

Given a point $(x, \varphi, v, z)\in \mathscr{X}$, we have the norm $\|(x, \varphi, v, z)\|_{\mathscr{X}}: = x+\int_{0}^{\infty}\varphi(a){\rm d}a+v+z.$

The organization of this paper is as follows. In the next section, we establish the asymptotic smoothness of the semi-flow generated by system (1.3). In Section 3, we calculate the immune-inactivated reproduction rate and the immune-activated reproduction rate and discuss the existence of feasible steady states of system (1.3) with the boundary condition (1.4). In Section 4, by analyzing the corresponding characteristic equations, we study the local asymptotic stability of an infection-free steady state and a CTL-inactivated infection steady state of system (1.3), respectively. In Section 5, we show that if the immune-activated reproduction rate is greater than unity, system (1.3) is uniformly persistent. In Section 6, we are concerned with the global stability (attractivity) of each of feasible steady states of system (1.3) by means of Lyapunov functionals and LaSalle's invariance principle. In Section 7, numerical examples are carried out to illustrate the feasibility of theoretical results. A brief conclusion is given in Section 8 to end this work.

2.   Boundedness and Asymptotic smoothness

In order to discuss the global dynamics of system (1.3) with the boundary condition (1.4), in this section, we are concerned with the boundedness of solutions of system (1.3) and the asymptotic smoothness of the semi-flow $\{\Phi(t)\}_{t\geq 0}$ generated by system (1.3).

2.1.   Boundedness of solutions

In this subsection, we prove the boundedness of semi-flow $\{\Phi(t)\}_{t\geq 0}$. Denote

and

Proposition 2.1. Let $\Phi_t$ be defined as in (1.6). Then the following statements hold.

(ⅰ) $\frac{{\rm d}}{{\rm d}t}\|\Phi_t(X_0)\|_{\mathscr{X}}\leq \Lambda+\bar{k}\max\left\{ \frac{\Lambda}{\mu_0}, \|X_0\|_{\mathscr{X}}\right\}-\mu_1 N(t)$ for all $t\geq 0$;

(ⅱ) $\|\Phi_t(X_0)\|_{\mathscr{X}}\leq \max\left\{ \frac{1}{\mu_1}\left[\Lambda+\bar{k}\max\left\{ \frac{\Lambda}{\mu_0}, \|X_0\|_{\mathscr{X}}\right\}\right], \|X_0\|_{\mathscr{X}}\right\}$ for all $t\geq 0$;

(ⅲ) $\limsup_{t \rightarrow +\infty}\|\Phi_t(X_0)\|_{\mathscr{X}}\leq \frac{1}{\mu_1}\left[\Lambda+\bar{k}\max\left\{ \frac{\Lambda}{\mu_0}, \|X_0\|_{\mathscr{X}}\right\}\right]$;

(ⅳ) $\Phi_t$ is point dissipative: there is a bounded set that attracts all points in $\mathscr{X}$.

Proof.. Let $\Phi_t(X_0) = \Phi(t, X_0): = (x(t), y(\cdot, t), v(t), z(t))$ be any nonnegative solution of system (1.3) with the boundary condition (1.4) and the initial condition (1.5). We derive from system (1.3) that

The variation of constants formula implies

which yields

for all $t \geq 0$. We derive from Eq (2.2) and the third and fourth equations of system (1.3) that

It follows from Eqs (2.1) and (2.3) that

Again, using variation of constants formula we have from Eq (2.4) that

for all $t\geq 0$. This completes the proof.

The following results are direct consequences of Proposition 2.1.

Proposition 2.2. If $X_0\in \mathscr{X}$ and $\|X_0\|_{\mathscr{X}}\leq K$ for some $K\geq \frac{1}{\mu_1}\left[\Lambda+\bar{k}\max\left\{ \frac{\Lambda}{\mu_0}, \|X_0\|_{\mathscr{X}}\right\}\right]$, then

for all $t\geq 0$.

Proposition 2.3. Let $C\in \mathscr{X}$ be bounded. Then

(1) $\Phi_t(C)$ is bounded;

(2) $\Phi_t$ is eventually bounded on $C$.

2.2.   Asymptotic smoothness

In this subsection, we show the asymptotic smoothness of the semi-flow $\{\Phi(t)\}_{t\geq 0}$.

Denote

It follows from (H1) and (H2) that $0 < e^{-(\bar{\mu}+\bar {p}K)a}\leq \pi(a)\leq e^{-\mu_0 a}$ for all $a\geq 0$. Clearly, $\pi(a)$ is a deceasing function.

Let $(x(t), y(\cdot, t), v(t), z(t))$ be a solution of system (1.3) with the boundary condition (1.4) and the initial condition (1.5). Integrating the second equation of system (1.3) along the characteristic line $t-a =$const., we have

where $L(t): = y(0, t) = \frac{ \beta x(t)v(t)}{1+ \alpha v(t)}$.

Using a similar argument as that in ^[12], it's easy to verify the following result.

Proposition 2.4. The function $L(t)$ is Lipschitz continuous on $\mathbb{R}^+$.

Before giving our main results, we need the following Lemmas.

Lemma 2.1. ^[26] The semi-flow $\Phi: \mathbb{R}^+\times \mathscr{X}_+ \rightarrow \mathscr{X}_+$ is asymptotically smooth if there are maps $\Theta, \Psi: \mathbb{R}^+\times \mathscr{X}_+ \rightarrow \mathscr{X}_+$ such that $\Phi(t, x) = \Theta(t, x)+\Psi(t, x)$ and the following hold for any bounded closed set $\mathcal {C}\subset \mathscr{X}_+$ that is forward invariant under $\Phi$:

(1) $\lim_{t \rightarrow +\infty}{\rm diam}\Theta(t, \mathcal {C}) = 0$;

(2) there exists $t_\mathcal {C}\geq 0$ such that $\Psi(t, \mathcal {C})$ has compact closure for each $t\geq t_\mathcal {C}$.

Lemma 2.2. ^[26] Let $\mathcal {C}$ be a subset of $L^1(\mathbb{R}^+)$. Then $\mathcal {C}$ has compact closure if and only if the following assumptions hold:

(ⅰ) $\sup_{f\in \mathcal {C}}\int_{0}^{\infty}|f(a)|da < \infty$;

(ⅱ) $\lim_{r \rightarrow \infty}\int_{r}^{\infty}|f(a)|da = 0$ uniformly in $f\in \mathcal {C}$;

(ⅲ) $\lim_{h \rightarrow 0^+}\int_{0}^{\infty}|f(a+h)-f(a)|da = 0$ uniformly in $f\in \mathcal {C}$;

(ⅳ) $\lim_{h \rightarrow 0^+}\int_{0}^h|f(a)|da = 0$ uniformly in $f\in \mathcal {C}$.

By applying Lemmas 2.1 and 2.2, we now prove the asymptotic smoothness of the semiflow $\Phi$ generated by system (1.3).

Theorem 2.1. The semi-flow $\Phi$ generated by system (1.3) is asymptotically smooth.

Proof. We first decompose the semi-flow $\Phi$ into two parts: for $t\geq 0$, let $\Psi(t, X_0): = \left(x(t), \tilde{y}(\cdot, t), v(t), z(t)\right), \Theta(t, X_0): = \left(0, \tilde{\phi}_{y}(\cdot, t), 0, 0\right)$, where

Clearly, we have $\Phi = \Theta+\Psi$ for $t\geq 0$.

Let $\mathcal {C}$ be a bounded subset of $\mathscr{X}$ and $K > \big[\Lambda+\bar{k}\max\{\frac{\Lambda}{\mu_0}, \|X_0\|_{\mathscr{X}}\}\big]{\big /}\mu_1$ the bound for $\mathcal {C}$. Let $\Phi(t, X_0) = (x(t), y(\cdot, t), v(t), z(t))$, where $X_0 = (x^0, y_{0}(\cdot), v^0, z^0)\in \mathcal {C}$. Then

Letting $a-t = \sigma$, it follows from (2.9) that

yielding $\lim_{t \rightarrow +\infty}\|\Theta(t, X_0)\| = 0$, hence, $\lim_{t \rightarrow +\infty}{\rm diam}$ $\Theta(t, \mathcal {C}) = 0$ and the assumption (1) in Lemma 2.1 holds.

In the following we show that $\Psi(t, \mathcal {C})$ has compact closure for each $t\geq t_\mathcal {C}$ by verifying the assumptions (ⅰ)–(ⅳ) of Lemma 2.2. From Proposition 2.2 we see that $x(t), v(t)$ and $z(t)$ remain in the compact set $[0, K]$. Next, we show that $\tilde{y}(a, t)$ remain in a pre-compact subset of $L_+^1$ independent of $X_0$. It is easy to show that $\tilde{y}(a, t)\leq \bar{L}e^{-\mu_0a},$ where $\bar{L} = \beta K^2/(1+ \alpha K)$. Therefore, the assumptions (ⅰ), (ⅱ) and (ⅳ) of Lemma 2.2 follow directly. We need only to verify that (ⅲ) of Lemma 2.2 holds. Since we are concerned with the limit as $h \rightarrow 0$, we assume that $h\in (0, t)$. In this case, we have

By Proposition 2.2., there is positive constant $M_L$ such that

It follows from (2.11) and (2.12) that

Hence, the condition (ⅲ) of Lemma 2.2 holds. By Lemma 2.1, the asymptotic smoothness of the semi-flow $\Phi$ generated by system (1.3) follows. This completes the proof.

The following result is immediate from Theorem 2.33 in ^[26] and Theorem 2.1.

Theorem 2.2. There exists a global attractor $\mathcal {A}$ of bounded sets in $\mathscr{X}$.

3.   Steady states and basic reproduction number

In this section, we are concerned with the local stability of each of feasible steady states of system (1.3) with the boundary condition (1.4).

Clearly, system (1.3) always has an infection-free steady state $E_0(\Lambda/d, 0, 0, 0)$. If system (1.3) has a CTL-inactivated infection steady state $E_1(x_1, y_1(a), v_1, 0)$, then it must satisfy the following equations:

We derive from the first equation of (3.1) that

It follows from the second equation of (3.1) that

where $\phi_1(a) = e^{-\int_0^a\mu(s){\rm d}s}.$ We obtain from the third equation of (3.1) and (3.3) that

On substituting (3.2)–(3.4) into the fourth equation of (3.1), we have

where

Here, $\mathscr{R}_0$ is called the immune-inactivated reproduction rate of system (1.3), which represents the number of newly infected cells produced by one infected cell during its lifespan. Hence, if $\mathscr{R}_0 > 1$, in addition to the infection-free steady state $E_0$, system (1.3) admits a CTL-inactivated infection steady state $E_1(x_1, y_1(a), v_1, 0)$, where

Further, if system (1.3) has a CTL-activated infection steady state $E^* (x^*, y^*(a), v^*, z^*)$, then it must satisfy the following equations:

It follows from the first equation of (3.7) that

We obtain from the second equation of (3.7) that

where $\phi_2(a, z^*) = e^{-\int_0^a p(s)z^*{\rm d}s}$. When $z^*\neq 0$, it follows from (3.9) and the fourth equation of (3.7) that

We derive from the third equation of (3.7) and (3.10) that

On substituting (3.8), (3.10) and (3.11) into the first equation of (3.7), we have that

where

From (3.13), it is easy to show that

Denote

Clearly

where

Here, $\mathscr{R}_1$ is called the immune-activated reproduction rate which expresses the CTL load during the lifespan of a CTL cell. Clearly, if $\mathscr{R}_1 > 1$, it therefore follows from (3.14) and (3.16) that $\Phi(0) > 0$. Further, for $z > 0$ sufficiently large, we note that

Then by (3.15), for $z > 0$ sufficiently large, there exists a $z_0^* > 0$ such that $\Phi(z_0^*) < 0$. Therefore, if $\mathscr{R}_1 > 1$, there exists a $z^*\in(0, z_0^*)$ such that $\Phi(z^*) = 0$. Hence, if $\mathscr{R}_1 > 1$, in addition to the infection-free steady state $E_0$ and the CTL-inactivated infection steady state $E_1$, system (1.3) exists a unique infection steady state $E^*(x^*, y^*(a), v^*, z^*)$.

4.   Local stability

In this section, we are concerned with the local stability of the infection-free steady state $E_0$ and the CTL-inactivated infection steady state $E_1$ of system (1.3), respectively.

We first consider the local stability of the infection-free steady state $E_0(\Lambda/d, 0, 0, 0)$.

Let $x(t) = x_0(t)+\Lambda/d, y(a, t) = y_0(a, t), v(t) = v_0(t), z(t) = z_0(t)$. Linearizing system (1.3) at the steady state $E_0$, it follows that

Looking for solutions of system (4.1) of the form $x_0(t) = x_{01}e^{ \lambda t}, y_0(a, t) = y_{01}(a)e^{ \lambda t}, v_0(t) = v_{01}e^{ \lambda t},$ $z_0(t) = z_{01}e^{ \lambda t}$, where $x_{01}, y_{01}(a), v_{01}$ and $z_{01}$ will be determined later, one obtains the characteristic equation of system (1.3) at the steady state $E_0$ of the form:

where

Clearly, Eq (4.2) always has one negative real root $\lambda = -b$, other roots of (4.2) are determined by equation

Clearly, we have $f_1(0) = \mathscr{R}_0$. It is easy to show that $f_1^{ \prime}(\lambda) < 0$ and $\lim_{ \lambda \rightarrow +\infty}f_1(\lambda) = 0$. Hence, $f_1(\lambda)$ is a decreasing function. Therefore, if $\mathscr{R}_0 > 1$, then $f_1(\lambda) = 1$ has a unique positive root. Hence, if $\mathscr{R}_0 > 1$, the steady state $E_0$ is unstable.

Now, we claim that all roots of Eq (4.3) have negative real parts if $\mathscr{R}_0 < 1$. If not, there exists a root $\lambda_1 = a_1+{\rm i} b_1$ with $a_1\geq 0$. In this case, substituting $\lambda_1$ into (4.3), we obtain

a contradiction. Hence, if $\mathscr{R}_0 < 1$, all roots of equation (4.2) have negative real parts. Accordingly, the steady state $E_0(\Lambda/d, 0, 0, 0)$ is locally asymptotically stable if $\mathscr{R}_0 < 1$.

Now, we consider the local stability of the CTL-inactivated infection steady state $E_1(x_1,$ $y_1(a), v_1, 0)$.

Let $x(t) = x_1(t)+x_1, y(a, t) = y_1(a, t)+y_1(a), v(t) = v_1(t)+v_1, z(t) = z_1(t)$. Linearizing system (1.3) at the steady state $E_1$, we obtain that

Looking for solutions of system (4.4) of the form $x_1(t) = x_{11}e^{ \lambda t}, y_1(a, t) = y_{11}(a)e^{ \lambda t}, v_1(t) = v_{11}e^{ \lambda t},$ $z_1(t) = z_{11}e^{ \lambda t}$, where $x_{11}, y_{11}(a), v_{11}$ and $z_{11}$ will be determined later, we obtain the following linear eigenvalue problem:

We derive from the fourth equation of (4.5) that

Clearly, If $\mathscr{R}_1 > 1$, $\lambda > 0$, in this case, $E_1$ is unstable. If $\mathscr{R}_1 < 1 < \mathscr{R}_0$, it follows from the fourth equation of (4.4) that $z_1\rightarrow 0$, hence, in the following discussion, we only consider the simplified system

It follows from the first and the second equations of system (4.7) that

and

We derive from the third equation of system (4.5) that

On substituting (4.8)–(4.10) into the fifth equation of system (4.7), one obtains that

where

We claim that all roots of Eq (4.11) have negative real parts. Otherwise, Eq (4.11) has at least one root $\lambda_2 = a_2 +{\rm i}b_2$ satisfying $a_2 \geq 0$. In this case, we have

Clearly, if $\mathscr{R}_0 > 1$, then $1+\frac{ \alpha d}{(\alpha d+ \beta)}(\mathscr{R}_0-1) > 1$, which mean that $\left| f_2(\lambda_2)\right| < 1,$ a contradiction. Therefore, if $\mathscr{R}_1 < 1 < \mathscr{R}_0$, the CTL-inactivated infection steady state $E_1$ is locally asymptotically stable.

In conclusion, we have the following result.

Theorem 4.1. For system (1.3) with the boundary condition (1.4), if $\mathscr{R}_0 < 1$, the infection-free steady state $E_0(\Lambda/d, 0, 0, 0)$ is locally asymptotically stable; if $\mathscr{R}_1 < 1 < \mathscr{R}_0$, $E_0$ is unstable and the CTL-inactivated infection steady state $E_1(x_1, y_1(a), v_1, 0)$ exists and is locally asymptotically stable.

5.   Uniform persistence

In this section, we investivate the uniform persistence of the semi-flow $\{\Phi(t)\}_{t\geq 0}$ generated by system (1.3) when the immune-activated reproduction rate $\mathscr{R}_1 > 1$.

Define

Noting that $k(\cdot), c(\cdot)\in L_+^\infty(0, \infty)$, we have $\bar{a}_1 > 0, \bar{a}_2 > 0$.

Denote

and

By ^[36] and using a similar argument as in the proof of Theorem 5.1 in ^[38], we have the following result.

Proposition 5.1. The subsets $\mathcal {Y}$ and $\partial \mathcal {Y}$ are both positively invariant under the semi-flow $\{\Phi(t)\}_{t\geq 0}$, namely, $\Phi(t, \mathcal {Y})\subset \mathcal {Y}$ and $\Phi(t, \partial \mathcal {Y})\subset \partial \mathcal {Y}$ for $t\geq 0$.

The following result is helpful to the proof of uniform persistence of the semi-flow $\{\Phi(t)\}_{t\geq 0}$ generated by system (1.3).

Theorem 5.1. The infection-free steady state $E_0(A/\mu, 0, 0, 0)$ is globally asymptotically stable for the semi-flow $\{\Phi(t)\}_{t\geq 0}$ restricted to $\partial \mathcal {Y}$.

Proof. Let $\left(x^0, y_0(\cdot), v^0, z^0\right)\in \partial \mathcal {Y}$. Then $(y_0(\cdot), v^0, z^0)\in \partial\tilde{\mathcal {Y}}$. We consider the following system

Since $\limsup_{t \rightarrow +\infty}x(t)\leq \Lambda/d$, by comparison principle, we have

where $\hat{y}(a, t), \hat{v}(t)$ and $\hat{z}(t)$ satisfy the following auxiliary system

Solving the first equation of system (5.3), we have

where

On substituting (5.5) into the second and the third equations of (5.3), it follows that

where

Since $(y_0(\cdot), v^0, z^0)\in \partial\tilde{\mathcal {Y}}$, we have $L_i(t)\equiv 0 (i = 1, 2)$ for all $t\geq 0$. It therefore follows from (5.6) that

It is easy to show that system (5.7) has a unique solution $\hat{v}(t) = 0, \hat{z}(t) = 0, \hat{L}(t) = 0$.

We obtain from (5.4) that $\hat{y}(a, t) = 0$ for $0\leq a < t$. For $a\geq t$, we have

which yields $\lim_{t \rightarrow +\infty}\hat{y}(a, t) = 0$. By comparison principle, it follows that $\lim_{t \rightarrow +\infty}y(a, t) = 0$ and $v(t) = 0, z(t) = 0$ as $t$ tends to infinity. We obtain from the first equation of system (1.3) that $\lim_{t \rightarrow +\infty}x(t) = \Lambda/d$. This completes the proof.

Using a similar argument as that in the proof of Theorem 5.1, we have the following result.

Theorem 5.2. The CTL-inactivated infection steady state $E_1(x_1, y_1(a), v_1, 0)$ is globally asymptotically stable for the semi-flow $\{\Phi(t)\}_{t\geq 0}$ restricted to $\partial \mathcal {Y}$.

Theorem 5.3. If $\mathscr{R}_1 > 1$, then the semi-flow $\{\Phi(t)\}_{t\geq 0}$ generated by system (1.3) is uniformly persistent with respect to the pair $(\mathcal {Y}, \partial \mathcal {Y})$; that is, there exists an $\varepsilon > 0$ such that $\liminf_{t \rightarrow +\infty}\|\Phi(t, x)\|_{\mathscr{X}}\geq \varepsilon$ for $x\in \mathcal {Y}$. Furthermore, there is a compact subset $\mathcal {A}_0\subset \mathcal {Y}$ which is a global attractor for $\{\Phi(t)\}_{t\geq 0}$ in $\mathcal {Y}$.

Proof. By Theorems 5.1 and 5.2, we see that the infection-free steady state $E_0(A/\mu, 0, 0, 0)$ and the CTL-inactivated infection steady state $E_1(x_1, y_1(a), v_1, 0)$ are globally asymptotically stable in $\partial \mathcal {Y}$. Hence, applying Theorem 4.2 in ^[35], in the following, we verify that

where

Here, we only show $W^s(E_1)\cap \mathcal {Y} = \emptyset$ holds since the proof of $W^s(E_0)\cap \mathcal {Y} = \emptyset$ is simple. Assume $W^s(E_1)\cap \mathcal {Y}\neq \emptyset$. Then there exists a solution $w\in \mathcal {Y}$ such that $\Phi(t, w) \rightarrow E_1$ as $t \rightarrow \infty$. In this case, one can find a sequence $\{w_n\}\subset \mathcal {Y}$ such that

where $\bar{w} = (x_1, y_1(a), v_1, 0)$.

Denote $\Phi(t, w_n) = (x_n(t), y_n(\cdot, t), v_n(t), z_n(t))$ and $w_n = (x_n(0), y_n(\cdot, 0), v_n(0), z_n(0))$. Since $\mathscr{R}_1 > 1$, we can choose $n$ sufficiently large satisfying $x_1-\frac{1}{n} > 0$ and

For such an $n > 0$, there exists a $T_1 > 0$ such that for $t > T_1$,

Consider the following auxiliary system

It is easy to show that if $\mathscr{R}_1 > 1$, system (5.10) has a unique steady state $E_0(0, 0, 0)$.

Looking for solutions of system (5.10) of the form $\tilde{y}(a, t) = \tilde{y}_1(a)e^{ \lambda t}, \tilde{v}(t) = \tilde{v}_1 e^{ \lambda t}, \tilde{z}(t) = \tilde{z}_1 e^{ \lambda t},$ where the function $\tilde{y}_1(a)$ and the constants $\tilde{v}_1, \tilde{z}_1$ will be determined later, we obtain the following linear eigenvalue problem:

We derive from the third equation of (5.11) that

Clearly, if $\mathscr{R}_1 > 1$, Eq (5.11) has at least one positive root $\lambda_0$, which yields the solution $(\tilde{y}(\cdot, t), \tilde{v}(t), \tilde{z}(t))$ of system (5.10) is unbounded. By comparison principle, the solution $\Phi(t, y_n)$ of system (1.3) is unbounded, which contradicts Proposition 5.1. Therefore, the semi-flow $\{\Phi(t)\}_{t\geq 0}$ generated by system (1.3) is uniformly persistent. Furthermore, there is a compact subset $\mathcal {A}_0\subset \mathcal {Y}$ which is a global attractor for $\{\Phi(t)\}_{t\geq 0}$ in $\mathcal {Y}$. This completes the proof.

6.   Global stability

In this section, we discuss the global stability of each of feasible steady states of system (1.3). The strategy of proofs is to use suitable Lyapunov functionals and LaSalles invariance principle. Further, we employ a Volterra type functional defined by $G(x) = x-1-\ln x$ in ^[37], which is positive and attains minimum value $0$ at $x = 1$.

We first give a result on the global stability of the infection-free steady state $E_0(\Lambda/d, 0, 0, 0)$ of system (1.3).

Theorem 6.1. If $\mathscr{R}_0 < 1$, the infection-free steady state $E_0(\Lambda/d, 0, 0, 0)$ of system (1.3) is globally asymptotically stable.

Proof. Let $(x(t), y(a, t), v(t), z(t))$ be any positive solution of system (1.3) with the boundary condition (1.4). Denote $x_0 = \Lambda/d.$

Define

where the positive constant $k_{1}$ and the nonnegative kernel function $F_1(a)$ will be determined later.

Calculating the derivative of $V_1(t)$ along positive solutions of system (1.3), it follows that

On substituting $\Lambda = d x_0$, $\frac{ \partial y(a, t)}{ \partial t} = -(\mu(a)+p(a)z(t))y(a, t)-\frac{ \partial y(a, t)}{ \partial a}$ into Eq (6.2), one obtains

Choose

Then, we have

We therefore obtain from (6.3)–(6.4) that

Clearly, if $\mathscr{R}_0 < 1$, we obtain from (6.5) that $V_1^{ \prime}(t)\leq 0$ and $V_1^{ \prime}(t) = 0$ implies that $x = x_0, y(a, t) = 0$ and $v = 0$. Hence, the largest invariant subset of $\{V_1^{ \prime}(t) = 0\}$ is the singleton $(x_0, 0, 0)$. Further, for $\varepsilon > 0$ sufficiently small satisfying $\int_{0}^{\infty}c(a)\varepsilon {\rm d}a-b < 0$, there is a $T > 0$, such that if $t > T$, $y(a, t) < \varepsilon$. It therefore follows from the fourth equation of system (1.3) that for $t > T$,

By comparison, we derive that

From Section 4, we see that if $\mathscr{R}_0 < 1$, $E_0$ is locally asymptotically stable. Accordingly, the global asymptotic stability of $E_0$ of system (1.3) follows from LaSalle's invariance principle. This completes the proof.

In the following, we establish the global asymptotic stability of the CTL-inactivated infection steady state $E_1(x_1, y_1(a), v_1, 0)$ and the global attractivity of the CTL-activated infection steady state $E^*(x^*, y^*(a), v^*, z^*)$ of system (1.3), respectively.

Denote

In order to guarantee the Lyapunov functional in proving the global stability of $E_1$ and $E^*$ is well-defined in infinite dimension, we make the following assumption:

$(H4)$ $x^0 > 0, v^0 > 0, z^0 > 0, \int_0^\infty \mid \ln y_0(a)\mid {\rm d}a < +\infty.$

We now define a positive function

Then, we have

and

Theorem 6.2. Assume there exists a positive constant $k_{2}$ satisfying $F_2(a)p(a) = k_{2}c(a)$. If (H4) holds, then the CTL-inactivated infection steady state $E_1(x_1, y_1(a), v_1, 0)$ of system (1.3) is globally asymptotically stable if $\mathscr{R}_1 < 1 < \mathscr{R}_0$.

Proof. Let $(x(t), y(a, t), v(t), z(t))$ be any positive solution of system (1.3) with the boundary condition (1.4).

Define

Using a similar argument as that in the proof of Lemmas 7.1 and 7.2 in ^[27], one can show that all integrals involved in $V_2(t)$ are finite.

Calculating the derivative of $V_2(t)$ along positive solutions of system (1.3), it follows that

On substituting $\Lambda = d x_1+ \beta x_1v_1/(1+ \alpha v_1)$ and $\frac{ \partial y(a, t)}{ \partial t} = -(\mu(a)+p(a)z(t))y(a, t)-\frac{ \partial y(a, t)}{ \partial a}$ into Eq (6.10), one obtains

A direct calculation shows that

On substituting Eq (6.12) into Eq (6.11), we have

Using integration by parts, it follows from Eq (6.13) that

On substituting Eqs (6.7)–(6.8) into Eq (6.14), and noting that $y_1'(a) = -\mu(a)y_1(a),$ $y_1(0) = \beta x_1v_1/(1+ \alpha v_1)$ and $y(0, t) = \beta x(t)v(t)/(1+ \alpha v(t))$, we obtain from Eq (6.14) that

Noting that $\frac{ \beta x_1}{u(1+ \alpha v_1)}\int_{0}^{\infty}k(a)y_1(a){\rm d}a = \frac{ \beta x_1}{u(1+ \alpha v_1)}uv_1 = \frac{ \beta x_1v_1}{1+ \alpha v_1}$, we have from Eq (6.15) that

Since the function $G(x) = x-1-\ln x\geq 0$ for all $x > 0$ and $G(x) = 0$ holds iff $x = 1$. Hence, $V_2^{ \prime}(t)\leq 0$ holds if $\mathscr{R}_1 < 1$. It is readily seen from (6.16) that $V_2^{ \prime}(t) = 0$ if and only if

for all $a\geq 0$. It is easy to verify that the largest invariant subset of $\{V_2^{ \prime}(t) = 0\}$ is the singleton $E_1$. By Theorem 4.2, we see that if $\mathscr{R}_1 < 1 < \mathscr{R}_0$, $E_1$ is locally asymptotically stable. Therefore, using LaSalle's invariance principle, we see that if $\mathscr{R}_1 < 1 < \mathscr{R}_0$ and (H4) hold, the global asymptotic stability of $E_1$ follows. This completes the proof.

In the following, we define a positive function

It is easy to show that

and

Theorem 6.3. Assume there exists a positive constant $k_{3}$ satisfying $F_3(a)p(a) = k_{3}c(a)$. If $(H4)$ holds, then the CTL-activated infection steady state $E^*(x^*, y^*(a), v^*, z^*)$ of system (1.3) is globally attractive if $\mathscr{R}_1 > 1$.

Proof. Let $(x(t), y(a, t), v(t), z(t))$ be any positive solution of system (1.3) with the boundary condition (1.4).

Define

Using a similar argument as that in the proof of Lemmas 7.1 and 7.2 in ^[27], one can show that all integrals involved in $V_3(t)$ are finite.

Calculating the derivative of $V_3(t)$ along positive solutions of system (1.3), it follows that

On substituting $\Lambda = d x^*+ \beta x^*v^*/(1+ \alpha v^*)$ and $\frac{ \partial y(a, t)}{ \partial t} = -(\mu(a)+p(a)z(t))y(a, t)-\frac{ \partial y(a, t)}{ \partial a}$ into Eq (6.22), one obtains

A direct calculation shows that

On substituting Eq (6.24) into Eq (6.23), we get

Using integration by parts, it follows from Eq (6.25) that

On substituting Eqs (6.19)–(6.20) into Eq (6.26), and noting that

and

we obtain from Eq (6.26) that

Since the function $G(x) = x-1-\ln x\geq 0$ for all $x > 0$ and $G(x) = 0$ holds iff $x = 1$. Hence, $V_3^{ \prime}(t)\leq 0$ holds if $\mathscr{R}_1 > 1$. It is readily seen from (6.27) that $V_3^{ \prime}(t) = 0$ if and only if

for all $a\geq 0$. We now look for the invariant subset $\mathscr{M}$ within the set

Because $x(t) = x^*, y(a, t) = y^*(a)$ and $v(t) = v^*$ on $\mathscr{M}$ and consequently, it follows from the second equation of system (1.3) that

which yields $z(t) = z^*.$ It is easy to verify that the largest invariant subset of $\{V_3^{ \prime}(t) = 0\}$ is the singleton $E^*$. Therefore, using LaSalle's invariance principle, we see that if $\mathscr{R}_1 > 1$ and (H4) hold, the global attractivity of $E^*$ follows. This completes the proof.

7.   Numerical simulations

In this section, we give some numerical examples for system (1.3) to illustrate the theoretical results in Sections 3 and 4. Based on the works of ^{[28,29,30,31,32,33]}, parameter values of system (1.3) are summarized in Table 2. In the following, we will use the finite difference method ^[34] for all numerical simulations. Further, to ensure the precision of numerical simulations, time- and age-steps are both set as $0.05$.

As argued by Markowitz et al. ^[30], the faster rate of loss of virus-producing cells shows that the generation time for HIV-1 in vivo is correspondingly shorter, $\sim2.0$ days, which is obtained by summing up some factors, such as the eclipse time of $\sim1.0$ $day$. This value indicates that HIV-1 typically undergoes $180$ generations per year in an infected person. Thus, the death rate of infected cells $\mu_m$ in Table 2 is set as $0.7$ ${\rm day}^{-1}$.

7.1.   Dynamical behaviors of system (1.3)

When the viruses invade through cytomembrane, infected cells cannot die immediately, due to that it takes some time for viruses to replicate, transcribe and translate. For this reason, we assume that the death rate of infected cells increases from $0$ to a peak value $\mu_m$ with the infection age. The age-dependent per capita death rate is set as

where ${a_0}$ denotes the mean value of the time for viruses to replicate, transcribe and translate (in this section, $a_0$ is set as $5$ day). Further, the maturing rate of new T cells and the kill ratio by T cells are selected as follows:

where $c_m$ and $p_m$ are the peak levels of $c(a)$ and $p(a)$, respectively. As for the viral production rate of infected cells, it keeps at $0$ for a short time $a_1$, and then increases from $0$ to a peak value $k_m$. Based on the works of ^[9,11], the specific function is set as follows

where $\theta$ determines how quickly $k(a)$ reaches the saturation level $k_m$. For simplicity, we assume that $\theta = 1$ and ${a_1} = 0.5$ day in the following numerical simulations.

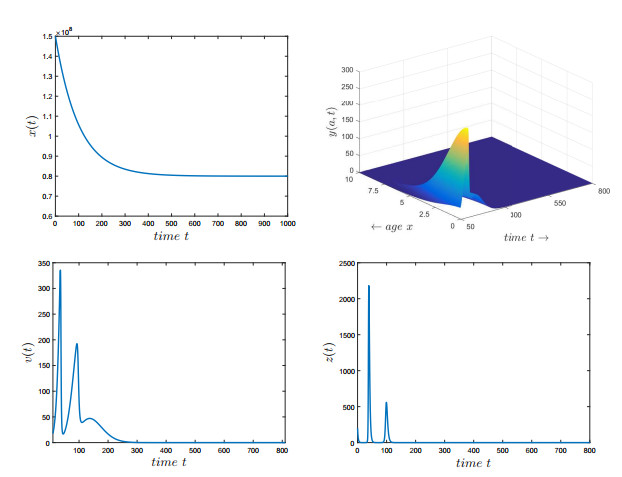
We first choose parameter values as in Case 1 of Table 2. Then we have the basic reproduction number $\mathscr{R}_0 = 0.8227 < 1$. By Theorem 4.1, we see that the infection-free steady state $E_0(80003167.69, 0, 0, 0)$ is locally asymptotically stable. Numerical simulation illustrates this fact (see Figure 1).

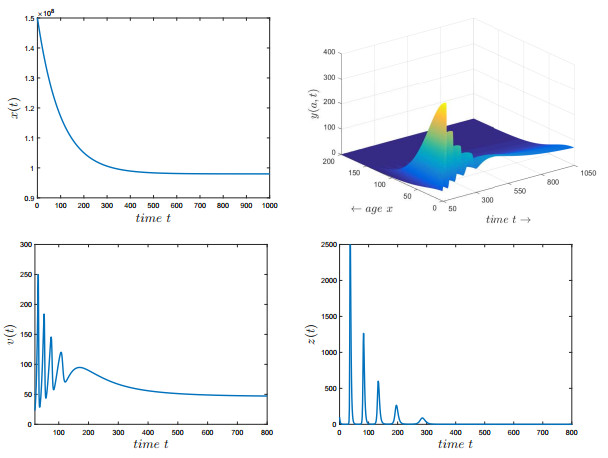
Next, we choose parameter values as in Case 2 of Table 2. By direct calculation, we get the basic reproduction number $\mathscr{R}_0 = 1.0079 > 1$ and the immune response reproduction number $\mathscr{R}_1 = 0.9939 < 1$. By Theorem 4.1, we see that in addition to the infection-free steady state $E_0(80003167.69, 0, 0, 0)$, system (1.3) has a CTL-inactivated infection steady state $E_1(97994001.72, 59.69\phi_1(a), 47.19, 0)$ which is locally asymptotically stable. Numerical simulation illustrates this fact (see Figure 2).

Remark 7.1. For system (1.3), a direct calculation shows that the characteristic equation of system (1.3) at the CTL-activated infection steady state $E^*$ is of the form

where

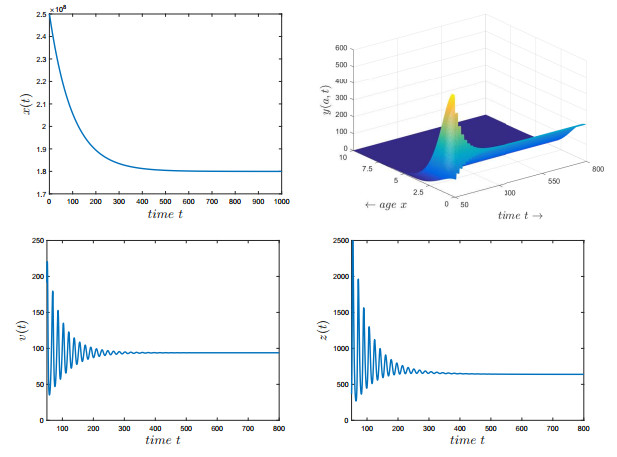
We failed in studying the local asymptotic stability of $E^*$ due to the complexity of Eq (7.4). In particular, we choose parameter values as in Case 3 of Table 2. By calculation, we have the immune-activated reproduction rate $\mathscr{R}_1 = 1.8256 > 1$. As can be seen from the discussion in Section 3, in addition to the infection-free steady state $E_0$ and the CTL-inactivated infection steady state $E_1$, system (1.3) has a unique CTL-activated infection steady state $E^*(179981502.93,216.65\phi_1(a)$ $\phi_2(a, 638.84), 93.90,638.84)$. Numerical simulation indicates that if $\mathscr{R}_1 > 1$, the CTL-activated infection steady state $E^*$ is locally asymptotically stable in some special cases (see Figure 3).

7.2.   The effects of CTL response

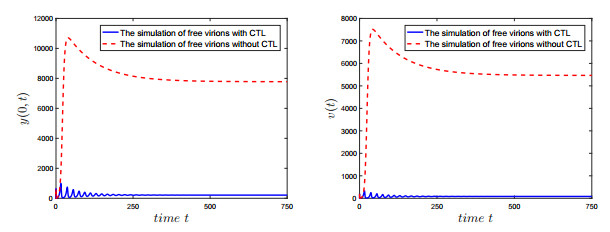
In order to investigate the effects of CTL immune response, we carry out the following numerical simulations. For convenience, parameter values are chosen as in Table 2. From Figure 4, it is clear that the concentrations of infected cells and free virions with CTL immune response is obviously lower than those without CTL immune response, which indicates that CTL immune response indeed has an important impact on infected cells and free virions and can help our body to eliminate the virions.

From Figure 5, we further observe that when the proliferate rate of virus-specific CTL cells $c_m$ increase from $0.002$ to $0.004$ $({\rm day}^{-1})$, both infected cells and free virions decreases to lower levels. This implies that CTL response can effectively reduce the quantity of infected cells and the serum viral load.

Remark 7.2. In our model, the death rate and the viral production rate of infected cells, the killing rate of infected cells by CTL and the proliferate rate of virus-specific CTL cells are assumed to vary according to the time a cell has been infected. Compared with the standard CTL response models without age structure, age-structure has more realistic representations of the biology of HIV-1 infection.

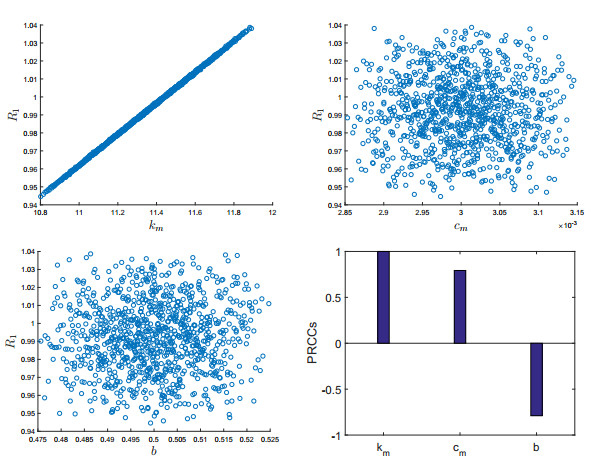
We now carry out the sensitivity analysis of $\mathscr{R}_1$. Through analysis of the sample derived from Latin hypercube sampling, we can obtain large efficient data in respect to different parameters of $\mathscr{R}_1$. The first three figures in Figure 6 shows the scatter plots of $\mathscr{R}_1$ in respect to $k_m$, $c_m$ and $b$, respectively, which implies that $k_m$ and $c_m$ are both positive correlative variables with $\mathscr{R}_1$; $b$ is negative correlative variable with $\mathscr{R}_1$. It is worth mentioning that $k_m$ contributes more to $\mathscr{R}_1$ compared to $c_m$, namely, $k_m$ is a more important factor in $\mathscr{R}_1$. The last figure in Figure 6 shows a tornado plot of partial rank correlation coefficients with respect to $\mathscr{R}_1$, indicating the importance of each parameter's uncertainty in contributing to $\mathscr{R}_1$ in the time to eradicate infection, which has the similar results to the first three figures in Figure 6.

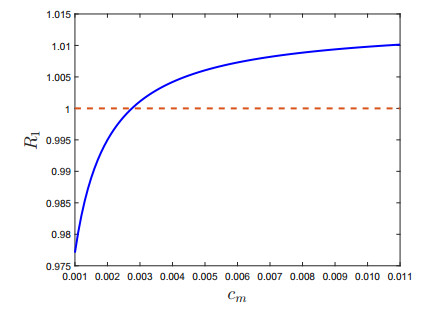
In the following, we carry out corresponding numerical simulations about the relation between the immune-activated reproduction rate $\mathscr{R}_1$ and the proliferate rate of virus-specific CTL cells $c_m$. As shown in Figure 7, we find that as the proliferate rate $c_m$ decreases, the value of $\mathscr{R}_1$ changes from greater than one to less than one.

8.   Conclusion

In this work, we have investigated an age-structured HIV-1 infection model with CTL immune response. The model allows the production rate of viral particles, the death rate of productively infected cells, the removed rate of infected cells and the proliferate rate of virus-specific CTLs to vary and depend on the infection age. By constructing suitable Lyapunov functionals and using LaSalle's invariance principle, we have investigated the global dynamics of each of feasible steady state of system (1.3). By Theorem 6.1, we see that if the immune-inactivated reproduction rate ${\mathscr{R}_0}$ is less than unity, the infection-free steady state is globally asymptotically stable. In this case, the virus is finally cleared up. By Theorem 6.2, we know that if the immune-activated reproduction rate ${\mathscr{R}_1}$ satisfies ${\mathscr{R}_1} < 1 < {\mathscr{R}_0}$, sufficient conditions are derived for the global stability of the CTL-inactivated infection steady state. In this case, the infection becomes chronic but without CTL immune response. If ${\mathscr{R}_1} > 1$, by Theorem 6.3, sufficient conditions are obtained for the global attractivity of the CTL-activated infection steady state. In this case, the infection turns to chronic with CTL immune response. We would like to point out here that Theorems 6.2 and 6.3 have room for improvement, we leave this for future work.

Acknowledgments

This work was supported by the National Natural Science Foundation of China (Nos. 11871316, 11801340, 11371368), the Natural Science Foundation of Shanxi Province (Nos. 201801D121006, 201801D221007).

Conflict of interest

The authors declare that they have no competing interests.