Citation: Cheng-Cheng Zhu, Jiang Zhu, Xiao-Lan Liu. Influence of spatial heterogeneous environment on long-term dynamics of a reaction-diffusion SVIR epidemic model with relaps[J]. Mathematical Biosciences and Engineering, 2019, 16(5): 5897-5922. doi: 10.3934/mbe.2019295
| [1] | Cheng-Cheng Zhu, Jiang Zhu . Spread trend of COVID-19 epidemic outbreak in China: using exponential attractor method in a spatial heterogeneous SEIQR model. Mathematical Biosciences and Engineering, 2020, 17(4): 3062-3087. doi: 10.3934/mbe.2020174 |
| [2] | Yongli Cai, Yun Kang, Weiming Wang . Global stability of the steady states of an epidemic model incorporating intervention strategies. Mathematical Biosciences and Engineering, 2017, 14(5&6): 1071-1089. doi: 10.3934/mbe.2017056 |
| [3] | Danfeng Pang, Yanni Xiao . The SIS model with diffusion of virus in the environment. Mathematical Biosciences and Engineering, 2019, 16(4): 2852-2874. doi: 10.3934/mbe.2019141 |
| [4] | Pengfei Liu, Yantao Luo, Zhidong Teng . Role of media coverage in a SVEIR-I epidemic model with nonlinear incidence and spatial heterogeneous environment. Mathematical Biosciences and Engineering, 2023, 20(9): 15641-15671. doi: 10.3934/mbe.2023698 |
| [5] | Azmy S. Ackleh, Keng Deng, Yixiang Wu . Competitive exclusion and coexistence in a two-strain pathogen model with diffusion. Mathematical Biosciences and Engineering, 2016, 13(1): 1-18. doi: 10.3934/mbe.2016.13.1 |
| [6] | Youshan Tao, J. Ignacio Tello . Nonlinear stability of a heterogeneous state in a PDE-ODE model for acid-mediated tumor invasion. Mathematical Biosciences and Engineering, 2016, 13(1): 193-207. doi: 10.3934/mbe.2016.13.193 |
| [7] | Wenzhang Huang, Maoan Han, Kaiyu Liu . Dynamics of an SIS reaction-diffusion epidemic model for disease transmission. Mathematical Biosciences and Engineering, 2010, 7(1): 51-66. doi: 10.3934/mbe.2010.7.51 |
| [8] | Nalin Fonseka, Jerome Goddard Ⅱ, Alketa Henderson, Dustin Nichols, Ratnasingham Shivaji . Modeling effects of matrix heterogeneity on population persistence at the patch-level. Mathematical Biosciences and Engineering, 2022, 19(12): 13675-13709. doi: 10.3934/mbe.2022638 |
| [9] | Liqiong Pu, Zhigui Lin . A diffusive SIS epidemic model in a heterogeneous and periodically evolvingenvironment. Mathematical Biosciences and Engineering, 2019, 16(4): 3094-3110. doi: 10.3934/mbe.2019153 |
| [10] | Baoxiang Zhang, Yongli Cai, Bingxian Wang, Weiming Wang . Dynamics and asymptotic profiles of steady states of an SIRS epidemic model in spatially heterogenous environment. Mathematical Biosciences and Engineering, 2020, 17(1): 893-909. doi: 10.3934/mbe.2020047 |
To the Editor,
With great interest, we have read the article by Romilda et al. entitled "Human biological monitoring of mercury for exposure assessment" that is published in the journal of AIMS Environmental Science Volume 4, Issue 2, 251–276 [1]. In this article, the authors have reviewed the different human biological monitoring (HBM) approaches for short-and long-term exposure to different chemical forms of mercury. The paper authored by Romilda et al. is a well-structured article which can be considered a significant contribution in this field. However, this commentary addresses another aspect of Hg biomonitoring and human health. Today, growing advances in modern technologies such as telecommunication and the rapid rise in the use of wireless systems have drastically increased the human exposure to different sources of electromagnetic fields (EMFs). Over the past several years, we have shown that dental amalgam fillings exposed to EMFs produced by magnetic resonance imaging (MRI) or those generated by very common sources such as mobile phones and Wi-Fi routers, show higher levels of mercury release from dental amalgam restorations [2,3]. It is worth noting that our experiments were not limited to in-vitro studies (using extracted teeth) [4] and our in-vivo [2,3] studies showed the same findings. Furthermore, it cannot be claimed that in our studies the release of mercury from dental amalgam fillings was under the influence of electromagnetic fields which cannot be extrapolated to in-vivo data from human biomonitoring (blood, urine, hair). Due to great advances in telecommunication technology and wireless systems, people are now continuously exposed to inescapable manmade electromagnetic fields in in the home or workplace.
Findings obtained by other researchers who later studied the microleakage of amalgam further confirmed the results of our early studies which indicated EMF-induced accelerated mercury release [5,6]. Furthermore, moving to the high energy ionizing portion of the wide spectrum of electromagnetic fields, it was shown that x-rays are also capable of increasing the mercury release from dental amalgam fillings [7]. It should be noted that the mercury levels which normally are released from amalgam fillings, even in the EMFs are present, are not high enough to cause toxicity. However, hypersensitive subpopulation, pregnant women and children may be affected by these low levels of mercury. Mortazavi and Mortazavi have recently reviewed these findings [8]. Considering the evidence provided above, the role of modern telecommunication technology and the substantial evidence which shows the role of exposure to EMFs on the release of mercury from dental amalgam restorations should be taken into account in future studies.
The authors declare there is no conflict of interest.
| [1] | F. Brauer and C. Castillo-Chavez, Mathematical Models in Population Biology and Epidemiology (Second Edition), Springer, 2012. |
| [2] | K. Dietz, The first epidemic model: A historical note on P.D. En'ko, Australian J. Stat., 30 (1988), 56–65. |
| [3] | H. F. Huo and X. M. Zhang, Complex dynamics in an alcoholism model with the impact of Twitter, Math. Biosci., 281 (2016), 24–35. |
| [4] | H. F. Huo and C. C. Zhu, Influence of relapse in a giving up smoking model, Abstr. Appl. Anal., (2013), 525461. |
| [5] | H. F. Huo and X. M. Zhang, Modelling effects of treatment at home on tuberculosis transmission dynamics, Appl. Math. Model., 40 (2016), 9474–9484. |
| [6] | C. Vargas-De-León, On the global stability of SIS, SIR and SIRS epidemic models with standard incidence, Chaos Solitons Fractals, 44 (2011), 1106–1110. |
| [7] | C. C. Zhu and J. Zhu, Stability of a reaction-diffusion alcohol model with the impact of tax policy, Comput. Math. Appl., 74 (2017), 613–633. |
| [8] | Z. Hu, L. Chang, Z. Teng, et al., Bifurcation analysis of a discrete SIRS epidemic model with standard incidence rate, Adv. Differ. Equ., (2016) 2016, 155. |
| [9] | Z. Jiang, W. Ma and J. Wei, Global hopf bifurcation and permanence of a delayed SEIRS epidemic model, Math. Comput. Simul., 122 (2016), 35–54. |
| [10] | A. M. Yousef and S. M. Salman, Backward bifurcation in a fractional-order SIRS epidemic model with a nonlinear incidence rate, Int. J. Nonlinear Sci. Numer. Simul., 17 (2016), 401–412. |
| [11] | H. Zhao and M. Zhao, Global hopf bifurcation analysis of an susceptible-infective-removed epi-demic model incorporating media coverage with time delay, J. Biol. Dyn., 11 (2016), 8–24. |
| [12] | S. Whang, S. Choi and E. Jung, A dynamic model for tuberculosis transmission and optimal treatment strategies in South Korea, J. Theor. Biol., 279 (2011), 120–131. |
| [13] | S. Choi and E. Jung, Optimal Tuberculosis Prevention and Control Strategy from a Mathematical Model Based on Real Data, Bull. Math. Biol., 76 (2014), 1566–1589. |
| [14] | Y. Hosono and B. Ilyas, Travelling waves for a simple diffusive epidemic model, Math. Model Meth. Appl. Sci., 5 (1995), 935–966. |
| [15] | W. T. Li, G. Lin, C. Ma, et al., Traveling wave solutions of a nonlocal delayed SIR model without outbreak threshold, Discrete Contin. Dyn. Syst. Ser. B, 19 (2014), 467–484. |
| [16] | R. Peng and X. Q. Zhao, A reaction–diffusion SIS epidemic model in a time-periodic environment, Nonlinearity, 25 (2012), 1451–1471. |
| [17] | X. Q. Zhao, Global dynamics of a reaction and diffusion model for Lyme disease, J. Math. Biol., 65 (2012), 787–808. |
| [18] | Y. X. Zhang and X. Q. Zhao, A reaction-diffusion Lyme disease model with seasonality, SIAM J. Appl. Math., 73 (2013), 2077–2099. |
| [19] | Y. J. Lou and X. Q. Zhao, A reaction–diffusion malaria model with incubation period in the vector population, J. Math. Biol., 62 (2011), 543–568. |
| [20] | G. Webb, A reaction–diffusion model for a deterministic diffusive models, J. Math. Anal. Appl., 84 (1981), 150–161. |
| [21] | W. E. Fitzgibbon, M. E. Parrott and G. F. Webb, Diffusion epidemic models with incubation and crisscross dynamics, Math. Biosci., 128 (1985), 131–155. |
| [22] | H. R. Thieme, Spectral bound and reproduction number for infinite-dimensional population struc-ture and time heterogeneity, SIAM J. Appl. Math., 70 (2009), 188–211. |
| [23] | W. Wang and X. Q. Zhao, A nonlocal and time-delayed reaction-diffusion model of dengue trans-mission, SIAM J. Appl. Math., 71 (2011), 147–168. |
| [24] | W. Wang and X. Q. Zhao, Basic reproduction numbers for reaction-diffusion epidemic models, SIAM J. Appl. Dyn. Syst., 11 (2012), 1652–1673. |
| [25] | Z. F. Xie, Cross-diffusion induced Turing instability for a three species food chain model, J. Math. Anal. Appl., 388 (2012), 539–547. |
| [26] | K. Hattaf and N. Yousfi, Global stability for reaction–diffusion equations in biology, Comput. Math. Appl., 67 (2014), 1439–1449. |
| [27] | E. Latosa and T. Suzuki, Global dynamics of a reaction–diffusion system with mass conservation, J. Math. Anal. Appl., 411 (2014), 107–118. |
| [28] | J. B. Wang, W. T. Li and F. Y. Yang, Traveling waves in a nonlocal dispersal SIR model with nonlocal delayed transmission, Commun. Nonlinear Sci. Numer. Simul., 27 (2015), 136–152. |
| [29] | Z. Xu, Traveling waves for a diffusive SEIR epidemic model, Comm. Pure Appl. Anal., 15(2016), 871–892. |
| [30] | Z. Xu and C. Ai, Traveling waves in a diffusive influenza epidemic model with vaccination, Appl. Math. Model., 40 (2016), 7265–7280. |
| [31] | C. C. Zhu, W. T. Li and F. Y. Yang, Traveling waves in a nonlocal dispersal SIRH model with relapse, Comput. Math. Appl., 73 (2017), 1707–1723. |
| [32] | C. C. Zhu, W. T. Li and F. Y. Yang, Traveling waves of a reaction-diffusion SIRQ epidemic model with relapse, J. Appl. Anal. Comput., 7 (2017), 147–171. |
| [33] | X. Bao, W. Shen and Z. Shen, Spreading speeds and traveling waves for space-time periodic nonlocal dispersal cooperative systems, Commun. Pure Appl. Anal., 18 (2019) 361–396. |
| [34] | K. Yamazaki and X. Wang, Global well-posedness and asymptotic behavior of solutions to a reaction-convection-diffusion cholera epidemic model, Discrete Contin. Dyn. Syst. Ser. B, 21 (2016), 1297–1316. |
| [35] | L. J. S. Allen, B. M. Bolker, Y. Lou, et al., Asymptotic profiles of the steady states for an SIS epidemic reaction-diffusion model, Discrete Contin. Dyn. Syst., 21 (2008), 1–20. |
| [36] | R. Peng, Asymptotic profiles of the positive steady state for an SIS epidemic reaction–diffusion model. Part I, J. Differ, Eq,, 247 (2009), 1096–1119. |
| [37] | R. Peng and S. Liu, Global stability of the steady states of an SIS epidemic reaction-diffusion model, Nonlinear Anal., 71 (2009), 239–247. |
| [38] | R. Peng and F. Yi, Asymptotic profile of the positive steady state for an SIS epidemic reaction–diffusion model: effects of epidemic risk and population movement, Phys. D, 259 (2013), 8–25. |
| [39] | T. Kuniya and J. Wang, Lyapunov functions and global stability for a spatially diffusive SIR epidemic model, Appl. Anal., (2016) http://dx.doi.org/10.1080/00036811.2016.1199796. |
| [40] | K. Deng and Y. Wu, Dynamics of a susceptible–infected–susceptible epidemic reaction–diffusion model, Proc. Roy. Soc. Edinburgh Sect. A, 146 (2016), 929–946. |
| [41] | Y. Wu and X. Zou, Asymptotic profiles of steady states for a diffusive SIS epidemic model with mass action infection mechanism, J. Differential Equations, 261 (2016), 4424–4447. |
| [42] | H. Li, R. Peng and F. B. Wang, Varying total population enhances disease persistence: Qualitative analysis on a diffusive SIS epidemic model, J. Differ. Equ., 262 (2017), 885–913. |
| [43] | Y. Cai, Y. Kang, M. Banerjeed, et al., Complex Dynamics of a host–parasite model with both horizontal and vertical transmissions in a spatial heterogeneous environment, Nonlinear Anal. Real World Appl., 40 (2018), 444–465. |
| [44] | Y. Tong and C. Lei, An SIS epidemic reaction–diffusion model with spontaneous infection in a spatially heterogeneous environment, Nonlinear Anal. Real World Appl., 41 (2018), 443–460. |
| [45] | R. Temam, Infinite-Dimensional Systems in Mechanics and Physics, Springer-Verlag, New York, 1997. |
| [46] | J. C. Robinson, Infinite-Dimensional Dynamical Systems: An Introduction to Dissipative Parabolic PDEs and the Theory of Global Attractors, Cambridge University Press, 2001. |
| [47] | M. Yang and C. Y. Sun, Exponential attractors for the strongly damped wave equations, Nonlinear Anal. Real World Appl., 11 (2010), 913–919. |
| [48] | T. Ma, The theory and method of partial differential equation, Science Press, Beijing, 2011. |
| [49] | T. Ma and S. Wang, Phase Transition Dynamics, Springer Science+Business Media, LLC 2014. |
| [50] | Y. Li, H. Wu and T. Zhao, Necessary and sufficient conditions for the existence of exponential attractors for semigroups, and applications, Nonlinear Anal., 75 (2012), 6297–6305. |
| [51] | Y. Zhong and C. K. Zhong, Exponential attractors for reaction-diffusion equations with arbitrary polynomial growth, Nonlinear Anal., 71(2009), 751–765. |
| [52] | J. Zhang and C. K. Zhong, The existence of global attractors for a class of reaction–diffusion equations with distribution derivatives terms in Rn, J. Math. Anal. Appl., 427 (2015), 365–376. |
| [53] | J. Zhang, P. E. Kloeden, M. Yang, et al., Global exponential κ-dissipative semigroups and expo-nential attraction, Discrete Contin. Dyn. Syst. Ser., 37(2017), 3487–3502. |
| [54] | J. Zhang, Y. Wang and C. K. Zhong, Robustness of exponentially κ-dissipative dynamical systems with perturbation, Discrete Contin. Dyn. Syst. Ser. B, 22 (2017), 3875–3890. |
| [55] | X. Wang and J. Wang, Analysis of cholera epidemics with bacterial growth and spatial movement, J. Biol. Dyn., 9 (2015), 233–261. |
| [56] | N. K. Vaidya, F. B. Wang and X. Zou, Avian in influenza dynamics in wild birds with bird mobility and spatial heterogeneous environment, Discrete Contin. Dyn. Syst. Ser. B, 17 (2012), 2829–2848. |
| [57] | K. Yamazaki, Global well-posedness of infectious disease models without life-time immunity: the cases of cholera and avian in influenza, Math. Med. Biol., 35 (2018), 428–445. |
| [58] | Q. X. Ye, Z. Y. Li, M. X. Wang, et al., Introduction to reaction–diffusion equations, second edition, Science Press, Beijing, 2011 (in Chinese). |
| [59] | H. L. Smith, Monotone dynamical systems, Mathematical Surveys and Monographs, vol.41, American Mathematical Society, Providence, RI, 1995. |
| [60] | I. I. Vrabie, C0 semigroups and application, Elsevier Science B.V., New York, 2003. |
| [61] | C. V. Pao, Nonlinear Parabolic and Elliptic Equations, New York, London: Plenum, 1992. |
| [62] | Z. Du and R. Peng, A priori L∞ estimates for solutions of a class of reaction-diffusion systems, J. Math. Biol., 72 (2016), 1429–1439. |
| [63] | Global hepatitis report, 2017. Available from: http://www.who.int/hepatitis/publications/global-hepatitis-report2017/en/. 64. World Health Statistics, 2013. Available from: http://www.who.int/. |
| 1. | Cheng-Cheng Zhu, Jiang Zhu, Dynamic analysis of a delayed COVID-19 epidemic with home quarantine in temporal-spatial heterogeneous via global exponential attractor method, 2021, 143, 09600779, 110546, 10.1016/j.chaos.2020.110546 | |
| 2. | Jinliang Wang, Ran Zhang, Toshikazu Kuniya, A reaction–diffusion Susceptible–Vaccinated–Infected–Recovered model in a spatially heterogeneous environment with Dirichlet boundary condition, 2021, 190, 03784754, 848, 10.1016/j.matcom.2021.06.020 | |
| 3. | Cheng-Cheng Zhu, Jiang Zhu, The effect of self-limiting on the prevention and control of the diffuse COVID-19 epidemic with delayed and temporal-spatial heterogeneous, 2021, 21, 1471-2334, 10.1186/s12879-021-06670-y | |
| 4. | Mohamed Mehdaoui, Abdesslem Lamrani Alaoui, Mouhcine Tilioua, Analysis of an optimal control problem for a spatio‐temporal SIR model with nonlinear density dependent diffusion terms, 2023, 0143-2087, 10.1002/oca.2976 | |
| 5. | Cheng-Cheng Zhu, Jiang Zhu, Spread trend of COVID-19 epidemic outbreak in China: using exponential attractor method in a spatial heterogeneous SEIQR model, 2020, 17, 1551-0018, 3062, 10.3934/mbe.2020174 | |
| 6. | Séverin Foko, Calvin Tadmon, Analysis of a delayed spatiotemporal model of HBV infection with logistic growth, 2024, 103, 0003-6811, 2283, 10.1080/00036811.2023.2291813 | |
| 7. | Yantao Luo, Yunqiang Yuan, Zhidong Teng, ANALYSIS OF A DEGENERATED DIFFUSION SVEQIRV EPIDEMIC MODEL WITH GENERAL INCIDENCE IN A SPACE HETEROGENEOUS ENVIRONMENT, 2024, 14, 2156-907X, 2704, 10.11948/20230346 | |
| 8. | Vasyl’ Davydovych, Vasyl’ Dutka, Roman Cherniha, Reaction–Diffusion Equations in Mathematical Models Arising in Epidemiology, 2023, 15, 2073-8994, 2025, 10.3390/sym15112025 | |
| 9. | Chang Zhai, Ping Chen, Zhuo Jin, Tak Kuen Siu, Epidemic modelling and actuarial applications for pandemic insurance: a case study of Victoria, Australia, 2024, 18, 1748-4995, 242, 10.1017/S1748499523000246 | |
| 10. | Cheng-Cheng Zhu, Jiang Zhu, Jie Shao, Epidemiological Investigation: Important Measures for the Prevention and Control of COVID-19 Epidemic in China, 2023, 11, 2227-7390, 3027, 10.3390/math11133027 | |
| 11. | Cheng-Cheng Zhu, Jiang Zhu, The existence conditions for global exponential attractor of non-autonomous evolution equations and applications, 2023, 173, 09600779, 113711, 10.1016/j.chaos.2023.113711 | |
| 12. | Pengfei Liu, Yantao Luo, Zhidong Teng, Role of media coverage in a SVEIR-I epidemic model with nonlinear incidence and spatial heterogeneous environment, 2023, 20, 1551-0018, 15641, 10.3934/mbe.2023698 | |
| 13. | Linhe Zhu, Yue Li, Le He, Shuling Shen, Pattern formation of network epidemic model and its application in oral medicine, 2025, 264, 01692607, 108688, 10.1016/j.cmpb.2025.108688 |
Figures(5) / Tables(2)
Cheng-Cheng Zhu, Jiang Zhu, Xiao-Lan Liu. Influence of spatial heterogeneous environment on long-term dynamics of a reaction-diffusion SVIR epidemic model with relaps[J]. Mathematical Biosciences and Engineering, 2019, 16(5): 5897-5922. doi: 10.3934/mbe.2019295
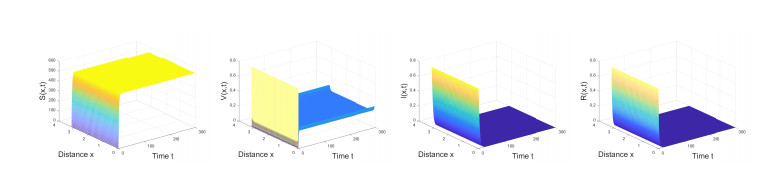
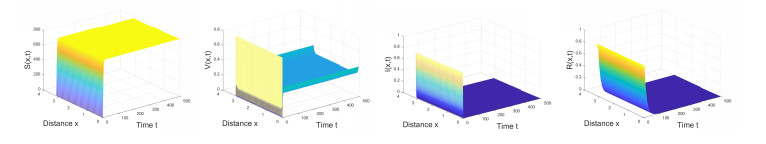
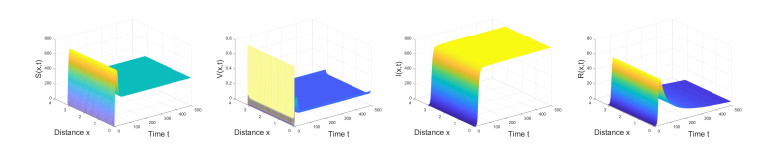
DownLoad: