Host heterogeneities such as space, gender, and age etc are intrinsic characters for investigating diseases mechanisms and transmission routes. First, we incorporate inter-group, intra-group and age structure to propose a multi-group SIVS epidemic model. Then we obtain the basic reproduction number of the system which is the spectral radius of the next generation operator by the renewal equation. Based on some assumptions for parameters, we obtain the existence and uniqueness of endemic equilibrium. By means of integral semigroup theory and Lyapunov methods, we show that the threshold dynamics of the system is completely determined by the basic
1.
Introduction
As history indicated, infectious diseases have been becoming a main enemy affecting human's health and economic development. Mathematical modelling is an useful tool to investigate the mechanisms of transmission of diseases and make optimal control measures [1]. There are many ways to suppress the disease transmission, such as media propagation, vaccination, quarantine and so on [2]. As we know, vaccination is one of effective methods to control and prevent disease prevalence. Indeed, vaccination has succeeded in slowing down transmission of diseases such as tuberculosis, hepatitis, and some children diseases [3]. However, it has been reported that vaccination immunity waning has caused some diseases reemergence such as measles, rubella and pertussis. There is no doubt that vaccine waning has great effects on understanding the evolution of diseases. Based on the epidemic compartment knowledge in Kermack and McKendrick [4,5,6], an SIVS epidemic model can be written as follows [7]:
where the total population is splited into four classes (Susceptible, Infected, Recovered and Vaccinated). b is the birth rate, μ is the natural death rate, β is the transmission rate, γ is the recovery rate, p is the vaccinated rate, ϵ is the vaccine waning rate. In [7], Li and Yang investigated two vaccine strategies consisting of continuous and impulsive styles, and they obtained the global stability of equilibria by constructing Lyapunov functionals. Zaleta and Hernández proposed an SIVS model with a standard incidence rate and a disease-induced death rate [8]. They showed that their model exhibits a backward bifurcation. Based on model (1), many researchers have evolved many different structures and successfully captured the key characters of diseases transmission and evaluated the risk of their prevalence (see, for examples, [9,10,11]).
We note that all models mentioned above are based on the homogeneous assumptions for host population. However, host heterogeneity plays an important role in exploring their dynamics. Many diseases such as tuberculosis, hepatitis C, HIV/AIDS and so on infect their host for a long time and sometimes for the duration of lifespan. During the long infectious period, the variability of infectivity with age-since-infection has been studied most extensively in HIV infection [12]. The immunity waning process of pertussis satisfies Gamma distributions [13], which can be expressed by the vaccinated age. In this case, the densities of the infected and vaccinated in time t and age a are denoted by i(t,a), and v(t,a), respectively. The parameters β,γ, and ϵ in system (1) are associated with age a. Based on system (1), the model can be described as follows:
where R+=(0,+∞), and L1+(R+) denotes the space of all the integral functions in L1 and maintaining positivity after integral. Obviously, system (2) is a hybrid system combining an ordinary differential equation and two partial differential equations. Global dynamics of such a system has been becoming a challenging issue due to lack of well posed mathematical techniques.
On the other hand, some diseases such as mumps, measles, gonorrhea, HIV/AIDS etc exhibit heterogeneity in host populations. Groups can be geographical such as counties, cities, communities, or epidemiological as different infectivity and multi-stain agents. Many authors introduced an irreducible matrix and summing elements of the matrix together as a kernel function to describe the inter-group and intra-group infections. In [14], Lajmanovich and Yorke proposed the earliest multi-group model for gonorrhea spread in a community and investigated the global stability. They assumed that the total size of population doesn't change and maintain a constant. Under this assumption their model can be simplified to just consider the infected classes. Since then, many multi-group epidemic models have been studied (see, for example [15,19,20]). In [21], Guo et al proposed a multi-group SIR epidemic model and used the graph-theoretic approach to investigate the global stability of endemic equilibrium. This method combining with nonnegative irreducible matrix is an effective tool in solving the global behavior of endemic equilibrium. In [20], Kuniya considered a multi-group SVIR model to explore the global behavior of equilibria by constructing Lyapunov functional and using a developed graph-theoretic method. There are few literatures incorporating age structure into multi-group epidemic models [15].
Motivated by the discussions above, we separate the total population into n groups and four compartments: susceptible, infected, and vaccinated, recovered, denoted by Sk(t),ik(t,a) and vk(t,a) and Rk(t), respectively. ik(t,a) denotes the infected individuals at time t and infection age a in group k. vk(t,a) denotes the vaccinated individuals at time t and vaccinated age a in group k. Rk(t) represents the recovered individuals at time t. Susceptible individuals in group k can be infected by infected individuals in group j at rate βkj(a). Hence, we denote the incidence rate in group k in the form of
Susceptible individuals in group k can be vaccinated at rate pk and become vaccinated individuals with immunity. The vaccinated individuals in group k lose its immunity at rate ϵk(a) and become susceptible individuals. A multi-group SIRVS epidemic model is formulated by the following differential equations:
where bk denotes the birth rate, γk is the recovery rate with respect to infection age a, μk is the natural death rate in group k. In order to satisfy the biological meaning, all the parameters are assumed to be nonnegative and bk>0 and μk>0. Note that the total population Nk(t)=Sk(t)+∫∞0ik(t,a)da+Rk(t)+∫∞0vk(t,a)da satisfies the following equation:
which yields limt→∞Nk(t)=bkμk. Without loss of generality, we assume that the total population is Nk=bkμk. Since the first four equations in (3) do not contain the variable Rk, we can consider the following closed subsystem:
Once behaviors of Sk(t), ik(t,a) and vk(t,a) are known, those of Rk(t) can be derived from the fourth equation in (3). For convenience, we make the following assumption:
Assumption 1.1. For system (5), we assume
(ⅰ) For each j,k∈{1,2,⋯,n}, βjk(a),γk(a),ϵk(a)∈L∞+(0,∞). That is, there exist positive constants β+jk and ϵ+k such that
(ⅱ) For each j,k∈{1,2,⋯,n}, βjk(a) satisfies the following property:
(ⅲ) For each j,k∈{1,2,⋯,n}, there exists an ε0>0 such that for almost all a∈[0,+∞),βjk(a)≥ε0.
(ⅳ) For each j,k∈{1,2,⋯,n}, the matrix (βjk)n×n is irreducible.
The assumptions above on the parameters in system (5) are naturally satisfied for some real diseases. Evidence exists that the infectivity βjk(a) has been addressed by Gamma and Log-normal distributions for smallpox [16,17], Weibull distribution for Ebola [18]. It is easy to find that all of these probability distribution functions have peak values and they are continuous. Hence, (ⅰ) and (ⅱ) in Assumption 1.1 readily hold. As for (ⅲ) in Assumption 1.1, we can modify it in a more generalized form:
(ⅲ)' There exists a positive constant}aβ such that βjk(a)(k,j∈N) is positive in a neighbourhood of aβ.
Obviously, the distribution functions mentioned above have this property. If we assume that every group keeps up close exchanges in mutual contact, then the generated graph is strongly connected. (ⅳ) in Assumption 1.1 is satisfied automatically.
2.
Well-posedness of the problem
In order to investigate the dynamic behavior of system (5), define the functional spaces
and
with the norm
where ψi=(ψi1,ψi2,⋯,ψin)T∈Rn or L1(R+,Rn)(i=1,2,3). In addition, denote X+,Y+ and Z+ as the positive cones of X,Y and Z. We define
Under Assumption 1.1 we see that the set
is invariant, where S=(S1,S2,⋯,Sn)T, i=(i1,i2,⋯,in)T and v=(v1,v2,⋯,vn)T. In the following, we just assume that all the initial values are taken from Ω.
Next, we will show that system (5) has a globally classic solution in Ω. Let b,p,μ,γ(a), and ϵ(a) be diagonal matrixes given by
Then let us define a linear operator Ai:D(Ai)⊂X→X as
and
where D(Aj)={(0,ψ)∈X0|ψ is obsolutely continuous,ψ′∈L1(R+,Rn),ψ(0)=0}, j=i,v. If λ∈ρ(Ai) (ρ(Ai) denotes the resolvent set of Ai), for any initial value (θi,ϕi)T∈X, we have
Similarly, for any λ∈ρ(Av) and any initial value (θv,ϕv)T∈X, we have
Furthermore, define two nonlinear operators as
where Bi(ϕ)=Sn∑j=1∫∞0βj(a)ϕij(a)da and Bv(ϕ)=pϕS. We further define another nonlinear operator
If we set u=(S,(0i),(0v))T∈Z, A=diag(−μ,Ai,Av), and F=(Fs,Fi,Fv), (5) can be rewritten as the following abstract Cauchy problem
Proposition 2.1. There exists a uniquely determined semiflow {U(t)}t≥0 on Z0+ such that, for each u=(S(t),(0i(t,⋅)),(0v(t,⋅)))∈Z0+, there exists a unique continuous map U∈C(R+,Z0+) which is an integral solution of the Cauchy problem (9), that is, for all t≥0,
Proof. By the definition of A and equations (7) and (8), A is dense in part of X0 (see Page 1117, , [22]), and the resolvent operator is bounded. From Proposition 3.2 in, [23], we need only to verify that F satisfies Lipschitz condition. Denote
and
By calculation, we have
Similarly, we have ‖Flk(xk)−Flk(ˉx)‖≤Llk‖x−ˉx‖,l=i,v, where Lik=bkμkn∑j=1β+kj, and Lvk=pk. Therefore, ‖F(x)−F(ˉx)‖≤n∑k=1‖Fk(xk)−F(ˉxk)‖≤L‖x−ˉx‖, where L=max{LSk,Lik,Lvk}.
In order to prove the positivity of the solution of (5), we first rewrite the operator Av and the nonlinear function Fvk as follows:
and
Denote A′=diag(μ,Ai,A′v), and F′=(FS,Fi,F′v). Then system (9) can be written as
It is obvious that eA′tu0∈Ω+ if u0∈Ω+, where Ω is the positive cone of Ω. Then we need to show that
For any ψlk∈R and ϕlk∈Ω+, it follows from the definitions of A′ and F′ that
On the other hand, choose a κk∈R+ and redefine the operator in (12) as follows:
Aκk=diag(−(μk+κk),Aiκk,Avκk), where
and
The nonlinear functions in (12) are defined as Flκk(ϕ)=Flk+κk‖ϕk‖(l=S,i,v) for any ϕ∈Z. Hence, we have
and
If we choose κk>max{pk+n∑j=1β+kj‖ϕij‖,ϵ+k}, it follows from (14)-(16) that
From what has been discussed above, we have the following result.
Proposition 2.2.
If Assumption 1.1 holds, system (5) has a unique positive solution in Ω.
3.
The basic reproduction number
In this section, we show the computation process of the basic reproduction number R0, which is the average number of secondary cases produced by a classical infected individual during its infectious period in a fully susceptible population. Many literatures have given different methods to solve this problem. In this paper, it follows from the renewal process mentioned in Diekman et al in [24] that its value is determined by a next generation operator. Note that E0=(ˆS0,0,0,0,ˆv0(a)) is the disease-free equilibrium of system (5) where ˆS0=(S10,S02,⋯,S0n) and ˆv0(a)=(v01(a),v02(a),⋯,v0n(a)) with S0k=bkμk+pk(1−K1k),v0k(a)=pkS0kπ1k(a), and π1k(a)=e−∫a0(μk+ϵk(s))ds, K1k=∫∞0ϵk(a)π1k(a)da. Linearizing system (5) at the disease-free equilibrium E0, the subsystem (5) can be rewritten as
where
and
Let B:D(B)⊂X→X be a linear operator defined by
where μ and γ are defined in (6). Based on the above definition, (7) can be rewritten as the following linear Cauchy problem in Z0:
Let u(t)=etB be the C0 semigroup generated by the generator B. By the variation of constants formula, we have
Mapping S0Λ on both sides of the above equation yields
where v(t)=S0ΛI denotes the density of newly infectives in the linear invasion phase, f(t)=S0Λu(t)I0 and Ψ(s)=ΛS0u(s). Then the next generation operator is defined by
where we used the relation (z−B)−1=∫∞0e−zsV(s)ds, for z∈ρ(B)(ρ(B) denotes the resolvent set of B) and 0∈ρ(B). In fact, the next generation operator is calculated as follows:
where
Based on Diekmann et al [24], the basic reproduction number R0=r(K) is the spectral radius of the next generation operator K, where r(A) denotes the spectral radius of a bounded operator A. It follows from Inaba [25] that Malthusian parameter or asymptotic growth rate of infectives is positive if R0>1, otherwise it is negative.
4.
Existence of endemic equilibria
In this section, we focus on the existence of the endemic equilibrium E∗ of system (5). It follows from Section 2 that equilibria of system (5) satisfy Au∗+F(u∗)=0. Actually, it satisfies the following equations
From the last two equations of (23), we have
Substituting (24) into the first equation of (23) yields
It follows from the second and the third equations of (23) that
Note that
where Kkj=∫∞0βkj(a)πj(a)da. Hence, we can define a nonlinear operator
where
In fact, it follows from (26) that the endemic equilibrium of (23) is a positively nontrivial fixed point of the operator H. Note that the Fréchet derivative of H at ϕ=0 is given by
Let
where
Consequently, H′[0] is equal to the next generation operator K defined by (21).
Next, we show that R0 determines the existence of the positive fixed point of operator H. Under Assumption 1.1, the following lemma holds.
Lemma 4.1.
Let K be defined by (21). We have
(a) K is compact.
(b)K is nonsupporting.
Proof. Assume that B0 is an arbitrary bounded subset of R. Then there exists a positive constant c0 such that ‖ϕ‖≤c0 for all ϕ∈B0. Note that
(28) implies that the operator K is bounded. It follows from Fréchet-Kolmogorov Theorem [31] that the operator K is compact. It is obvious that K is nonsupporting under (ⅲ) or (ⅲ)' of Assumption 1.1.
Lemma 4.1, together with the monotonicity of the operator H with respect to ϕ manifests that the following lemma holds.
Lemma 4.2. Let H be defined (27).
H is compact and H(R+) is bounded.
Employing Theorem 4.11 in [26] (Krasnoselskii fixed theorem) and Krein-Rutman Theorem in [27], Lemma 4.2 implies that r(K) is the uqiue eigenvalue of the operator K associated with a positive eigenvector and there is no eigenvector of K associated with eigenvalue 1. As a consequence of Corollary 5.2 in [15], the following result is immediate.
Proposition 4.1.
If R0>1, then H has at least one nontrivial fixed point in Z0+.
Corollary 4.1.
If R0>1, then system (5) has at least one positive endemic equilibrium E∗=(S∗,0,i∗,0,v∗)∈Z0+, where S∗=(S∗1,S∗2,⋯,S∗n),i∗=(i∗1,i∗2,⋯,i∗n),v∗=(v∗1,v∗2,⋯,v∗n).
In order to determine the uniqueness of the solution of system (23), we define two operators by (ⅱ) and (ⅲ) of Assumption 1.1 as follows:
and for a small positive value δ
For convenience, define
and
It is obvious that 0≤H−(ϕ)e≤H(ϕ)e≤H+(ϕ)e, where e=(1,1,⋯,1)T.
Theorem 4.3.
If R0>1, then the operator H defined in (27) has at most one nontrivial fixed point in Z0+.
Proof. By Proposition 4.1, we assume that there are two different nontrivial fixed points in R+, denoted by ϕ∗ and ˆϕ∗. Borrowing the definitions of H− and H+, we have
Hence, there exists a positive constant q=sup{r≥0,ϕ∗≥rˆϕ∗}>0. Suppose 0<q<1, for all ϕ∈R+ and k∈N, then
where ξk(ϕ,q)=q(1−q)n∑j=1bjϕj(μj+pj(1−K1j)+ϕj)(μj+pj(1−K1j)+qϕj)Kkj. It follows from 0<q<1 and (ⅲ) of Assumption 1.1 that ξk is positive for all k∈N+ and ϕ∈R+. Furthermore,
Inequality (30), together with the monotonicity of H implies that
This contradicts the definition of q. Therefore, q≥1 and ϕ∗≥qˆϕ∗≥ˆϕ∗. Exchanging the role of ϕ∗ and ˆϕ∗, we can prove ϕ∗≤ˆϕ∗. This implies that ϕ∗=ˆϕ∗.
5.
Asymptotic smoothness
In this section, we will show the relative compactness of the positive orbit {U(t,u0)}t≥0 defined by (5). This process is spurred by Lemma 19 in [28] and Theorem 2.46 in [29]. To apply them, we define
and
Then we can divide the solution of semigroup U(t) into two parts:
and
where
This implies that U(t)u0=V(t)u0+W(t)u0.
Theorem 5.1.
The semiflow U:R+×X0→X0 is asymptotically smooth if there are maps V(t),W(t):R+×X0→X0 such that U(t)u0=V(t)u0+W(t)u0, and the following statements hold for any bounded closed set Ω that is forward invariant under U:
(i) For any u0∈Ω, there exists a function δ:R+×R+→R+ such that for any r>0 limt→+∞δ(t,r)=0 with ‖u0‖Ω≤r, then ‖V(t,u0)‖Ω≤δ(t,r);
(ii) there exists a tΩ≥0 such that W(t)(Ω) has a compact closure for each t≥tΩ.
Lemma 5.2.
There exists a function δ:R+×R+→R+ such that for any r>0,
and
Proof. Integrating ik and vk equations along the characteristic line t−a= const. yields
for all k∈N. Hence for any u0∈Y and ‖u0‖Y≤r,
where δ(t,r)=e−μ_tr and μ_=mink∈N{μk}. Obviously, ‖V(t)u0‖Ω approaches 0 as t goes to infinity.
Lemma 5.3.
W(t) maps any bounded subsets of Ω into sets with compact closure in Y.
Proof. It follows from the first equation of (5) that Sk(t) remains in the compact set {ϕ∈Rn+|0≤ϕk≤bkμk,k∈N} for all t≥0. Therefore, we need to show that ˜i and ˜v still remain in pre-compact subsets of Y0 which is independent of u0. Note that
Since for all k∈N+, 0≤˜ik(t,a)=ik(t−a,0)πk(a)≤bkμ_2n∑j=1β+kjbje−μ_a:=Δke−μ_a where Δk=bkμ_2n∑j=1β+kjbj, and 0≤˜vk(t,a)≤bkpkμke−μ_a. It is easy to see that (ⅰ) - (ⅲ) of Theorem B.2 in [29] hold.
In what follows, we need to show that (iv) of Theorem B.2 in [29] also holds. Assume that h∈(0,t) without loss of generality. Then
Similarly,
Obviously, both (36) and (48) uniformly converge to 0 as h\rightarrow0 which is independent of u_0.
Lemmas 5.2 and 5.3, together with \|\mathcal U(t)u_0\|_{Z_0}\le\sum\limits_{k = 1}^n\frac{b_k}{\mu_k}, imply that \mathcal U(t) has compact closure in Z for u_0\in\Omega. It follows from Proposition 3.13 in [32] that the solution orbit is relatively compact and the semiflow \mathcal U(t) is asymptotically smooth.
Proposition 5.1. The semiflow \mathcal U(t) defined in (10) is asymptotically smooth.
6.
Uniform persistence
In this section, we establish the uniform persistence of (5) when \mathcal R_0>1. This property guarantees the well-definition of the Lyapunov functionals in Section 7. For some k\in\mathbb N, define \rho_k: \Gamma\to \mathbb{R}_+ and u_{0} = ({\bf S_{0}, {\bf 0}, i_0(\cdot), {\bf 0}, v_0(\cdot)})\in \Omega_0 by
Let
Obviously, if u_0\in \Omega\setminus \Omega_0, then ({\bf S}(t), {\bf0, i}(t, \cdot), {\bf0, v}(t, \cdot))\to E_0 as t\to\infty. Hence, if \mathcal U has a global compact attractor in \Omega_0, then it also has a global compact attractor in \Omega.
Assumption 6.1. The support of the initial age-since-infection value i_{k0}(a)\in L^1(\mathbb R_+) lies to the right of the support the infectivity function \beta_{kj} for all k, j\in\mathbb N.
Proposition 6.1.
If \mathcal {R}_0>1 and Assumption 6.1 hold, then system (5) is uniformly weakly \rho-persistent for some k\in\mathbb N.
Proof. Since \mathcal{R}_0>1, there exists an \eta_{j0}>0 and \phi_j\in \mathbb R_+ for k, j\in\mathbb N such that
where \tilde S_k^0(\eta_{k0}) = \frac{b_k}{\mu+\eta_{k0}+p_k(1-K_k^1)}-\eta_{k0}(>0) and \widehat{K_{kj}(\cdot)} = \int_0^\infty e^{-\lambda a}\beta_{kj}(a) \pi_k(a)da.
Suppose that, for any \eta_{k0}>0, there exists an u_{0}\in \Omega_0 such that
and show a contradiction. Therefore, there exists a t_0\in\mathbb{R}_+ such that \rho_k(\mathcal U(t, u_0))\le \eta_{k0} for t\ge t_0 and all k\in\mathbb N. Without loss of generality, we shift the time to t_0 = 0. Then \lambda_k(t)\le \eta_{k0} for t\ge t_0 = 0 and k\in\mathbb N.
Next, we show that S_{k{\infty}} \ge S^0_k(\eta_{k0}): = \frac{b_k}{\mu_k+\eta_{k0}+p_k(1-K_k^1)}, where S_{k{\infty}} = \liminf\limits_{t\rightarrow\infty}S_k(t). By the Fluctuation Lemma [35], we can pick up a sequence \{t_n\} such that S_k(t_n)\to S_{k{\infty}}, \frac{dS_k(t_n)}{dt}\to 0 as n\to\infty. Then from the first equation of (5), it follows that
This, combined with (40), gives
Letting n\to\infty leads to
This implies that S_{k\infty}\ge S_k^0(\eta_{k0}).
Finally, since S_{k\infty}\ge S_k^0(\eta_{k0}), there exists a t_1\in\mathbb{R}_+ such that S_k(t)\ge \tilde S_k^0(\eta_{k0}) for t\ge t_1. Again, without loss of generality, we can assume t_1 = 0. Solving i_k(t, a) by the characteristic line method yields
where b_k(t) = S_k(t)\lambda_k(t). Then
Taking Laplace transforms on both sides of the above inequality gives
This inequality holds for any \xi and \eta_{k0}. If we take \xi and \eta_{k0} small enough, then this is a contradiction with (38). This completes the proof.
Clearly, for all k\in\mathbb N, \rho_k is a continuous function on \mathbb R_+. Proposition 5.1 implies that \{\mathcal U\}_{t\ge0} has a global attractor. From Theorem A.34 in [34], we need to show that for any bounded total orbit h(t+s) = \mathcal U(s, u(t)) of \mathcal U_t such that \rho_k(h(t))>0 for all t\in\mathbb R and s\in\mathbb R_+. For the total trajectory, we have
for t\in\mathbb R and a\in\mathbb R_+. In order to prove the strongly uniform persistence, the following lemma is helpful.
Lemma 6.1.
Let ({\bf S}(t), {\bf 0, i}(t, \cdot), {\bf0, v}(t, \cdot)) be a solution of (5). Then S_k^{\infty}\le S_k^0, where S_k^{\infty} = \limsup\limits_{t\rightarrow\infty}S_k(t) for k\in\mathbb N.
Proof. By Fluctuate Lemma in [35], there exists \{t_n\} such that t_n\to\infty, S_k(t_n)\to S_k^{\infty}, and \frac{dS_k(t_n)}{dt}\to 0 as n\to\infty. Integrating v_k equation in (5) along the characteristic equation t-a = \mathrm{const.}, we have
Substituting v_k into S_k equation yields
Applying Fluctuate Lemma, we immediately obtain
or S_k^{\infty}\le \frac{b_k}{\mu_k+p_k(1-K_k^1)} = S_k^0 as required. This completes the proof.
Lemma 6.2. Let us define \Theta(b(t)) = \sum\limits_{k = 1}^nb_k(t). Suppose that Assumption 6.1 holds, then \Theta(b(t)) for total trajectory h(t) is identically zero on \mathbb R, or it is strictly positive on \mathbb R.
Proof. Suppose that there exists a t_1>0 such that b_k(t) = 0 for all t\le t_1. By the definition of \lambda_k, we have
where \bar \beta = \max\limits_{j, k\in\mathbb N}\{\beta_{kj}^+\}. Summing k from 1 to n on both sides of (41) yields
for all t> t_1. It follows from Gronwall inequality that \Theta(b(t)) = 0 for all t\ge t_1.
Suppose there doesn't exist a t_1 such that \Theta(b(t)) = 0 for all t\le t_1. Thus, there exists a sequence \{t_m\} towards -\infty such that \Theta(b(t_m))>0 for each m. That means that b_{k}(t_m)>0 for each m. Moreover, there exists a sequence a_m such that i_k(t_m, a_m) = i_k(t_m-a_m, 0)\pi_k(a_m)>0 for each m. In view of the first equation, with the dissipative property of system (5), we obtain
Hence, there exists a positive constant \zeta>0 such that S_k(t)>\zeta>0 holds for all t\in\mathbb R. Let b_{km}(t) = b_k(t+t_m^*) for each n, where t_m^* = t_m-a_m. Recalling equation (41), we arrive at
where \varepsilon_0 is defined in (ⅲ) of Assumption 1.1 and
Consequently,
Since \tilde\Theta_m(0) = \sum\limits_{k = 1}^n\sum\limits_{j = 1}^n\int_0^\infty \beta_{kj}(a) i_{jm0}(a)da>0 and \tilde\Theta_m(t) is continuous at t = 0, it follows from Assumption [29] and Gronwall inequality that \Theta(b_m(t)) and \tilde\Theta_m(t) are positive for sufficiently small t. Furthermore, from Corollary B.6 [29], we conclude that there exists a constant l>0 such that \Theta(b_m(t))>0 for all t>l. Since \Theta(b_m(t)) is a time shift of \Theta(b(t)) by t_m^* with t_m^*\rightarrow-\infty as m\rightarrow\infty, it follows that \Theta(b(t))>0 for all t\in\mathbb R. This completes the proof.
Corollary 6.1.
Suppose that Assumption 6.1 holds. Then for all k\in\mathbb N, b_k(t) for the total trajectory h(t) is strictly positive for every t\in\mathbb R.
From Propositions 5.1 and 6.1, together with Corollary 6.1, we apply Theorem 5.2 in [29] to illustrate the \rho- strongly uniform persistence of system (5).
Lemma 6.3.
Suppose that \mathcal R_0>1 and the assumption of Corollary 6.1 hold. Then system (5) persists uniformly strongly, in this sense, there exists some \eta_{k0} such that
for some k\in\mathbb N and \lambda_k(0)\neq0.
Proof. From Corollary 6.1, we readily see that \inf \lambda_k(0)>0 for some k\in\mathbb N. Applying Theorem A.34 in [34], we conclude that there exists a constant \eta_{k0}>0 such that \liminf\limits_{t\rightarrow+\infty}\rho_k(\mathcal U(t, u_0))>\eta_{k0}.
By Lemma 6.3, we present the following theorem to state the uniformly strong persistence of system (5).
Theorem 6.4.
Suppose that \mathcal R_0>1 and Assumption 6.1 hold. There exist some positive constants \eta_{k0}>0(k\in\mathbb N) such that for all t\in\mathbb R and a\in\mathbb R_+
7.
Global attractivity of the equilibria
In this section, we will show the global behavior of the equilibria of system (5). To achieve this goal, we employ a Volterra type functional defined by g(x) = x-1-\ln x in [22], which is positive and attains minimum value 0 at x = 1. In what follows, we check this Volterra type functional is well-defined in infinite dimension and make the following assumption.
Assumption 7.1. For all j\in\mathbb N_+, S_{j0}\in \mathbb R_+, \displaystyle\int_0^\infty|\ln h_{j0}(a)|da < +\infty, h = i, v.
Lemma 7.1.
If Assumption 7.1 holds, then \displaystyle\int_0^\infty v_j^0(a)\ln\frac{v_j(t, a)}{v_j^0(a)}da is bounded.
Proof. For t>a,
For t\le a,
It follows from (44) - (45) that
Therefore, it follows from Assumption 7.1 that \displaystyle\int_0^\infty v_j^0(a)\ln\frac{v_j(t, a)}{v^0_j(a)}da is bounded.
Theorem 7.2.
Let Assumption 7.1 hold. If \mathcal R_0 = r(\mathcal K) < 1, the disease-free equilibrium E^0 is a global attractor in \Omega.
Proof. For j\in\mathbb N, define
where \alpha_{kj}(a) = \int_a^\infty\beta_{kj}(s)\frac{\pi_j(s)}{\pi_j(a)}ds and \delta_k(a) = \int_a^\infty\varepsilon_k(s)v^0_k(s)da. Lemma 7.1 ensures V_j(t) is well-defined. Then
From Lemma 7.1, together with 6.4, V_j(t) is well-defined. Deviating it along the solution of (5) yields
Note that \mathcal R_0 = r(\mathcal K) < 1 implies that \sum\limits_{k = 1}^nS_k^0K_{jk} < 1. Therefore, V'_j(t)\le0 and it is easy to see that the equality holds if and only if ({\bf S}(t), {\bf 0}, {\bf i}(t, \cdot), {\bf 0}, {\bf v}(t, \cdot)) = ({\bf S^0}, {\bf 0}, {\bf 0}, {\bf 0}, {\bf v^0}(\cdot)). This implies that the largest positive invariant subset of \{u(t)\in \Omega|V'_j(t) = 0\} is the singleton \{({\bf S}^0, {\bf 0}, {\bf 0}, {\bf 0}, {\bf v^0}(\cdot))\}. This shows that the disease-free equilibrium E_0 is a global attractor.
In the following, we give a lemma to show the boundedness of the Lyapunov functional for proving the global attractivity of the endemic equilibrium E^*.
Lemma 7.3. If Assumption 7.1 holds, then \displaystyle\int_0^\infty h^*_j(a)\ln\frac{h_j(t, a)}{h^*_j(a)}da (h = i, v) is bounded.
Proof. Prior to this proof, denote \beta_j^+ = \max\limits_{k\in\mathbb N}ess.\sup\limits_{a\in\mathbb R_+}\beta_{jk}(a) for all j\in\mathbb N. For t>a, it follows from b_j(t)\le\beta^+_j\frac{\Lambda_j^2}{\mu_j^2} that
For a>t,
Then,
By the assumption, it follows that \displaystyle\int_0^\infty i^*_j(a)\ln\frac{i_j(t, a)}{i^*_j(a)}da is bounded.
Similarly, \displaystyle\int_0^\infty v^*_j(a)\ln\frac{v_j(t, a)}{v^*_j(a)}da is also bounded.
Assume that f(t, a)\in\mathbb R\times L^1(\mathbb R_+) is a solution of the following system
where m(a), \eta(a)\in L^1(\mathbb R_+) and L(t)\in\mathbb R. Obviously, the nontrivial equilibrium E_1^* of system (52) satisfies the following equations:
Define a Lyapunov functional V_1(t) = \int_0^\infty M(a)g\left(\frac{f(t, a)}{f^*(a)}\right)da, where M(a) = \int_a^\infty\beta(s) f^*(s)ds.
Lemma 7.4.
Suppose that \displaystyle\int_0^\infty|\ln f_0(a)|da is bounded. There exists a positive value \eta_0>0 such that f(t, a)>\eta_0e^{-\int_0^am(s)ds}, then
where M(a) = \displaystyle\int_a^\infty\eta(s)f^*(s)da.
Proof. Note that
where we denote h'_l(s, l) = \frac{\partial h(s, l)}{\partial l}.
It follows from the proving process of Lemma 7.3, together the assumption of Lemma 7.4 that V_1(t) is well-defined. Deviating it along the solution of (52), we obtain
Observing (55), we have
With the help of integral by parts, we obtain
here we used the fact M(0) = \int_0^\infty\eta(a)f^*(a)da, and M'(a) = -\eta(a)f^*(a).
Next, we will give the global attractivity of the endemic equilibrium E^*.
Theorem 7.5. Suppose \mathcal R_0>1, (iv) of Assumption 1.1 and Assumption 7.1 hold. Then the endemic equilibrium E^* is a global attractor in \Omega_0.
Proof. Define
where \alpha_j(a) = \sum\limits_{k = 1}^n\int_a^\infty\beta_{jk}(s)i^*_k(s)da and \delta_j(a) = \int_a^\infty\varepsilon_j(s)v^*_j(s)ds. \kappa_j will be determined later and considered as a weighted coefficient. The well-definition of V(t) follows from Lemma 7.3. From the definitions of \alpha_j(a) and \delta_j(a), it follows that
and
Assisting with Lemma 7.4 and deviating V(t) along the solution of (5) yield
Note that
Equation (60) implies that
Noting that \int_0^\infty\epsilon_k(a)v_k^*(a)da = p_kS_k^*\int_0^\infty\epsilon_k(a)\pi_k^1(a)da = p_kS_k^*K_k^1, it follows from (60) and (61) that
where H_j(i) = \displaystyle\frac{i_j(t, a)}{i^*_j(a)}-\ln\frac{i_j(t, a)}{i^*_j(a)}. Define \Theta_{jk} = S_j^*\int_0^\infty\beta_{jk}(a)i_k^*(a)[H_k(i)-H_j(i)]da, (j, k = 1, 2, \cdots, n) and a Laplacian matrix
By Lemma 2.1 in [21], we have that the solution space of the linear system \Theta\kappa = 0 is 1 and one of its basis is given by
where c_{jj}>0 (j = 1, 2, \cdots, n) denotes the cofactor of the j-th diagonal element of matrix \Theta. This implies that \sum\limits_{k = 1}^n\theta_{jk}\kappa_j = \sum\limits_{j = 1}^n\theta_{kj}\kappa_k and
Employing the graph-theoretic approach mentioned in [21], (63) is equal to zero. Therefore, V'(t)\le0. The equality holds if and only if \frac{i_k(t, a)}{i_k^*(a)} = \frac{i_j(t, 0)}{i_j^*(0)} = \frac{i_j(t, a)}{i_j^*(a)}. This implies that \mathbf i = \mathbf ci^*. It follows from the first equation of (5) with respect to the monotonicity of \mathbf c that \mathbf c = \mathbf 1. Hence, the largest invariant set of \{u(t)\in \Omega_0| V'(t) = 0\} is the singleton E^*. Combining the relative compactness of the solution orbit (see Lemma 5.3) with the invariance principle (see [Theorem 4.2, [30]]), we see that the endemic equilibrium E^* is a global attractor in \Omega_0.
8.
Simulations
In this section, we perform some numerical experiments to illustrate our theoretical results. For the experimental operability, we set a_{max} = 10 instead of infinity. For simplicity, we assume system (5) describes some sexually transmission diseases, such as Zika, Ebola and genital warts etc, which consist of two groups - male group and female group. As some news reported in [33], human papillomaviruses (HPV) is an effective and safe vaccine to control some sexually transmitted diseases inducing by virus. In order to illustrate system (5), we firstly fix the demographic parameters as follows:
Hence the total male population maintains the size N_1(t) = 2050 and the size of the total female population is N_2(t) = 4050. It has been reported that nearly half of newly infections are diagnosed as females in age range between 15 to 24. Therefore, we assume that the vaccination rates p_1 = 0.21 for male and p_2 = 0.22 for female. Other parameters are associated with infection age in the form of
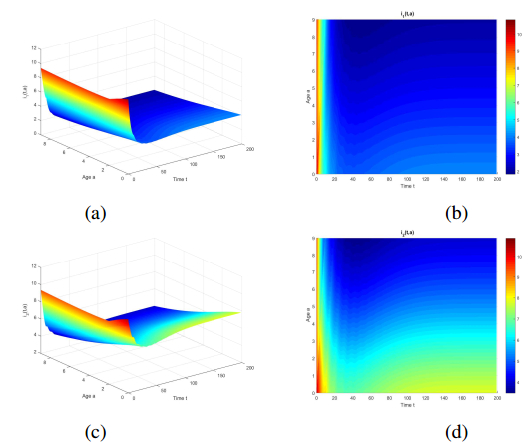
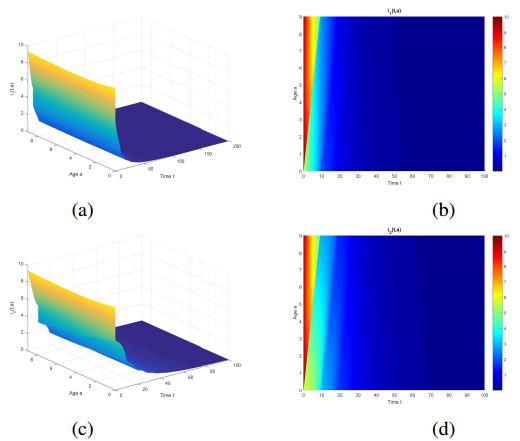
where \Gamma(n) = (n-1)!, or \Gamma(z) = \int_0^\infty x^{z-1}e^{-x}dx (z\in\mathbb N). Obviously, y(x, A, B) satisfies (ⅲ)' of Assumption 1.1. We fix \gamma(a) = y(a, 2, 5), \varepsilon(a) = y(a, 1, 1) and verify the transmission rate \beta(a) = y(a, 6, B). If we choose B = 9, this implies that the mean of the transmission function is 54 and the variance is 486. It follows from Figure 1 that the disease-free equilibrium E_0 is asymptotically stable. Then we decrease B = 8 associated with the variance changed as 384. Figure 2 shows that the endemic equilibrium E^* is asymptotically stable.
Comparing infected male and female populations in Figure 2, we find that the total infected number for female population is larger than that for male population. However, the first peak time for female population is later than the time for male population. Although the vaccination rate for female designed is higher than such rate for male, the level of infected peak and the total number for female population are still larger than those for male population. Consequently, the government should pay more attentions to female population.
9.
Discussion
In this paper, we proposed a multi-group SIVS epidemic model with age-since-infection. We calculated the basic reproduction number \mathcal R_0 by the renewal equation, which is the spectral radius of the next generation operator \mathcal K. From Theorems 7.2 and 7.5, we see that the global attractivity of system (5) is totally determined by \mathcal R_0. This implies that the basic reproduction number is a sharp threshold determining that the disease prevails or vanishes. Implicit Euler method performed illustrates the theoretical results. Numerical experiment shows that the number of the infected females is larger than the number of the infected male although the vaccination rate for female group is higher than that for male group. The government should pay much more concerns on female group for suppressing sexual diseases prevalence.
Irreducibility of the transmission matrix \beta_{jk}, j, k\in\mathbb N has been highly influential in analyzing the global stability of equilibria. This assumption is still a basic assumption of epidemics spreading on scale free networks. Therefore, we hope our analysis method proving theoretical results and numerical method can be generalized to investigate the dynamics of some epidemic models on complex networks [36]. Besides, oscillation is one of the important phenomena in diseases transmission. What mechanisms resulting in oscillation has been becoming an increasing trend in investigating epidemic models with age-since-infection [37]. We leave this for our future work.
Acknowledgements
The authors are very grateful to the Editor-in-Chief, Professor Yang Kuang, and the anonymous referees for their valuable comments and suggestions, which helped us to improve the presentation of this work significantly.
This work was supported by the National Natural Science Foundation of China (Nos. 61573016, 11871316), Shanxi Scholarship Council of China (2015-094), Shanxi Scientific Data Sharing Platform for Animal Diseases, and Startup foundation for High-level Personal of Shanxi University.
Conflict of interest
The authors declare there is no conflict of interest.









 DownLoad:
DownLoad: