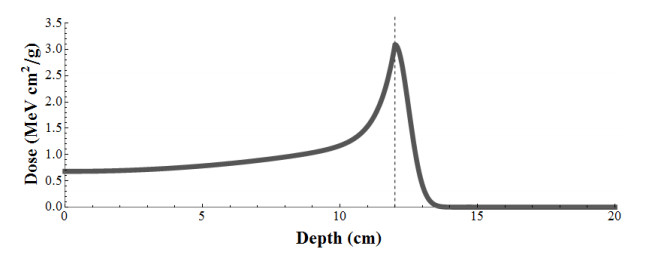
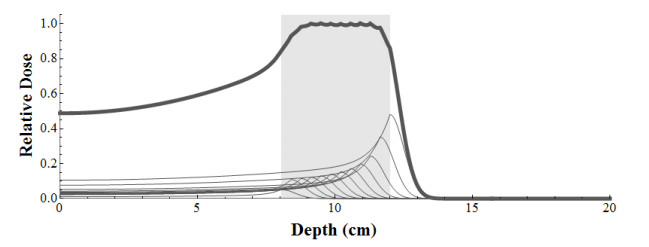
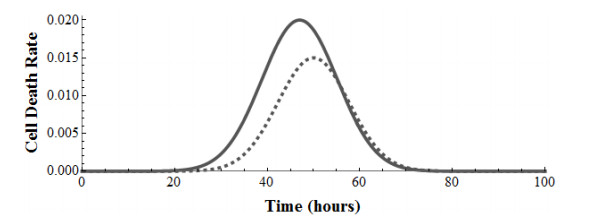
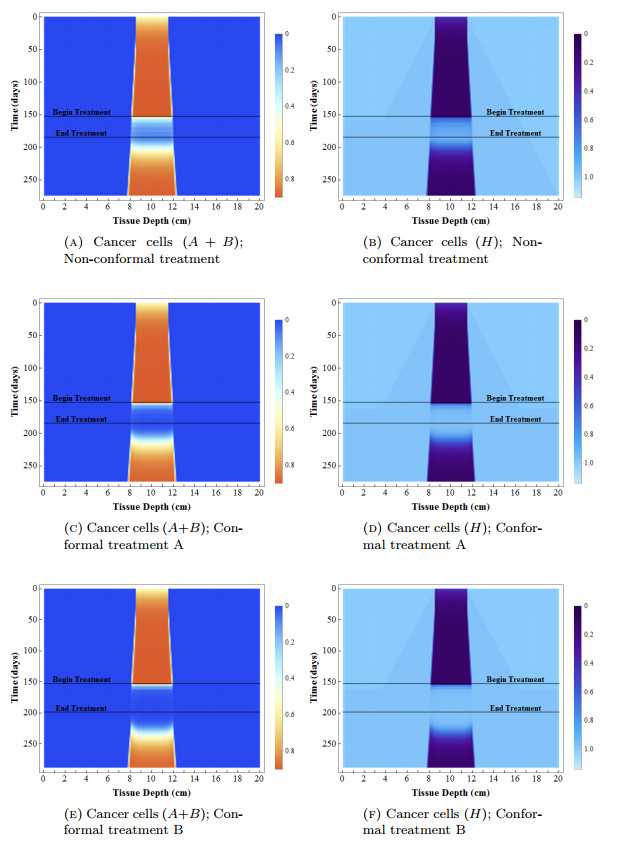
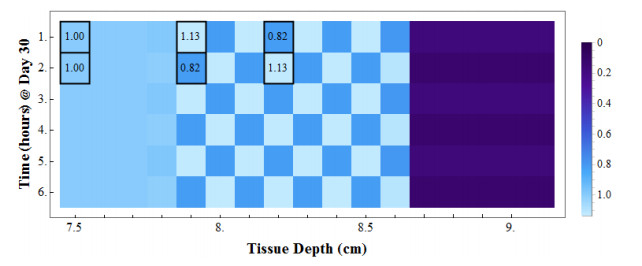
Proton therapy is a type of radiation therapy used to treat cancer. It provides more localized particle exposure than other types of radiotherapy (e.g., x-ray and electron) thus reducing damage to tissue surrounding a tumor and reducing unwanted side effects. We have developed a novel discrete difference equation model of the spatial and temporal dynamics of cancer and healthy cells before, during, and after the application of a proton therapy treatment course. Specifically, the model simulates the growth and diffusion of the cancer and healthy cells in and surrounding a tumor over one spatial dimension (tissue depth) and the treatment of the tumor with discrete bursts of proton radiation. We demonstrate how to use data from in vitro and clinical studies to parameterize the model. Specifically, we use data from studies of Hepatocellular carcinoma, a common form of liver cancer. Using the parameterized model we compare the ability of different clinically used treatment courses to control the tumor. Our results show that treatment courses which use conformal proton therapy (targeting the tumor from multiple angles) provides better control of the tumor while using lower treatment doses than a non-conformal treatment course, and thus should be recommend for use when feasible.
1.
Introduction
Ship dynamic positioning is a system used in ships and offshore vessels to automatically maintain the ship's positions and heading by thrusters and propulsion systems [1]. Eliminating the need for traditional anchoring, dynamic positioning ship (DPS) is equipped with a sophisticated system that allows it to stay in a specific location or follow a set track. And DPS has been used in various maritime applications, including offshore oil and gas exploration, subsea construction, cable laying, and so on. Recently, there is a wealth of research in the academic literature on the control for DPS ([2,3,4,5,6,7]), and the control algorithms and strategies include that proportional integral derivative (PID) control, adaptive control, intelligent control, etc. [2]. The research direction includes sensor fusion and position reference systems [3]; robust control [4]; fault tolerance control [5]; cooperative control and networked system [6]; simulation and validation [7] etc. For example, in [3], the technology of sensor fusion and the selection and optimization of position reference systems for DPS are discussed, which emphasizes the requirements of system accuracy and reliability. Reference [4] uses H∞ robust control techniques for DPS to obtain a robust controller with better performance than traditional dynamic positioning systems. Trajectory preservation is achieved through appropriate weighting functions. Reference [5] discusses the asymptotic stability conditions for DPS with partial actuator failure. The saturation proportional differential controller has been designed, and the asymptotic stability conditions are given by using the LKF approaches. In [6], a new event triggered collaborative DP control algorithm is proposed for multiple surface vessels. This algorithm adopts an undirected interactive topology to construct a communication network between surface vessels, so that all followers can obtain leader information. Reference [7] proposes a multi physics modeling method for a comprehensive electric propulsion system used for DPS, which can run in real-time and connect to control hardware.
In recent years, due to the advantages of discreteness, high efficiency, and low communication costs, the sampled-data control system has received widespread attention and it has been widely applicated in various systems, such as the hypersonic vehicle system [8,9], Lur'e system [10,11,12], neural networks system [13,14,15,16,17], Markovian jump system [18,19] and so on [20,21]. It can be applied to practical system such as fast train [22], unmanned aerial vehicle [23], large cruise liner [24,25], small unmanned ships [26], etc. Nowadays, the Takagi-Sugeno fuzzy system (TSFS) has received widespread attention due to their significant effectiveness in describing the complex nonlinear system, and considerable sampled-data control methods are reported for TSFS [27,28]. For example, in [29], the sampled-data control issue for TSFS with random time delay and actuator faults is studied using the input-delay method; In [30], the stability issue for nonlinear sampled-data TSFS is studied using the LKF method, which is validated by a single link robotic arm with an electric motor model. In [31], the stability characteristics of TSFS under sampling-data control are studied. The system is considered as a variable time delay system based on the input delay method. An appropriate LKF candidates and quadratic delay product terms are provided. Reference [32] studies the nonfragile sampling control problem for TSFS with parameter uncertainty. A new augmented LKF with sufficient sample data information has been constructed, which results in less conservative results.
Recently, the research on sampled-data control systems for DPS has attracted widespread attention. In [33], A semi-globally practically asymptotically stable controller is designed using the integration and backstepping technique of the Euler approximation models. In [34], based on the Euler approximation model and nonlinear sampling control theory, a state feedback controller and an output feedback controller based on a reduced order observer are designed for DPS to stabilize the tracking error. In [35], the sampling control system was applied to the dynamic positioning ship, and the input delay method was combined with the Wirtinger integral inequality to obtain results with less conservatism. In [36], the control problem of a sampling system for DPS with actuator failure is discussed, and a sampling controller is designed to ensure the exponential stability of the system and meet the H∞ performance index. In [37], the fuzzy robust stability criterion of sampled-control systems for DPS is studied, and a fuzzy sampling controller is designed using an input time delay method to ensure that the system remains stable under external disturbances. In [38], the reliable tracking control problem for DPS based on nonperiodic measurement information is studied and the mean square exponential stability criterion is obtained.
However, there is still much room for further study for the sampled-data DPS. Firstly, some important characteristics are still overlooked, which include the features of sampling pattern and the effective characteristics of the constructed LKF. Secondly, the positivity limit of the Lyapunov matrix is strict, which will result in the conservatism of the system.
Inspired by the above discussions, we aim to study the stability condition and controller design for sampled-data DPS with the T-S fuzzy model. In addition, we will construct an improved LKF by adding the new useful terms and introducing the reciprocally convex method. The main advantages of this article are be listed that:
(1) To capture the features of sampling pattern fully, some novel terms, like (tk+1−t)t∫tke2αs[x(s)x(tk)]T[R1R2∗R3][x(s)x(tk)]ds are introduced in the constructed LKF.
(2) The tighter technique of reciprocally convex combination is introduced during the differentiation of LKF. Hence, the conservatism of stability criteria can be greatly reduced.
(3) A second-order term (tk+1−t)(t−tk)[xT(tk)N1x(tk)] related to time t is added during the construction of V(t), thereby a less conservative result is obtained.
Notations: Rm denotes m-dimensional Euclidean space, and " T " represents the matrix transposition. "*" is a symmetric block matrix.
2.
Problem formulation
Consider the dynamic equations for DPS as follows:
where
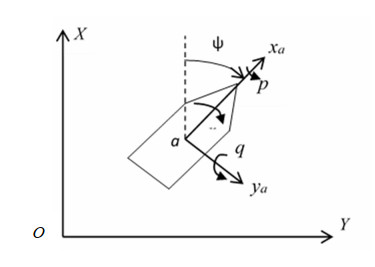
where η(t)=[xa(t)ya(t)ψ(t)]T is the positions and heading angle in the northeast coordinate system respectively. υ(t)=[p(t)v(t)r(t)]T represents the surge, sway, and yaw velocity vector in the attached coordinate system (see Figure 1). J(ψ) is the coordinate rotation matrix. u(t) is the control force vector; M and D denote the inertial matrix and damping matrix, respectively. G is the mooring force matrix, and w(t)=[w1(t),w2(t),w3(t)] is the environmental disturbance including wind, waves, and currents.
Remark 1. During the modelling of the ship, it can be considered that the interaction forces between the various points of action of the ship are ignored, that is, the ship is considered as a rigid body. Hence, the combination of forward, lateral, and turning motions with heave, roll, and pitch motions is neglected, and we only consider the three degrees of freedom plane motion of the DPS.
Define
Let
Substitute (2) and (3) into (1) such that
where
where a11,a22,a23,a32,a33,d11,d22,d23,d32,d33,b11,b22,b23,b32,b33 are constant.
The global fuzzy models can be described as follows:
Mode Rule i: IF z1(t) is fi1, ⋯zn(t) is fin, THEN
where z1(t),z2(t),…,zn(t) represent the premise variables, fij is the fuzzy set, and n is the rules number.
Assume the yaw angle satisfies ψ(t)=[−π/π22,π/π22], then the controller rules are considered such that:
Model Rule 1:
IF ψ(t)=0, THEN
Model Rule 2:
IF ψ(t)=π2, THEN
Model Rule 3:
IF ψ(t)=−π2, THEN
where
where α=sin(2∘), β=cos(88∘). The global fuzzy models are inferred such that:
where
The membership function of ψ(t) is shown in Figure 2.
Assuming that the states of the DPS are obtained at each sampling instant tk, the sampling time is assumed to be
The structure of the system is depicted in Figure 3.
The system stability process is shown in Figure 4. It can be seen that the DPS fuzzy controller calculates the ship's control force and moment based on the deviation between the desired and actual position and velocity of DPS. Then, the thrust allocation distributes control force and moment for DPS to generate the actual position and velocity under the environmental disturbances, which achieves the stability target of the system.
Let d represent the upper bound of the sampling interval, i.e.,
then we consider the following sampled-data controller
where K represents the controller matrix. Then the T-S fuzzy controller can be described that:
Controller Rule 1:
IF ψ(t)=0, THEN
Controller Rule 2:
IF ψ(t)=π2, THEN
Controller Rule 3:
IF ψ(t)=−π2, THEN
Hence, the overall fuzzy controller is inferred to be:
Substitute (14) into (11)
When the system has an uncertainty parameter, the system is converted into
where ΔA and ΔB are the uncertainty parameter matrix, and satisfy
D,Ea,Eb are known dimensional constant matrices, and F(t) is unknown time-varying matrices with Lebesgue measurable elements that satisfy
For the further deriving process, the two lemmas are given.
Lemma 1 [39] For any matrix H>0, and a vector with appropriate dimension μ(s), the inequality satisfies
where, matrix F and vector ξt are not dependent on integral variables and have any dimensions.
Lemma 2 [40] For any matrix J,R=RT>0, constant d1⩽d(t)⩽d2, and vector function ˙x:[d2,d1]→Rn, the following integral inequality holds:
where
Lemma 3 [41] Given matrices ΩT=Ω,Γ,X, for any F satisfying FTF⩽L, the inequality is established
and only if there is a scalar ε>0, then
3.
Main results
3.1. Stability analysis
In the section, a novel LKF is constructed, and the stabilization condition of system (15) is proposed. To begin, the notation is defined as follows:
Theorem 1: The system (15) with w(t)=0 is asymptotically stable, if there exist positive-definite matrices X=[XT1XT2XT3XT4XT5]T, P=[P1P2∗P3],Q,Z,U,S,R,N1,N2,M=[M11M12∗M22], and given scale d>0, such that
where
Proof. Consider the following LKF:
Take the derivative of V(t), which yields
By Lemma 1, it can be obtained that
Then
By Lemma 2, we have
where
then
For any matrixes G1,G2, we have
For any diagonal matrix D>0, it can be obtained that
Then, from (27) to (36), we have
where
Ψ1,Ψ2,Ψ3 is defined in Theorem 1.
By Lemma 2, we can derive a linear convex combination about τ(t), for all τ(t)∈[0,d], if
Then, Ψ(τ(t)) is negative definite.
Following the Schur complement, (24) and (25) imply (38) and (39), which means that ˙V(t)<0. This proof is completed.
Remark 1. In the constructed LKF, V1(t) and V2(t) are the general energy function of the system state, and it represents the energy changes of the system state and derivative from t−d to t. It is the foundation of the entire LKF. V5(t) symbolizes the relationship between state derivatives and time delay, and it is the foundation of time delay dependent stability standards. Additionally, V3(t) and V4(t) include the characteristic of time delay, and they play an important role in obtaining the final solution.
Remark 2. Compared with literature [42], two (tk,tk+1) dependent terms like (tk+1−t)t∫tke2αs[x(s)x(tk)]T[R1R2∗R3][x(s)x(tk)]ds and (tk+1−t)(t−tk)[xT(tk)N1x(tk)] are added in the LKF, which means that the available characteristic of sampling patterns are fully captured, and the LKF has a more general form.
Remark 3. In some previously published papers (such as [43,44]), they used overly relaxed delimitation techniques when estimating some integral terms such as −d∫tkt−d˙xT(s)Z˙x(s)ds. However, this paper directly uses a tighter technique by reciprocally convex combination in Lemma 2. Besides, free matrices G and G1 are proposed during the derivation procedure of LKF, which improves the design flexibility. Therefore, the conservatism of stability criteria can be greatly reduced.
3.2. H∞ performance analysis
To prove the robustness of the system, the following H∞ performance function is proposed
where w(t)≠0 and w(t)∈L2[0,∞), and y(t) denotes the system output, and satisfies that
Then, based on the Theorem 2, the system (15) can be proved to satisfy the robust performance with disturbances.
Theorem 2: the system (15) is asymptotically stable, if there exist positive-definite matrices X=[XT1XT2XT3XT4XT5]T, P=[P1P2∗P3],Q,Z,U,S,R,N1,N2,M=[M11M12∗M22], and given scale d>0, such that
where
Ξ12,Ξ13,Ξ14,Ξ15,Ξ22,Ξ23,Ξ33,Ξ34,Ξ35,Ξ44 are defined in Theorem 1.
Prove:
where
The proof is finished.
3.3. Mode uncertainty analysis
Next, Theorem 3 provides the stability conditions for systems with norm bounded uncertainty.
Theorem 3: the system (15) is asymptotically stable, if there exist positive-definite matrices X=[XT1XT2XT3XT4XT5]T, P=[P1P2∗P3],Q,Z,U,S,R,N1,N2,M=[M11M12∗M22], and given scale d>0,ε>0, such that
where
Ξ12,Ξ13,Ξ14,Ξ15,Ξ22,Ξ23,Ξ33,Ξ34,Ξ35,Ξ44 are defined in Theorem 1.
Prove: replace A and Ad in (25) with A+DF(t)Ea and B+DF(t)Eb, respectively. It can be obtained that:
where
According to Lemma 3, if there exist a scale ε>0, the following inequality holds
According to Schu's complement, Eq (48) is equivalent to Eq (50). The proof is completed.
3.4. Controller design
Then, the controller method can be obtained according to Theorem 4.
Theorem 4: for a given scale d>0, if there exist positive-definite matrices X=[XT1XT2XT3XT4XT5]T, P=[P1P2∗P3],Q,Z,U,S,R,N1,N2,M=[M11M12∗M22], such that
where
then the system (15) with w(t)=0 is asymptotically stable. Moreover, the controller gain is
Proof: Define
Let diag{ G,G,G,G,G,G}T and diag{ G,G,G,G,G,G}. By pre- and post-multiplying (24) respectively, (52) can be obtained. Let diag{ G,G,G,G,G,G,G}T and diag{ G,G,G,G,G,G,G}, pre- and post-multiplying (25) respectively, (53) can be obtained. This completes the proof.
Remark 4. The system (15) can obtain less conservativeness than that in [45], which is mainly due to two results. First, during the construction of V(t), a second-order term (tk+1−t)(t−tk)[xT(tk)N1x(tk)] related to time t is also added, which can reduce the conservatism of the results to some extent. Second, during the derivative of the LKF, the reciprocally convex combination method is used, which can obtain a more stringent derivative upper limit than Jensen's inequality, thereby less conservativeness will be achieved.
4.
Numerical examples
To demonstrate the merits of the method, a practical example is carried out. The M and D is considered as follows [46].
Let α=sin20 and β=cos880, then
The allowable maximum sampling interval is an important control performance index of the sampled-data system. A longer sampling period indicates a lower communication burden and fewer time consumption of the actuator, which helps to save energy, time and so on. Table 1 lists the results which uses different methods to obtain the maximum sampling internal. From Table 1, it can be seen that the sampling internal in Theorem1 improves [36,37,38] about 189.2%, 173.86%, and 35.9%, respectively, which means that the designed fuzzy controller is beneficial to obtain a larger maximum sampling interval.
Assume the initial value of the system that xs(t)=[15m15m0.2∘0m/s0m/s0∘/s].
Then, the gain can be computed such that
To demonstrate the control performance of the proposed method, Figures 5–10 present the comparison results with LKF in [36]. From Figures 5–10, it illustrates that the system state xs(t) reaches the expected values within approximately 10 seconds, while [36] achieves the desired target and requires a longer time. This indicates that the response speed in the proposed method is faster. Besides, the system's oscillation amplitude is smaller, which indicates that our result has good anti-interference ability under environment disturbance. In addition, Figures 5–10 further illustrate that the proposed method has more preferable control performance.
5.
Conclusions
The stabilization issue for fuzzy DPS with sampled-data is studied. To reduce the conservative and improve the sampling interval, a novel LKF is constructed by adding some integral terms, which can utilize the information of actual sampling modes fully. Then a fuzzy sampling control scheme is proposed. By introducing free matrices and reciprocally convex approach, the conservatism of the system is reduced. Simulation research demonstrates that the proposed method can achieve better control performance. However, the proposed methodologies didn't consider the lower bound of the sampling interval, and some matrix in the LKF are still required to be positive definite. Additionally, how to avoid the emergence of hierarchical phenomena in the system is an urgent problem to be solved. Furthermore, we will improve the LKF and discuss the aperiodic sampled-data control problem for DPS, which both considers the lower and upper bound of the sampling interval.
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Acknowledgments
This study was funded by National Natural Science Foundation of China (52371369); the Key Projects of National Key R & D Program (No.2021YFB390150), the National Science Project of Fujian Province (No. 2020J01659, 2022J01323, 2021J01822, 2020J01660, 20230019), the Fuzhou-Xiamen-Quanzhou In-dependent Innovation Region Cooperated Special Foundation (No: 3502ZCQXT2021007); Funds of Fujian Provincial for Promoting High Quality Development of Marine and Fisheries Industry (No. FJHYF-ZH-2023-10).
Conflict of interest
The authors declare there is no conflict of interest.









 DownLoad:
DownLoad: