Adaptive sliding mode fault-tolerant attitude control for flexible satellites based on T-S fuzzy disturbance modeling

1.   Introduction

The rapid advancement of space technology and the growing demand for aerospace applications, a new generation of satellites, space stations, manned spacecrafts and space probes generally have large flexible accessories such as solar panels, mechanical arms and antennas ^[1,2]. Nowadays, one of the most cutting-edge science and technology in the world is aerospace technology. With the complexity and diversity of space missions, the attitude control of flexible satellites is required to be more accurate. Therefore, there is no doubt that ACS is playing an important role in various satellite subsystems ^[3]. High-precision attitude control algorithms often determine the success or failure of scientific tasks. Robust control ^[4], PD (proportional-derivative) control ^[5], sliding mode control (SMC) technology ^[6] and ACS ^[7,8] have been commonly used in the ACS of flexible satellites.

In addition, exogenous disturbances widely exist in robotic manipulators ^[9], aerospace systems and motion control systems ^[10] and so on. In order to protect the system performance from external disturbances, many scholars have adopted different methods to solve the anti-disturbance control problems in ACS ^[11,12]. For the framework of DOBC, an observer is designed to estimate some unknown disturbances and then equivalently compensate the disturbances in the controller ^[13,14]. However, the existing DOBC method cannot accurately describe nonlinear or irregular disturbances. Therefore, it is particularly important to study a new disturbance modeling method. Reference ^[15] has a very interesting research work, an event-triggered anti-disturbance attitude control approach is proposed for the ACS with multiple disturbances. Meanwhile, a disturbance observer is designed to estimate uncertain modeling disturbance and norm-bounded equivalent disturbance, and its effectiveness is verified by simulation. In ^[16,17,18], the TSFDO was used to describe some irregular and nonlinear disturbances. Compared with the traditional disturbance observer approaches, T-S fuzzy disturbance observers can effectively estimate nonlinear disturbances.

In the past decades, fault-tolerant control (FTC) has become a research hotspot in the field of aerospace, and it is also an effective way to improve the reliability of satellite ACS ^[19]. Satellites that have been in orbit for a long time are likely to fail ^[20,21,22]. In ^[23], an adaptive variable structural FTC scheme is introduced to address sensors and thrusters faults in spacecraft formations. In ^[24], the synthesis of reliable state feedback control for T-S fuzzy systems with sensor multiplicative faults is studied. Different from the existing research on sensor failure, the influence of sensor failure on the premise variables is taken into account. In ^[25], an adaptive FTC approach based on disturbance observer is presented for ACS with actuator faults, elastic modal and environmental disturbance torque. In order to compensate for external disturbances, uncertainties and time-varying faults, a time-converged robust sliding mode fault-tolerant controller (RSMFTC) is designed. Unlike general fault diagnosis observers (FDO), the fault-tolerant controller did not require fault detection ^[26]. In ^[27], a fault diagnosis observer and a disturbance observer are proposed to observe the actuator failure and system disturbances. Furthermore, an adaptive FTC method is proposed to improve the stability and reliability of rigid satellites. In ^[28], based on a robust fault tolerant controller, system output can track the desired signals for faulty nonlinear systems subject to parameter uncertainties and no full-state measurements. An adaptive sliding mode fault-tolerant control scheme is proposed for flexible satellites with saturation and partial failure of the actuator in ^[29], where an estimator and the neural network are applied to estimate fault and the nonlinear dynamic, respectively.

This paper addresses an ASMFTC approach for for flexible satellite ACS subject to the loss of actuator effectiveness and multiple disturbances. Compared to previous DOBC results ^[1,30], the disturbance is not restricted to be a constant or represented by a linear exo-system. In addition, compared with references ^[1,27], the multi-source disturbance caused by flexible accessories is taken into account. Firstly, the T-S fuzzy model (TSFM) is applied to describe the irregular and nonlinear disturbances caused by the elastic modal, and a fuzzy disturbance observer (FDO) is further designed to estimate the elastic modal. Then, based on the TSFDO, an ASMFTC method is investigated for ACS of flexible satellites. Finally, the simulation results show that the introduction of the disturbance observer and the proposed ASMFTC method can guarantee that the system reaches a steady state quickly and show the efficiency.

The main chapters in this paper are arranged as follows. In Section 2, the structural diagram of flexible satellite is introduced, and the mathematical model with multi-source disturbances and partial actuator failure is given. Meanwhile, the T-S fuzzy disturbance modeling is discussed and designed. In Section 3, a TSFDO is designed to estimate the disturbance which generated by the elastic modal. Then, an ASMFTC is constructed for a flexible satellite. In Section 4, the effectiveness of the control method is verified by comparing simulation examples. Last but not the least, the concluding comments in this paper can be found in Section 5.

2.   Mathematical model and and problem description

2.1.   Mathematical model

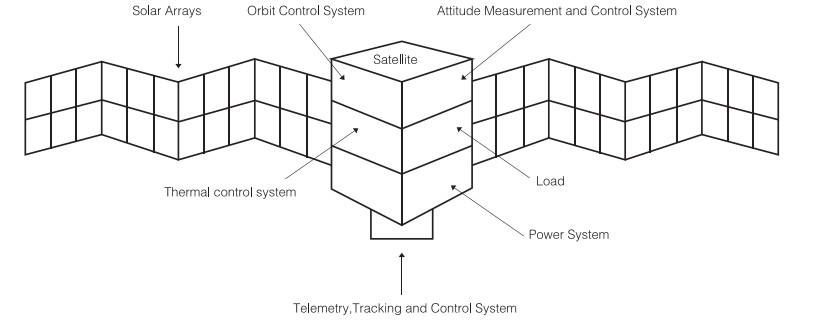
Flexible satellite system is more complex, usually composed of satellite body, flexible accessories, attitude control system, electronic power system, power system, etc. Among them, attitude control system is the most important subsystem (see Figure 1). The mathematical model of a flexible satellite includes a flexible appendage and a rigid body structure ^[25,31]. The Lagrange method is used to describe the attitude dynamics equation as

where $J$ is three-axis inertia moment. Variable $\theta$ represents three attitude angles. $T_z(t) = T_c(t)+T_d(t)$ represent command control torques, including external disturbance torque $T_d(t)$ and control torque $T_c(t)$. $H$ is the rigid-elastic coupling matrix and $\eta$ is the flexible modal coordinate. $E = diag\left\{{n_i^2, i = 1, 2, ..., n}\right\}$ represents the stiffness matrix, where ${n_i}\left({i = 1, 2, .....n}\right)$ represent the modal frequency. $C = diag\left\{ {2{\varepsilon _i}{n_{i}}, i = 1, 2, ......n} \right\}$ is the damping matrix, where ${\varepsilon _i}\left({i = 1, 2, ..., n} \right)$ is the damping ratio. The attitude dynamics equation (2.1) can be rewritten as the following matrix form by modal truncation of flexible structure.

The disturbance $d_0(t)$ generated by the flexible appendages can be written as

Define $x(t) = \left[\begin{array}{cc}\theta^T(t) & \dot \theta^T(t)\end{array}\right]^T$. Taking into account the multiple disturbances and partial actuator failure in the flexible satellite, Eq (2.2) can also be rewritten as

where $x(t)$ is the state variable of the system, $u(t)$ is the system control input and

$I$ is a unit matrix with suitable dimensions. $d_0(t)$ is the modelable disturbance generated by flexible appendages. $d_1(t)$ is an equivalent energy bounded disturbance, which satisfies $\left|d_1(t) \right|\leq{\overline d _1}$. Its upper bound $\overline d _1$ is positive and unknown. $\rho (t)$ represents the actuator failure factor and $\rho(t) = diag\left\{{\begin{array}{*{20}{c}} \rho _1(t) & \rho _2(t) & \rho _3(t)\end{array}} \right\}$, $0 < {\rho _i} \le 1$, $i = 1, 2, 3$. It is worth noting that $\rho _i(t){\rm{ = }}1$ means the $i-th$ actuator is healthy, and $0 < \rho _i(t) < 1$ means that the $i-th$ actuator is working but partially fails. Therefore, only partial failure and complete health of the actuator are considered here, Eq (2.4) is rewritten as follows by a simple transformation.

where $\Delta \rho(t) = I-\rho(t)$. Here it can be assumed that $\rho _0 = \mathop {\min}\limits_{i = 1, 2, 3}\rho _i(t)$, then it can be concluded that $\sigma = \left\|\Delta \rho(t)\right\| = 1-\rho _0$. Define $e_\theta(t) = \theta _d(t)-\theta(t)$, where $\theta_d(t)$ represents the desired attitude and $\theta(t)$ is the actual attitude. For clarity, $e_\theta(t)$ is marked as $e_\theta$.

2.2.   T-S Disturbance model

TSFDO is described by a set of fuzzy If-Then rules, and the nonlinear and irregular disturbance $d_0(t)$ cannot be described by a linear external system ^[1,27]. Since T-S fuzzy model has strong approximation ability, a TSFDO with $r$ rule will be applied to approximate nonlinear interference $d_0(t)$.

Rule $j$: If ${\vartheta _1}$ is $\mu_{1j}$, $\vartheta_2$ is $\mu_{2j}$, $\cdots$ and ${\vartheta _n}$ is $\mu_{nj}$, then

where $V_j$ and $W_j$ are two known parameter matrices. $\vartheta_i$ and $\mu_{ij}$ ($i = 1, \cdots, n$; $j = 1, \cdots, r$) represent the premise variables and the fuzzy sets. $r$ is the number of If-Then rules and $n$ is the number of premise variables. The whole TSFM is obtained based on fuzzy blending.

where $\vartheta = [\vartheta_1, \ldots, \vartheta_n]$, ${h_j}\left(\vartheta \right) = \frac{{{\Upsilon _j}\left(\vartheta \right)}}{{\sum\limits_{j = 1}^r {{\Upsilon _j}\left(\vartheta \right)} }}$, ${\Upsilon _j}\left(\vartheta \right) = \prod\limits_{i = 1}^n {{\mu _{ij}}\left({{\vartheta _j}} \right)}$, and $\mu_{ij}(\cdot)$ is the grade of the membership function of $\mu_{ij}$. Here it can be supposed that

for any $\vartheta$. Therefore, $h_j(\vartheta)$ satisfies the following formula

Remark 1: It is noted that the existing DOBC approaches can observe some unknown constant and harmonic signals ^[32,33]. However, in practical control systems, the disturbance is usually irregular or nonlinear. Therefore, the traditional DOBC method is no longer applicable. The main task of anti-disturbance control is to compensate or weaken disturbance, just as T-S fuzzy observer has the ability to estimate nonlinear or irregular disturbances ^[34,35]. In this paper, the TSFDM is applied to describe the elastic modal with high precision.

2.3.   Control objectives

In this paper, an ASMFTC method based on TSFDO is proposed to deal with the fault-tolerant problem of flexible satellites under actuator failure and multiple disturbances. By designing Lyapunov function and selecting appropriate control parameters, the sliding surface can be guaranteed to approach zero. The effectiveness of the proposed ASMFTC can be further proved by simulation verification. The diagram of the ACS for the flexible satellite as shown in Figure 2.

3.   Anti-disturbance fault tolerant controller design

3.1.   Design of T-S fuzzy disturbance observer

It can be obtained from Eq (2.7) and Remark 1, regular disturbance $d_0(t)$ can be easily estimated by constructing T-S fuzzy disturbance observer

where $\hat d_0(t)$ is the estimation of $d_0(t)$. Matrix $L_1$ is the unknown T-S fuzzy disturbance observer gain to be designed later. $\varepsilon(t)$ is the auxiliary function of design. The disturbance estimation error $e_w(t)$ is defined as

From Eqs (2.4), (2.7) and (3.1), and computing the time derivative along the trajectory of Eq (2.7), the disturbance estimation error equation satisfies

Theorem 1: If there exist matrices $P_1 > 0$, $R$ satisfying

$j = 1, \cdots, r$, then with the disturbance observer gain matrix $L_1 = RP_1^{-1}$, the estimation error system Eq (3.3) is stable and ultimately uniformly bounded (UUB), where

Proof: Consider the following Lyapunov candidate function as

Computing the time derivative along the trajectory of Eq (3.3), it can be shown that

where

Next by using Schur's complement formula that if $\Omega_j < 0$, $\Psi_a > 0$ and $\Gamma < 0$, the estimation error system $e_w(t)$ is stable and ultimately uniformly bounded.

3.2.   Design of non-singular terminal sliding mode controller

In this paper, the TSFDO is used to observe the disturbance generated by elastic modal, and the variable structure method is used to design the sliding mode controller. Finally, the modeled disturbances are compensated in the designed controller to ensure reliable operation of the system.

Remark 2: In linear sliding mode control, the sliding mode variable is a linear function of the system state, so the linear sliding mode control method can only make the system state converge asymptotically. In order to realize the finite time convergence of the system state, this paper proposes a terminal sliding mode control method. In terminal sliding mode control, the sliding mode variable is a nonlinear function of the system state.

Select the terminal sliding surface as follows

where $s(t) = \left[\begin{array}{ccc} s_1&s_2&s_3 \end{array}\right]^T$ is the sliding mode function, $N \in {R^{3 \times 3}}$ is a reversible positive constant diagonal matrix. $k_1$ and $k_2$ are odd and positive numbers, and satisfy $1 < {k_1}/{k_2} < 2$.

Remark 3: It can be obtained from the above proof that the disturbance estimation error $e_w(t)$ is stable and bounded. From the definition $m(t) = \bar d_1(t)+V_je_w(t)$, $m(t)$ is a continuous function and it has an upper bound $f$.

Define $\lambda = 1/(1-\sigma)$, where $\sigma = \left\|\Delta \rho(t)\right\|$. From the fuzzy disturbance observer equation (3.1), a compound controller based on terminal sliding mode can be designed as follows

where

$\hat d_0$ is the estimation of $d_0$, $\hat f$ is the estimation of $f$. $\alpha$ and $\beta$ are two positive adaptive parameters, and

where $I_0$ is a positive constant.

The derivative of the sliding surface $s(t)$ can be written as

Proof: Select the Lyapunov function as:

where $\tilde f = f-\hat f$, $\tilde \lambda = \lambda-\hat \lambda$.

The derivative of $V_2(t)$ is calculated as:

It is not difficult to seen that the sliding surface $s(t)$ is uniformly bounded and $\mathop {\lim }\limits_{t \to \infty } {V_2}\left(t \right) = {V_2}\left(\infty \right)$ holds. According to the Barbalat's lemma, when $t \to \infty$, then have $\left\| {s\left(t \right)} \right\| \to 0$. Furthermore, $s\left(t \right) \to 0$. This completes the proof.

Remark 4: For general terminal sliding control, the sliding surface can be selected as follows:

where $s_1(t)$ is the sliding mode function, $N \in {R^{3 \times 3}}$ is a positive constant diagonal matrix. $k_1$ and $k_2$ are positive odd numbers and satisfy ${k_1} > {k_2}$. There are some non-linear terms when deriving the sliding surface, such as $\frac{{{k_2}}}{{{k_1}}}Ne_\theta ^{{k_2}/{k_1} - 1}{\dot e_\theta }$. A non-singular terminal sliding mode method is utilized to avoid this similar situation in this paper.

Remark 5: In practical engineering applications, adaptive control methods usually add negative feedback links to ensure reliable and stable closed-loop systems.

where ${\gamma _1}$ and ${\gamma _2}$ are two small positive numbers.

Remark 6: In fact, the chattering problem in a sliding mode variable structure control system must exist, and the elimination of the chattering eliminates the anti-perturbation and anti-disturbance of the variable structure control. In order to remove this undesirable phenomenon in sliding mode control, people usually make corrections to minimize or eliminate chattering. The commonly used methods to eliminate tremor include quasi-sliding mode method, dynamic sliding mode method and high-order sliding mode method ^[36,37].

Therefore, it is impossible to completely eliminate the chattering, and it can only weaken it to a certain extent to some extent. The nonlinear function in Eq (3.10) can be approximated by the nonlinear function $s/(\left\| s \right\|+\gamma _3)$ in practical engineering applications. Therefore, Eq (3.10) can be further rewritten as:

4.   Simulation examples

Numerical simulations are presented in this section to verify the effectiveness of the proposed ASMFTC control method. The controller is used in ACS of a flexible satellite. Assume that the flexible satellite operates at a height of 900 km ^[25,27,31]. The orbit angular rate $n_0 = 0.0011\; rad/s$. The moments of inertia are

Including sunlight pressure moments, combined with the actual engineering situation, the space environment disturbance torque can be as follows

Take the pitch angle of the flexible satellite as an example of numerical simulation. Since the low-frequency vibration mode accounts for the main part of the vibration energy of the accessory. Therefore, this paper only considers the effects of disturbances caused by low-order modes. The first-order and second-order elastic modes are considered in this paper. Considering in a practical flexible satellite system, the two elastic modes are $n_1 = 3.17$ and $n_2 = 7.38$. The corresponding damping are ${\varepsilon _1} = 0.001$ and ${\varepsilon _2} = 0.0016$. The coupling matrix is selected as $H(t) = \left[\begin{array}{cc} 1.27806 & 0.91758\end{array} \right]$. Selecting the following Gaussian function as the membership function of the T-S fuzzy system:

The nonlinear disturbance in Eq (2.3) can be described as:

where $\sigma_{1}^{2} = 0.5$, $\sigma_{2}^{2} = 1$.

Selecting $N = 5$, $k_1 = 5$, $k_2 = 3$, $I_0 = 0.5$, $\alpha = \beta = 1$, $\gamma_1 = \gamma_2 = \gamma_3 = 1$. From Theorem 1, the gain of the TSFDO is

Since the vibration generated by the flexible appendages mainly comes from the low-frequency vibration mode, the first-order and second-order elastic modes of the flexible appendages are shown in Figures 3 and 4, respectively. From the definition of $d_0(t)$ in Eq (2.3), the responses of the disturbance $d_0(t)$ and its estimation are demonstrated in Figure 5. From Figure 6, it shows the estimation error of the disturbance $d_0(t)$. From Figures 5 and 6, it can be seen that the T-S fuzzy disturbance observer proposed in this paper can effectively estimate the nonlinear disturbance generated by the flexible appendages.

From Figure 7, it demonstrates the control torque under Eq (3.10). It can be seen from Figure 7 that the control torque remains stable after the system fails. However, the control input of the system still has chattering and the chattering is still very large. Therefore, the quasi-sliding mode method is used to reduce chattering as shown in Figure 8. As can be seen from Figure 8, the control input chattering of the system is greatly reduced under the control method of Eq (3.21).

In Figures 9 and 10, the loss of actuator effectiveness is assumed to occur at 20th second with failure factors 0.4, 0.8 and 1.0, respectively. From Figures 9 and 10, it can show the responses of the pitch angle and pitch angular rate under different failure factors. The solid line represents the curve of failure factor 0.4, dash line is the curve of failure factor 0.8, and dot line is curve of failure factor 1.0. From Figures 9 and 10, it can be seen that the proposed ASMFTC control method has has an anti-disturbance and fault accommodation ability. The curve of failure factor 1.0 can still maintain high accuracy after 20th second, meanwhile the curves of failure factors 0.8 and 0.4 are not as good as the counterpart of 1.0. Among these, the control accuracy of failure factor 0.4 is the worst. In other words, the smaller the failure factor, the worse the stability and fault accommodation accuracy of the system. It can be seen that the the responses of pitch angle and pitch angle rate under the two methods in Figures 11 and 12, with the proposed control method (ASMFTC), both pitch angle and pitch angular rate can be stabilized quickly, and the anti-disturbance of the system performance is better.

5.   Conclusions

In this paper, the problem of anti-disturbance control for a flexible satellite subject to actuator partial failure and multiple disturbances have been investigated. An ASMFTC approach based on TSFDO is presented for ACS with actuator partial fault, environmental disturbance torque and elastic modal. Firstly, the TSFM technology is applied to describe elastic modal, where the modeled disturbance is not restricted to be a linear exogenous system. Secondly, a flexible satellite rather than a rigid one subject to multiple disturbances is considered. Thirdly, the composite fault tolerant controller consists of TSFDO and an ASMFTC. Numerical simulations show that the designed controller can effectively improve the attitude control performance of flexible satellites, and have certain engineering practical value.

Acknowledgments

Jiangsu University "Blue Project" Outstanding Young Key Teachers Fund.

Conflict of interest

The authors declare there is no conflict of interest.