Separated boundary value problems via quantum Hilfer and Caputo operators

1.   Introduction

The concepts of fractional calculus have gained widespread recognition as an important area within pure mathematics. The concepts within fractional calculus have been studied by numerous mathematicians, given their significance across various fields of knowledge, see ^[1,2,3,4]. Theoretical aspects of fractional differential equations have attracted considerable interest since the advent of fractional calculus. Owing to the nonlocal nature of fractional-order differential equations, researchers possess the flexibility to choose and apply the most suitable operator, thereby accurately representing complex real-world phenomena. However, there are numerous versions of integrals and derivatives of arbitrary fractional order, employing various types of operators, as detailed in^{[5,6,7,8,9,10,11]}.

Fixed-point theorems serve as valuable tools for establishing both the existence and uniqueness of solutions to fractional differential equations. The methodology has been extensively studied by numerous mathematicians, and vast results have been published, see ^{[12,13,14,15,16,17,18]} and references therein. Furthermore, in the physical and life sciences, fractional calculus has widespread applicability across various domains, including image processing, complex systems, traffic flow, etc. Numerous researchers have studied many different kinds of initial and boundary value problems with various types of fractional operators, using techniques based on fixed-point theorems, ^{[19,20,21,22,23,24,25,26]}.

The concept of sequential fractional derivatives was first introduced in ^[27]. Since its inception, numerous researchers have made significant contributions to the advancement of this field. As a result, several research articles have been published. For more research insight, we refer to ^{[28,29,30,31,32,33,34]}.

Wang et al. ^[35], studied the turbulent flow model within the framework of the Caputo-Hadamard fractional derivatives of the form:

In ^[36], the authors discussed the existence and uniqueness of positive solutions for a class of Caputo fractional differential equations described by:

In 2018, Tariboon et al. ^[37] considered the sequential Caputo and Hadamard fractional nonseparated boundary value problem given by:

The investigation employed Banach and Krasnoselskii's fixed-point theorems, alongside Leray-Schauder's nonlinear alternative, to establish the existence and uniqueness of the results.

Asawasamrit et al. ^[38] studied the nonlocal boundary value problem involving the Hilfer fractional derivative of the form:

Jackson ^[39,40] initiated the idea of $q$-difference calculus. For more basic concepts of $q$-difference calculus, see ^[41,42]. Since then, numerous researchers have delved into the theoretical analysis of $q$-fractional-order differential equations, see ^{[43,44,45,46,47,48]}.

Allouch et al. ^[49] studied the $q$-difference equation with nonlinear integral boundary conditions of the form:

Measures of noncompactness and Mönch's fixed point theorems were utilized to derive the results.

Inspired by recent publications, we propose a new type of separated boundary value problems and investigate its theoretical analysis. The problem under consideration takes the form of

where ${{^{C}}\mathcal{D}}^{\delta}_q(\cdot)$ and ${^{H}}\mathcal{D}^{\alpha, \beta}_{q}(\cdot),$ respectively, are the Caputo and Hilfer fractional derivatives of orders $\delta, \; \alpha$, and type $\beta$ such that $\gamma = \alpha+\beta(1-\alpha)$ with $\gamma+\delta > 1,$ and $f:[0, T]\times\mathbb{R}\rightarrow \mathbb{R}$ is a continuous function.

The existence and uniqueness of solutions to $q$ sequential fractional-order boundary value problems have not been extensively studied. In our study, we introduce a new class of sequential $q$-Hilfer and $q$-Caputo fractional differential equations with separated boundary conditions and provide a comprehensive theoretical analysis.

The novelty of our study lies in the fact that we consider a sequential fractional boundary value problem combining $q$-Hilfer and $q$-Caputo fractional derivative operators subjected to non-separated boundary conditions. To the best of our knowledge, this is the first paper to appear in the literature. The method used is standard, but its configuration in the Hilfer-Caputo sequential boundary value problem (1.6) is new. The results are new and significantly enrich the existing results in the literature on Hilfer boundary value problems.

The rest of the paper is organized as follows: Section 2 revisits essential definitions, lemmas, and theorems. Section 3 focuses on establishing an integral equivalent form of the proposed problem, which enables us to prove the existence and uniqueness of results. In Section 4, two examples are presented. Section 5 provides the conclusion of the paper.

2.   Preliminaries

The section includes prerequisite facts, definitions, and key lemmas that will assists in proving the main results. The space $\mathcal{X} = \mathcal{C}([0, T], \mathbb{R})$ constitutes a Banach space comprising all continuous functions over $[0, T]$ with

Recall that for $q\in(0, 1)$ and $g, h\in\mathbb{R},$ the following properties holds ^[42]:

and

Moreover, for $\alpha\in\mathbb{R}$, we have

The $q$ analog gamma function is given by

such that $\Gamma_{q}(\alpha+1) = [\alpha]_{q}\Gamma_{q}(\alpha).$

For $u: [0, T]\rightarrow \mathbb{R}$ and $0 < q < 1,$ the $q$-derivative of $u$ is defined by

Moreover, the higher-order $q$-derivative shows

For setting $J_{t} = \{tq^{n}: n\in\mathbb{N}, \; t\ge0\}\cup\{0\}$, the analog $q$-integral of a function $u: J_{t}\rightarrow \mathbb{R}$ is of the form:

provided that the right-hand side converges. Note that $\mathcal{D}_{q}\left(\mathcal{I}_{q}u\right)(t) = u(t),$ and if $u$ is continuous at $0,$ then

Definition 2.1. ^[45] Let $w : [0, T]\rightarrow \mathbb{R}, \; \vartheta\in[0, T],$ and $\alpha > 0.$ The integral operator

is called the $q$-fractional-order integral in the Riemann-Liouville sense of order $\alpha > 0$ for the function $w,$ and $\mathcal{I}^{0}_qw(\vartheta) = w(\vartheta).$

Lemma 2.1. ^[45] For $0\le\alpha < \infty$ and $\sigma\in(-1, +\infty).$ If $w(\vartheta) = (\vartheta-a)^{(\sigma)},$ then

also

Definition 2.2. ^[50] Let $w : [0, T]\rightarrow \mathbb{R}, \; \vartheta\in[0, T], \; 0 < \alpha < 1.$ The derivative operator

is called the $q$-fractional-order derivative in Riemann-Liouville sense of order $\alpha$ for the function $w.$

Definition 2.3. ^[50] Let $w\in\mathcal{C}^{1}_{q}([0, T], \mathbb{R}), \; \vartheta\in[0, T], \; 0 < \alpha < 1.$ The derivative operator

is called the $q$-fractional-order derivative in the Caputo sense of order $\alpha.$

Lemma 2.2. ^[50] Let $w:[0, T]\rightarrow \mathbb{R}$ and $\alpha, \sigma\ge 0.$ Thus

Definition 2.4. ^[51] Let $w\in\mathcal{C}^{1}_{q}([0, T] \mathbb{R})$ and $0 < \alpha < 1, \; 0\leq \beta\leq1.$ The operator

is called $q$-Hilfer fractional derivative of order $\alpha$ with a parameter $\beta.$ Note that ${^{H}}\mathcal{D}_{q}^{\alpha, \beta}$ can be written as

Lemma 2.3. ^[50] Suppose that $0 < \alpha < 1.$ Then we have

and moreover,

Remark 2.1. Note that if $\beta = 0,$ from problem (1.6), we have

and if $\beta = 1$, we have

which are the $q$ sequential Riemann-Liouville and Caputo derivatives with separated boundary conditions.

3.   Main results

In this part, we begin by employing techniques from Lemma 2.3 to establish an integral equation associated with problem (1.6). To this end, we introduce the lemma, addressing a linear variant of problem (1.6), which serves as the fundamental tool for transforming the problem into a fixed-point problem.

Lemma 3.1. Let $0 < \alpha, \delta < 1$, $0\le\beta\leq1$, $\gamma = \alpha+\beta(1-\alpha)$, $\lambda_{1}, \lambda_{2}\in \mathbb{R}$ and $0 < q < 1$ and

Suppose $g\in C([0, T], \mathbb{R}).$ If $z\in\mathit{C}_q^2([0, T], \mathbb{R}),$ then, the linear problem

is equivalent to the integral equation:

Proof. Suppose $z\in\mathit{C}_q^2([0, T], \mathbb{R})$ satisfies problem (3.1), then, we show that $z$ is satisfies the integral (3.2). Since

then, make use of Eq (3.3), the first equation of (3.1) can be simplified as

Taking $\mathcal{I}_{q}^{\alpha}$ to both sides of Eq (3.4) and utilize the techniques in Lemma 2.3, yields

Also, taking $\mathcal{I}^{\delta}_q$ to both sides of Eq (3.5) and utilize the techniques in Lemma 2.3, we get

where $B\in \mathbb{R}$ is an arbitrary constant. Applying the operator ${^{C}}\mathcal{D}^{\gamma+\delta-1}_q$ to both sides of Eq (3.6), yields

Thus, from the condition $z(0)+\lambda_{1}{{^{C}}\mathcal{D}^{\gamma+\delta-1}_{q}}z(0) = 0,$ Eqs (3.6) and (3.7), we get

From $z(T)+\lambda_{2}{{^{C}}\mathcal{D}^{\gamma+\delta-1}_{q}}z(T) = 0$, Eqs (3.6) and (3.7), we obtain

Upon simplification and substituting $B = -\lambda_{1}A$ in Eq (3.9), we get

and

□

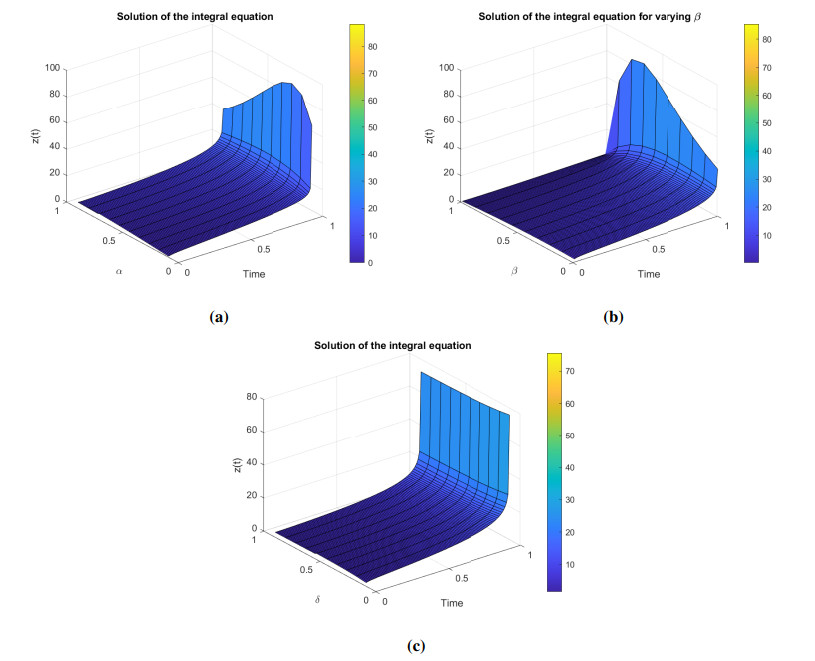
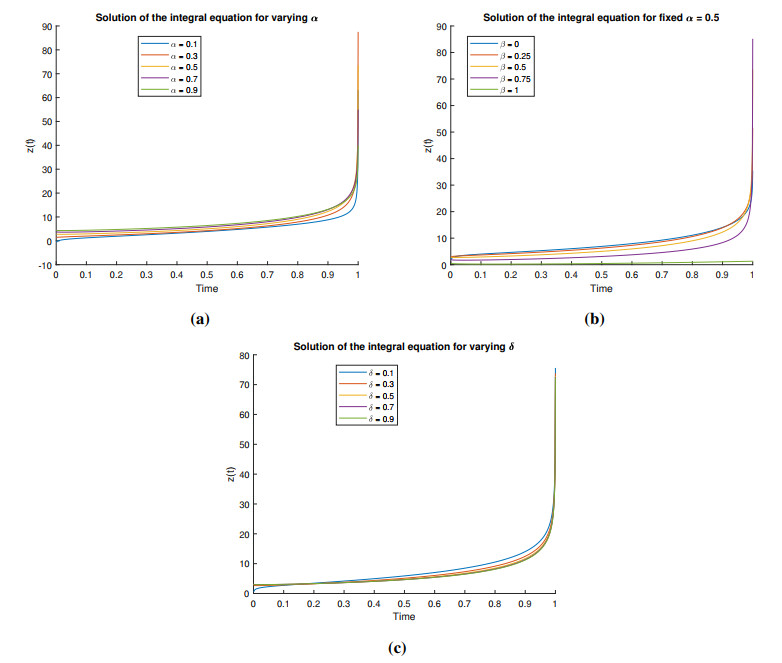
To explore the numerical behavior of the integral solution of the proposed problem (1.6), we vary the fractional-orders associated with the problem. The following parameters, $\alpha, \beta,$ and $\delta,$ respectively, were considered. The respective graphical analysis are shown in Figures 1 and 2, respectively. Figures 1a–1c illustrate the behavior of the solution of the integral (3.2) when varying the fraction-order $\alpha$. Moreover, the corresponding 3D plots is displayed in Figures 2a–2c, respectively.

3.1.   Existence results

By employing Krasnoselskii's fixed-point theorem and Leray-Schauder's nonlinear alternative, in this subsection, we will present the proof of the existence results of (1.6).

Lemma 3.2. (Krasnoselskii's fixed point theorem) ^[52] Let $\mathit{M}\subset\mathcal{X}$ be closed, bounded, convex, and nonempty. Suppose $\mathcal{F}_{1}, \; \mathcal{F}_{2}$ be operators such that:

(i) $\mathcal{F}_{1}z+\mathcal{F}_{2}z_{1}\in\mathit{M}$ whenever $z, z_{1}\in\mathit{M};$

(ii) $\mathcal{F}_{2}$ is a contraction mapping;

(iii) $\mathcal{F}_{1}$ is compact and continuous.

Then there exists $w\in\mathit{M}$ such that $w = \mathcal{F}_{1}w+\mathcal{F}_{2}w.$

Theorem 3.1. Let $0 < \alpha, \delta < 1$, $0\leq\beta\leq1,$ and $\gamma = \alpha+\beta(1-\alpha)$. Suppose that the function $f:[0, T]\times\mathbb{R}\rightarrow \mathbb{R}$ is continuous and satisfies:

$(\mathcal{H}_{1})$ There exists $L > 0, \; (constant)$ such that

$(\mathcal{H}_{2})$ $|f(t, z)|\le\Psi(t), \forall\in(t, z)\in[0, T]\times\mathbb{R}$ and $\Psi\in\mathcal{C}([0, T], \mathbb{R}^+);$

Then there exists at least one solution of the quantum Hilfer and Caputo separated boundary value problem (1.6) on $[0, T],$ provided that

where

Proof. Now, from equation 3.2, define $\mathcal{F}:\mathcal{X}\rightarrow \mathcal{X}$ by

Suppose $\sup_{t\in[0, T]}\Psi(t) = \|\Psi\|$ and $\sigma\ge\|\Psi\|\Delta$ such that $\mathit{B}_{\sigma} = \left\lbrace x\in\mathcal{X}: \|z\|\le\sigma\right\rbrace.$ Now, we set

and

Then, for any $z, z_{1}\in\mathcal{B}_{\sigma},$ we get

Hence $\|\mathcal{F}_{1}z+\mathcal{F}_{2}z_{1}\|\le \sigma,$ which shows that $\mathcal{F}_{1}z+\mathcal{F}_{2}z_{1}\in\mathit{B}_{\sigma}.$ Therefore, the condition $(i)$ of Lemma 3.2 is satisfied.

To show condition $(ii)$ of Lemma 3.2, we proceed as follows, for any $z, z_{1}\in\mathcal{C}([0, T], \mathbb{R}),$ gives

Consequently, $\|(\mathcal{F}_{2}z)-(\mathcal{F}_{2}z_{1})\|\le L\Big(\Delta-\frac{T^{(\alpha+\delta)}}{\Gamma_{q}(\alpha+\delta+1)}\Big)\|z-z_{1}\|,$ and hence, by (3.10), $\mathcal{F}_{2}$ is a contraction. Hence, condition $(ii)$ of Lemma 3.2 is satisfied.

Moreover, since $f\in\mathcal{C}([0, T], \mathbb{R}),$ the operator $\mathcal{F}_{1}$ is continuous and it is uniformly bounded, as

Set $\sup_{(t, z)\in[0, T]\times\mathcal{B}_{\sigma}}|f(t, z)| = \hat{f}.$ Then

$\rightarrow0.$ as $t_{2}-t_{1}\rightarrow 0,$ independently of $z.$ Hence, as a consequence of the Arzelá-Ascoli theorem, this shows that $\mathcal{F}_{1}$ is compact on $\mathcal{B}_{\sigma}.$

Therefore, since all the assumptions of Lemma 3.2 are satisfied, we conclude that there exists at least one solution of quantum Hilfer and Caputo separated boundary value problem (1.6) on $[0, T].$ □

The next existence result relies on Leray-Schauder's nonlinear alternative.

Lemma 3.3. (Leray-Schauder's Nonlinear Alternative) ^[53] Let $\mathcal{C}\subset\mathcal{X}$ be closed and convex of $\mathcal{X}$, $\mathcal{U}\subset\mathcal{C}$ be open, and $0\in\mathcal{U}.$ Suppose $\mathit{F}:\bar{\mathcal{U}}\rightarrow \mathcal{C}$ is a continuous and compact map. Then either

(i) $\mathcal{F}$ has a fixed point in $\bar{\mathcal{U}}$ or

(ii) $\exists z\in\partial{\mathcal{U}}$ and $\omega\in(0, 1)$ with $z = \omega\mathcal{F}(z).$

Theorem 3.2. Suppose that the function $f:[0, T]\times\mathbb{R}\rightarrow \mathbb{R}$ is continuous and satisfies:

$(\mathcal{H}_{3})$ there exists a function $\varLambda : [0, \infty)\rightarrow (0, \infty)$ continuous and nondecreasing and a function $G\in\mathcal{C}([0, T], \mathbb{R}_{+})$ such that

$(\mathcal{H}_{4})$ There exists $C > 0\; (constant)$ such that

Then, there exists at least one solution of problem (1.6) on $[0, T].$

Proof. Firstly, we show that $\mathcal{F}$ maps a bounded set into bounded sets in $\mathcal{X}.$ For any $k > 0,$ let $\mathbb{B}_{k} = \{z\in\mathcal{X}: \|z\|\le k\}$ be a bounded set in $\mathcal{X}.$ Then, for $t\in[0, T]$ yields

which implies that

Moreover, for $t_{1}, t_{2}\in[0, T]$ with $t_{1} < t_{2}$ and $z\in\mathbb{B}_{k},$ we obtain

This proves the equicontinuity of the set $\mathcal{F}(\mathbb{B}_{k}),$ and by the Arzelá-Ascoli theorem, it is relatively compact. Thus, $\mathcal{F}: \mathcal{X}\rightarrow \mathcal{X}$ is completely continuous.

Now, for $t\in[0, T],$ as in step 1, yields

which gives

By $(\mathcal{H}_{4})$ $\exists C$ such that $C\ne\|z\|.$ Let

Then, $\mathcal{F}:\bar{\mathcal{U}}\rightarrow \mathcal{C}$ is both continuous and completely continuous. From $\mathcal{U}$, there exists no $z\in\bar{\mathcal{U}}$ such that $z = \omega\mathcal{F}(z)$ for any $\omega\in(0, 1).$ Thus, as a consequence of Lemma 3.3, we conclude that $\mathcal{F}$ has a fixed point $z\in\bar{\mathcal{U}}$ which is a solution of problem (1.6). □

3.2.   Uniqueness result

We proceed to establish the uniqueness of problem (1.6) using the Banach contraction principle ^[54].

Theorem 3.3. Let $0 < \alpha, \delta < 1$, $0\leq\beta\leq1,$ and $\gamma = \alpha+\beta(1-\alpha)$. Suppose that $f: [0, T]\times \mathbb{R}\to \mathbb{R}$ fulfills the assumption $(\mathcal{H}_{1}).$ If

where $\Delta$ is defined by (3.11), then, there exists a unique solution of problem (1.6) on $[0, T].$

Proof. To do so, problem (1.6) can be viewed as a fixed-point problem, $z = \mathcal{F}z,$ where $\mathcal{F}$ is defined as in (3.1). Next, we show that $\mathcal{F}$ has a unique fixed point. Indeed, let $\sup_{t\in[0, T]}|f(t, 0)| = \mathcal{K} < \infty$ and $\frac{\mathcal{K}\Delta}{1-L\Delta}\le k.$ First, we show that $\mathcal{F}\mathcal{B}_{k}\subset\mathcal{B}_{k},$ where $\mathcal{B}_{k} = \{z\in \mathcal{X}: \|z\|\le k\}.$ Given $z\in\mathcal{B}_{k},$ gives

and hence $\|(\mathcal{F}z)\|\le k,$ which means that $\mathcal{F}\mathcal{B}_{k}\subset\mathcal{B}_{k}.$

Subsequently, for $t\in[0, T]$ and any $z, z_{1}\in\mathcal{C}([0, T], \mathbb{R}),$ we get

Therefore, $\|(\mathcal{F}z)-(\mathcal{F}z_{1})\|\le L\Delta\|z-z_{1}\|,$ and hence, by (3.13), $\mathcal{F}$ is a contraction, and hence, problem (1.6) has a unique solution on $[0, T]$. □

3.3.   Special cases of the proposed problem.

Case Ⅰ. If $\lambda_{1} = \lambda_{2} = 0,$ problem (1.6) reduces to sequential $q$-Hilfer problems of the form:

Corollary 3.1. Let $0 < \alpha, \delta < 1$, $0\le\beta\leq1,$ and $\gamma = \alpha+\beta(1-\alpha)$. Suppose $f:[0, T]\times\mathbb{R}\rightarrow \mathbb{R}$ is a continuous function. If $z\in\mathit{C}^2_q([0, T], \mathbb{R}),$ then $z$ satisfies the problem (3.14) if and only if $z$ satisfies the integral equation:

Case Ⅱ. If $\lambda_{1} = \lambda_{2} = 1,$ problem (1.6) reduces to sequential $q$-Hilfer problems of the form:

Corollary 3.2. Let $0 < \alpha, \delta < 1$, $0\le\beta\leq1,$ and $\gamma = \alpha+\beta(1-\alpha)$. Suppose $f:[0, T]\times\mathbb{R}\rightarrow \mathbb{R}$ is a continuous function. If $z\in\mathit{C}^2_q([0, T], \mathbb{R}),$ then $z$ satisfies the problem (3.16) if and only if $z$ satisfies the integral equation:

Case Ⅲ. Let $\beta = 1,$ then $\gamma = 1,$ and problem (1.6) reduces to the sequential $q$-Caputo fractional-order differential equation given by

Corollary 3.3. Let $0 < \alpha, \delta < 1$ be orders of fractional derivative and $0 < q < 1$ be quantum number. Suppose $f:[0, T]\times\mathbb{R}\rightarrow \mathbb{R}$ is a continuous function. If $z\in\mathit{C}^2_q([0, T], \mathbb{R}),$ then $z$ satisfies the problem (3.18) if and only if $z$ satisfies the integral equation:

Case Ⅳ. Let $\beta = 0,$ then $\gamma = \alpha,$ and problem (1.6) reduces to the sequential $q$-Riemann and Caputo fractional-order differential equation given by

Corollary 3.4. Let $0 < \alpha, \delta < 1$, and $f:[0, T]\times\mathbb{R}\rightarrow \mathbb{R}$ is a continuous function. If $z\in\mathit{C}([0, T], \mathbb{R}),$ then $z$ satisfies the problem (3.20) if and only if $z$ satisfies the integral equation:

where $Q^* = T^{(\alpha+\delta-1)}+(\lambda_{2}-\lambda_{1})\Gamma_{q}(\alpha+\delta)$.

Case Ⅴ. If $q = 1,$ then problem (1.6) reduces to the sequential Hilfer and Caputo boundary value problem of the form:

Corollary 3.5. Let $0 < \alpha, \delta < 1$, $0\le\beta\leq1,$ and $\gamma = \alpha+\beta(1-\alpha)$. Suppose $f:[0, T]\times\mathbb{R}\rightarrow \mathbb{R}$ is a continuous function. If $z\in\mathit{C}^2([0, T], \mathbb{R}),$ then $z$ satisfies the problem (3.22) if and only if $z$ satisfies the integral equation:

4.   Examples

Example 4.1. Consider the sequential fractional differential equation involving $q$-Hilfer and $q$-Caputo fractional derivatives:

Now, we choose constants as $\alpha = 3/5$, $\beta = 2/5$, $\delta = 4/5$, $q = 1/2$, $T = 9/7$, $\lambda_{1} = 11/23$, $\lambda_{2} = 13/29$. Then we compute $\gamma = 19/25$, which yields $\gamma+\delta-1 = 14/25$. These information can be used to find that $Q\approx1.123420003$, $\Delta\approx3.821919331,$ and $\Delta-(T^{(\alpha+\delta)}/\Gamma_{q}(\alpha+\delta))\approx2.578777897$.

Case $(i)$. Let the nonlinear bounded function $f(t, z)$ be presented by

where $h:[0, 9/7]\to\mathbb{R},$ and $p$ is a positive constant.

Thus,

and

for all $t\in[0, 9/7]$ and $z, z_1\in \mathbb{R}$. Therefore, conditions $(\mathcal{H}_{1})$ and $(\mathcal{H}_{2})$ in Theorem 3.1 are satisfied with $L = 1/p$. Thus, from Theorem 3.1, we say that problem (4.1) with (4.2) has at least one solution on $[0, 9/7]$ if $p > 2.578777897$. In addition, the unique solution of problem (4.1) with (4.2), can be guaranteed if $p > 3.821919331$ by applying the result in Theorem 3.3.

Case $(ii)$. If the nonlinear, unbounded function $f(t, z)$ is expressed as

then it is easy to check that condition $(\mathcal{H}_{1})$ is fulfilled by inequality

with $L = 1/4$, which leads to

Hence, problem (4.1) with (4.3) has a unique solution on $[0, 9/7]$.

Example 4.2. Consider the sequential boundary value differential equations in the frame of $q$-Hilfer and $q$-Caputo fractional derivatives given by:

Here $\alpha = 5/7$, $\beta = 3/4$, $\gamma = 13/14$ (by computing), $q = 2/3$, $\delta = 6/7$, $T = 8/9$, $\lambda_{1} = 17/31$, $\lambda_{2} = 19/37,$ and $\gamma+\delta-1 = 11/14$. From all constants, we have $Q\approx0.8787426597$ and $\Delta\approx2.500884518$.

Now, we see that the nonlinear non-Lipschitzian function $f(t, z)$ shown in the right-side of the first equation in (4.4), is bounded by

Choosing $G(t) = 1/(t+2)$ and $\Lambda(u) = (1/4)u^2+(1/3)$, we have $\|G\| = 1/2,$ and we can find that $\exists C\in(0.492701921, 2.706166297)$ satisfying $(\mathcal{H}_4)$. Thus, by applying Theorem 3.2, we say that problem (4.4) has at least one solution on $[0, 8/9]$.

5.   Conclusions

Since the appearance of fractional operators, many research articles have been dedicated to improving and generalizing those operators. This paper investigates the existence and uniqueness of the results of a sequential boundary value problem in the setting of $q$-Hilfer and $q$-Caputo fractional derivatives with separated boundary conditions. The proposed problem is new and can be visualized as a generalization of Hilfer, $q$-Caputo, Caputo, $q$-Riemann-Liouville, and Riemann-Liouville fractional differential equations.

Author contributions

All authors contributed equally and significantly to writing this article. All authors read and approved the final manuscript.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

The first author was supported by King Mongkut's University of Technology North Bangkok with contract no. KMUTNB-Post-67-08. This research budget was allocated by National Science, Research and Innovation Fund (NSRF) and King Mongkut's University of Technology North Bangkok with Contract no. KMUTNB-FF-67-B-02.

Conflicts of interest

The authors declare no conflict of interest.