1.
Introduction
Due to production fluctuation, the strength of a component, say X, might fluctuate substantially and unexpectedly. When the component is put to work, it experiences stress, denoted by Y. If X is smaller than Y, the component will fail rapidly since its strength is insufficient to bear the stress. If Y is less than X, the component's strength can sustain the stress, and it functions appropriately. Since the initial study of Birnbaum and McCarty [1], the topic of evaluating the stress–strength index R=P(Y<X) and its related deductions when X and Y are two independent random variables has received a lot of attention. Because R indicates the link between a system's stress and strength, it can also be referred to as the system's stress–strength index. Kotz et al. [2] provided many examples of the stress–strength model in their book, including the reliability of rocket engines, earthquake resistance, and the reaction of leprosy patients to medicine. For example, the likelihood of successfully firing the engine can be represented by R=P(Y<X), where X is the strength of the rocket chamber and Y is the maximum chamber pressure generated when a solid propellant is burned. Recent research has focused on evaluating the stress–strength model using various statistical methods. See, for example, Al-Mutairi et al. [3], Sharma et al. [4], Hassan et al. [5], Pak et al. [6], Alsadat et al. [7], Nassar et al. [8], and Quintino et al. [9].
1.1. Inverted Chen distribution
When evaluating the stress–strength index, it is crucial to choose the appropriate statistical distribution for modelling the random variables X and Y. Choosing the best model relies on accurately evaluating the stress–strength index R. In this paper, we assume that the random variables X and Y are independent and follow the inverted Chen (IC) distribution. Srivastava and Srivastava [10] were the first to introduce the IC distribution, while Agiwal [11] extensively examined its properties. For the random variable X, for instance, the probability density function (PDF) of the IC distribution takes the form
where a and b are the shape parameters and η(x;b)=1−ex−b. The cumulative distribution function (CDF) related to (1.1) is expressed as follows:
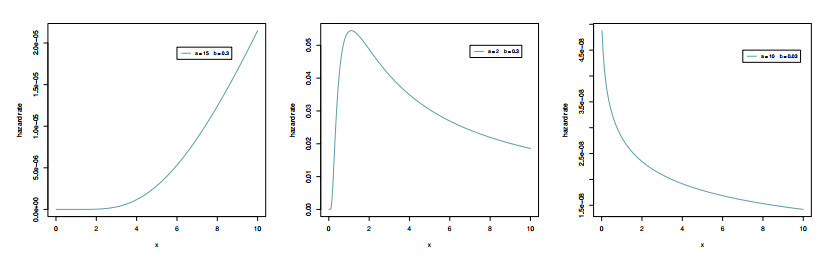
Agiwal [11] indicated that the IC distribution can be used to model the data with an upside-down bathtub hazard rate function (HRF). The form of the HRF that corresponds to the IC distribution is given by
Figure 1 shows plots of the HRF for the IC distribution, using selected parameter values. The figure demonstrates that the IC distribution can model data with hazard rate shapes that increase or decrease, not just the upside-down bathtub shape as pointed out by Agiwal [11].
The variability in the HRF enhances the flexibility of the IC model and makes it a suitable choice for reliability and other studies.
Kumar et al. [12] conducted a study on inferences in two IC populations using joint type-Ⅱ censored samples, one of the few studies to employ the IC distribution. Assume the stress and strength random variables are independent and have the IC distribution, i.e., X∼IC(a1,b) and Y∼IC(a2,b). Then, the stress–strength index R takes the following form, according to Agiwal [11],
1.2. Adaptive progressive type-Ⅱ censoring
When performing reliability studies, it is not expected to gather data on the failure times of all tested items, particularly for highly trustworthy items. In these scenarios, the researcher gathers data from a subset of the tested units. This is referred to in the literature as censored samples. There are numerous censoring samples in the literature, including single and multi-stage censoring plans. One of the most popular censorship strategies is known as progressive type-Ⅱ censoring (PTIIC). This method enables researchers to remove live items using a specified removal pattern R_=(R1,R2,…,Rm), where m represents the required number of failures taken from a random sample of size M. At the time of each failure, say Xi:m:n,i=1,…,m, Ri items are randomly chosen and eliminated from the test. Immediately, after observing the mth failure, the test is terminated and all remaining items Rm are discarded. Several works considered the PTIIC plan; see, for more detail, Aggarwala and Balakrishnan [13], Balakrishnan and Lin [14], Singh et al. [15], Dey et al. [16], and Chacko and Mohan [17]. Ng et al. [18] proposed a broader censorship strategy, with the traditional PTIIC approach standing as a particular case. They termed their strategy adaptive progressive type-Ⅱ censoring (APTIIC). This strategy makes certain that we will complete the test upon we have reached the specified amount of failures and that the total test length will be near the optimal prefixed time, indicated by T. This plan runs similarly to the PTIIC plan but with two choices for stopping the experiment. In the first scenario, when Xm:m:n<T, the experiment terminates at Xm:m:n, resulting in the standard PTIIC method. Otherwise, if T happens earlier than Xm:m:n, the removal pattern is adjusted by setting Ri=0,i=d+1,…,m−1, where d represents the number of detected failures recorded before T. In this situation, at Xm:m:n, all the leftover items are eliminated from the experiment, i.e., R∗=M−m−∑Ji=1Ri. Many authors incorporated the APTIIC plan; see, for example, Nassar et al. [19], Du and Gui [20], Dey et al. [21], Alotaibi et al. [22] and Lv et al. [23], Xiao et al. [24], Kumari et al. [25], and Nassar et al. [26], among others.
1.3. Problem statement and assumptions
Despite the IC distribution's usefulness due to its flexible HRF, little interest has been given in the literature to the estimations of its parameters or any function that includes these parameters, particularly when censoring samples are involved. The only study that has investigated the estimation issues of the stress–strength index is the study of Agiwal [11]. However, this study only considered the complete sample case, which is not practical due to its time-consuming nature, especially when dealing with highly reliable products. Additionally, that study focused solely on point estimation and did not provide any information regarding interval estimation for either parameters or the reliability measure. Another limitation is that they assumed the shape parameter has a non-informative prior in the Bayesian estimation framework. This assumption has drawbacks, particularly concerning the resulting improper posterior distribution. As a result, one can see that the current literature reveals a gap in research focusing on estimating the parameters as well as the stress–strength index involving the IC distribution in censoring schemes. This study aims to fill this gap by investigating both classical and Bayesian estimation approaches of the IC distribution based on the APTIIC scheme. This can be performed using the following assumptions:
(1) The strength X and stress Y are random variables that each follow the IC distribution with PDF and CDF defined in Eqs (1.1) and (1.2), respectively.
(2) The shape parameter, which is unknown, is the same for both random variables X and Y.
(3) In Bayesian estimation, the random variables a1,a2 and b are independent random variables and each follows a gamma distribution.
The limitations mentioned are addressed in this study as follows:
(ⅰ) Utilizing a general censoring plan, specifically the APTIIC scheme, allows for the collection of the necessary data, with the complete sample serving as a special case.
(ⅱ) Investigating two classical confidence interval approaches and two Bayes intervals for estimating unknown parameters and the stress–strength index.
(ⅲ) Studying the Bayesian estimation of the various parameters when the shape parameter follows the gamma prior distribution, which includes the non-informative prior as a special case.
1.4. Motivation, objectives and contributions of the study
Motivated by (1) the flexibility of the IC distribution in modelling increasing, decreasing, and upside-down bathtub data, (2) the efficiency of the APTIIC scheme in collecting data, particularly when the product under consideration is reliable, and (3) the significance of estimating the stress–strength index in various engineering and industrial applications. We encourage dealing with the estimations of the IC distribution using APTIIC samples. First, we focus on the estimations of the unknown parameters and then move on to the stress–strength index R=P(Y<X). To achieve this, from a classical perspective, the method of maximum likelihood is used to obtain point estimates of various parameters due to its desirable properties. Approximate confidence intervals (ACIs) are derived from the asymptotic properties of these estimates. However, since the stress–strength index is in the interval [0,1], this approach may produce confidence intervals with negative lower bounds or upper bounds exceeding one. To address this issue, a normal approximation of the log-transformed estimates is also employed to get the suitable intervals. The maximum likelihood approach is known to provide inefficient estimates when dealing with small sample sizes. To address this limitation, we employ the Bayesian estimation method, which combines prior knowledge about the unknown parameters with available data to derive Bayes estimates and Bayes intervals. In this context, we utilize two loss functions to obtain the Bayes point estimates, along with the Bayes credible intervals (BCIs). These intervals are based on the assumption that the posterior distribution is symmetric; however, they may not perform well in cases of asymmetric posterior distributions. To overcome this issue, we use the highest posterior density (HPD) credible intervals. For more detail about Bayesian estimation, see Li et al.[27,28,29] and Ni et al. [30].
Based on the above discussion, we can summarize the main objectives of this study as follows:
(1) Getting the maximum likelihood estimates (MLEs) and two sorts of ACIs for the unknown parameters a1,a2 and b.
(2) Acquiring MLE and two types of ACIs employing the delta method for the stress–strength index R=P(Y<X).
(3) Studying the Bayesian estimations of a1, a2, b, and R=P(Y<X) leveraging both symmetric squared error (SSE) and asymmetric LINEX loss functions. The Bayes estimates are computed by drawing samples from posterior distribution via implementing the so–called Markov Chain Monte Carlo (MCMC) technique.
(4) Obtaining the BCIs and HPD credible intervals for a1, a2, b, and R=P(Y<X).
(5) Conducting simulation research with multiple experimental designs to investigate the efficacy of classical and Bayesian estimations. The assessments are based on several common precision measurements.
(6) Investigating a pair of real-world data sets to put the estimation strategies presented into practice.
The contributions of this study can be listed below:
(1) Compare the classical point estimates of the parameters and the stress–strength index of the IC distribution with two Bayes estimates, utilizing the SSE and LINEX loss functions. This comparison will highlight how different loss functions impact the precision and bias of the parameter estimates.
(2) This study compares four interval estimates to assess their effectiveness in quantifying uncertainty in stress–strength analysis.
(3) This study examines the accuracy of different estimators under various censoring schemes and parameter settings through extensive simulation studies. The goal is to recommend the most suitable estimation approach for estimating the stress–strength index of the IC distribution.
(4) Demonstrating the applicability of the IC distribution allows for the analysis of real-world data sets and the estimation of the stress–strength index. As indicated later, the data analysis of two applications shows that the IC distribution is suitable for modelling two engineering data sets.
Following this introduction, the paper will be organised as follows: Section 2 examines the MLEs of a1, a2, and b, as well as the stress–strength index R and two types of corresponding ACIs. In Section 3, we utilize the MCMC technique to obtain Bayesian estimates of the various parameters, using both the SSE and the LINEX loss functions, in addition to two types of credible intervals. Section 4 consists of a simulation study to evaluate the accuracy of the estimates obtained. Section 5 presents an analysis of two actual data sets. Finally, in Section 6, the paper is concluded.
2.
Classical inference
This section focuses on the maximum likelihood estimation of the IC distribution, specifically the unknown parameters a1, a2 and b, as well as the stress–strength index R. The point estimates for these parameters, as well as two ACIs, are covered.
2.1. Point estimation
Let X_=(X1:m1:M1,…,Xm1:m1:M1) be an APTIIC sample of size m1 picked from the IC(a1,b) population, where X1:m1:M1<⋯<Xd1:m1:M1<T1<Xd1+1:m1:M1<⋯<Xm1:m1:M1, with removal pattern R_=(R1,…,Rd1,0,…,0,R∗). In addition, let Y_=(Y1:m2:M2,…,Ym2:m1:M2) be an observed APTIIC sample of size m2 taken from the IC(a2,b) population, such that Y1:m2:M2<⋯<Yd2:m2:M2<T2<Yd2+1:m2:M2<⋯<Ym2:m2:M2, with progressive censoring plan S_=(S1,…,Sd2,0,…,0,S∗). Here, d1 and d2 stand for the number of recorded failures before time T1 and T2, respectively. For brevity, we use Xi and Yi instead of Xi:m1:M1 and Yi:m2:M2, respectively, in this work. Let x_ and y_ be the realizations of X_ and Y_, respectively, then the joint likelihood function of the observed data can be expressed as
where C1 and C2 are constants.
From (1.1), (1.2), and (2.1), the likelihood function can be formed as
where m=m1+m2, Q=∑m1i=1log(xi)+∑m2i=1log(yi) and ψ(xi;a1,b)=1−ea1η(xi;b). The natural logarithm of (2.2), presented by L=logL(a1,a2,b|x_,y_), is
The three normal equations that correspond to (2.3) are given by
and
where ψ1(xi;a1,b)=η(xi;b)1−e−a1η(xi;b) and ψ2(xi;a1,b)=a1x−bilog(xi)ex−bi1−e−a1η(xi;b). To obtain the MLEs of (a1,a2,b), indicated by (ˆa1,ˆa2,ˆb), a computational approach is required to solve the above system of equations in (2.4)–(2.6), as there is no theoretical solution available. For this purpose, we recommend implementing the Newton-Raphson iterative method via 'maxNR()' function of maximization in the 'maxLik' package, which was proposed by Henningsen and Toomet [31].
After computing the MLEs (ˆa1,ˆa2,ˆb), we can use the invariance principle of the MLEs to get the MLE of the stress–strength index R as follows:
2.2. Interval estimation
Here, we first derive the ACIs of the parameters a1,a2, and b using two ways. The first is the ACIs that use the MLEs' normal approximation (ACIs-NA). The second type uses the normal approximation of log-transformed MLEs to get the ACIs (ACIs-NT). Then, we use the same two approaches to get the required interval ranges for the stress–strength index R. The ACIs for the parameters a1,a2, and b are derived via the asymptotic normality of the MLEs, which implies that (ˆa1,ˆa2,ˆb)∼N[(a1,a2,b),Σ], where Σ is the variance-covariance matrix, which is obtained through taking the inverse of the Fisher information matrix. Practically, we estimate the variance-covariance matrix by inverting the observed Fisher information matrix as given below.
The second derivative elements in (2.7) are given by
and
where
and
Following the asymptotic normality of the MLEs, the 100(1−α)% ACIs-NA of a1, a2, and b can be computed as
where zα/2 is the upper (α/2)th percentile point of the standard normal distribution. The interval estimations in (2.8) may yield negative lower bounds. Thus, the ACIs-NT can address this issue. For any parameter, say, for example a1, the ACI-NT can be acquired as follows:
On the other hand, in order to get the ACI-NA and ACI-NT of the stress–strength index R, we first need to obtain the variance of ˆR. Here, we utilize the estimated variance-covariance matrix in (2.7) through the delta method to approximate the estimated variance of ˆR.
where
Upon acquiring ˆVR, the 100%(1−α) ACI-NA and ACI-NT of the stress–strength index R can be obtained, respectively, as
3.
Bayesian inference
In this part, the Bayesian estimation methodology is applied to investigate the Bayes estimates for the unknown parameters a1,a2, and b, as well as the stress–strength index R of the IC distribution with APTIIC data. To begin the Bayesian analysis, we should give our knowledge regarding the unknown parameters a1,a2, and b using prior distributions. Because no conjugate priors are available for the IC distribution, we use the gamma distribution as the prior distribution for the three unknown parameters. The gamma distribution is flexible and can model a wide variety of shapes based on its shape parameter. It shares the same support as the true unknown parameters a1,a2, and b. Additionally, it is commonly used in practice, which facilitates both analytical and numerical Bayesian analysis. The closed-form expressions for the mean and variance of the gamma distribution allow for easy determination of hyperparameter values in numerical parts.
Furthermore, the closed form of its variance enables an examination of how the variation in the prior distribution affects estimation performance. For more detail about using Bayesian estimation, see Zhuang et al. [32]. Assuming the independence of the three parameters and that each follows the gamma prior distribution. Then, we can formulate the joint prior distribution as
where θk,βk>0,k=1,2,3. By linking the likelihood function in (2.2) with the joint prior distribution in (3.1), the posterior distribution of α, β, and λ can possibly be expressed in the following way:
where A refers to the normalized constant obtained as follows
The loss function plays a crucial role in Bayesian analysis by capturing both overestimation and underestimation in investigations. While the symmetric loss function treats overestimation and underestimation equally, the asymmetric loss function assigns different weights to these two situations. In real-world scenarios, the asymmetric loss function is often more practical and beneficial. For instance, in reliability evaluations, overestimating the stress–strength index can have far more severe consequences than underestimating it. In our study, we consider both symmetric and asymmetric loss functions. The first is the SSE loss function, with the Bayes estimator representing the posterior mean. The second is the LINEX loss function, an asymmetric loss function that was introduced by Klebanov [33] and used by Varian [34]. From the posterior distribution in (3.2), the Bayes estimator for any parametric function, say ξ(a1,a2,b), using the SSE and LINEX loss functions are obtained, respectively, as
and
where the parameter τ indicates the direction and degree of symmetry of the LINEX loss function. It is evident from Eqs (3.3) and (3.4) that closed-form expressions for the Bayes estimators cannot be obtained due to the complicated ratio of integrals. To address this issue, we employ the MCMC technique to sample from the posterior distribution and utilize the obtained samples to compute the necessary Bayes estimates, BCIs, and HPD credible intervals. In order to implement the MCMC technique, we first need to determine the full conditional distributions of the unknown parameters. These distributions can be derived from (3.2) for the parameters a1, a2, and b, respectively, as
and
The initial examination of the full conditional distributions in (3.5)–(3.7) indicates that these distributions are unfamiliar and cannot be represented by any known distributions. By simulated one APTIIC random sample from IC(a1,a2,b) population, when (a1,a2,b)=(0.4,0.8,0.5), Mi=100, mi=50, Ti=0.5 (for i=1,2), and Ri=Si=1, i=1,2,...,m, Figure 2 shows that the collected samples of a1, a2, or b from (3.5), (3.6), and (3.7), respectively, behave similar to the Gaussian density.
Therefore, we utilize the Metropolis-Hastings (MH) technique, using a normal proposal distribution (NPD), in order to obtain samples from these distributions. The next processes show how the MH algorithm produces posterior samples.
Step 1. Set k=1 and put (a(0)1,a(0)2,b(0))=(ˆa1,ˆa2,ˆb) as initial guesses.
Step 2. From (3.5) with NPD N(a(k−1)1,ˆΣ11), utilize the MH steps to simulate a(k)1.
Step 3. Use (3.6) and NPD N(a(k−1)2,ˆΣ22) to get a(k)2 via the MH steps.
Step 4. Generate b(k) via the MH steps from (3.7) and NPD N(b(k−1),ˆΣ33).
Step 5. Use (a(k)1,a(k)2,b(k)) to compute the stress–strength index as
Step 6. Put k=k+1.
Step 7. Repeat steps 2-6, M times to obtain
Before getting the Bayes estimates, BCIs, and HPD credible intervals, we remove the first B created samples to guarantee convergence and erase any bias caused by starting value selection. For the stress–strength index R, as an example, and one can simply extend the next formulas to the other parameters, the Bayes estimates using the SSE and LINEX loss functions can be computed, respectively, as
and
To obtain the BCI and HPD credible interval of the stress–strength index R, we first sort R(k) for k=B+1,…,M. Then, the 100%(1−α) BCI is
For the HPD credible interval, specify k∗,k∗=B+1,…,M, where
where [v] refers to the largest integer that is less than or equal to v. Then, the 100%(1−α) HPD credible interval for R is
4.
Monte Carlo comparisons
This part presents extensive Monte Carlo simulations to evaluate the validity of the offered estimations of all new theoretical results reported in the preceding sections.
4.1. Comparison scenarios
Each acquired point (or interval) estimator of ai, i=1,2, b, and R is assessed based on 1,000 APTIIC samples gathered from two different groups of IC(a1,a2,b) population, namely: Set-Ⅰ:(0.4, 0.8, 0.5) and Set-Ⅱ:(1.5, 1.2, 1). At the same time, the plausible value of R using Set-Ⅰ and Set-Ⅱ is considered to be 0.3333 and 0.5555, respectively. To achieve this objective, besides different fashions of progressive censoring (PC) R_ and S_, several comparison designs of Mi, mi, and Ti for i=1,2 are presented in Table 1. For distinction, in Table 1, we present four PC designs, called PC[A], PC[B], PC[C], and PC[D] as uniformly, left-, center-, and right-censored patterns, respectively. For brevity, in Table 1, the progressive pattern PC[A]:{(1⋆20), (1⋆15)} (as an example) means that one survival element will be removed for twenty times in R_ and for fifteen times in S_.
To collect an APTIIC sample of sizes mi, i=1,2, after assigning the level of Mi, Ti, i=1,2, and PC:{R_,S_}, do the following procedure:
Step 1: Fix the actual values of IC(a1,b) population.
Step 2: Draw a traditional PTIIC sample as:
(a) Simulate ζ independent items (say ζ1,ζ2,…,ζm1) from uniform U(0,1) distribution.
(b) Set Λi=ζ(i+∑m1j=m1−i+1Rj)−1i, for i=1,2,…,m1.
(c) Set Ui=1−Λm1Λm1−1⋯Λm1−i+1 for i=1,2,…,m1.
(d) Collect a PTIIC sample (with size m1) from IC(a1,b) distribution by putting:
Step 3: Determine d1 at predetermined T1.
Step 4: Discard the remaining sample (Xd1+2,…,Xm1) when T<Xm1.
Step 5: Use the truncated f(x;a1,b)[1−F(xd1+1;a1,b)]−1 distribution to get (Xd1+2,…,Xm1) order statistics with size M1−d1−∑d1j=1Rj−1.
Step 6: Redo Steps 1–5 for IC(a2,b) population.
Once 1,000 APTIIC samples are gathered, we install two recommended packages in R software, namely:
● A 'maxLik' package (by Henningsen and Toomet [31]) to calculate the frequentist results.
● A 'coda' package (by Plummer et al. [35]) to calculate the Bayes' results.
The primary challenge in a Bayesian setting is determining the hyper-parameter values. To do this, we will select values for the hyper-parameters θi and βi for i=1,2, of ai, i=1,2, in the joint gamma density prior using the past-sample data approach. In this case, we create 10,000 past-complete samples when M1=M2=50. As a result, we assign the values of (θ1,β1,θ2,β2) as (32.3375, 80.4758, 43.5030, 53.1782) and (34.6828, 22.0867, 39.8016, 31.9652) for Set-Ⅰ and Set-Ⅱ, respectively. Additionally, without loss of generality, we shall fix (θ3,β3) of b as (1, 2) and (1, 1) for Set-Ⅰ and Set-Ⅱ, respectively. To run the MCMC technique proposed in Section 3, to ignore the effect of initial guessing, the first 2000 iterations (out of a total of 12000 MCMC iterations) are produced. Following that, the Bayes estimates and BCI/HPD interval estimates of ai, i=1,2, b, and R are obtained.
All estimates of R (as an example) are assessed based on their root mean squared-errors (RMSEs), average relative absolute biases (ARABs), average interval lengths (AILs), and coverage percentages (CPs) as
and
respectively, where J∙(⋅) is the indicator function and (L(⋅),U(⋅)) denotes the (lower, upper) sides of the (1−α)% frequentist (or Bayesian) interval estimate of R. Similarly, the RMSE, ARAB, AIL, and CP values of ai, i=1,2, and b can be easily computed.
4.2. Comparison results
In Tables 2–9, the RMSEs (reported in first-column) and ARABs (reported in second-column) for the maximum likelihood and Bayes estimates (from SSE and LINEX(τ(=−3,+3))) of a1, a2, b, and R are listed, respectively. In Tables 10–17, the AILs (reported in first-column) and CPs (reported in second-column) for the ACI-NA/ACI-NT and BCI/HPD interval estimates (at α=5%) of the same parameters are listed, respectively.
From Tables 2–17, in terms of the lowest level of simulated RMSE, ARAB, and AIL values, we report the following facts:
● A general note of this study is that all offered estimates of ai, i=1,2, b, and R derived by the suggested estimation methods perform well and produce.
● As Mi(or mi) for i=1,2, increases, the associated accuracy of all unknown quantities becomes even better. A similar comment is also noted when ∑m1i=1Ri (or ∑m2i=1Si) narrowed down.
● As Ti, i=1,2, grows, the simulated RMSE, ARAB, and AIL values of all estimates of ai, i=1,2, b, or R decrease while those CPs increase; thus, their estimates behaved satisfactory.
● To acquire high-quality assessments of any unknown lifespan factor in the presence of the suggested censored data, the investigator ought to maximize the total test length while accounting for the test's cost.
● Due to prior gamma knowledge, all Bayes' MCMC results of ai, i=1,2, b, or R perform better than other competitor estimates, as predicted. A similar comment is also reached when comparing the asymptotic (ACI-NA/ACI-NT) with credible (BCI/HPD) methods.
● Comparing the proposed point and interval procedures, it is clear that:
– All results developed from the Bayes' MCMC and HPD interval methods outperformed frequentist estimates. It is the best we expected.
– The 95% ACI-NA results of ai, i=1,2, and R performed superior compared to those developed from 95% ACI-NT, whereas the 95% ACI-NT results of b performed superior compared to 95% ACI-NA results.
– The 95% HPD results of ai, i=1,2, b, or R performed superior compared to those developed from 95% BCI results.
– The estimates obtained using the LINEX loss function for ai, i=1,2, b, or R outperform those acquired using the classical approach or the Bayes estimates obtained using the SSE loss function.
● As a1, a2, and b increase, it is observed that:
– The RMSE and ARAB values of ai, i=1,2 and R decrease; while those of b increase.
– The AIL values of ai, i=1,2, b, or R increase, while the associated CPs decrease.
● Comparing the proposed schemes PC[A], PC[B], PC[C], and PC[D], for Set-Ⅰ or Set-Ⅱ, it is observed that the offered estimates of ai, i=1,2, or R perform well using PC[B] 'left-censoring' whereas those of b perform well using PC[A] 'uniform-censoring' than others.
● As a summary, in the case of data generated using the proposed sampling mechanism, the Bayesian method via the MH procedure is recommended to analyze the IC parameters or the stress–strength index.
5.
Real-world data analysis
This part offers two examples that demonstrate how the proposed methodologies can be utilized in a practical environment, using various actual data sets from the engineering field.
5.1. Light-emitting diode
A light-emitting diode (LED) is a semiconductor device that emits infrared or visible light when charged with an electric current. Recently, organic white LEDs (WOLEDs) have emerged as a promising technology for low-power lighting applications because they combine the benefits of high efficiency and low manufacturing costs with the attractive qualities of large surfaces that radiate high-quality white light; see Farinola and Ragni [36]. This application illustrates the applicability of the proposed methodologies for evaluating the lifetime of the M00071 WOLEDs mixed with red, green, and blue colors under two stress levels, namely: 9.64mA (with M1=10) and 17.09mA (with M2=10). In Table 18, each data point has been divided by 1000 for computational purposes. Also, in Table 18, we assume the data set at 9.64mA by X and the other by Y. This data set was originally reported by Zhang et al. [37] and rediscussed by Nassar et al. [38]. To determine if the IC distribution is suitable for the WOLED data sets, the MLEs (with their standard errors (Std.Errs)) of IC parameters a and b are derived first to create the Kolmogorov-Smirnov (KS) statistic with its P-value; see Table 18. Since the computed P-value is far from the desired significance percentage (5%), the IC distribution matches the WOLED data sets satisfactorily.
Additionally, in Figure 3, four fitting diagrams are also investigated to examine the goodness-of-fit results, namely: (1) scaled total-time-on-test (TTT) transform, (2) fitted IC reliability, (3) quantile-quantile, and (4) contour for the log-likelihood of a and b. For both groups X and Y, Figure 3 offers that the fitted TTT-transforms indicate that the WOLED data sets provide an increasing failure rate, the fitted reliability (or quantile-quantile) line captured its empirical-line adequately, and that the acquired values of ˆa and ˆb used in KS calculations existed and are unique. As a result, all subplots in Figure 3 support our appropriate conclusion.
From Table 18, for each group of WOLED data, several artificial APTIIC samples (symbolized by S[i], i=1,2,3,) are generated based on m1=m2=5 and various selects of Ti,i=1,2, R_ and S_; see Table 19. For each sample in Table 19, the point estimations (with their Std.Errs) and 95% interval estimates (with their interval widths (IWs)) developed by the likelihood and Bayes approaches of ai, i=1,2, b, and R are evaluated; see Table 20. Due to our lack of prior information about the IC parameters from the WOLED data, all Bayes calculations will be created using the joint improper gamma prior against (from SSE and LINEX(for τ(=−5,−0.05,+5)). Without loss of generality, we assign M=50,000 and B=10,000 to develop all acquired Bayes' inferences of ai, i=1,2, b, and R. Here, the estimated values of ˆa and ˆb (in Table 20) are used as starting points in MCMC iterations. It is noted, from Table 20, that the estimated values of ai, i=1,2, b, and R obtained from the MCMC method behaved well compared to those obtained from the MCMC method in terms of the smallest estimated Std.Err values. Similar behavior is also noted, from Table 21, if one compares the credible (from BCI/HPD) intervals with the asymptotic (from ACI-NA/ACI-NT) intervals in terms of the smallest estimated IW values.
To see the existence and uniqueness of the reported likelihood estimates of a1, a2, and b in Table 20, for S[i], i=1,2,3, the profile log-likelihoods of a1, a2, and b are plotted; see Figure 4. It shows that all values of ˆa1, ˆa2, and ˆb (in Table 20) existed and are unique. Using Sample S[1] (as an example), Figure 5 displays the trace and density plots of a1, a2, b, and R using their staying 40,000 draws after burn-in. To distinguish, in Figure 5, the sample mean (or Bayes' estimate from SSE) and the two HPD bounds of a1, a2, b, and R are defined by red-soled and red-dashed lines, respectively. It shows that the simulated MCMC variates for all unknown parameters are appropriately mixed and that the associated conditional PDFs are approximately symmetric.
5.2. Pump motor
A pump motor is an electrical induction device that transfers electrical energy into mechanical energy. In centrifugal pumps, the motor drives the rotating movement of the pump shaft, which is linked to the pump's impeller. This application analyzes a real-data set that consists of the failure times (in hours) of a motor for a pump that can pump multiple liquids at various rates. The liquid density was used to determine stress and failure times under two stress levels, namely: 1.0g/ml (with M1=10) and 1.4g/ml (with M2=10); see Abdel Ghaly et al. [39]. In Table 22, each failure time point has been divided by 1000 for computational requirements. Also, in Table 22, we assume the data set at 1.0g/ml by X and the other by Y.
Similar to the same fitting scenarios discussed in Application 6, from the entire pump's data sets, Table 22 shows that the IC lifetime distribution fits the pump's data sets well. Figure 6(a), for Group-X, emphasizes that the Group-X data set has an increasing hazard rate. Figure 6(d), for Group-X, shows that the estimates ˆa≅12.895 and ˆb≅3.3477 (provided in Table 22) existed and are unique. Figure 6(b) and (c) support the same fact. A similar fitting information from Figure 6 is also observed for Group-Y.
Now, from the complete pump's data sets, different APTIIC samples with m1=m2=5 are generated; see Table 23. For S[i] for i=1,2,3, the MLE and Bayesian estimations of ai, i=1,2, b, and R (with their Std.Errs) are obtained and reported in Table 24. Further, 95% ACI-NA/ACI-NT and 95% BCI/HPD interval estimates (with their IWs) of the same subjects are also presented in Table 25. In this application, all Bayes settings are set to be the same as those described in Subsection 5.1. Regarding the lowest Std.Errs, the results (in Table 24) showed that the offered Bayes' estimations of ai, i=1,2, b, and R (created from SSE or LINEX) perform better than those obtained from the competitive likelihood approach. In terms of minimum IWs, from Table 25, the same observation is also reached when comparing asymptotic intervals with credible intervals.
The log-likelihoods of a1, a2, and b for S[i], i=1,2,3, are displayed in Figure 7 to demonstrate the presence and uniqueness of ˆa1, ˆa2, and ˆb in Table 24. It demonstrates that all values for the MLEs of a1, a2, or b (in Table 25) existed and are distinct. Using Sample S[1] (as an instance), Figure 8 depicts the trace and density diagrams of a1, a2, b, and R after 40,000 draws following burn-in. It demonstrates that the created MCMC variates of a1, a2, b, and R are effectively mixed, and that the corresponding conditional PDFs are roughly symmetric.
6.
Conclusions
In this research, we explored different methods for estimating the stress–strength index. We considered the strength and stress components as independent random variables, following inverted Chen distributions. These distributions had similar shape parameters but separate scale parameters. The estimations are constructed using adaptive progressive type-Ⅱ censoring samples. We used the maximum likelihood method, a traditional approach, to estimate the model parameters, as well as the stress–strength index and two types of approximate confidence intervals. The delta method is used to approximate the variance of the stress–strength index. The Bayes estimates of the model parameters and the stress–strength index are estimated using gamma priors under squared error and LINEX loss functions. Given the complexity of the posterior distribution, the Markov Chain Monte Carlo technique is employed to generate samples from the conditional distributions and then compute the Bayes estimates. Bayesian credible intervals and the highest posterior density credible intervals are also calculated. We perform a comprehensive numerical analysis to assess the provided estimates using various testing plans, considering factors such as root mean squared error, interval lengths, and coverage probabilities. Two illustrative examples are investigated to provide a better understanding of the suggested approaches. The numerical results demonstrate that Bayes estimates using the LINEX loss function are superior to other Bayes and classical estimates for estimating model parameters and the stress–strength index. Furthermore, the highest posterior density credible intervals exhibit a minimum interval length compared to Bayes credible intervals and the two classical confidence intervals for various parameters. The real data analysis shows the effectiveness of the inverted Chen distribution in fitting two engineering data sets as well as in modelling the stress–strength index derived from these data. Although we have not come across any related work developing the asymptotic (or credible) intervals for the inverted Chen parameters or its stress–strength index in the presence of datasets gathered from the proposed sampling strategy, we advise to extend the new results presented in this work to other sampling strategies. Finally, it is important to note that this study assumes the stress and strength random variables are independent. However, in cases where these variables may be dependent, it is crucial to incorporate that relationship into the model. This idea requires further investigation in future work. See for more detail, James and Chandra [40], Chandra et al. [41], and Shang and Yan [42].
Author contributions
Refah Alotaibi: Conceptualization, methodology, investigation, funding acquisition, writing – original draft; Mazen Nassar: Conceptualization, methodology, writing – review and editing, writing – original draft; Zareen A. Khan: Conceptualization, investigation, writing – review and editing; Ahmed Elshahhat: Methodology, software, witing – original draft. All authors have read and approved the final version of the manuscript for publication.
Funding
The authors extend their appreciation to the Deanship of Scientific Research and Libraries in Princess Nourah bint Abdulrahman University for funding this research work through the Research Group project, Grant No. (RG-1445-0011).
Acknowledgments
The authors would like to express thank to the Editor-in-Chief and anonymous referees for their constructive comments and suggestions. The authors extend their appreciation to the Deanship of Scientific Research and Libraries in Princess Nourah bint Abdulrahman University for funding this research work through the Research Group project, Grant No. (RG-1445-0011). We would like to thank Wejdan Alajlan for her careful reading of the paper and constructive suggestions for improving its results.
Conflict of interest
There is no conflict of interest.









 DownLoad:
DownLoad:










