The traveling wave solution and dynamics analysis of the fractional order generalized Pochhammer–Chree equation

1.   Introduction

In the late 19th and early 20th centuries, some mathematicians began to study fractional order differential equations. However, due to the limitations of mathematical tools and the lack of practical application requirements at that time, research progress in this field was relatively slow. In the past few decades, with the advancement of science and technology and the increasing demand for practical applications, the study of fractional order differential equations has gradually emerged. More and more scholars are paying attention to this field and have achieved many important research results. At present, the research on fractional differential equations has involved multiple directions, including the definition, properties, calculation methods, solution methods, stability analysis, control strategies, etc. of fractional derivatives. In addition, the application of fractional differential equations in various fields is constantly expanding and deepening. Fractional differential equations have unique characteristics, wide application fields, and important historical significance. With the continuous advancement of science and technology and the increasing demand for practical applications, it is believed that fractional differential equations will play a more important role in the future.

Compared with classical integer order differential equations ^[1,2], fractional order differential equations ^{[3,4,5,6,7,8,9,10,11,12,13]} have significant characteristics: nonlocality and memory; power law characteristics and low-rate decay; fractal structure and multiple time scales. Fractional differential equations have shown their importance in multiple fields. Compared with integer order differential equations, fractional-order differential equations can more accurately describe the dynamic behavior of certain complex systems. For example, in fields such as rheology, thermal systems, acoustics, and mechanics, fractional calculus models can provide clearer physical meanings and more concise expression processes. Fractional order differential equations have wide applications in control theory, signal processing, image processing, biomedical engineering, and other fields. For example, in biomedical engineering, fractional differential equations can be used to describe physical processes such as electrical conductivity and thermal conductivity of biological tissues. The study of fractional differential equations has not only promoted the development of mathematics and physics; but also facilitated research in related interdisciplinary fields. For example, the combination of fractional calculus with fractional dynamics, fractional thermodynamics, fractional electromagnetics, and other fields provides new research perspectives and methods for these areas.

In recent years, nonlinear problems in the fields of communication, traffic control, physics, and chemistry can usually be simulated using fractional order partial differential equations (FPDEs) ^[3,4,5,6,7]. Therefore, obtaining numerical simulations and exact solutions for such FPDEs is one of the key areas of current research ^[8,9,10]. Many predecessors devoted themselves to the research of this field of work. Moreover, analyzing the qualitative behavior of such equations without solving their solutions is also a hot topic in the current academic community, and many important theoretical studies have been reported ^[11,12,13]. On the one hand, as early as 2010, Professor Liu ^[14] proposed a polynomial complete discriminant system method to solve traveling wave solutions of nonlinear partial differential equations. In recent years, many experts and scholars have applied this method to the traveling wave reconstruction of FPDEs. On the other hand, Professor Li ^[15] transformed nonlinear partial differential equations into ordinary differential equations through traveling wave transformation, and transformed the ordinary differential equation into a two-dimensional planar dynamical system. By studying the phase diagram of the two-dimensional dynamical system, the dynamic behavior of the ordinary differential equation was analyzed. This article will consider the qualitative behavior and traveling wave solutions of a very typical FPDE based on the research work in the above two aspects.

In this section, we review the definition of conformable fractional derivatives.

Definition 1.1. ^[16] Let $f:[0, \infty)\rightarrow \mathbf{R}$. Then, the conformable fractional derivative of $f$ of order $\varrho$ is defined as

the function $f$ is $\varrho$-conformable the differentiable at a point $t$ if the limit in Eq (1.1) exists.

Proposition 1.2. ^[16] The conformable fractional derivative possesses the following properties:

(i) $D_{t}^{\varrho}(t^{\mu}) = \mu t^{\mu-\varrho}, \forall\mu\in\mathbf{R}$.

(ii) $D_{t}^{\varrho}(af(t)+bg(t)) = aD_{t}^{\varrho}f(t)+bD_{t}^{\varrho}g(t), \forall a, b \in\mathbf{R}$.

(ii) $D_{t}^{\varrho}(f\circ g)(t) = t^{1-\varrho}g(t)^{\varrho-1}g^{'}(t)D_{t}^{\varrho}(f(t))|_{t = g(t)}$.

The conformable fractional derivative has many important properties. The detailed proof is given in the reference ^[17].

The fractional order generalized Pochhammer–Chree equation is an important model in the fields of mathematics and physics, which combines the theory of fractional calculus and nonlinear partial differential equations to describe wave and diffusion phenomena in complex systems. In the future, with the continuous development of mathematical theory, numerical methods, and computational techniques, greater progress and breakthroughs will be made in the research and application of fractional order generalized Pochhammer–Chree equations. In this paper, we present the conformable space-time fractional order generalized Pochhammer–Chree equation ^[18] as follows:

where $q = q(x, t)$ is the longitudinal displacement, which is a function of $x$ and $t$; here $x$ is the abscissa of the particle and $t$ is time. $\mu$, $\theta$, $\nu$ are real parameters. Equation (1.3) is widely used in the field of ion acoustic waves and has attracted the interest of many scholars. It is a model equation that describes the longitudinal deformation wave propagation of an elastic rod under certain limitations, such as under incompressible or approximate conditions. In ^[18], Aniqa Zulfiqar and his collaborators first studied Eq (1.2) using the exp-function method.

When $\varrho = 1$, Eq (1.2) is simplified into an integer-order generalized Pochhammer–Chree equation ^[19] as follows:

where the generalized Pochhammer–Chree equation is a nonlinear partial differential equation that simulates the propagation of elastic waves in a cylinder. Equation (1.3) is a special case of Eq (1.2), which has a wider applicability. In this paper, we will study Eq (1.2).

Specifically, many interesting methods are still studying the traveling wave solution of Eq (1.3), for example, the Sardar sub-equation method ^[19], the Galilean transformation ^[20], the extended direct algebraic method ^[21], the Weierstrass elliptic function method ^[22], the planar dynamical system method ^[23], etc. However, further research is still needed on Eq (1.2). In this article, we plan to continue studying Eq (1.2) using the methods of planar dynamic equilibrium systems and polynomial complete discriminant systems ^[24,25].

The structure of this article is as follows: In Section 2, we perform a traveling wave transformation on Eq (1.2) and perform a series of identity transformations and simplifications, providing a complete discriminant system for fourth-order polynomials. In Section 3, we conduct a study on the dynamic properties of the equations and draw some three-dimensional (3D), two-dimensional (2D) phase portraits and Lyapunov exponent diagram. In Section 4, we obtain the traveling wave solutions of Eq (1.2) by using the complete discriminant system method of quartic polynomials. In Section 5, we conduct numerical analysis and draw 2D graphics, 3D graphics, and contour plots. In Section 6, we summarize the work of this article.

2.   Mathematical preliminaries

We perform the following traveling wave transformation on Eq (1.2).

where $q = q(x, t)$ is a function of $x$ and $t$, $Q = Q(\chi)$ is a real function of $\chi$, $k$ and $\rho$ are parameters.

Through Eq (2.1), we can calculate the following results:

where $Q^{'}$ represents $\frac{dQ}{d\chi}$, $Q^{''}$ represents $\frac{d^{2}Q}{d \chi^{2}}$, $Q^{(4)}$ represents $\frac{d^{4}Q}{d \chi^{4}}$.

Integrating $\chi$ twice on both sides of Eq (2.2), and then taking the integration constant as zero, we can obtain

Assuming $\Xi = Q^{n}$, we can obtain

where $\Xi = \Xi(\chi)$ is a real function of $\chi$, $\Xi^{'}$ represents $\frac{d\Xi}{d \chi}$, $\Xi^{''}$ represents $\frac{d^{2}\Xi}{d \chi^{2}}$.

If we set

then

where $c_{0}$ is the integral constant.

Substituting Eqs (2.5) and (2.6) into Eq (2.4) again yields

So,

where $b_{4} = -\frac{n^{2}\nu}{(n+1)k^{2}\rho^{2}}$, $b_{3} = -\frac{2n^{2}\theta}{(n+2)k^{2}\rho^{2}}$, $b_{2} = \frac{n^{2}(\rho^{2}-\mu)}{k^{2}\rho^{2}}$.

3.   Dynamics analysis

We will analyze the dynamic behavior of Eq (2.5). First, we need to change Eq (2.5) to the following form

where $a_{3} = -\frac{2n^{2}\nu}{(n+1)k^{2}\rho^{2}}, a_{2} = -\frac{3n^{2}\theta}{(n+2)k^{2}\rho^{2}}, a_{1} = \frac{n^{2}(\rho^{2}-\mu)}{k^{2}\rho^{2}}.$

Next, we will study the chaotic behavior caused by periodic disturbances using the following balanced dynamical system.

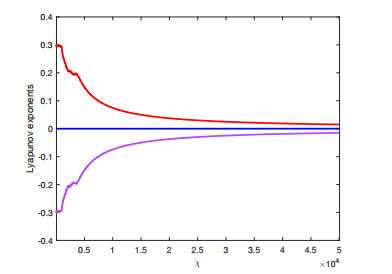
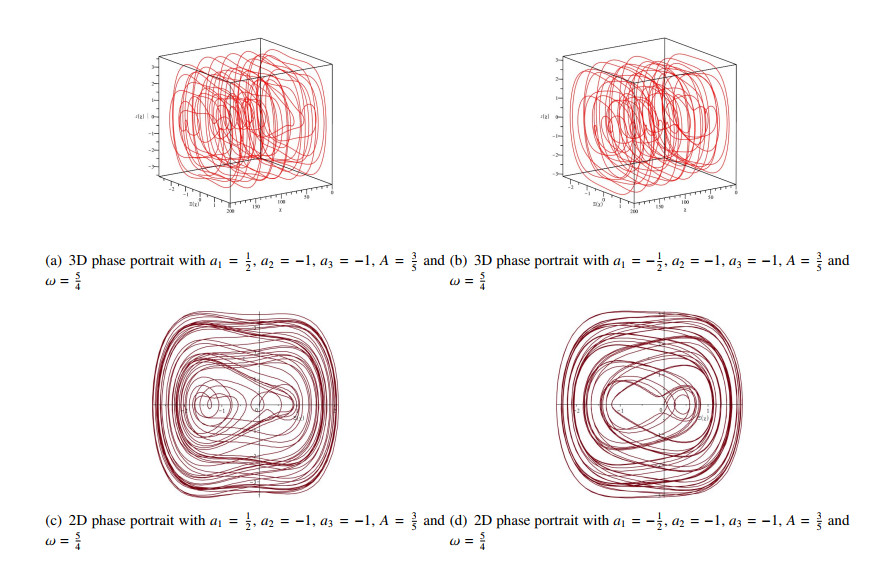
where $A\sin(\omega \chi)$ is disturbance factor, $A$ is amplitude and $\omega$ is frequency. We can use mathematical software to draw the 3D and 2D phase portraits and the Lyapunov exponent diagram of Eq (3.2), as shown in Figures 1 and 2.

The Lyapunov exponent is an important tool for analyzing chaotic systems. It provides a way to quantify a system's response to changes in initial conditions, thereby helping us predict the long-term behavior of the system. The three Lyapunov exponents of Eq (3.2) are shown in Figure 2, which approach 0.014949, 0, and -0.014903, respectively. From the graph, it can be seen that the maximum exponent is a positive number, indicating that the system Eq (3.2) exhibits chaotic behavior.

4.   Traveling wave solutions of Eq (1.2)

If $b_{4} > 0$, we set

where $\Phi = \Phi(\xi)$ is a function of $\xi$ and $\Xi = \Xi(\chi)$ is a function of $\chi$.

Substituting Eq (4.1) into Eq (2.8), we can obtain

where $\Phi^{'} = \frac{d\Phi}{d\xi}$, $\varrho_{1} = (b_{2}-\frac{3b_{3}^{2}}{8b_{4}})(b_{4})^{-\frac{1}{2}}$, $\varrho_{2} = (\frac{b_{3}^{3}}{8b_{4}^{2}}-\frac{b_{2}b_{3}}{2b_{4}})(b_{4})^{-\frac{1}{4}}$, $\varrho_{3} = -\frac{3b_{3}^{4}}{256b_{4}^{3}}+\frac{b_{2}b_{3}^{2}}{16b_{4}^{2}}$.

If $b_{4} < 0$, we set

where $\Phi = \Phi(\xi)$ is a function of $\xi$ and $\Xi = \Xi(\chi)$ is a function of $\chi$.

Substituting Eq (4.3) into Eq (2.8), we can obtain

where $\Phi^{'} = \frac{d\Phi}{d\xi}$, $\varrho_{1} = (-b_{2}+\frac{3b_{3}^{2}}{8b_{4}})(-b_{4})^{-\frac{1}{2}}$, $\varrho_{2} = (-\frac{b_{3}^{3}}{8b_{4}^{2}}+\frac{b_{2}b_{3}}{2b_{4}})(-b_{4})^{-\frac{1}{4}}$, $\varrho_{3} = \frac{3b_{3}^{4}}{256b_{4}^{3}}-\frac{b_{2}b_{3}^{2}}{16b_{4}^{2}}$.

We provide the complete discriminant system for the quartic polynomial $F(\Phi) = \Phi^{4}+\varrho_{1}\Phi^{2}+\varrho_{2}\Phi+\varrho_{3}$ as follows:

We firstly integrate Eqs (4.2) and (4.4) into one equation as follows:

where $\tau = \pm 1$ and $\xi_{0}$ is any integral constant.

Using the complete discriminant system of quartic polynomials as Eq (4.5), we can obtain solutions of Eqs (4.6) and (1.2) in nine different scenarios (see ^[14]).

Case 1. When $I_{2} < 0$, $I_{3} = 0$, $I_{4} = 0$, $F(\Phi) = (\Phi^{2}+\alpha^{2})^{2}$, where $\alpha$ is a real number and $\alpha > 0$. If $\tau = 1$, we can obtain

Thus, the solution of Eq (4.2) is

and the solution of Eq (1.2) is

Case 2. When $I_{2} = 0$, $I_{3} = 0$, $I_{4} = 0$, $F(\Phi) = \Phi^{4}$. If $\tau = 1$, we can obtain

So the solution of Eq (4.2) is

and the solution of Eq (1.2) is

Case 3. When $I_{2} > 0$, $I_{3} = 0$, $I_{4} = 0$, $I_{5} > 0$, $F(\Phi) = (\Phi^{2}-\alpha^{2})^{2}$, where $\alpha$ is a real number and $\alpha > 0$. If $\tau = 1$, we can obtain

When $\Phi > \alpha$ or $\Phi < -\alpha$, the solution of Eq (4.2) is

Thus, the solution of Eq (1.2) is

When $-\alpha < \Phi < \alpha$, the solution of Eq (4.2) is

Then, the solution of Eq (1.2) is

Case 4. When $I_{2} > 0$, $I_{3} = 0$, $I_{4} = 0$, $I_{5} = 0$, $F(\Phi) = (\Phi-\alpha)^{3}(\Phi-\beta)$, where $\alpha$, $\beta$ are real numbers and $3\alpha+\beta = 0$. If $\tau = 1$, when $\Phi > \alpha$ and $\Phi > \beta$, or when $\Phi < \alpha$ and $\Phi < \beta$, the solution of Eq (4.2) is

Therefore, the solution of Eq (1.2) is

If $\tau = -1$, when $\alpha < \Phi < \beta$, or $\beta < \Phi < \alpha$, the solution of Eq (4.4) is

Thus, the solution of Eq (1.2) is

Case 5. When $I_{2}I_{3} < 0$, $I_{4} = 0$, $F(\Phi) = (\Phi-\alpha)^{2}[(\Phi+\alpha)^{2}+\beta^{2}]$, where $\alpha$ and $\beta$ are real numbers. If $\tau = 1$, we can obtain

where $\zeta = \frac{3\alpha}{\sqrt{4\alpha^{2}+\beta^{2}}}$, $\vartheta = \sqrt{4\alpha^{2}+\beta^{2}}-\frac{3\alpha^{2}}{\sqrt{4\alpha^{2}+\beta^{2}}}$, $\xi_{0}$ is an integral constant.

Then, the solution of Eq (4.2) is

Thus, the solution of Eq (1.2) is

Case 6. When $I_{2} > 0$, $I_{3} > 0$, $I_{4} > 0$, $F(\Phi) = (\Phi-\alpha)(\Phi-\beta)(\Phi-\gamma)(\Phi-\delta)$, where $\alpha$, $\beta$, $\gamma$, $\delta$ are real numbers, $\alpha+\beta+\gamma+\delta = 0$ and $\alpha > \beta > \gamma > \delta$. If $\tau = 1$, when $\Phi > \alpha$ or $\Phi < \delta$, we let

when $\gamma < \Phi < \beta$,

Here, we assume that

Then, we have

where $m^{2} = \frac{(\alpha-\delta)(\beta-\gamma)}{(\alpha-\gamma)(\beta-\delta)}$.

Let

Therefore, the solutions of Eq (4.2) are

where $m^{2} = \frac{(\alpha-\delta)(\beta-\gamma)}{(\alpha-\gamma)(\beta-\delta)}$.

Thus, the solutions of Eq (1.2) are

If $\tau = -1$, when $\alpha > \Phi > \beta$, we assume that

When $\delta < \Phi < \gamma$, we assume that

Thus, the solutions of Eq (4.4) are

where $m^{2} = \frac{(\alpha-\beta)(\gamma-\delta)}{(\alpha-\gamma)\beta-\delta)}$.

Then, the solutions of Eq (1.2) are

Case 7. When $I_{2}I_{3}\geq0$, $I_{4} < 0$, $F(\Phi) = (\Phi-\alpha)(\Phi-\beta)[(\Phi-\gamma)^{2}+\delta^{2}]$, where $\alpha$, $\beta$, $\gamma$, $\delta$ are real numbers, $\alpha+\beta+2\gamma = 0$ and $\alpha > \beta$, $\gamma > 0$, $\delta > 0$. If $\tau = 1$, we let

where $\kappa_{1} = \frac{1}{2}(\alpha+\beta)\kappa_{3}-\frac{1}{2}(\alpha-\beta)\kappa_{4}$, $\kappa_{2} = \frac{1}{2}(\alpha+\beta)\kappa_{4}-\frac{1}{2}(\alpha-\beta)\kappa_{3}$, $\kappa_{3} = \alpha-\gamma-\frac{\delta}{\kappa_{6}}$, $\kappa_{4} = \alpha-\gamma-\delta\kappa_{6}$, $\kappa_{5} = \frac{\delta^{2}+(\alpha-\gamma)(\beta-\gamma)}{\delta(\alpha-\beta)}$, $\kappa_{6} = \kappa_{5}+\sqrt{(\kappa_{5})^{2}+1}$. We obtain

where $(\kappa_{7})^{2} = \frac{1}{1+(\kappa_{6})^{2}}$.

Thus, we can get

So, the solution of Eq (4.2) is

So, the solution of Eq (1.2) is

If $\tau = -1$, we let

where $\kappa_{1} = \frac{1}{2}(\alpha+\beta)\kappa_{3}-\frac{1}{2}(\alpha-\beta)\kappa_{4}$, $\kappa_{2} = \frac{1}{2}(\alpha+\beta)\kappa_{4}-\frac{1}{2}(\alpha-\beta)\kappa_{3}$, $\kappa_{3} = \alpha-\gamma-\frac{\delta}{\kappa_{6}}$, $\kappa_{4} = \alpha-\gamma-\delta\kappa_{6}$, $\kappa_{5} = \frac{\delta^{2}+(\alpha-\gamma)(\beta-\gamma)}{\delta(\alpha-\beta)}$, $\kappa_{6} = \kappa_{5}-\sqrt{(\kappa_{5})^{2}+1}$.

Let

where $(\kappa_{7})^{2} = \frac{1}{1+(\kappa_{6})^{2}}$.

Thus, we can obtain

So, the solution of Eq (4.4) is

So, the solution of Eq (1.2) is

Case 8. When $I_{2}I_{3}\leq0$, $I_{4} > 0$, $F(\Phi) = [(\Phi-\alpha)^{2}+\beta^{2}][(\Phi-\gamma)^{2}+\delta^{2}]$, where $\alpha$, $\beta$, $\gamma$, $\delta$ are real numbers, $\alpha+\gamma = 0$ and $\beta\geq\delta > 0$. If $\tau = 1$, we let

where $\kappa_{1} = \alpha\kappa_{3}+\beta\kappa_{4}$, $\kappa_{2} = \alpha\kappa_{4}-\beta\kappa_{3}$, $\kappa_{3} = -\beta-\frac{\delta}{\kappa_{6}}$, $\kappa_{4} = \alpha-\gamma$, $\kappa_{5} = \frac{(\alpha-\gamma)^{2}+\beta^{2}+\delta^{2}}{2\beta\delta}$, $\kappa_{6} = \kappa_{5}+\sqrt{(\kappa_{5})^{2}-1}$. We can get

where $(\kappa_{7})^{2} = \frac{(\kappa_{6})^{2}-1}{(\kappa_{6})^{2}}$.

Let

Thus, the solution of Eq (4.2) is

where $\kappa_{8} = \frac{\delta\sqrt{((\kappa_{3})^{2}+(\kappa_{4})^{2})((\kappa_{3}\kappa_{6})^{2}+(\kappa_{4})^{2})}}{(\kappa_{3})^{2} +(\kappa_{4})^{2}}$.

Thus, the solution of Eq (1.2) is

Case 9. When $I_{2} > 0$, $I_{3} > 0$, $I_{4} = 0$, $F(\Phi) = (\Phi-\alpha)^{2}(\Phi-\beta)(\Phi-\gamma)$, where $\alpha$, $\beta$, $\gamma$ are real numbers, $2\alpha+\beta+\gamma = 0$ and $\beta > \gamma$. If $\tau = 1$, when $\alpha > \beta$ and $\Phi > \beta$, or $\alpha < \gamma$ and $\Phi < \gamma$, the solution of Eq (4.2) is

When $\alpha > \beta$ and $\Phi < \gamma$, or $\alpha < \gamma$ and $\Phi > \beta$, the solution of Eq (4.2) is

When $\alpha > \beta > \gamma$, the solution of Eq (4.2) is

If $\tau = -1$, when $\alpha > \beta$ and $\Phi > \beta$, or $\alpha < \gamma$ and $\Phi < \gamma$, the solution of Eq (4.4) is

When $\alpha > \beta$ and $\Phi < \gamma$, or $\alpha < \gamma$ and $\Phi > \beta$, the solution of Eq (4.4) is

When $\beta > \alpha > \gamma$, the solution of Eq (4.4) is

5.   Discussion and physical explanation

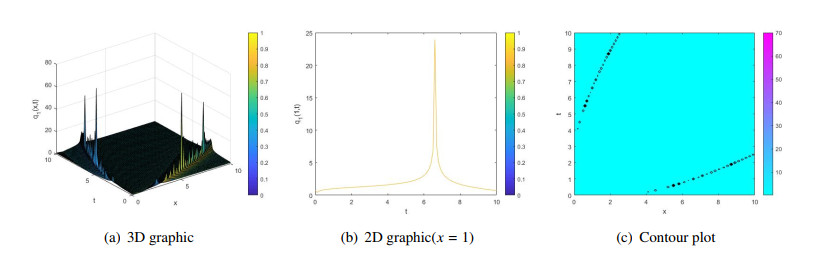
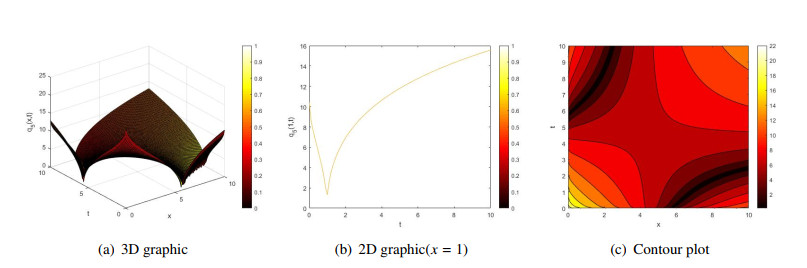
We use mathematical software to draw 2D graphics, 3D graphics, and contour plots of partial solutions to Eq (1.2) under specific parameters as shown in Figures 3–5.

Remark 5.1. Through Figures 3–5, we obtained the 3D graphics, 2D graphics, and contour plots of the trigonometric function solution $q_{1}(x, t)$ and the hyperbolic function solution $q_{3}(x, t)$ for Eq (1.2) with parameters $n = 2$, $\nu = -\frac{3}{4}$, $\theta = -2$, $\mu = 0$, $\kappa = \rho = \alpha = 1$, $\xi_{0} = 0$, $\varrho = \frac{1}{2}$, and the 3D graphic, 2D graphic, and contour plot of the rational function solution $q_{5}(x, t)$ for Eq (1.2) with parameters $n = 2$, $\nu = -\frac{3}{4}$, $\theta = 2$, $\mu = 1$, $\kappa = \rho = \alpha = 1$, $\beta = -3$, $\xi_{0} = 0$, $\varrho = \frac{1}{2}$. These graphs visually display the distribution and changes of data, and can also help readers better understand the above solutions.

6.   Conclusions

This article investigates the chaotic behavior and traveling wave solutions of the fractional order generalized Pochhammer–Chree equation. In the paper, we applied appropriate traveling wave transformations to Eq (1.2) and obtained 3D and 2D phase portraits and a Lyapunov exponent diagram displaying chaotic behavior. We also used a complete discriminant system of quartic polynomials to solve Eq (1.2) and obtained a richer variety of solutions, including rational, hyperbolic, triangular, Jacobian elliptic function solutions, and implicit function solutions. We conducted numerical analysis and used mathematical software to draw 2D graphics, 3D graphics, and contour plots of a trigonometric function solution $q_{1}(x, t)$, a hyperbolic function solution $q_{3}(x, t)$, and a rational function solution $q_{5}(x, t)$ under specific parameters as shown in Figures 3–5. Compared with existing literature ^[21], the polynomial complete discriminant system method used in the paper for solving equations has clearer classification, simpler solution forms, and a wider variety of solution types. This is the advantage and highlight of using a fully discriminative system to solve equations. However, the polynomial complete discriminant system method is currently only applicable to solving partial differential equations that can be converted into ordinary differential equations. In the future, we will discuss the traveling wave solutions and chaotic behavior of more complex partial differential equations.

Conflicts of interest

The author declares no conflicts of interest.