A non-linear fractional neutral dynamic equations: existence and stability results on time scales

1.   Introduction

Fractional calculus emerges from the conventional concepts of calculus, namely integral and derivative operators, similarly to how fractional exponents develop from integer exponents ^[1]. Many people are aware that integer-order derivatives and integrals have distinct interpretations related to physical properties and geometric characteristics. On the other hand, when dealing with fractional-order integration and differentiation, which encompass a swiftly expanding domain in both theory and practical applications to real-life issues, contradict this notion ^[2]. It has been used in many scientific and engineering fields recently, including the study of fluid flow, rheology, diffusive transport, electrical networks, electromagnetic theory and probability, as well as research on viscoelastic materials ^[3,4]. Due to their frequent occurrence in fields including physics, chemistry and engineering, fractional differential equations (FDEs) have attracted attention from numerous studies. Various techniques have been devised to address FDEs, including the widely used Laplace transform approach, the iterative method, the Fourier transform technique, and the operational method ^[5,6]. However, most of these techniques are limited to certain classes of FDEs, especially linear ones with constant coefficients. Linear fractional differential equations with variable coefficients based on the Reimann-Liouville fractional derivative have been solved using the decomposition approach^[7,8,9]. In recent years, academics have paid a lot of attention to the study of fractional differential equations. This is because fractional differential equations are used in many different engineering and scientific fields, such as fluid dynamics, fractal theory, diffusion in porous media, fractional biological neurons, traffic flow, polymer rheology, neural network modelling, viscoelastic panels in supersonic gas flow, real systems characterised by power laws, electrodynamics of complex media, sandwich system identification and the nonlinear oscillation of the earth ^[10,11].

A set that does not contain any closed subset that is empty within the set of real numbers is referred to as time scale ^[12,13]. The introduction of time scale is to serve, unite and improve differential equations theory, as well as various other designated systems of differences. In 1990, Hilger proposed the implementation of time scales as a means to integrate and expand the theory of differential equations, discrete equations and other systems of differential equations defined on a closed subset of the real line that is not empty, and he demonstrated it. Initial value problems involving differentials are characterized by their exclusive existence and one of a kind nature in time scale equations. The combination of separate, closed intervals on time scale functions is an exceptional structure to examine populace dynamics ^{[14,15,16,17]}.

When max$\{n_1, n_2, ......, n_k\} = n$, neutral differential equations (NDEs) arise. NDEs, compared to retarded differential equations in that they depend on derivatives with delays, determine the function's past and present values ^[18]. In high speed computers, elastic networks are modelled by neutral type differential equations ^[19]. Specifically, for the purpose of connecting switching circuits. Since neutral differential equations are found in many branches of practical mathematics, they have received a lot of attention recently. Many researchers have worked on developing neutral differential systems, noting various fixed point techniques, mild solutions, and nonlocal circumstances ^[20,21].

Conversely, the nonlocal problem for abstract evolution equations has been studied by several writers. The question of whether an abstract Cauchy differential equation with nonlocal conditions could have a solution in a Banach space was initially investigated by Byszewski. Since nonlocal conditions are typically more accurate for physical estimations than the classical beginning condition, nonlocal conditions in physical science may be connected with better effect in applications than the classical initial condition ^[22,23,24]. Given that dynamic equations provide a cogent framework for analysing both differential equations and finite difference equations, it can only be considered the presence of dynamic equations along nonlocal initial conditions ^[25,26,27].

In ^[28], using fixed point techniques, the authors investigated on time scales: the existence of a solution to a nonlinear fractional dynamic equation along initial and boundary conditions. In our study, we analyse the existence and stability results of a nonlinear neutral fractional dynamic equation utilising the time scale.

Here, $\mathcal{D}^{\gamma}$ denotes the Riemann-Liouville fractional derivative of order $0 < \gamma < 1$ & $[0, {\mathit{T}}] \in \mathbb{T}$. $\Psi : [m_o, 0]\rightarrow \mathfrak{R}$ are the continuous functions. The functions ${\mathit{g}}(\alpha, {\mathfrak{h}})$ and $\mathcal{L}(\alpha, {\mathfrak{h}})$ are continuous in ${\mathfrak{h}}$, and in ${\mathfrak{h}}$ and ${\mathfrak{i}}$ respectively. $\alpha \in \mathscr{T} = [0, {\mathit{T}}]_\mathbb{T}$ where $\mathscr{T}$ is a time scale interval s.t.,

2.   Preliminaries

In the real numbers $\mathfrak{R}$, a closed subset is called a time scale $\mathbb{T}$. A backward jump operator is a function $\rho = sup\{\alpha \in \mathbb{T}: \rho (\alpha) < \alpha\}$, for $\rho (\alpha) < \alpha$, and $\alpha \in \mathbb{T}$ is called a left scattered point of time scale $\mathbb{T}$ and for $\alpha >$ inf $\mathbb{T}$ and $\rho(\alpha) = \alpha$, then $\alpha$ is called a left dense(ld) point of $\mathbb{T}$.

Left dense(ld) continuous function does not appears in $\mathbb{T}$ for any $\nabla$-derivative. Then, define an operator kappa $\mathbb{T}_{\kappa}$ of $\mathbb{T}$ as: for $\mathbb{T}_{\kappa}$ = $\mathbb{T} \setminus\{{\mathfrak{t}}\}$, else $\mathbb{T}_{\kappa} = \mathbb{T}$ is a left scattered minimum say in time scale ${\mathfrak{t}}$.

Definition 2.1 (^[29]). If $\forall$ left dense points in $\mathscr{T}$, there exists a continuous function, then a function $\mathcal{G}: \mathscr{T}\rightarrow \mathfrak{R}$ is called an ld-continuous function, and in the right dense point the right sided limit appears.

An ld continuous space function is the set of every function from $\mathscr{T}$ to $\mathfrak{R}$ and is denoted by $\mathcal{C}(\mathscr{T}, \mathfrak{R})$.

Remark 2.2. Let a space function $\mathcal{C}(\mathscr{T}, \mathfrak{R}) = \mathscr{X}$ from a Banach space with the norm be defined as:

for $\alpha \in \mathscr{T}$.

Definition 2.3 (^[30]). Consider ${\mathit{g}}(\alpha)$ as a $\nabla$-integrable function to be defined on $\mathscr{T}$. Then,

Definition 2.4 (RL $\nabla$-derivative). ^[31] An ld-continuous function ${\mathit{g}}: \mathbb{T}_{{\mathfrak{k}}^{{\mathfrak{m}}}}\rightarrow \mathfrak{R}$. The RL fractional $\nabla$-derivative of order $\gamma(\geq 0) \in \mathfrak{R}$ is defined as

Remark 2.5. From Definition 2.4 we also get $\mathcal{D}_{0^{+}}^{\gamma}{\mathit{g}}(\alpha) = \mathscr{T}_{0^{+}}^{{\mathfrak{m}}-\gamma}\mathcal{D}_{0^{+}}^{{\mathfrak{m}}}$, where ${\mathfrak{m}} = [\gamma] +1$.

Definition 2.6 (^[24]). Assume $\mathcal{D}\subset \mathcal{C}({\mathit{T}}, \mathfrak{R})$ is a set. If $\mathcal{D}$ is simultaneously bounded and equicontinuous, then it is relatively compact.

Definition 2.7 (^[24]). Assume a set $\mathfrak{B}\subseteq \mathcal{A}$ is bounded and $\mathcal{G}(\mathfrak{B})$ in $\mathcal{A}$ is relatively compact. Then, a mapping $\mathcal{G}:\mathcal{A}\rightarrow \mathfrak{B}$ is completely continuous.

Lemma 2.8 (^[32]). Consider $\mathbb{T}$ is a time scale s.t. $\varphi_{1}, \varphi_{2} \in \mathbb{T}$ with $\varphi_{1} \leq \varphi_{2}$. For a non-decreasing continuous function ${\mathit{z}}: \mathfrak{R}\rightarrow \mathfrak{R}$, we have

Lemma 2.9 (^[33]). Assume ${\mathit{z}}:{[{\mathfrak{a}}, {\mathfrak{b}}]_{\mathbb{T}}}\rightarrow \mathfrak{R}$ is an integrable function. We then have that

holds.

Lemma 2.10 (Krasnoselskii fixed point theorem). ^[34] Assume a Banach space $S$. Consider a closed, bounded, convex and non-empty subset $\mathcal{W}$. Also, $\mathcal{M}, \mathcal{N}$ are operators s.t.,

(a) $\mathcal{M}{\mathfrak{u}} +\mathcal{N}{\mathfrak{v}} \in \mathcal{W}$ whenever ${\mathfrak{u}}, {\mathfrak{v}} \in \mathcal{W}$;

(b) $\mathcal{M}$ is continuous and compact;

(c) $\mathcal{N}$ is a contraction mapping. So, ${\mathit{z}}\in\mathcal{ W}$ s.t. ${\mathit{z}} = \mathcal{M}{\mathit{z}} + \mathcal{N}{\mathit{z}}$.

Lemma 2.11 (^[35]). For $\alpha \in \mathscr{T}$,

where $\xi$ be a positive number, i.e., $\xi > 0.$

Definition 2.12 (^[35]). Equation (1.1) is known as Ulam-Hyers (UH) stable when $\exists$ constant $\mathbb{H}_{({\mathcal{E}_{\mathcal{L}}}, {\mathcal{E}_{{\mathit{g}}}}, \gamma)}\xi > 0$ s.t., for ${\mathfrak{i}}$ of Lemma 2.11 and for $\xi > 0$, there appears a unique solution ${\mathfrak{h}}$ of Eq (1.1)

Definition 2.13 (^[35]). Equation (1.1) is known as generalised UH stable when there appears constant $\mathbb{H}_{({\mathcal{E}_{\mathcal{L}}}, {\mathcal{E}_{{\mathit{g}}}}, \gamma)}\xi = 0$ s.t. for ${\mathfrak{i}}$ of Lemma 2.11, there appears a unique solution ${\mathfrak{h}}$ of Eq (1.1)

3.   Main results

For the results of Eq (1.1), we need some assumptions to obtain the main results and to provide the examples of practical systems, for e.g., evolutionary computation.

(A1) For a continuous function ${\mathit{g}}$ : $\mathscr{T} \times \mathfrak{R} \rightarrow \mathfrak{R}$

There appears a constant $\mathfrak{L}$ $>$ 0 s.t. $|g(\alpha, {\mathfrak{h}})-g(\alpha, {\mathfrak{i}})| \leq \mathfrak{L}|{\mathfrak{h}} - {\mathfrak{i}}|$ for every $\alpha \in \mathscr{T}$, ${\mathfrak{h}}$ $\in \mathfrak{R}$.

(A2) For a continuous function $\mathcal{L}$: $\mathscr{T} \times \mathfrak{R} \rightarrow \mathfrak{R}$

(ⅰ) There appears a constant $\mathcal{M} _{\mathcal{L}}$ $>$ 0 s.t. $|\mathcal{L}(\alpha, {\mathfrak{h}})| \leq \mathcal{M} _{\mathcal{L}}$(1 + $|{\mathfrak{h}}|$) for every $\alpha \in \mathscr{T}$, ${\mathfrak{h}}$ $\in \mathfrak{R}$.

(ⅱ) There appears a constant $\mathcal{E} _{\mathcal{L}}$ $>$ 0 s.t. $|\mathcal{L}(\alpha, {\mathfrak{h}})-\mathcal{L}(\alpha, {\mathfrak{i}})| \leq \mathcal{E} _{\mathcal{L}}|{\mathfrak{h}} - {\mathfrak{i}}|$ for every $\alpha \in \mathscr{T}$, ${\mathfrak{h}}$ $\in \mathfrak{R}$.

(A3) ${\mathfrak{k}}_{1} < 1$, where ${\mathfrak{k}}_{1} < 1$ = $\dfrac{T^\gamma \mathcal{M} _{\mathcal{L}}}{\Gamma(\gamma + 1)}$.

(A4) For $0 < \gamma < 1$, ${\mathfrak{ h}} \in \mathcal{X} \cap {\mathcal{L}_\nabla} (\mathscr{T}, \mathfrak{R})$, and $\mathcal{L}(\alpha, {\mathfrak{h}}(\alpha), D^{\gamma}{\mathfrak{h}}(\alpha)) \nabla \alpha$ = $\mathcal{L}(\alpha, {\mathfrak{h}}(\alpha))\omega({\mathfrak{s}})\nabla \alpha$ for every $\alpha \in \mathscr{T}$, ${\mathfrak{h}}$ $\in \mathfrak{R}$.

(A5) Let there be a set $\mathcal{C}$ = $\{ {\mathfrak{h}} = \mathcal{X}: ||{\mathfrak{h}}|| \leq \upsilon \}$ $\subseteq \mathcal{X}$ and an operator $\Omega : \mathcal{C} \rightarrow \mathcal{C}$, defined as

Theorem 3.1. An ld-continuous function $\mathcal{L} :\mathscr{T} \times \mathfrak{R} \times \mathfrak{R} \rightarrow \mathfrak{R}$. If a function ${\mathfrak{h}} \in C([m_o, 0])$, then ${\mathfrak{h}}(\alpha)$ is said to be a solution of the Eq (1.1) if and only if

for $\alpha \in [0, \mathcal{T}]$ and ${\mathfrak{h}}(\alpha) = \psi(\alpha)$ for $\alpha \in [m_o, 0]$.

Proof. From Eq (1.1),

By (A4), put $\mathcal{D}^{\gamma}{\mathfrak{h}}(\alpha) = \omega(\alpha)$. Then,

The Riemann-Liouville integral equation defines

which implies

Then, by Lemma 2.9 we have

Hence,

From the initial condition, and the condition that holds,

which implies,

Substituting the value of ${\mathfrak{c}}_{1}$ in the above equation, we get,

Hence the solution. □

Theorem 3.2. Assume (A1)–(A5) holds and

Then Eq (1.1) contains a unique solution.

Proof. For $\varsigma = \dfrac{{\mathfrak{k}}_{1}}{1 - {\mathfrak{k}}_{1}}$, we consider

Define $\Omega: \mathfrak{B}\rightarrow \mathfrak{B}$ as

Here, $\Omega$: $\mathfrak{B} \rightarrow \mathfrak{B}$ is well defined. Then, $\alpha \in \mathscr{T}$ and ${\mathfrak{h }}\in \mathfrak{B}$ gives

By using the Lemma 2.8, we have,

Hence,

$\therefore$ $\Omega$: $\mathfrak{B} \rightarrow \mathfrak{B}$ is well defined. Also, we show that the operator $\Omega$ : $\mathfrak{B} \rightarrow \mathfrak{B}$ is contractive and, for $\alpha \in \mathscr{T}$, we have

By Lemma 2.8,

Hence,

where

which implies

$\therefore$ $\Omega$ has an exact contraction mapping. Applying the Banach contraction theorem, $\Omega$ contains a unique fixed point and is said to be a solution for Eq (1.1). □

Theorem 3.3. Assume (A1) and (A2) hold. Then Eq (1.1) contains at least one solution, with the assumptions being satisfied when $\mathfrak{L} + \mathcal{E}_{\mathcal{L}} < 1$.

Proof. To prove the result, we take two maps $\Omega _{1}$ and $\Omega_{2}$ such that

Here $\Omega = \Omega _{1} + \Omega _{2}$ and the following methods are proved.

Step 1: $\Omega _{1}$ is a contraction mapping since

Step 2: For each $h \in \mathfrak{B}$, we know $\Omega = \Omega _{1} + \Omega _{2}$ where $\Omega :\mathfrak{B}\rightarrow \mathfrak{B}$. Then, we have $\Omega _{1}{\mathfrak{h}} + \Omega _{2}{\mathfrak{h}} \in \mathfrak{B}$.

Step 3: Define an operator $\Omega_{2} :\mathfrak{B}\rightarrow \mathfrak{B}$ as

By using Lemma 2.8, we have,

Hence,

$\therefore$ $\Omega_{2}$ :$\mathfrak{B} \rightarrow \mathfrak{B}$ is well defined.

Step 4: To prove the operator $\Omega$ is continuous, consider a sequence ${\mathfrak{h}}_{{\mathfrak{n}}}$ then ${\mathfrak{h}}_{{\mathfrak{n}}} \rightarrow {\mathfrak{h}}_{{\mathfrak{n}}}$ in $\mathcal{C}(\mathscr{T}, \mathfrak{R})$ for any $\alpha \in \mathscr{T}$. Then we have

Since functions $\mathcal{L}$ and ${\mathit{g}}$ are continuous with respect to ${\mathfrak{ h}}$, we have $||(\Omega_{2} {\mathfrak{h}}_{{\mathfrak{n}}}) - (\Omega_{2} {\mathfrak{h}})||_{{\mathfrak{c}}} \rightarrow 0$ as ${\mathfrak{n}}\rightarrow 0$.

$\therefore$ $\Omega_{2}$ is continuous.

Step 5: Let $\vartheta_{1}, \vartheta_{2} \in \mathscr{T}$ such that $\vartheta_{1} < \vartheta_{2}$. Then we have

As $(\varphi - {\mathfrak{s}})^ {\gamma - 1}$ is continuous, $|(\Omega_{2} {\mathfrak{h}})(\alpha_{2}) - (\Omega_{2} {\mathfrak{h}})(\alpha_{1})|\rightarrow 0$ when $\vartheta_{1}\rightarrow \vartheta_{2}$. The proof is same for $\alpha \leq \varphi < {\mathit{T}}$. Thus, the operator $\Omega_{2}$ is equicontinuous. From the followed steps and by the Arzela-Ascoli Theorem, we find that $\Omega_{2}(\mathfrak{B})$ is compact and, from the above steps, we find that Krasnoselskii's fixed point theorem holds and Eq (1.1) contains at least one solution in $\mathfrak{B}$. □

Theorem 3.4. Consider (A1)and (A2) and that inequality (2.1) holds. Then Eq (1.1) is UH stable.

Proof. Let ${\mathfrak{h}}$ be a unique solution of Eq (1.1) and ${\mathfrak{i}}$ be the solution of the inequality

Then by (1.1), we have

Then,

Hence,

Thus,

where

$\therefore$ Equation (1.1) is UH stable.

Setting,

$\therefore$ Equation (1.1) is generalised UH stable. □

4.   Non-linear fractional neutral dynamic equations with nonlocal condition

The inspiration for the nonlocal condition, which extends beyond the classical condition, stemmed from physical issues. Byszewski is credited with conducting the groundbreaking research on nonlocal conditions. Byszewski initially proposed and provided evidence on the result concerning the existence and uniqueness of solutions to abstract Cauchy problems with nonlocal initial conditions mentioned in ^[23]. Various articles have examined the topic of existence and uniqueness outcomes in various types of nonlinear differential equations. Neutral differential equations are found in various fields of applied mathematics and have gained significant prominence in recent times. In ^[36], the authors discussed the Existence and approximation of solutions to neutral fractional differential equations with nonlocal conditions. In ^[37], the authors discussed fractional neutral evolution equations with nonlocal conditions. In ^[38], the authors discussed semilinear neutral fractional stochastic integro-differential equations with nonlocal conditions. In ^[39], the authors discussed existence and data dependence results for neutral fractional order integro-differential equations. Inspired by the above work, we discuss the results for non-linear fractional neutral dynamic equations with non-local conditions.

where ${\mathit{g}}: \mathscr{T} \times \mathbb{T}\rightarrow \mathbb{T}$ which satisfies the below assumption.

(A6) There appears a constant $\mathcal{P} > 0$ s.t., $||{\mathit{g}}({\mathfrak{h}}) - {\mathit{g}}({{\mathfrak{i}}})|| \leq \mathcal{P}||{\mathfrak{h}} - {\mathfrak{i}}||,$ for ${\mathfrak{h}}, {\mathfrak{i}} \in ([0, {\mathit{T}}], \mathfrak{R}).$

Using the nonlocal condition in physics yields a more advantageous outcome compared to the classical initial condition $({\mathfrak{h}}) = {\mathfrak{h}}_{o}$. For example ${\mathit{g}}({\mathfrak{h}})$ is written as,

where ${\mathfrak{c}}_{{\mathfrak{n}}}({\mathfrak{n}} = 1, 2, ....{\mathfrak{n}})$ are known constants and $0 < {\mathfrak{t_{1}}} < ..... < {\mathfrak{t}}_{{\mathfrak{n}}}\leq {\mathit{T}}$. Compared with the initial condition, the nonlocal condition can be more useful.

Theorem 4.1. Assume $0 < \gamma \leq 1$. In Eq (1.1) for ${\mathfrak{h}} \in \mathfrak{X} \cap \mathcal{L}_\nabla(\mathscr{T}, \mathfrak{R})$, ${\mathfrak{h}}$ is the solution of integral equation, and applying the nonlocal condition where the assumption (A6) holds,

Proof. Assume $\mathcal{D}^{\gamma} {\mathfrak{h}}(\alpha) = {\mathit{g}}(\alpha)$. Then, using Eq (1.1) in the above equation we get,

By using the technique used in Theorem 3.2, one can show that $\Omega$ has a fixed point. Then, one can show that the fractional dynamic equation of (1.1) has a fixed point by employing the technique applied in Theorems 3.2 and 3.3. The proof is same as Theorems 3.2 and 3.3 and is omitted. □

5.   Example

Our theoretical conclusions are shown by the example that follows. We will also present the path of the suggested numerical strategy in order to find the numerical solution to the nonlinear problem (1.1).

Example 5.1. Consider a neutral fractional dynamic equation with a time scale along the initial condition ${\mathit{T}} = [0, 1] \cup [2, 3]$ s.t.

where $\alpha \in [0, 2]\cap{\mathit{T}}_{{\mathfrak{k}}}, \gamma = \frac{1}{2}$ and ${\mathfrak{h}}(\alpha)$ is an ld-function which is continuous on ${\mathit{T}}$. Then, for $\varphi_{1}, \varphi_{2} \in \mathfrak{R}$, we set

which satisfies the condition

Then,

and

which implies

Thus, from (5.3) and (5.4), one can get $\mathcal{E} _{\mathcal{L}} = \dfrac{1}{10}$ and $\mathfrak{L} = \dfrac{1}{10}$. Therefore, Eq (5.1) confirms assumptions (A1)–(A3). Substituting the given points in Theorem 3.2, we have

$\therefore$ Equation (5.1) has a solution which is unique in the time scale interval $[0, 2]\cap{\mathit{T}}_{{\mathfrak{k}}}$.

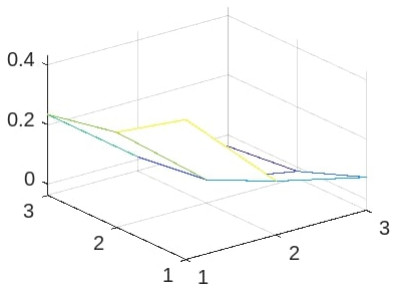
Figure 1 reveals a good agreement between the numerical solution and exact solution across the entire interval.

6.   Conclusions

This work explores nonlinear fractional neutral dynamic equations results that incorporate the Riemann-Liouville nabla ($\nabla$) derivative and includes initial conditions on time scales. Fixed point theory is applied to investigate results of existence, uniqueness and stability.

In the future we look forward to developing new mathematical and computational methods for analysing and modelling the dynamic equation, and applying these methods to solve real-world problems. Some specific areas of interest include chaos theory, nonlinear dynamics, and network science. Additionally, we look forward on the analysis of dynamic equations in integration of machine learning and artificial intelligence techniques.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

The authors extend their appreciation to Prince Sattam bin Abdulaziz University for funding this research work through the project number (PSAU/2023/01/2189822).

Conflict of interest

The authors declares that they have no conflicts of interest.