Synchronization and fluctuation of a stochastic coupled systems with additive noise

1.   Introduction

Caraballo and Kloeden^[1] considered the following two stochastic differential equations (SDEs) in $\mathbb R^{2d}$

where $\alpha, \beta \in \mathbb R^{d}$ are constant vectors with no components equal to zero, $W^1_{t}, W^2_{t}$ are independent two-sided scalar Wiener processes and the continuously differentiable functions $f$, $g$ satisfy the one-sided dissipative Lipschitz conditions. And then the corresponding coupled system is

with a coupling coefficient $\nu > 0$. They proved that the coupled system has a unique stationary solution $({X}^{\nu}_{t}, {Y}^{\nu}_{t})$, which is pathwise globally asymptotically stable. Moreover,

where ${Z}_{t}$ is the unique stationary solution of the averaged system

This phenomenon is that the unique asymptotically stationary solution of the coupled system converges to the unique asymptotically stationary solution of the averaged system, which also called synchronization.

Synchronization is motivated by a wide range of applications in physics, control and biology, see e.g., ^[2,3,4]. The synchronization of deterministic coupled dynamical systems has been presented in both autonomous systems ^[5,6] and nonautonomous systems ^[7]. Caraballo and Kloeden ^[1] and Al-Azzawi et al. ^[8] investigated the effect of additive noise on the synchronization of coupled dissipative systems through the theory of stochastic dynamical systems. Besides, a almost everywhere convergence rate of convergence is established in ^[8]. Liu and Zhao did research on synchronization of coupled systems with additive fractional Brownian motion ^[9] and normal deviation of synchronization of stochastic coupled systems ^[10]. It is worth mentioning that all the above problems are studied from the perspective of dynamic systems.

To be more precise, in this paper we consider the following system.

where $\alpha, \beta \in \mathbb R^{d\times n }$ are constant matrices, $W_{t}$ is a two-sided $\mathbb R^{n}$ valued Wiener process and the continuously differentiable functions $f$, $g$ satisfy some assumption. And then the corresponding coupled system is

with a coupling coefficient $\nu > 0$. We will proved that

where ${Z}_{t}$ is the unique solution of the averaged system

This result can be viewed as a version of the law of large numbers. The central limit theorem corresponds to the law of large numbers, so that the following problem is to prove the central limit theorem for the coupled system.

From the above, to the best knowledge of the authors, the existing literature about synchronization only shows the results of synchronization and the corresponding convergence rate, leaving the central limit theorem of synchronization unsolved. Therefore, this paper mainly introduces the central limit theorem of synchronized system. We show the normalized difference ${\nu}^{\frac{1}{4}}({X}^{\nu}_{t}-Z_t)$ converges weakly to ${Z}^\infty_t$ as $\nu$ tends to infinity, where ${Z}^\infty(t)$ is the unique solution of the SDE

Comparing with the synchronization conclusions in previous articles, these results provide a better approximation of the limit behavior of the synchronized system.

In order to solve these problems, we mainly transforms the coupled system (1.1) to a multi-scale system and then discusses the synchronization under the framework of the averaging principle of the multi-scale system. We can construct some equivalence relations and convert the synchronized system (1.1) into the multi-scale system, as shown below.

Substituting $\hat{X}_t^\nu = X^\nu_t$ and $\hat{Y}_t^\nu = X^\nu_t-Y^\nu_t$ into the SDEs (1.1), and then

Let $\frac{1}{\nu} = \epsilon$, $\tilde{X}_t^\epsilon = \hat{X}^\nu_t$ and $\tilde{Y}_t^\epsilon = \sqrt{\nu}\hat{Y}^\nu_t$,

Thus, to achieve the synchronization and fluctuation of the coupled system (1.1), one needs to verify when $\epsilon$ tends to zero, $X^\epsilon_t$ converges in four square sense to ${Z}_{t}$, and to verify when $\epsilon$ tends to zero, $\frac{1}{{\epsilon}^{\frac{1}{4}}}(\tilde{X}_t^\epsilon-{Z}_{t})$ converge weakly to a SDE

Similarly, the synchronization and fluctuation result of $Y^\nu_t$ is obtained only by $\frac{1}{\nu} = \epsilon$, $\tilde{Y}_t^\epsilon = \hat{Y}^\nu_t$ and $\tilde{X}_t^\epsilon = \sqrt{\nu}\hat{X}^\nu_t$.

The theory of averaging principle which can be regarded as the law of large numbers has been intensively studied in both the deterministic $\alpha = \beta = 0$, see e.g., ^[11,12] and the references therein. For the fluctuation of multi-scale system with singular coefficients, refer to ^[9,13,14]. Note that we can not directly apply the arguments about the averaging principle that have been presented in the previous literature. The key reason is that the relation between singular parameters of fast slow system is not satisfied in the above literature. When $\alpha_\epsilon = \sqrt{\epsilon}$ and $\gamma_\epsilon = \sqrt{\epsilon}$, the $\lim_{\epsilon\rightarrow0}\frac{\alpha_\epsilon}{\gamma_\epsilon} = 1\neq 0$. So that we cannot solve such problems by constructing proper Poisson's equation.

We will make some assumptions.

Assumption 1.1. (Lipschitz condition) For all $x, y$, there exists a constant $L > 0$ such that

Assumption 1.2. (Linear growth condition) For all $x$, there exists a constant $K > 0$ such that

Throughout this paper, the capital letter $C$ denotes a constant (independent of $\epsilon$) whose value may change from line to line.

A brief outline of the paper is as follows. Section 2 contains proofs of results related to synchronization of coupled system (1.1) as the coupled coefficient $\nu$ tends to infinity, including supporting lemma. Section 3 introduces the central limit theorem of synchronized system. Moreover, we give an example to illustrate the utility of our results in Section 4 and a conclusion of this paper in Section 5.

2.   Synchronization of stochastic coupled system

In this section, we will prove that the unique solution to coupled system (1.1) converges in an $L^4$ to the unique solution of averaged system (1.2). Moreover, the convergence rate of synchronization is obtained respectively.

Theorem 2.1. Let ${\tilde{X}}^{\epsilon}_{t}$ and ${Z_t}$ be the unique solutions of (1.3) and (1.2) respectively. If Assumptions 1.1 and 1.2 are satisfied, then

Lemma 2.2. Let $({X}^{\nu}_{t}, Y^{\nu}_{t})$ and ${Z_t}$ be the unique solutions of (1.1) and (1.2) respectively. If Assumptions 1.1 and 1.2 are satisfied, then

Before discussing the synchronization of the stochastic coupled system in detail, we give some conclusions which are used in the next proof.

Lemma 2.3. Let ${\tilde{Y}}^{\epsilon}_{t}$ be the unique solutions of (1.3). Assume that Assumptions 1.1 and 1.2 hold, there exists a constant $C > 0$, such that for any $t\in[0, T]$,

Proof. By (1.3), a simple computation shows that

One gets

And then,

The Grownall lemma yields that

It then follows that

Since the estimate of $E|X_t^\epsilon|^4$ is quite similar to that of $E|Y_t^\epsilon|^4$. Substitute the above inequality obtain

The proof is completed.

With the help of the preceding lemma, Theorem 2.1 is proved.

Proof of Theorem 2.1. Note from (2.1) that

Thus

The proof is completed.

Theorem 2.1 implies in particular that synchronization of the stochastic coupled system. In addition, through a simple example in Section 4 will explicitly illustrate that synchronization for SDE is valid.

3.   Fluctuation of stochastic coupled system

In this section, we will establish a limit in distribution of the fluctuation of ${X}^{\nu}_{t}$ about its typical behavior ${Z_t}$. Before discussing the synchronization of stochastic coupled system in detail, we give some conclusions which are used in the next proof.

Lemma 3.1. The family of process $\{Z^\epsilon_t, 0{\leqslant} t {\leqslant} T, 0 < \epsilon {\leqslant} 1\}$ is weakly compact in $C([0, T];\mathbb R^d)$.

Proof. There exists a convenient criterion for tightness: Kolmogorov's criterion of Remark A.5 in ^[15]. What we only need to verify is that there exist $\alpha$, $\beta$, $C > 0$ such that $E|Z^{\epsilon}_{t + h}- Z^{\epsilon}_t|^\beta{\leqslant} Ch^{1+\alpha}$ for all $t\in[0, T]$.

By (1.3), a simple computation shows that

Using Hölder's inequality, Jensen's inequality, some elementary inequalities and the linear growth conditions of $f$ and $g$, one gets

Taking Lemma 2.3 into consideration, then

Using Hölder's inequality, Jensen's inequality, some elementary inequalities and the Lipschitz conditions of $f$ and $g$, then

Taking Theorem 2.1 and Lemma 2.3 into consideration, then

This estimate guarantees the weak compactness of the family of the processes $\{Z^\epsilon_t, 0{\leqslant} t {\leqslant} T, 0 < \epsilon \leq\; 1\}$.

Denote ${\lambda}^{\epsilon}_{t} = \frac{1}{2}\left(f(\tilde{X}_t^\epsilon)+g(\tilde{X}_t^\epsilon)\right)$ and ${\lambda}_{t} = \frac{1}{2}\left(f(Z_t)+g(Z_t)\right)$. By Taylor's theorem for ${\lambda}_{t}$, one can then derive that

We then have the following decomposition

where, for $0{\leqslant} t{\leqslant} T$,

Theorem 3.2. Let ${\tilde{X}}^{\epsilon}_{t}$ and ${Z_t}$ be the unique solutions of (1.3) and (1.2) respectively. If Assumptions 1.1 and 1.2 are satisfied, then $Z^\epsilon(t): = \frac{X^\epsilon_t-Z_t}{\epsilon^{\frac{1}{4}}}$ converges weakly to $Z_t^0$ in the space $C([0, T];\mathbb R^d)$, where $Z_t^0$ is the solution of

Proof. We split the proof of theorem into two subsection. To begin, the process $\{Z^\epsilon, 0{\leqslant} t {\leqslant} T\}$ is tight in $C([0, T])$ in Lemma 3.1. In view of Prohorov's theorem, we can extract every sequence of such process contains a subsequence converging to a process; Next, we identify the limit via martingale problem.

Let $Q^\epsilon$ be the probability measure of $Z^\epsilon(t)$ in $C([0, T];\mathbb R^d)$. By the Itô formula to a function $\phi\in C^2_b(\mathbb{R}^d)$ with $Z^\epsilon(t)$, one has

where

We will consider the above cases separately. Firstly, by the linear growth of $f$ and $g$, $E|X^{\epsilon}_s-Z_s|^4{\leqslant} C\epsilon$, Lemma 2.3, one gets that

Secondly, the stochastic integrals in $R_2(\epsilon, t, \omega)$ are square integrable. The expected value vanishes by Doob's inequality, that is

Thirdly,

Above all, combine (3.1)–(3.3), then

This concludes the proof of Theorem 3.2.

Theorem 3.3. Let $({X}^{\nu}_{t}, Y^{\nu}_{t})$ and ${Z_t}$ be the unique solutions of (1.1) and (1.2) respectively. If Assumptions 1.1 and 1.2 are satisfied, then $Z^\nu(t): = \nu^{\frac{1}{4}}\left(X^\nu_t-Z_t\right)$ converges weakly to $Z_t^\infty$ in the space $C([0, T];\mathbb R^d)$, where $Z_t^\infty$ is the solution of

Proof. Because of $\nu = \frac{1}{\epsilon}$,

The result of this theorem follows from Theorem 3.2.

Theorem 3.3 implies in particular that fluctuation of the stochastic coupled system.

4.   Numerical simulations

Example 4.1. Consider the following equation

where $W_t$ is a two-sided Wiener process.

The corresponding averaged SDE is

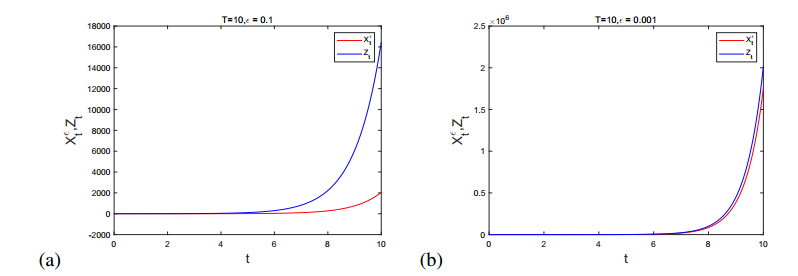
Let's first illustrate the averaging principle through images.

Figure 1 are slow variable $X_t^\epsilon$ and averaged variable $Z_t$ between the same initial value $X_0^\epsilon = 1$, $Z_0 = 1$ and the different $\epsilon$ ($(a)\epsilon = 0.1, (b)\epsilon = 0.01$), respectively. This means that the smaller $\epsilon$ is, the closer $Z_t$ is to $X_t^\epsilon$.

5.   Conclusions

This paper investigates the synchronization and fluctuation of stochastic coupled system with additive Gaussian noise. Through a transformation, such stochastic coupled system is converted into stochastic slow-fast system. The synchronization of the stochastic coupled system is then viewed as the convergence of the corresponding stochastic slow-fast system to its averaged system. The fluctuation considered in this paper is a central limit-type result of the fluctuation between the coupled system and its averaged system. Moreover, we derives the fluctuation of synchronization for the stochastic coupled system by verifying the conditions proposed for the stochastic fast-slow system. In the further research, we will try using the multiscale analysis to consider the synchronization, bifurcation (codimension-1 and codimension-2) which based on the the existence and uniqueness^[16], stability ^[17,18], bifurcation ^[19] of the stochastic reaction-diffusion system. In addition, the numerical solution method in ^[20] may provides theoretical support for our numerical simulation.

Acknowledgments

This work was supported by the Scientific Research Foundation of Anhui Provincial Education Department (No.KJ2020A0483) and the PhD Research Startup Fund for Anhui Jianzhu University (NO.2019QDZ25, 2022QDZ19).

Conflict of interest

The authors declare that there is no conflict of interest.