Some properties for 2-variable modified partially degenerate Hermite (MPDH) polynomials derived from differential equations and their zeros distributions

1.   Introduction

This paper is devoted to study the expressions forms of the solutions and periodic nature of the following third-order rational systems of difference equations

with initial conditions are non-zero real numbers.

In the recent years, there has been great concern in studying the systems of difference equations. One of the most important reasons for this is a exigency for some mechanization which can be used in discussing equations emerge in mathematical models characterizing real life situations in economic, genetics, probability theory, psychology, population biology and so on.

Difference equations display naturally as discrete peer and as numerical solutions of differential equations having more applications in ecology, biology, physics, economy, and so forth. For all that the difference equations are quite simple in expressions, it is frequently difficult to realize completely the dynamics of their solutions see ^{[1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19]} and the related references therein.

There are some papers dealed with the difference equations systems, for example, The periodic nature of the solutions of the nonlinear difference equations system

has been studied by Cinar in ^[7].

Almatrafi ^[3] determined the analytical solutions of the following systems of rational recursive equations

In ^[20], Khaliq and Shoaib studied the local and global asymptotic behavior of non-negative equilibrium points of a three-dimensional system of two order rational difference equations

In ^[9], Elabbasy et al. obtained the form of the solutions of some cases of the following system of difference equations

In ^[12], Elsayed et al. have got the solutions of the systems of rational higher order difference equations

and

Kurbanli ^[25,26] investigated the behavior of the solutions of the following systems

In ^[32], Yalçınkaya has obtained the conditions for the global asymptotically stable of the system

Zhang et al. ^[39] investigated the persistence, boundedness and the global asymptotically stable of the solutions of the following system

Similar to difference equations and systems were studied see ^{[21,22,23,24,27,28,29,30,31,32,33,34,35,36,37,38]}.

2.   The system: $x_{n+1}=\dfrac{y_{n-1}z_{n}}{z_{n}+x_{n-2}}, \; y_{n+1}= \dfrac{z_{n-1}x_{n}}{x_{n}+y_{n-2}},$ $z_{n+1}=\dfrac{x_{n-1}y_{n}}{ y_{n}+z_{n-2}}$

In this section, we obtain the expressions form of the solutions of the following three dimension system of difference equations

where $n\in \mathbb{N}_{0}$ and the initial conditions are non-zero real numbers.

Theorem 1. We assume that $\{x_{n}, y_{n}, z_{n}\}$ are solutions of system (1).Then

and

where $x_{-2} = a, \ x_{-1} = b,$ $x_{0} = c,$ $y_{-2} = d,$ $y_{-1} = e,$ $y_{0} = f,$ $z_{-2} = g,$ $z_{-1} = h$ $and$ $z_{0} = k.$

Proof. For $n = 0$ the result holds. Now assume that $n > 1$ and that our assumption holds for $n-1$, that is,

and

It follows from Eq (1) that

Then we see that

Also, we see from Eq (1) that

Then

Finally from Eq (1), we see that

Thus

By similar way, one can show the other relations. This completes the proof.

Lemma 1. Let $\{x_{n}, y_{n}, z_{n}\}$ be a positive solution of system (1), then all solution of (1) is bounded and approaching to zero.

Proof. It follows from Eq (1) that

we see that

Then all subsequences of $\{x_{n}, y_{n}, z_{n}\}$ (i.e., for $\{x_{n}\}$ are $\{x_{6n-2}\}$, $\{x_{6n-1}\}$, $\{x_{6n}\},$ $\{x_{6n+1}\}$, $\{x_{6n+2}\}$, $\{x_{6n+3}\}\ $ are decreasing and at that time are bounded from above by $K, L$ and $M$ since $K = \max \{x_{-2}, x_{-1}, x_{0}, x_{1}, x_{2}, x_{3}\},$ $L = \max \{y_{-2}, y_{-1}, y_{0}, y_{1}, y_{2}, y_{3}\}$ and $M = \max \{z_{-2}, z_{-1}, z_{0}, z_{1}, z_{2}, z_{3}\}$.

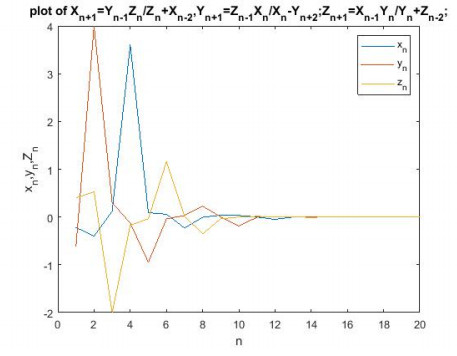
Example 1. We assume an interesting numerical example for the system (1) with $x_{-2} = -.22, \; x_{-1} = -.4, \ x_{0} = .12, \; y_{-2} = -.62,$ $y_{-1} = 4, \ y_{0} = .3, \; z_{-2} = .4, \; z_{-1} = .53\ and\; z_{0} = -2$. (See Figure 1).

3.   The system: $x_{n+1}=\dfrac{y_{n-1}z_{n}}{z_{n}+x_{n-2}}, \; y_{n+1}= \dfrac{z_{n-1}x_{n}}{x_{n}+y_{n-2}},$ $z_{n+1}=\dfrac{x_{n-1}y_{n}}{ y_{n}-z_{n-2}}$

In this section, we get the solution's form of the following system of difference equations

where $n\in \mathbb{N}_{0}$ and the initial values are non-zero real numbers with $x_{-2}\neq \pm z_{0}, \neq -2z_{0},$ $z_{-2}\neq y_{0}, \neq 2y_{0}, \neq 3y_{0}$ and $y_{-2}\neq 2x_{0}, \neq \pm x_{0}.$

Theorem 2. Assume that $\{x_{n}, y_{n}, z_{n}\}$ are solutions of (2). Then for $n = 0, 1, 2, ...,$

and

where $x_{-2} = a, \ x_{-1} = b,$ $x_{0} = c,$ $y_{-2} = d,$ $y_{-1} = e,$ $y_{0} = f,$ $z_{-2} = g,$ $z_{-1} = h$ $and$ $z_{0} = k.$

Proof. The result is true for $n = 0$. Now suppose that $n > 0$ and that our claim verified for $n-1$. That is,

and

Now from Eq (2), it follows that

Also, we see from Eq (2) that

Also, we can prove the other relations.

Example 2. See below Figure 2 for system (2) with the initial conditions $x_{-2} = 11, \; x_{-1} = 5, \ x_{0} = 13, \; y_{-2} = 6,$ $y_{-1} = 7, \ y_{0} = 3, \; z_{-2} = 14,$ $z_{-1} = 9$ $and\; z_{0} = 2.$

4.   The system: $x_{n+1}=\dfrac{y_{n-1}z_{n}}{z_{n}+x_{n-2}}, \; y_{n+1}= \dfrac{z_{n-1}x_{n}}{x_{n}-y_{n-2}},$ $z_{n+1}=\dfrac{x_{n-1}y_{n}}{ y_{n}+z_{n-2}}$

Here, we obtain the form of solutions of the system

where $n\in \mathbb{N}_{0}$ and the initial values are non-zero real numbers with $x_{-2}\neq \pm z_{0}, \neq 2z_{0},$ $z_{-2}\neq \pm y_{0}, \neq -2y_{0}$ and $y_{-2}\neq x_{0}, \neq 2x_{0}, \neq 3x_{0}.$

Theorem 3. If $\ \{x_{n}, y_{n}, z_{n}\}$ are solutions of system (3) where $x_{-2} = a, \ x_{-1} = b,$ $x_{0} = c,$ $y_{-2} = d,$ $y_{-1} = e,$ $y_{0} = f,$ $z_{-2} = g,$ $z_{-1} = h$ $and$ $z_{0} = k.$ Then for $n = 0, 1, 2, ...,$

and

Proof. As the proof of Theorem 2 and so will be left to the reader.

Example 3. We put the initials $x_{-2} = 8, \; x_{-1} = 15, \ x_{0} = 13, \; y_{-2} = 6, \; y_{-1} = 7,$ $y_{0} = 3, \; z_{-2} = 14, \; z_{-1} = 19$ $and\; z_{0} = 2,$ for the system (3), see Figure 3.

The following systems can be treated similarly.

5.   The system: $x_{n+1}=\dfrac{y_{n-1}z_{n}}{z_{n}-x_{n-2}}, \; y_{n+1}= \dfrac{z_{n-1}x_{n}}{x_{n}+y_{n-2}},$ $z_{n+1}=\dfrac{x_{n-1}y_{n}}{ y_{n}+z_{n-2}}$

In this section, we deal with the solutions of the following system

where $n\in \mathbb{N}_{0}$ and the initial values are non-zero real with $x_{-2}\neq z_{0}, \neq 2z_{0}, \neq 3z_{0},$ $z_{-2}\neq \pm y_{0}, \neq 2y_{0}$ and $y_{-2}\neq \pm x_{0}, \neq -2x_{0}.$

Theorem 4. The solutions of system (4) are given by

and

where $x_{-2} = a, \ x_{-1} = b,$ $x_{0} = c,$ $y_{-2} = d,$ $y_{-1} = e,$ $y_{0} = f,$ $z_{-2} = g,$ $z_{-1} = h$ $and$ $z_{0} = k.$

Example 4. Figure 4 shows the behavior of the solution of system (4) with $x_{-2} = 18, \; x_{-1} = -15, \ x_{0} = 3, \; y_{-2} = 6,$ $y_{-1} = .7, \ y_{0} = -3,$ $z_{-2} = 4, \; z_{-1} = -9\ and\; z_{0} = 5$.

6.   The system: $x_{n+1}=\dfrac{y_{n-1}z_{n}}{z_{n}-x_{n-2}}, \; y_{n+1}= \dfrac{z_{n-1}x_{n}}{x_{n}-y_{n-2}},$ $z_{n+1}=\dfrac{x_{n-1}y_{n}}{ y_{n}-z_{n-2}}$

In this section, we obtain the solutions of the difference system

where the initials are arbitrary non-zero real numbers with $x_{-2}\neq z_{0},$ $z_{-2}\neq y_{0}$ and $y_{-2}\neq x_{0}.$

Theorem 5. If $\ \{x_{n}, y_{n}, z_{n}\}$ are solutions of system (5) where $x_{-2} = a, \ x_{-1} = b,$ $x_{0} = c,$ $y_{-2} = d,$ $y_{-1} = e,$ $y_{0} = f,$ $z_{-2} = g,$ $z_{-1} = h$ $and$ $z_{0} = k$. Then

and

Example 5. Figure 5 shows the dynamics of the solution of system (5) with $x_{-2} = 18, \; x_{-1} = -15, \; x_{0} = 3, y_{-2} = 6, y_{-1} = .7,$ $y_{0} = -3, z_{-2} = 4, z_{-1} = -9\ and\; z_{0} = 5.$

7.   Conclusions

This paper discussed the expression's form and boundedness of some systems of rational third order difference equations. In Section 2, we studied the qualitative behavior of system $x_{n+1} = \dfrac{y_{n-1}z_{n}}{z_{n}+x_{n-2}}, \; y_{n+1} = \dfrac{z_{n-1}x_{n}}{x_{n}+y_{n-2}}, \ z_{n+1} = \dfrac{x_{n-1}y_{n} }{y_{n}+z_{n-2}},$ first we have got the form of the solutions of this system, studied the boundedness and gave numerical example and drew it by using Matlab. In Section 3, we have got the solution's of the system $x_{n+1} = \dfrac{y_{n-1}z_{n}}{z_{n}+x_{n-2}}, \; y_{n+1} = \dfrac{z_{n-1}x_{n}}{ x_{n}+y_{n-2}}, \ z_{n+1} = \dfrac{x_{n-1}y_{n}}{y_{n}-z_{n-2}},$ and take a numerical example. In Sections 4–6, we obtained the solution of the following systems respectively, $x_{n+1} = \dfrac{y_{n-1}z_{n}}{z_{n}+x_{n-2}}, \; y_{n+1} = \dfrac{z_{n-1}x_{n}}{ x_{n}-y_{n-2}}, \ z_{n+1} = \dfrac{x_{n-1}y_{n}}{y_{n}+z_{n-2}},$ $x_{n+1} = \dfrac{y_{n-1}z_{n}}{z_{n}-x_{n-2}}, \; y_{n+1} = \dfrac{z_{n-1}x_{n}}{ x_{n}+y_{n-2}}, \ z_{n+1} = \dfrac{x_{n-1}y_{n}}{y_{n}+z_{n-2}},$ and $x_{n+1} = \dfrac{y_{n-1}z_{n}}{z_{n}-x_{n-2}}, \; y_{n+1} = \dfrac{z_{n-1}x_{n}}{ x_{n}-y_{n-2}}, \ z_{n+1} = \dfrac{x_{n-1}y_{n}}{y_{n}-z_{n-2}}.$ Also, in each case we take a numerical example to illustrates the results.

Acknowledgements

This project was funded by the Deanship of Scientific Research (DSR) at King Abdulaziz University, Jeddah, under grant no. (G: 233–130–1441). The authors, therefore, acknowledge with thanks DSR for technical and financial support.

Conflict of interest

All authors declare no conflicts of interest in this paper.