1.
Introduction
MS-algebras were considered by T. S. Blyth and J. C. Varlet[15] as a common properties of de Morgan algebras and Stone algebras. T. S. Blyth and J. C. Varlet[16] described the lattice Λ(MS) of all subclasses of the class MS of all MS-algebras by identities.
A. Badawy, D. Guffovˊa and M. Haviar[10] introduced and characterized the class of principal MS-algebras and the class of decomposable MS-algebras by means of principal MS-triples and decomposable MS-triples, respectively. They obtained a one-to-one correspondence between principal MS-algebras (decomposable MS-algebras) and principal MS-triples (decomposable MS-triples). Moreover, they proved that the class of principal MS-algebras is a subclass of the class of decomposable MS-algebras.
A. Badawy[6] established the relationship between de Morgan filters and congruences of a decomposable MS-algebra. A. Badawy[3] introduced the notion of dL-filters of a principal MS-algebra and characterized certain congruences in terms of dL-filters. Recently, A. Badawy, M. Haviar and M. Ploˇsˇcica[11] introduced and described the notions of congruence pairs and perfect extensions of principal MS-algebras. They characterized the lattice of congruences of a principal MS-algebra in terms of congruence pairs. In 2021, S. El-Assar and A. Badawy[19] characterized permutability of congruences and strong extensions of decomposable MS-algebras by means of congruence pairs.
We review in Section 2 many basic concepts and results that we are using throughout this article. We give an example (Example 2.1) to determine the principsl MS-triple (L∘∘,D(L),φL) assosiated with a certain principal MS-algebra L. In Section 3, we give equivalent conditions for a pair of congruence (θ1,θ2)∈Con(L∘∘)×Conlat(D(L)) to become an MS-congruence pair of a principal MS-algebra L, where θ1 is a congruence on a de Morgan algebra L∘∘ and θ2 is a lattice congruence on the lattice D(L). Also, we characterize the lattice of all MS- congruence pairs which are induced by the Boolean elements of a principal MS-algebra. In Section 4, we discuss many properties of the congruence relation Ψ which defined on a principal MS-algebra L by
We prove that Ψ permutes with each congruence relation θ on L. Also, we characterize 2-permutability of congruences (briefly permutability) by means of MS-congruence pairs. We illustrate Examples 3.1 and 4.1 to clarify Theorems 3.1 and 4.4, respectively. Also, Example 4.2 introduces certain principal MS-algebra L, which has not 2-permutable congruences as well as D(L) has not also 2-permutable congruences. In Section 5, A characterization of n-permutability of congruences of a principal MS-algebra is given, which is a generalization of the characterization of 2-permutability of congruences of such algebras.
2.
Preliminaries
In this section, we recall some specific definitions and remarkable results which are discussed in previous articles [10,14,15,16,17,19].
Definition 2.1. [16] A de Morgan algebra is an algebra (L;∨,∧,−,0,1) of type (2, 2, 1, 0, 0), where (L;∨,∧,0,1) is a bounded distributive lattice and the unary operation − satisfying:
Definition 2.2. [15] A Stone algebra is an algebra (L;∨,∧,∗,0,1) of type (2,2,1,0,0), where (L;∨,∧,0,1) is a bounded distributive lattice and the unary operation ∗ satisfying:
Definition 2.3. [15] An MS-algebra is an algebra (L;∨,∧,∘,0,1) of type (2, 2, 1, 0, 0), where (L;∨,∧,0,1) is a bounded distributive lattice and a unary operation ∘ satisfying:
The class MS of all MS-algebras is equational. A de Morgan algebra is an MS-algebra satisfying the identity, a=a∘∘. The class S of a Stone algebra is a subclass of MS satisfying a∧a∘=0.
The basic properties of MS-algebras which were shown in[15] are given in the following theorem.
Theorem 2.1. Let a,b be any two elements of an MS-algebra L. Then
(1) 0∘∘=0 and 1∘∘=1,
(2) a≤b⇒b∘≤a∘,
(3) a∘∘∘=a∘,
(4) a∘∘∘∘=a∘∘,
(5) (a∨b)∘=a∘∧b∘,
(6) (a∨b)∘∘=a∘∘∨b∘∘,
(7) (a∧b)∘∘=a∘∘∧b∘∘.
An element a of an MS-algebra L is called a closed element of L if a=a∘∘ and an element d∈L is called a dense element of L if d∘=0.
Theorem 2.2. Let L be an MS-algebra. Then
(1) the set L∘∘={a∈L:a=a∘∘} of all closed elements of L is a de Morgan subalgebra of L, see [17],
(2) the set D(L)={a∈L:a∘=0} of all dense elements of L is a principal filter of L, see [10].
Definition 2.4. [10] An MS-algebra (L;∨,∧,∘,0,1) is called a principal MS-algebra if it satisfies the following conditions:
(1) the filter D(L) is principal, that is, there exists an element dL∈L such that D(L)=[dL),
(2) a=a∘∘∧(a∨dL), for all a∈L.
A principal MS-algebra L is a principal Stone algebra if x∘∨x∘∘=1, for all x∈L.
Definition 2.5. [8] A principal MS-triple is (M,D,φ), where
(1) M is a de Morgan algebra,
(2) D is a bounded distributive lattice,
(3) φ is a (0,1)-lattice homomorphism from M into D.
Lemma 2.1. [10] Let L be a principal MS-algebra. Define a map φL:L∘∘→[dL) by the following rule
Then φL is a (0,1)-lattice homomorphism.
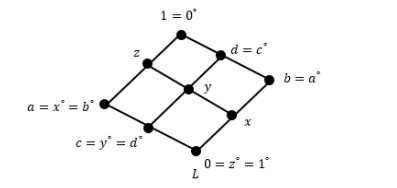
Example 2.1. Consider the MS-algebra L in Figure 1.
It is clear that L is a principal MS-algebra with the smallest dense element z. Then the principal triple (L∘∘,D(L)=[z),φL) which associated with L is given in Figure 2.
Definition 2.6. [22] An equivalence relation θ on a lattice L is called a lattice congruence if (a,b)∈θ and (c,d)∈θ imply (a∨c,b∨d)∈θ and (a∧c,b∧d)∈θ.
Definition 2.7. An equivalence relation θ on an MS-algebra L is called a congruence on L if
(1) θ is a lattice congruence,
(2) (a,b)∈θ implies (a∘,b∘)∈θ.
We use Conlat(L) for the lattice of all lattice congruences of a lattice (L;∨,∧) and Con(L) for the lattice of all congruences of an MS-algebra (L;∨,∧,∘,0,1).
Definition 2.8. Let L be a lattice and θ∈Conlat(L). Then we define the principal congruence generated by (a,b) which denoted by θ(a,b) as follows:
If L is an MS-algebra and θ∈Con(L), then
Let L be an MS-algebra. Then (θL∘∘,θD(L))∈Con(L∘∘)×Conlat(D(L)), where θL∘∘ and θD(L) are the restrictions of θ∈Con(L) to L∘∘ and D(L), respectively. It is clear that θL∘∘ is a congruence relation on a de Morgan algebra L∘∘ and θD(L) is a lattice congruence on a lattice D(L).
The symbols ▽L and △L will be used for the universal congruence L×L and the equality congruence on L, respectively.
The concept of MS-congruence pairs is given as follows:
Definition 2.9. [11] Let L be a principal MS-algebra with a smallest dense element dL. A pair of congruences (θ1,θ2)∈Con(L∘∘)×Conlat(D(L)) will be called an MS-congruence pairs if
A characterization of congruences on a principal MS-algebra via MS-congruence pairs is given in the following theorem.
Theorem 2.3. [11] Let L be a principal MS-algebra with the smallest dense element dL. For every congruence θ on L, the restrictions of θ to L∘∘ and D(L) determine the MS-congruence pair (θL∘∘,θD(L)).
Conversely, every MS-congruence pair (θ1,θ2) uniquely determines a congruence θ on L satisfying θL∘∘=θ1 and θD(L)=θ2. Such congruence can be defined by the rule
Throughout this paper, dL denotes to the smallest dense element of a principal MS-algebra L.
For extra information of MS-algebras, principal MS-algebras and decomposable MS-algebras, we refer the reader to[1,2,3,4,5,6,7,8,9,12,13,14,15,16,17,18,20,21,22,23,24].
3.
Characterization of MS-congruence pairs
In this section, we characterize MS-congruence pairs of a principal MS-algebra and a principal Stone algebra. Also, we describe the lattice of all MS-congruence pairs which induced by the Boolean elements of a principal MS-algebra, in fact such lattice forms a Boolean algebra on it is own.
Lemma 3.1. Let L be a principal MS-algebra and (θ1,θ2) be an MS-congruence pair. Then
Proof. Let (a,b)∈θ1 and (x,y)∈θ2. Then (a∨dL,b∨dL)∈θ2 (by Definition 2.9) and (x,y)∈θ2 imply that (a∨x∨dL,b∨y∨dL)∈θ2. Therefore (a∨x,b∨y)∈θ2 as x,y≥dL. □
Now, we give an important characterization of MS-congruence pairs of a principal MS-algebra.
Theorem 3.1. Let L be a principal MS-algebra with the smallest dense element dL. Then the following statements are equivalent:
(1) (θ1,θ2) is an MS-congruence pair of L,
(2) ConφL(L)⊆θ2, where
Proof. (1⇒2) Let (θ1,θ2) be an MS-congruence pair of L and (x,y)∈ConφL(θ1). Then (x,y)=(φL(a),φL(b))=(a∨dL,b∨dL), where (a,b)∈θ1. Since (a,b)∈θ1, then (a∨dL,b∨dL)∈θ2, by (1). Thus (x,y)∈θ2. So ConφL(θ1)⊆θ2.
(2⇒1) Let Con(θ1)⊆θ2. We prove that (θ1,θ2) is an MS-congruence pair of L. Let (a,b)∈θ1. Then by (2), (a∨dL,b∨dL)=(φL(a),φL(b))∈θ2. □
A characterization of congruence pairs of a principal Stone algebra is given in the following.
Theorem 3.2. Let L be a principal Stone algebra. Let (θ1,θ2)∈Con(L∘∘)×Conlat(D(L)). Then the following statements are equivalent:
(1) (θ1,θ2) is an MS-congruence pair of L,
(2) ConφL(θ1)⊆θ2,
(3) (a,1)∈θ1 and u≥a,u∈D(L) imply (u,1)∈θ2.
Proof. In Theorem 3.1, we proved that (1) and (2) are equivalent.
(2⇒3) Let ConφL(θ1)⊆θ2. Let (a,1)∈θ1 and u≥a,u∈D(L). Then (φL(a),φL(1))∈θ2 by (2). Thus (a∨dL,1)∈θ2.
Now (a∨dL,1)∈θ2 and (u,u)∈θ2,u≥a,u∈D(L) imply (a∨u∨dL,1∨u)∈θ2. Thus (u,1)∈θ2 as u≥a,dL.
(3⇒1) Since L is a Stone algebra then L∘∘ is a Boolean subalgebra of L and hence a∨a∘=1 for each a∈L∘∘. Suppose (3) holds and (a,b)∈θ1. Then (b∘,b∘)∈θ1 implies (a∨b∘,b∨b∘)=(a∨b∘,1)∈θ1 and (a∨a∘,b∨a∘)=(b∨a∘,1)∈θ1. Therefore (β,1)∈θ1, where β=(a∨b∘)∧(a∘∨b). It is clear that β∈L∘∘ and β∧a=β∧b=a∧b. Since β≤β∨dL∈D(L) and (β,1)∈θ1 then (β∨dL,1)∈θ2 by (3). Thus (a∨dL,a∨dL)∧(1,β∨dL)∈θ2 implies (a∨dL,(a∧β)∨dL)=(a∨dL,(a∧b)∨dL)∈θ2. Similarly, we can get (b∨dL,(b∧β)∨dL)=(b∨dL,(a∧b)∨dL)∈θ2. Then (b∨dL,b∨dL)∈θ2. □
Example 3.1. Consider the principal MS-algebra L in Example 2.1 (Figure 1). The lattices Con(L) and A(L) of all congruences of L and all MS-congruence pairs of L are given in Figure 3, respectively.
Where,
And
It is clear that Con(L) isomorphic to A(L) under the isomorphism θ⟼(θL∘∘,θD(L)).
A subset I of a lattice L with 0 is called an ideal of L if
A principal ideal of L generated by a∈L is defined by
A subset F of a lattice L with 1 is called a filter of L if
A principal filter of L generated by a∈L is defined by
Definition 3.1. Let θ be a lattice congruence on a bounded lattice L. Then we have the following important subsets
(i) The Kernel of θ (Kerθ) is the set {x∈L:(x,0)∈θ}, which is an ideal of L,
(ii) The Cokernel of θ (Cokerθ) is the set {x∈L:(x,1)∈θ}, which is a filter of L.
Definition 3.2. [7] An element c of an MS-algebra L is called a Boolean element of L if c∨c∘=1.
It is ready seen that the set B(L)={c:c∨c∘=1} of all Boolean elements of L forms a Boolean subalgebra of L∘∘.
Lemma 3.2. Let c be a Boolean element of a principal MS-algebra L. Then
(1) θ(0,c) is a principal congruence relation on L∘∘ with Kerθ(0,c)=(c], where
(2) θ(φL(c),1) is a principal congruence relation on D(L) with Cokerθ(φL(c),1)=[φL(c)), where
Proof.
(1) It is easy to check that θ(0,c) is a principal congruence relation on L∘∘ with Kerθ(0,c)=(c].
(2) It is easy to show that θ(φL(c),1) is an equivalence relation on D(L). Let (x,y),(m,n)∈θ(φL(c),1). Thus x∧(c∨dL)=y∧(c∨dL) and m∧(c∨dL)=n∧(c∨dL). Then, we get (x∧m,y∧n)∈θ(φL(c),1) and (x∨m,y∨n)∈θ(φL(c),1). Therefore θ(φL(c),1) is a principal congruence on the lattice D(L). Also, we have
□
Now, we observe that every Boolean element c of a principal MS-algebra L associated with the MS-congruence pair of the form (θ(0,c),θ(φL(c∘),1)).
Theorem 3.3. Let L be a principal MS-algebra and c∈L∘∘. Then c is a Boolean element of L if and only if (θ(0,c),θ(φL(c∘),1)) is an MS-congruence pair of L.
Proof. Let c be a Boolean element of L. We proved that θ(0,c) and θ(φL(c∘),1) are MS-congruence on L∘∘ and lattice congruence on D(L), respectively (Lemma 3.2). To show that (θ(0,c),θ(φL(c∘),1)) is an MS-congruence pair, let (x,y)∈θ(0,c). Then
Conversely, let (θ(0,c),θ(φL(c∘),1)) be an MS-congruence pair. Since (0,c)∈θ(0,c) then c∧c∘ = 0∧c∘=0. Now, c∨c∘=(c∘∧c)∘=1. Therefore c is a Boolean element. □
The basic properties of principal congruence relations θ(0,a) and θ(φL(a),1),∀a∈B(L) are given in the following:
Lemma 3.3. Let a,b be Boolean elements of a principal MS-algebra L. Then
(1) a≤b if and only if θ(0,a)⊆θ(0,b),
(2) a=b if and only if θ(0,a)=θ(0,b),
(3) θ(0,0)=△L∘∘ and θ(0,1)=▽L∘∘,
(4) θ(0,a)∨θ(0,b)=θ(0,a∨b),
(5) θ(0,a)∩θ(0,b)=θ(0,a∧b).
Lemma 3.4. Let L be a principal MS-algebra. Then for every a,b∈B(L), we have
(1) a≤b implies θ(φL(a∘),1)⊆θ(φL(b∘),1),
(2) θ(φL(a∘),1)∨θ(φL(b∘),1)=θ(φL(a∨b)∘,1),
(3) θ(φL(a∘),1)∧θ(φL(b∘),1)=θ(φL(a∧b)∘,1),
(4) θ(φL(0),1)=▽D(L) and θ(φL(1),1)=△D(L).
Proof.
(1) Let a≤b. Then a∘≥b∘. Let (x,y)∈θ(φL(a∘),1) then x∧(a∘∨dL)=y∧(a∘∨dL). Now
Then (x,y)∈θ(φL(b∘),1). Therefore θ(φL(a∘),1)⊆θ(φL(b∘),1).
(2) Since a,b≤a∨b then by (1), we have
Then θ(φL(a∨b)∘,1) is an upper bound of θ(φL(a∘),1) and θ(φL(b∘),1). Let θ(φL(c∘),1) be an upper bound of θ(φL(a∘),1) and θ(φL(b∘),1).
Then θ(φL(a∘),1),θ(φL(b∘),1)⊆θ(φL(c∘),1) implies φL(c∘)≤φL(a∘), φL(b∘). Thus φL(c∘)≤φL(a∘∧b∘)=φL(a∨b)∘ and hence θ(φL(a∨b)∘,1)⊆θ(φL(c∘),1). Therefore θ(φL(a∨b)∘,1) is the least upper bound of θ(φL(a∘),1) and θ(φL(b∘),1).
(3) Since a∧b≤a,b then by (1) θ(φL(a∧b)∘,1)⊆θ(φL(a∘),1),θ(φL(b∘),1). Then θ(φL(a∧b)∘,1) is a lower bound of θ(φL(a∘),1) and θ(φL(b∘),1). Let θ(φL(c∘),1) be a lower bound of θ(φL(a∘),1) and θ(φL(b∘),1). Then θ(φL(c∘),1)⊆θ(φL(a∘),1),θ(φL(b∘),1) implies φL(a∘),φL(b∘)≤φL(c∘). Thus φL(a∧b)∘=φL(a∘)∨φL(b∘)≤φL(c∘) and hence θ(φL(c∘),1)⊆θ(φ(a∧b)∘,1). Therefore θ(φL(a∧b)∘,1) is the greatest lower bound of θ(φL(a∘),1) and θ(φL(b∘),1).
(4) Since dL,1∈θ(dL,1), then θ(dL,1)=D(L)×D(L)=▽D(L). Also, let (x,y)∈θ(φL(1),1) then x∧(1∨dL)=y∧(1∨dL). It follows, x=y and hence θ(φL(1),1)=△D(L). □
Theorem 3.4. Let L be a principal MS-algebra. Consider the subsets H and G of Con(L∘∘) and Con(D(L)), respectively as follows:
Then we have
(1) (H;∨,∧,′,△L∘∘,▽L∘∘) is a Boolean algebra, where (θ(0,a))′=θ(a∘,0),
(2) (G;∨,∧,′,△D(L),▽DL) is a Boolean algebra, where (θ(φL(a),1))′=θ(φL(a∘),1).
Proof.
(1) Since θ(0,0)=△L∘∘ and θ(0,1)=▽L∘∘. Then ▽L∘∘,△L∘∘∈H. Let θ(0,a),θ(0,b)∈H. Then we get
and
Therefore H is a bounded lattice. Since B(L) is a Boolean algebra, then a∘∈B(L) for all a∈B(L). Thus θ(0,a∘)∈H. Then we have
and
Therefore (H;∨,∧,′,△L∘∘,▽L∘∘) is a Boolean algebra.
(2) We have △D(L)=θ(φL(1),1)∈G and ▽D(L)=θ(φL(0),1)=▽D(L)∈G. Let θ(φL(a),1),θ(φL(b),1)∈G. Then we get
and
Therefore G is a bounded lattice. Since B(L) is a Boolean algebra, then a∘∈B(L) for all a∈B(L). Thus θ(φL(a∘),1)∈G. Then we have
and
Therefore (G;∨,∧,′,△DL,▽DL) is a Boolean algebra. □
Let L be a principal MS-algebra. Let A(L) be the lattice of all MS-congruence pairs of L. We consider a subset A′(L) of A(L) as follows:
From the above results, we observe that the set A′(L) of all MS-congruence pairs induced by the Boolean elements of a principal MS-algebra forms bounded sublattice of the lattice A(L). Moreover A′(L) is a Boolean algebra on its own.
Theorem 3.5. Let L be a principal MS-algebra. Then (A′(L);∨,∧,′,0A′(L),1A′(L)) is a Boolean algebra, where
Example 3.2. Consider the principal MS-algebra L in Example 2.1. The lattices B(L) and A′(L) of all Boolean elements of L and all MS-congruence pairs of L of the form (θ(0,a),θ(φL(a∘),1)),a∈B(L) are given in the following Figure 4, respectively. It is clear that B(L) and A(L) are isomorphic Boolean algebras under the isomorphism a⟼(θ(0,a),θ(a∘∨z,1)). Also, A′(L) is a bounded sublattice of A(L).
4.
2-permutability of principal MS-algebras
In this section, we characterize the notion of 2-permutability of congruences of a principal MS-algebra by means of MS-congruence pairs.
Let L be an algebra. We say the congruences θ,Φ∈Con(L) are 2-permutable if θ∘Φ=Φ∘θ, that is, (x,a)∈θ and (a,y)∈Φ, x,y,a∈L imply (x,b)∈Φ and (b,y)∈θ for some b∈L. We call the algebra L has 2-permutable congruences (briefly permutable) if every pair of congruences of L permute.
Lemma 4.1. A principal MS-algebra L has 2-permutable congruences if and only if every pair of principle congruences of L permute.
Proof. Assume that every pair of principal congruences on L permute. Let θ and Φ be arbitrary congruences on L and (a,b)∈(θ∘Φ),a,b∈L. Then (a,t)∈θ and (t,b)∈Φ for some t∈L. Then (a,t)∈θ(a,t) and (t,b)∈θ(t,b) imply that (a,b)∈θ(a,t)∘θ(t,b). Thus (a,b)∈θ(t,b)∘θ(a,t). Since θ(a,t)⊆θ and θ(t,b)⊆Φ, then (a,b)∈(Φ∘θ). Therefore θ and Φ permute. The second implication is obvious. □
Define a relation Ψ on a principal MS-algebra L as follows:
Theorem 4.1. Let L be a principal MS-algebra with the smallest dense element dL. Then
(1) Ψ is a congruence relation on L with KerΨ={0} and CokerΨ=D(L),
(2) Ψ is closed congruence on L, that is, (x,x∘∘)∈Ψ,∀x∈L,
(3) max [x]Ψ=x∘∘,x∈L, where [x]Ψ={y∈L:y∘∘=x∘∘} is the congruence class of x modulo Ψ.
Proof.
(1) One can check that Ψ is a congruence relation on L. Now, we have
and
(2) Since x∘∘∘∘=x∘∘ then x∘∘∈[x]Ψ. Thus (x∘∘,x)∈Ψ.
(3) Let y∈[x]Ψ. Then y≤y∘∘=x∘∘ and x∘∘∈[x]Ψ. Thus x∘∘ is the greatest member of the congruence class [x]Ψ. □
Theorem 4.2. Let L be a principal MS-algebra. Then
(1) L/Ψ is a de Morgan algebra,
(2) L/Ψ and L∘∘ are isomorphic de Morgan algebras.
Proof.
(1) It is ready seen that (L/Ψ;∨,∧,{0},D(L)) is a bounded distributive lattice, where L/Ψ={[a]Ψ:a∈L} is the set of all congruence classes module Ψ and
Define ◻ on L/Ψ by ([a]Ψ)◻=[a∘]Ψ,∀a∈L. We have
Then L/Ψ is a de Morgan algebra.
(2) Define g:L∘∘→L/Ψ by
Let a,b∈L∘∘ and a=b. Then a∘∘=b∘∘ implies [a]Ψ=[b]Ψ. It follows that g is well defined map of L∘∘ into L/Ψ. Let a,b∈L∘∘, we get
and
Let [a]Ψ=[b]Ψ. Then a∘∘=b∘∘ implies a=b. Therefore g is an injective map. Let [a]Ψ∈L/Ψ. Then [x]Ψ=[x∘∘]Ψ=g(x). Then g is a surjective map. This deduce that g is an isomorphism of de Morgan algebras. □
Now, we observe that Ψ satisfies the following property,
Theorem 4.3. Let L be a principal MS-algebra. Then Ψ permutes with each congruence of L.
Proof. We prove that Ψ∘θ=θ∘Ψ for all θ∈Con(L). Let (a,b)∈Ψ∘θ. Then (a,z)∈Ψ and (z,b)∈θ for some z∈L. It follows that a∘∘=z∘∘ and (z,b)∈θ. Now
Since [b∘∘∧(a∨dL)]∘∘=b∘∘, then (b∘∘∧(a∨dL),b)∈Ψ. Therefore (a,b∘∘∧(a∨dL))∈θ and (b∘∘∧(a∨dL),b)∈Ψ. imply (a,b)∈θ∘Ψ. Then Ψ∘θ=θ∘Ψ,∀θ∈Con(L). □
Lemma 4.2. Let L be a principal MS-algebra. Then
(1) △L permutes with every congruence on L,
(2) ▽L permutes with every congruence on L.
Proof.
(1) Let (a,b)∈θ∘△L. Then (a,t)∈θ and (t,b)∈△L for some t∈L. Thus (a,t)∈θ and t=b. Then (a,a)∈△L and (a,b)∈θ imply (a,b)∈△L∘θ. Therefore θ∘△L=△L∘θ.
(2) It is obvious. □
In the following theorem, we characterize 2-permutability of congruences of a principal MS-algebra L using MS-congruence pairs.
Theorem 4.4. Let L be a principal MS-algebra. Then the following conditions are equivalent:
(1) L has 2-permutable congruences.
(2) L∘∘ and D(L) have 2-permutable congruences.
Proof. To prove the conditions (1) and (2) are equivalent, we show that the two congruences θ,Φ∈Con(L) are 2-permutable if and only if their restrictions θL∘∘,ΦL∘∘ and θD(L),ΦD(L) have 2-permutable congruences on L∘∘ and D(L), respectively. Firstly, suppose that θ,Φ have 2-permutable congruences on L. Let (x,z)∈θL∘∘∘ΦL∘∘. Then there exist y∈L∘∘ such that (x,y)∈θL∘∘ and (y,z)∈ΦL∘∘. Then we have (x,y)∈θ and (y,z)∈Φ. Since θ,Φ are 2-permutable, then there exists a∈L such that (x,a)∈Φ and (a,y)∈θ. We get (x,a∘∘)=(x∘∘,a∘∘)∈ΦL∘∘ and (a∘∘,z∘∘)∈θL∘∘. Therefore θL∘∘,ΦL∘∘ are 2-permutable. Also, to prove that θD(L),ΦD(L) are 2-permutable, let (x,z)∈θD(L)∘ΦD(L). Then there exist y∈D(L) such that (x,y)∈θD(L) and (y,z)∈ΦD(L). Then we have (x,y)∈θ and (y,z)∈Φ. Since θ,Φ are 2-permutable, there exists a∈L such that
Thus θD(L),ΦD(L) have 2-permutable congruences on D(L).
Conversely, let θ,Φ∈Con(L). Assume that θL∘∘,ΦL∘∘ and θD(L),ΦD(L), have 2-permutable congruences on L∘∘ and D(L), respectively. Let (x,z)∈θ∘Φ. Suppose that x,y,z∈L with (x,y)∈θ and (y,z)∈Φ. Then, by using Theorem 2.3, we get the following statements.
Since θL∘∘,ΦL∘∘ have 2-permutable congruences on L∘∘, there exists an element a∈L∘∘ such that
Since θD(L),ΦD(L) have 2-permutable congruences on D(L), there exists e∈D(L) such that (x∨dL,e)∈ΦDL and (e,z∨dL)∈θDL). It follows that (x∘∘,a)∈Φ,(a,z∘∘)∈θ and (x∨dL,e)∈Φ,(e,z∨dL)∈θ. Since L is a principal MS-algebra we have
Since θ,Φ are compatible with the ∧ operation, then (x∘∘,a)∈Φ and (x∨dL,e)∈Φ imply (x,a∧e)=(x∘∘∧(x∨dL),a∧e)∈Φ. Also (a,z∘∘)∈θ and (e,z∨dL)∈θ imply (a∧e,z)=(a∧e,z∘∘∧(z∨dL))∈θ. Consequently, we deduce that (x,a∧e)∈Φ and (a∧e,z)∈θ,a∧e∈L. Then (x,z)∈Φ∘θ, and hence θ,Φ are 2-permutable. □
Now, we construct two examples to clarify the above theorem.
Example 4.1. Consider the principal MS-algebra L in Example 2.1 (Figure 1). From Table 1, we show that L has 2-permutable congruences. Also, Tables 2 and 3 show that L∘∘andD(L) have 2-permutable congruences, respectively. Where αL∘∘=βL∘∘ and δL∘∘=△L∘∘.
In the following example, we give a principal MS-algebra L which has not 2-permutable congruences as well as D(L) has not also 2-permutable congruences.
Example 4.2. Consider the principal MS-algebra L in Figure 5.
It is clear that D(L)={z,z1,1} and L∘∘={0,c,a,b,d,1}. Consider θ,Φ∈Con(L) as follows:
We observe that θ∘Φ≠Φ∘θ as (z,1)∈Φ∘θ but (z,1)∉θ∘Φ.
Then L has not 2-permutable congruences and D(L) has not 2-permutable congruences as θD(L)∘ΦD(L)≠ΦD(L)∘θD(L).
5.
n-permutability of principal MS-algebras
We generalize the concept of 2-permutability to the concept of n-permutability of congruences of principal MS-algebras.
Definition 5.1. A principal MS-algebra L is said to have n-permutable congrunces if every two congruences θ,Φ of L are n-permutable, that is,
At first, we need the following two lemmas.
Lemma 5.1. Let L be a principal MS- algebra with the smallest dense element dL. Let θ,Φ be two congruences on L. Then we have
(i) (θ∘Φ∘θ∘...)L∘∘=θL∘∘∘ΦL∘∘∘...(n times),
(ii) (θ∘Φ∘θ∘...)D(L)=θD(L)∘ΦD(L)∘...(n times).
Proof.
(i) Recall that θL∘∘,ΦL∘∘ are the restrictions of θ,Φ on L∘∘, respectively. Let a,b∈L∘∘ and (a,b)∈(θL∘∘∘ΦL∘∘∘...). Then there exist c1,c2,...,cn−1∈L∘∘ such that
Then
Then (a,b)∈(θ∘Φ∘θ∘...) and hence (a,b)∈(θ∘Φ∘θ∘...)L∘∘. Therefore
Conversely, let a,b∈L∘∘ such that (a,b)∈(θ∘Φ∘θ∘...)L∘∘. Then (a,b)∈(θ∘Φ∘θ∘...). Then there exist c1,c2,...,cn−1∈L such that
Then we get
Since c1∘∘,c2∘∘,...,cn−1∘∘∈L∘∘, we have
Therefore
and hence
Then we get
(ii) Take x,y∈D(L), let (x,y)∈(θD(L)∘ΦD(L)∘ΦD(L)∘θD(L)∘...). Then (x,y)∈(θ∘Φ∘θ∘...) and hence (a,b)∈(θ∘Φ∘θ∘...)D(L). Then (θ∘Φ∘θ∘...)⊆(θ∘Φ∘θ∘...)D(L).
Conversely, let (x,y)∈(θ∘Φ∘θ∘...)D(L), then (x,y)∈(θ∘Φ∘...). There exist d1,d2,...dn−1∈L, such that
Since x,y≥dL, we get,
Hence (x,y)∈(θD(L)∘ΦD(L)∘θD(L)∘...). Then the required equality is proved. □
Lemma 5.2. Let θ and Φ be congruences on a principal MS-algebra L. Let ψ denoted to the relation θ∘Φ∘θ∘...(n times). Then (a,b)∈ψ and (c,d)∈ψ imply (a∧c,b∧d)∈ψ.
Proof. Let (a,b)∈ψ and (c,d)∈ψ. Then there exist a1,a2,...,an−1,c1,c2,...,cn−1∈L such that
And
Since θ,Φ are congruence on L then we get,
Thus (a∧c,b∧d)∈ψ. □
Now, a characterization of n-permutability of principal MS-algebras is given.
Theorem 5.1. Two congruences θ,Φ on a principal MS-algebra L are n-permutable if and only if their restrictions θL∘∘,ΦL∘∘ and θD(L),ΦD(L) with respect to L∘∘ and D(L) respectively are n-permutable.
Proof. Let L has n-permutable congruences. Let θ,Φ be any two congruences on L. Then by (i) and (ii) of Lemma 5.1, respectively, we have
and
Therefore L∘∘ and D(L) have n-permutable congruences.
Conversely, let L∘∘ and D(L) have n-permutable congruences. If (a,b)∈(θ∘Φ∘θ∘...), using Theorem 2.3, we get
and
By lemma 5.1, we get
and
Since θL∘∘,ΦL∘∘ and θD(L),ΦD(L) are n-permutable on L∘∘,D(L), respectively, we have
and
It follows that, (a∘∘,b∘∘)∈(Φ∘θ∘Φ∘...) and (a∨dL,b∨dL)∈(Φ∘θ∘Φ∘...). Since L is a principal MS-algebra, then a=a∘∘∧(a∨dL) and b=b∘∘∧(b∨dL). By Lemma 5.2, we get
Thus (θ∘Φ∘θ∘...)⊆(Φ∘θ∘Φ∘...). Similarly we can show that (Φ∘θ∘Φ∘...)⊆(θ∘Φ∘θ∘...). Then L has n-permutable congruences. □
6.
Conclusions
With the aid of the technique of MS-congruence pairs, many properties were investigated for principal MS-algebras, deal with a characterization of congruence pairs of a principal MS-algebra (a Stone algebra), a description of the lattice A(L) of all MS-congruence pairs of L via Boolean elements of L, characterizations of 2-permutability and n-permutability of congruences of a principal MS-algebra. This work leads us in the future to study many aspects of principal MS-algebras and related structures, for instance, it can be applied to triple construction of principal MS-algebra, affine and locally complete of principal MS-algebras.
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Conflict of interest
The authors declare no conflict of interest.









 DownLoad:
DownLoad:







