We present a multi-step spectral collocation method to solve Caputo-type fractional integro-differential equations (FIDEs) involving weakly singular kernels. We reformulate the problem as the second type Volterra integral equation (VIE) with two different weakly singular kernels. Based on these integral equations, we construct a multi-step Legendre-Gauss spectral collocation scheme for the problem. The hp-version convergence is established rigorously. To demonstrate the effectiveness of the suggested method and the validity of the theoretical results, the results of some numerical experiments are presented.
Citation: Chuanli Wang, Biyun Chen. An hp-version spectral collocation method for fractional Volterra integro-differential equations with weakly singular kernels[J]. AIMS Mathematics, 2023, 8(8): 19816-19841. doi: 10.3934/math.20231010
| [1] | Obaid Algahtani, M. A. Abdelkawy, António M. Lopes . A pseudo-spectral scheme for variable order fractional stochastic Volterra integro-differential equations. AIMS Mathematics, 2022, 7(8): 15453-15470. doi: 10.3934/math.2022846 |
| [2] | A. H. Tedjani, A. Z. Amin, Abdel-Haleem Abdel-Aty, M. A. Abdelkawy, Mona Mahmoud . Legendre spectral collocation method for solving nonlinear fractional Fredholm integro-differential equations with convergence analysis. AIMS Mathematics, 2024, 9(4): 7973-8000. doi: 10.3934/math.2024388 |
| [3] | Dumitru Baleanu, Babak Shiri . Generalized fractional differential equations for past dynamic. AIMS Mathematics, 2022, 7(8): 14394-14418. doi: 10.3934/math.2022793 |
| [4] | Xiaojun Zhou, Yue Dai . A spectral collocation method for the coupled system of nonlinear fractional differential equations. AIMS Mathematics, 2022, 7(4): 5670-5689. doi: 10.3934/math.2022314 |
| [5] | Mohammed A. Almalahi, Satish K. Panchal, Fahd Jarad, Mohammed S. Abdo, Kamal Shah, Thabet Abdeljawad . Qualitative analysis of a fuzzy Volterra-Fredholm integrodifferential equation with an Atangana-Baleanu fractional derivative. AIMS Mathematics, 2022, 7(9): 15994-16016. doi: 10.3934/math.2022876 |
| [6] | Imran Talib, Md. Nur Alam, Dumitru Baleanu, Danish Zaidi, Ammarah Marriyam . A new integral operational matrix with applications to multi-order fractional differential equations. AIMS Mathematics, 2021, 6(8): 8742-8771. doi: 10.3934/math.2021508 |
| [7] | Ahmed M. Rajab, Saeed Pishbin, Javad Shokri . Analyzing the structure of solutions for weakly singular integro-differential equations with partial derivatives. AIMS Mathematics, 2024, 9(9): 23182-23196. doi: 10.3934/math.20241127 |
| [8] | Zahra Pirouzeh, Mohammad Hadi Noori Skandari, Kamele Nassiri Pirbazari, Stanford Shateyi . A pseudo-spectral approach for optimal control problems of variable-order fractional integro-differential equations. AIMS Mathematics, 2024, 9(9): 23692-23710. doi: 10.3934/math.20241151 |
| [9] | Khadijeh Sadri, David Amilo, Kamyar Hosseini, Evren Hinçal, Aly R. Seadawy . A tau-Gegenbauer spectral approach for systems of fractional integro-differential equations with the error analysis. AIMS Mathematics, 2024, 9(2): 3850-3880. doi: 10.3934/math.2024190 |
| [10] | Mahmoud A. Zaky, Weam G. Alharbi, Marwa M. Alzubaidi, R.T. Matoog . A Legendre tau approach for high-order pantograph Volterra-Fredholm integro-differential equations. AIMS Mathematics, 2025, 10(3): 7067-7085. doi: 10.3934/math.2025322 |
We present a multi-step spectral collocation method to solve Caputo-type fractional integro-differential equations (FIDEs) involving weakly singular kernels. We reformulate the problem as the second type Volterra integral equation (VIE) with two different weakly singular kernels. Based on these integral equations, we construct a multi-step Legendre-Gauss spectral collocation scheme for the problem. The hp-version convergence is established rigorously. To demonstrate the effectiveness of the suggested method and the validity of the theoretical results, the results of some numerical experiments are presented.
In this paper, we focus on numerical methods to solve FIDEs involving weakly singular kernels, i.e., equations of the form
| C0Dαxu(x)=g(x)+p(x)u(x)+∫x0(x−t)−βq(x,t)u(t)dt,0<x<T, | (1.1) |
| u(0)=u0, | (1.2) |
where 0<α,β<1, u0 is any real number, g(x) and p(x) are continuous and bounded functions on IT:=[0,T], q(x,t) is nonzero continuous function of x and t defined on
| △T:={(x,t)∈R2:0≤t≤x≤T}. |
The symbol C0Dαx denotes a Caputo-type fractional derivative defined by [3]
| C0Dαxϕ(x)=1Γ(n−α)∫x0ϕ(n)(s)(x−s)α−n+1ds,n−1<α<n, n∈N. |
Correspondingly,
| Dαxϕ(x)=dnϕ(x)dxn,α=n∈Z+. |
During the last few decades, fractional-order derivatives has been widely used to model physical phenomena in many different contexts (see [1,2,3,4,5]). It is usually very hard to find exact solutions of fractional order differential equations and much attention has been devoted to develop efficient approaches to approximately solve these problems. Khader et al. [6] developed a Chebyshev pseudo-spectral method based on a new formula of Caputo derivative to solve FIDEs systems numerically. In [7], Zhao et al. transformed FIDEs to Volterra integral equations with weakly singular kernels and presented a piecewise polynomials collocation method to solve the problem. Wang [8] presented an one-step spectral collocation method for nonlinear fractional boundary value problems. Dehestani et al. [9] investigated fractional Genocchi functions (FGFs) and their Pseudo-operational matrix, and construct the collocation method based on FGFs to find the numerical solution of the fractional partial integro-differential equations with variable-order. Sadri et al. [10] explored the two-variable Vieta-Fibonacci polynomials and their operational matrices to derive a collocation method for time-fractional telegraph equations.
Relevant results may be found in [11,12,13,14,15,16,17,18]. Most of of the approaches correspond to p-version methods, which are usually inefficient to deal with local weak singularities and always result in lower order of accuracy nearby the singular points.
Recently, Wang have obtained results using multi-step or hp-version spectral methods for Volterra integral equations (see [19,20,21,22]). Guo and Wang [23,24] investigated multi-step spectral collocation methods based on classical shifted Jacobi polynomials for single order and multi-order fractional differential equations. Yao et al. [25] developed a hybrid spectral element method to solve VIEs with vanishing delays, using different kinds of meshes and different orthogonal functions as basis to obtain higher accuracy. Based on these results we further explore hp-version spectral collocation approaches for FIDEs (1.1) and (1.2). Our main results may be summarized as follows:
(ⅰ) The original problems (1.1) and (1.2) are recasted as a VIE with two different singular kernels, and an hp-version Legendre spectral collocation method is proposed. The time step h and polynomial degree M in the scheme may be chosen arbitrarily, according to the requirements of accuracy or storage. The explicit form and the iteration method make the new scheme highly convenient.
(ⅱ) The priori error estimate for the new method is derived rigorously for 12<α<1. The convergence characteristic of our method is verified numerically on the basis of several typical examples, which fits the theoretical results well. The numerical comparison illustrates that our hp-version spectral collocation approaches also suits well to the case 12<α<1, and allows one to achieve higher accuracy than other piecewise polynomial collocation methods.
The article is structured as follows: Section 2 is for preliminaries. In Section 3, the FIDEs is transformed into Volterra integral equation and its piece-wised form. Then, a multi-step spectral collocation scheme based on the reformulated problem is constructed. In Section 4, the hp-version convergence of the proposed method is analyzed in the L2 function space. In Section 5, we present the results of some numerical experiments to illustrated the suitability of this method. Finally, some concluding remarks were presented at the end of the paper.
To make a preparation for the later work, some basic definitions and properties of the shifted Legendre polynomials shall be introduced at first. We make an arbitrary partition on the given interval I=[0,1], which can be denoted by
| Ih:={xn:0=x0<x1<⋯<xN=1}. |
We set In=(xn−1,xn], hn=xn−xn−1.
Lk(t), t∈(−1,1) denotes the standard Legendre polynomial of the degree k. The shifted form of Legendre polynomial with degree k over In is defined by [22]
| Ln,k(x)=Lk(2x−xn−1−xnhn),x∈In, k≥0. |
We denote the set of polynomials of degree not greater than Mn on the sub-interval In by PMn(In). Let {tn,j,ωn,j}Mnj=0 be the nodes and the corresponding quadrature weights for the standard Legendre-Gauss interpolation over (−1,1), and xn,j be the nodes for the shifted form of Legendre-Gauss quadrature on the sub-interval In,
| xn,j=12(hntn,j+xn−1+xn),0≤j≤Mn. |
It has been known that [22]
| ∫InLn,k(x)Ln,j(x)dx=hn2k+1δk,j, | (2.1) |
where δk,j is the Kronecker function. Furthermore, it can be verified that [21]
| ∫Inϕ(x)dx=hn2Mn∑j=0ϕ(xn,j)ωn,j,∀ϕ∈P2Mn+1(In), | (2.2) |
and
| Mn∑i=0Ln,k(xn,i)Ln,j(xn,i)ωn,i=22k+1δk,j,∀0≤k+j≤2Mn+1. | (2.3) |
Let Ix,Mn:C(In)→PMn(In) denote the shifted Legendre-Gauss interpolation operator implemented on the n-th sub-interval In, such that
| Ix,Mnv(xn,i)=v(xn,i),i=0,1,⋯,Mn. | (2.4) |
For later uses, we transfer the integral interval (xn−1,x] to In via the following transformation:
| ξ=ξ(λ,x):=xn−1+(λ−xn−1)(x−xn−1)hn,ξ∈(xn−1,x], λ∈In. | (2.5) |
Then, we introduce another interpolation operator
| I(xn−1,x]ξ,Mn:C(xn−1,x)→PMn(xn−1,x), |
defined by
| I(xn−1,x]ξ,Mnv(ξn,i)=v(ξn,i),i=0,1,⋯,Mn, |
where ξn,i are the shifted Legendre-Gauss points on the interval (xn−1,x). Obviously,
| I(xn−1,x]ξ,Mnv(ξ)|ξ=ξ(λ,x)=Iλ,Mnv(ξ(λ,x)). |
Due to the presence of the weak singularity, we naturally introduce the interpolatory quadrature formula with weight depending on the weakly singular kernel (x−t)δ, which was mentioned in [26] and defined as follows: For any ϕ(t)∈PMk(Ik), one has
| ∫Ik(x−t)δϕ(t)dt=Mk∑q=0ϕ(xk,q)˜ωδk,q(x),x∈In, 1≤k≤n−1, | (2.6) |
where
| ˜ωδk,q(x)=∫Ik(x−t)δhk,q(t)dt |
and {hk,q(t)}Mkq=0 are Lagrange interpolation basis function corresponding to the quadrature points {xk,q}Mkq=0.
In this part, we first convert the original problem to VIE and its piecewise form equivalently. Then we shall present the multi-step Legendre-Gauss spectral collocation approach to solve numerically the reformulated problem (3.7).
To obtain the reformulation of the discussed problem, we recall the equation of the classical form
| C0Dαxu(x)=f(x,u(x)),α>0,u(i)(0)=ui0,i=0,1,⋯,n−1. | (3.1) |
The equivalence between (3.1) and the second kind VIEs is given by the following statements:
Lemma 1. Assume that α>0, n−1≤α<n, n∈N, f is a continuous function, then the Eq (3.1) is equvalent to the following Volterra Integral equation of the second kind:
| u(x)=n−1∑i=0ui0+1Γ(α)∫x0(x−t)α−1f(t,u(t))dt. | (3.2) |
Applying the above Lemma into the problems (1.1) and (1.2), we can deduce that
| u(x)=u0+1Γ(α)∫x0(x−t)α−1[g(t)+p(t)u(t)+∫t0(t−s)−βq(t,s)u(s)ds]dt=u0+1Γ(α)∫x0(x−t)α−1g(t)dt+1Γ(α)∫x0(x−t)α−1p(t)u(t)dt+1Γ(α)∫x0(x−t)α−1∫t0(t−s)−βq(t,s)u(s)dsdt. | (3.3) |
This is an equivalent VIE to the original problem. By exchanging the integration order of the last part, (3.3) can be expressed as follows:
| 1Γ(α)∫x0(x−t)α−1∫t0(t−s)−βq(t,s)u(s)dsdt=1Γ(α)∫x0(∫xs(x−t)α−1(t−s)−βq(t,s)dt)u(s)ds. | (3.4) |
By introducing variable substitution t=s+τ(x−s), the above formula can be transformed into
| 1Γ(α)∫x0(x−t)α−1∫t0(t−s)−βq(t,s)u(s)dsdt=1Γ(α)∫x0(x−s)α−β(∫10(1−τ)α−1τ−βq(s+τ(x−s),s)dτ)u(s)ds=1Γ(α)∫x0(x−t)α−βK(x,t)u(t)dt, | (3.5) |
where
| K(x,t)=∫10(1−τ)α−1τ−βq(t+τ(x−t),t)dτ. |
Since q(x,t) is bounded and continuous in △T, assuming that qmax is the maximum of q for (x,t)∈△T, and qmin is the minimum, correspondingly, then
| qminB(α,1−β)≤∫10(1−τ)α−1τ−βq(t+τ(x−t),t)dτ≤qmaxB(α,1−β), |
where B(⋅,⋅) is Beta function defined by
| B(a,b)=∫10sa−1(1−s)b−1ds. | (3.6) |
Thus K(x,t) is a bounded and continuous founction of x and t in △T.
As a result, Eqs (1.1) and (1.2) can be reformulated as the following equivalent form:
| u(x)=u0+1Γ(α)[∫x0(x−t)α−1g(t)dt+∫x0(x−t)α−1p(t)u(t)dt+∫x0(x−t)α−βK(x,t)u(t)dt]. | (3.7) |
According to the basic results of VIEs (Theorem 6.1.2 in [26]), the above equation possesses a unique solution u∈C(I). Equation (3.7) is different from the reformation in [7]. We have two different singular kernels which can be treated respectively. That means we can choose suitable collocation methods for each kernel for the sake of solving the problem more conveniently and efficiently.
Now, we aim to construct an efficient multi-step spectral collocation scheme for (1.1) and (1.2). We set T=1 for the sake of convenience and simplicity. Denote by un(x) the exact solution to (1.1) and (1.2) on the n-th sub-interval, that is,
| un(x)=u(x),x∈In, 1≤n≤N, |
where In is defined in Section 2. From (1.1) we know that for any x∈In
| un(x)=u0+1Γ(α)[n−1∑k=1∫Ik(x−t)α−1g(t)dt+∫xxn−1(x−t)α−1g(ξ)dξ+n−1∑k=1∫Ik(x−t)α−1p(t)uk(t)dt+∫xxn−1(x−ξ)α−1p(ξ)un(ξ)dξ+n−1∑k=1∫Ik(x−t)α−βK(x,t)uk(t)dt+∫xxn−1(x−ξ)α−βK(x,ξ)un(ξ)dξ]. | (3.8) |
Using the transformation in (2.5), the Eq (3.8) becomes
| un(x)=u0+1Γ(α)[n−1∑k=1∫Ik(x−t)α−1g(t)dt+n−1∑k=1∫Ik(x−t)α−1p(t)uk(t)dt+n−1∑k=1∫Ik(x−t)α−βK(x,t)uk(t)dt+(x−xn−1hn)α∫In(xn−λ)α−1g(ξ(λ,x))dλ+(x−xn−1hn)α∫In(xn−λ)α−1p(ξ(λ,x))un(ξ(λ,x))dλ+(x−xn−1hn)α−β+1∫In(xn−λ)α−βK(x,ξ(λ,x))un(ξ(λ,x))dλ]. | (3.9) |
The multi-step spectral collocation method to solve (3.7) based on Legendre-Gauss points is to seek Un(x)∈PMn(In), such that
| Un(x)=u0+1Γ(α)Ix,Mn[n−1∑k=1∫Ik(x−t)α−1It,Mkg(t)dt+n−1∑k=1∫Ik(x−t)α−1It,Mkp(t)Uk(t)dt+n−1∑k=1∫Ik(x−t)α−βIt,MkK(x,t)Uk(t)dt+(x−xn−1hn)α∫In(xn−λ)α−1Iλ,Mng(ξ(λ,x))dλ+(x−xn−1hn)α∫In(xn−λ)α−1Iλ,Mnp(ξ(λ,x))Un(ξ(λ,x))dλ+(x−xn−1hn)α−β+1∫In(xn−λ)α−βIλ,MnK(x,ξ(λ,x))Un(ξ(λ,x))dλ], | (3.10) |
where Uk(x) is the approximate solution of uk(x) on the element Ik. To describe the numerical implementations in details for scheme (3.10), we set
| Un(x)=Mn∑p=0ZnpLn,p(x),Ix,Mn˜ωα−1k,q(x)=Mn∑p=0ˆωk,α−1p,qLn,p(x),Ix,MnK(x,xα−βk,q)˜ωα−βk,q(x)=Mn∑p=0ˆvk,α−βp,qLn,p(x),Ix,MnIλ,Mn((x−xn−1)αp(ξ(λ,x))Un(ξ(λ,x)))=Mn∑p=0Mn∑q=0Bnp,qLn,p(x)Ln,q(λ),Ix,MnIλ,Mn((x−xn−1)α−β+1K(x,ξ(λ,x))Un(ξ(λ,x)))=Mn∑p=0Mn∑q=0Dnp,qLn,p(x)Ln,q(λ),Ix,MnIλ,Mn(x−xn−1)αg(ξ(λ,x))=Mn∑p=0Mn∑q=0Gnp,qLn,p(x)Ln,q(λ). | (3.11) |
Then by applying (2.4), (2.6) and (3.11), one has
| Ix,Mnn−1∑k=1∫Ik(x−t)α−1It,Mk(p(t)Uk(t))dt=n−1∑k=1Ix,MnMk∑q=0(p(xk,q)Uk(xk,q))˜ωα−1k,q(x)=n−1∑k=1Mk∑q=0Mn∑p=0p(xk,q)Uk(xk,q)ˆωk,α−1p,qLn,p(x)=Mn∑p=0n−1∑k=1AkpLn,p(x). | (3.12) |
Using similar techniques, we can obtain
| Ix,Mnn−1∑k=1∫Ik(x−t)α−βIt,Mk(K(x,t)Uk(t))dt=n−1∑k=1Mk∑q=0Uk(xk,q)Ix,MnK(x,xk,q)˜ωα−βk,q(x)=Mn∑p=0n−1∑k=1Mk∑q=0Uk(xk,q)ˆvk,α−βp,qLn,p(x)=Mn∑p=0n−1∑k=1CkpLn,p(x) | (3.13) |
and
| Ix,Mnn−1∑k=1∫Ik(x−t)α−1It,Mkg(t)dt=n−1∑k=1Ix,MnMk∑q=0(g(xk,q))˜ωα−1k,q(x)=n−1∑k=1Mk∑q=0Mn∑p=0g(xk,q)ˆωk,α−1p,qLn,p(x)=Mn∑p=0(n−1∑k=1Mk∑q=0g(xk,q)ˆωk,α−1p,q)Ln,p(x)=Mn∑p=0n−1∑k=1EkpLn,p(x). | (3.14) |
Using (2.2)–(2.4) and (3.11), we have
| Ix,Mn(x−xn−1hn)α∫In(xn−λ)α−1Iλ,Mnp(ξ(λ,x))Un(ξ(λ,x))dλ=1hαnMn∑p=0Mn∑q=0Bnp,q∫In(xn−λ)α−1Ln,q(λ)dλLn,p(x)=Mn∑p=0¯BnpLn,p(x) | (3.15) |
and
| Ix,Mn(x−xn−1hn)α−β+1∫In(xn−λ)α−βIλ,MnK(x,ξ(λ,x))Un(ξ(λ,x))dλ=1hα−β+1nMn∑p=0Mn∑q=0Dnp,qLn,p(x)∫In(xn−λ)α−βLn,q(λ)dλ=Mn∑p=0¯DnpLn,p(x). | (3.16) |
In addition
| Ix,Mn(x−xn−1hn)α∫In(xn−λ)α−1Iλ,Mng(ξ(λ,x))dλ=1hαnMn∑p=0Mn∑q=0Gnp,qLn,p(x)∫In(xn−λ)α−1Ln,q(λ)dλ=Mn∑p=0¯GnpLn,p(x). | (3.17) |
One can easily verify that
| ˆωk,α−1p,q=2p+12Mn∑i=0˜ωα−1k,q(xn,i)Ln,p(xn,i)ωn,i,ˆvk,α−βp,q=2p+12Mn∑i=0K(xn,i,xk,q)˜ωα−βk,q(xn,i)Ln,p(xn,i)ωn,i,Akp=Mk∑q=0p(xk,q)Uk(xk,q)ˆωk,α−1p,q,Ckp=Mk∑q=0Uk(xk,q)ˆvk,α−βp,q,Ekp=Mk∑q=0g(xk,q)ˆωk,α−1p,q,Bnp,q=(2p+1)(2q+1)4Mn∑i=0Mn∑j=0(xn,i−xn−1)αp(ξ(xn,j,xn,i))×Un(ξ(xn,j,xn,i))Ln,p(xn,i)Ln,q(xn,j)ωn,iωn,j,Dnp,q=(2p+1)(2q+1)4Mn∑i=0Mn∑j=0(xn,i−xn−1)α−β+1K(xn,i,ξ(xn,j,xn,i))×Un(ξ(xn,j,xn,i))Ln,p(xn,i)Ln,q(xn,j)ωn,iωn,j,ˉBnp=12αMn∑q=0Bnp,qMn∑j=0Ln,q(xα−1n,j)ωα−1n,j,ˉDnp=12α−β+1Mn∑q=0Dnp,qMn∑j=0Ln,q(xα−βn,j)ωα−βn,j,Gnp,q=(2p+1)(2q+1)4Mn∑i=0Mn∑j=0(xn,i−xn−1)αg(ξ(xn,j,xn,i))Ln,p(xn,i)Ln,q(xn,j)ωn,iωn,j,ˉGnp=12αMn∑q=0Gnp,qMn∑j=0Ln,q(xα−1n,j)ωα−1n,j. | (3.18) |
Next, using (3.10)–(3.17), we deduce that
| Mn∑p=0ZnpLn,p(x)=u0+1Γ(α)Mn∑p=0[n−1∑k=1(Akp+Ckp+Ekp)+¯Bnp++¯Dnp+¯Gnp]Ln,p(x). | (3.19) |
Comparing the expansion coefficients on the two sides of (3.19), yields
| Znp=1Γ(α)[n−1∑k=1(Akp+Ckp+Ekp)+¯Bnp+¯Dnp+¯Gnp],p=1,2,⋯,Mn,Znp=u0+1Γ(α)[n−1∑k=1(Akp+Ckp+Ekp)+¯Bnp+¯Dnp+¯Gnp],p=0. | (3.20) |
The system (3.20) is obviously implicit, which always couldn't be easily solved by a direct method. Actually, we can employ an iterative method to solve out the expansion coefficients Znp. Here, we prefer to using the successive substitution method for its simplicity and convenience. In short, we compute the successive coefficients {Znp}Mnp=0 based on the previously obtained quantities {Zkp}Mkp=0,k=1,⋯,n−1.
In this part, some useful results are introduced, which are fundamental for the convergence analysis.
For any given interval Λ, we denote by L2(Λ) the space of measurable functions whose square is Lebesgue integrable on Λ. Let Hm(Λ) be the standard Sobolev space with the integer order m.
Lemma 1. ([22]) Assume that v∈Hm(In) is any given function with 1≤m≤Mn+1, there holds
| ‖v−Ix,Mnv‖2L2(In)≤ch2mnM−2mn‖∂mxv‖2L2(In). |
Lemma 2. ([22]) Let the assumption in Lemma 1 holds. Then we have
| ‖v−I(xn−1,x]ξ,Mnv‖2L2(xn−1,x)≤ch2mnM−2mn‖∂mtv‖2L2(In). |
In order to discuss the convergence of singular solutions, we next consider the error estimates for Legendre-Gauss interpolation of xν-type functions which have singularity at the endpoint x=0.
Lemma 3. ([21]) Let Mn=M≥0 and hn≈h. Suppose that u(x)=xν with a non-integer ν satisfying ν>0. Then for α>−1, M>ν−1,
| ‖u−Ix,Mu‖2L2(I1)≤ch2ν+11(M+1)−4ν−2. |
In addition, since xν only has a singular point at the left end of the first interval, we have
| n−1∑k=1‖u−Ix,Mu‖2L2(Ik)≤ch2ν+1(M+1)−4ν−2. |
Lemma 4. ([19]) Given that {rk}, {qk} and {ρk}(k≥0) are non-negative sequences. {en} is the sequence satisfying e0≤ρ0 and
| en≤ρn+n−1∑k=0qk+n−1∑k=0rkek,n≥1. |
Then there holds that
| en≤ρn+n−1∑k=0(qk+rkρk)exp(n−1∑k=0rk),n≥1. |
In this part, we shall analyze the error bounds in the L2(I) function space for smooth solutions. Thus, the term ‖Un−Ix,Mnun(x)‖L2(In) would be estimated first. According to (3.8), one has
| Ix,Mnun(x)=u0+1Γ(αIx,Mn[n−1∑k=1∫Ik(x−t)α−1g(t)dt+n−1∑k=1∫Ik(x−t)α−1p(t)uk(t)dt+n−1∑k=1∫Ik(x−t)α−βK(x,t)uk(t)dt+∫In(x−ξ)α−1g(ξ)dξ+∫In(x−ξ)α−1p(ξ)un(ξ)dλ+∫In(x−ξ)α−βK(x,ξ)un(ξ)dξ]. | (4.1) |
Subtracting (3.10) from (4.1) and using the inverse of transformation (2.5), we can obtain that
| Un(x)−Ix,Mnun(x)=1Γ(α)[B1+B2+B3+B4+B5], | (4.2) |
where
| B1=n−1∑k=1Ix,Mn∫Ik(x−t)α−1(It,Mkp(t)Uk(t)−p(t)uk(t))dt,B2=Ix,Mn∫xxn−1(x−ξ)α−1(I(xn−1,x]ξ,Mnp(ξ)Un(ξ)−p(ξ)un(ξ))dξ,B3=n−1∑k=1Ix,Mn∫Ik(x−t)α−β(It,MkK(x,t)Uk(t)−K(x,t)uk(t))dt,B4=Ix,Mn∫xxn−1(x−ξ)α−β(I(xn−1,x]ξ,MnK(x,ξ)Un(ξ)−K(x,ξ)un(ξ))dξ,B5=Ix,Mn(n−1∑k=1∫Ik(x−t)α−1(It,Mkg(t)−g(t))dt+∫xxn−1(x−ξ)α−1(I(xn−1,x]ξ,Mng(ξ)−g(ξ))dξ). | (4.3) |
We estimate {Bj}5j=1 one by one. For simplicity, we make the assumption (A) as follows:
● q(x,t) is continuous, for any given x∈I, q(x,t)|t∈Ik∈Hmk(Ik) with the integer 1≤mk≤Mk+1.
● g,p,u is continuous on I, g|t∈Ik, p|t∈Ik, uk∈Hmk(Ik) with the integer 1≤mk≤Mk+1.
It can be found that the first item of (A) implies the same result for K(x,t).
Lemma 5. Under the assumption (A). If α∈(12,1), then for any 1≤n≤N, we have
| ‖B1‖2L2(In)≤chnn−1∑k=1h2mkkM−2mkk(‖∂mkt(pu)‖2L2(Ik)+‖∂mktu‖L2(Ik))+chnn−1∑k=1‖U−u‖2L2(Ik). | (4.4) |
Proof. For the sake of convenience and simplification, we make the notation as follows:
| V(t)|t∈Ik:=It,Mkp(t)Uk(t)−p(t)uk(t)). |
Applying this formula and (2.2) to the first formula of (4.3), we have
| ‖B1‖2L2(In)=‖Ix,Mn∫xn−10(x−t)α−1(It,Mkp(t)Uk(t)−p(t)uk(t))dt‖2L2(In)=hn2Mn∑i=0wn,i(∫xn−10(xn,i−t)α−1V(t)dt)2. | (4.5) |
Using Cauchy-Schwarz inequality, we can easily get
| ‖B1‖2L2(In)≤hn2Mn∑i=0wn,i∫xn−10(xn,i−t)2(α−1)dt∫xn−10V2(t)dt. | (4.6) |
Since α∈(12,1) and ∑Mni=0ωn,i=2, we can deduce that
| ‖B1‖2L2(In)≤cT2α−1hn2n−1∑k=1∫Ik(It,Mkp(t)Uk(t)−p(t)uk(t)))2dt≤B11+B12, | (4.7) |
where
| B11=cT2α−1hnn−1∑k=1∫Ik(It,Mkp(t)(Uk(t)−uk(t)))2dt,B12=cT2α−1hnn−1∑k=1∫Ik((It,Mk−I)p(t)uk(t))2dt. | (4.8) |
Next, we shall estimate B1 by evaluating B11 and B12 one by one. Using (2.2) again, one has
| B11=chnn−1∑k=1∫Ik(It,Mkp(t)(Uk(t)−uk(t)))2dt=chnn−1∑k=1hk2Mk∑j=0(p(xk,j)(Uk(xk,j)−uk(xk,j)))2wk,j≤chnn−1∑k=1hk2Mk∑j=0((Uk(xk,j)−uk(xk,j)))2wk,j≤chnn−1∑k=1∫Ik(It,Mk(Uk(t)−uk(t)))2dt. | (4.9) |
Clearly, by triangle inequality, the above becomes
| B11≤chnn−1∑k=1∫Ik(Uk(t)−uk(t)))2dt+chnn−1∑k=1∫Ik((It,Mk−I)uk(t))2dt≤chnn−1∑k=1(‖U−u‖2L2(Ik)+‖(It,Mk−I)u‖2L2(Ik)). | (4.10) |
By Lemma 1, we get
| ‖(It,Mk−I)u‖2L2(Ik)≤h2mkkM−2mkk‖∂mktu‖L2(Ik),B12≤chnn−1∑k=1h2mkkM−2mkk‖∂mkt(pu)‖L2(Ik). | (4.11) |
Combining (4.7), (4.10) with (4.11), the desired estimate follows.
Lemma 6. Under the assumption (A). If α∈(12,1), then there holds that
| ‖B2‖2L2(In)≤ch2αn‖U−u‖2L2(In)+ch2mn+2αnM−2mnn(‖∂mnxu‖2L2(In)+‖∂mnx(pu)‖2L2(In)). |
Proof. Using the Triangle Inequality to the second formula of (4.3), one has
| ‖B2‖2L2(In)≤chnMn∑i=0wn,i(∫xn,ixn−1(xn,i−ξ)α−1(I(xn−1,xn,i]ξ,Mnp(ξ)Un(ξ)−p(ξ)un(ξ))dξ)2≤B21+B22, | (4.12) |
where
| B21=chnMn∑i=0wn,i(∫xn,ixn−1(xn,i−ξ)α−1I(xn−1,xn,i]ξ,Mnp(ξ)(Un(ξ)−un(ξ))dξ)2,B22=chnMn∑i=0wn,i(∫xn,ixn−1(xn,i−ξ)α−1(I(xn−1,xn,i]ξ,Mn−I)p(ξ)un(ξ))dξ)2. | (4.13) |
Since α∈(12,1), according to Cauchy-Schwarz inequality and (2.2), we obtain that
| B21≤chnMn∑i=0wn,i∫xn,ixn−1(xn,i−ξ)2α−2dξ⋅∫xn,ixn−1(I(xn−1,xn,i]ξ,Mnp(ξ)(Un(ξ)−un(ξ))2dξ≤ch2αnMn∑i=0wn,i∫xn,ixn−1(I(xn−1,xn,i]ξ,Mnp(ξ)(Un(ξ)−un(ξ)))2dξ≤ch2αnMn∑i=0wn,ixn,i−xn−12Mn∑j=0(p(ξn,j)(Un(ξn,j)−un(ξn,j)))2wn,j≤ch2αnMn∑i=0wn,ixn,i−xn−12Mn∑j=0((Un(ξn,j)−un(ξn,j)))2wn,j≤ch2αnMn∑i=0wn,i∫xn,ixn−1(I(xn−1,xn,i]ξ,Mn(Un(ξ)−un(ξ)))2dξ≤ch2αnMn∑i=0wn,i[∫xn,ixn−1(Un(ξ)−un(ξ))2dξ+∫xn,ixn−1((I(xn−1,xn,i]ξ,Mn−I)un(ξ))2dξ]. | (4.14) |
From Lemma 2, we deduce that
| B21≤ch2αn‖U−u‖2L2(In)+ch2mn+2αnM−2mnn‖∂mnxu‖2L2(In). | (4.15) |
Furthermore, applying the Cauchy-Schwarz inequality, we get
| ‖B22‖2L2(In)≤chnMn∑i=0wn,i∫xn,ixn−1(xn,i−ξ)2α−2dξ∫xn,ixn−1((I(xn−1,xn,i]ξ,Mn−I)p(ξ)un(ξ)))2dξ≤ch2αnMn∑i=0wn,i∫xn,ixn−1((I(xn−1,xn,i]ξ,Mn−I)p(ξ)un(ξ))2dξ≤ch2mn+2αnM−2mnn‖∂mnξ(pu)‖2L2(In). | (4.16) |
Thus, by (4.15) and 4.16), the desired result is obtained.
Lemma 7. Under the assumption (A), suppose that α∈(12,1), 0<β<1, then we have
| ‖B3‖2L2(In)≤chnn−1∑k=1h2mkkM−2mkk(‖∂mkt(Ku)‖2L∞(In,L2(Ik)+‖∂mntu‖L2(Ik))+chnn−1∑k=1‖U−u‖2L2(Ik). | (4.17) |
Proof. We define
| W(x,t)|:=It,MkK(x,t)Uk(t)−K(x,t)uk(t)),x∈In, t∈Ik. |
Thus
| ‖B3‖2L2(In)=‖Ix,Mn∫xn−10(x−t)α−β∫xn−10(x−t)α−βW(x,t)‖2L2(In)=hn2Mn∑i=0wn,i(∫xn−10(xn,i−t)α−βW(x,t)dt)2. | (4.18) |
Since α∈(12,1) and 0<β<1, we easily deduce that −1<2(α−β)<2. Using Cauchy-Schwarz Inequality, we get
| ‖B3‖2L2(In)≤hn2Mn∑i=0wn,i∫xn−10(xn,i−t)2(α−β)dt∫xn−10W2(xn,i,t)dt≤cT2α−2β+1hn2Mn∑i=0wn,in−1∑k=1∫Ik(It,MkK(xn,i,t)Uk(t)−K(xn,i,t)uk(t)))2dt≤B31+B32, | (4.19) |
where
| B31=chnMn∑i=0wn,in−1∑k=1∫Ik(It,MkK(xn,i,t)(Uk(t)−uk(t)))2dt,B32=chnMn∑i=0wn,in−1∑k=1∫Ik((It,Mk−I)K(xn,i,t)uk(t))2dt. | (4.20) |
Using (2.2) and triangle inequality again, we get
| B31=chnMn∑i=0wn,in−1∑k=1hk2Mk∑j=0(K(xn,i,xk,j)(Uk(xk,j)−uk(xk,j)))2wk,j≤chnMn∑i=0wn,imax | (4.21) |
By Lemma 1, we get
| \begin{equation} \begin{split} B_{32}&\leq ch_n \sum\limits_{i = 1}^{M_n}w_{n,i} \sum\limits_{k = 1}^{n-1}h_k^{2m_k}M_k^{-2m_k}\|\partial^{m_k}_t\big(K(x_{n,i},\cdot) u\big)\|_{L^2(I_k)} \\& \leq ch_n \sum\limits_{k = 1}^{n-1}h_k^{2m_k}M_k^{-2m_k}\max\limits_{x\in I_n}\|\partial^{m_k}_t\big(K(x,\cdot) u\big)\|_{L^2(I_k)}. \end{split} \end{equation} | (4.22) |
Plugging (4.21) and (4.22) into (4.19), we complete the proof.
Lemma 8. Under the assumption \mathcal{(A)} , suppose that \alpha \in \big(\frac{1}{2}, 1\big) , 0 < \beta < 1 , then we have
| \begin{equation} \begin{split} \|B_4\|^2_{L^2(I_n)}\leq ch_n^{2m_n+2\alpha-\beta+2}M_n^{-2m_n}\Big(\|\partial^{m_n}_{\xi}u\|^2_{L^2(I_{n})}+\|\partial^{m_n}_{\xi}(K u)\|^2_{L^{\infty}(I_{n},L^2(I_n))}\Big) +ch_n^{2\alpha-2\beta+2}\|U-u\|^2_{L^2(I_n)}. \end{split} \end{equation} | (4.23) |
Proof. Since -1 < 2\alpha-2\beta < 2 , using Cauchy-Schwarz inequality and (2.2), we obtain
| \begin{equation} \begin{split} \|B_4\|^2_{L^2(I_{n})} &\leq ch_n \sum\limits_{i = 0}^{M_n}w_{n,i}\Big( \int^{x_{n,i}}_{x_{n-1}}(x_{n,i}-\xi)^{\alpha-\beta}(\mathcal{I}^{(x_{n-1},x_{n,i}]}_{\xi,M_n}K(x_{n,i},\xi)U_n(\xi)-K(x_{n,i},\xi)u_n(\xi))d\xi\Big)^2 \\& \leq ch_n^{2\alpha-2\beta+2} \sum\limits_{i = 0}^{M_n}w_{n,i} \int^{x_{n,i}}_{x_{n-1}}(\mathcal{I}^{(x_{n-1},x_{n,i}]}_{\xi,M_n}K(x_{n,i},\xi)U_n(\xi)-K(x_{n,i},\xi)u_n(\xi))d\xi \\& \leq ch_n^{2\alpha-2\beta+2}(B_{41}+B_{42}), \end{split} \end{equation} | (4.24) |
where
| \begin{equation} \begin{split} &B_{41} = c \sum\limits_{i = 0}^{M_n}w_{n,i} \int^{x_{n,i}}_{x_{n-1}}\Big(\mathcal{I}^{(x_{n-1},x_{n,i}]}_{\xi,M_n}K(x_{n,i},\xi)(U_n(\xi)-u_n(\xi))\Big)^2d\xi, \\&B_{42} = c \sum\limits_{i = 0}^{M_n}w_{n,i} \int^{x_{n,i}}_{x_{n-1}}\Big(\mathcal{I}^{(x_{n-1},x_{n,i}]}_{\xi,M_n}-\mathcal{I})K(x_{n,i},\xi)u_n(\xi))\Big)^2d\xi. \end{split} \end{equation} | (4.25) |
By computing directly, we obtain
| \begin{equation} \begin{split} B_{41}&\leq c \sum\limits_{i = 0}^{M_n}w_{n,i} \int^{x_{n,i}}_{x_{n-1}}\Big(\mathcal{I}^{(x_{n-1},x_{n,i}]}_{\xi,M_n}K(x_{n,i},\xi_{n,j})(U_n(\xi)-u_n(\xi))\Big)^2d\xi \\&\leq c \sum\limits_{i = 0}^{M_n}w_{n,i} \frac{x_{n,i}-x_{n-1}}{2} \sum\limits_{j = 0}^{M_n}\Big(K(x_{n,i},\xi_{n,j})(U_n(\xi_{n,j})-u_n(\xi_{n,j}))\Big)^2w_{n,j} \\& \leq c \sum\limits_{i = 0}^{M_n}w_{n,i} \frac{x_{n,i}-x_{n-1}}{2} \sum\limits_{j = 0}^{M_n}\Big((U_n(\xi_{n,j})-u_n(\xi_{n,j}))\Big)^2w_{n,j} \\&\leq c \sum\limits_{i = 0}^{M_n}w_{n,i}\Big[ \int^{x_{n,i}}_{x_{n-1}}(U_n(\xi)-u_n(\xi))^2d\xi+ \int^{x_{n,i}}_{x_{n-1}}\Big((\mathcal{I}^{(x_{n-1},x_{n,i}]}_{\xi,M_n}-\mathcal{I})u_n(\xi)\Big)^2d\xi\Big] \\& \leq c\|U-u\|^2_{L^2(I_{n})}+ch_n^{2m_n}M_n^{-2m_n}\|\partial^{m_n}_xu\|^2_{L^2(I_{n})}. \end{split} \end{equation} | (4.26) |
Furthermore, applying the Cauchy-Schwarz inequality, we get
| \begin{equation} \begin{split} \|B_{42}\|^2_{L^2(I_{n})}&\leq c \sum\limits_{i = 0}^{M_n}w_{n,i} \int^{x_{n,i}}_{x_{n-1}}\Big((\mathcal{I}^{(x_{n-1},x_{n,i}]}_{\xi,M_n}-\mathcal{I})K(x_{n,i},\xi_{n,j})u_n(\xi))\Big)^2d\xi \\& \leq c \sum\limits_{i = 0}^{M_n}w_{n,i} \int^{x_{n,i}}_{x_{n-1}}\Big((\mathcal{I}^{(x_{n-1},x_{n,i}]}_{\xi,M_n}-\mathcal{I})K(x_{n,i},\xi)u_n(\xi)\Big)^2d\xi \\& \leq ch_n^{2m_n}M_n^{-2m_n}\max\limits_{x\in I_n}\|\partial^{m_n}_{\xi}K(x,\cdot) u\|^2_{L^2(I_{n})} \sum\limits_{i = 0}^{M_n}w_{n,i} \leq ch_n^{2m_n}M_n^{-2m_n}\|\partial^{m_n}_{\xi}(K u)\|^2_{L^{\infty}(I_n,L^2(I_{n})}. \end{split} \end{equation} | (4.27) |
Thus, by (4.24), (4.26) and (4.27), the desired result is obtained.
Using the same skills as above, we deduce the following estimates:
Lemma 9. Under the assumption \mathcal{(A)} , suppose that \alpha \in \big(\frac{1}{2}, 1\big) , 0 < \beta < 1 , there holds
| \begin{equation} \begin{split} \|B_5\|^2_{L^2(I_n)}&\leq c h_n \sum\limits_{k = 1}^{n-1}h_k^{2m_k}M_k^{-2m_k}\|\partial_t^{m _k}g\|^2_{L^2(I_k)}+ch_n^{2m_n+2\alpha}M_n^{-2m_n}\|\partial^{m_n}_tg\|_{L^2(I_n)}. \end{split} \end{equation} | (4.28) |
Combining the above estimates for \{B_j\}^5_{j = 1} , we obtain local convergence as follows:
Theorem 1. Under the assumption \mathcal{(A)} , suppose that \alpha \in \big(\frac{1}{2}, 1\big) , 0 < \beta < 1 , then for any 1\leq n \leq N , we have
| \begin{equation} \begin{split} \|U_n-u_n\|^2_{L^2(I_{n})}&\leq c h_n \exp(cT) \sum\limits_{k = 1}^{n-1}h_k^{2m_k}M_k^{-2m_k}\Big(\|\partial_t^{m_k} (p\cdot u)\|^2_{L^2(I_k)}\\&+\|\partial^m_tu\|_{L^2(I_k)} +\|\partial_t^{m_k} (K(x,\cdot)u)\|^2_{L^{\infty}(I_n,L^2(I_k)} +\|\partial_t^{m_k} g\|^2_{L^2(I_k)}\Big) \\& +ch_n^{2m_n}M_n^{-2m_n}\Big(\|\partial^{m_n}_{\xi}u\|^2_{L^2(I_{n})}+\|\partial^{m_n}_{\xi}(p u)\|^2_{L^2(I_{n})}+\|\partial^{m_n}_{\xi}g\|_{L^2(I_n)}\Big). \end{split} \end{equation} | (4.29) |
Proof. Obviously, it follows from the triangle inequality that
| \begin{equation} \begin{split} \|U_n-u_n\|^2_{L^2(I_{n})} \leq 2\|U_n-\mathcal{I}_{x,M_n}u_n\|^2_{L^2(I_{n})}+ 2\|\mathcal{I}_{x,M_n}u_n-u_n\|^2_{L^2(I_{n})}.\\ \end{split} \end{equation} | (4.30) |
According to Lemma 1, one has
| \begin{equation} \|\mathcal{I}_{x,M_n}u_n-u_n\|^2_{L^2(I_{n})}\leq ch_n^{2m_n}M_n^{-2m_n}\|\partial^{m_n}_x u\|_{L^2(I_n)}. \end{equation} | (4.31) |
The above formula along with (4.2), Lemmas 5–9 lead to
| \begin{equation} \begin{split} \|U_n-u_n\|^2_{L^2(I_{n})}&\leq c h_n \sum\limits_{k = 1}^{n-1}h_k^{2m_k}M_k^{-2m_k}\Big(\|\partial_t^{m_k} (pu)\|^2_{L^2(I_k)}+\|\partial^{m_k}_tu\|_{L^2(I_k)} \\&+\|\partial_t^{m_k} (K(x,\cdot)u)\|^2_{L^{\infty}(I_n,L^2(I_k)} +\|\partial_t^{m_k} g\|^2_{L^2(I_k)}\Big) \\& +ch_n^{2m_n}M_n^{-2m_n}\Big(\|\partial^{m_n}_{\xi}u\|^2_{L^2(I_{n})}+\|\partial^{m_n}_{\xi}(pu)\|^2_{L^2(I_{n})}+\|\partial^{m_n}_{\xi}g\|_{L^2(I_n)}\Big) \\&+ch_n \sum\limits_{k = 1}^{n-1}\big\|U-u\big\|^2_{L^2(I_k)} +c\big(h_n^{2\alpha}+h_n^{2\alpha-2\beta+2}\big)\|U-u\|^2_{L^2(I_n)}. \end{split} \end{equation} | (4.32) |
Assume that \alpha \in \big(\frac{1}{2}, 1\big) , 0 < \beta < 1 and h_n is small enough, such that
| c\big(h_n^{2\alpha}+h_n^{2\alpha-2\beta+2}\big) < 1. |
Then we can obtain from (4.32) that
| \begin{equation} \begin{split} \|U_n-u_n\|^2_{L^2(I_{n})}&\leq ch_n \sum\limits_{k = 1}^{n-1}\big\|U-u\big\|^2_{L^2(I_k)}+c h_n \sum\limits_{k = 1}^{n-1}h_k^{2m_k}M_k^{-2m_k}\Big(\|\partial_t^{m_k} (p u)\|^2_{L^2(I_k)}\\&+\|\partial^{m_k}_tu\|_{L^2(I_k)} +\|\partial_t^{m_k} (K(x,\cdot)u)\|^2_{L^{\infty}(I_n,L^2(I_k)} +\|\partial_t^{m_k} g\|^2_{L^2(I_k)}\Big) \\& +ch_n^{2m_n}M_n^{-2m_n}\Big(\|\partial^{m_n}_{\xi}u\|^2_{L^2(I_{n})}+\|\partial^{m_n}_{\xi}(pu)\|^2_{L^2(I_{n})}+\|\partial^{m_n}_{\xi}g\|_{L^2(I_n)}\Big). \end{split} \end{equation} | (4.33) |
Using the Lemma 4, we can obtain that
| \begin{equation} \begin{split} \|U_n-u_n\|^2_{L^2(I_{n})}&\leq c h_n \exp(cT) \sum\limits_{k = 1}^{n-1}h_k^{2m_k}M_k^{-2m_k}\Big(\|\partial_t^{m_k} (p u)\|^2_{L^2(I_k)}\\&+\|\partial^{m_k}_tu\|_{L^2(I_k)} +\|\partial_t^{m_k} (K(x,\cdot)u)\|^2_{L^{\infty}(I_n,L^2(I_k)}+\|\partial_t^{m_k} g\|^2_{L^2(I_k)}\Big) \\&+ch_n^{2m_n}M_n^{-2m_n}\Big(\|\partial^{m_n}_{\xi}u\|^2_{L^2(I_{n})}+\|\partial^{m_n}_{\xi}(p u)\|^2_{L^2(I_{n})}+\|\partial^{m_n}_{\xi}g\|_{L^2(I_n)}\Big). \end{split} \end{equation} | (4.34) |
This is the desired result.
Summing (4.29) from n = 1 to N , we get the following L^2 -norm error estimate on the entire interval I .
Theorem 2. Under the assumption \mathcal{(A)} , if \alpha \in \big(\frac{1}{2}, 1\big) , 0 < \beta < 1 , then we have
| \begin{equation} \begin{split} \|U-u\|^2_{L^2(I)}&\leq c \exp(cT) \sum\limits_{k = 1}^Nh_k^{2m_K}M_k^{-2m_K}\Big(\|\partial_t^{m_K} (p u)\|^2_{L^2(I_k)}+\|\partial^{m_K}_tu\|_{L^2(I_k)} \\& +\|\partial_t^{m_K} g\|^2_{L^2(I_k)} +\|\partial_t^{m_K} (K(x,\cdot)u)\|^2_{L^{\infty}(I_n,L^2(I_k)}\Big). \end{split} \end{equation} | (4.35) |
Remark 1. Theorem 2 established the hp -version error bounds for sufficiently smooth solutions. However, for 0 < \alpha < 1 in the Volterra integral Eq (3.7), smooth functions p, q, g result in the weakly singular solution u(x) which typically has the form of u(x) = O(x^{\alpha}) near x = 0 (see [26]). Thus, applying the Lemma 3 and executing the same procedure as above, we can obtain the following results:
| \|U-u\|_{L^2(I)}\leq ch^{\alpha+\frac{1}{2}}M^{-2\alpha-1}, |
on the uniform meshes ( h_n = h ) and uniform approximation degree ( M_n = M ).
Remark 2. For the sake of getting higher order methods to solve the problems with weakly singular kernels, some special partitions (such as graded meshes or geometric meshes) are always considered. Here we implemented the proposed method on the graded meshes of the form x_n = \big(\frac{i}{N}\big)^rT . Thus M order convergence for h can be obtained under the condition r = (M+1)/\alpha , where M is the degree of interpolation polynomials on I_n and M+1 is the number of collocation points.
To demonstrate the effectiveness of the proposed method and the validity of the theoretical results derived in the previous sections, here we present some numerical experiments.
Example 1. We first consider the following FIDE with sufficiently smooth solution:
| \begin{equation} \left\{\begin{split} &{}_0^CD_x^{2/3}u(x) = g(x)+p(x)u(x)+ \int_0^x(x-t)^{-\frac{5}{6}}q(x,t)u(t)dt, \quad x\in(0,1),\\ & u(0) = 0, \end{split}\right. \end{equation} | (5.1) |
where
| g(x) = \frac{\Gamma(22/3)}{\Gamma(20/3)}x^{17/3}-x^{19/3}e^{-x}-B(1/6,25/3)x^{51/6},\quad p(x) = e^{-x}, \ q(x,t) = xt. |
The solution of this problem can be written as u(x) = x^{19/3} , which is sufficiently smooth on the interval [0, 1]. We can transform this problem into the equivalent form:
| \begin{equation} u(x) = u(0)+ \frac{1}{\Gamma(2/3)}\Big[ \int_0^x(x-t)^{-1/3}g(t)dt+ \int_0^x(x-t)^{-1/3}p(x)u(t)dt + \int_0^x(x-t)^{-1/6}K(x,t)u(t)dt\Big], \end{equation} | (5.2) |
where
| K(x,t) = B(5/3,1/6)t^2+B(2/3,7/6)xt. |
In Figure 1, we show the discrete L^2 -errors for decreasing h and fixed polynomial degree M = 1, 2, 3, 4. These approximately diagonal lines indicate that the h -version convergence is algebraic in h . In Table 1, the discrete L^2 -norm errors in h -version and the order of convergence are summarized. Results are agreement with the theoretical results of Theorem 2.
| h | M | L^2{\rm -error} | \rm order | M | L^2{\rm -error} | \rm order | M | L^2{\rm -error} | \rm order |
| 1/64 | 9.89E-05 | 2.00 | 6.35E-07 | 3.00 | 2.42E-09 | 4.00 | |||
| 1/128 | 2.47E-05 | 2.00 | 7.94E-08 | 3.00 | 1.52E-10 | 4.00 | |||
| 1/256 | 1 | 6.18E-06 | 2.00 | 2 | 9.92E-09 | 3.00 | 3 | 9.47E-12 | 4.00 |
| 1/512 | 1.54E-06 | 2.00 | 1.24E-09 | 3.00 | 5.92E-13 | 4.00 | |||
| 1/1024 | 3.86E-07 | 2.00 | 1.55E-10 | 3.00 | 3.70E-14 | 4.00 |
In Figure 2, we show the discrete L^2 -errors of the p -version with increasing polynomial degree M at fixed time step, h = 1, 1/2, 1/4, 1/8, respectively. We see that exponential convergence of p -version is achieved, which confirms the results of Theorem 2.
Example 2. We use the proposed method to solve the following problem with weakly singular solution:
| \begin{equation} \left\{\begin{split} &{}_0^CD_x^{2/3}u(x) = g(x)+p(x)u(x)+ \int_0^x(x-t)^{-\frac{1}{2}}q(x,t)u(t)dt, \quad x\in(0,1),\\ & u(0) = 0, \end{split}\right. \end{equation} | (5.3) |
where
| \begin{equation} \begin{split} &g(x) = \Gamma(5/3)-e^{-x}x^{2/3}-B(1/2,5/3)x^{7/6}, \\&p(x) = e^{-x},\ q(x,t) = 1. \end{split} \end{equation} | (5.4) |
The above equation has the exact solution u(x) = x^{\frac{2}{3}}, which has a weak singularity at the left endpoint x = 0.
In Figure 3, we present the discrete L^2 -errors for various h and fixed polynomial degree M = 1, 2, 3, 4 , respectively. They indicates that the h -version convergence of the proposed method is algebraic for decreasing h . In Table 2, we list the discrete L^2 -errors of the h -version and the convergence order. The convergence order is about 1.17 , which corresponds to the theoretical results about h -version predicted in Remark 1 (the h -version convergence rate is \mathcal{O}(h^{\alpha+\frac{1}{2}} ) for singular solutions).
| h | M | L^2{\rm -error} | \rm order | M | L^2{\rm -error} | \rm order | M | L^2{\rm -error} | \rm order |
| 1/64 | 2.79E-04 | 1.19 | 1.04E-04 | 1.18 | 5.38E-05 | 1.18 | |||
| 1/128 | 1.23E-04 | 1.18 | 4.62E-05 | 1.17 | 2.39E-05 | 1.17 | |||
| 1/256 | 1 | 5.47E-05 | 1.17 | 2 | 2.05E-05 | 1.17 | 3 | 1.06E-05 | 1.17 |
| 1/512 | 2.43E-05 | 1.17 | 9.12E-06 | 1.17 | 4.72E-06 | 1.17 | |||
| 1/1024 | 1.08E-05 | 1.17 | 4.06E-06 | 1.17 | 2.10E-06 | 1.17 |
In Figure 4, the discrete L^2 -errors in the p -version for Example 2 are shown. The mode M is increasing for each fixed time step h = 1, 1/2, 1/4, 1/8 . This means that the p -version convergence for different h is algebraic and the convergence orders are similar since the solution is weakly singular.
In Table 3, p -version error bounds and convergence order are presented in details. The convergence order for the L^2 -errors is about 2.3, which coincides with the p -version results predicted in Remark 1 ( p -version convergence rate is \mathcal{O}(M^{-2\alpha-1}) ) and is twice as fast as the h -version results.
| M | h | L^2{\rm -error} | \rm order | h | L^2{\rm -error} | \rm order |
| 6 | 2.56E-03 | 2.36 | 9.84E-04 | 2.13 | ||
| 7 | 1.79E-03 | 2.32 | 7.07E-04 | 2.15 | ||
| 8 | 1 | 1.31E-03 | 2.33 | 1/2 | 5.28E-04 | 2.18 |
| 9 | 9.98E-04 | 2.33 | 4.07E-04 | 2.21 | ||
| 10 | 7.79E-04 | 2.36 | 3.22E-04 | 2.24 |
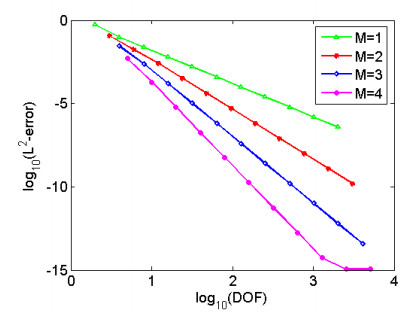
To obtain higher convergence order and improve the effectiveness of our method, we make use of graded meshes x_i = (i/N)^r for i = 0, 1, \cdots, N with r = (M+1)/\alpha , where the spectral collocation method uses shifted Legendre polynomials of degree M . The value M = 1, 2, 3 are examined in our experiments. In Figure 5, we plot the discrete L^2 -errors against the degrees of freedom. In Table 4, the discrete L^2 -errors in h -version and the corresponding convergence order are listed in details, for increasing grid points number N for each fixed mode M = 1, 2, 3, 4 , respectively. Clearly, M+1 -order convergence is achieved, as predicted in Remark 2.
| N | M | L^2{\rm -error} | \rm order | M | L^2{\rm -error} | \rm order | M | L^2{\rm -error} | \rm order |
| 64 | 1.82E-05 | 2.00 | 3.24E-07 | 3.00 | 1.06E-08 | 4.00 | |||
| 128 | 4.55E-06 | 2.00 | 4.06E-08 | 3.00 | 6.62E-10 | 4.00 | |||
| 256 | 1 | 1.14E-06 | 2.00 | 2 | 5.07E-09 | 3.00 | 3 | 4.14E-11 | 4.00 |
| 512 | 2.84E-07 | 2.00 | 6.34E-10 | 3.00 | 2.59E-12 | 4.00 | |||
| 1024 | 7.11E-08 | 2.00 | 7.92E-11 | 3.00 | 1.62E-13 | 4.00 |
Example 3. We apply the proposed method to solve the following problem with weakly singular solution in [7]:
| \begin{equation} \left\{\begin{split} &{}_0^CD_x^{1/3}u(x) = g(x)+p(x)u(x)+ \int_0^x(x-t)^{-\frac{1}{2}}q(x,t)u(t)dt, \quad x\in(0,1),\\ & u(0) = 0, \end{split}\right. \end{equation} | (5.5) |
where
| \begin{equation} \begin{split} &g(x) = \frac{6x^{8/3}}{\Gamma(11/3)}+\Big( \frac{32}{35}- \frac{\Gamma(1/2)\Gamma(7/3)}{\Gamma(17/6)}\Big)x^{11/6}+\Gamma(7/3)x, \\&p(x) = - \frac{32}{35}x^{1/2}, \qquad q(x,t) = 1. \end{split} \end{equation} | (5.6) |
The exact solution is u(x) = x^3+x^{\frac{4}{3}}, which has weak singularity at the left endpoint x = 0.
Figures 6 and 7 show that the discrete L^2 -error in both h and p -version converges algebraically as they behaved in Example 2, when the problem is solved on the uniform meshes. In Figure 8, one can find that the discrete L^2 -errors on graded meshes converge approximately exponentially. We compare the maximum errors on uniform meshes of our method and the h -version collocation method (45) in [7]. Table 5 indicates that the accuracy of our method are higher than those of the method (45) in [7]. Moreover, the time step h and polynomial degree M in our hp -version spectral collocation method may be chosen arbitrarily, according to the demands of users. Thus hp -version spectral collocation method is much more convenient and flexible to be implemented.
| N | M | [7] | our method | M | [7] | our method |
| 16 | 7.81E-05 | 5.00E-06 | 3.98E-05 | 1.45E-06 | ||
| 32 | 2 | 1.53E-05 | 1.12E-06 | 3 | 8.05E-06 | 3.20E-07 |
| 64 | 2.99E-06 | 2.50E-07 | 1.62E-06 | 7.11E-08 | ||
| 128 | 5.89E-07 | 5.55E-08 | 3.23E-07 | 1.58E-08 |
In this paper, we have addressesed an hp -version spectral collocation method for FIDEs (1.1) and (1.2) with singular kernels. We have converted the problem into a piece-wised Volterra integral equations and then presented a multi-step Legendre-Gauss spectral collocation scheme for the problem. The L^2 -norm error bounds have been established for \alpha \in \big(\frac{1}{2}, 1\big) . Numerical experiments has demonstrated the effectiveness of the suggested approach and the validity of the theoretical results. As a matter of fact, theoretical and numerical results expected to hold for \alpha \in \big(0, 1). In the further research, we will analyze the convergence for \alpha \in \big(0, 1\big) and the design of corresponding numerical methods for efficiently solve nonlinear FIDEs.
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
This research was funded by the National Natural Science Foundation of China (Grant No. 11901509) and the Natural Science Foundation of the Jiangsu Higher Education Institutions of China (Grant No. 19KJB110023). The authors are thankful to the referees for their constructive suggestions which helped to improve the presentation of this paper.
The authors declare no conflicts of interest.
| [1] | F. Mainardi, Fractional calculus and waves in linear viscoleasticity, Word Scientific, 2010. http://doi.org/10.1142/p614 |
| [2] | R. Hilfer, Applications of fractional calculus in physics, World Scientific, 1999. https://doi.org/10.1142/3779 |
| [3] | A. A. Kilbas, H. M. Srivastava, J. J. Trujillo, Theory and applications of fractional differential equations, Elsevier, 2006. https://doi.org/10.1016/s0304-0208(06)x8001-5 |
| [4] | I. Podlubny, Fractional differential equations, Academic Process, 1999. |
| [5] | K. Diethelm, The analysis of fractional differential equations, Springer, 2010. https://doi.org/10.1007/978-3-642-14574-2 |
| [6] |
M. M. Khader, N. H. Sweilam, On the approximate solutions for system of fractional integro-differential equations using Chebyshev pseudo-spectral method, Appl. Math. Modell., 37 (2013), 9819–9828. https://doi.org/10.1016/j.apm.2013.06.010 doi: 10.1016/j.apm.2013.06.010

|
| [7] |
J. Zhao, J. Xiao, N. J. Ford, Collocation methods for fractional integro-differential equations with weakly singular kernels, Numer. Algorithms, 65 (2014), 723–743. https://doi.org/10.1007/s11075-013-9710-2 doi: 10.1007/s11075-013-9710-2

|
| [8] |
C. Wang, Z. Wang, L. Wang, A spectral collocation method for nonlinear fractional boundary value problems with a Caputo derivative, J. Sci. Comput., 76 (2018), 166–188. https://doi.org/10.1007/s10915-017-0616-3 doi: 10.1007/s10915-017-0616-3

|
| [9] |
H. Dehestani, Y. Ordokhani, M. Razzaghi, Pseudo-operational matrix method for the solution of variable-order fractional partial integro-differential equations, Eng. Comput., 37 (2020), 1791–1806. https://doi.org/10.1007/s00366-019-00912-z doi: 10.1007/s00366-019-00912-z

|
| [10] |
K. Sadri, K. Hosseini, D. Baleanu, S. Salahshour, A high-accuracy Vieta-Fibonacci collocation scheme to solve linear time-fractional telegraph equations, Waves Random Complex Media, https://doi.org/10.1080/17455030.2022.2135789 doi: 10.1080/17455030.2022.2135789

|
| [11] |
K. Sadri, K. Hosseini, E. Hincal, D. Baleanu, S. Salahshour, A pseudo-operational collocation method for variable-order time-space fractional KdV-Burgers-Kuramoto equation, Math. Meth. Appl. Sci., 46 (2023), 8759–8778. https://doi.org/10.1002/mma.9015 doi: 10.1002/mma.9015

|
| [12] |
H. Dehestania, Y. Ordokhania, M. Razzaghib, Numerical solution of Variable-order time fractional weakly singular partial integro-difffferential equations with error estimation, Math. Modell. Anal., 25 (2020), 680–701. https://doi.org/10.3846/mma.2020.11692 doi: 10.3846/mma.2020.11692

|
| [13] |
H. Cai, A fractional spectral collocation for solving second kind nonlinear Volterra integral equations with weakly singular kernels, J. Sci. Comput., 80 (2019), 1529–1548. https://doi.org/10.1007/s10915-019-00987-2 doi: 10.1007/s10915-019-00987-2

|
| [14] |
S. Chen, J. Shen, L. L. Wang, Generalized Jacobi functions and their applications to fractional differential equations, Math. Comput., 85 (2016), 1603–1638. https://doi.org/10.1090/mcom3035 doi: 10.1090/mcom3035

|
| [15] |
X. Li, C. Xu, A space-time spectral method for the time fractional diffusion equation, SIAM J. Numer. Anal., 47 (2009), 2108–2131. https://doi.org/10.1137/080718942 doi: 10.1137/080718942

|
| [16] |
S. Esmaeili, M. Shamsi, A pseudo-spectral scheme for the approximate solution of a family of fractional differential equations, Commun. Nonlinear Sci. Numer. Simul., 16 (2011), 3646–3654. https://doi.org/10.1016/j.cnsns.2010.12.008 doi: 10.1016/j.cnsns.2010.12.008

|
| [17] |
P. Mokhtary, F. Ghoreishi, The L^2-convergence of the Legendre spectral tau matrix formulation for nonlinear fractional integro-differential equations, Numer. Algorithms, 58 (2011), 475–496. https://doi.org/10.1007/s11075-011-9465-6 doi: 10.1007/s11075-011-9465-6

|
| [18] |
C. Li, F. Zeng, F. Liu, Spectral approximations to the fractional integral and derivative, Fract. Calculus Appl. Anal., 15 (2012), 383–406. https://doi.org/10.2478/s13540-012-0028-x doi: 10.2478/s13540-012-0028-x

|
| [19] |
C. T. Sheng, Z. Q. Wang, B. Y. Guo, A multistep Legendre-Gauss spectral collocation method for nonlinear Volterra integral equations, SIAM J. Numer. Anal., 52 (2014), 1953–1980. https://doi.org/10.1137/130915200 doi: 10.1137/130915200

|
| [20] |
C. L. Wang, Z. Q. Wang, H. L. Jia, An hp-version spectral collocation method for nonlinear Volterra integro-differential equation with weakly singular kernels, J. Sci. Comput., 72 (2017), 647–678. https://doi.org/10.1007/s10915-017-0373-3 doi: 10.1007/s10915-017-0373-3

|
| [21] |
Z. Wang, Y. Guo, L. Yi, An hp-version Legendre-Jacobi spectral collocation method for Volterra integro-differential equations with smooth and weakly singular kernels, Math. Comput., 86 (2017), 2285–2324. https://doi.org/10.1090/mcom/3183 doi: 10.1090/mcom/3183

|
| [22] |
Z. Wang, C. Sheng, An hp-spectral collocation method for nonlinear Volterra integral equations with vanishing variable delays, Math. Comput., 85 (2016), 635–666. https://doi.org/10.1090/mcom/3023 doi: 10.1090/mcom/3023

|
| [23] |
Y. Guo, Z. Wang, An hp-version Chebyshev collocation method for nonlinear fractional differential equations, Appl. Numer. Math., 158 (2020), 194–211. https://doi.org/10.1016/j.apnum.2020.08.003 doi: 10.1016/j.apnum.2020.08.003

|
| [24] |
Y. Guo, Z. Wang, An hp-version Legendre spectral collocation method for multi-order fractional differential equations, Adv. Comput. Math., 47 (2021), 37. https://doi.org/10.1007/s10444-021-09858-7 doi: 10.1007/s10444-021-09858-7

|
| [25] |
G. Yao, D. Tao, C. Zhang, A hybrid spectal method for the nonlinear Volterra integral equations with weakly singular kernel and vanishing delays, Appl. Math. Comput., 417 (2022), 126780. https://doi.org/10.1016/j.amc.2021.126780 doi: 10.1016/j.amc.2021.126780

|
| [26] | H. Brunner, Collocation methods for Volterra integral and related functional equations, Cambridge University Press, 2004. https://doi.org/10.1017/cbo9780511543234 |
| 1. | Li-Bin Liu, Limin Ye, Xiaobing Bao, Yong Zhang, A second order numerical method for a Volterra integro-differential equation with a weakly singular kernel, 2024, 19, 1556-1801, 740, 10.3934/nhm.2024033 | |
| 2. | Babak Azarnavid, A kernel-based method for fractional integro-differential equations with a weakly singular kernel in multi-dimensional complex domains, 2024, 159, 09557997, 1, 10.1016/j.enganabound.2023.11.015 | |
| 3. | M. Fardi, B. Azarnavid, S. Mohammadi, Numerical simulation of nonlinear fractional integro-differential equations on two-dimensional regular and irregular domains: RBF partition of unity, 2025, 181, 08981221, 21, 10.1016/j.camwa.2024.12.019 |
| h | M | L^2{\rm -error} | \rm order | M | L^2{\rm -error} | \rm order | M | L^2{\rm -error} | \rm order |
| 1/64 | 9.89E-05 | 2.00 | 6.35E-07 | 3.00 | 2.42E-09 | 4.00 | |||
| 1/128 | 2.47E-05 | 2.00 | 7.94E-08 | 3.00 | 1.52E-10 | 4.00 | |||
| 1/256 | 1 | 6.18E-06 | 2.00 | 2 | 9.92E-09 | 3.00 | 3 | 9.47E-12 | 4.00 |
| 1/512 | 1.54E-06 | 2.00 | 1.24E-09 | 3.00 | 5.92E-13 | 4.00 | |||
| 1/1024 | 3.86E-07 | 2.00 | 1.55E-10 | 3.00 | 3.70E-14 | 4.00 |
| h | M | L^2{\rm -error} | \rm order | M | L^2{\rm -error} | \rm order | M | L^2{\rm -error} | \rm order |
| 1/64 | 2.79E-04 | 1.19 | 1.04E-04 | 1.18 | 5.38E-05 | 1.18 | |||
| 1/128 | 1.23E-04 | 1.18 | 4.62E-05 | 1.17 | 2.39E-05 | 1.17 | |||
| 1/256 | 1 | 5.47E-05 | 1.17 | 2 | 2.05E-05 | 1.17 | 3 | 1.06E-05 | 1.17 |
| 1/512 | 2.43E-05 | 1.17 | 9.12E-06 | 1.17 | 4.72E-06 | 1.17 | |||
| 1/1024 | 1.08E-05 | 1.17 | 4.06E-06 | 1.17 | 2.10E-06 | 1.17 |
| M | h | L^2{\rm -error} | \rm order | h | L^2{\rm -error} | \rm order |
| 6 | 2.56E-03 | 2.36 | 9.84E-04 | 2.13 | ||
| 7 | 1.79E-03 | 2.32 | 7.07E-04 | 2.15 | ||
| 8 | 1 | 1.31E-03 | 2.33 | 1/2 | 5.28E-04 | 2.18 |
| 9 | 9.98E-04 | 2.33 | 4.07E-04 | 2.21 | ||
| 10 | 7.79E-04 | 2.36 | 3.22E-04 | 2.24 |
| N | M | L^2{\rm -error} | \rm order | M | L^2{\rm -error} | \rm order | M | L^2{\rm -error} | \rm order |
| 64 | 1.82E-05 | 2.00 | 3.24E-07 | 3.00 | 1.06E-08 | 4.00 | |||
| 128 | 4.55E-06 | 2.00 | 4.06E-08 | 3.00 | 6.62E-10 | 4.00 | |||
| 256 | 1 | 1.14E-06 | 2.00 | 2 | 5.07E-09 | 3.00 | 3 | 4.14E-11 | 4.00 |
| 512 | 2.84E-07 | 2.00 | 6.34E-10 | 3.00 | 2.59E-12 | 4.00 | |||
| 1024 | 7.11E-08 | 2.00 | 7.92E-11 | 3.00 | 1.62E-13 | 4.00 |
| h | M | L^2{\rm -error} | \rm order | M | L^2{\rm -error} | \rm order | M | L^2{\rm -error} | \rm order |
| 1/64 | 9.89E-05 | 2.00 | 6.35E-07 | 3.00 | 2.42E-09 | 4.00 | |||
| 1/128 | 2.47E-05 | 2.00 | 7.94E-08 | 3.00 | 1.52E-10 | 4.00 | |||
| 1/256 | 1 | 6.18E-06 | 2.00 | 2 | 9.92E-09 | 3.00 | 3 | 9.47E-12 | 4.00 |
| 1/512 | 1.54E-06 | 2.00 | 1.24E-09 | 3.00 | 5.92E-13 | 4.00 | |||
| 1/1024 | 3.86E-07 | 2.00 | 1.55E-10 | 3.00 | 3.70E-14 | 4.00 |
| h | M | L^2{\rm -error} | \rm order | M | L^2{\rm -error} | \rm order | M | L^2{\rm -error} | \rm order |
| 1/64 | 2.79E-04 | 1.19 | 1.04E-04 | 1.18 | 5.38E-05 | 1.18 | |||
| 1/128 | 1.23E-04 | 1.18 | 4.62E-05 | 1.17 | 2.39E-05 | 1.17 | |||
| 1/256 | 1 | 5.47E-05 | 1.17 | 2 | 2.05E-05 | 1.17 | 3 | 1.06E-05 | 1.17 |
| 1/512 | 2.43E-05 | 1.17 | 9.12E-06 | 1.17 | 4.72E-06 | 1.17 | |||
| 1/1024 | 1.08E-05 | 1.17 | 4.06E-06 | 1.17 | 2.10E-06 | 1.17 |
| M | h | L^2{\rm -error} | \rm order | h | L^2{\rm -error} | \rm order |
| 6 | 2.56E-03 | 2.36 | 9.84E-04 | 2.13 | ||
| 7 | 1.79E-03 | 2.32 | 7.07E-04 | 2.15 | ||
| 8 | 1 | 1.31E-03 | 2.33 | 1/2 | 5.28E-04 | 2.18 |
| 9 | 9.98E-04 | 2.33 | 4.07E-04 | 2.21 | ||
| 10 | 7.79E-04 | 2.36 | 3.22E-04 | 2.24 |
| N | M | L^2{\rm -error} | \rm order | M | L^2{\rm -error} | \rm order | M | L^2{\rm -error} | \rm order |
| 64 | 1.82E-05 | 2.00 | 3.24E-07 | 3.00 | 1.06E-08 | 4.00 | |||
| 128 | 4.55E-06 | 2.00 | 4.06E-08 | 3.00 | 6.62E-10 | 4.00 | |||
| 256 | 1 | 1.14E-06 | 2.00 | 2 | 5.07E-09 | 3.00 | 3 | 4.14E-11 | 4.00 |
| 512 | 2.84E-07 | 2.00 | 6.34E-10 | 3.00 | 2.59E-12 | 4.00 | |||
| 1024 | 7.11E-08 | 2.00 | 7.92E-11 | 3.00 | 1.62E-13 | 4.00 |
| N | M | [7] | our method | M | [7] | our method |
| 16 | 7.81E-05 | 5.00E-06 | 3.98E-05 | 1.45E-06 | ||
| 32 | 2 | 1.53E-05 | 1.12E-06 | 3 | 8.05E-06 | 3.20E-07 |
| 64 | 2.99E-06 | 2.50E-07 | 1.62E-06 | 7.11E-08 | ||
| 128 | 5.89E-07 | 5.55E-08 | 3.23E-07 | 1.58E-08 |