Pathless directed topology in connection to the circulation of blood in the heart of human body

1.   Introduction

General topology and graph theory are main subjects in discrete mathematics. Leonhard Euler, in 1736 ^[6], introduced the graph theory to give some solutions of problems in discrete mathematics. In the graph theory, the notion of topologizing the vertices set or the edges set can was introduced by many researchers. For example, in 2013, Amiri ^[2] used the special neighborhoods of a locally finite graph to construct a topology on its vertices set, called graphic topology, on the set $\mathcal{V}$ of vertices of simple graph $\mathcal{G}_{1} = (\mathcal{V}, \mathcal{E})$ by giving the subbasis family $S_{G} = \{A_{x}:x\in V\}$ such that $A_{x}$ is the set of all adjacent vertices of $x$. For the directed graphs, Abdu and Kiliciman ^[1], structured the topologies on the set of edges in directed graphs, called incompatible edge and compatible topologies. In 2019, Nianga and Canoy ^[13] used the Hop neighborhoods of a graph to structure a finite topological space, that is, they presented a way of structuring a topology on a graph via the hop neighborhoods of a graph. In ^[14], Nianga and Canoy described some topologies induced by the complements of simple undirected graphs. In 2019, Gamorez et al. ^[10], described topologies induced by the corona, edge corona and tensor product of two graphs. In 2020, Sari and Kopuzlu ^[17] structured some topologies by using simple undirected graphs on the set of vertices. In 2021, Anabel et al. ^[3] constructed topologies on a vertices set by using monophonic eccentric neighborhoods of the graphs.

Nianga and Canoy ^[13] studied graph structure in finite topological spaces by Hop neighbourhood. In nano topology, Othman ^[15] studied some new graph structures. Recently, Sari and Kopuzlu ^[17] generated topological spaces from simple undirected graphs and investigated several properties. Graph theoretical structures in Alexandroff topology can be found in ^[20]. Similar studies also can be obtained in rough set ^[16] theoretical settings. El Atik et al. ^[4] studied rough set based graph theoretical structures in neighbourhood systems. Moreover, one may refer to ^[8] for graph theoretical attribute reduction in terms of rough set theory. Moreover, several results were investigated in digraphs from the perspective of rough set theory in ^[7]. A directed graph ^[5,6], (shortly, dirgraph) $\mathcal{G}$ is a pair $(\mathcal{V}, \mathcal{E})$ of a non-empty vertices set $\mathcal{V}$ and a set $\mathcal{E}$ of directed edges $E_{x_{1}x_{2}}$ such that $x_{1}\in \mathcal{V}$ is called the initial vertex of a directed edge $E_{x_{1}x_{2}}$ and $x_{2}\in \mathcal{V}$ is called the terminal vertex of a directed edge $E_{x_{1}x_{2}}$. By $Ends(E_{x_{1}x_{2}})$ we mean the set $\{x_{1}, x_{2}\}$ of ends vertices of $E_{x_{1}x_{2}}$. The adjacent edges are distinct edges that have a common vertex. Two directed adjacent edges $E_{x_{1}x_{2}}$ and $E'_{x'_{1}x'_{2}}$ are said to have the different directed (or adjacent different directed edges) if $x_{1} = x'_{1}$ or $x_{2} = x'_{x}$. An alternate sequence of directed edge of the form $\{E^{1}_{x_{1}x_{2}}, E^{2}_{x_{2}x_{3}}, E^{3}_{x_{3}x_{4}}, ....\}$ is called directed path. For $x\in \mathcal{V}$, the directed edge $E_{xx}$ is called a loop. The parallel edges are directed edges which have the same started vertex and the same end vertex. The dirgraph which has no parallel edges or loops is called simple.

In this work we give the notion of pathless property of subsets of vertices set in directed graph to structure topology on vertices set. In Section 2 we structure pathless directed topological spaces of directed graphs and study Alexandroff property on this structure. In Section 3, we study relation between the relative topologies and pathless directed topological spaces of E-generated subdirected graphs with giving the relation of connectedness in graphs and in pathless directed topological spaces. In Section 4, we present the relation between isomorphically in graphs and homeomorphically pathless directed topological spaces.

2.   Pathless directed topological spaces

Let $\mathcal{G} = (\mathcal{V}, \mathcal{E})$ be a dirgraph. A set $\mathcal{H}\subseteq \mathcal{V}$ is called C-set in $\mathcal{V}$ if $|\mathcal{H}|\geq 2$ and for every $u\in \mathcal{H}$ there is at last one vertex $v\in \mathcal{H}$ such that there is directed edge between $u$ and $v$.

For any digraph $\mathcal{G} = (\mathcal{V}, \mathcal{E})$ and for any C-set $\mathcal{H}\subseteq \mathcal{V}$, we define $\mathcal{H}_{\mathcal{E}} = \{E_{x_{1}x_{2}}\in \mathcal{E}: x_{1}, x_{2}\in \mathcal{H}\}$. $|\mathcal{H}_{\mathcal{E}}|$ denotes the number of adjacent different directed edges in $\mathcal{E}(\mathcal{H})$. If $\mathcal{E}(\mathcal{H})$ is single then we consider $|\mathcal{H}_{\mathcal{E}}| = 1$. For any directed edge $E_{x_{1}x_{2}}\in \mathcal{E}$, $(E_{x_{1}x_{2}})_{\mathcal{E}}$ denotes the set of all adjacent edges with $E_{x_{1}x_{2}}$ in the different direction.

Definition 2.1. Let $\mathcal{G} = (\mathcal{V}, \mathcal{E})$ be a digraph. A C-set $\mathcal{H}$ in $\mathcal{V}$ is called pathless set in $\mathcal{G}$ if the following conditions hold:

$(1)$ $0 < |\mathcal{H}_{\mathcal{E}}| < 3$;

$(2)$ if $E\in \mathcal{H}_{\mathcal{E}}$ and $E'$ is adjacent different directed edge with $E$ then $E'\in \mathcal{H}_{\mathcal{E}}$.

The pathless directed topological space of $\mathcal{G}$ is a pair $(\mathcal{V}, T_{P\mathcal{G}})$, where $T_{P\mathcal{G}}$ is a topology on $\mathcal{V}$ induced by a subbasis $P_{\mathcal{G}}$, where $P_{\mathcal{G}}$ denotes the collection of all pathless sets in $\mathcal{G}$.

Remark 2.2. It is clear from the above definition that $1 < |\mathcal{H}| < 4$, for any pathless set $\mathcal{H}$ in $\mathcal{G}$.

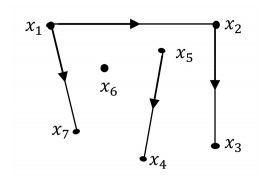
Example 2.3. In Figure 1, we consider a digraph $\mathcal{G} = (\mathcal{V}, \mathcal{E})$, where $\mathcal{V} = \{x_{1}, x_{2}, x_{3}, x_{4}, x_{5}, x_{6}, x_{7} \}$. We have $P_{\mathcal{G}} = \{\{x_{1}, x_{2}, x_{7}\}, \{x_{4}, x_{5}\}, \{x_{2}, x_{3}\}\}.$ Thus, the pathless directed topology $T_{P\mathcal{G}}$ is given below.

Example 2.4. For the digraph of Figure 2, the pathless directed topology $T_{P\mathcal{G}}$ is an indiscrete.

Theorem 2.5. Let $\mathcal{G} = (\mathcal{V}, \mathcal{E})$ be a digraph. Then, $Ends(E_{x_{1}x_{2}})$ is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$, for all $E_{x_{1}x_{2}}\in \mathcal{E}$ with $|(E_{x_{1}x_{2}})_{\mathcal{E}}| = 0$ or $|(E_{x_{1}x_{2}})_{\mathcal{E}}|\geq 2$.

Proof. If $|(E_{x_{1}x_{2}})_{\mathcal{E}}| = 0$, then it is clear by definition of $P_{\mathcal{G}}$ that $Ends(E_{x_{1}x_{2}})\in P_{\mathcal{G}}$. It means the set $Ends(E_{x_{1}x_{2}})$ is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$. Let $|(E_{x_{1}x_{2}})_{\mathcal{E}}|\geq 2$. Then, there is at least two directed edges $E^{1}_{y_{1}y_{2}}, E^{2}_{z_{1}z_{2}}\in (E_{x_{1}x_{2}})_{\mathcal{E}}$ such that one of the following cases occurs. They cases are as follow: (i) $x_{2} = y_{2} = z_{2}$ or (ii) $x_{1} = y_{1} = z_{1}$ or (iii) $z_{2} = x_{2}$ and $y_{1} = x_{1}$ or (iv) $y_{2} = x_{2}$ and $x_{1} = z_{1}$.

If $x_{2} = y_{2} = z_{2}$, then $\{y_{1}, x_{2} = y_{2} = z_{2}, x_{1}\}, \{z_{1}, x_{2} = y_{2} = z_{2}, x_{1}\}\in P_{\mathcal{G}},$ i.e.,

Similarly, we get $\{x_{1}, x_{2}\} = Ends(E_{x_{1}x_{2}})\in T_{P\mathcal{G}}$ for the other three cases. Thus, $Ends(E_{x_{1}x_{2}})$ is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$.

Corollary 2.6. Let $\mathcal{G} = (\mathcal{V}, \mathcal{E})$ be a digraph. If one of the following conditions holds.

$(1)$ $|(E_{xy_{1}})_{\mathcal{E}}| = 0$ and $|(E'_{xy_{2}})_{\mathcal{E}}| = 0$,

$(2)$ $|(E_{xy_{1}})_{\mathcal{E}}| = 0$ and $|(E'_{xy_{2}})_{\mathcal{E}}|\geq 2$,

$(3)$ $|(E_{xy_{1}})_{\mathcal{E}}|\geq 2$ and $|(E'_{xy_{2}})_{\mathcal{E}}| = 0$,

$(4)$ $|(E_{xy_{1}})_{\mathcal{E}}|\geq 2$ and $|(E'_{xy_{2}})_{\mathcal{E}}|\geq 2$,

for all $y_{1}\neq y_{2}, x \in \mathcal{V}$and $E_{xy_{1}}, E'_{xy_{2}}\in \mathcal{E}$, then $\{x\}$ is anopen set in $(\mathcal{V}, T_{P\mathcal{G}})$.

Proof. Using Theorem 2.5, we get that $Ends(E_{xy_{1}})$ and $Ends(E'_{xy_{2}})$ are open sets in $(\mathcal{V}, T_{P\mathcal{G}})$. Thus, $Ends(E_{xy_{1}})\cap Ends(E'_{xy_{2}}) = \{x\}$ is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$.

Theorem 2.7. Let $\mathcal{G} = (\mathcal{V}, \mathcal{E})$ be a digraph. Then, the pathless directed topological space $(\mathcal{V}, T_{P\mathcal{G}})$ of$\mathcal{G}$ is an Alexandroff space.

Proof. To prove that $(\mathcal{V}, T_{P\mathcal{G}})$ is an Alexandroff space, it is enough to prove that arbitrary intersection of elements of $P_{\mathcal{G}}$ is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$. Let $\{F_{\alpha}: \alpha\in I \}$ be the collection of elements of $P_{\mathcal{G}}$. Then, it clear by the definition of $P_{\mathcal{G}}$ that $|F_{\alpha}| = 2$ or $|F_{\alpha}| = 3$ for all $\alpha\in I$. Then, one of the following conditions holds: $\cap_{\alpha\in I }F_{\alpha} = \emptyset$ or $\cap_{\alpha\in I }F_{\alpha} = \{u, x\}$ or $\cap_{\alpha\in I }F_{\alpha} = \{u\}$, for some $u, x\in\mathcal{V}$. If $\cap_{\alpha\in I }F_{\alpha} = \emptyset$, then $\cap_{\alpha\in I }F_{\alpha}$ is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$. If $\cap_{\alpha\in I }F_{\alpha} = \{u, x\}$, for some $u, x\in\mathcal{V}$ then by Theorem 2.5, $\cap_{\alpha\in I }F_{\alpha}$ is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$. If $\cap_{\alpha\in I }F_{\alpha} = \{u\}$, for some $u\in\mathcal{V}$ then, one of the following three cases holds:

where $|F_{1}| = |F_{2}| = 3$ or $|F_{1}| = 2$ and $|F_{2}| = 3$ or $|F_{1}| = 2$ and $|F_{2}| = 2.$

Case 1: If $|F_{1}| = |F_{2}| = 3$, then $F_{1}, F_{2}\in P_{\mathcal{G}}$. Hence, $\cap_{\alpha\in I }F_{\alpha}$ is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$.

Case 2: If $|F_{1}| = 2$ and $|F_{2}| = 3$, then $F_{2}\in P_{\mathcal{G}}$ and hence, $F_{2}$ is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$. For $|F_{1}| = 2$, we have one of the following conditions in both cases: $F_{1}\in P_{\mathcal{G}}$ or $F_{1} = \mathcal{H}_{1}\cap \mathcal{H}_{2}$, where $|\mathcal{H}_{1}| = |\mathcal{H}_{2}| = 3$, i.e., $\mathcal{H}_{1}, \mathcal{H}_{2}\in P_{\mathcal{G}}$. Thus, $F_{1}$ is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$. Therefore, $\cap_{\alpha\in I }F_{\alpha}$ is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$.

Case 3: If $|F_{1}| = 2$ and $|F_{2}| = 2$, then we have one of the following conditions: $F_{1}, F_{2}\in P_{\mathcal{G}}$ or $F_{2}\in P_{\mathcal{G}}$ and $F_{1} = \mathcal{H}_{1}\cap \mathcal{H}_{2}$, where $|\mathcal{H}_{1}| = |\mathcal{H}_{2}| = 3$, i.e., $\mathcal{H}_{1}, \mathcal{H}_{2}\in P_{\mathcal{G}}$ or $F_{1}\in P_{\mathcal{G}}$ and $F_{2} = \mathcal{H}_{1}\cap \mathcal{H}_{2}$, where $|\mathcal{H}_{1}| = |\mathcal{H}_{2}| = 3$, $\mathcal{H}_{1}, \mathcal{H}_{2}\in P_{\mathcal{G}}$ or $F_{1} = \mathcal{H}_{1}\cap \mathcal{H}_{2}$ and $F_{2} = \mathcal{H}'_{1}\cap \mathcal{H}'_{2}$, where

i.e., $\mathcal{H}_{1}, \mathcal{H}_{2}, \mathcal{H}'_{1}, \mathcal{H}'_{2}\in P_{\mathcal{G}}$. Thus, $\cap_{\alpha\in I }F_{\alpha}$ is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$.

Let $\mathcal{G} = (\mathcal{V}, \mathcal{E})$ be a digraph. For every $x\in \mathcal{V}$, we write $U_{\mathcal{G}}(x)$ to denote the intersection of all open sets in $(\mathcal{V}, T_{P\mathcal{G}})$ containing $x$. From above theorem, $(\mathcal{V}, T_{P\mathcal{G}})$ is Alexandroff space and thus, $U_{\mathcal{G}}(\mathcal{H})$ is the smallest open set in $(\mathcal{V}, T_{P\mathcal{G}})$ containing $x$. It is clear that the collection $P_{\mathcal{G}}(\mathcal{V}) = \{U_{\mathcal{G}}(x):x\in \mathcal{V}\}$ forms a minimal basis of $(\mathcal{V}, T_{P\mathcal{G}})$. For every $\mathcal{H} \subseteq \mathcal{V}$, $U_{\mathcal{G}}(\mathcal{H})$ denotes the intersection of all open sets in $(\mathcal{V}, T_{P\mathcal{G}})$ containing $\mathcal{H}$.

Theorem 2.8. If $\mathcal{G} = (\mathcal{V}, \mathcal{E})$ is a digraph, then $U_{\mathcal{G}}(\mathcal{H}) = \cap\{G\in P_{\mathcal{G}}:\mathcal{H} \subseteq G\}$, for all $\mathcal{H} \subseteq \mathcal{V}$.

Proof. It is clear that $G$ is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$, for all $G\in P_{\mathcal{G}}$. Using Theorem 2.7, we have that $\cap\{G\in P_{\mathcal{G}}:\mathcal{H} \subseteq G\}$ is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$. Since $\mathcal{H} \subseteq G$ for all $G\in \{G\in P_{\mathcal{G}}:\mathcal{H} \subseteq G\},$ thus $\mathcal{H} \subseteq \cap\{G\in P_{\mathcal{G}}:\mathcal{H} \subseteq G\}$ and so $U_{\mathcal{G}}(\mathcal{H})\subseteq \cap\{G\in P_{\mathcal{G}}:\mathcal{H} \subseteq G\}.$ Again, the collection of all intersections of members of $P_{\mathcal{G}}$ forms a basis for $(\mathcal{V}, T_{P\mathcal{G}})$, thus $\cap\{G\in P_{\mathcal{G}}:\mathcal{H} \subseteq G\}\subseteq U_{\mathcal{G}}(\mathcal{H}).$ Hence, $U_{\mathcal{G}}(\mathcal{H}) = \cap\{G\in P_{\mathcal{G}}:\mathcal{H} \subseteq G\}$.

Corollary 2.9. If $\mathcal{G} = (\mathcal{V}, \mathcal{E})$ is a digraph then for all $x\in \mathcal{V}$, $U_{\mathcal{G}}(x) = \cap\{G\in P_{\mathcal{G}}:x\in G\}.$

Corollary 2.10. If $\mathcal{G} = (\mathcal{V}, \mathcal{E})$ be a digraph, $E \in {\mathcal{E}}$ and $| (E)_{\mathcal{E}}| = 0$ or $| (E)_{\mathcal{E}}|\geq 2$. Then, $U_{\mathcal{G}}(Ends(E)) = Ends(E).$

Proof. Let $\mathcal{G} = (\mathcal{V}, \mathcal{E})$ be a digraph and $E\in \mathcal{E}$. If $| (E)_{\mathcal{E}}| = 0$, then $Ends(E)\in P_{\mathcal{G}}$ is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$. So, $U_{\mathcal{G}}(Ends(E)) = Ends(E)$.

If $| (E)_{\mathcal{E}}|\geq 2$, then by Theorem 2.5, $Ends(E)$ is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$. So, $U_{\mathcal{G}}(Ends(E)) = Ends(E)$.

Corollary 2.11. If $|(E)_{\mathcal{E}}| = 1$, then there is $E'\in (E)_{\mathcal{E}}$such that $U_{\mathcal{G}}(Ends(E)) = Ends(E)\cup Ends(E').$

Proof. If $| (E)_{\mathcal{E}}| = 1$, then there is $E'\in (E)_{\mathcal{E}}$ such that $Ends(E)\cup Ends(E')\in P_{\mathcal{G}}$. So, $U_{\mathcal{G}}(Ends(E)) = Ends(E)\cup Ends(E').$

Corollary 2.12. Let $E, E'\in \mathcal{E}$ be two directed edges in a digraph $\mathcal{G} = (\mathcal{V}, \mathcal{E})$. Then, $(E)_{\mathcal{E}} = \{E'\}$ if andonly if $Ends(E')\subseteq U_{\mathcal{G}}(Ends(E))$

Proof. Suppose that $E, E'\in \mathcal{E}$ and $(E)_{\mathcal{E}} = \{E'\}$, i.e., $| (E)_{\mathcal{E}}| = 1$. Then, $U_{\mathcal{G}}(Ends(E)) = Ends(E)\cup Ends(E').$ Hence, $Ends(E')\subseteq U_{\mathcal{G}}(Ends(E))$.

Conversely, suppose that $Ends(E')\subseteq U_{\mathcal{G}}(Ends(E))$. Since, $Ends(E)\subseteq U_{\mathcal{G}}(Ends(E))$, then $Ends(E') = Ends(E)\mbox{ or }Ends(E')\neq Ends(E).$ If $Ends(E') = Ends(E)$, then the proof is complete. If $Ends(E')\neq Ends(E)$ and we have $Ends(E)\cup Ends(E')\subseteq U_{\mathcal{G}}(Ends(E))$, then $|Ends(E)\cup Ends(E')| = 3$, since $U_{\mathcal{G}}(Ends(E))$ is the smallest open set in $(\mathcal{V}, T_{P\mathcal{G}})$ containing $Ends(E)$. Then, $U_{\mathcal{G}}(Ends(E))\in P_{\mathcal{G}}$ and $(E)_{\mathcal{E}} = \{E'\}$, since if $E''\in (E)_{\mathcal{E}}$, then by Corollary 2.10, $U_{\mathcal{G}}(Ends(E)) = Ends(E)$.

Throughout the paper, $\overline{A}$ denotes closure of a subset $A$ in a topological space.

Corollary 2.13. Let $\mathcal{G} = (\mathcal{V}, \mathcal{E})$ be a digraph and $E, E'\in \mathcal{E}$. Then, $(E')_{\mathcal{E}} = \{E\}$ if andonly if $Ends(E')\subseteq \overline{U_{\mathcal{G}}(Ends(E))}$.

Proof. Suppose that $Ends(E')\subseteq \overline{U_{\mathcal{G}}(Ends(E))}$.Then for all open set $A$ containing $Ends(E')$, $A\cap U_{\mathcal{G}}(Ends(E))\neq \emptyset$. So, $U_{\mathcal{G}}(Ends(E'))\cap U_{\mathcal{G}}(Ends(E))\neq \emptyset.$ Then, $Ends(E)\cup Ends(E')\in P_{\mathcal{G}}$ and $(E')_{\mathcal{E}} = \{E\}$, since if $E''\in (E')_{\mathcal{E}}$, then by Corollary 2.10, we have $U_{\mathcal{G}}(Ends(E')) = Ends(E')$.

Conversely, suppose that $(E')_{\mathcal{E}} = \{E\}$. Then by Corollary 2.12, $Ends(E)\subseteq U_{\mathcal{G}}(Ends(E'))$. Thus for all open set $A$ containing $Ends(E')$, we have $Ends(E)\subseteq A$ and $A\cap Ends(E) = Ends(E)\neq \emptyset.$ Since $Ends(E) \subseteq U_{\mathcal{G}}(Ends(E))$ then $A\cap U_{\mathcal{G}}(Ends(E))\neq \emptyset$. Hence, $Ends(E')\subseteq \overline{U_{\mathcal{G}}(Ends(E))}$.

Let $\mathcal{G} = (\mathcal{V}, \mathcal{E})$ be a digraph. Recall ^[9] that an Alexandroff space $(\mathcal{V}, T_{P\mathcal{G}})$ is $T_{0}$-space if and only if $U_{\mathcal{G}}(x)\neq U_{\mathcal{G}}(u)$, for all $u\neq x\in \mathcal{V}$. An Alexandroff space $(\mathcal{V}, T_{P\mathcal{G}})$ is $T_{1}$-space if and only if $U_{\mathcal{G}}(x) = \{x\}$, for all $u\neq x\in \mathcal{V}$ i.e., if and only if $(\mathcal{V}, T_{P\mathcal{G}})$ is discrete.

Proposition 2.14. If $\mathcal{G} = (\mathcal{V}, \mathcal{E})$ is a digraph, then $\cup_{E\in \mathcal{E}}\{Ends(E): | (E)_{\mathcal{E}}| = 0\mathit{\mbox{or}}| (E)_{\mathcal{E}}|\geq 2\}$ is anopen set in $(\mathcal{V}, T_{P\mathcal{G}})$.

Proof. By Theorem 2.5, for $E\in \mathcal{E}$ with $| (E)_{\mathcal{E}}| = 0$ or $| (E)_{\mathcal{E}}|\geq 2$, $Ends(E)$ is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$. So, $\cup_{E\in \mathcal{E}}\{Ends(E):| (E)_{\mathcal{E}}| = 0\mbox{ or }| (E)_{\mathcal{E}}|\geq 2\}$ is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$.

Proposition 2.15. If $\mathcal{G} = (\mathcal{V}, \mathcal{E})$ is a digraph, then $\cup_{E\in \mathcal{E}}\{Ends(E):| (E)_{\mathcal{E}}| = 1\}$ is a closed set in $(\mathcal{V}, T_{P\mathcal{G}})$.

Proof. Let $C = \cup_{E\in \mathcal{E}}\{Ends(E):| (E)_{\mathcal{E}}| = 1\}.$ It is clear that $\overline{C} = \cup_{E\in \mathcal{E}}\{\overline{Ends(E)}:| (E)_{\mathcal{E}}| = 1\}.$ By Proposition 2.14, $\overline{Ends(E)}\subseteq C$, for all $Ends(E)\subseteq C$. So, $\overline{C}\subseteq C$. Thus, $\overline{C} = C$ and hence, $C$ is a closed set in $(\mathcal{V}, T_{P\mathcal{G}})$.

Let $\mathcal{G} = (\mathcal{V}, \mathcal{E})$ be a digraph. For $x\in \mathcal{V}$, $\mathcal{E}(x)$ denotes the set of all $E\in \mathcal{H}$ such that $x\in Ends(E)$ and $\mathcal{V}(x)$ denotes the set of all $x'\in \mathcal{V}$ such that $x$ is joined with $x'$ by a directed edge.

Proposition 2.16. If $\mathcal{G} = (\mathcal{V}, \mathcal{E})$ is a digraph, then$\cap_{E\in \mathcal{E}(x)}U_{\mathcal{G}}(Ends(E)) = U_{\mathcal{G}}(x),$ for $x\in \mathcal{V}$.

Proof. It is clear that $U_{\mathcal{G}}(x) \subseteq\cap_{E\in \mathcal{E}(x)}U_{\mathcal{G}}(Ends(E)).$ Since, $U_{\mathcal{G}}(x)$ is the intersection of all open sets in $(\mathcal{V}, T_{P\mathcal{G}})$ containing $x$ and $P_{\mathcal{G}}$ is the subbasis of $(\mathcal{V}, T_{P\mathcal{G}})$, thus $U_{\mathcal{G}}(x) = \cap_{E\in \mathcal{E'} }U_{\mathcal{G}}(Ends(E))$, for some subset $\mathcal{E'}$ of $\mathcal{E}$. Then, $x\in U_{\mathcal{G}}(Ends(E))$, for all $E\in \mathcal{E'}$. Hence, $E\in \mathcal{E}(x)$, for all $E\in \mathcal{E'}$ i.e., $\mathcal{E'}\subseteq \mathcal{E}(x)$. So, $\cap_{E\in \mathcal{E}(x)}U_{\mathcal{G}}(Ends(E))\subseteq U_{\mathcal{G}}(x)$. Hence, the proof is done.

3.   Relative topology and subdigraph

A digraph $\mathcal{H}$ is called subdigraph of a digraph $\mathcal{G} = (\mathcal{V}, \mathcal{E})$, if the direction function of $\mathcal{H}$ is the restriction of the direction function of $\mathcal{G}$ on $\mathcal{H}_{\mathcal{E}}$ and all edges and vertices of $\mathcal{H}$ are in $\mathcal{G}$. A collection of the edges in a digraph $\mathcal{G} = (\mathcal{V}, \mathcal{E})$ together with their terminals is called E-generated subdigraph of $\mathcal{G} = (\mathcal{V}, \mathcal{E})$. For any E-generated subdigraph $\mathcal{G}_{\mathcal{H}}$ of $\mathcal{G} = (\mathcal{V}, \mathcal{E})$, $\mathcal{V}_{\mathcal{H}}$ denotes the set of all vertices of $\mathcal{G}_{\mathcal{H}}$, $\mathcal{E}_{\mathcal{H}}$ denotes the set of all edges of $\mathcal{G}_{\mathcal{H}}$, $T_{P\mathcal{GH}}$ denotes the pathless directed topology of $\mathcal{G}_{\mathcal{H}}$ and $P_{\mathcal{GH}}$ is the subbasis of $(\mathcal{V}_{\mathcal{H}}, T_{P\mathcal{GH}})$.

Theorem 3.1. For any E-generated subdigraph$\mathcal{G}_{\mathcal{H}}$ of$\mathcal{G} = (\mathcal{V}, \mathcal{E})$, $T_{P\mathcal{GH}}\subseteq T_{P\mathcal{G}}|_{\mathcal{V}_{\mathcal{H}}}$, where$T_{P\mathcal{G}}|_{\mathcal{V}_{\mathcal{H}}}$ is the relativetopology of $T_{P\mathcal{G}}$ on $\mathcal{V}_{\mathcal{H}}$.

Proof. Let $G\in T_{P\mathcal{GH}}$. We will prove that $G = F\cap \mathcal{V}_{\mathcal{H}}$, for some open set $F$ in $(\mathcal{V}, T_{P\mathcal{G}})$. Let $F' = \cap\{D\in T_{P\mathcal{G}}: G\subseteq D\}.$ Then by Theorem 2.7, $F'$ is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$ and $F'\cap \mathcal{V}_{\mathcal{H}} = G$. Thus, $G\in T_{P\mathcal{G}}|_{\mathcal{V}_{\mathcal{H}}}$.

In general, for any E-generated subdigraph $\mathcal{G}_{\mathcal{H}}$ of $\mathcal{G} = (\mathcal{V}, \mathcal{E})$, we have $T_{P\mathcal{GH}}\neq T_{P\mathcal{G}}|\mathcal{V}_{\mathcal{H}}$, we have the following example for it.

In Figure 3, $\mathcal{V} = \{x_{1}, x_{2}, x_{3}, x_{4} \}$ and $T_{P\mathcal{G}} = \{\emptyset, \mathcal{V}, \{x_{1}, x_{2}, x_{3}\}, \{x_{2}, x_{3}, x_{4}\}, \{x_{2}, x_{3}\}\}.$ We consider $\mathcal{V}_{\mathcal{H}} = \{x_{1}, x_{2}, x_{3}\}$ and $\mathcal{E}_{\mathcal{H}} = \{x_{1}x_{2}, x_{3}x_{2}\}$. Thus, we find that $T_{P\mathcal{GH}} = \{\emptyset, \mathcal{V}_{\mathcal{H}}\} \mbox{ and } T_{P\mathcal{G}}|\mathcal{V}_{\mathcal{H}} = \{\emptyset, \mathcal{V}_{\mathcal{H}}, \{x_{2}, x_{3}\}\}.$

An E-generated subdigraph $\mathcal{G}_{\mathcal{H}} = (\mathcal{V}_{\mathcal{H}}, \mathcal{E}_{\mathcal{H}})$ of $\mathcal{G} = (\mathcal{V}, \mathcal{E})$ is called an adjacent with $\mathcal{G}$ if $| (E)_{\mathcal{E}}|\geq 2$ in $\mathcal{G}$ implies $| (E)_{\mathcal{E}_{\mathcal{H}}}|\geq 2$ in $\mathcal{G}_{\mathcal{H}}$, for all $E\in\mathcal{E}_{\mathcal{H}}$.

Theorem 3.2. Let $\mathcal{G}_{\mathcal{H}} = (\mathcal{V}_{\mathcal{H}}, \mathcal{E}_{\mathcal{H}})$ be an E-generated subdigraph of $\mathcal{G} = (\mathcal{V}, \mathcal{E})$. Then, $\mathcal{G}_{\mathcal{H}}$ is an adjacent with$\mathcal{G}$ if and only if $T_{P\mathcal{GH}} = T_{P\mathcal{G}}|_{\mathcal{V}_{\mathcal{H}}}$.

Proof. Suppose that $\mathcal{G}_{\mathcal{H}}$ is an adjacent with $\mathcal{G}$. Let $G\in T_{P\mathcal{G}}|_{\mathcal{V}_{\mathcal{H}}}$ and $G\notin T_{P\mathcal{GH}}$. Since $\mathcal{V}_{\mathcal{H}}\in T_{P\mathcal{GH}}\cap T_{P\mathcal{G}}|_{\mathcal{V}_{\mathcal{H}}}$, then $G = Ends(E)$, for some $E\in \mathcal{E}_{\mathcal{H}}$ such that $| (E)_{\mathcal{E}}|\geq 2$ in $\mathcal{G}$ and $| (E)_{\mathcal{E}_{\mathcal{H}}}| = 1$ in $\mathcal{G}_{\mathcal{H}}$. This is a contradiction to the hypothesis, that $T_{P\mathcal{G}}|_{\mathcal{V}_{\mathcal{H}}}\subseteq T_{P\mathcal{GH}}$. For the other hand, $T_{P\mathcal{GH}}\subseteq T_{P\mathcal{G}}|_{\mathcal{V}_{\mathcal{H}}}$ by Theorem 5.7. Thus, $T_{P\mathcal{GH}} = T_{P\mathcal{G}}|_{\mathcal{V}_{\mathcal{H}}}$.

Conversely, let $T_{P\mathcal{GH}} = T_{P\mathcal{G}}|_{\mathcal{V}_{\mathcal{H}}}$. Suppose that there is $E\in\mathcal{E}_{\mathcal{H}}$ such that $| (E)_{\mathcal{E}}|\geq 2$ in $\mathcal{G}$ and $| (E)_{\mathcal{E}_{\mathcal{H}}}| < 2$ in $\mathcal{G}_{\mathcal{H}}$. Then, by Theorem 2.5, $Ends(E)$ is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$. Hence, $Ends(E)\cap \mathcal{V}_{\mathcal{H}} = Ends(E)$ is an open set in $(\mathcal{V}_{\mathcal{H}}, T_{P\mathcal{G}}|_{\mathcal{V}_{\mathcal{H}}})$ but $Ends(E)$ is not open set in $(\mathcal{V}_{\mathcal{H}}, T_{P\mathcal{GH}})$. This is a contradiction to the hypothesis, that $\mathcal{G}_{\mathcal{H}}$ is an adjacent with $\mathcal{G}$.

Theorem 3.3. Let $\mathcal{G} = (\mathcal{V}, \mathcal{E})$ be adigraph without isolated points. If the pathless directedtopological space $(\mathcal{V}, T_{P\mathcal{G}})$ is connectedspace, then $\mathcal{G}$ is a connected digraph.

Proof. Suppose that the digraph $\mathcal{G}$ is a disconnected graph. Let $\{\mathcal{G}_{\alpha}:\alpha\in \Delta\}$ be the collection of all directed subgraphs of $\mathcal{G}$. Then for every $\alpha\in \Delta$, we have $\mathcal{V}_{\mathcal{G}_{\alpha}} = \cup\{Ends(E):E\in \mathcal{E}(\mathcal{G}_{\alpha})\}$, which is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$. Since $\mathcal{G}$ has no isolated points, thus $\mathcal{V}^{c}_{\mathcal{G}_{\alpha}} = \mathcal{V}-\mathcal{V}_{\mathcal{G}_{\alpha}}$ is an open set in $(\mathcal{V}, T_{P\mathcal{G}})$ and $\mathcal{V} = \mathcal{V}^{c}_{\mathcal{G}_{\alpha}}\cup \mathcal{V}_{\mathcal{G}_{\alpha}}$. Thus, the pathless directed topological space $(\mathcal{V}, T_{P\mathcal{G}})$ is disconnected space. This is a contradiction to the hypothesis. So, graph $\mathcal{G}$ is a connected digraph.

Converse of the above theorem above is not true in general. For example, in Figure 4, the pathless directed topological space $(\mathcal{V}, T_{P\mathcal{G}})$ is disconnected space but the graph $\mathcal{G}$ is connected, where $T_{P\mathcal{G}} = \{\emptyset, \mathcal{V}, \{x_{1}, x_{2}\}\{x_{3}, x_{2}\}, \{x_{3}, x_{4}\},$ $\{x_{2}\}, \{x_{3}\}, \{x_{1}, x_{2}, x_{3}\}, \{x_{2}, x_{3}, x_{4}\}\}.$

4.   Digraph isomorphic property

For any two digraphs $\mathcal{G}_{1} = (\mathcal{V}_{1}, \mathcal{E}_{1})$ and $\mathcal{G}_{2} = (\mathcal{V}_{2}, \mathcal{E}_{2})$, by graph function of $\mathcal{G}_{1}$ into $\mathcal{G}_{2}$ we mean a pair $(\Phi_{\mathcal{V}_{1}\mathcal{V}_{2}}, \Phi_{\mathcal{E}_{1}\mathcal{E}_{2}}):\mathcal{G}_{1}\to \mathcal{G}_{2}$ of two functions $\Phi_{\mathcal{V}_{1}\mathcal{V}_{2}}:\mathcal{V}_{1}\to \mathcal{V}_{2}$ and $\Phi_{\mathcal{E}_{1}\mathcal{E}_{2}}:\mathcal{E}_{1}\to \mathcal{E}_{2}$. Recall ^[19] that the isomorphism of $\mathcal{G}_{1}$ onto $\mathcal{G}_{2}$ is a graph function $(\Phi_{\mathcal{V}_{1}\mathcal{V}_{2}}, \Phi_{\mathcal{E}_{1}\mathcal{E}_{2}}):\mathcal{G}_{1}\to \mathcal{G}_{2}$ of two bijective functions $\Phi_{\mathcal{V}_{1}\mathcal{V}_{2}}:\mathcal{V}_{1}\to\mathcal{V}_{2}$ and $\Phi_{\mathcal{E}_{1}\mathcal{E}_{2}}:\mathcal{E}_{1}\to \mathcal{E}_{2}$ such that $\Phi_{\mathcal{E}_{1}\mathcal{E}_{2}}(E_{x_{1}x_{2}}) = \Phi_{\mathcal{E}_{1}\mathcal{E}_{2}}(E_{\Phi_{\mathcal{V}_{1}\mathcal{V}_{2}}(x_{1})\Phi_{\mathcal{V}_{1}\mathcal{V}_{2}}(x_{2})})$, for all $E_{x_{1}x_{2}}\in\mathcal{E}_{1}$ and $x_{1}, x_{2}\in \mathcal{V}_{1}$. Here, $E_{x_{1}x_{2}}\in\mathcal{E}_{1}$ is an edge directed from $x_{1}$ to $x_{2}$ in $\mathcal{G}_{1}$ if and only if $\Phi_{\mathcal{E}_{1}\mathcal{E}_{2}}(E_{x_{1}x_{2}})$ is an edge directed from $\Phi_{\mathcal{V}_{1}\mathcal{V}_{2}}(x_{1})$ to $\Phi_{\mathcal{V}_{1}\mathcal{V}_{2}}(x_{2})$ in $\mathcal{G}_{2}$. If there exists an isomorphism of $\mathcal{G}_{1}$ onto $\mathcal{G}_{2}$, then we say that $\mathcal{G}_{1}$ and $\mathcal{G}_{2}$ are isomorphic and write $\mathcal{G}_{1}\cong\mathcal{G}_{2}$.

Theorem 4.1. If the two digraphs $\mathcal{G}_{1} = (\mathcal{V}_{1}, \mathcal{E}_{1})$ and$\mathcal{G}_{2} = (\mathcal{V}_{2}, \mathcal{E}_{2})$ are isomorphic, then the two pathless directed topological spaces $(\mathcal{V}_{1}, T_{P\mathcal{G}_{1}})$ and $(\mathcal{V}_{2}, T_{P\mathcal{G}_{2}})$ are homeomorphic.

Converse of Theorem 4.1 is not true in general. In Figure 5, two pathless directed topological spaces $(\mathcal{V}_{1}, T_{P\mathcal{G}_{1}})$ and $(\mathcal{V}_{2}, T_{P\mathcal{G}_{2}})$ are homeomorphic, where $\mathcal{V}_{1} = \{x_{1}, x_{2}\}, \mbox{ }\mathcal{V}_{2} = \{y_{1}, y_{2}\}, \mbox{ }T_{P\mathcal{G}_{1}} = \{\emptyset, \mathcal{V}_{1}\}\mbox{ and } T_{P\mathcal{G}_{2}} = \{\emptyset, \mathcal{V}_{2}\},$ but the two graphs $\mathcal{G}_{1}$ and $\mathcal{G}_{2}$ are not isomorphic.

Theorem 4.2. If $\mathcal{G}_{1}$ and$\mathcal{G}_{2}$ are two simple digraphs and$\mu:\mathcal{V}_{1}\to \mathcal{V}_{2}$ is continuous function, then$(E_{yx})_{\mathcal{E}_{1}} = \{E_{zx}\} \mathit{\mbox{}} (\mathit{\mbox{resp.}}(E_{yx})_{\mathcal{E}_{1}} = \{E_{yz}\})$ implies $(E_{\mu(y)\mu(x)})_{\mathcal{E}_{2}} = \{E_{\mu(z)\mu(x)}\}\mathit{\mbox{}}(\mathit{\mbox{resp.}}$$(E_{\mu(y)\mu(x)})_{\mathcal{E}_{2}} = \{E_{\mu(y)\mu(z)}\})$, for all $y, x, z\in \mathcal{V}_{1}$.

Proof. Suppose that $\mu:\mathcal{V}_{1}\to \mathcal{V}_{2}$ is continuous function and $y, x, z\in \mathcal{V}_{1}$ such that $(E_{yx})_{\mathcal{E}_{1}} = \{E_{zx}\}$. By Corollary 2.14, $Ends(E_{yx})\subseteq \overline{U_{\mathcal{G}_{1}}(Ends(E_{zx}))}$. Then, $\mu[Ends(E_{yx})]\subseteq \mu[\overline{U_{\mathcal{G}_{1}}(Ends(E_{zx}))}].$ i.e. $Ends(E_{\mu(y)\mu(x)})\subseteq \mu[\overline{U_{\mathcal{G}_{1}}(Ends(E_{zx}))}].$ Since $\mu$ is continuous, thus $\mu\big(\overline{U_{\mathcal{G}_{1}}(Ends(E_{zx}))}\big) \subseteq \overline{U_{\mathcal{G}_{1}}(Ends(E_{\mu(z)\mu(x)}))}.$ Hence, $Ends(E_{\mu(y)\mu(x)})\subseteq \overline{U_{\mathcal{G}_{1}}(Ends(E_{\mu(z)\mu(x)}))}.$ Thus, $(E_{\mu(y)\mu(x)})_{\mathcal{E}_{2}} = \{E_{\mu(x)\mu(z)}\}$ from Corollary 2.14. Similarly, we prove the other case.

Converse of the above theorem is not true in general. For example, in Figure 6 we consider $\mathcal{V} = \{x_{1}, x_{2}, x_{3}\}, \mbox{ }\mathcal{V'} = \{x'_{1}, x'_{2}, x'_{3}, x'_{4}\},$ $T_{P\mathcal{G}} = \{\emptyset, \mathcal{V}\}\mbox{ and }T_{P\mathcal{G'}} = \{\emptyset, \mathcal{V'}, \{x'_{1}, x'_{2}, x'_{3}\}, \{x'_{3}, x'_{4}\}, \{x'_{3}\}\}.$ Let $\mu:\mathcal{V}\to \mathcal{V'}$ be a function given by $\mu(x_{1}) = x'_{1}, \mbox{ }\mu(x_{2}) = x'_{2}, \mbox{ and }\mu(x_{3}) = x'_{3}.$ Note that $\mu$ is not continuous while $(E_{x_{1}x_{2}})_{\mathcal{E}} = \{E_{x_{2}x_{3}}\}$ implies $(E_{\mu(x_{1})\mu(x_{2})})_{\mathcal{E}'} = \{E_{\mu(x_{2})\mu(x_{3})}\}$ and $(E_{x_{2}x_{3}})_{\mathcal{E}} = \{E_{x_{1}x_{2}}\}$ implies $(E_{\mu(x_{2})\mu(x_{3})})_{\mathcal{E}'} = \{E_{\mu(x_{1})\mu(x_{2})}\}$.

5.   Pathless directed topology in human heart

Recently, many ideas were generated in topology using graph theory, in particular neighbourhood of a vertex in a graph. In this section, we consider Figures 7 and 8 from ^[12] and Nada et al. ^[11] respectively. Before going further, we procure following definition.

Definition 5.1. ^[5] A graph $H$ is a subgraph of a graph $G$ if $V(H)\subseteq V(G)$ and $E(H)\subseteq E(G)$ so that the edges in $E(H)$ use only vertices in $V(H)$. We write $H \subseteq G$ and say $G$ contains $H$. An induced subgraph is a subgraph $G[S]$ with vertex $S \subseteq V(G)$ and all edges with both ends in $S$.

In Shokry and Aly ^[18], open-path in graph and topology were connected. As an application, Shokry and Aly ^[18] studied the circulation process of blood in a human heart. It is important to note that they were unable to write all the elements of the topology $\tau$ in Section 5.1. Thus, it is raising question on the validation of their claims $cl(X(p)) = \{v_1, v_2, v_3, e_1, e_2, e_3\}$, where $X(p) = \{v_1, v_2, e_1. e_2, e_3\}$. Similarly, their claim $int(X(p)) = \{v_4, e_4, v_5, e_6\}$, where $X(p) = \{v_4, e_4, v_5, e_6, v_6\}$ is also doubtful. Moreover, it is unclear to us about their biological justifications in Section 5.1 from $cl(X(p))$ and $int(X(p))$ which fail when any of $v_6$ or $v_7$ remains in $X(p)$. Thus, it clearly indicates that their methodology is not biologically feasible to justify the circulation process of blood in a human heart. In 2017, Nada et al. ^[11] introduced a new kind of topological structure using graphs and proved some fundamental properties. They introduced a topology on a set of vertices by considering post class for each vertex as an element of the subbasis. Moreover, they applied their results to the circulation process of blood in a human heart. Similar to ^[18], Nada et al. ^[11] also did not mention ways to get biological implications regarding the circulation of blood in a human heart from closure and interior of a subset from a subgraph. While developing the theory, they considered topology on $V$ of the graph $G = (V, E)$. At the time of application, Nada et al. ^[11] considered $H = \{v_1, v_2, e_1, e_2, e_3\}$ as a subgraph and found $cl(V(H))$, where $V(H) = \{v_1, v_2\}$. But from Definition 5.1, it is easy to find that $H$ is not a subgraph. Thus, their application is not flawless.

Again, Nawar et al. ^[12] and Othman ^[15] introduced nano topology induced by different neighbourhoods. Their methodology was inspired from upper approximation and lower approximation of rough set theory, but in neighbourhood sense. It was claimed from Figure 4 and Tables 4–7 of ^[12], that blood flows into a heart in a directed path. It means that blood must be passed through each successive point until completing its cycle. We agree with the flow process of blood in a heart as a directed graph, but we have disagreement with their mathematics since they never stated whether the open sets indicate the parts of the heart from which blood flows in a directed path. For example, from Table 4 of ^[12], it can be easily seen that if $V(H) = \{v_2, v_6\}$, then $\tau_{N_r}(V(H)) = \{V(G), \emptyset, \{v_2, v_5, v_6\}\}$. Now, because of topology defined Definition 2.3 of ^[12], it is necessary that $V(G)\in \tau_{N_r}(V(H))$. But, it is not clear to us about the biological interpretation of $\{v_2, v_5, v_6\}$ since, if we consider two parts of the heart $v_2$ and $v_6$, then according to Figure 8, blood does not flow from $v_2$ to $v_6$ via only $v_5$. Thus their claim "From Figure 4 and Tables 4–7, we show that the blood flows into a heart in a directed path, that's mean the blood must be passed through each successive point until completing its cycle" is not correct in case of $V(H) = \{v_2, v_6\}$. Thus, we are unable to agree with their mathematical ideas for biological conclusions. Hence, the theories of ^[11,12,18] in biological applications are doubtful. We shall show that pathless directed topological spaces are useful to describe the above biological process in better ways than ^[11,12,18].

Nada et al. ^[11] procured same notions as of Shokry and Aly ^[18] to identify different parts of a heart of human body for their calculations. For our purpose, we procure same notions of Shokry and Aly ^[18]. Let us consider superior vena cava, inferior vena cava, right atrium, right ventricle, pulmonary trunk, right lung, left lung, left atrium, left ventricle and aorta by the vertices $v_1, v_2, v_3, v_4, v_5, v_6, v_7, v_8, v_9$ and $v_{10}$, respectively. Figures 7 and 8 represent a digram of a heart and a diagraph representation of the circulation of blood in a heart respectively. Let $G = (V, E)$ represents the digraph representation of the heart of human body. Then, $V = \{v_1, v_2, v_3, v_4, v_5, v_6, v_7, v_8, v_9, v_{10}\}$ and $E = \{e_1, e_2, e_3, e_4, e_5, e_6, e_7, e_8, e_9, e_{10}\}$. In this case, we have $P_{\mathcal{G}} = \{\{v_1, v_2, v_3\}, \{v_3, v_4\}, \{v_4, v_5\},$ $\{v_5, v_6, v_7\}, \{v_6, v_7, v_8\}, \{v_8, v_9\}, \{v_9, v_{10}\}\}$. Now, to proceed further we shall find the topology $\tau_ {P_{\mathcal{G}}}$ generated from the subbasis $P_{\mathcal{G}}$.

Then, $\tau_{P_G}$ = $\{ V, \emptyset, \{v_1, v_2, v_3\},$ $\{v_3, v_4\},$ $\{v_4, v_5\},$ $\{v_5, v_6, v_7\},$ $\{v_6, v_7, v_8\},$ $\{v_8, v_9\},$ $\{v_{10}, v_9\},$ $\{v_3\},$ $\{v_4\},$ $\{v_5\},$ $\{v_6, v_7\},$ $\{v_8\},$ $\{v_9\},$ $\{v_1, v_2, v_3, v_4\},$ $\{v_1, v_2, v_3, v_4, v_5\},$ $\{v_1, v_2, v_3, v_5, v_6, v_7\},$ $\{v_1, v_2, v_3, v_6, v_7, v_8\},$ $\{v_1, v_2, v_3, v_8, v_9\},$ $\{v_1, v_{10}, v_2, v_3, v_9\},$ $\{v_1, v_2, v_3, v_5\},$ $\{v_1, v_2, v_3, v_6, v_7\},$ $\{v_1, v_2, v_3, v_8\},$ $\{v_1, v_2, v_3, v_9\},$ $\{v_3, v_4, v_5\},$ $\{v_3, v_4, v_5, v_6, v_7\},$ $\{v_3, v_4, v_6, v_7, v_8\},$ $\{v_3, v_4, v_8, v_9\},$ $\{v_{10}, v_3, v_4, v_9\},$ $\{v_3, v_4, v_6, v_7\}, \{v_3, v_4, v_8\},$ $\{v_3, v_4, v_9\},$ $\{v_4, v_5, v_6, v_7\},$ $\{v_4, v_5, v_6, v_7, v_8\}$ $, \{v_4, v_5, v_8, v_9\},$ $\{v_{10}, v_4, v_5, v_9\},$ $\{v_4, v_5, v_8\},$ $\{v_4, v_5, v_9\},$ $\{v_5, v_6, v_7, v_8\},$ $\{v_5, v_6, v_7, v_8, v_9\},$ $\{v_{10}, v_5, v_6, v_7, v_9\},$ $\{v_3, v_5, v_6, v_7\},$ $\{v_5, v_6, v_7, v_9\},$ $\{v_6, v_7, v_8, v_9\},$ $\{v_{10}, v_6, v_7, v_8, v_9\},$ $\{v_3, v_6, v_7, v_8\},$ $\{v_4, v_6, v_7, v_8\},$ $\{v_{10}, v_8, v_9\},$ $\{v_3, v_8, v_9\},$ $\{v_4, v_8, v_9\},$ $\{v_5, v_8, v_9\},$ $\{v_{10}, v_3, v_9\},$ $\{v_{10}, v_4, v_9\},$ $\{v_{10}, v_5, v_9\},$ $\{v_{10}, v_6, v_7, v_9\},$ $\{v_3, v_5\},$ $\{v_3, v_6, v_7\},$ $\{v_3, v_8\},$ $\{v_3, v_9\},$ $\{v_4, v_6, v_7\},$ $\{v_4, v_8\},$ $\{v_4, v_9\},$ $\{v_5, v_8\},$ $\{v_5, v_9\}$ $, \{v_6, v_7, v_9\},$ $\{v_1, v_2, v_3, v_4, v_5, v_6, v_7\},$ $\{v_1, v_2, v_3, v_4, v_6, v_7, v_8\},$ $\{v_1, v_2, v_3, v_4, v_8, v_9\},$ $\{v_1, v_{10}, v_2, v_3, v_4, v_9\},$ $\{v_1, v_2, v_3, v_4, v_6, v_7\},$ $\{v_1, v_2, v_3, v_4, v_8\},$ $\{v_1, v_2, v_3, v_4, v_9\},$ $\{v_1, v_2, v_3, v_4, v_5, v_6, v_7, v_8\},$ $\{v_1, v_2, v_3, v_4, v_5, v_8, v_9\},$ $\{v_1, v_{10}, v_2, v_3, v_4, v_5, v_9\},$ $\{v_1, v_2, v_3, v_4, v_5, v_8\},$ $\{v_1, v_2, v_3, v_4, v_5, v_9\},$ $\{v_1, v_2, v_3, v_5, v_6, v_7, v_8\},$ $\{v_1, v_2, v_3, v_5, v_6, v_7, v_8, v_9\},$ $\{v_1, v_{10}, v_2, v_3, v_5, v_6, v_7, v_9\},$ $\{v_1, v_2, v_3, v_5, v_6, v_7, v_9\}$ $, \{v_1, v_2, v_3, v_6, v_7, v_8, v_9\},$ $\{v_1, v_{10}, v_2, v_3, v_6, v_7, v_8, v_9\},$ $\{v_1, v_{10}, v_2, v_3, v_8, v_9\},$ $\{v_1, v_2, v_3, v_5, v_8, v_9\},$ $\{v_1, v_{10}, v_2, v_3, v_5, v_9\},$ $\{v_1, v_{10}, v_2, v_3, v_6, v_7, v_9\},$ $\{v_1, v_2, v_3, v_5, v_8\},$ $\{v_1, v_2, v_3, v_5, v_9\}$ $, \{v_1, v_2, v_3, v_6, v_7, v_9\}, \{v_3, v_4, v_5, v_6, v_7, v_8\},$ $\{v_3, v_4, v_5, v_8, v_9\},$ $\{v_{10}, v_3, v_4, v_5, v_9\},$ $\{v_3, v_4, v_5, v_8\},$ $\{v_3, v_4, v_5, v_9\},$ $\{v_3, v_4, v_5, v_6, v_7, v_8, v_9\},$ $\{v_{10}, v_3, v_4, v_5, v_6, v_7, v_9\}, \{v_3, v_4, v_5, v_6, v_7, v_9\},$ $\{v_3, v_4, v_6, v_7, v_8, v_9\},$ $\{v_{10}, v_3, v_4, v_6, v_7, v_8, v_9\},$ $\{v_{10}, v_3, v_4, v_8, v_9\},$ $\{v_{10}, v_3, v_4, v_6, v_7, v_9\},$ $\{v_3, v_4, v_6, v_7, v_9\},$ $\{v_4, v_5, v_6, v_7, v_8, v_9\},$ $\{v_{10}, v_4, v_5, v_6, v_7, v_9\},$ $\{v_4, v_5, v_6, v_7, v_9\},$ $\{v_{10}, v_4, v_5, v_6, v_7, v_8, v_9\},$ $\{v_{10}, v_4, v_5, v_8, v_9\},$ $\{v_{10}, v_5, v_6, v_7, v_8, v_9\},$ $\{v_3, v_5, v_6, v_7, v_8\},$ $\{v_3, v_5, v_6, v_7, v_8, v_9\},$ $\{v_{10}, v_3, v_5, v_6, v_7, v_9\},$ $\{v_3, v_5, v_6, v_7, v_9\},$ $\{v_3, v_6, v_7, v_8, v_9\},$ $\{v_4, v_6, v_7, v_8, v_9\},$ $\{v_{10}, v_3, v_6, v_7, v_8, v_9\},$ $\{v_{10}, v_4, v_6, v_7, v_8, v_9\},$ $\{v_{10}, v_3, v_8, v_9\},$ $\{v_{10}, v_4, v_8, v_9\},$ $\{v_{10}, v_5, v_8, v_9\},$ $\{v_3, v_5, v_8, v_9\},$ $\{v_{10}, v_3, v_5, v_9\},$ $\{v_{10}, v_3, v_6, v_7, v_9\},$ $\{v_{10}, v_4, v_6, v_7, v_9\},$ $\{v_3, v_5, v_8\},$ $\{v_3, v_5, v_9\},$ $\{v_3, v_6, v_7, v_9\},$ $\{v_4, v_6, v_7, v_9\},$ $\{v_1, v_2, v_3, v_4, v_5, v_6, v_7, v_8, v_9\},$ $\{v_1, v_{10}, v_2, v_3, v_4, v_5, v_6, v_7, v_9\},$ $\{v_1, v_2, v_3, v_4, v_5, v_6, v_7, v_9\},$ $\{v_1, v_2, v_3, v_4, v_6, v_7, v_8, v_9\},$ $\{v_1, v_{10}, v_2, v_3, v_4, v_6, v_7, v_8, v_9\},$ $\{v_1, v_{10}, v_2, v_3, v_4, v_8, v_9\},$ $\{v_1, v_{10}, v_2, v_3, v_4, v_6, v_7, v_9\},$ $\{v_1, v_2, v_3, v_4, v_6, v_7, v_9\},$ $\{v_1, v_{10}, v_2, v_3, v_4, v_5, v_6, v_7, v_8, v_9\}, \{v_1, v_{10}, v_2, v_3, v_4, v_5, v_8, v_9\},$ $\{v_1, v_{10}, v_2, v_3, v_5, v_6, v_7, v_8, v_9\},$ $\{v_1, v_{10}, v_2, v_3, v_5, v_8, v_9\}, \{v_{10}, v_3, v_4, v_5, v_6, v_7, v_8, v_9\},$ $\{v_{10}, v_3, v_4, v_5, v_8, v_9\},$ $\{v_{10}, v_3, v_5, v_6, v_7, v_8, v_9\},$ $\{v_{10}, v_3, v_5, v_8, v_9\}\}$

Now, before going further we procure following Definitions 5.5 and 5.6 from ^[18].

Definition 5.2. ^[18] The closure of the path on the graph is:

$cl(X(p)) = X(p)\cup \{\{v_i, e_i\}: v_i\in V, N(v_i)\cap X(p)\neq \emptyset$ and $\{e_i\}\cap X(p)\neq \emptyset\}$.

Definition 5.3. ^[18] The interior of any path on the graph is:

$int(X(p)) = \{\{v_i, e_i\}: v_i\in V, e_i\in E, N(v_i)\subseteq X(p)$ and $\{e_i\}\in X(p)\}$.

Here, $p$ indicates a path and thus, $X(p)$ indicates the set containing vertices and edges of the path $p$.

But, it is natural to find that the Definition 5.1 does not follow the following property of closure of a subset ^[21] in a topological space $(X, \tau)$.

Theorem 5.4. Let $(X, \tau)$ be a topological space and $A\subseteq X$. Then, $x\in cl(A)$ if and only if $A\cap U\neq \emptyset$ $\forall U\in \tau, x\in U.$

Similarly, the Definition 5.6 does not follow property of interior in a topological space because no neighbourhood of $e_i$ was considered in this definition. The reason follows from $X = V\cup E$ of ^[18]. Thus, neighborhoods of edges must play the role to define closure and interior of $X(p)$ of any path $p$. Thus, we rectify their notions below.

Definition 5.5. The closure of the path on the graph is:

$cl(X(p)) = X(p)\cup \{\{v_i, e_i\}: v_i\in V, N(v_i)\cap X(p)\neq \emptyset$ and $N(e_i)\cap X(p)\neq \emptyset\}$.

Definition 5.6. The interior of any path on the graph is:

$int(X(p)) = \{v_i, e_i: v_i\in V, e_i\in E, N(v_i)\subseteq X(p)$ and $N(e_i)\subseteq X(p)\}$.

Now, we procure following two subsets to compare the circulation of blood in a heart of human body with refer to ^[18]. For this purpose, we prepare the following Table 1 using the subset H and its closure in the sense of ^[18] and us.

Thus, it can be checked that our method is more precise than ^[18] and in our method, $cl(H) = \{v_1, v_2\}$, i.e., blood flows from $v_1$ and $v_2$. Since, the flow of blood is a digraph, thus this can be easily concluded. Moreover, Theorem 5.7 clearly concludes that $cl(H) = \{v_1, v_2, v_3, e_1, e_2, e_3\}$ is not correct because it does not satisfy this fundamental property. Moreover, our calculation $cl(H) = \{v_1, v_2\}$ clearly tells the above phenomenon.

Again, we prepare the following table using the subset H and its interior in the sense of ^[18] and us.

In Table 2, Shokry and Aly ^[18] found two interiors $\{v_4, e_4, v_5, e_6, v_6 \}$, $\{ v_4, e_4, v_5, e_6\}$ of $\{v_4, e_4, v_5, e_6, v_6\}$. But, due to the medical application, they neglected $\{v_4, e_4, v_5, e_6, v_6\}$. But, it is to remember that neither general topology nor any of its generalized form supports existence of two interiors of a same subset. Thus, their methodology is neither mathematically feasible nor biologically correct. Similar flaws can be observed in Nada et al. ^[11]. We procure the following definition of Nada et al. ^[11].

Definition 5.7. Let $G = (V, E)$ be a graph and $R$ be a relation on $G$, then for each $v_i\in V$ as defined above, we define the post class for each $v_i$ as the open neighbourhood of $v_i$ in $R$. It is denoted by $v_iR$. We construct a subbase for a topology by $S_G = \bigcup \{v_iR: v_i\in V(G)\}$. By the intersection of members of $S_G$, we define a base $\beta_G$ and by the arbitrary union of members of $\beta_G$ which is the topological structure $\tau_G$ on a grpah $G$.

In case of a heart, Nada et al. ^[11] considered a subgraph $H = \{v_1, v_2, e_1, e_2, e_3\}$, where $V(H) = \{v_1, v_2\}$. They calculated $cl(V(H)) = \{v_1, v_2, v_3\}$. But, we observed two interesting facts in Nada et al. ^[11]. At first, $H$ is not a subgraph by Definition 5.1 and after recalculation, we found that $cl(V(H)) = \{v_1, v_2\}$, not $\{v_1, v_2, v_3\}$ of Nada et al. ^[11]. Thus, both the claims of Nada et al. ^[11] are not true. Thus, their biological claims also have flaws while describing the biological phenomena regarding the circulation of blood in a heart.

Now, we shall discuss our observation regarding $int(H)$ and $cl(H)$ from our methods in terms of biological phenomena of the circulation of blood in a heart. If either $v_1$ or $v_2$ is in $H$, then $int(H)$ contains neither $v_1$ or $v_2$. It practically indicates that blood flows from both $v_1$ and $v_2$ but not from only anyone of theses two vertices. Moreover, the flow-path of blood splits from the vertex $v_5$ and blood reaches to $v_8$ either by passing through $v_6$ or $v_7$ or by both. Moreover, since the flow-path of blood splits from the vertex $v_5$, thus it is uncertain to us about $v_6$ or $v_7$. If both $v_1$ and $v_2$ is in $H$, then $int(H)$ contains both $v_1$ and $v_2$. If either $v_6$ or $v_7$ is in $H$, then $int(H)$ contains neither $v_6$ or $v_7$. It shows that uncertainty is present in the flow-path after reaching the vertex $v_5$. We observed that $int(H)$ in general contains those points which are either forming directed paths or only the isolated vertices (but not the starting points of blood flow, i.e., $v_1$ and $v_2$). Thus, $int(H)$ is assuring us the directed paths or isolated points which are important for a healthy heart functioning. We procure some examples. We have $int(\{v_1, v_2\}) = \emptyset$, which indicates that there is no vertex in $\{v_1, v_2\}$ which may need medical treatments to be cured if blood flows from both $v_1$ and $v_2$. Again, $int(\{v_1, v_2, v_3\}) = \{v_1, v_2, v_3\}$ and it indicates that if blood flows from $v_1$ and $v_2$, then it will reach to $v_3$, but the directed paths $v_1$ to $v_3$ and $v_2$ to $v_3$ are both needed to be functioned well for the well functioning of the remaining vertices. Interestingly, $int(\{v_1, v_3\}) = \{ v_3\}$ and $int(\{v_2, v_3\}) = \{ v_3\}$. These indicates that blood can't flow from only $v_1$ or $v_2$, we need both of them. But $v_3$ must be functioned well, if blood flows from $v_1$ or $v_2$ for the well functioning of the next points. Similarly, we can find that $int(\{v_2, v_3, v_5\}) = \{v_3, v_5\}$. Here, $v_2$ and $v_3$ are connected by a directed path and $v_5$ is an isolated vertex, if we consider a subgraph having three vertices $v_2, v_3, v_5$ and the directed edge $e_2$. Thus, $int(\{v_2, v_3, v_5\}) = \{v_3, v_5\}$ indicates that blood will pass through $v_3$ and $v_5$, but they should be well functioned for the well functioning of the remaining vertices, but since the blood flows from both $v_1$ and $v_2$, thus $v_2$ is excluded. Now, we show an interesting fact. We find that $int(\{v_2, v_3, v_5, v_6\}) = \{v_3, v_5\}$, $int(\{v_2, v_3, v_5, v_7\}) = \{v_3, v_5\}$, $int(\{v_2, v_3, v_5, v_7, v_8\}) = \{v_3, v_5, v_8\}$, $int(\{v_2, v_3, v_5, v_7, v_9\}) = \{v_3, v_5, v_9\}$, $int(\{v_2, v_3, v_5, v_7, v_9, v_{10}\}) = \{v_3, v_5, v_9, v_{10}\}$ and so on. One may observe that the flow-path of blood splits from $v_5$ and uncertainty is present between the paths to reach blood at $v_8$. Thus all the interiors do not contain anyone of $v_6$ or $v_7$, but we can observe that $int(\{v_2, v_3, v_5, v_6, v_7\}) = \{v_3, v_5, v_6, v_7\}$, $int(\{v_2, v_3, v_5, v_6, v_7, v_8\}) = \{v_3, v_5, v_6, v_7, v_8\}$, $int(\{v_2, v_3, v_5, v_6, v_7, v_9\}) = \{v_3, v_5, v_6, v_7, v_9\}$, $int(\{v_2, v_3, v_5, v_6, v_7, , v_9, v_{10}\}) = \{v_3, v_5, v_6, v_7, v_9, v_{10}\}$ and so on. Thus, it can be concluded that our methodology to define the subbase $\mathcal{P}_G$ and then finding interior of a subset has biological feasibility in case of the circulation of blood in a heart of human body.

Now, we discuss about closure from the same perspective of a heart. In case of the circulation of blood in a heart, $cl(H)$ indicates the flow-path of blood from the vertices of $H$ alongwith the starting vertices $v_1$ or $v_2$, if $v_3\in H$. Since, the flow-path splits after reaching $v_5$, thus $cl(H)$ will also reflect this biological phenomena of the heart via our methodologies. We only write closures of some subsets without discussing briefly. We find that $cl(\{v_1, v_2\}) = \{v_1, v_2 \}$, $cl(\{v_1, v_2, v_3\}) = \{v_1, v_2, v_3\}$, $cl(\{v_1, v_2, v_3, v_4\}) = \{v_1, v_2, v_3, v_4\}$, $cl(\{v_1, v_2, v_3, v_4, v_5\}) = \{v_1, v_2, v_3, v_4, v_5\}$, $cl(\{v_1, v_3, v_4, v_5\}) = \{v_1, v_2, v_3, v_4, v_5\}$, $cl(\{v_2, v_3, v_4, v_5\}) = \{v_1, v_2, v_3, v_4, v_5\}$, $cl(\{v_3, v_4, v_5\}) = \{v_1, v_2, v_3, v_4, v_5\}$, $cl(\{ v_4, v_5\}) = \{v_4, v_5\}$, $cl(\{ v_4, v_5, v_6\}) = \{v_4, v_5, v_6, v_7\}$, $cl(\{ v_4, v_5, v_7\}) = \{v_4, v_5, v_6, v_7\}$, $cl(\{ v_4, v_6\}) = \{v_4, v_6, v_7\}$, $cl(\{ v_4, v_7\}) = \{v_4, v_6, v_7\}$, $cl(\{ v_4, v_6, v_7\}) = \{v_4, v_6, v_7\}$ and so on. It is easy to check that both interior and closure explain the flow-path very well and accurately in comparison to Shokry and Aly ^[18], Nada et al. ^[11] and Nawar et al. ^[12].

6.   Conclusions

In this paper, we defined a new kind of topology named "pathless directed topology". This topological structure was defined over a digraph. Later, we proved some results connecting to Alexandroff space. We also established connections between pathless directed topology and connected digraph. Several properties were studied related to digraph isomorphicity. At the end, we proved that our methodologies describe the circulation of blood in a heart of human body more accurately than Shokry and Aly ^[18], Nada et al. ^[11] and Nawar et al. ^[12]. We also found that our methodologies of pathless directed topology is biologically feasible.

Acknowledgment

The authors extend their appreciation to the Deanship of Scientific Research at King Khalid University for funding this work through General Research Project under grant number (R.G.P.2/48/43).

The authors would also like to thank the Deanship of Scientific Research at Umm Al-Qura University for supporting this work by Grant Code: 22UQU4330052DSR05.

Conflict of interest

The authors declare that there is no conflict of interest.