1.
Introduction
Differential equations (DEs) are a powerful tool that can be used to model and understand a wide variety of systems. It plays a crucial role in solving real-world problems in many fields; see [1,2]. During the 20th century, the rapid progress of science resulted in applications across biology, population studies, chemistry, medicine, social sciences, genetic engineering, economics, and more. Many of the phenomena that appear in these fields are modeled using delay differential equations (DDEs). This led to many disciplines being elevated, and significant discoveries were made with this type of mathematical modeling.
The DEs that have the delayed argument in the highest derivative of the state variable are known as neutral differential equations (NDEs). NDEs have an extremely diverse historical background. In reality, they have a wide range of uses in natural science, as in the process of chemical reactions. Time-delayed transitions may be seen in chemical reaction kinetics, especially in complex processes. These kinetics are described by NDDEs; see [3]. The presence of the delay term in NDDs, which expresses the need for historical information, expands the solution space, and complicates numerical methods. This led to studying the qualitative behavior of these equations because finding closed solutions is often impossible due to their complexity. In recent years, there has been significant research focused on the asymptotic behavior of solutions to DEs; see [4,5,6]. By examining the asymptotic properties, researchers can forecast the future behavior of systems modeled by DEs from simple physical processes to complicated biological and economic systems. This part of the study supports the practical use of theoretical models in a variety of scientific and engineering domains in addition to aiding in their refinement; see [7]. In recent years, one of the most significant branches of qualitative theory has been oscillation theory; it was introduced in a pioneering paper of Fite; see [8,9,10]. This theory answers a lot of questions regarding the oscillatory behavior and asymptotic properties of DE solutions.
Finding adequate criteria to guarantee that all DE solutions oscillate while eliminating positive solutions is one of the main objectives of oscillation theory; see [11,12,13]. One of the main characteristics of oscillation theory is the variety of mathematical and analytical approaches it uses; see [14,15,16]. Over the past decade, there has been significant progress in the study of the oscillatory properties of DEs; see [17,18,19]. This interest stems from the fact that comprehending mathematical models and the phenomena they describe is made easier by examining the oscillatory and asymptotic behavior of these models; also, see [20,21,22]. Moreover, oscillation theory is abundant with fascinating theoretical problems that require the tools of mathematical analysis. In recent decades, oscillation theory has attracted the attention of many researchers, resulting in numerous books and hundreds of studies on several kinds of functional DEs; see [23,24,25]. Due to the critical roles of NDDE in various fields, such as civil engineering and application-oriented research that can support research with the potential to develop the ship-building, airplane, and rocket industries, the study of the oscillatory properties of these equations has advanced significantly. This makes them extremely important practically in addition to their abundance of interesting analytical problems. For more recent results regarding the oscillatory properties of NDDE solutions; see [26,27].
1.1. Fourth-order NDE
In this paper, we examine the oscillatory behavior of solutions to the neutral equation
where Ω(u)=x(u) + p(u)x(ζ(u)). Here, we accomplish our important results by considering the next conditions:
(H1) p,ζ∈C4([u0,∞)), 0<p(u)<p0<∞, ζ(u)≤u, limu→∞ζ(u)=∞, and ζ(u) invertible;
(H2) θ, q∈C([u0,∞)), q(u)>0, θ(u)≤u, limu→∞θ(u)=∞;
(H3) r(u)∈C1([u0,∞)), r(u)>0 and satisfy
By a solution of (1.1), we mean a function x∈C3([ux,∞)) for ux≥u0, which has the properties r(Ω′′′)∈C1([ux,∞)), and satisfies (1.1) on [ux,∞). We only take into account the solutions x of (1.1) that satisfy Sup{|x(u)|:u≥u∗}>0 for all u∗ ≥ ux.
Definition 1.1. [22] A solution of (1.1) is called oscillatory if it has arbitrarily large zeros on [u0,∞); otherwise, it is called non-oscillatory. Equation (1.1) is said to be oscillatory if all its solutions are oscillatory.
1.2. Literature review
The majority of studies have focused on establishing a condition that assures excluding increasing positive solutions using a variety of techniques. The oscillations of higher-order NDE have been investigated by many researchers, and many techniques have been presented for establishing oscillatory criteria for these equations. A lot of research has been conducted regarding the canonical condition; see [28,29,30]. We will now outline some of the results from previous papers that have contributed to an important part in advancing research on fourth-order NDEs, particularly Moaaz et al. in [31] established criteria for oscillation of solutions of NDDE
by applying two Riccati substitutions in each case of the derivatives of the corresponding function Ω. This criteria guarantees that all solutions oscillate under the canonical condition, where β≥α and 0≤p(u)<p0<∞.
In [32], Bazighifan et al. obtained the Philos type the oscillation criteria to ensure oscillation of solutions of the equation
and by employing the well-known Riccati transformation, they established an asymptotic criterion that enhances and supplements previous results, where 0≤p(u)<p0<1.
To comprehend the asymptotic and oscillatory behavior of solutions to NDEs, it is essential to understand the relationship between the solution x and its associated function Ω. Through this relationship, many researchers discovered several criteria that simplified and enhanced their earlier results. Here, we will present some of the relationships identified from previous research. For p(u)=p0, the conventional relationship:
is generally employed for second-order equations under the condition (1.2), while in [33,34] they applied the following relationship
in the non-canonical case. Moaaz et al. [35] in the canonical condition, they obtained some oscillation criteria for the next form of the equation
by optimizing the relationship (1.4), where α, β∈Q+odd. They provided for p0>1 the following relationship
for m is even, while for p0<1 they provided the relationship
for m is odd. In [36], Hassan et al. enhanced the relationship (1.5) by the next one
for m an odd integer, when examined, the oscillatory properties of the equation
where α∈Q+odd. In [37], Moaaz et al. improved the relationship (1.4) to the following
and they obtained a criterion to ensure that there are no Kneser solutions of the equation of third-order NDDE
by comparing (1.7) with a first-order DDE (comparison technique).
Moaaz and Alnafisah [38] examined the oscillatory behavior of solutions to DE
and derived inequalities and relationships, by enhancing the relationship (1.4) considering the two cases p0>1 and p0<1 without restrictions on the delay functions. Then, using an improved approach, they obtained new monotonic properties for the positive solutions.
Recently, Bohner et al. [39], by considering two cases ζ≤u and ζ≥u, studied the NDDE
and improved the relationship (1.5) by getting the next one
where
where k∈N.
In addition, recently, for higher-order some research improved the relationship of (1.4). Among these research, Alnafisah et al. [40] presented the following relationship:
by investigating the asymptotic and oscillatory behaviors of solutions to the NDEs
where n≥4 and α is the ratio of two positive odd integers.
The key to our contribution to this work, We categorize the positive solutions to the studied equation based on the signs of its derivatives. After that, we obtain new monotonic properties in certain cases of positive solutions. Based on these properties, we discover the relationship between the solution and its corresponding function Ω of our Eq (1.1) in the two cases p0>1 and p0<1. Additionally, we use these new relationships to exclude positive solutions by obtaining some oscillation criteria. The results are illustrated by an example. These results obtained extend and improve upon previous findings in the literature, providing a more comprehensive framework for analyzing these equations.
The paper is organized as follows: In Section 2, we present the fundamental notation and definitions that will be used in our proofs. In Section 3, we present a series of lemmas that enhance the monotonicity properties of nonoscillatory solutions. In Section 4, we establish oscillation criteria for (1.1) as our main result. Lastly, we illustrate our results with an example. In conclusion, briefly discuss what we have done in this research and the results we have obtained.
2.
Preliminaries
In this section, we will display the following constants and functions that are used in this paper. The class of all positive non-oscillatory solutions to (1.1) is denoted by the symbol S+.
Notation 2.1. For any integer k≥0. In order to present the results, we will need the following notation:
for i=1,2,....
Lemma 2.1. [41] Let ϝ∈Cκ([u0,∞),R+) and ϝ(κ) be of constant sign, eventually. Then there are a ux≥u0 and a j∈Z, 0 ≤j≤κ, with κ+j even for ϝ(κ)(u)≥0, or κ+j odd for ϝ(κ)(u)≤0 such that
And j≤κ−1 implies that (−1)j+lϝ(l)(u)>0 for u≥ux, l=j,j+1,.......κ−1.
Lemma 2.2. [42] Assume ϝ is stated in Lemma 2.1. If ϝ(κ−1)(u)ϝ(κ)(u)≤0, eventually, and limu→∞ϝ(u)≠0, then, there exists uk∈[u1,∞) for every ϵ∈(0,1), such that
Lemma 2.3. [43] If the Eq (1.1) has a solution x that is eventually positive, then
for k≥0.
Lemma 2.4. [41] If h∈Cκ([u0,∞),R+), h(i)(u)>0 for i=0,1,2,...,κ, and h(κ+1)(u)≤0, then eventually,
3.
Results and discussion
We will introduce the next lemma that describes the behavior of positive solutions.
Lemma 3.1. Assume that x∈S+. Then, eventually, we have two cases for Ω eventually:
Case (1)
Case (2)
Proof. Suppose that x is a positive solution of (1.1); we obtain Ω(4)(u)≤0 from (1.1). From the Lemma 2.1 Cases (1) and (2), and their derivatives, are obtained. □
Notation 3.1. We will refer to the symbol ℵ1 as the class of all eventually positive solutions of Eq (1.1) whose corresponding function satisfies Case (1) and ℵ2 as the class of all eventually positive solutions of Eq (1.1) whose corresponding function satisfies Case (2). Moreover, we will use the following notation throughout the proof of our lemmas.
Notation 3.2. For any positive integer k, we define the functions
and
and
For every k∈ N0, we assume that
It is clear that β∗k is positive. Our reasoning will usually rely on the obvious truth that a u1≥u0 is large enough such that for fixed but arbitrary βk∈(0,β∗k), we have
on [u1,∞).
3.1. Asymptotic and monotonic properties
This section contains several lemmas regarding the asymptotic properties of solutions that are part of the classes ℵ1 and ℵ2.
Lemma 3.2. Suppose that x∈S+. If Ω′′(u)>0 eventually, then,
(I) Ω(u)≥13uΩ′(u).
However, if Ω′′(u)<0, eventually, then
(II) Ω(u)≥ϵuΩ′(u), for ϵ∈(0,1).
Proof. Suppose that x is a positive solution of (1.1) and for u≥u1, Ω′′(u)>0. By applying Lemma 2.4 with F=Ω and κ≥3, we obtain
which gives (Ⅰ). Next, for u≥u1, Ω′′(u)<0. Then, u2>u1 exists, such that
which gives (Ⅱ), for all ϵ∈(0,1) and u≥u2. □
3.1.1. Properties in the case where p(u)≤p0<1
Lemma 3.3. Let β∗0>0 and x∈ℵ1. Then, for u large enough,
(A1) limu→∞r(u)Ω′′′(u)=limu→∞Ω(k)(u)/π2−k(u)=0, k=0,1,2;
(A2) Ω′′(u)/π0(u) is decreasing;
(A3) Ω′(u)/π1(u) is decreasing;
(A4) Ω(u)/π2(u) is decreasing.
Proof. Assume that x∈ℵ1. From the definition of Ω
we have
Since Ω(u)>x(u), Ω′(u)>0, and ζ(u)≤u, we have
which with (1.1) we have
(A1): Since we have Ω′′′(u) as a non-increasing and positive function, then
Assume ℓ>0; then r(u)Ω′′′(u)≥ℓ>0, by integrating three times
From (3.1) with R(u;0)=(1−p(u)) and (3.3) we obtain
From (3.4) into (3.5) we obtain
By integrating the above inequality from u3 to u, we obtain
which is
we find that there is a contradiction; therefore, ℓ=0. When x∈ℵ1, we have Ω(u)⟶∞, Ω′(u)⟶∞ as u⟶∞ and Ω′′(u)/π0(u)⟶0 as u⟶∞ such that Ω′′(u)>0 for k=2 is increasing, then by l'Hôpital's rule we find that (A1) satisfied.
(A2): As r(u)Ω′′′(u) is nonincreasing in ℵ1, we are able to say that
that is
Since Ω′′(u)>0, and r(u)Ω′′′(u) converges to zero by (A1), there exists u4>u3 such that
so we obtain
Therefore,
then Ω′′(u)/π0 is decreasing; that proves (A2).
(A3): From (A1) and (A2), we have Ω′′(u)/π0 decreasing and tending to zero, then we find
then we obtain
for u5>u4. Hence
from that we arrive at (A3).
(A4): Likewise, since Ω′(u)/π1(u) is decreasing and tends to zero, we obtain
then we arrive at
for u6>u5, so
that proves (A4). □
Lemma 3.4. Let β∗0>0 and x∈ℵ1. Then, the corresponding function Ω eventually satisfies
Proof. By using the facts that ζ[2i+1](u)≤ζ[2i](u)<u and Ω′(u)>0, we find that
By utilizing (Ω(u)/π2(u))′<0, we arrive at
this leads to
From this inequality in (2.1), we obtain
From this and (1.1) we obtain
This is completes the proof.
□
Lemma 3.5. Let β∗k>0 for some k∈N, and x∈ℵ1. Then, for u large enough, (A1)–(A4) (in Lemma 3.3) hold.
Proof. Replacing inequality (3.5) in Lemma 3.2 (Ⅱ) and proceeding in the same manner, we obtain properties in (A1)–(A4). □
Lemma 3.6. Assume that β∗k>0 and x∈ℵ2. If Ω′′(u)<0, eventually, then
Proof. Suppose that x∈ℵ2. For u≥u1, Ω′′(u)<0. From the facts Ω′(u)>0 and (II), we obtain
and
Then, Eq (2.1) becomes
which together with (1.1) gives (3.9).
□
Lemma 3.7. Let β∗k>0 for some k∈ N and x∈ℵ1. Then,
Proof. Follows from Lemmas 3.3, 3.6, and 3.4 with (1.1) gives (3.10). □
3.1.2. Properties in the case where p(u)≥p0>1
Lemma 3.8. Let x∈ℵ1∪ℵ2. Then there exists k such that
Proof. From the definition Ω(u), we find that
hence, we obtain
After k steps we arrive at there exists a k such that
This concludes the proof. □
Lemma 3.9. Let x∈ℵ1∪ℵ2. Then there exists k such that (1.1) implies,
When
Proof. Suppose that x∈ℵ1. From the fact that Ω′(u)>0 and from (I) in Lemma 3.2, we obtain
and
Then, from Lemma (3.8), Eq (3.11) becomes
in view of the fact that Ω(ζ[−2i](u))≥Ω(u), the above inequality becomes
which is together with (1.1), we obtain
Suppose that x∈ℵ2. For u≥u1, Ω′′(u)<0. From the facts Ω′(u)>0 and (II) in Lemma 3.2, we obtain
and
Then, from Lemma (3.8), Eq (3.11) becomes
which is together with (1.1), we obtain
Followed by (3.14) and (3.15) gives (3.13). □
4.
Oscillation theorems
In the following theorem, we will obtain oscillation criteria for Eq (1.1) in the case where p0<1.
For clarity, we will define that:
Theorem 4.1. Assume that there is ϵ∈(0,1) and p0<1 such that, if x∈ℵ1, then
and, if x∈ℵ2, then
where
Then, Eq (1.1) is oscillatory.
Proof. Assume the contrary, that (1.1) has a non-oscillatory solution x. Then, there exists a u1≥u0 such that x(u)>0, x(θ(u))>0, and x(ζ(u))>0 for u≥u1. There are two possible classes from Lemma 3.1: ℵ1 and ℵ2. Assume ℵ1 holds. From (3.8) in Lemma 3.4 we have
Introduce Riccati substitutions
We observe that ω(u)>0 for u≥u1; by differentiating (4.4), we obtain
from (4.3) we obtain
From (I) in Lemma 3.2, we have
then we obtain
by applying Lemma 2.2 for every ϵ∈(0,1), we obtain
Therefore, from (4.5)–(4.7), we obtain
which is
By integrating the above inequality, from u to∞, we obtain
since ω>0 and ω′<0, we have
which is
Let λ=infu≥u∗ω(u)/˜M1(u), then from (4.8) we notice
which contradicts thatλ≥1 in (4.8).
Assume ℵ2 holds. From (3.9) in Lemma 3.6 we have
By introducing Riccati substitutions
Integrating (4.9) from u to ∞, we obtain
From (II) in Lemma 3.2, we have
hence
By using (4.12) in (4.11), we obtain
by integrating again this inequality from u to ∞, we obtain
by differentiating ϖ(u) in (4.10), we obtain
for u≥u2.
Then, from (4.13) and (4.10), we obtain
by integrating the above inequality from u to ∞
from this we obtain
The rest of the proof is done as in the case ℵ1. As a result, the theorem is established. □
The next theorem provides two oscillation conditions for Eq (1.1), which require that p0>1. These conditions are established by using a comparison method with a first-order equation, under the next constraints: g(u)≤θ(u).
Theorem 4.2. Let p0>1 hold, if there exists ϵ∈(0,1) and k such that ρ2 and ˆρ2 are defined, such that the two first-order DDEs
and when g(u)≤θ(u)
where
are oscillatory, then (1.1) is oscillatory.
Proof. Assume the contrary, that (1.1) has a non-oscillatory solution x. Then, there exists a u1≥u0 such that x(u)>0, x(θ(u))>0, and x(ζ(u))>0 for u≥u1. There are two possible classes from Lemma 3.1: ℵ1 and ℵ2. Assume ℵ1 holds. Then from Eq (3.14) in Lemma 3.9, we have
From (4.7) and (4.16) we notice
If we set ψ(u)=r(u)Ω′′′(u), then ψ(u) is a positive solution of the first-order delay differential inequality
By [44, Theorem 1], the DDE (4.14) also has a positive solution; this leads to a contradiction.
Assume ℵ2 holds. Then from Eq (3.15) in Lemma 3.9, we have
Since g(u)≤θ(u), we find
by integrating (4.18) from u to ∞ we obtain
which is
Integrating (4.20) again from u to ∞, we obtain
From (II) in Lemma 3.2, we have
Let ν(u)=Ω′(u) and by using (4.22) in (4.21), we find that ν(u) is a positive solution of the inequality
But according to [44, Theorem 1], the condition (4.15) also has a positive solution ν(u); this leads to a contradiction. □
Corollary 4.1. If p0>1 such that
and
Then (1.1) is oscillatory.
5.
Applications and discussion
Example 5.1. Let the fourth-order NDE
where p0, q0 are positive and δ, β∈(0,1). We note that ζ−1(θ(u))=βuδ, r(u)=1. As a result, it is clear that ζ[−2i](u)=δ−2iu, ζ[−2i+1](u)=δ−2i+1u. Thus, for p0>1, we define
for k>0. Then, the condition (4.23) in Corollary 4.1, becomes
Also, we have
Then, the condition (4.24) simplifies to
which is
Using Corollary 4.1, Eq (5.1) is oscillatory if
Example 5.2. Now, consider the fourth-order NDE
where p0, q0 are positive, λ, μ∈(0,1), r(u)=1, θ(u)=μu, q(u)=q0u4, p(u)=p0 and ζ(u)=λu.
As a result, it is clear that π0(u)=u, π1(u)=u22, π2(u)=u36 and π2(ζ[2i](u))=(λ2iu)36, π0(ζ[2i](u))=λ2iu, then for p0<1, we define
and
By applying condition (4.1) in Theorem 4.1 when x∈ℵ1, we see
this implies
which get all solutions of (5.5) are oscillatory if
By applying condition (4.2) in Theorem 4.1 when x∈ℵ2, we see
which obtain all solutions of (5.5) are oscillatory if
Remark 5.1. Consider a special case of Eq (5.5) in the form
when talking ϵ=p0=0.5. By using our conditions (5.6) and (5.7), then Eq (5.8) is oscillatory if
By applying [32, Corollary 1], we see that
Then, by choosing θ(t)=t4 and ϕ(t)=t2, we find that (5.8) is oscillatory if
While [4, Theorem 2.1] ensures the oscillation of Eq (5.8) if
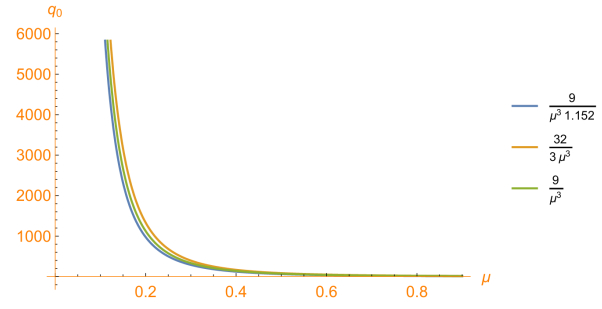
Figures 1 illustrates the efficiency of the conditions (5.9) in studying the oscillation of the solutions of (5.8) for values of μ∈(0,1). Thus, our results present a better criterion for oscillation.
6.
Conclusions
Finding conditions that exclude each of the cases of the derivatives of the positive solution is often the foundation of the idea of establishing oscillation criteria for differential equations. This study examines the oscillatory behavior of fourth-order NDEs in the canonical case. The relationship between the solution and the corresponding function is vital to the oscillation theory of NDEs. Therefore, we improve these relationships by applying the modified monotonic properties of positive solutions. The conditions that we obtained using these relationships subsequently proved that there are no positive solutions in categories ℵ1 and ℵ2. Then, using the newly deduced relationships and properties, we employed a number of approaches by using different techniques, including recatti and comparison techniques, to develop a set of oscillation criteria. Additionally, we provided examples that illustrated and clarified the importance of our results; they were compared with some previous results in the literature. In the future, we can try to develop new conditions that ensure that every solution of (1.1) is oscillatory in the noncanonical case.
Author contributions
H. Salah, C. Cesarano, and E. M. Elabbasy: Conceptualization, methodology, writing-original draft; M. Anis, S. S. Askar, and S. A. M. Alshamrani: Formal analysis, investigation, writing-review and editing. All authors have read and approved the final version of the manuscript for publication
Acknowledgments
The authors present their appreciation to King Saud University for funding this research through Researchers Supporting Project number (RSPD2024R533), King Saud University, Riyadh, Saudi Arabia.
Conflict of interest
The authors declare that there is no conflict of interest.









 DownLoad:
DownLoad:



