1.
Introduction
China's economic growth relies heavily on traditional fossil fuels, which has raised concerns among the governments about balancing economic development and environmental protection (Carlson et al., 2021; Su et al., 2023; Zhao et al., 2022). Because of the nature of the environment as a public good, the government plays an important role in environmental governance (Cheng and Xu, 2023). Extensive research has explored the relationship between fiscal support and green production. Fiscal subsidies can significantly promote corporate research and development investment and renewable energy production (Niesten et al., 2018; Sim, 2018; Yu et al., 2016). Investment subsidy programs have an incentive effect in promoting firms to absorb employment and expand renewable energy applications (Decramer and Vanormelingen, 2016). Government subsidies for energy-saving products are also beneficial in reducing carbon emissions and energy consumption (Yi and Li, 2018).
However, existing studies have not focused on the relationship between fiscal policy and green finance. In the context of a modern financial economy, financing capacity is becoming increasingly important for green governance (Qin et al., 2023; Zhang et al., 2023). Since the central bank established green credit (GC) services in local banks in 2007, GC has steadily risen and become China's largest green financial product (Xu and Li, 2020). GC is designed to encourage companies to invest in renewable energy and pollution control by providing financial incentives. Banks evaluate environmental governance performance based on the information disclosed by the company and then allocate the GC quota accordingly (Xu, 2020; Xu and Li, 2020).
However, existing studies have suggested that banks and enterprises lack incentives to participate in GC (Aizawa and Yang, 2010; Zhang et al., 2011). Due to information asymmetry, it is difficult for banks to be accurately informed of the business situation of enterprises (Ma et al., 2023). Banks tend to be cautious in GC services out of liquidity and risk considerations (Wen et al., 2021). Moreover, to improve the efficiency of social capital applied to environmental governance, the Chinese government introduced an environmental regulation policy for GC in 2012 called GC Guidelines (Hu et al., 2021). The policy asked banks to strengthen the assessment of the environmental risks of enterprises when granting credit, thus raising the threshold for obtaining credit financing (Liu et al., 2019; Xu and Li, 2020). Neoclassical economic theory suggests that environmental regulation will increase corporate compliance costs, thus limiting their ability to invest and green innovation (Gollop and Roberts, 1983; Wagner, 2007; Xu et al., 2022). In the presence of credit pressure, some enterprises may discharge waste illegally despite the risk of being penalized (Xu and Kim, 2022). Environmental regulation will substantially increase the costs of environmental governance for high-polluting enterprises and restrict their financing capacity (Yu et al., 2022). Therefore, GC may pose a paradox, as the needier the enterprises are, the harder it becomes to acquire GC (Ma et al., 2023).
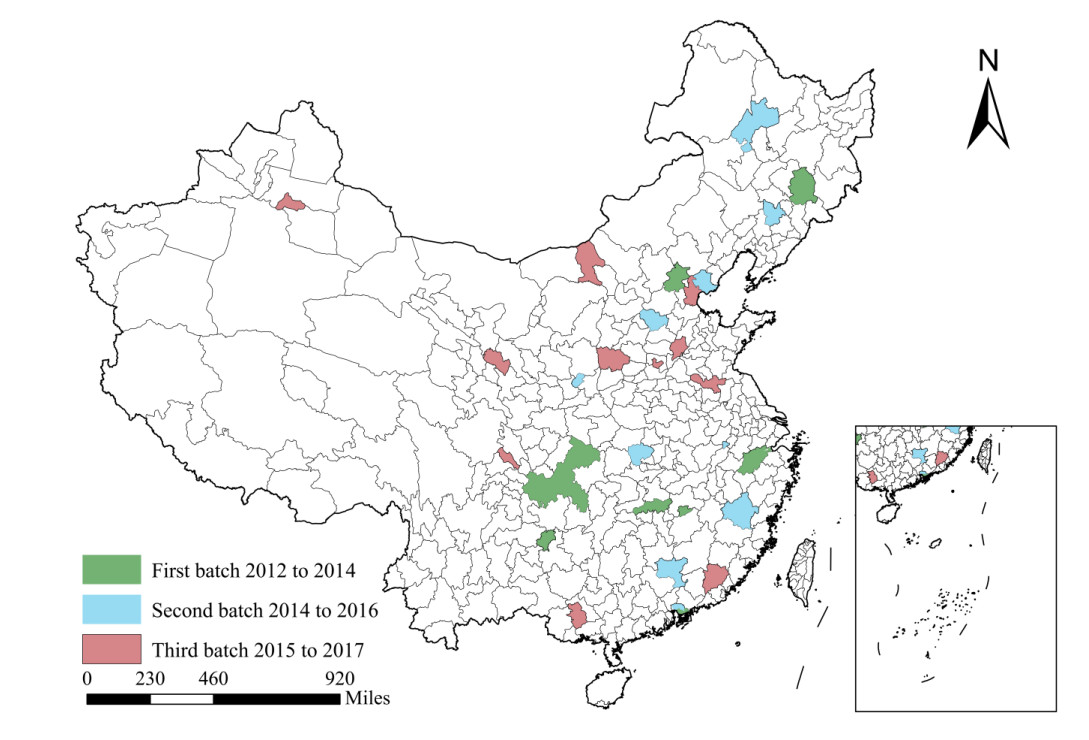
Therefore, it is necessary to explore the role of government support in addressing the information asymmetry and enterprise credit pressure. This study takes the instance of a fiscal policy implemented in China for energy conservation and emission reduction (ECER) to explore its impact on corporate GC. The policy aimed to establish ECER pilots in a total of 30 cities in three batches over the period from 2012 to 2017. Each batch of pilots lasted three years and was supported by funds from the Ministry of Finance. The program aimed to eliminate outdated production facilities by encouraging the priority development of renewable energy. According to the regional environmental governance performance, the central government allocated 400 to 600 million yuan per year to each city for the ECER project. A detailed distribution of the demonstration cities is presented in Figure 1.
In this paper, based on the staggered difference-in-difference (DID) model considering policy withdrawal, the effects of fiscal policy on enterprise GC and the mechanisms are explored by adopting the construction of ECER demonstration cities as a quasi-natural experiment. The main finding is that the scale of corporate GC in the ECER demonstration cities has increased significantly compared to the non-demonstration cities. This paper also explores the determinants and mechanisms. Government subsidies provide direct financial incentives for firms to participate in GC. Moreover, government participation also helps to avoid information asymmetry between firms and banks and alleviates corporate financing constraints. Lastly, this paper examines the policy effect heterogeneity from different aspects. It is found that the enterprises belonging to the late-intervention batches, high environmental awareness cities, high-polluting industries, and non-state-owned enterprises have better GC performance.
Compared with existing studies, the contributions of this study mainly have the following aspects. First, extensive research has discussed the relationship between fiscal policy and corporate cleaner production and concluded that government subsidies and regulations help to encourage enterprises to promote green production technologies and achieve emission reductions (Wang and Qiu, 2021; Yi and Li, 2018; Yu et al., 2016). However, few studies have explored the impact of government involvement on corporate financing behavior. This study is the first to examine the role of fiscal policy on corporate GC and its underlying path. The results and findings of this paper suggest that government participation is conducive to alleviating information asymmetry in the financing market. Moreover, fiscal subsidies also provide direct financial support for corporate financing. Therefore, this paper contributes empirical evidence for a better understanding of the role of the government in the GC market.
Second, this paper considers an econometric issue that may lead to biased estimates in the staggered DID identification strategy. Extensive literature only estimated the staggered DID model based on the two-way fixed-effect (TWFE) model (Agyeman and Lin, 2023; Bao et al., 2023; Beck et al., 2010). However, some recent literature has shown that the estimates of the TWFE model may be biased owing to the presence of heterogeneous treatment effects. The problem of "bad control groups" causes the results of parallel trend tests to be unreliable (Athey and Imbens, 2022; Goodman-Bacon, 2021). For this reason, this paper simultaneously adopts several popular DID estimators that consider heterogeneous treatment effects to enhance the robustness of the conclusions.
Lastly, current research on fiscal policy rarely considers enterprise performance after policy withdrawal. Recent studies have found that some enterprises will implement temporary green governance to obtain fiscal support (Shi et al., 2023; Tatomir et al., 2023). Therefore, the long-term effects of the policy and the enterprise performance may be uncertain. A successful fiscal policy should exhibit leading and long-term effects rather than treating fiscal subsidies as corporate non-operating income (Cheng et al., 2023). By applying a recently proposed DIDM estimator (De Chaisemartin and D'Haultfoeuille, 2020), the long-term effects after policy withdrawal are also explored in this paper. The estimate of policy withdrawal effects also provides a more comprehensive assessment of the role of the ESER demonstration cities policy.
The remainder of the paper has the following structure: Section 2 presents the theoretical analysis and main hypotheses. Section 3 provides the research design while offering solutions to two estimated problems of our interest: the heterogeneous treatment effects and the policy withdrawal effects. Section 4 is the empirical results. Section 5 reports the conclusions and discussion, and policy implications are presented in Section 6.
2.
Theoretical analysis and hypothesis
Corporate cost theory suggests that increased operating costs will limit corporate investment and financing options by reducing corporate cash flow and raising repayment risk (Kouvelis et al., 2019; Liu et al., 2023). The increase in pollution control costs will have a "crowding-out effect" on the investment of productive activities under environmental regulations (Jorgenson and Wilcoxen, 1990), thus resulting in lower demand for GC. Unlike environmental regulations, fiscal policies alleviate internal capital constraints and provide financial support for repayment by directly expanding capital sources through subsidies, tax rebates, and government investments (Gonzalez and Pazo, 2008; Xie et al., 2019).
The ECER demonstration cities policy encourages environmental governance mainly through fiscal grants. The central government first pays environmental governance funds to local governments. Then, local governments establish environmental governance projects and provide enterprises with cash and asset subsidies. In addition, fiscal appropriations are not transferred at once but dynamically adjusted based on the completion of green governance projects through an assessment system. Suppose a project does not meet the acceptance criteria; the central government will withdraw the fiscal appropriations or even reclaim past subsidies. The combination of supervision and incentives will help regulate corporate behavior and guide enterprises to utilize subsidies provided for environmental governance, thus expanding GC financing (Liu et al., 2021; Zhang et al., 2020).
Hypothesis 1. The ECER demonstration cities policy promotes corporate GC through fiscal subsidies.
From the perspective of short-term operating goals, the long return cycle, high risk, and uncertain benefits of green spending do not meet the short-term corporate profitability objective. As the main instrument to compensate for market failures, fiscal policy provides government support for corporate green spending (Bi et al., 2016; Huang et al., 2019). Moreover, the stakeholder theory argues that enterprise operations should consider stakeholders' requirements regarding long-term operating strategies (Barney and Harrison, 2020; Friedman and Miles, 2002). Corporate activities should be consistent with shareholders' profitability growth objectives and climate and environmental governance, satisfying public and government interests. Expanding green spending helps to obtain short-term subsidies while shaping the corporate social figure and enhancing government trust in enterprises (Freudenreich et al., 2020). Fiscal policy is conducive to establishing long-term strategic plans and reinforcing confidence in green spending.
The issuance of GC is based on the assessment of corporate pollution governance and green spending. Commercial banks grant credit to enterprises that are more favorable to environmental protection by using differentiated pricing (Nandy and Lodh, 2012). The long- and short-term goals of corporate green spending tend to be unified with fiscal support, thus releasing more positive environmental governance information to commercial banks and increasing the quota of bank credit (Forcadell et al., 2020).
Hypothesis 2. The ECER demonstration cities policy promotes corporate GC by increasing enthusiasm for green spending.
Corporate financing constraints are caused by external financing exceeding internal asset costs under information asymmetry (Kaplan and Zingales, 1997). Asymmetric information theory argues that one party holds more information and the other party lacks confidence in the transaction owing to moral hazard consideration. Asymmetric information will eventually lead to welfare loss for both sides (Oliner and Rudebusch, 1992). This phenomenon is prevalent in modern finance (Myers and Majluf, 1984). In many emerging markets and developing countries, frauds and bad debts are formed mainly due to asymmetric information between banks and firms. Enterprises with more private information than banks intend to apply for a higher credit line by overstating their operational capabilities. However, banks intentionally raise the credit threshold for fear that enterprises may send fake information. The game between the two sides eventually leads to harsh credit conditions and a shrinking credit market (Fazzari et al., 1988; Pawlina and Renneboog, 2005).
The ECER demonstration cities policy provides an implicit government guarantee mechanism for enterprises to obtain GC lines. With the help of government credibility, corporate fraud is restrained while sending credible market signals to commercial banks (Hong et al., 2020; Wu, 2017). Therefore, under fiscal support, the engagement of governments as a third party will help alleviate enterprise financing constraints.
Hypothesis 3. The ECER demonstration cities policy promotes corporate GC by alleviating financing constraints.
Combining Hypotheses 1-3, this study proposes Hypothesis 4:
Hypothesis 4. The ECER demonstration cities policy has a positive effect on enterprise GC.
A pilot policy is usually a trial-and-error tool before the policy is fully rolled out. The actual effects of a pilot policy are often unknown and uncertain before implementation (Cheng et al., 2023). The ECER demonstration cities policy provides a rich experience for policy evaluation and optimization through a batch-based strategy. Therefore, the effects of different batches may be different.
The market positioning theory argues that a firm's product supply and development strategies should be considered in light of market demand preferences (Cui et al., 2017; Songailiene et al., 2011). There may be differences in consumer concern for environmental governance in different regions, leading to differences in investment and financing decisions.
Based on the output theory, the disposability of different outputs is asymmetric, implying that environmental governance sacrifices more potential capacity for high-polluting firms (Fare et al., 1989). Therefore, the same intensity of fiscal intervention may have different impacts on different industries.
Finally, the property rights theory argues that clearly defined property rights are more conducive to solving the externality problem (Coase, 1981). Non-state-owned enterprises (non-SOEs) usually have clearer property rights than state-owned enterprises (SOEs) and are more sensitive to policy interventions and cost changes (Tan et al., 2022).
Hypothesis 5. The impact of the ECER demonstration cities policy on GC may vary at the batch, regional, industry, and enterprise levels.
3.
Research design
3.1. Model settings
Existing studies mostly measured fiscal policy using proxy variables (Yi and Li, 2018; Yu et al., 2016). The endogeneity problem of such an empirical strategy will seriously affect the robustness of the estimated results (Cheng and Xu, 2023). Since the DID identification strategy can estimate the "net effect" of policy interventions more accurately, it is widely adopted in policy assessment (Lee and Nie, 2023). This study leverages the staggered implementation of the ECER demonstration cities policy in three batches from 2012 to 2017 to conduct the research design. The policy intervention is considered a quasi-natural experiment, and a DID identification strategy is adopted to estimate causal effects. The strategy compares the differences in the sizes of enterprises' GC between demonstration cities and non-demonstration cities before and after the policy implementation (Heckman and Robb, 1985; Sloczynski and Wooldridge, 2018). In the baseline regression, the following TWFE model is developed:
where GCit represents the GC size of enterprise i in year t, Policyit represents the core explanatory variable, and Controlit is the relevant control variable. λi and μt indicate enterprise and year fixed effects, respectively. εit indicates the random error term that is subject to identical independent distribution. We use ordinary least squares (OLS) to estimate Equation (1), and cluster standard errors are set at the industry level.
3.2. Variables and data
3.2.1. Variables and definitions
Explained variable: We use the share of GC of the total credit of a firm to measure (GCit).
Core explanatory variable: ECER demonstration cities (Policyit). If the city to which firm i belongs is selected as a demonstration city in year t, then Policyit=1; otherwise, Policyit=0.
Control variables: Referring to existing studies (Chen et al., 2021; Tan et al., 2022), the control variables mainly reflect the characteristics of city and enterprise levels. At the city level, this study considers socio-economic and environmental variables that may affect the level of GC, including GDP per capita (PGDP), natural population growth rate (POP), and the overall utilization rate of general industrial solid waste (USW). At the enterprise level, this study mainly selects relevant indicators that reflect enterprises' operating performance and financial status. These include enterprise size, measured by total assets (SIZE); operating and development performance, measured by total assets growth rate (TAGR) and return on assets (ROA); and investment and debt payment capability, measured by investment expenditure rate (INV) and total debt rate (TDR).
3.2.2. Data and descriptions
As GC was launched in 2007, and the ECER demonstration cities policy ended in 2017, the listed enterprise data for 285 cities from 2007 to 2017 is used as the dataset for the benchmark regression. GC and other enterprise data are from annual reports of listed enterprises and the CSMAR database. City data are available from the China City Statistical Yearbook.
To adapt them to the study of this paper, the following preliminary treatments were performed on the listed company samples: (1) All special treatment enterprises (ST), special treatment enterprises with the risk of termination of listing (*ST), and particular transfer enterprises (PT) were excluded. Because of abnormal cash flow and operating losses, these are usually not the target enterprises for GC. (2) All banking and securities industries were excluded as these enterprises are only the issuing and managing sectors of GC. (3) All observations of continuous-type variables at the 1% and 99% quantiles were excluded to avoid the interference of outliers on the robustness of the estimates.
As each batch of demonstration cities lasts only three years, the research design of this paper also has a policy withdrawal problem. Specifically, there was no policy intervention for the first batch in 2015 and later, and there was no policy intervention for the second batch in 2017 and later. Therefore, we cannot directly define the treatment and control groups as in a common DID identification strategy. This paper proposes the following two solutions: (1) There is directly excluding the observations of the first batch in 2015 and later and the observations of the second batch in 2017, thus transforming the data structure into a standard staggered DID version. The scheme was initially adopted in the benchmark regression and proved generalizable to other problems of our interest by subsequent robustness tests. (2) This study still wants to capture differences between the treatment and control groups after policy withdrawal, which has important implications for assessing policy effects. For this purpose, a recently proposed DIDM estimator that proved robust to the policy withdrawal problem is introduced. The detailed application strategy is described in Section 3.3. The descriptive statistics of the dataset used for the benchmark regression are shown in Table 1.
3.3. Limitations of TWFE models and solutions
For the general single-point-in-time 2 × 2 DID model, parallel trends are the core assumption for the estimate by applying the TWFE model similar to Equation (1). However, in the case of staggered DID with policy withdrawal that we are addressing, the TWFE regression may be a biased estimator. The estimate obtained is a weighted average of all possible 2 × 2 DID components between groups treated at different points in time (Goodman-Bacon, 2021). This paper solves both the "bad control group" and policy withdrawal problems by adopting the following strategies that have been proven to be effective:
This study uses the cohort-specific average treatment effect on the treated (CATT) proposed by Sun and Abraham (2021) and the imputation estimator proposed by Borusyak et al. (2021). Both methods address the heterogeneous treatment effects by preventing "bad control groups" from entering the estimation.
This study uses the DIDM estimator proposed by De Chaisemartin and D'Haultfoeuille (2020). The dataset of the benchmark model is expanded, and two new datasets, 2007 to 2017 and 2007 to 2019, are used to compute the DIDM estimator. This approach estimates the average treatment effect of policy transitions, effectively addressing the heterogeneous treatment effects while helping us study the impact of policy withdrawal.
As a straightforward and effective method, this study also shuts down the 2 × 2 DID components from the newly treated and already treated groups in the heterogeneity tests (Callaway and Sant'Anna, 2021). This will help to estimate the standard 2 × 2 DID version of each batch of policy interventions separately.
3.4. Other robustness issues
This study also provides a placebo test and PSM-DID test for the problem of unobservable factor interference and self-selection in the DID identification strategy. See Section 4.2.3 and Section 4.2.4 for details.
The complete study design of this paper is shown in Figure 2.
4.
Empirical research
4.1. Benchmark results
Table 2 reports estimates of β in the benchmark regression and presents that the ECER demonstration cities policy had a significantly positive effect on enterprise GC. Column (1) introduces year fixed effects to rule out the impact of unobservable factors that vary over time. The results show that the policy contributed to the expansion of GC at the 1% significance level. Columns (2) and (3) further introduce the control variables and enterprise fixed effects to exclude the impact of unobservable factors that vary over individuals. The estimates remain robust and show that firm GC in the demonstration cities has significantly increased by 0.2178.
The TWFE model can avoid the impairment of causal inference by unobservable effects (Braghieri et al., 2022). For instance, differences in macro energy supply and consumption structure do not vary with individuals. Differences in the attitudes of enterprise managers toward clean energy promotion and environmental protection do not change over time. Therefore, this study adopts column (3), the result that considers enterprise and year fixed effects, as the preferred model to serve as a reference value in subsequent tests.
4.2. Robustness tests
4.2.1. Event study figure
Common trends and homogeneity of treatment effects are core assumptions for obtaining unbiased estimators with the DID approach. This study first attempts an event study estimator based on the TWFE model, which may not be robust in staggered DID, and the model is built as follows:
Policyjit is the time variable relative to the year the demonstration policy is implemented. Policyjit=1 for enterprises in demonstration cities; otherwise, Policyjit=0. The previous policy implementation year is set as the base year.
To further consider the issue of heterogeneous treatment effects, the event study figure based on two popular robust estimators is provided. Finally, to address the policy withdrawal problem in this paper, the DIDM-based estimators are presented for two expansion samples.
Figure 3 reports the event study version of all estimators within the 95% confidence interval. All estimators show that the parallel trend assumption holds. The coefficients are all close to zero before the policy intervention and display no trend while increasing consistently after the policy intervention. The Borusyak, Jaravel, and Spiess (2021) estimator is significantly above zero in the first year of policy implementation (T+0). TWFE and De Chaisemartin and D'Haultfoeuille (2020) estimators are significantly larger than zero in the second year of policy implementation (T+1). The Sun and Abraham (2021) estimator is significant in the third year of policy intervention (T+2). In addition, treatment effects of DIDM estimators increase continuously after the policy withdrawal, implying a strong boost effect of the ECER demonstration cities policy. The increase in treatment effects after the policy withdrawal is mainly explained by the following: (1) There are still a return visit and evaluation from the central government after the withdrawal of the incentive. Enterprises must maintain the investment and promotion of renewable energy under supervision. (2) Policy incentives and the construction of supporting infrastructure are conducive to solving the problem of financial constraints and the difficulty of realizing ecological benefits in the early stages. This implies that fiscal policy can encourage follow-up investment and credit. (3) Policy intervention breaks the "path-dependence" of enterprises on traditional fossil energy and further enhances the preference for renewable energy and emission control.
4.2.2. Point estimates of robust estimators
Table 3 presents the point estimates of the three robust estimators mentioned above. The Borusyak, Jaravel, and Spiess (2021) and Sun and Abraham (2021) estimates are slightly smaller than our preferred model. Both DIDM estimates of De Chaisemartin and D'Haultfoeuille (2020) are larger than the preferred estimate due to persistent influences of the ECER policy. However, all point estimates are significant at the 95% level, indicating that the estimate in the preferred model is little influenced by heterogeneous treatment effects.
4.2.3. Placebo test
To further remove the effects of unknown variables on causal inference and improve the robustness of the result, a placebo test is adopted to identify the randomness of the intervention effect. We generated a "pseudo" policy variable with 500 repeated samples and then estimated them by benchmark model. Figure 4 illustrates the coefficients and kernel density distribution of the "pseudo" policy variable and indicates that all estimates are close to zero and far smaller than the preferred model. Thus, the estimated result is unaffected by stochastic factors.
4.2.4. PSM-DID test
The application of the DID model calls for excluding endogeneity caused by self-selection bias (Wang and Yi, 2021). In reality, however, the ability of a region to become an ECER demonstration city is influenced by the socio-economic level of the region, the energy structure and environmental performance, and government preferences. Such influence can permeate the enterprise level, thus making a significant difference between the treatment and control groups before the policy intervention. Therefore, according to the PSM-DID approach, this study re-matched the treatment and control groups with 1:1 neighbor matching. Table 4 presents the results of the new sample after matching and shows that the estimated coefficients remain significantly positive at the 1% significance level. Therefore, the conclusions in the benchmark regression are still robust.
In summary, all the results above are in support of Hypothesis 4.
4.3. Mechanism
4.3.1. Government subsidy
This section examines whether the ECER demonstration cities policy promotes corporate GC through government subsidies. Typically, government subsidies include direct cash subsidies and asset subsidies (Liu et al., 2021). Direct cash subsidies are measured by the share of cash subsidies of operating income, and the share of asset subsidies of total assets is used as the indicator of asset subsidies. Following Cheng et al. (2023) and Lee et al. (2023), a three-stage regression is used for the estimations, where the preferred model has given the first step of the regression.
Table 5 shows that the ECER demonstration cities policy promotes both types of subsidies. However, only cash subsidies can significantly promote corporate GC. The effect of asset subsidies is insignificant, and the coefficient value is negative (−0.0479). It may be explained that asset subsidies have a substitution effect on corporate GC. Enterprises that can obtain environmental governance production equipment directly from governments are no longer dependent on financing. However, the estimated effect of the policy on cash subsidies is 0.0524, while the regression coefficient for asset subsidies is only 0.0059. The result indicates that the ability of fiscal policy to provide asset subsidies is limited. Because of the positive externality of environmental governance, the government assumes the main responsibility for allocating relevant assets (Cheng and Xu, 2023). However, the results of this study find that the government is more interested in providing cash subsidies, making it inefficient in carrying out the government's duties.
4.3.2. Green spending
Enterprises' spending on environmental governance usually includes green expenses and green investments. Green expenses belong to the account of profit and loss, such as sewage charges and pollution control costs. Green investments belong to the account of assets, such as research and development of clean production equipment (Sueyoshi and Goto, 2009). This study uses the share of green expenses of management expenses and the share of green investments of total assets to measure them, respectively. Table 6 shows that the ECER demonstration cities policy promotes corporate GC through green expenses and investments. This result shows that fiscal policy can stimulate corporate environmental governance and increase green expenses, which has the same effect as environmental regulation (Gollop and Roberts, 1983). Moreover, fiscal policy can also promote corporate green investments and prevent the "crowding-out effect" caused by increased environmental costs (Jorgenson and Wilcoxen, 1990). Therefore, fiscal policy can work better on corporate GC.
4.3.3. Financing constraints
The FC index is used as a proxy for corporate financing constraints. The FC index ranges from 0 to 1. A larger value means a more serious financing constraint (Fee et al., 2009). Table 7 shows that the ECER demonstration cities policy can significantly improve corporate GC by reducing financing constraints. Under the influence of information asymmetry in the capital market, enterprises face an increasingly severe external financing environment. The findings suggest that fiscal intervention by governments is effective in enhancing trust between enterprises and banks and increasing the scale of GC by improving the external financing environment.
All the above results support Hypotheses 1–3.
4.4. Heterogeneity
4.4.1. Policy batch heterogeneity
The exploration of policy batch heterogeneity has a dual significance. Since the policy of the ECER demonstration cities spans six years and three batches from the beginning to the end, the policy specification may change over the duration. This paper focuses on the policy intensities and the corresponding differences in treatment effects between the early, middle, and later stages by transforming the staggered DID model into a standard 2 × 2 DID model. At the same time, as a complement to the robustness test, such a transformation will also shut down the 2 × 2 DID components from the newly treated and already treated samples, thus avoiding the appearance of negative weights in the treatment effects.
Table 8 reports the average treatment effects for each of the three batches before and after. The results show that the first batch is insignificant, the second batch is significantly positive at the 5% level, and the third batch is significantly positive at the 1% level. Moreover, the average treatment effect increases with the batches. In Figure 5, this study also provides an event study version of the three batches based on the TWFE estimator. It can be observed that the coefficients of all three batches prior to the policy intervention are close to zero, show no clear trend, and appear to have no significant differences from each other. However, after the policy implementation, the coefficients have a definite trend of increasing with the batches. All these statistical phenomena indicate that the policy intervention in the later period is significantly better than in the earlier period. The reason for this can be seen in two main aspects: (1) With the first batch of demonstration cities construction, the governments are inexperienced. The policy specification develops more reasonably and efficiently with the accumulation of practical lessons. (2) With the promotion of renewable energy and general environmental awareness, the demonstration cities policy is less difficult to implement and less resistant. In addition, there are actually only eight demonstration cities in the first batch, expanded to ten in the second batch and further expanded to twelve in the third batch. We cannot exclude that the policy is initially designed with a plan to advance from weak to strong intervention gradually.
4.4.2. Urban environmental awareness heterogeneity
The level of public concern about energy and environmental issues reflects a city's interest in green development (Ge et al., 2021; Zhang et al., 2023). With "environmental pollution" as the keyword, we calculated the frequency of public searches on Baidu, which is the largest search engine in China. This helps us construct an urban environmental awareness index. This study sets the boundary at the 50% quantile, and cities above the boundary are considered a high environmental awareness group, while cities below the boundary are considered a low environmental awareness group. Table 9 reports the subgroup regressions and shows that the ECER demonstration cities policy has only significantly promoted enterprise GC in the group with high environmental awareness. This study mainly considers the following reasons: (1) The environmental governance philosophy of the public. The public with higher environmental awareness is more willing to participate in the construction of green projects, thus enabling a relaxed credit atmosphere for enterprises. (2) Differences in consumer demand. Consumers with higher environmental awareness also have a stronger preference for green products (Cui et al., 2017). To provide goods and services that meet market demand, the enthusiasm of enterprises to be involved in GC and investment also increases.
4.4.3. Industry heterogeneity
Based on the Catalogue of Listed Enterprises for Environmental Verification Industry Classification and Management, the sample enterprises are classified by industry type into high-polluting industries1 and the opposite non-high-polluting industries for examining industry nature heterogeneity. Table 10 shows that only the policy interventions for high-polluting industries can significantly affect GC. There are two main reasons for this: (1) Due to regular assessment by the central government, local governments are forced to pay more attention to high-polluting industries. Therefore, more fiscal support is granted to high-polluting enterprises. (2) High-polluting enterprises have a heavier cost burden on environmental governance, and fiscal support is more effective in alleviating financial constraints. Therefore, with the same amount of subsidy, high-polluting enterprises are more sensitive to policy intervention.
1 The high-polluting industries include thermal power, iron and steel, cement, electrolytic aluminum, coal, metallurgy, chemicals, petrochemicals, building materials, paper, brewing, pharmaceuticals, fermentation, textiles, leather, and mining.
4.4.4. Enterprise heterogeneity
Table 11 examines the difference in the impact of policy intervention on SOEs and non-SOEs and shows that the ECER demonstration cities policy can only promote the scale of GC for non-SOEs. This paper cannot exclude that SOEs have other access to environmental governance funds since central planning control and administrative orders guide the operations of these enterprises (Wu et al., 2012). However, the results show that non-SOEs are more effective in using fiscal resources for GC financing. This suggests that the ECER demonstration cities policy is conducive to breaking the market monopoly of SOEs and providing non-SOEs with more opportunities for green development.
All the above results support Hypothesis 5.
5.
Conclusions and discussion
This study uses the construction of energy conservation and emission reduction (ECER) demonstration cities as a quasi-natural experiment to examine the effect of fiscal policy on the scale of GC for listed enterprises in China. The main findings are as follows:
(1) The ECER demonstration cities policy can significantly increase the size of corporate GC. The result remains robust after considering the heterogeneous treatment effects. The further test based on the DIDM estimator suggests that the intervention effect is persistent after policy withdrawal, which indicates that fiscal support has a long-term effect and can continuously realize GC diffusion. This result implies that the government can achieve greater environmental benefits with less investment through financial leverage. Furthermore, this study also finds that fiscal subsidies can continuously incentivize firms to increase related green spending, thus broadening the role of micro participants in environmental governance.
(2) The policy intervention works mainly through providing direct cash subsidies, corporate green spending, and the reduction of external financing constraints. It is beneficial for governments to take the lead in building green projects to reduce the costs of environmental governance and enhance the trust between enterprises and banks. Government subsidies expand the willingness and ability of enterprises to participate in green credit by alleviating their cost constraints, improving the efficiency of environmental governance, and reducing bank credit concerns. However, this study also finds that the government is not sufficiently interested in providing asset subsidies and helping enterprises improve their environmental governance facilities.
(3) The effects of policy intervention vary with the implementation batches, urban environmental awareness, industry types, and corporate property rights. First, it is a successful attempt to implement the pilot policy in batches. This strategy ensures that governments can get a richer experience of environmental governance by evaluating policy effects and reducing the cost of trial and error. Second, to adapt to regional market demand, enterprises located in cities with higher environmental awareness are more willing to engage in green investment and credit. Third, high-polluting enterprises and non-SOEs face higher green governance costs, which makes fiscal support more conducive to these enterprises.
6.
Policy implications
With the development of environmental governance, the contribution of financial instruments has become increasingly prominent. This study has important implications in exploring the role of fiscal policy in the development of green finance. First, the government should pay more attention to fiscal policy instead of relying too much on environmental regulations. The demonstration policy should be further extended to the whole country, and the government should provide more assistance to enterprise green financing. In addition, a major concern is that the central government has perhaps assumed excessive pressure on fiscal expenditures, as all funds are provided by the central government. Therefore, it is necessary to promote the role of local governments and to force them to actively participate in green governance through central supervision.
Second, the government should optimize its policy subsidy programs and develop relevant environmental industries by allocating government assets, thereby creating a more efficient social financing environment and reducing enterprise cost concerns. Moreover, as an authoritative organization, the government can disclose more credible corporate environmental information, thus guiding the flow of bank credit to qualified enterprises. Therefore, it is essential for governments to establish public environmental performance disclosure platforms to reduce the loss of benefits due to information asymmetry.
Third, local governments in different regions should strengthen communication and cooperation. The demonstration cities should share their experience in green financing with the non-demonstration cities, thus reducing the cost of trial and error and improving the efficiency of green credit promotion in the whole country. In addition, the government should also strengthen environmental advocacy and raise awareness of urban environmental protection.
Lastly, high-polluting enterprises and non-SOEs are more urgent in fiscal support for green financing due to the constraints of capital liquidity and enterprise performance. Therefore, the government should pay more attention to these enterprises and create a fairer market competition environment. The role of green finance in environmental governance can be realized more efficiently by encouraging all types of enterprises to participate in green credit.
Use of AI tools declaration
The authors affirm that no artificial intelligence (AI) tools were used in the creation of this work.
Conflict of interest
All authors declare no conflicts of interest in this paper.









 DownLoad:
DownLoad: