Accelerated modified inertial Mann and viscosity algorithms to find a fixed point of $\alpha -$inverse strongly monotone operators

1.   Previous contributions to the inclusion problem

Assume that $C$ is a nonempty closed convex subset of a Hilbert space $\gimel$. A self-mapping $T:C\rightarrow C$ is called nonexpansive if

for all $\kappa, \omega \in C.$ The set $F(T) = \{\kappa \in C:\ T\kappa = \kappa \}$ denote the set of fixed points of a mapping $T.$

In this paper, we discuss the following inclusion problem: Find $\tilde{ \kappa}\in \gimel$ such that

where $\Xi :\gimel \rightarrow \gimel$ is an operator and $\Pi :\gimel \rightarrow \{{2^{\gimel }\}}$ is a set-valued operator. There are many real-world applications to various mappings in the fixed point theory, for example, many problems can be revisited as: Convex optimization and feasibility problems, image restoration problems, and monotone variational inequalities (see ^[1,2,3]). To be more precise, some concrete problems in machine learning and the linear inverse problem can be modeled mathematically with this formulation.

The classical approach to problem (1.1) (which is denoted by $((\Xi +\Pi)^{-1}(0))$ is the forward-backward splitting method ^[4,5,6,7,8], which is presented as follows: $\kappa _{1}\in \gimel$ and

where $\tau > 0$ and $I$ is the identity mapping. In this visibility, the step containing $\Xi$ refers to the forward step and $\Pi$ is the backward step, but not the sum of $\Xi$ and $\Pi$. In special cases, this technique includes exciting results in the gradient method ^[9,10] and the proximal point algorithm ^[11,12].

In 1979, a strong splitting algorithms in a real Hilbert space were built by Lions and Mercier ^[13] as follows:

and

where $J_{\tau }^{\Omega } = (I+\tau \Omega)^{-1}.$ Mostly, the two kinds of algorithms (1.3) and (1.4) called a Peaceman-Rachford algorithm ^[7] and Douglas-Rachford algorithm ^[14], respectively. Generally, both algorithms are weakly convergent ^[15].

Another direction concerning with the problem (1.1) of two monotone and accretive mappings in Hilbert and Banach spaces, a stationary solution to the following initial value-problem:

can be rewritten as (1.1) when the governing maximal monotone $\Re$ takes the form $\Re = \Xi +\Pi$ ^[13]. In optimization, it often needs ^[4] to solve a minimization problem of the form

where $h, m:\gimel \rightarrow (-\infty, \infty]$ are proper (that is, the inverse image of a compact set is compact) and lower semi-continuous convex functions such that $h$ is differentiable with $L$ -Lipschitz gradient, and the proximal mapping of $m$ is

In particular, if $\Xi = \nabla h$ and $\Pi = \partial m,$ where $\nabla h$ is the gradient of $h$ and $\partial m$ is the subdifferential of $m$ which is defined by $\partial m(\kappa) = \{q\in \gimel :m(\omega)\geq m(\kappa)+\langle q, \omega -\kappa \rangle,$ for all $\omega \in \gimel \}$, therefore problem (1.1) becomes (1.5) and (1.2) becomes

where $\tau > 0$ is the step-size and $prox_{\tau m} = (I+\tau \partial m)^{-1}$ is the proximity operator of $m.$

The rest of the paper is organized as follows. Section 2 describes a compilation of previously existing algorithms related to the well-known Mann iteration and some of its modifications and extensions. Section 3 gives some preliminary lemmas and definition which are then used to derive the main results of Section 4. The new main strong convergence results and their associated iterative algorithms are given in Section 4. In particular, the so-called inertial CQ-projection algorithm and the so-called inertial shrinking CQ-projection viscosity algorithm.

2.   Compilation of exciting algorithms

The iteration $\kappa _{n+1} = T\kappa _{n} = ... = T^{n}\kappa _{0}$ is called a Picard iteration where $\kappa _{0}$ is a starting point. It is one of the simplest iterative methods, but it has a defect, that its convergence cannot be guaranteed even in the weak topology. To overcome this defect, Mann iteration algorithm is one of the effective ways for that, which generates iterative sequence $\{\kappa _{n}\}$ through the following convex combination:

For nonexpansive mappings, the iteration (2.1) is useful for solving the fixed point problem and provides a unified framework for different algorithms. Also it has shortcomings, although it is defined in a Hilbert space, under certain conditions, the iterative sequence $\{\kappa _{n}\}$ defined by (2.1) has only weak convergence. Previously, many attempts to obtain a strong convergence were presented in ^[16,17,18].

In 2001, a heavy ball method applied to inertial proximal point algorithm by Alvarez and Attouch ^[19]. This method under maximal monotone operators was introduced by Poylak ^[20] for proximal point algorithm. The algorithm takes the form

It was proved that if $\{\tau _{n}\}$ is nondecreasing and $\{\theta _{n}\}\subset \lbrack 0, 1)$ with

then algorithm (2.2) converges weakly to a zero of $\Pi$. In particular, the condition (2.3) is true for $\theta _{n} < 1/3$. Here $\theta _{n}$ is an extrapolation factor and the inertia is represented by the term $\theta _{n}(\kappa _{n}-\kappa _{n-1})$.

The concepts of single-valued, co-coercive and Lipschitz continuous operator $\Xi$ added to the inertial proximal point algorithm by Moudafi and Oliny ^[21] to built the following algorithm:

Via condition (2.3) a weak convergence result using algorithm (2.4) was obtained provided that $\tau _{n} < \frac{2}{L}$, where $L$ is a Lipschitz constant of $\Xi$. It is noted that for $\hbar _{n} > 0$ the algorithm (2.4) does not take the form of a forward–backward splitting algorithm, since operator $\Xi$ is still evaluated at the point $\kappa _{n}$.

Of course, strong convergence is much better than weak convergence because it is often much more desirable for academic researchers since the obtained convergence results are more efficient and robust in potential application.

A strong algorithm for modified Mann algorithm was presented by Nakajo and Takahashi ^[16], which is called CQ-algorithm:

for each $n\geq 0$ and $C$ is defined in the above section. They obtained the strong convergence of the sequence $\{\kappa _{n}\}$ to $P_{Fix(T)}\kappa _{0},$ provided that the sequence $\{\hbar _{n}\}$ is bounded above by $1$. For more good results of the CQ-algorithms of nonexpansive mappings, we highly mention to ^[22].

Motivated by the algorithm (2.5), Dong et al. ^[23] discussed a strong convergence result involving an inertial forward-backward algorithm for monotone inclusions: Let $\Xi :\gimel$ $\rightarrow \gimel$ be an $\alpha -$inverse strongly monotone operator and $\Pi :\gimel \rightarrow 2^{\gimel }$ be a maximal monotone operator such that $(\Xi +\Pi)^{-1}(0)\neq \emptyset.$ Let $\{\alpha _{n}\}\in < /p > < p > \mathbb{R} < /p > < p >$ and the sequence $\{\kappa _{n}\}\subset \gimel$ be generated by $\kappa _{\circ }, \kappa _{1}\in \gimel$ and for all $n\geq 1$

Recently, Kim and Xu ^[17] proposed the following modified Mann iteration algorithm based on the Halpern iterative algorithm ^[24] and the Mann iteration algorithm(2.1):

for some fixed point $\kappa \in C,$ where $\Im :C\rightarrow C$ is a nonexpansive mapping with $Fix(\Im)\neq \emptyset$ and $\{\alpha _{n}\}$, $\{\zeta _{n}\}$ are sequences in (0, 1). Under mild conditions the sequence $\{\kappa _{n}\}$ generated by (2.6) converges to a fixed point of $\Im.$ Many authors worked in this directions and obtained strong convergence for a fixed point under a appropriate conditions, see, ^{[25,26,27,28]}.

Chen et al. ^[18] generalized the results ^[24] by introducing a new modified Mann iteration algorithm by combining the viscosity approximation algorithm ^[29] and the modified Mann iteration algorithm ^[17]. They established strong convergence result under fewer restrictions. The above results were circulated to more general operators and wider Banach spaces such as quasi-nonexpansive, asymptotically quasi-nonexpansive and strict pseudo-contractions mappings, see for instance ^{[30,31,32,33,34,35,36]}.

Inspired by the contributions of ^[16,17,23], new algorithms by overlapping the concepts of inertial Mann forward-backward method, CQ-Shrinking projection method and the viscosity algorithm were obtained and strong convergence results under these algorithms were discussed. At the last, numerical results are discussed to present the applications and a good acceleration performance of our algorithms. Our results extend and unify a lot of papers in this direction like Kim and Xu ^[17], Chen et al. ^[18], Suzuki ^[37] and the paper cited ^[38,39,40].

3.   Necessary lemmas and definition

In this paper, we shall refer to $\{\kappa _{n}\}$ is a sequence in $\gimel,$ $"\rightarrow "$ is the strong convergence, $"\rightharpoonup "$ is the weak convergence and $P_{C}:\gimel \rightarrow C$ is the nearest point projection, that is for all $\kappa \in \gimel$ and $\omega \in C,$ $\Vert \kappa -P_{C}\kappa \Vert \leq \Vert \kappa -\omega \Vert.$ $P_{C}$ is called the metric projection. It's obvious that $P_{C}$ achieves the following inequality,

for all $\kappa, \omega \in \gimel$. In other words, the metric projection $P_{C}$ is firmly nonexpansive. Hence $\langle \kappa -P_{C}\kappa, \omega -P_{C}\omega \rangle \leq 0$ holds for all $\kappa \in \gimel$ and $\omega \in C$, see ^[41].

Lemma 3.1. ^[41] Suppose that $\gimel$ is a real Hilbert space. Then for each $\kappa, \omega \in \gimel$ and a real number $\mho$, we have

(i) $\left\Vert \kappa +\omega \right\Vert ^{2}\leq \left\Vert \kappa \right\Vert ^{2}+2\langle \omega, \kappa +\omega \rangle,$

(ii) $\left\Vert \mho \kappa +(1-\mho)\omega \right\Vert ^{2} = \mho \left\Vert \kappa \right\Vert ^{2}+(1-\mho)\left\Vert \omega \right\Vert ^{2}-\mho (1-\mho)\left\Vert \kappa -\omega \right\Vert ^{2}$.

Lemma 3.2. [42,Theorem 1.9.10,p. 39 and Theorem 2.2.13,p. 57] Suppose that $\gimel$ is a real Hilbert space and $\{\kappa _{n}\}$ is a sequence in $\gimel$. Then the following properties are fulfilled:

(i) If $\kappa _{n}\rightharpoonup \kappa$ and $\Vert \kappa _{n}\Vert \rightarrow \Vert \kappa \Vert$ as $n\rightarrow \infty$, then $\lim_{n\rightarrow \infty }\kappa _{n} = \kappa$; that is, $\gimel$ has the Kadec-Klee property.

(ii) If $\kappa _{n}\rightharpoonup \kappa$ as $n\rightarrow \infty$, then $\Vert \kappa \Vert \leq \liminf_{n\rightarrow \infty }\Vert \kappa _{n}\Vert.$

Lemma 3.3. ^[43] Let $C\neq \emptyset$ be closed convex subset of a real Hilbert space $\gimel$. Then for each $\kappa, \omega, \upsilon \in \gimel$ and $\eth \in \mathbb{R}$, the following set is closed and convex:

Lemma 3.4. ^[21] Let $C\neq \emptyset$ be closed convex subset of a real Hilbert space $\gimel$ and $P_{C}:\gimel \rightarrow C$ be the metric projection. Then

for all $\kappa \in \gimel$ and $\omega \in C.$

Lemma 3.5. ^[44] Let $T$ be a nonexpansive self-mapping of a closed convex subset $C$ of a Hilbert space $\gimel$. Then the mapping $I-T$ is demiclosed; that is, whenever $\{\kappa _{n}\}$ is a sequence in $C$ which weakly converges to some $\kappa \in C$ and the sequence $\{(I-T)(\kappa _{n})\}$ strongly converges to some $\omega$, it follows that $(I-T)(\kappa) = \omega$.

Definition 3.6. Suppose that $D(\Xi)\subset \gimel$ and $R(\Xi)\subset \gimel$ are the domain and the range of an operator $\Xi,$ respectively. An operator $\Xi$ is called:

1). monotone if

2). maximal monotone if it is monotone and its graph

is not a proper subset of one of any other monotone mapping,

3). $\beta -$strongly monotone if there exists $\beta > 0$ such that

4). $\alpha -$inverse strongly monotone (for short $\alpha$-ism$)$ if there exists $\alpha > 0$ such that

Lemma 3.7. ^[5] Let $\gimel$ be a real Hilbert space, $\Xi : \gimel \rightarrow \gimel$ be an $\alpha$-inverse strongly monotone operator and $\Pi :\ \gimel \rightarrow 2^{\gimel }$ be a maximal monotone operator. For each $\tau > 0,$ we define

then, we get

(i) For $\tau > 0,$ $F(T_{\tau }) = (\Xi +\Pi)^{-1}(0);$

(ii) For $0 < s\leq \tau$ and $\kappa \in \gimel$, $\left\Vert \kappa -T_{s}\kappa \right\Vert \leq 2\left\Vert \kappa -T_{\tau }\kappa \right\Vert.$

Lemma 3.8. ^[45] Let $\gimel$ be a real Hilbert space, $\Xi :\ \gimel \rightarrow \gimel$ be an $\alpha -$inverse strongly monotone operator and $\Pi : \gimel \rightarrow 2^{\gimel }$ be a maximal monotone operator. For each $\tau > 0,$ we have

for all $\kappa, \omega \in \gimel$.

4.   Inertial shrinking projection and CQ-Mann-Halpern with viscosity algorithms

According to the notions of inertial CQ and shrinking projection technique with the Halpern algorithm and viscosity algorithm, respectively, we build two new algorithms in this section and their strong convergence in a Hilbert space is discussed.

Theorem 4.1. (Inertial shrinking projection algorithm). Let $\gimel$ be a real Hilbert space, $\Xi :\gimel \rightarrow \gimel$ be an $\alpha -$ inverse strongly monotone operator, $\Pi :\gimel \rightarrow 2^{\gimel }$ be a maximal monotone operator such that $\Theta = (\Xi +\Pi)^{-1}(0)\neq \emptyset$ and $\{\alpha _{n}\}$ is a bounded sequence$.$ For given two sequences $\{\lambda _{n}\}$ and $\{\rho _{n}\}\ $in $(0, 1)$. A sequence $\{\kappa _{n}\}$ is generated by

for each $n\geq 1$ and $\kappa _{\circ }, \kappa _{1}\in \gimel,$ where $\Upsilon _{n} = (I+\tau _{n}\Pi)^{-1}(I-\tau _{n}\Xi).$ If the following hypotheses hold:

$(\blacktriangleright _{1})$ $\sum_{n = 0}^{\infty }\rho _{n} = \infty$ and $\lim_{n\rightarrow \infty }\rho _{n} = 0,$

$(\blacktriangleright _{2})$ $0 < \lim \inf_{n\rightarrow \infty }\tau _{n}\leq \lim \sup_{n\rightarrow \infty }\tau _{n} < 2\alpha,$

then the sequence $\{\kappa _{n}\}$ generated by (4.1) converges strongly to a point $\theta = P_{\Theta }(\kappa _{1}).$

Proof. We split the proof into the following steps:

Step (i). Show that $P_{C_{n+1}}\kappa _{1}$ is bounded for each $\kappa _{1}\in \gimel,$ $n\geq 1$ and $\Theta \subset C_{n+1}.$ It follows from the condition $(\blacktriangleright _{2})$ and Lemma 3.8 that $T_{\tau _{n}} = (I+\tau _{n}\Pi)^{-1}(I-\tau _{n}\Xi)$ is a nonexpansive mapping. Lemma 3.7 guarantees that $\Theta$ is closed and convex set, and Lemma 3.3, clarifies that $C_{n+1}$ is closed and convex, for all $n\geq 1.$

Let $p\in \Theta,$ we have

Furthermore, by Lemma 3.1 (ii) and Lemma 3.8, we can write

Also, by Lemma 3.1 (i), we have

Applying (4.2) and (4.3) in (4.4), and since $(1-\rho _{n}) < 1,$ we get

It is clear that $\Theta \subset C_{1} = \gimel$. Assume that $\Theta \subset C_{n}$ for some $n\geq 1$. Then $p\in C_{n}$ and by (4.5), we have for all $n\geq 1,$ $p\in C_{n+1}.$ Thus $\Theta \subset C_{n+1}$ for all $n\geq 1,$ that is, $P_{C_{n+1}}\kappa _{1}$ is well-defined.

Step (ii).Prove that $\{\kappa _{n}\}$ is bounded. Since $\Theta \neq \emptyset$, closed and convex subset of $\gimel,$ there is a unique $\wp \in \Theta$ such that $\wp = P_{\Theta }\kappa _{1}.$ This implies that, $\kappa _{n} = P_{C_{n}}\kappa _{1},$ $C_{n+1}\subset C_{n}$ and $\kappa _{n+1}\in C_{n}$ for all $n\geq 1,$ we can get

Further, as $\Theta \subset C_{n},$ we obtain

It follows by (4.6) and (4.7), that $\lim_{n\rightarrow \infty }\Vert \kappa _{n}-\kappa _{1}\Vert$ exists. This leads to $\{\kappa _{n}\}$ is bounded.

Step (iii).Fulfillment $\lim_{n\rightarrow \infty }\kappa _{n} = \theta.$ By the definition of $C_{n},$ for $m > n$, we observe that $\kappa _{m} = P_{C_{m}}\kappa _{1}\in C_{m}\subset C_{n}.$ From Lemma 3.4, we have

Apply Step (ii), we conclude that $\lim_{n, m\rightarrow \infty }\Vert \kappa _{m}-\kappa _{n}\Vert ^{2} = 0$. Thus $\{\kappa _{n}\}$ is a Cauchy sequence. Hence, $\lim_{n\rightarrow \infty }\kappa _{n} = \theta,$ as $n\rightarrow \infty$. As well as, we get

Step (iv). Prove that $\theta \in \Theta.$ It follows from the boundedness of the sequence $\{\alpha _{n}\}$ and (4.8) that

By (4.5), (4.8) and the condition $(\blacktriangleright _{1}),$ we get

Applying (4.8)–(4.10), we can write

By (4.3) and (4.11), we observe that

The inequalities (4.12) and (4.13) lead to

and

Now, we have

It follows from (4.14) and (4.15) that

Since $\liminf_{n\rightarrow \infty }\tau _{n} > 0,$ there is $\varepsilon > 0$ such that $\tau _{n}\geq \varepsilon$ and $\varepsilon \in (0, 2\alpha)$ for all $n\geq 1.$ Then by Lemma 3.7 (ii) and (4.16), we have

From (4.10), since $\kappa _{n}\rightarrow \theta,$ we also have $\omega _{n}\rightarrow \theta.$ Since $T_{\varepsilon }$ is a nonexpansive and continuous mapping, from (4.17), we have $\theta \in \Theta$.

Step (v). Show that $\theta = P_{\Theta }(\kappa _{1}).$ Since $\kappa _{n} = P_{C_{n}}\kappa _{1},$ and $\Theta \subset C_{n},$ we can get

Setting $n\rightarrow \infty$ in (4.18), we have

This show that $\theta = P_{\Theta }(\kappa _{1})$. The proof is finished.

Theorem 4.2. (Inertial CQ-projection algorithm) (ICQMHA). Assume that all requirements of Theorem 4.1 are met. Then the sequence $\{\kappa _{n}\}$ generated by

converges strongly to a point $\theta = P_{\Theta }(\kappa _{1}).$

Proof. The proof is divided into the following steps:

Step (1). Demonstrate that $\{\kappa _{n}\}_{n = 0}^{\infty }$ is well-defined for each $\kappa _{1}\in \gimel$ and for all $n\geq 1,$ $\Theta \subset Q_{n}\cap C_{n}$.

It is clear that by Lemma 3.3, $C_{n}$ is closed and convex subset of $\gimel$. Also, if we rewrite the set $Q_{n}$ as shown

we obtain that $Q_{n}$ is also closed and convex subset of $\gimel$. So $Q_{n}\cap C_{n}$ is closed and convex, for all $n\geq 1.$ Assume that $p\in \Theta$. By the same manner of Theorem 4.1, we have

Thus, $p\in C_{n}$ for all $n\geq 1,$ it implies that $\Theta \subset C_{n}$ for all $n\geq 1.$

For $n = 1$, we have $Q_{1} = \gimel$ and hence $\Theta \subset C_{1}\cap Q_{1}$. Suppose that $\Theta \subset C_{l}\cap Q_{l}$ for some $l\geq 1$. Since $\kappa _{l+1} = P_{C_{l}\cap Q_{l}}(\kappa _{1})$. Then we get

for each $b\in C_{l}\cap Q_{l}.$ Since $\Theta \subseteq C_{l}\cap Q_{l},$ and $p\in \Theta,$ we have

This leads to $p\in Q_{l+1}$, and hence $\Theta \subseteq Q_{l+1}.$ Hence, we get $\Theta \subseteq C_{l+1}\cap Q_{l+1}$. Based on the above results, $\{\kappa _{n}\}$ is well defined and $\Theta \subset C_{n}\cap Q_{n}.$

Step (2). Clarify that $\{\kappa _{n}\}$ is bounded. By Algorithm (4.19), we can write

This implies that, $\kappa _{n} = P_{Q_{n}}(\kappa _{1}).$ Since $\Theta \subset Q_{n},$ we get

Again, since $\kappa _{n+1}\in Q_{n},$ we have

It follows from (4.20) and (4.21) that $\lim_{n\rightarrow \infty }\Vert \kappa _{n}-\kappa _{1}\Vert$ exists. Hence $\{\kappa _{n}\}$ is bounded.

Step (3). Prove that $\lim_{n\rightarrow \infty }\Vert \kappa _{n+1}-\kappa _{n}\Vert = 0$. Since $\kappa _{n+1}\in Q_{n}$ and $\kappa _{n} = P_{Q_{n}}(\kappa _{1})$, it follows from Lemma 3.4 that

This implies that $\lim_{n\rightarrow \infty }\Vert \kappa _{n+1}-\kappa _{n}\Vert = 0$.

Step (4).Show that $\theta \in \Theta.$ Follows immediately by Step (iv) Theorem 4.1.

Step (5). Illustrate that $\theta = P_{\Theta }(\kappa _{1})$. By the same scenario of {Step (iv)} Theorem 4.1, we obtain that

where $\varepsilon \in (0, 2\alpha)$. The nonexpansivity of $T_{\varepsilon }$ yields,

From (4.22) and (4.23), we can obtain

Since $\{\kappa _{n}\}$ is bounded, there is a subsequence $\{\kappa _{n_{k}}\}$ of $\{\kappa _{n}\}$ such that $\kappa _{n_{k}}\rightharpoonup \kappa ^{\ast }$. This combines with (4.24) and by using Lemma 3.5, we obtain that $\kappa ^{\ast }\in F(T_{\varepsilon })$, that is, $\kappa ^{\ast }\in \Theta$.

Since $\theta = P_{\Theta }(\kappa _{1})$ and $\kappa ^{\ast }\in \Theta$, (4.20) and Lemma 3.2 (ii) imply that

Using the uniqueness of the nearest point $\theta$, we now see that $\theta = \kappa ^{\ast }$. We also have $\Vert \kappa _{n_{k}}-\kappa _{1}\Vert \rightarrow \Vert \kappa _{1}-\theta \Vert$ and from Lemma 3.2 (i), we get that $\kappa _{n_{k}}\rightarrow \theta$ as $k\rightarrow \infty$. Using again the uniqueness of $\theta$, we deduce that $\kappa _{n}\rightarrow \theta$ as $n\rightarrow \infty$.

This ends the proof.

If we replace $\kappa _{1}$ with $\Im (\kappa _{1}),$ where $\Im :C\rightarrow C$ is a contractive mapping in (4.1) and (4.19) we have the following inertial shrinking CQ-projection viscosity algorithms:

Theorem 4.3. (Inertial shrinking projection viscosity algorithm) Assume that all requirements of Theorem 4.1 are satisfied. Let $\Im :C\rightarrow C$ be a $\mu -$contraction with $\mu \in \lbrack 0, 1),$ that is $\left\Vert \Im \kappa -\Im \omega \right\Vert \leq \mu \left\Vert \kappa -\omega \right\Vert$ for all $\kappa, \omega \in C.$ Then the sequence $\{\kappa _{n}\}$ generated by

converges strongly to a point $\theta = P_{\Theta }(\kappa _{1}).$

Theorem 4.4. (Inertial shrinking CQ-projection viscosity algorithm) (ICQMVA). Suppose that all requirements of Theorem 4.1 are verified. Let $\Im :C\rightarrow C$ be a $\mu -$contraction with $\mu \in \lbrack 0, 1).$ Then the sequence $\{\kappa _{n}\}$ generated by

converges strongly to a point $\theta = P_{\Theta }(\kappa _{1}).$

Remark 4.5 If we neglect CQ-shrinking projection terms, then the proposed algorithms in this manuscript extend and improve the results of ^[38,39,40], Kim and Xu ^[17] (if $\alpha _{n} = 0$ and $\Upsilon _{n} = I$ (Identity mapping) in algorithms (4.1) and (4.19)), Chen et al. ^[18] (if $\alpha _{n} = 0$ and $\Upsilon _{n} = I$ in (4.25) and (4.26)) and Suzuki ^[37].

5.   Computational experiments

In this section, the numerical comparison between strong convergence of our algorithms and the modified inertial Mann Halpern and viscosity algorithms ^[46] are illustrated. Through numerical calculations we found that our methods accelerate and more effective than methods of ^[46]. The codes used here to obtain numerical results are the MATLAB codes run in MATLAB version 9.5 (R2018b) on Intel(R) Core(TM)i5-6200 CPU PC @ 2.30GHz 2.40GHz, RAM 8.00 GB.

For simplicity:

${\text (1)}$ For Tan et. al. ^[46] (shortly, MIMHA) (shortly, MIMVA);

${\text (2)}$ For our proposed algorithms (shortly, ICQMHA) (shortly, ICQMVA).

Example 5.1. For any nonempty closed convex set $C_{i} \subset \mathbb{R}^{n}$ for each $i = 0, 1, ..., m.$ We are now considering the convex feasibility problem of finding

Define a map $T : \mathbb{R}^{n} \rightarrow \mathbb{R}^{n}$ by

where $P_{C_i}$ $(i = 0, 1, \cdots, m)$ denotes the metric projection upon $C_{i}.$ Since $P_{C_i}$ $(i = 0, 1, \cdots, m)$ is nonexpansive, then the mapping $T$ defined by (5.1) is also nonexpansive. Moreover, we can see that

During this experiment, we use $C_{i}$ $(i = 0, 1, \cdots, m)$ as a closed ball with center $c_{i} \in \mathbb{R}^{n}$ and radius $r_{i} > 0.$ Thus $P_{C_i}$ can be determined as

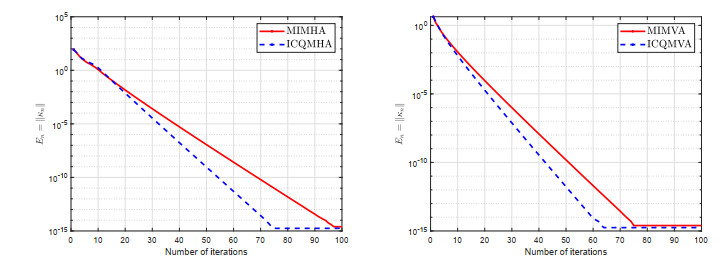
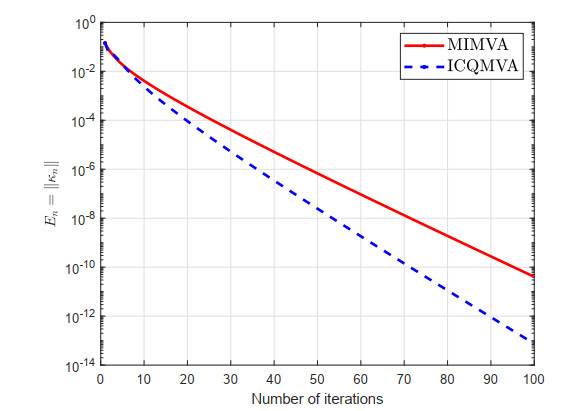
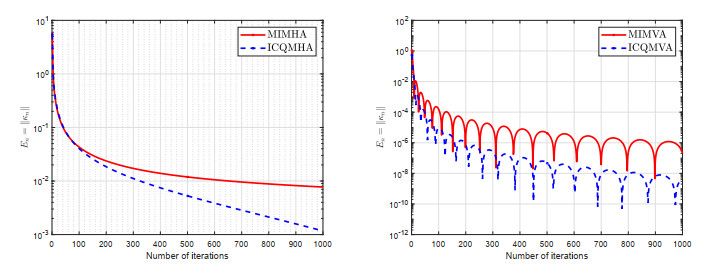
Choose $r_{i} = 1$ $(i = 0, 1, \cdots, m)$, $c_{0} = (0, 0, \cdots, 0),$ $c_{1} = (1, 0, \cdots, 0),$ and $c_{2} = (-1, 0, \cdots, 0).$ Moreover, $c_{i} \in \Big(\frac{-1}{\sqrt{n}}, \frac{-1}{\sqrt{n}} \Big)^{n}$ $(i = 3, 4, \cdots, m)$ are randomly chosen. From the choice of $c_{1}, c_{2}, r_{1}, r_{2},$ given that $Fix(T) = {0}.$ Moreover, we use $\kappa_{0} = \kappa_{1} = (1, 1, \cdots, 1),$ $\alpha_{n} = \frac{10}{(n+1)^{2}},$ $\eta = 4,$ $\lambda_{n} = \frac{1}{100(n+1)^{2}},$ $\rho_{n} = \frac{1}{n+1}$ and $f(\kappa) = 0.1\kappa_{n}.$ The numerical results are shown in Figures 1–2.

Example 5.2. Let $F:C\subset \mathbb{\gimel }\rightarrow \mathbb{\gimel }$ be an operator and the variational inequality problem is define in the following way:

Define $T:C\subset \mathbb{\gimel }\rightarrow \mathbb{\gimel }$ by

where $0 < \lambda < \frac{2}{L}$ and $L$ is the Lipschitz constant of the mapping $F.$ Let $F : H = \mathbb{R}^{2} \rightarrow \mathbb{R}^{2}$ defined by

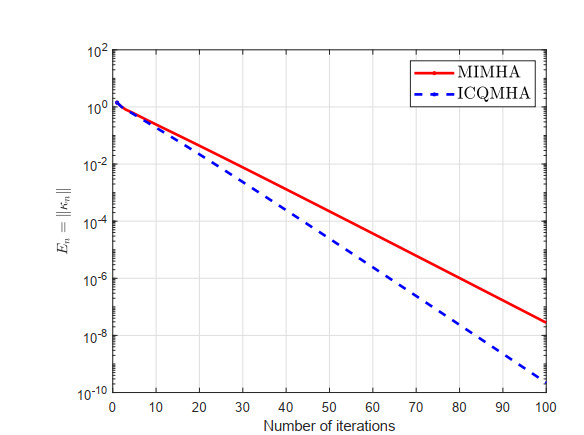
The authors in ^[47] showed that $F$ is Lipschitz continuous with $L = \sqrt{26}$ and strongly monotone. Therefore the variational inequality (5.4) has a unique solution (see, e.g. ^[48]) and $(0, 0)$ is its solution. We use $\alpha_{n} = \frac{10}{(n+1)^{2}},$ $\eta = 4,$ $\lambda_{n} = \frac{1}{100(n+1)^{2}},$ $\rho_{n} = \frac{1}{n+1}$ and $f(\kappa) = 0.1\kappa_{n}.$ The numerical results are shown in Figures 3–5.

Example 5.3. We assume that the Fermat-Weber (FW) problem, it is a well-known model of location theory. In mathematical terms, Fermat-Weber is described as follows:

in which $a_{i} \in \mathbb{R}^{n}$ are anchor points as well as $\omega_{i}$ were non-negative weights (see ^[49] for more details). The above problem can be described as fixed point problems as follows: A mapping $T : \mathbb{R}^{n} \rightarrow \mathbb{R}^{n}$ is defined by

where $\omega_{i} = 1$ for all $i.$ Moreover, we consider a three dimensional example with $n = 3$, $m = 8$ and collection $\Pi$ of anchor points are

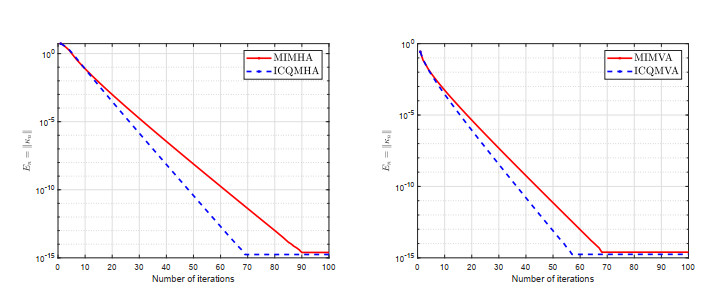
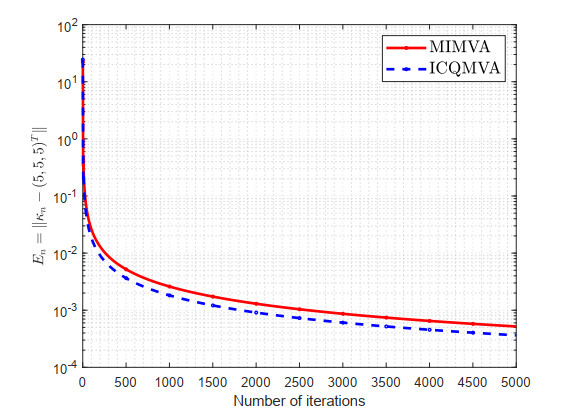
From above assumptions it follows that the optimal value of above problem is $\kappa^{*} = (5, 5, 5)^{T}.$ During this example, we use fixed element $\kappa_{1} = (1, 2, 3)^{T}$ and $\alpha_{n} = \frac{10}{(n+1)^{2}},$ $\eta = 4,$ $\lambda_{n} = \frac{1}{100(n+1)^{2}},$ $\rho_{n} = \frac{1}{n+1}$ and $f(\kappa) = 0.19\kappa_{n}.$ The numerical results are shown in Figures 6–7.

Example 5.4. Let $C = \{\kappa \in \mathbb{R}^{3} : \|\kappa\| \leq 1 \}$ be the unit closed ball and $T : C \rightarrow C$ ^[50] be defined by

We use $\alpha_{n} = \frac{10}{(n+1)^{2}},$ $\eta = 4,$ $\lambda_{n} = \frac{1}{100(n+1)^{2}},$ $\rho_{n} = \frac{1}{n+1}$ and $f(\kappa) = 0.1\kappa_{n}.$ The numerical results are shown in Figures 8–9.

6.   Advantages of our methods

In the study of algorithms, the efficiency and effectiveness of algorithms are measured by two main factors: The first is reaching the desired point with the fewest iterations possible, and the second factor is the time. When the time taken to obtain strong convergence is short, results are better. There is no doubt that the paper ^[46] addressed a lot of algorithms and proved, under suitable stipulation, that its algorithm accelerates better than the previous one. Here according to Examples 5.1–5.4, we were able to verify that our algorithm converges faster than the algorithm ^[46], so it converges faster than all the algorithms included in the paper ^[46]. Also, the numerical results (images) shows that our algorithms need a small number of iterations to get the desired target. This makes our method successful in speeding up the algorithm presented in Paper ^[46].

7.   Conclusions

In this manuscript, the strong convergence results for $\alpha$inverse strongly monotone operators under new algorithms in the framework of Hilbert spaces have been discussed and several algorithms have been developed. The proposed algorithms combine the inertial Mann forward-backward method with the CQ-shrinking projection method and viscosity algorithm. The main algorithms which are presented and discussed are so-called "Inertial CQ-projection algorithm" (ICQMHA) and "Inertial shrinking CQ-projection viscosity algorithm "(ICQMVA). It has been theoretically proved that our algorithms lead to an acceleration of the previous modified inertial Mann-Halpern and viscosity algorithms. Also, some numerical examples have been performed to illustrate the applications and to test the computational performance and its effectiveness of the proposed algorithms.

Acknowledgments

The authors thank the Basque Government for Grant IT1207-19.

Conflicts of interest

The authors declare that they have no competing interests concerning the publication of this article