Dynamical behaviors to the coupled Schrödinger-Boussinesq system with the beta derivative

1.   Introduction

It is clear that most of the events that occur in mathematical physics and engineering areas can be described by partial differential equations. The physical phenomena of nonlinear partial differential equations (NLPDEs) can connect to a lot of areas of sciences, for example, plasma physics, optical fibers, nonlinear optics, fluid mechanics, chemistry, biology, geochemistry, and engineering sciences ^[1].

Scientists have been used and improved many methods to obtain the analytic, semi analytic and numerical solution of (NLPDEs), such as shooting and Runga-Kutta fourth order technique ^[2,3], Adomian decomposition method ^[4], homotopy perturbation method ^[5], Adams-Bashforth-Moulton method ^[6], sine-Gordon expansion method ^[7,8], sinh-Gordon expansion method ^[9,10], an extended trial equation method ^[11], the degenerate Darboux transformation ^[12,13], the multiplier approach ^[14], the improved Bernoulli sub-equation function method ^[15,16,17], a modified simple equation method ^[18], method of undetermined coefficients ^[19], a functional variable method ^[20], the trial equation method ^[21], couple of integration schemes ^[22], lie symmetries along with $\left({}^{{{G}'}}/{}_{G} \right)$-expansion method ^[23], improved $\text{tan }\left(\phi \left(\xi \right)/2 \right)$-expansion method ^[24,25], inverse mapping method ^[26], Wronskian determinants ^[27], the simple equation method ^[28], tanh function method ^[29], the extended homoclinic test method ^[30], Jacobi elliptic function anzätz method ^[31], the decomposition-Sumudu-like-integral-transform method ^[32], hypothetical method ^[33], exp$\left(-\varphi \left(\xi \right) \right)$-expansion method ^[34,35], symbolic computational method ^{[36,37,38,39]}, the Thomson scattering ^[40], the Lie group analysis method ^[41], and Darboux covariant Lax pairs and Bäcklund transformations ^[42].

The fractional differential equation is a generalization of the classical integer differential equation that has many distinct advantages. In contrast to the classic integer derivative model, the fractional derivative model has more precise mathematical physical structure simulations ^[43]. Wang et al. ^[44] studied a wick-type stochastic fractional nonlinear Schrödinger equation and constructed its fractional optical solitons. Nabti and Ghanbari ^[45] presented a global stability analysis of a fractional SVEIR epidemic model. Ismael et al. ^[46] investigated the Lakshmanan-Porsezian-Daniel model include conformable fractional derivative. Lu et al. ^[47] used the fractional Riccati method and fractional bifunction method to study the fractional complex Ginzburg-Landau equation. Fang et al. ^[48] found discrete fractional soliton solutions of conformable fractional discrete complex cubic Ginzburg-Landau equation. Ghanbari and Kumar ^[49] offered the existence of chaos in a fractional predator-prey-pathogen model. Ghanbari ^[50] explored the dynamics of an eco-epidemiological system using a nonlinear fractional differential equation system. Yu et al. ^[51] used the fractional mapping equation method and fractional bi-function method to investigate a space-time fractional nonlinear Schrödinger equation, and exact to a suggested equation was driven by using the Mittag-Leffler function.

The Boussinesq equation was introduced by Boussinesq ^[52] to describe two-dimensional irrotational flows of an inviscid liquid in a uniform rectangular channel as well as it was the first equation proposed in the research paper to describe a large range of physical phenomena. The Schrödinger-Boussinesq system raised in laser and plasma physics also has been attracted by many mathematicians and physicists. Manafian and Aghdaei ^[53] used the improved $\tan \left({}^{\phi \left(\xi \right)}/{}_{2} \right)$ -expansion method and reported some exact solutions to the Schrödinger-Boussinesq system. Osman et al. ^[54] studied the variable-coefficients coupled Schrödinger-Boussinesq equation by using a unified method. MU and QIN ^[55] constructed rational solutions, breather, and the second-order rational solution by employing the Hirota technique. Bai and Wang ^[56] used the time-splitting Fourier spectral method for the coupled Schrödinger-Boussinesq equations and Ray in Ref. ^[57] used the time-Splitting Spectral Technique for the suggested system include Riesz fractional derivative. Banquet et al. ^[58] found the existence of local and global solutions for coupled Schrödinger-Boussinesq systems involving singular initial data. Liang ^[59] studied modulational instability and reported some stationary waves for the coupled generalized Schrodinger-Boussinesq system. Kılıcman and Reza Abazari ^[60] addressed travelling wave solutions of the Schrödinger-Boussinesq System via $\left({}^{{{G}'}}/{}_{G} \right)$-expansion method.

In this paper, we use the modified auxiliary expansion method to seek novel soliton solutions of the coupled Beta derivative of the Schrödinger-Boussinesq system that occur during the stationary propagation of coupled nonlinear magnetosonic waves and upper-hybrids in magnetized plasmas. The new solutions are presented as the family solution and expressed in hyperbolic, trigonometric, exponential and fractional function. Finally, the linear stability analysis and instability modulation Schrödinger-Boussinesq system are also presented.

This paper is organized as follows. In section 2, some basic definitions, properties, and the theorem about the Beta derivative are given. The structures of the modified auxiliary expansion method are given in section 3. Soliton solutions are constructed for coupled Schrödinger-Boussinesq system with Beta derivative in section 4. Linear Stability Analysis of coupled Schrödinger-Boussinesq system is presented in section 5. In section 6, we provide a conclusion to the studied system.

2.   The beta derivative

In this section, we introduce some basic definitions, properties, and the theorem about the beta derivative of a function of order $\alpha$ ^[61].

Definition 1. Let $f$ be a function, then the beta derivative of a function $f$ of order $\alpha$ is defined as

${}_{0}^{\text{A}}D_{t}^{\alpha }\left(f\left(t \right) \right) = \underset{\Delta \to 0}{\mathop{\lim }}\, \frac{f\left(t\, +\Delta \left(t+\frac{1}{\Gamma \left(\alpha \right)} \right) \right)-f\left(t \right)}{\Delta }, \, \, \, \, \, \, \, \, \, \, \, \, \, \text{for all}\, \, \, \, \, t > 0, \, \, \, \, 0 < \alpha < 1.\, \,$

Theorem 1. Suppose that $0 < \beta \le 1, \, \, \beta > 0$, and the function $f, \, g\,$ are $\alpha$ -differentiable at a point $t > 0$, then

I. ${}_{0}^{\text{A}}D_{t}^{\alpha }\left(af\left(t \right)+bg\left(t \right) \right) = a{}_{0}^{\text{A}}D_{t}^{\alpha }f\left(t \right)+b{}_{0}^{\text{A}}D_{t}^{\alpha }g\left(t \right), \, \, \, a, b\in \mathbb{R}.$

II. ${}_{0}^{\text{A}}D_{t}^{\alpha }\left(f\left(t \right).g\left(t \right) \right) = f\left(t \right){}_{0}^{\text{A}}D_{t}^{\alpha }g\left(t \right)+g\left(t \right){}_{0}^{\text{A}}D_{t}^{\alpha }f\left(t \right).$

III.

IV. ${}_{0}^{\text{A}}D_{t}^{\alpha }\left(C \right) = 0$, where $C$ is a constant function.

V. Suppose that $f$ is differentiable function, $\Delta = {{\left(x+\frac{1}{\Gamma \left(\alpha \right)} \right)}^{\alpha -1}}h, \, \, h\to 0$ when $\Delta \to 0$ then

VI.

where $l$ is a constant and $\eta = \frac{l}{\alpha }{{\left(x+\frac{1}{\Gamma \left(\alpha \right)} \right)}^{\alpha }}$.

3.   General form of method

Assume, we have the following nonlinear partial differential equation (NLPDE)

To find explicit exact solutions of coupled Schrödinger-Boussinesq system, we use the following transformation

where $\nu$ is arbitrary constant and $\xi$ is the symbol of the wave variable. Inserting Eq (2) to Eq (1), we get a nonlinear ordinary differential equation (NLODE)

The trial equation of solution for Eq (3) is given by

where ${{a}_{0}}, \, {{a}_{i}}$ and ${{b}_{i}}$ are non-zero constants and $\Phi \left(\xi \right)$ is the auxiliary ODE given by

where $\mu$, $\lambda$ are constants and $K > 0, \, K\ne 1$. The auxiliary ODE has the general solution:

I. When ${{\lambda }^{2}}-4\mu > 0,$ then $f\left(\xi \right) = {{\log }_{K}}\left(-\lambda -\sqrt{{{\lambda }^{2}}-4\mu }\tanh \left(\frac{1}{2}\sqrt{{{\lambda }^{2}}-4\mu }\left(C+\xi \right) \right) \right)$.

II. When ${{\lambda }^{2}}-4\mu < 0,$ then

III. When ${{\lambda }^{2}}-4\mu \ne 0$, $\lambda = 0$ and$\mu < 0$, then

IV. When ${{\lambda }^{2}}-4\mu \ne 0$, $\lambda = 0$ and$\mu > 0$, then

V. When ${{\lambda }^{2}}-4\mu > 0$ and $\mu = 0$, then

VI. When ${{\lambda }^{2}}-4\mu = 0, \lambda \ne 0$ and $\mu \ne 0$, then

VII. When ${{\lambda }^{2}}-4\mu = 0, \lambda = 0$ and $\mu = 0$, then

4.   The coupled Schrödinger-Boussinesq system with the beta derivative

Consider the coupled Schrödinger-Boussinesq system ^[62] with the Beta Derivative as follows:

where $\beta$ and $\gamma$ are real constants, $E\left(x, t \right)$ is a complex function, and $N\left(x, t \right)$ is a real function. To find the explicit exact solutions of coupled S-B system, we use the following transformation

where $\delta$ is arbitrary constant and $\kappa$ is the symbol of the soliton wave number, $\omega$ represents the soliton frequency and $l$ symbolize the phase constant. Substituting Eq (4.3) into Eqs (4.1) and (4.2), we obtain

where Eq (4.5) is found by integrating twice with respect to $\xi$ and $A$ is the arbitrary constant of integration. By Separate Eq (4.4) into real and imaginary parts, we get

and

Inserting Eq (4.7) into Eqs (4.5) and (4.6), we get

From Eq (4.8), we have

By using the homogeneous balance principle between the highest-order derivatives and nonlinear terms appearing in Eqs (4.9) and (4.10), we get $m = 2$ and $n = 2$ for $U$ and $V$, respectively. We assume that the solutions of Eqs (4.9) and (4.10) have the following form

By inserting Eqs (4.10) and (4.11) into Eqs (4.8) and (4.9) and collecting all terms with the same order of ${{ \mathrm{K} }^{-f\left(\xi \right)}}$ together, putting each coefficient of each polynomial to zero, we conclude the following cases:

Case One. When ${{a}_{0}} = \frac{\lambda \sqrt{2{{\lambda }^{2}}{{\mu }^{2}}-8{{\mu }^{3}}+\sqrt{{{\mu }^{4}}\left(12A+\beta {{2}^{2}}+24{{\beta }_{2}}{{\kappa }^{2}}+144{{\kappa }^{4}}+3{{\lambda }^{4}}-24{{\lambda }^{2}}\mu +48{{\mu }^{2}} \right)}}}{\sqrt{2}\mu }, {{a}_{2}} = 0$, ${{a}_{1}} = \sqrt{2}\sqrt{2{{\lambda }^{2}}{{\mu }^{2}}-8{{\mu }^{3}}+\sqrt{{{\mu }^{4}}\left(12A+{{\beta }_{2}}^{2}+24{{\beta }_{2}}{{\kappa }^{2}}+144{{\kappa }^{4}}+3{{\lambda }^{4}}-24{{\lambda }^{2}}\mu +48{{\mu }^{2}} \right)}}$, ${{b}_{1}} = 0$, ${{b}_{2}} = 0$, ${{c}_{0}} = \frac{-{{\beta }_{2}}{{\mu }^{2}}-12{{\kappa }^{2}}{{\mu }^{2}}+3{{\lambda }^{2}}{{\mu }^{2}}+\sqrt{{{\mu }^{4}}\left(12A+{{\beta }_{2}}^{2}+24{{\beta }_{2}}{{\kappa }^{2}}+144{{\kappa }^{4}}+3{{\lambda }^{4}}-24{{\lambda }^{2}}\mu +48{{\mu }^{2}} \right)}}{6{{\mu }^{2}}}, {{c}_{1}} = 2\lambda \mu$, ${{c}_{2}} = 2{{\mu }^{2}}$, ${{d}_{1}} = 0$, ${{d}_{2}} = 0$, We obtain the following families of solutions.

Family 1. When $\Delta = {{\lambda }^{2}}-4\mu > 0$, $2{{\lambda }^{2}}{{\mu }^{2}}-8{{\mu }^{3}}+{{ \mathrm{H} }_{1}} > 0$, $-\frac{{{\lambda }^{2}}}{2}+2\mu > 0$, $\left(12A+{{\beta }_{2}}^{2}+24{{\beta }_{2}}{{\kappa }^{2}}+3\left(48{{\kappa }^{4}}+{{\left({{\lambda }^{2}}-4\mu \right)}^{2}} \right) \right){{\mu }^{4}} > 0$ and $\mu \ne 0$, then

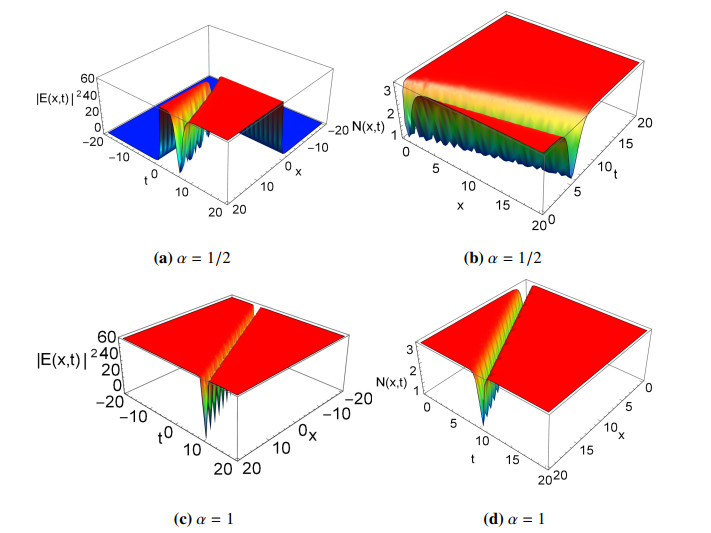
where ${{ \mathrm{H} }_{1}} = \sqrt{\left(12A+{{\beta }_{2}}^{2}+24{{\beta }_{2}}{{\kappa }^{2}}+3\left(48{{\kappa }^{4}}+{{\Delta }^{2}} \right) \right){{\mu }^{4}}}$. Eq (4.12) and Eq (4.13) are dark soliton solutions as shown in Figure (1).

Family 2. When $\Delta = {{\lambda }^{2}}-4\mu < 0$, $2{{\lambda }^{2}}{{\mu }^{2}}-8{{\mu }^{3}}+{{H}_{1}} > 0$, $-\frac{\Delta }{2} > 0$, $\left(12A+{{\beta }_{2}}^{2}+24{{\beta }_{2}}{{\kappa }^{2}}+3\left(48{{\kappa }^{4}}+{{\Delta }^{2}} \right) \right){{\mu }^{4}} > 0$ and $\mu \ne 0$, then

Equations (4.14) and (4.15) are singular soliton solutions as seen in Figure (2).

Family 3. When $\lambda = 0$, $\mu < 0$, ${{H}_{2}} > \mu$ and ${{\mu }^{4}}\left(12A+{{\beta }_{2}}^{2}+24{{\beta }_{2}}{{\kappa }^{2}}+48\left(3{{\kappa }^{4}}+{{\mu }^{2}} \right) \right) > 0$, then

where ${{ \mathrm{H} }_{2}} = \sqrt{{{\mu }^{4}}\left(12A+{{\beta }_{2}}^{2}+24{{\beta }_{2}}{{\kappa }^{2}}+48\left(3{{\kappa }^{4}}+{{\mu }^{2}} \right) \right)}$.

These solutions are singular soliton solutions as presented in Figure (3).

Family 4. When $\lambda = 0$, $\mu > 0$, ${{H}_{2}} > \mu$ and ${{\mu }^{4}}\left(12A+{{\beta }_{2}}^{2}+24{{\beta }_{2}}{{\kappa }^{2}}+48\left(3{{\kappa }^{4}}+{{\mu }^{2}} \right) \right) > 0$, then

These solutions are singular soliton solutions as presented in Figure (4).

Family 5. When ${{\lambda }^{2}}-4\mu > 0$ and $\mu = 0$, then the solution of this family could not be found because is located at the denominator of ${{a}_{0}}, {{c}_{0}}$.

Family 6. When ${{\lambda }^{2}}-4\mu = 0, \lambda \ne 0$, $2{{\lambda }^{2}}{{\mu }^{2}}-8{{\mu }^{3}}+{{H}_{1}} > 0$ and $\mu \ne 0$, then

As shown in Figure (5), Eq (4.20) and Eq (4.21) are singular solutions, too.

Family 7. When ${{\lambda }^{2}}-4\mu = 0, \lambda = 0$ and $\mu = 0$, then the solution of this family could not be found because $\mu = 0$ is located at the denominator of ${{a}_{0}}, {{c}_{0}}$.

Case Two. When ${{a}_{0}} = \frac{\lambda \sqrt{2\Delta {{\mu }^{2}}}}{\mu }, {{a}_{1}} = -2\sqrt{2{{\mu }^{2}}\Delta }$, ${{a}_{2}} = 0, {{b}_{1}} = 0$, ${{b}_{2}} = 0$, ${{c}_{0}} = \frac{1}{6}\left(5{{\lambda }^{2}}-8\mu -\sqrt{{{\lambda }^{4}}-8{{\lambda }^{2}}\mu +16{{\mu }^{2}}-12A} \right)$, ${{c}_{1}} = 2\lambda \mu$, ${{c}_{2}} = 2{{\mu }^{2}}$, ${{d}_{1}} = 0$, ${{d}_{2}} = 0$, $\omega = \frac{1}{12}\left(12{{\beta }_{1}}+{{\beta }_{2}}-10{{\lambda }^{2}}+40\mu +\sqrt{{{\lambda }^{4}}-8{{\lambda }^{2}}\mu +16{{\mu }^{2}}-12A} \right)$, $\kappa = -\frac{\sqrt{\sqrt{{{\lambda }^{4}}-8{{\lambda }^{2}}\mu +16{{\mu }^{2}}-12A}-{{\beta }_{2}}}}{2\sqrt{3}}$, we obtain the following families of solutions.

Family 1. When $\Delta = {{\lambda }^{2}}-4\mu > 0$, $\beta 2 > 0$, $\beta 2 > {{H}_{3}}$ and ${{\Delta }^{2}}-12A > 0$, then

where ${{H}_{3}} = \sqrt{{{\Delta }^{2}}-12A}$.

Family 2. When$\Delta < 0$, ${{\beta }_{2}} > 0$, $\beta 2 > {{H}_{3}}$ and ${{\Delta }^{2}}-12A > 0$, then

Family 3. When $\lambda = 0$, $\mu < 0$, $A > 0$ and $-{{\beta }_{2}}-2\sqrt{4{{\mu }^{2}}-3A} > 0$, then

where ${{H}_{4}} = \sqrt{-{{\beta }_{2}}-2\sqrt{4{{\mu }^{2}}-3A}}$.

Family 4. When $\lambda = 0$, $\mu < 0$, $A < 0$ and $-{{\beta }_{2}}-2\sqrt{4{{\mu }^{2}}-3A} > 0$, then

Family 5. When $\Delta > 0$ and $\mu = 0$, then the solution of $E\left(x, t \right)$could not be found because $\mu = 0$ is located at the denominator of ${{a}_{0}}$.

Family 6. When $\Delta = 0, \lambda \ne 0$, $-\beta 2-H > 0$ and $\mu \ne 0$,

Family 7. When $\Delta = 0, \lambda = 0$ and $\mu = 0$, then the solution $E\left(x, y, t \right)$ could not be found because $\mu = 0$ is located at the denominator of ${{a}_{0}}$.

Case Three. When ${{a}_{0}} = \frac{\lambda \sqrt{{{\lambda }^{2}}{{c}_{0}}+8\mu {{c}_{0}}-3c_{0}^{2}-A-2{{\lambda }^{2}}\mu -4{{\mu }^{2}}}}{\sqrt{{{\lambda }^{2}}-2{{c}_{0}}}}$, ${{a}_{1}} = 0$, ${{a}_{2}} = 0$, ${{b}_{1}} = \frac{2\sqrt{{{\lambda }^{2}}{{c}_{0}}+8\mu {{c}_{0}}-3c_{0}^{2}-A-2{{\lambda }^{2}}\mu -4{{\mu }^{2}}}}{\sqrt{{{\lambda }^{2}}-2{{c}_{0}}}}$, ${{b}_{2}} = 0$, ${{c}_{1}} = 0$, ${{c}_{2}} = 0$, ${{d}_{1}} = 2\lambda$, ${{d}_{2}} = 2$, $\omega = \frac{2A+12{{\beta }_{1}}{{\lambda }^{2}}+{{\beta }_{2}}{{\lambda }^{2}}-{{\lambda }^{4}}+20{{\lambda }^{2}}\mu +8{{\mu }^{2}}-24{{\beta }_{1}}{{c}_{0}}-2{{\beta }_{2}}{{c}_{0}}-6{{\lambda }^{2}}{{c}_{0}}-48\mu {{c}_{0}}+18c_{0}^{2}}{12\left({{\lambda }^{2}}-2{{c}_{0}} \right)}$, $\kappa = \frac{\sqrt{{{\lambda }^{4}}+4{{\lambda }^{2}}\mu -8{{\mu }^{2}}+2{{\beta }_{2}}{{c}_{0}}-6{{\lambda }^{2}}{{c}_{0}}+6c_{0}^{2}-2A-{{\beta }_{2}}{{\lambda }^{2}}}}{2\sqrt{3\left({{\lambda }^{2}}-2{{c}_{0}} \right)}}$, we obtain the following families of solutions.

Family 1. When $\Delta = {{\lambda }^{2}}-4\mu > 0$ and $\left({{\lambda }^{2}}+8\mu \right){{c}_{0}}-3c_{0}^{2}-A-2\mu \left({{\lambda }^{2}}+2\mu \right) > 0$, then

where ${{H}_{5}} = \sqrt{\left({{\lambda }^{2}}+8\mu \right){{c}_{0}}-3c_{0}^{2}-A-2\mu \Delta }$.

Family 2. When $\Delta < 0$ and $\left({{\lambda }^{2}}+8\mu \right){{c}_{0}}-3c_{0}^{2}-A-2\mu \left({{\lambda }^{2}}+2\mu \right) > 0$, then

Family 3. $\lambda = 0$, $\mu < 0$, ${{c}_{0}} < 0$ and $8\mu {{c}_{0}}-3c_{0}^{2}-A-4{{\mu }^{2}} > 0$

where ${{H}_{6}} = \sqrt{8\mu {{c}_{0}}-3c_{0}^{2}-A-4{{\mu }^{2}}}$.

Family 4. When $\lambda = 0$, $\mu > 0$ and $8\mu {{c}_{0}}-3c_{0}^{2}-A-4{{\mu }^{2}} > 0$, then

Family 5. When $\Delta > 0$, ${{\lambda }^{2}}-2{{c}_{0}} > 0$, ${{\lambda }^{2}}{{c}_{0}}-3c_{0}^{2}-A > 0$ and $\mu = 0$, then

Family 6. When $\Delta = 0, \lambda \ne 0$, ${{\lambda }^{2}}-2{{c}_{0}} > 0$, $\left({{\lambda }^{2}}+8\mu \right){{c}_{0}}-3c_{0}^{2}-A-2\mu \left({{\lambda }^{2}}+2\mu \right) > 0$ and $\mu \ne 0$, then

Family 7. When $\Delta = 0, \lambda = 0$, $\mu = 0$, ${{c}_{0}} > 0$, and $-A-3c_{0}^{2} > 0$, then

5.   Linear stability analysis

In this section, we construct the modulation instability (MI) of the stationary solutions of Eqs (4.1) and (4.2) via the virtue of linear stability analysis. The MI may consist of exponential growth of small disturbances in the amplitude or optical wave phase ^[63]. It is essential that we can observe MI in the nonlinear physics of the wave. Suppose that Eqs (4.1) and (4.2) have the following stationary solutions ^[64]:

where $a$ and $b$ are arbitrary real constants. Putting Eq (5.1) into Eqs (4.1) and (4.2), we get $\varphi = \left({{\beta }_{1}}-b \right)$. Suppose that the perturbed stationary solution has the form:

where $U\left(x, t \right)$ is complex fractional function, and $V\left(x, t \right)$ is real fractional function. Putting Eq (5.2) into Eqs (4.1) and (4.2), the results satisfy the following linear equations.

Where $*$ is the symbol of the conjugate and so, Eqs (5.3) and (5.4) can be written as

Where $W$ denotes the complex frequency, $M$ is real disturbance wave-number, and ${{U}_{1}}, {{U}_{2}}, {{V}_{1}}, {{V}_{2}}$ are the coefficients of the linear combination. Substituting Eqs (5.5) and (5.6), we get the following homogeneous equations

Evaluating the determinant and equaling to zero, we get the following relationship:

According to Eq (5.8), we can discuss the following cases of the MI for Eqs (4.1) and (4.2) as follows

Case 1. In case

we observe that the modulation instability of the Eqs (4.1) and (4.2) occurs when the wave number contains an imaginary value, therefor

Case 2. In case

we observe that the modulation instability of the Eqs (4.1) and (4.2) occurs when either

or

Moreover, we investigate the modulation Instability gain spectrum $G\left(W \right)$, which is determined by the maximum absolute value for the imaginary part of the wave number and defined as

and

The effect of the arbitrary constants $a$ and $b$ are illustrated graphically as seen in Figures (6) and (7).

6.   Conclusions

In this research, we constructed the new periodic, singular solutions of the coupled Schrödinger-Boussinesq system with beta derivative via a modified auxiliary expansion method. We found and investigated the several new family's solutions and one family are shown graphically in 2-D and 3-D; to more understands their physical characteristics. The novel solutions included hyperbolic function, trigonometric function, rational function, and constant function. The linear stability analysis of coupled Schrödinger-Boussinesq are studied and the modulation instability of two cases are analyzed. Moreover, the two cases of instability modulation and its gain spectrum are illustrated graphically. These new solutions and results might appreciate in laser and plasma sciences.

Acknowledgments

This projected work was partially (not financial) supported by the Scientific Research Project Fund of Harran University with the project number HUBAP:21132.

Conflict of interest

The authors declare that they have no conflict of interest.