Fractal form of the partition functions p (n)

1.   Introduction

In the theory of numbers, it would be useful to find a general form for the coefficients in the fractal family $\left\{p\left(n, k\right), k\in \mathbb{N} \right\}$ which defines the rule to calculate the number of partitions for $n\in \mathbb{N}$, in which $n$ can be decomposed on exactly $k$ parts. Each $k$ represents one class, from the total number of partitions for $n$. In this case, we can state that $p\left(n\right) = \sum_{k = 1}^{n}p\left(n, k\right)$, where $p\left(n\right)$ is the partition function. If all the partition class functions $p\left(n, k\right)$ have the same form, then the form of the partition function remains the same form due to the superposition property applied to the sum of the partition class functions. In addition, it is known that $p\left(n\right) = p\left(2n, n\right)$ ^[1], so as soon as the general form of $p\left(n, k\right)$ is determined, the form of $p\left(n\right)$ is also solved, as done in Section 6.

There are many different partition number restrictions. Two of them are apparently similar but also different. Using the notations as in ^[2], with $p_k\left(n\right)$ denote a function that represents the number of all partitions of the number $n$ with at most $k$ parts and with $p\left(n, k\right)$ function that represents the number of all partitions of the number $n$ into exactly $k$ parts.

Significant contributions to the study of $p_k\left(n\right)$ were made by: Cayley ^[3], Sylvester ^[4], Glaisher ^[5] and Gupta ^[6]. For historical notes, see Gupta ^[7]. Furthermore, the theory of q-partial fractions and its formula was developed in Munagi ^[8]. All the aforementioned are characterized by the fact that they primarily used Sylvester's Theorem and investigated $p_k\left(n\right)$. None of the mentioned papers have been noticed: 1. Fractal form of the partition function; 2. The common general form of all coefficients within the same class functions $p\left(n, k\right)$; 3. Recurrent relationship and the common general form of all the first, second, ..., coefficients of all $\{p\left(n, k\right), k \in \mathbb{N}\}$; 4. The ability to form a function that generates partitions function, similar to the Gamma function for a factorial. All of these are the basic content of this paper. In addition, the access to this issue through recurrent connections in this paper is unique.

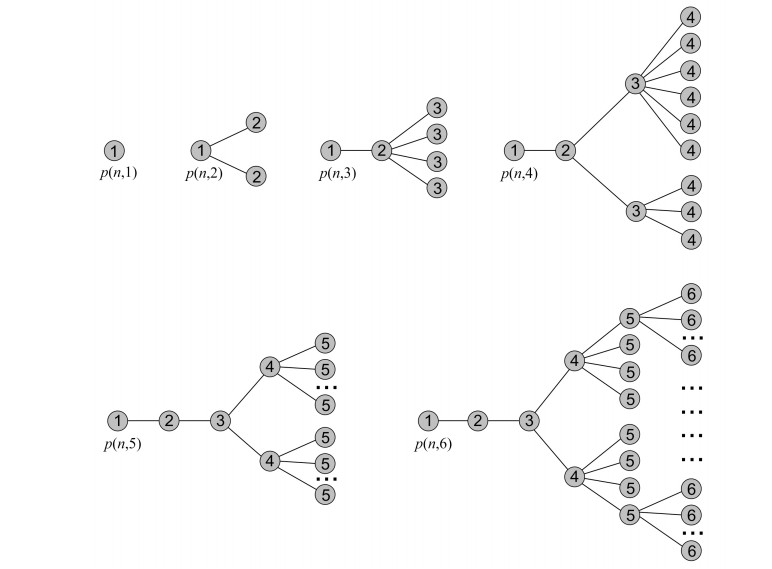
Each polynomial written down by the value defined coefficients is not a fractal. However, it is possible to justify the use of the terms: fractal polynomial and fractal family. For the first analogy we can use graphs. Based on result in ^[1] (part of Appendix C) we assign one graph to each quasi-polynomials $\{p\left(n, k\right), k = 1, 2, \dots, 6\}$. Each polynomials member is one vertex of the graph. Let us number the vertices of the graph in the way they form individual polynomials. The vertices that belong to the same single polynomial are joined by the edges. All the mention results correspond to the following graphs are in Figure 1.

Within each graph, each complete branch starting from vertex with index one represents one polynomial. All together a quasi-polynomial. Each quasi-polynomial contains a specified number of polynomials and is not fractal. However, the fractal structure of quasi-polynomial, when $k$ unlimitedly increase is quite obvious. The partition function for each $n$, unifies from each quasi-polynomial only one polynomial, $p\left(2n, n\right)$. The partition function does not have a finite form and is formed from the apparent fractal. Therefore, the partition function is a fractal polynomial and partitions class functions $p\left(n, k\right)$ have a fractal form. In this case, each individual polynomial (which form partition function) has discrete values and is a true polynomial (as in Appendix D). However, this needs to be understood only in this context. If the search for the partition function is accessed in the manner described above, the answer is given. The result obtained does not exclude that another approach leads to a different conclusion. Here I refer to the result obtained in Section 6 given by the function $first\left(n, i\right)$. Also in Section 6, we give a fractal form of a partition function in one unique form where for each value of $n$, a polynomial of a different degree and coefficients from general formulas are obtained. That is, a unique formula defines infinitely many different polynomials that all together form one fractal polynomial - partition function.

All of this can be more severe and argued in the manner proposed in ^[9]. Consider an infinite series of graphs corresponding to the quasi-polynomials of each partition class $\{p\left(n, k\right), k\in \mathbb{N}\}$

ⅰ) The graphs in Figure 1 are self-similar.

ⅱ) Clearly, every part of each graph is contained in all the other parts.

ⅲ) When $k$ increase the length of the graphs and the number of its branches tend to infinity.

ⅳ) Each next graph is obtained by a recursive procedure. Each subsequent graph is obtained from the previous one, as will be shown in detail in Section 3. We will prove that all: first, second, ... coefficients are obtained by unique general formulas.

ⅵ) The coefficients of the polynomials correspond to vertices in each graph: $1, 2, 3, \dots$ have a unique recurrent formula which they can be obtained with. This was explained in detail in Section 4 and Section 5. In this case, the polynomials corresponding to the graph in Figure 1 are not real polynomial because they contain factorials. (Thus polynomials: $q_1\left(n\right) = \frac{1}{n!}n^{k}$ and $q_1\left(n\right) = \frac{1}{\left(n-1\right)!}n^{k-1}$ are not of the same degree but are identical).

ⅶ) The dimensions of each graph, starting from the second in Figure 1, is greater than 1 (has different branches) but lesser than two (does not have a surface).

According to ^[9], these are quite sufficient reasons to call some object a fractal. The partition function is an infinite part of the previous fractal, and therefore also a fractal.

There are several different approaches when attempting to determine the general form of $p\left(n, k\right)$. In ^[10], the form for $k = 2, 3, 4$ is given, but from such an approach it is not possible to even observe the form for $k = 5$. By a completely different approach, Ekhad ^[11] determined the shape up to $k = 60$, but the general form is not determined. The third approach (similar to that in ^[12]) is presented in ^[1] and the process of determining $p\left(n, k\right)$ becomes quite difficult when increasing the class $k$. It is advisable to look for some other partition features that allow for determining the coefficients of $p\left(n, k\right)$ needed to calculate the values of an individual class. In this paper, we will develop the recurrent relations that generalize the form of all coefficients with the highest degrees of all the fractal polynomials $\left\{p\left(n, k\right)\right\}$. For some fixed $k$, common coefficients of a fractal polynomial $p\left(n, k\right)$ will be found using the theorem of a horizontal total collecting from the left ^[13,1]. Then, using the same theorem, we will show that within a single fractal polynomial $p\left(n, k\right)$, of some fixed class, there exists a general form for all of its common coefficients.

The formula for varying coefficients of fractal polynomials $\left\{p\left(n, k\right)\right\}$ will be found using the theorem of vertical $k$-collecting ^[1] in the following manner: within class 2, every second value is found using the same polynomial; within class 3, every sixth value is found using the same polynomial; within class 4, every twelfth value is found using same polynomial etc. ^[1]. Therefore, the values for the second class are found using two polynomials, the third class using six, the fourth class using twelve and so forth. In general, for the class $k$ we need at most $LCM\left(1, 2, 3, \dots, k\right)$-(the least common multiple) different polynomials. Therefore, using the fact that $p\left(n, k\right)$ is a fractal polynomial of the $(k-1)$-th degree ^[1], and knowing the necessary values of $p\left(n, k\right)$ within the same class $k$ (that we may obtain using Theorem 1 ^[1]), we may set up the corresponding system of equations and determine the unknown values for the rest of the variable and unknown coefficients.

Appendix A gives the general form of the first six coefficients of a fractal polynomials $p(n, k)$.

Appendix B gives the final form of the first six polynomials $Q_{k}\left(n\right)$.

Appendix C gives the final form of the first ten fractal polynomials $p(n, k)$.

Appendix D gives the special form of partition function for the first ten natural numbers using the fractal family $\left\{p\left(n, k\right)\right\}$.

Appendix E gives the special form of partition function for the first twelve natural numbers using the general form of the coefficients $a\left(n, k\right)$, from Appendix A.

2.   A total horizontal collecting and $p\left(n, k\right)$

In ^[1] (Theorem 2 - a total horizontal collecting), it is shown that for the number of partition for the class $k$, denoted with $p\left(n, k\right)$, the following is valid:

In ^[1] it has been proven that the fractal polynomials $\left\{p(n, k), k \in \mathbb{N}\right\}$ are of degree $k-1$. Also, for each class, there are at most $LCM\left(1, 2, 3, \dots, k\right)$ different polynomials which calculate all values of the same class (creating a unique quasi-polynomial). Within each polynomial $p\left(n, k\right)$ all highest level coefficients ending with degree $\lambda = \left[\frac{k}{2}\right]$ have a common values (total $k-\lambda$ coefficients), while the remaining coefficients vary (the remaining $\lambda$ coefficients). ($\lambda$ will retain the same meaning throughout this paper.) As stated, the first variable coefficient has up to two values. The second variable coefficient has up to six values and so forth. A free member always has a variability up to $LCM\left(1, 2, 3, \dots, k\right)$ values ^[1](not necessarily different).

The expression (1) can be applied to determine the coefficients of the fractal polynomials $\left\{ p\left(n, k\right), k\in \mathbb{N}\right\}$ which allow for an explicit calculation for the number of partitions of the class $k$.

For every $n, k\in \mathbb{N}$, we will look for the polynomial $p\left(n, k\right)$ of the form:

where $a_{k, 1}, a_{k, 2}, ..., a_{k, k-\lambda}$ represent the coefficients of the common part which depends only on $k$, and $b_{j_1}, b_{j_2}, \dots$ represent the coefficients of the variable part of the polynomial $p\left(n, k\right)$ which depends on $k$ and the remainder of the division of $n$ with $LCM\left(2, 3, \dots, j_i\right)$ ^[1].

Let us now determine the general form of some $p\left(n, k\right)$. For example: for $k = 1$, we have: $p\left(n, 1\right) = 1$, for each $n\in \mathbb{N}$. Hence, $a_{1, 1} = 1$. For $k = 2$, we have $p\left(n, 2\right)$ in the form of the polynomial $a_{2, 1}n+b_{j_1}, {j_1}\in \left\{1, 2\right\}$, $a_{2, 1}, b_{j_1}\in \mathbb{Q}$. According to (2.1) we can state:

Comparing the degrees of the polynomials and equating the coefficients gives $a_1 = \frac{1}{2}$. We can conclude that:

Variable coefficients $\left\{b_1, b_2\right\}$ cannot be determined by this procedure. Firstly, as stated earlier, for class 2 the following holds: $p\left(2m-1, 2\right) = m-1$, and $p\left(2m, 2\right) = m$, for all $m\in \mathbb{N}$. Substituting the variable $n$ into the expression (3), first with a random odd number, and then with a random even number, we obtain: $b_1 = -\frac{1}{2}$ and $b_2 = 0$, that is, the polynomials of class 2 are given by:

For $k = 3$, applying the same procedure with the assumption that the required polynomial is of the form $a_{3, 1}n^2+a_{3, 2}n+b_j$, $j\in \left\{1, 2, \dots, 6\right\}$ by substituting in the relation (1) for $k = 3$, $p\left(n+3, 3\right) = p\left(n, 3\right)+p\left(n, 2\right)+p\left(n, 1\right)$ we obtain

In the formula above, there are more coefficients of the required quadratic polynomial which are not uniquely determined. The last relation leads to the value of the coefficient $a_1 = \frac{1}{12}$. Coefficients $\left\{a_{3, 2}, b_1, \dots, b_6\right\}$ cannot be directly determined by this procedure. Similarly, for the third class it holds that all the values can be divided into six groups, modulo six, such that each group can be calculated using the same polynomial ^[1]. Therefore, all values, for example, $p\left(6m, 3\right), m \in \mathbb{N}$ belong to the same polynomial. Using two given values, we can find $a_{3, 2}$, for example, $p\left(6, 3\right) = \frac{1}{12}6^2+a_{3, 2}\cdot 6+b_6 = 3$ and $p\left(12, 3\right) = \frac{1}{12}12^2+a_{3, 2}\cdot 12+b_6 = 12$. When we subtract them, only the coefficient $a_{3, 2}$ remains as unknown. So, we find $a_{3, 2} = 0$. Each of the unknowns $\left\{b_j\right\}$ we find by using some known values: $p\left(6m, 3\right), p\left(6m+1, 3\right), \dots, p\left(6m+5, 3\right)$. Finally, we obtain all six polynomials needed to calculate the third class given by:

For $k = 10$ see ^[17]. In the following section, we will focus on finding the general form of the common coefficients $a_{k, i}$ of the polynomials $p\left(n, k\right)$.

Now, we will look for the general form of all the first $k-\lambda$ coefficients within the same fractal polynomial $p\left(n, k\right)$.

3.   Coefficients ${a_{k, 1}, a_{k, 2}, \dots, a_{k, k-\lambda}}$ of $p\left(n, k\right)$ have a unique general form

Let us show that it is possible to find the general forms of the coefficients of $p\left(n, k\right)$ for degree $k$, $k-1$, $\dots$ $\lambda$. The following can be proved.

Theorem 1. (A vertical connection of the coefficients)

All first, second, third, $\dots$, $(k-\lambda)$th coefficients of the fractal family ${p\left(n, k\right)}$, (2.2) have a unique general form.

Proof. The proof will be carried out by method of mathematical induction on $k$ - the degree of the fractal family ${p\left(n, k\right)}$.

Replacing the form of some $p\left(n, k\right)$ given in (2.2), in formula (2.1), we have:

Unifying the coefficients with same degrees on each side respectively and equating them, in the last equation, we obtain the following: $\left(k-1\right)$ degree coefficients on both sides cancel each other out; With the $\left(k-2\right)$ degree, the coefficient on the left is $(k-1)ka_{k, 1}$, and on the right $a_{k-1, 1}$. Hence,

With degree $k-3$, the coefficient on the left is $\binom{k-1}{2}k^2 a_{k, 1}+\binom{k-2}{1}k a_{k, 2}$, and on the right $a_{k-1, 2}+a_{k-2, 1}$. Hence,

To generalize, comparing coefficients with degree $k-m-1$, the recurrent equation takes form:

Now, we can find the general form of all the largest coefficients of the family ${p\left(n, k\right)}$. Solving the recurrent relations (3.1) by $k$, it is easily found that

Hence, knowing that $a_{1, 1} = 1$ we obtain the general form of the first coefficients of all $\left\{ p\left(n, k\right), 1\le k\le n\right\}$.

The relation (3.4) represents the general formula for the coefficients of the highest degree of all fractal polynomials $p\left(n, k\right)$ defined in (2.2).

In the same manner, resolving the relation (3.2) by $k$, we can conclude that the second coefficient of the two adjacent fractal polynomials $p\left(n, k\right)$ depends only on the first and the second corresponding coefficient in the previous polynomial, relative to $k$ (the second common coefficient first appears from $k = 3$). Using (3.4), relation (3.2) can be written as follows:

The coefficient $a_{k, 2}$, can be calculated from the recurrent relation (3.5) by $k$. Introducing the substitution $a_{k, 2} = \frac{1}{k!\left(k-2\right)!} b_{k, 2}$ into (3.5) gives

The resulting expression allows the explicit calculation of the coefficient $a_{k, 2}$ in the general form. We have already found that $a_{3, 2} = 0$, because of the substitution, and $b_{3, 2} = 0$. A simple transformation leads to

Hence,

Even though (3.6) can be simplified we will not do that because the coefficient $a_{k, 2}$ exists starting from $k = 3$ and can be calculated using the given formula, which would be impossible after simplifying.

Each subsequent recurrence relation becomes more complex to calculate, since it depends on the increasing number of coefficients of the preceding polynomials. However, note that the general form of each coefficient $a_{k, j}$ can be obtained from (3.3).

Suppose we found the first $m-1$ coefficients. Writing relation (3.3), on the left side only the last coefficient is unknown. On the right side only the first coefficient is unknown, which is recurrently related to the corresponding coefficient on the left side. The obtained recurrence relation can be solved in the manner defined by $a_{k, 2}$. (In Theorem 2, the procedure will be described in detail here, only the possibility of obtaining a general form is sufficient.)

Therefore, all the first, second, ..., $\left(k-\lambda\right)$-th coefficients of all fractal polynomials $p\left(n, k\right)$ have a unique general form.

4.   General form of the coefficients of the fractal family $p\left(n, k\right)$

Using the proof of Theorem 1, it is possible to establish the hypothesis of the general form of coefficients $a_{k, i}$ and prove it.

Theorem 2. The first $k-\lambda$, coefficients of $p\left(n, k\right)$, (2.2) are given in the form

where $Q_{2j-3}\left(k\right)$ is a polynomial of $k$ with degree $2j-3$.

Proof. The proof involves the method of transfinite induction on $j$, $j\in \mathbb{N}$.

For $j = 1$, from (3.4), the first coefficient is: $a_{k, 1} = \frac{k^{-1}}{\left(k-1\right)!\left(k-1\right)!}, \enspace Q_{-1}\left(k\right) = \frac{1}{k}.$

For $j = 2$, from (3.6), we get: $a_{k, 2} = \frac{k-3}{4\left(k-1\right)!\left(k-2\right)!}, \enspace Q_{1}\left(k\right) = \frac{1}{4}k-\frac{3}{4}.$

The statement is true for $j = 1, 2$. We will prove it to be true for all $1\le j\le k-\lambda$.

Suppose that the statement is true for all $j$ from 1 to $i-1, i > 2$. All the coefficients $a_{k, j}, j\le i-1$, have an assumed form, for example $a_{k-2, j-1} = \frac{Q_{2j-5}\left(k-2\right)}{\left(\left(k-2\right)-1\right)!\left(k-2-\left(j-1\right)\right)!} = \frac{Q_{2j-5}\left(k-2\right)}{\left(k-3\right)!\left(k-j-1\right)!}$.

Let us prove that the statement is true for $j = i$. We will start from formula (3.3) in a slightly different form ($i$ instead of $m$)

This formula is used to calculate the coefficient $a_{k, i}$ from the previous coefficients. We keep the last member on the left side and first on the right, in the previous formula, in their places and move all the others from the left to the right in the following way:

Each algebraic expression in brackets, in (4.1), contains all the coefficients $a_{k, j}$ in which $j\le i-1$ and all of them have an assumed form (inductive hypothesis). Using this fact, we can write an expression for everything in the brackets as a single fraction. Thus, we obtain the following

The degree of the polynomial in the numerator (4.2) is $2i-3$, since the degree of $Q_{2i-5}\left(k\right)$ is $2i-5$. Similarly, in each subsequent bracket, the degree of the polynomial is smaller by one.

The highest degree of the polynomial in the numerator in (4.3) is $2i-4$, etc. In the last bracket the highest degree is $i-1$ so we have

Each of the previous resulting fractions had the same expression in the denominator. Summing up all the previous fractions and bearing in mind that the numerator is a polynomial of degree $2i-3$, we can write down the following

Dividing equation (4.4) by $k\left(k-i\right)$ gives

Introducing the substitution

to (4.5) and simplifying, gives a simple recurrence equation by $k$

The resulting recurrence equation (4.7) can be solved by $k$ and its solution is expressed by the sums of degrees from $0$ to $2i-3$ of all natural numbers from $1$ to $k$. Written as $W_{2i-3}\left(k\right)$ by using its coefficients $w_1, w_2, \dots, w_{2i-2}$, we obtain the recursion solution (4.7) in the form

For the proof, it is sufficient to determine only the degree of the polynomial obtained in (4.8). For this we need the following general known lemma and two of its consequences.

Lemma 1. Let $S_{n}^{j} = 1^j+2^j+\dots+n^j$ denote the sum of the $j^{th}$ degrees of all the natural numbers from $1$ to $n$, where $n\in \mathbb{N}$. Then, the sums $\left\{S_{n}^{j}\right\}$, $1\le j\le m$, $m\in \mathbb{N}$, satisfy the recurrence formula

Corollary 1. The sum $S_{n}^{m}$ expanded with powers of $n$ can be obtained from the last equation and can be expressed as:

(This formula is known as Faulhaber's formula and its general form is

where $B_k$ is the $k$-th Bernoulli number. In this paper, it is written in a way that is more appropriate to the context used.)

Corollary 2. Each of the resulting sums $\left\{S_{n}^{j}\right\}$ is divisible by both $n$ and $n+1$. The proof can be obtain using mathematical induction.

According to Lemma 1, the sum next to each coefficients $w_j, \enspace j = 1, 2, \dots,$ $2i-2$ is always a polynomial of degree one higher than the degree of the numbers to be added. The highest degree is in the $w_1\sum_{j = 1}^{k}j^{2i-3}$ and its sum is a polynomial of degree $2i-2$. Also, it has already been found that the resulting polynomial is always divisible by at least one $k$ (Corollary 2). When we return to (4.6) and make the shortening nominator and denominator with $k$ we obtain that the general form of the coefficients of the required polynomial is given by

For $i = 1$, the value corresponds to the obtained result but the procedure is not shown in this proof.

5.   Determining coefficients of the polynomial $Q_{2i-3}\left(k\right), i \ge 1$

In order to determine the coefficient of the polynomial $Q_{2i-3}\left(k\right), i > 1$, we shall start from the first $k-\lambda$ coefficients of some $p\left(n, k\right)$ that can be written as

where $Q_{2i-3}\left(k\right), 1\le i\le k-\lambda$, are polynomials of order $2i-3$, with rational coefficients, which has been proven previously. Let $q_{i, 1}, q_{i, 2}, \dots\ q_{i, 2i-2}$ be coefficients of $Q_{2i-3}\left(k\right)$ polynomials, respectively.

To determine unknown coefficients, we will use a procedure similar to the proof of Theorem 2 with some more detailing. Using this procedure, as in the proof of Theorem 2, we obtain (4.4). First, we will calculate the coefficients of the polynomial $W_{2i-3}\left(k\right)$ : $W_{2i-3}\left(k\right) = w_1\cdot k^{2i-3} + w_2\cdot k^{2i-4} + \dots + w_{2i-2}$. The value of these coefficients can be exactly found using expressions: (4.2), (4.3), $\dots$ however, we will find only the first few. While arranging expression (4.2) it should be taken into consideration that, for example, $Q_{2i-5}\left(k-2\right)$ represents:

Multiplying and expanding the parenthesis in (4.2), (4.3), etc., developing the numerators by degrees of $k$ and integrating values with the same degree gives us the first few coefficients:

as the coefficient with degree $2i-3$,

as the coefficient with degree $2i-4$ and

as the coefficient with degree $2i-5$.

as coefficient with degree $2i-6$.

The procedure is continued as in Theorem 2. We divide (4.4) with $k\left(k-i\right)$, introduce the substitution (4.5) and finally solve the recurrence equation (4.7) by $k$. The solution is given by (4.8). Summing up (4.8) in terms of degrees with respect to powers of $k$ (Corollary 1), for example

$\dots$

we obtain:

It is still necessary to cancel one $k$ in all members (with the common denominator) on the right side in the previous formula, and equating the coefficients on the left and right with $Q_{2i-3}\left(k\right)$ gives:

We will calculate four coefficients due to the fact that the procedure for calculating even and odd coefficients differs. We'll show that is more difficult to calculate the general form of even coefficients. Solving (5.2) as a recurrent equation by $i$, we obtain the coefficient of the highest degree of $Q_{2i-3}\left(k\right)$.

Therefore, the general form of the first coefficient is:

Now, the rest of the recurrent relations can be additionally simplified. Hence, the expression (5.3) becomes:

Introducing the substitution: $q_{i, 2} = \frac{1}{2^{i-2}\left(2i-3\right)!!}r_{i, 2}$ and considering:

the last expression, after multiplication with $2^{i-2}\left(2i-3\right)!!$ can be rewritten and simplified in the form:

The last expression can be solved as a recurrent equation by $i$ when converted into the sum below, which is further divided into two more simple ones. Introducing $m = j-2$ further simplifies the expression.

Knowing that $\frac{1}{4^m}\binom{2m}{m} = \binom{-\frac{1}{2}}{m}$, the last sums can be found using the formulae ^[14]:

and

Therefore, we find the sum (replacing $r$ with $-\frac{1}{2}$) where the result is used starting from $i = 2$.

If we keep the result already obtained, the general form of the second coefficient is:

By introducing the Gamma function ^[15] the results could be expressed as follows:

Finally, we obtain the general form of the second coefficient:

The coefficient $q_{i, 3}$ is determined from relation (5.4), which can be written as:

Similarly to the above analysis, by introducing the substitution

the previous relation is reduced to a recurrent relation with the solution in the form of a sum to be additionally determined. After simplification

The coefficient $q_{i, 3}$ exists from $i \ge 3$. Therefore, the solution of this recurrence equation is expressed by the sum

By returning substitution we obtain

In order to see the difference in the procedure for determining even and odd coefficients, we have to find the fourth coefficient. If a similar manner we start from (5.5) we obtain

The substitution for even coefficients is different (in regards to odd)

Now we use that

By substitution, we obtain a recurrence equation:

whose solution is the sum:

In addition to what has already been said in obtaining the coefficient $q_2$ should be applied in this case as well. First, we introduce substitution $m = j-3$, we obtain

and knowing that $\frac{1}{4^m}\binom{2m}{m} = \binom{-\frac{1}{2}}{m}$ there is a need to finding the sums:

Formulas for sums are found inductively, starting from (5.6). We found that

We determine the other three sums by using equals:

and the following transformation of the sum of binomial coefficients

Similarly, we find the other sums.

By substituting in the last recurrence equation we found

By introducing the Gamma function using the formula ^[15]

each of the sums $I_0$, $I_1$,$\dots$, $I_4$ can be further simplified in the following way

Finely, we obtain

and by replacement substitution we obtain the fourth coefficient

The above procedure defines the general algorithm to find all the coefficients of the polynomial $Q_{2i-3}\left(k\right)$. Although recurrent equations become more complex, it is still possible to explicitly resolve each of them. Similarly, as in the determination of the general form of odd coefficients $a_{k, i}$, except for recurrent equations, only the sums of the degree of natural numbers appear. But for even, except for a different substitution, a much more complex sum is obtained. Thus, transcendence occurs only in even members of the partition function. From the first few results it is possible to inductively conclude the general form of all subsequent coefficients.

where $\phi = LCM\left(\phi_1, \phi_2, \phi_3, \phi_4\right)$.

$\phi_1$ is common denominator in (4.2), (4.3), $\dots$ and is equal $\left(k+1\right)!$. $\phi_2$ is common denominator in (5.3), (5.4), $\dots$ and it inherits the highest value of the preceding coefficient. $\phi_3$ is common denominator in (5.3), (5.4), $\dots$ and represents the least common multiple (LCM) of the first $k$ Bernoulli numbers. $\phi_4$ appears in even coefficients and represents the least common multiple of $\Gamma\left(\frac{3}{2}\right)$, $\Gamma\left(\frac{5}{2}\right)$, $\dots$ equals $\left(2k-1\right)!!$, ($\sqrt{\pi}$ is cancelled).

$k_1 = k+\left[\frac{k+1}{2}\right]-1$ represents the number of members within the polynomial $Q_{2i-1}\left(i\right)$.

$P_k\left(i\right)$ is $k$ degree polynomial.

Knowing that $p\left(n\right) = p\left(2n, n\right)$, what remains is to further "repackaged" this by using the fractal polynomial $p\left(n, k\right)$, whereby $k$ becomes $n$ and the whole polynomial changes both its degree and the coefficients.

6.   Fractal forms of the partition function $p\left(n\right)$

The general form of the coefficients of the fractal polynomial $p\left(n, k\right)$ was found. For each natural number $n$, in order to to find the total number of partitions, only one polynomial from its fractal family $\left\{p\left(n, k\right), k\in \mathbb{N} \right\}$ will be used. From everything that has been said so far (in this paper) the fractal form of the partition function $p\left(n\right)$ can be obtained in at least three different forms.

6.1.   The formation of fractal polynomials of the partition function $p\left(n\right)$ as special cases of $\left\{p\left(n, k\right)\right\}$

For each $n$, from fractal polynomials $\left\{p\left(m, n\right)\right\}$, it is necessary to choose only one polynomial belonging to the family which has the property $p\left(2n, n\right)$. All chosen polynomials make a new fractal family called the partition function $p\left(n\right)$. Several of the first members are in Appendix D. In this approach, the partition function is a real polynomial which has a fractal form. The fractal form of the partition function is qualitatively different from the fractal form of the partition functions of the classes. The fractal form of the partition functions of the classes represents a unique polynomial with higher degrees and different parts with lower degrees. In this case, the partition function for each value has a different polynomial.

To calculate the number of partitions of the number $n$, one polynomial of degree $n-1$ is required. All first, second, ... coefficients of all polynomials are determined by the unique formulas given in Appendix A. This establishes the vertical connection of all the polynomials within all the fractal families. This is especially important for the fractal family of the partition function. The fractal form in this case would have a form:

The last term is only for making the first half of the whole expression, the other half which contains the alternating members ${b_i}$ is not specified (see Appendix E). To find the number of partitions of the number $n$, the first $\left[\frac{n}{2}\right]$ required members is shown how to calculate. Other ${b_i}, i = 1, \dots, n-\lambda$ can be calculated as shown in section 1. In ^[17] this part for $n = 10$ was calculated.

It is important to note that despite the use of polynomials to calculate partition functions, the coefficients together with degrees do not behave asymptotically as expected. The main values are not located at the highest degrees, but are moving towards the centre in relation to the ordered polynomial. If we compare the two adjacent members starting with the highest degree, one can notice that:

The first pair satisfies the inequality for $n \ge 4$. The second pair satisfies the inequality for $n \ge 7$, etc. Therefore, this approach is not suitable for asymptotic assessment. This is because the partition function is not a real polynomial. The second problem is that the values of the members from the beginning of this polynomial tends to zero, when $n$ increases unlimitedly. In other words, because it is

$\dots$

In order to avoid this, certain repacking of the obtained polynomials is required. We want the values of the members of the partition function decrease together with the degrees or at least the first members do not tend to zero when $n$ increase. This leads us to the next section.

6.2.   Computation values of $p\left(n\right)$ with a polynomial whose coefficients are $a_{n, i}$

Instead of the first approach, using the general form of the entire family $p\left(n, k\right)$ (Appendix C) it is possible to get $p\left(n\right)$ in a formally different form. To that end it is necessary to additionally "repackaged" the general form of the coefficients (Appendix A) of $p\left(n, k\right)$. In this case, all $k$ are converted to $n$, and the general form is apparently changed. Using only the general forms of the coefficients $a_{n, k}$ given in Appendix A the values of the partition functions can be obtained as given in Appendix E.

Notice the general form of the polynomial $p\left(2n, n\right)$, $\lambda = \left[\frac{n}{2}\right]$ is given by the expression:

where $b_{j_1}, b_{j_2}, \dots$ represent the coefficients of the variable part of the fractal polynomial $p\left(2n, n\right)$ which depend on $n$ and the remainder of the division of $n$ by the $LCM\left(2, 3, \dots, j_i\right)$. We will only repack the fixed part of (6.2). The last member in (6.1) is of the degree $\lambda$, so we obtain

It can be seen (6.1) that the partition function is not a real polynomial, because its coefficients also depend on $n$. Such a polynomial has the characteristic that its greatest values do not have to be at the highest degree. If we look carefully at the expression (6.1), we will see that the degrees the polynomials in the numerators grow until the degrees of the main polynomial decrease. The highest degree is in the numerator of the last fixed member. The last member in the polynomial (6.2) has degree $2\left(n-\lambda\right)-3 + \lambda = 2n-\left[\frac{n}{2}\right]-3$. Hence, it is possible to "repack" the fractal polynomial (6.2) into a new, formally different which will give completely new general form of the partition function.

Let us consider the series of real polynomials $Q_{2j-3}\left(n\right)$:

Members within each of these polynomials have a form

In section 5 we have already seen that polynomials $Q_{2i-3}\left(n\right)$ have members that alternate and found the general forms a few first coefficients $q_{i, j}$. Each member in (6.2) with the degree $n-j$, $j = 1, 2, \dots$ additionally divided into individual addends

Using already obtained forms $q_{i, j}$ the general form of all the first, second and third members in each previous parentheses is:

Before we proceed, we notice the following fact that is particularly significant. Each of the obtained members: $first\left(n, i\right), second\left(n, i\right), third\left(n, i\right), \dots$ can be a formula for calculating the total number of partitions of all natural numbers. The following table illustrates this in the example of the first member. This is a fractal property that deserves further examination.

Table 1 indicates the possibility of obtaining the exact values of the number of partitions, and not the approximate ones. Thus, we obtain infinitely many different forms that are very similar (fractal) that contain all the exact values of the total number of all partitions of natural numbers.

In order to obtain a unique polynomial with the "non tend to zero" values in the first members, we will do the following. Let us unify all the first members $first\left(n, i\right)$, $i = 1, 2, \dots, n-\lambda$ into one single. Let us repeat this process for all the others in the same way. Thus, we get a polynomial representing the general fractal form of the partition function whose values in members from the beginning do not tend to zero.

When we unify all the $first\left(n, i\right)$, $second\left(n, i\right)$ and $third\left(n, i\right)$ members into a single sum we obtain

(Generating function of the last sum is $\frac{2^{x-1}x^{x-2}}{\left(x-1\right)!^2}\left(1+\frac{x}{8}\right)^{x-1}$.)

Although the following forth coefficients have been calculated in this paper, it is clear that without further writing how its form will look.

The general form of the partitions function is

The main disadvantage of this form is that there are large amplitudes of positive and negative values.($p_1$ is the sum of positive, and $p_2$ is the sum of negative members, etc.)

In order to alleviate this effect we can write another interesting fractal form of partition function. It is enough to write only the form (6.2) by decreasing degrees. It has already been established that the highest degree is in the last member and equal $2n-\left[\frac{n}{2}\right]-3$. Let the first $\lambda$ coefficients of the partition function be given with:

When the coefficients $q_{i, 1}, q_{i, 2}, \dots, q_{i, 2i-2}, i \in \left\{1, 2, \dots, \lambda\right\}$ are found (section 5) then all the unknown coefficients of the partition function are determined. When uniting values from several different polynomial in the numerator (6.2) it should be taken into account that the degrees of two neighbours members of (6.2) differ by two. According to the notations used in paper $i = n-\lambda = n-\left[\frac{n}{2}\right]$. Thus, we find

$\dots$

A set of coefficients with a unique general form is continued until the degree is $\left[\frac{n}{2}\right]$ and thus is obtained by repacking the remaining coefficients in (6.2). The variable part of $p\left(n\right)$ is obtained from the variable part of $p\left(2n, n\right)$ to which the unused fixed-coefficients are attached.

The coefficient with the highest degree $n^{2n-\left[\frac{n}{2}\right]-3}$, has already been defined in this study and equals

The next three coefficient are also determined

The general form of the partition function is

From this form it can be seen that the number of partitions for even numbers is slightly faster increase than the odd. And in this case (6.4) there are large amplitudes in the values of positive and negative members. The disadvantage in (6.4) is that it is easier to notice the general fractal form of all members in the previous form (6.3).

7.   Conclusion

With finding the fractal form $first\left(n, i\right) = \frac{2^{n-3i+2}n^{n+i-3}}{\left(n-1\right)!\left(n-i\right)!\left(i-1\right)!}$ should be of great interest in further research of partition function. From Table 1 it can be observed that there is a visible connection between $n$ and $i$ and in the case of precise determination that connection it is possible to obtain an extension of the partition function in the same way as the Gamma function from factorial. When $first\left(n, i\right)$ is converted to a single parameter function, the perfect asymptotic form of the partition function will be obtained.

If a similar Table were made for functions: $second\left(n, i\right)$, $third\left(n, i\right), \dots$ it would be noticed that as their index increases, an increasing number of initial values of partition function cannot be obtained by using them. Thus, $second\left(n, i\right)$ gives all values except the first three, $third\left(n, i\right)$ all except the first five etc.

Conflict of interest

The author declares no conflicts of interest.

Appendix A.   The first six coefficients of the fractal polynomials $\left\{p(n, k)\right\}$

● The coefficient with degree $k-1$

● The coefficient with degree $k-2$

● The coefficient with degree $k-3$

● The coefficient with degree $k-4$

● The coefficient with degree $k-5$

● The coefficient with degree $k-6$

Appendix B.   The coefficients of the first six polynomials $Q_{2i-3}(n)$

●

●

●

●

●

●

Appendix C.   The first ten fractal polynomials $p(n, k)$

● The first class $k = 1$

● The second class $k = 2$

● The third class $k = 3$

Values for $w_j$ are listed in order from $j = 0$ to $j = 5$, as is the case in all of the following examples.

● The fourth class $k = 4$

● The fifth class $k = 5$

$w_j$ are following numeric respectively:

● The sixth class $k = 6$

where

$w_j$ are given in the following table: $0$, $\frac{19319}{518400}$, $\frac{313}{32400}$, $\frac{87}{6400}$, $-\frac{244}{2025}$, $-\frac{2801}{20736}$, $\frac{89}{400}$, $\frac{30983}{518400}$, $-\frac{188}{2025}$, $-\frac{569}{6400}$, $-\frac{127}{1296}$, $\frac{45319}{518400}$, $\frac{3}{25}$, $-\frac{22153}{518400}$, $-\frac{2279}{32400}$, $-\frac{17}{256}$, $-\frac{1}{2025}$, $-\frac{7817}{518400}$, $\frac{57}{400}$, $-\frac{10489}{518400}$, $-\frac{14}{81}$, $\frac{199}{6400}$, $\frac{713}{32400}$, $\frac{3847}{518400}$, $\frac{1}{25}$, $-\frac{2545}{20736}$, $\frac{1609}{32400}$, $\frac{343}{6400}$, $-\frac{163}{2025}$, $-\frac{49289}{518400}$, $\frac{1}{16}$, $\frac{51719}{518400}$, $-\frac{107}{2025}$, $-\frac{313}{6400}$, $-\frac{1879}{32400}$, $-\frac{1505}{20736}$, $\frac{4}{25}$, $-\frac{1417}{518400}$, $-\frac{983}{32400}$, $-\frac{169}{6400}$, $-\frac{13}{81}$, $\frac{12919}{518400}$, $\frac{73}{400}$, $\frac{10247}{518400}$, $-\frac{269}{2025}$, $-\frac{33}{256}$, $\frac{2009}{32400}$, $\frac{24583}{518400}$, $\frac{2}{25}$, $-\frac{42889}{518400}$, $-\frac{143}{1296}$, $\frac{599}{6400}$, $-\frac{82}{2025}$, $-\frac{28553}{518400}$, $\frac{41}{400}$, $-\frac{1249}{20736}$, $-\frac{26}{2025}$, $-\frac{57}{6400}$, $-\frac{583}{32400}$, $-\frac{16889}{518400}$.

● The seventh class $k = 7$

The linear members show greater variability with

The first hundred $w_j$ (420 numbers) are: $0$, $-\frac{127}{72576}$, $\frac{76}{1575}$, $\frac{321}{22400}$, $\frac{334}{14175}$, $-\frac{1159}{8064}$, $-\frac{1}{7}$, $\frac{73511}{259200}$, $\frac{13}{1575}$, $-\frac{183}{22400}$, $-\frac{32}{567}$, $-\frac{655}{8064}$, $-\frac{11}{175}$, $\frac{69401}{1814400}$, $\frac{43}{225}$, $-\frac{23}{896}$, $-\frac{32}{567}$, $-\frac{12847}{201600}$, $-\frac{18}{175}$, $\frac{255377}{1814400}$, $-\frac{2}{63}$, $\frac{7}{128}$, $\frac{334}{14175}$, $-\frac{8311}{201600}$, $-\frac{11}{175}$, $-\frac{127}{72576}$, $-\frac{2}{63}$, $\frac{1217}{22400}$, $\frac{256}{2025}$, $-\frac{12847}{201600}$, $-\frac{1}{7}$, $\frac{4409}{72576}$, $\frac{76}{1575}$, $-\frac{1079}{22400}$, $\frac{334}{14175}$, $\frac{71}{1152}$, $-\frac{1}{7}$, $\frac{141977}{1814400}$, $\frac{13}{1575}$, $\frac{1217}{22400}$, $-\frac{32}{567}$, $-\frac{1159}{8064}$, $\frac{2}{5}$, $\frac{182801}{1814400}$, $\frac{76}{1575}$, $-\frac{79}{896}$, $-\frac{32}{567}$, $-\frac{247}{201600}$, $-\frac{18}{175}$, $\frac{57311}{259200}$, $-\frac{2}{63}$, $-\frac{23}{896}$, $\frac{334}{14175}$, $-\frac{20911}{201600}$, $-\frac{11}{175}$, $\frac{4409}{72576}$, $\frac{1}{9}$, $-\frac{183}{22400}$, $-\frac{233}{14175}$, $-\frac{247}{201600}$, $-\frac{1}{7}$, $-\frac{127}{72576}$, $\frac{76}{1575}$, $\frac{503}{3200}$, $\frac{334}{14175}$, $-\frac{1159}{8064}$, $-\frac{1}{7}$, $\frac{255377}{1814400}$, $\frac{13}{1575}$, $-\frac{183}{22400}$, $\frac{7}{81}$, $-\frac{655}{8064}$, $-\frac{11}{175}$, $\frac{69401}{1814400}$, $\frac{76}{1575}$, $-\frac{23}{896}$, $-\frac{32}{567}$, $\frac{2279}{28800}$, $-\frac{18}{175}$, $\frac{255377}{1814400}$, $-\frac{2}{63}$, $-\frac{79}{896}$, $\frac{334}{14175}$, $-\frac{8311}{201600}$, $\frac{2}{25}$, $-\frac{127}{72576}$, $-\frac{2}{63}$, $\frac{1217}{22400}$, $-\frac{233}{14175}$, $-\frac{12847}{201600}$, $-\frac{1}{7}$, $\frac{2111}{10368}$, $\frac{76}{1575}$, $-\frac{1079}{22400}$, $\frac{334}{14175}$, $-\frac{655}{8064}$, $-\frac{1}{7}$, $\frac{141977}{1814400}$, $\frac{34}{225}$, $\frac{1217}{22400}$, $-\frac{32}{567}$, $\dots$

● The eighth class $k = 8$

The first hundred $w_j$, $j\equiv n\mathrm{\enspace mod\enspace}840$ (840 numbers) are: $0$, $\frac{76117}{4064256}$, $\frac{49759}{6350400}$, $\frac{80301}{1254400}$, $-\frac{457}{31752}$, $\frac{120661}{4064256}$, $-\frac{377}{3136}$, $-\frac{330419}{2073600}$, $\frac{24694}{99225}$, $\frac{3013}{50176}$, $-\frac{2441}{254016}$, $\frac{140101}{4064256}$, $-\frac{1217}{9800}$, $-\frac{4400819}{101606400}$, $-\frac{281}{5184}$, $\frac{3253}{50176}$, $\frac{601}{3969}$, $\frac{3099469}{101606400}$, $-\frac{3089}{78400}$, $-\frac{319163}{4064256}$, $-\frac{577}{31752}$, $-\frac{43}{1024}$, $-\frac{55841}{6350400}$, $\frac{11713981}{101606400}$, $\frac{4}{49}$, $-\frac{172715}{4064256}$, $-\frac{3401}{254016}$, $-\frac{96851}{1254400}$, $-\frac{577}{16200}$, $\frac{452437}{4064256}$, $-\frac{121}{3136}$, $-\frac{153275}{4064256}$, $\frac{10681}{99225}$, $\frac{48701}{1254400}$, $-\frac{17993}{254016}$, $-\frac{2219}{82944}$, $-\frac{1}{392}$, $-\frac{4234931}{101606400}$, $\frac{428191}{6350400}$, $\frac{181}{50176}$, $\frac{358}{3969}$, $\frac{37717}{4064256}$, $-\frac{289}{1600}$, $\frac{4379581}{101606400}$, $\frac{2015}{31752}$, $\frac{1989}{50176}$, $-\frac{7625}{254016}$, $-\frac{2635331}{101606400}$, $\frac{74}{1225}$, $-\frac{8603}{82944}$, $\frac{17335}{254016}$, $\frac{2229}{50176}$, $-\frac{26977}{793800}$, $\frac{9154381}{101606400}$, $-\frac{313}{3136}$, $-\frac{402107}{4064256}$, $\frac{7}{81}$, $\frac{50749}{1254400}$, $\frac{322591}{6350400}$, $\frac{223045}{4064256}$, $-\frac{25}{392}$, $-\frac{255659}{4064256}$, $-\frac{468641}{6350400}$, $-\frac{451}{25600}$, $\frac{682}{3969}$, $\frac{369493}{4064256}$, $-\frac{185}{3136}$, $-\frac{9969731}{101606400}$, $\frac{33527}{793800}$, $-\frac{1083}{50176}$, $-\frac{473}{5184}$, $\frac{388933}{4064256}$, $\frac{76}{1225}$, $\frac{1819981}{101606400}$, $\frac{1783}{254016}$, $-\frac{843}{50176}$, $-\frac{1753}{31752}$, $-\frac{106019}{2073600}$, $\frac{1711}{78400}$, $-\frac{70331}{4064256}$, $\frac{667}{3969}$, $\frac{965}{50176}$, $-\frac{574241}{6350400}$, $\frac{3419581}{101606400}$, $-\frac{1}{8}$, $\frac{76117}{4064256}$, $\frac{12151}{254016}$, $-\frac{20051}{1254400}$, $\frac{14944}{99225}$, $\frac{120661}{4064256}$, $-\frac{377}{3136}$, $-\frac{9899}{82944}$, $\frac{34823}{793800}$, $\frac{125501}{1254400}$, $-\frac{2441}{254016}$, $\frac{140101}{4064256}$, $\frac{2}{49}$, $-\frac{12529331}{101606400}$, $-\frac{1841}{129600}$, $\frac{3253}{50176}$, $\frac{839}{31752}$, $\dots$

● The ninth class $k = 9$

The first hundred $w_j$, $j\equiv n\mathrm{\enspace mod\enspace}2520$ (2520 numbers) are: $0$, $\frac{22355}{11943936}$, $\frac{307571}{9144576}$, $-\frac{51469}{7225344}$, $\frac{1258961}{14288400}$, $-\frac{757021}{585252864}$, $-\frac{781}{112896}$, $-\frac{1470637}{11943936}$, $-\frac{97}{729}$, $\frac{4553891}{20070400}$, $\frac{459635}{9144576}$, $\frac{23130851}{585252864}$, $-\frac{55}{7056}$, $\frac{348899}{585252864}$, $-\frac{233701}{4665600}$, $-\frac{16285}{147456}$, $-\frac{1243}{35721}$, $\frac{35821283}{585252864}$, $\frac{2347}{12544}$, $\frac{295376939}{14631321600}$, $\frac{5335}{571536}$, $-\frac{7069}{147456}$, $-\frac{9901}{186624}$, $-\frac{25391389}{585252864}$, $-\frac{334}{11025}$, $\frac{48871139}{585252864}$, $\frac{120947}{9144576}$, $\frac{99867}{802816}$, $-\frac{391}{11664}$, $-\frac{629989}{298598400}$, $-\frac{781}{112896}$, $-\frac{48173341}{585252864}$, $-\frac{1837}{35721}$, $\frac{400115}{7225344}$, $\frac{25301051}{228614400}$, $-\frac{502957}{11943936}$, $\frac{1}{16}$, $\frac{348899}{585252864}$, $-\frac{450589}{9144576}$, $\frac{2021819}{180633600}$, $-\frac{1972}{35721}$, $\frac{47765219}{585252864}$, $-\frac{13}{2304}$, $-\frac{724141}{11943936}$, $\frac{438161}{14288400}$, $\frac{83483}{802816}$, $\frac{261347}{9144576}$, $-\frac{37335325}{585252864}$, $-\frac{22}{441}$, $\frac{12502811}{298598400}$, $-\frac{5149}{186624}$, $\frac{95987}{7225344}$, $\frac{4169}{571536}$, $\frac{23130851}{585252864}$, $\frac{38819}{313600}$, $-\frac{36229405}{585252864}$, $-\frac{97}{729}$, $\frac{2147}{147456}$, $\frac{646259}{9144576}$, $\frac{566327339}{14631321600}$, $\frac{233}{7056}$, $-\frac{11595037}{585252864}$, $-\frac{263965}{9144576}$, $\frac{11}{16384}$, $-\frac{1021}{18225}$, $\frac{47765219}{585252864}$, $\frac{3971}{112896}$, $\frac{12292835}{585252864}$, $-\frac{16999}{571536}$, $\frac{9625019}{180633600}$, $-\frac{9901}{186624}$, $-\frac{1249453}{11943936}$, $\frac{3}{49}$, $\frac{24983267}{585252864}$, $\frac{21499451}{228614400}$, $-\frac{51469}{7225344}$, $\frac{15833}{571536}$, $-\frac{502957}{11943936}$, $-\frac{157}{2304}$, $-\frac{320482261}{14631321600}$, $-\frac{3295}{35721}$, $\frac{166427}{802816}$, $\frac{459635}{9144576}$, $\frac{11186915}{585252864}$, $-\frac{31}{3600}$, $-\frac{724141}{11943936}$, $-\frac{263965}{9144576}$, $-\frac{503053}{7225344}$, $-\frac{514}{35721}$, $\frac{1480789939}{14631321600}$, $\frac{2091}{12544}$, $-\frac{724141}{11943936}$, $-\frac{823}{11664}$, $\frac{95987}{7225344}$, $\frac{6347051}{228614400}$, $-\frac{13447453}{585252864}$, $-\frac{31}{441}$, $\frac{36927203}{585252864}$, $-\frac{5149}{186624}$, $\frac{42259}{409600}$, $\frac{15833}{571536}$, $\dots$

● The tenth class $k = 10$

The first hundred $w_j$, $j\equiv n\mathrm{\enspace mod\enspace}4240$ (4240 numbers) are: $0$, $\frac{67771020491}{13168189440000}$, $\frac{112093001}{25719120000}$, $\frac{2620941641}{54190080000}$, $-\frac{1051471}{3214890000}$, $\frac{1294475515}{21069103104}$, $\frac{1498451}{105840000}$, $\frac{2802280763}{268736560000}$, $-\frac{30064892}{200930625}$, $-\frac{258248981}{2007040000}$, $\frac{8713177}{41150592}$, $\frac{554474300491}{13168189440000}$, $\frac{552779}{13230000}$, $\frac{793065538763}{13168189440000}$, $-\frac{12319207}{524880000}$, $-\frac{406439}{86704128}$, $-\frac{17493844}{200930625}$, $-\frac{7018318842613}{13168189440000}$, $-\frac{293591}{3920000}$, $\frac{1233988035659}{13168189440000}$, $\frac{784753}{5143824}$, $\frac{45465113}{1105920000}$, $\frac{480573001}{25719120000}$, $-\frac{80720141237}{13168189440000}$, $-\frac{57017}{826875}$, $-\frac{1165700869}{21069103104}$, $-\frac{702116407}{25719120000}$, $-\frac{32330133}{2007040000}$, $\frac{3852497}{65610000}$, $\frac{411640595659}{13168189440000}$, $\frac{20955}{169344}$, $\frac{914830140491}{13168189440000}$, $-\frac{20694083}{200930625}$, $-\frac{2667138359}{54190080000}$, $\frac{289718857}{25719120000}$, $\frac{877675}{429981696}$, $-\frac{35039}{490000}$, $\frac{869713757387}{13168189440000}$, $\frac{914949449}{25719120000}$, $\frac{322397513}{54190080000}$, $\frac{33560}{321489}$, $-\frac{193052019509}{13168189440000}$, $-\frac{16957}{2160000}$, $-\frac{257841421237}{13168189440000}$, $-\frac{285251471}{3214890000}$, $\frac{3355}{3211264}$, $-\frac{1474283593}{25719120000}$, $\frac{1070882317387}{13168189440000}$, $-\frac{28069}{826875}$, $-\frac{6525591109}{268738560000}$, $\frac{3395801}{41150592}$, $-\frac{991009463}{54190080000}$, $\frac{47105297}{3214890000}$, $-\frac{542711741237}{13168189440000}$, $-\frac{139863}{3920000}$, $\frac{2530605179}{21069103104}$, $-\frac{180206}{4100625}$, $-\frac{2351713591}{54190080000}$, $\frac{233669449}{25719120000}$, $\frac{435687875659}{13168189440000}$, $\frac{1675}{21168}$, $\frac{452838540491}{13168189440000}$, $-\frac{743264999}{25719120000}$, $-\frac{1888933}{40960000}$, $-\frac{5182006}{200930625}$, $-\frac{101969669}{21069103104}$, $\frac{5418451}{105840000}$, $\frac{356283917387}{13168189440000}$, $-\frac{29817647}{3214890000}$, $\frac{633037513}{54190080000}$, $\frac{98473}{839808}$, $-\frac{420947139509}{13168189440000}$, $-\frac{4153}{30625}$, $\frac{457764418763}{13168189440000}$, $-\frac{175961143}{25719120000}$, $\frac{6343769}{86704128}$, $\frac{171319121}{3214890000}$, $\frac{6941880763}{268738560000}$, $-\frac{4006957}{105841000}$, $-\frac{278910524341}{13168189440000}$, $\frac{5140}{321489}$, $-\frac{232558869}{2007040000}$, $\frac{2482893001}{25719120000}$, $\frac{944467698763}{13168189440000}$, $\frac{2747}{270000}$, $\frac{931455227}{21069103104}$, $-\frac{799316407}{25719120000}$, $\frac{1134126409}{54190080000}$, $-\frac{19744267}{200930625}$, $-\frac{849315564341}{13168189440000}$, $\frac{569}{6292}$, $\frac{23138822459}{268738560000}$, $-\frac{11104703}{3214890000}$, $\frac{514941641}{54190080000}$, $\frac{1242278857}{25719120000}$, $\frac{393361531}{21069103104}$, $-\frac{46433}{826875}$, $-\frac{391242402613}{13168189440000}$, $-\frac{30919399}{524880000}$, $-\frac{136728981}{2007040000}$, $\frac{839009}{5143824}$, $\dots$

Appendix D.   The formation of fractal polynomial of the partition function $p\left(n\right)$ as special cases of $\left\{p\left(2n, n\right)\right\}$

When a fractal family $\left\{p\left(m, n\right)\right\}$ is known, only one polynomial from that family counts $p\left(n\right)$ and that is $p\left(2n, n\right)$. In Appendix C, the forms of all the families are determined by $k = 10$. Using these, we can obtain the following results.

1) $p\left(1\right)$, we calculate from $p\left(n, 1\right) = 1$. So, $p\left(1\right) = 1$.

2) $p\left(2\right)$, we calculate from the fractal family $\left\{p\left(n, 2\right)\right\}$. The value for $n = 4$ is obtained from the function $p\left(n, 2\right) = \frac{n}{2}$. We find $p\left(2\right) = p\left(4, 2\right) = 2$.

3) $p\left(3\right)$, we calculate from the fractal family $\left\{p\left(n, 3\right)\right\}$. The value for $n = 6$ is obtained from the function $p\left(n, 3\right) = \frac{n^2}{12}$. We find $p\left(3\right) = p\left(6, 3\right) = 3$.

4) $p\left(4\right)$, we calculate from the fractal family $\left\{p\left(n, 4\right)\right\}$. The value for $n = 8$ is obtained from the function $p\left(n, 4\right) = \frac{1}{144}n^3+\frac{1}{48}n^2+\frac{16}{144}$.

We find $p\left(4\right) = p\left(8, 4\right) = 5$.

5) $p\left(5\right)$, we calculate from the fractal family $\left\{p\left(n, 5\right)\right\}$. The value for $n = 10$ is obtained from the function

We find $p\left(5\right) = p\left(10, 5\right) = 7$.

6) $p\left(6\right)$, we calculate from the fractal family $\left\{p\left(n, 6\right)\right\}$. The value for $n = 12$ is obtained from the function

We find $p\left(6\right) = p\left(12, 6\right) = 11$.

7) $p\left(7\right)$, we calculate from the fractal family $\left\{p\left(n, 7\right)\right\}$. The value for $n = 14$ is obtained from the function

We find $p\left(7\right) = p\left(14, 7\right) = 15$.

8) $p\left(8\right)$, we calculate from the fractal family $\left\{p\left(n, 8\right)\right\}$. The value for $n = 16$ is obtained from the function

We find $p\left(8\right) = p\left(16, 8\right) = 22$.

9) $p\left(9\right)$, we calculate from the fractal family $\left\{p\left(n, 9\right)\right\}$. The value for $n = 18$ is obtained from the function

We find $p\left(9\right) = p\left(18, 9\right) = 30$.

10) $p\left(10\right)$, we calculate from the fractal family $\left\{p\left(n, 10\right)\right\}$. The value for $n = 20$ is obtained from the function

We find $p\left(10\right) = p\left(20, 10\right) = 42$.

etc. See ^[16] for a different algebraic procedure.

Appendix E.   Calculating the total number of partitions for several first $n\in \mathbb{N}$ from the known coefficients $a_{k, i}$

To calculate the values of $p\left(n\right)$ we use the formula $p\left(n\right) = p\left(2n, n\right)$ and the record given with (2.2). If we take instead of $n$, $2n$ and instead of $k$, $n$ we get

where $a_{n, 1}, a_{n, 2}, ..., a_{n, n-\lambda}$ represent the coefficients of the common part which depends only on $n$. In calculation, we use only the coefficients of the common part (without the variable coefficients). The first few coefficients $a_{n, i}$ are determined by the general form in Appendix A. The number of coefficients $a_{n, i}$ to be taken is determined by $n-\lambda$, $\lambda = \left[\frac{n}{2}\right]$.

1) For $n = 1$, $\lambda = 0$, $p\left(1\right) = a_{1, 1}\cdot 1 = 1$.

2) For $n = 2$, $\lambda = 1$, only one coefficient is needed $p\left(2\right) = \frac{1}{2}\cdot 4 = 2$.

3) For $n = 3$, $\lambda = 1$, the first two coefficient are needed $p(3) = \frac{1}{12}\cdot 6^2+0\cdot 6 = 3$.

4) For $n = 4$, $\lambda = 2$, the first two coefficient are needed.

5) For $n = 5$, $\lambda = 2$, the first three coefficient are needed.

6) For $n = 6$, $\lambda = 3$, the first three coefficient are needed.

7) For $n = 7$, $\lambda = 3$, the first four coefficient are needed.

8) For $n = 8$, $\lambda = 4$, the first four coefficient are needed.

9) For $n = 9$, $\lambda = 4$, the first five coefficient are needed.

10) For $n = 10$, $\lambda = 5$, the first five coefficient are needed.

11) Case $n = 11$, $\lambda = 5$, the first six coefficient are needed.

12) Case $n = 12$, $\lambda = 6$, the first six coefficient are needed.