1.
Introduction
Anthrax is a contagious disease caused by bacteria produced by gram-positive endospores and can be spread between animals and humans [1]. The disease can infect all warm-blooded animals, especially herbivores such as cattle, sheep, goats, and horses [2,3,4,5]. When herbivores ingest a sufficient number of spores from soil and plants, they are usually infected with anthrax. Once infected, the animal will die within 24–48 hours [6,7]. Humans are infected with anthrax primarily through contact with diseased animals. Once the bacteria enters the human body, it can cause symptoms such as fever, skin lesions and breathing difficulties [7]. Anthrax spores have a strong ability to survive, which can result in contaminated soil and water becoming long-lasting sources of infection [9]. Anthrax occurs worldwide in 2004, a massive outbreak of anthrax killed up to 500 animals in Zimbabwe, and 109 animal anthrax deaths were reported in North Dakota in 2005. Between August 2009 and October 2010, there was an outbreak of 273 human cutaneous anthrax cases and 140 animal anthrax deaths in Bangladesh [10,11,12,13,14]. In a limited resource environment, anthrax has caused great harm to the development of animal husbandry and human health. As a result, anthrax has attracted widespread attention, and some progress has been made in disease control and vaccine research. In order to efficiently control the spread of anthrax, it is imperative to investigate the dynamics of the disease.
In the past few decades, various mathematical models have been intensively studied on anthrax dynamics. In previous studies, Hahn and Furniss analyzed how anthrax spreads and determined the threshold of the disease [15]. In relation to animal migration, Friedman and Yakubu addressed the basic reproduction number of the model. They examined the sufficient conditions for the presence of an endemic equilibrium point in the diffusion model [16]. Mushayabasa et al. investigated the global asymptotic stability of the endemic equilibrium point by analyzing a model that considers the vectorial impacts of anthrax transmission [17]. In fact, the spread of the virus is linked to climate, season, temperature and humidity. Due to the complexity of the environment system, the parameters of the system inevitably change randomly due to perturbations, thus making it random. Incorporating randomness into mathematical models of biological and biochemical processes is essential for gaining a deeper comprehension of the mechanisms that regulate biological systems [18]. From [19,20], Cai and Wang examined how changes in the environment affect the spread of diseases by analyzing the random fluctuations in a susceptible-infected-susceptible (SIS) model that includes media coverage. However, few studies have constructed stochastic processes into anthrax models, which facilitates the study in this paper.
Soil renovation, heavy rains, and flash floods can lead to a rise in spore levels in the surroundings, heightening the likelihood of infection [21]. It is crucial to take into account environmental disturbances when studying anthrax. Based on the existing research [7], we present a stochastic model of anthrax to explore the impact of stochastic disturbances on threshold dynamics. The stability of the new model and the probability density function in the case of disease persistence are worth studying. It is well known that the endemic equilibrium point and the basic reproduction number can reflect the persistence of the disease for identified systems. However, environmental perturbations are unpredictable, so there is no positive equilibrium in the stochastic system [22]. In this way, a stochastic anthrax model is investigated for the stationary distribution of disease persistence. In practice, the statistical nature of the model can lead to prevention and control the disease easier [22,23]. However, the resolution of the high-dimensional Fokker-Planck equation presents a challenging task, and only a limited number of research endeavors have been dedicated to addressing the computation of the probability density function. To close this gap, in this paper we employ the four-dimensional Fokker-Planck equation to investigate the probability density function for the stochastic anthrax model. Our article has the following contributions:
● On the basis of existing research [7], we propose a stochastic anthrax model. Sufficient conditions for the existence of an ergodic stationary distribution of globally positive solutions are established.
● The probability density function expression has been explored by few studies due to the difficulty of solving the high-dimensional Fokker-Planck equation. Therefore, this study investigates the precise representation of the probability density function in the vicinity of the quasi-equilibrium point of the model when a persistent disease is present.
The rest of our paper is arranged as follows. Section 2 introduces the mathematical models and gives the relevant notation and lemma. Section 3 proves the unique ergodic stationary distribution existence when Rs>1. In Section 4, when Rs>1, the probability density function is obtained by solving the Fokker-Planck equation in four dimensions. Section 5 provides the numerical simulations for our results of the analysis. The main results are discussed in Section 6.
2.
Model formulation and preliminaries
2.1. Anthrax model formulation
Understanding the transmission mechanisms of anthrax epidemics requires the use of mathematical models as an important tool. As it is known that there are different forms of anthrax models, here we consider the following anthrax model [7]:
where S(t) and I(t) are the number of susceptible and infected animals at time t, respectively. N(t)=S(t)+I(t) denotes the total live animal population at time t, A denotes grams of spores in the environment, and C denotes the number of infected carcasses. The birth rate of animals is r>0, K is the animal carrying capacity, and rN(1−NK) is born into the susceptible class per unit time. ηa is the transmission rate of environment to susceptible animals, ηc is the transmission rate of infected carcasses to susceptible animals, and ηi is the transmission rate of infected animals to susceptible animals, so ηa>0,ηc>0,ηi>0. μ is the death rate, γ is the disease death rate, α is the spore decay rate, β is the spore growth rate, δ the is carcass consumption rate, κ is the carcass decay rate, and τ denotes the recovery rate of infected animals.
Assume that ηiγ+μ+τ<1, otherwise the anthrax increase is unbounded. On account of the total live animal population as N(t)=S(t)+I(t), adding the equation S(t) and I(t) results in
and if r>μ, this implies that lim supt→∞N(t)⩽K(1−μr)=S0.
Assuming 0<N(0)⩽S0, the positive invariant is
The disease-free equilibrium point E0 and the endemic equilibrium point E∗ are obtained by solving the equation. E0=(S0,0,0,0),E∗=(S∗,I∗,A∗,C∗), where
N∗=S0−Kγr(1−1D) with D=ηiγ+μ+τ+γ+μγ+μ+τηcN∗δN∗+κ+γ+μγ+μ+τβηaN∗α(δN∗+κ), and the basic reproduction number of Model (2.1) is
According to Saad-Roy et al. [7], the main results about the stability of the equilibrium point are as follows:
(i) If R0<1, the disease-free eqilibrium E0=(S0,0,0,0) is globally asymptotically stable; thus a small outbreak of anthrax is eradicated. If R0>1, the disease-free equilibrium is unstable; thus, anthrax persists.
(ii) If R0>1, there has a unique endemic equilibrium with E∗=(S∗,I∗,A∗,C∗), which is globally asymptotically stable in Γ.
In real life, the dynamic behaviors of anthrax are disturbed by random factors in nature. In this paper, we consider random noise on the basis of the Model (2.1) and adopt the method used by Imhof et al. [24]. Our hypothesis posits a direct correlation between stochastic fluctuations and S(t),I(t),A(t),C(t). Thus, the stochastic model for anthrax transmission is to be described in the following form:
where σi (i=1,2,3,4) represents the intensities of the white noises and the definition of Bi(t) (i=1,2,3,4) is based on complete probability space and is independent of standard Brownian motions. The transmission diagram illustrating the dynamics of anthrax transmission is shown in Figure 1. After giving the basic information of the anthrax model, the relevant lemma is given in the following section.
2.2. Notations and lemmas
Throughout this paper, we use X(t)=(S(t),I(t),A(t),C(t)) as the solution of Model (2.2) with the initial value X(0)=(S(0),I(0),A(0),C(0)). Let (Ω,F,{Ft}t⩾0,P) be the probability space with the filtration {Ft}t⩾0 satisfying the usual conditions that are right continuous, and F0 contains all P-null sets. Let R4+ be an-dimensional Euclidean space and Un=[1n⋅n]×[1n⋅n]×[1n⋅n]×[1n⋅n].
Our focus in this section is on the existence and uniqueness of a globally positive solution. In order to achieve this goal, Lemma 2.1 is introduced to demonstrate the existence of a positive solution to the Model (2.2). The proof of the existence theorem for positive solution can be obtained in terms of Mao and Bahar [25,26], so the proof is omitted here.
Lemma 2.1. For any initial value X(0)=(S(0),I(0),A(0),C(0))⊆R4+, Model (2.2) has a unique global positive solution X(t)=(S(t),I(t),A(t),C(t)),t⩾0, and the solution will remain in R4+ with probability one.
Based on the existence of the positive solution, the next step we will concentrate on is the asymptotic estimate of the Model (2.2).
Lemma 2.2. If for all ϵ>1, the following inequalities hold
there exists a positive constant ˆT(ϵ) such that the solution X(t)=(S(t),I(t),A(t),C(t)) with initial value X(0)⊆R4+ has the following property lim supt→∞E|X(t)|⩽ˆT(ϵ). For this expression, the solution X(t) is stochastically ultimately bounded.
The proof of Lemma 2.2 is shown in the Appendix.
Let X(t) be a regular time-homogeneous Markov process in Rl described by the following stochastic differential eqaution: dX(t)=b(X)dt+∑kr=1gr(X)dBr(t). The diffusion matrix is defined as follows: B(X)=((bi,j(X))),bi,j(X)=∑kr=1girgjr(X), where X(t) is nonsingular [27], then we have the following lemma.
Lemma 2.3. (Khasminskii [27]). The Markov process X(t) has a unique ergodic stationary distribution v(⋅) if there exists a bounded open domain U⊂Rl with regular boundary Γ and if it has the following properties:
(i) There exists a positive ˉE such that ∑ni,j=1bij(X)ξiξj⩾ˉE|ξ|2, X∈U, ˉE∈Rl;
(ii) There exists a nonnegative C2-function V such that LV is negative for any X∈Rl∖U.
Thus the Markov process X(t) has a stationary distribution v(⋅). Let f(x) be a function integrable with respect to the measure π, for all x∈Rl. We can get
According to the Routh-Hurwitz stability principle and Zhou et al. [28], the following algebraic equation solving theory is given.
Lemma 2.4 (Zhou et al. [28]). For the four-dimensional real matrix D=diag(σ1,σ2,σ3,σ4), Z=(aij)4×4, the characteristic polynomial of Z is ωZ(ζ)=ζ4+s1ζ3+s2ζ2+s3ζ+s4. Assuming that Λ=(νij)4×4 is a symmetric matrix, we have the following algebraic equation
If Z has all negative real part eigenvaues, that is,
then Λ is a positive definite matrix.
Using Lemmas 2.5–2.7, we will develop solutions for the four dimensions as described by Tan et al. [23] and Zhou et al. [29].
Lemma 2.5 (Zhou et al. [29]). For the algebraic equation G20+B1X1+X1BT1=0 and G0=diag(1,0,0,0),
where r22=a32(a1(a2a3−a1a4)−a23),r13=−r22,r33=a1a3r22,r11=a2a3−a1a4a3r22,r24=−r33,r44=a1a2−a3a3a4r22. If a1>0, a3>0,a4>0 and a1a2−a3>0,a1(a2a3−a1a4)−a23>0, then the matrix X1 is postive definite.
Lemma 2.6 (Tan et al. [23]). For the algebraic equation G20+B2X2+X2BT2=0 and G0=diag(1,0,0,0),
where ˜r22=12(b1b2−b3),˜r13=−˜r22,˜r11=b2˜r22,˜r33=b1b3˜r22. If b1>0,b3>0 and b1b2−b3>0, then the matrix X2 is semi-postive definite.
Lemma 2.7 (Tan et al. [23]). For the algebraic equation G20+B2X2+X2BT2=0 and G0=diag(1,0,0,0),
where ˜r11=12c1,˜r22=12c1c2. If c1>0 and c2>0, then the matrix X3 is semi-postive definite.
For a stochastic model, stability is the basis for studying other properties, so the stationary distribution is an important research content. Thus, the ergodic stationary distribution of the Model (2.2) is studied in the following section.
3.
Stationary distribution
Due to the complex structure of nonlinear systems, it is generally difficult to solve their state equations. Therefore, the Lyapunov method has been widely applied [30,31,32,33,34,35]. In this section, within the framework of the stochastic Lyapunov method, we will provide sufficient conditions for the ergodicity of the global positive solution and the existence of a stationary distribution.
Define
where Rs is a stochastic threshold for Model (2.2). Next, its ergodicity is discussed using threshold.
Theorem 3.1. If Rs>1, for any initial value (S(0),I(0),A(0),C(0))∈R4+, then Model (2.2) has a unique stationary distribution v(·) and it follows the ergodic property.
Proof. The diffusion matrix of Model (2.2) is given by
where X=(S,I,A,C). Choose ˉE=min(SI,A,C)∈ˉU⊂R4+{σ21S2,σ22I2,σ23A2,σ24C2}, then we have ˉE>0, where ˉU=[1n⋅n]×[1n⋅n]×[1n⋅n]×[1n⋅n]. For any (S,I,A,C)∈ˉU and (ξ1,ξ2,ξ3,ξ4)∈R4+, from (3.1), we have
where |ξ|=(ξ21+ξ22+ξ23+ξ24)12, then the condition (i) in Lemma 2.3 holds.
Construct the following function:
and the positive constants mi (i=1,2,3,4) will be determined at a later stage. By Itˆo's fomular, it can be obtained that
Let
then we can get
Thus,
Define the function
where W2=S+I,W3=−lnS,W4=−lnI,W5=−lnA, and W6=1p+1(S+I)p+1. Select a suitable constant M>0 satisfying the following condiction
where
It is easy to check that
where Un=[1n⋅n]×[1n⋅n]×[1n⋅n]×[1n⋅n]. Furthermore, W(S,I,A,C) is a continuous function. Hence, (S,I,A,C) must have a minimum point (ˉS0,ˉI0,ˉA0,ˉC0) in the interior of R4+, then we define a nonnegative function ˉW:R4+→R as follows
By utilizing Itˆo's formula, we can derive the following result
and
Choose 0<p<min{1,μσ21∧μ+γσ22}, then we can see that
hence,
Next, we form a condensed subset D in order to satisfy the condition (ii) as outlined in Lemma 2.3. Define the bounded closed set
where ξ is a suffciently small constant. Choose ξ satisfying the following conditions
where
Divide R4+∖D into six domains:
We aim to demonstrate that the inequality LˉW(S,I,A,C)⩽−1 holds true on the domain R4+∖D. This is equivalent to establishing its validity on the six specified domains.
Case 1: If (S,I,A,C)∈D1, one can see that
Case 2: If (S,I,A,C)∈D2, one can see that
Case 3: If (S,I,A,C)∈D3, one can see that
Case 4: If (S,I,A,C)∈D4, one can see that
Case 5: If (S,I,A,C)∈D5, one can see that
Case 6: If (S,I,A,C)∈D6, one can see that
Hence, we obtain LˉW⩽−1 for all (S,I,A,C)∈R4+∖D. According to Lemma 2.3, it can be deduced that the Model (2.2) possesses a unique stationary distribution denoted as v(·). This serves to conclude the proof.
Remark 1. As we know, a deterministic system disease persistence is measured according to the basic reproduction number R0 and the endemic equilibrium point E∗. However, in a stochastic model, there is no positive equilibrium due to unpredictable noise perturbations. Therefore, the persistence of the disease in a stochastic system is reflected by the presence of an ergodicity stationary distribution. According to Theorem 3.1, Model (2.2) has a unique stationary distribution when Rs>1. Comparing the expressions for R0 and Rs, we find that Rs⩽R0 and the presence of disease in both the model and the stochastic model can be assessed using a consistent measure of Rs>1.
Remark 2. Model (2.2) characterizes the impact of random noise on the spread of anthrax, which is an extension of Model (2.1). When random noise is absent, i.e., σi=0 (i=1,2,3,4), Model (2.2) degenerates into Model (2.1).
4.
Probability density function
This section aims to derive the precise formulation of the density function for Model (2.2) at a quasi-equilibrium point. It is important to emphasize that the probability density function serves as a representation of the dynamic attributes of a stochastic system with statistical relevance. Our main goal is to ascertain the probability density function for the purpose of examining the dynamics of the model from a statistical perspective.
Before proving the probability density function, two equivalent differential equation forms for Model (2.2) are given. Let x1=lnS,x2=lnI,x3=lnA,x4=lnC. By Itˆo's formula, an equivalent model can be obtained:
Let b1=μ+σ212,b2=γ+μ+τ+σ222,b3=α+σ232,b4=κ+σ242. If
holds, Model (2.2) admits a quasi-steady ˉE1=(ˉS1,ˉI1,ˉA1,ˉC1)=(ex∗1,ex∗2,ex∗3,ex∗4), where ˉI1 is the positive root of the equtaion
and
In addition, assume if Rs>1, then ˉRs>1, which means that Model (2.2) admits a quasi-steady ˉE1 under the condition Rs>1. Let
where x∗1=lnˉS1,x∗2=lnˉI1,x∗3=lnˉA1,x∗4=lnˉC1, then the linearized system of (4.1) is as follows:
where
By employing the above lemmas, we will demonstrate the probability density function of the Model (2.2) near the quasi-steady equilibrium ˉE1.
Let Y=(y1,y2,y3,y4)T,D=diag(σ1,σ2,σ3,σ4), and B(t)=(B1(t),B2(t),B3(t),B4(t))T. We can rewrite Model (4.3) as the following form:
where
then the linearized Model (4.3) can be simiplified to dY=ZYdt+DdB(t). Based on the pertinent theory proposed by Zhou et al., it is postulated that there is a probability density function denoted as Φ(y1,y2,y3,y4) in the vicinity of the quasi-endemic equilibrium ˉE1.
Theorem 4.1. [Zhou et al. [22]] Assuming that Rs>1 for any initial value (S(0),I(0),A(0),C(0)∈R4+, the solution (S(t),I(t),A(t),C(t)) of the Model (2.2) follows the unique log-normal probability density function Φ(S,I,A,C) around the quasi-endemic equilibrium ˉE1, which is described by
where Λ is a positive definite matrix.
From Gardiner [36], the density function U(Y)=U(y1,y2,y3,y4) of the quasi-stationary distribution of Model (4.3) at the origin Y∗=(0,0,0,0) can be described by the four-dimensional Fokker-Planck equation as follows:
which can be approximated by a Gaussian distribution
ˉM is a real symmetric matrix, which is described by ˉMD2ˉM+ZTˉM+ˉMZ=0. If ˉM is positive definite, let ˉM−1=Λ, then
According to Tian et al. [37], (4.4) can be rewriten as the sum of the four equations as follows:
where D1=(σ1,0,0,0),D2=(0,σ2,0,0),D3=(0,0,σ3,0),D4=(0,0,0,σ4),Λ=Λ1+Λ2+Λ3+Λ4, D2=D21+D22+D23+D24. Consider the corresponding characteristic equation of Z as follows:
where
In s2, the following formula can be calculated
In s3, the following formula can be calculated
In s4, the following formula can be calculated
where τ−ηiˉS21ˉN21>0 and
while s1>0, s2>0, and s3>0. We can calculate s1s2−s3>0 and s1(s2s3−s1s4)−s23>0. As per the Routh-Hurwitz stability criterion [38], it can be deduced that the matrix Z possesses eigenvalues with negative real parts. According to the theory of matrix similar transformations, it is evident that ωZ(ζ) is a similarity invariant, indicating that s1,s2,s3, and s4 are also similarity invariants.
Next, the corresponding proof for the positive definiteness of Λ is divided into four steps.
Proof. Step 1. Consider the algebraic equation
Let Z1=U1ZU−11, where
and
where w1=−a33a41a21,w2=−a41−a42+a41a44a21−a24a241a221.
Case 1: When w1≠0 and w2≠0, there exists the elimination matrix U11 such that Z11=U11Z1U−111, where
then there is
where w3=w2w1a33w1−a33w2w1+w2w1a24a41−a21a44a21+a23a41a21.
Case 1-1: If w3≠0, in order to find the standard transformation matrix Q111 such that T111=Q111Z11Q−1111, where
with
Thus, we can get
Meanwhile, (4.6) has the following form:
That is, D20+T111Θ1+Θ1TT111=0, where Θ1=1ρ2111(Q111U11U1)Λ1(Q111U11U1)T, ρ111=a21w1w3σ1.
According to Lemma 2.5, we can obtain the semipositive definite matrix
Therefore, it can be calculated that
is positive definite.
Case 1-2: If w3=0, we can find the standard transformation matrix Q112 such that T112=Q112Z11Q−1112, where
with
We have
where bi(i=1,2,3,4) are marks, and we only care about their traits. According to the uniqueness of ωZ(ζ), it can be obtained that
The following results can be obtained that b1b2−b3>0, then (4.6) can be transformed into the following form:
That is, D20+T112Θ2+Θ2TT112=0, where Θ2=1ρ2112(Q112U11U1)Λ1(Q112U11U1)T, ρ112=a21w1σ1.
According to the Lemma 2.6, we can obtain the semipositive definite matrix
Therefore, by calculation,
is semi-postive definite.
Case 2: When w1≠0 and w2=0, the objective is to determine the standard transformation matrix Q12 that satisfies the equation T12=Q12Z1Q−112, where
with
We can obtain that T12=T111, and (4.6) can be transformed into
where Θ1=1ρ212(Q12U1)Λ1(Q12U1)T, ρ12=−a23a33a241a21σ1. Consequently,
is a positive definite matrix.
Case 3: When w1=0 and w2≠0, there exists the transformation matrix U13 such that Z13=U13Z1U−113, where
then we have
we are able to find the standard transformation matrix Q13 such that T13=Q13Z13Q−113, where
with
We can obtain that T13=T111, and (4.6) can be transformed into
where Θ1=1ρ213(Q13U13U1)Λ1(Q13U13U1)T, ρ13=−a21w2σ1. Consequently,
is a positive definite matrix.
Case 4: When w1=0 and w2=0, there exists the transformation matrix U14 such that T14=Q14Z1Q−114, where
with
We then have
c1=a11+a21+a24a41a21>0, c2=(a11+a12)a21+(a41+a42)a24−a14a41+a11a24a41a21+a23a33a41a21+a224a241a221>0. Thus, (4.6) can be transformed into
where Θ3=1ρ214(Q14U1)Λ1(Q14U1)T, ρ14=a21σ1. With Lemma 2.7, we can obtain the semi-postive definite matrix
Therefore, by calculation,
is semi-postive definite.
Step 2. For the following algebraic equation
consider the corresponding order matrix J2 and elimination matrix U2:
Let Z2=(U2J2)Z(U2J2)−1, then we can obtain that
where r1=−a41+a14a44+a42a44a33, r2=−a11+a13a14+a12a44+a214a33.
Case 1: When r1≠0,r2≠0, there exists the elimination matrix U21 such that Z21=U21Z2U−121, where
then we have
where r3=(a42+a44r2r1)r2r1−a12−(a13+a14)r2r1.
Case 1-1: If r3≠0, the standard transformation matrix Q211 can be determined in order to obtain the transformation T211 as T211=Q211Z21Q−1211, where
with
As a result, we have T211=T111, and (4.12) has the following form:
That is, D20+T211˜Θ1+˜Θ1T211=0, where ˜Θ1=1ρ2211(Q211U21U2J2)Λ2(Q211U21U2J2)T, ρ211=a33r1r3σ2. According to Lemma 2.5, we can obtain the semipositive definite matrix
Therefore, it can be obtained by calculation that
Case 1-2: If r3=0, we can find the standard transformation matrix Q212 such that T212=Q212Z21Q−1212, where
then we have
where
We can obtain ˜b1˜b2−˜b3>0, then (4.12) can be transformed into the following form:
That is, D20+T212˜Θ2+˜Θ2TT212=0, where ˜Θ2=1ρ2212(Q212U21U2J2)Λ2(Q212U21U2J2)T, ρ212=a33r1σ2. According to Lemma 2.6, we can obtain the semipositive definite matrix
Therefore, it can be obtained by calculation that
is semi-postive definite.
Case 2: When r1≠0 and r2=0, there exists the transformation matrix Q22 such that T22=Q22Z2Q−122, where
with
It can be obtained that T22=T111, and (4.12) can be transformed into
where ˜Θ1=1ρ222(Q22U2J2)Λ2(Q22U2J2)T, ρ22=−a21a33r1σ2. Consequently,
is a positive definite matrix.
Case 3: When r1=0 and r2≠0, there exists the transformation matrix U23 such that Z23=U23Z2U−123
then we have
We can find the standard transformation matrix Q23 such that T23=Q23Z23Q−123, where
with
It can be obtained by calculation that T23=T111, and (4.12) can be transformed into
where ˜Θ1=1ρ223(Q23U23U2J2)Λ2(Q23U23U2J2)T, ρ23=−a33a44r2σ2. Consequently,
is a positive definite matrix.
Case 4: When r1=0 and r2=0, there exists the transformation matrix Q24 such that T24=Q24Z2Q−124, where
then we have
where c1=a11+a21+a33+a44−a13−a14−a42>0, c2=s4a13a42+a14a42−a12a44>0. Thus, (4.12) can be transformed into
where ˜Θ3=1ρ224(Q24U2J2)Λ2(Q24U2J2)T, ρ24=a33σ2. With Lemma 2.7, we can obtain the positive semidefinite matrix
Therefore, it can be obtained by calculation that
is semi-postive definite.
Step 3. For the following algebraic equation
consider the corresponding order matrix J3 and elimination matrix U3 :
Let Z3=(U3J3)Z(U3J3)−1. It can be obtained by calculation that
where k1=a21+a21a23−a11a23a13+a12a223a213, k2=a24−a14a23a13.
Case 1: When k1=0 and k2≠0, there exists the standard transformation matrix Q31 such that T31=Q31Z3Q−131, where
with
We can obtain that T31=T111, and (4.18) can be transformed into
where ˉΘ1=1ρ231(Q31U3J3)Λ3(Q31U3J3)T, ρ31=(a13a41−a23a42)k2σ3, and ˉΘ1=Θ1. Consequently,
is positive definite matrix.
Case 2: When k1≠0, k2=0, there exists the transformation matrix U32 such that Z32=U32Z3U−132, where
then we have
where k3=(a13a44+a12a23+a13a21)k1a23a42−a13a41−a213a42k21(a23a42−a13a41)2.
Case 2-1: If k3≠0, we can find the standard transformation matrix Q321 such that T321=Q321Z32T−1321, where
with
It can be obtained by calculation that T321=T111, and (4.18) can be transformed into
where ˉΘ1=1ρ2321(Q321U32U3J3)Λ3(Q321U32U3J3)T, ρ321=(a13a41−a23a42)k3σ3. Consequently,
is positive definite matrix.
Case 2-2: If k3=0, we can find the standard transformation matrix Q322 such that T322=Q322Z32Q−1322, where
with
We can find
which means that
we can find that ˉb1ˉb2−ˉb3>0, and (4.18) can be transformed into the following form :
That is, D20+T322ˉΘ2+ˉΘ2TT322=0, where ˉΘ2=1ρ2322(Q322U32U3J3)Λ3(Q322U32U3J3)T, ρ322=(a13a41−a23a42)σ3. According to Lemma 2.6, we are able to obtain the semipositive definite matrix
Therefore, it can be obtained by calculation that
is semi-postive definite.
Case 3: When k1=0 and k2=0, there exists the transformation matrix Q33 such that T33=Q33Z3Q−133, where
with n∗3=−a13a33+a14(a11+a44−a12a23a13) and n∗4=a12(a11+a13a21a13)+a14a42, then we can find
where
Thus, (4.18) can be transformed into
where ˉΘ3=1ρ233(Q33U3J3)Λ3(Q33U3J3)T, ρ33=−a13σ3. With Lemma 2.7, we can obtain the positive semi-definite matrix
Therefore, it can be obtained by calculation that
is semi-postive definite.
Step 4. For the following algebraic equation
consider the corresponding order matrix J4 and elimination matrix U4:
Let Z4=(U4J4)Z(U4J4)−1. It can be obtained that
where p1=−a33+a21a33a12, p2=a33.
Case 1: When p1=0 and p2≠0, it is possible to determine the standard transformation matrix Q41 in order to satisfy the equation T41=Q41Z4Q−141, where
with
then it can be obtained by calculation that T41=T111, and (4.23) can be transformed into
where ˆΘ1=1ρ241(Q41U4J4)Λ4(Q41U4J4)T, ρ41=(a13a21−a14a221a12)a33σ4, and ˆΘ1=Θ1. Consequently,
is positive definite matrix.
Case 2: When p1≠0 and p2=0, there exists the transformation matrix U42, then Z42=U42Z4U−142, where
then we can find
where p3=a33+a33(a12−a21)(a12a24+a21a41)a12a13+a14a21−a12a233(a11+a12)(a12−a21)2a21(a12a13+a14a21)2.
Case 2-1: If p3≠0, we can find the standard transformation matrix Q421 such that T421=Q421Z42Q−1421, where
with
then we can obtain that T421=T111, and (4.23) can be transformed into
where ˆΘ1=1ρ2421(Q421U42U4J4)Λ4(Q421U42U4J4)T, ρ421=−a21(a12a13+a14a21)a12σ4. Consequently,
is positive definite matrix.
Case 2-2: If p3=0, we are able to find the standard transformation matrix Q422 such that T422=Q422Z42Q−1422, where
with
We then have
which means that
ˆb1ˆb2−ˆb3>0, and (4.23) can be transformed into the following form :
That is, D20+T422ˆΘ2+ˆΘ2TT422=0, where ˆΘ2=1ρ2422(Q422U42U4J4)Λ4(Q422U42U4J4)T, ρ422=−a21(a12a13+a14a21)a21σ4. According to Lemma 2.6, we can find the semipositive definite matrix
Therefore, we can obtain that
is semi-postive definite.
Case 3: When p1=0 and p2=0, there exists the transformation matrix Q42 such that T43=Q42Z4Q42, where
with
We then have
where
Thus, (4.23) can be transformed into
where ˆΘ3=1ρ243(Q43U4J4)Λ4(Q43U4J4)T, ρ43=−a12σ4. With Lemma 2.7, we can obtain the positive semidefinite matrix ˆΘ3
Therefore, it can be obtained by calculation that
is semi-postive definite.
In summary, Λ3, Λ4 are positive definite and Λ1,Λ1 are at least semi-positive definite. Hence, Λ=Λ1+Λ2+Λ3+Λ4 is a positive definite matrix, and there is a unique and precise density function surrounding the quasi-endemic equilibrium ˉE when Rs>1. Considering the transformation of (y1,y2,y3,y4)=(lnSˉS1,lnIˉI1,lnAˉA1,lnCˉC1) and (S,I,A,C), the unique ergodic stationary distribution v(⋅) of Model (2.2) approximately follows a unique log-normal probability density function
This completes the proof of Theorem 4.1.
Remark 3. By combining Theorems 3.1 and 4.1, it is possible to find evidence that the stationary distribution of Model (2.2) around the equilibrium ˉE1 is compatible with the probability density function Φ(S,I,A,C). Hence, a reasonable stochastic criterion for disease persistence can be achieved when Rs>1. From this we can see that accurately representing the density function can be an efficient method for preventing and managing numerous epidemics.
5.
Numerical simulations
In this section, we apply theoretical findings to analyze the dynamics of anthrax and investigate the impact of environmental disturbance on the transmission of the disease. Using by Milstein's method [], the discretization equation of Model (2.2) is given by
where the time increment is Δt>0, and χk,πk,ωk,ζk(k=1,...,n) are the independent Gaussian random variables following the distribution N(0,1). As stated by Saad-Roy et al. [7] and Mushayabasa et al. [17], Table 1 displays the relevant biological parameters and the initial value of Model (2.2). Next, our objective is to perform empirical examples to concentrate on the following three aspects:
1. If Rs>1, there is a unique ergodicity property stationary distribution.
2. Stochastic fluctuations have an impact on disease persistence in the Model (2.2).
3. Focus on the probability density function of Model (2.2).
5.1. Dynamical behaviors of Model (2.2) under Rs>1
The main parameters are given in Table 1. Let the stochastic perturbations be (σ1,σ2,σ3,σ4)=(0.005,0.005,0.005,0.005) in Model (2.2) then we can calculate that
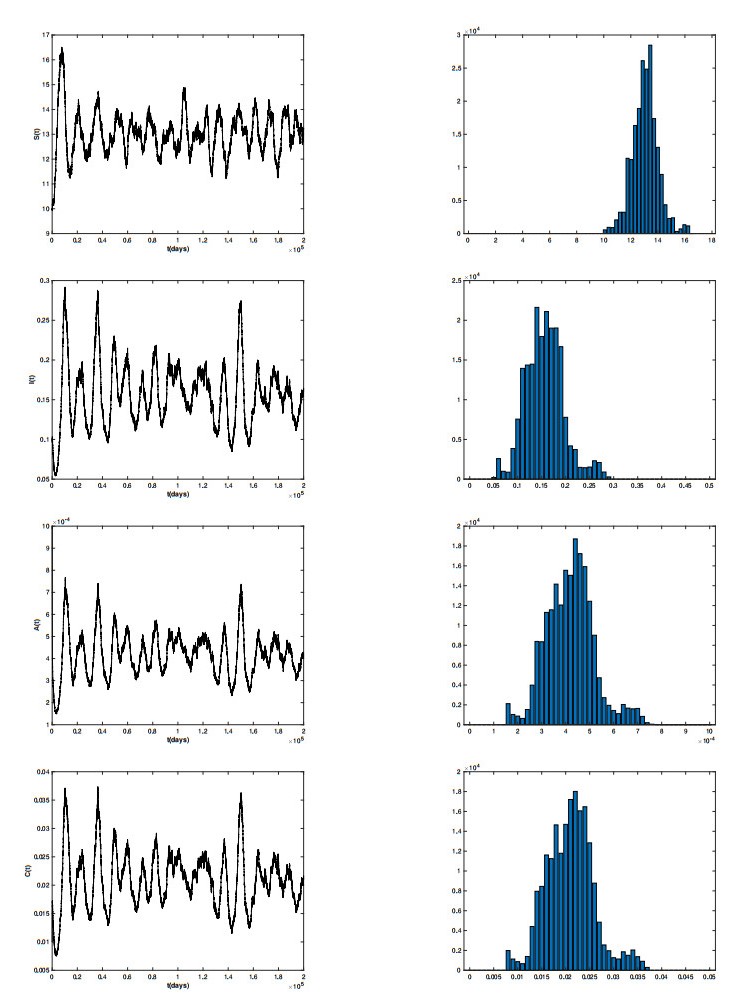
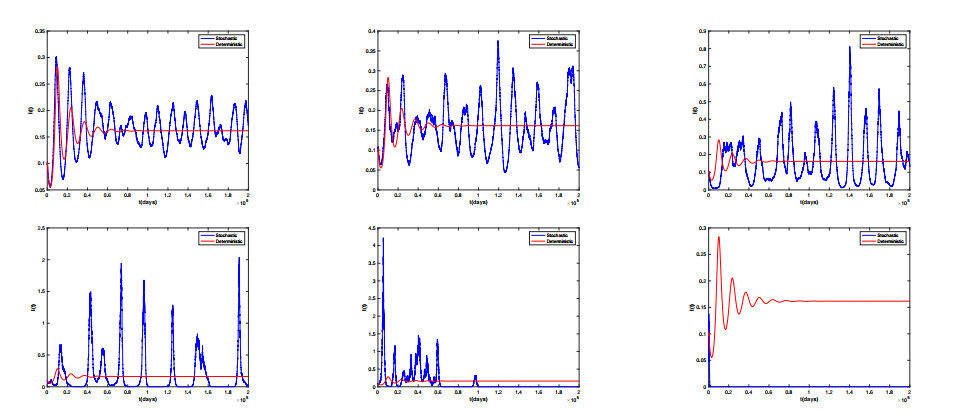
The disease has a tendency to persist in both deterministic and stochastic models according to Theorem 3.1. It can be inferred that Model (2.2) has a stationary distribution v(⋅). In Figure 2, we give the time series diagram of the model solution and its corresponding histogram. In the time series diagram in Figure 2, the curve fluctuates up and down, but the overall trend is stable. The corresponding interval of frequency can be seen in the histogram on the right. It is shown that the Model (2.2) has a unique ergodic stationary distribution, which is consistent with the result of Theorem 3.1. This indicates that the disease will endure. In real life, anthrax is not easy to be completely eradicated, but in a relatively stable state. Next, the dynamical behavior is observed when the parameters of the stochastic and deterministic models are identical. It is shown that the stochastic path fluctuates around the deterministic path, which indicates that the stochastic Model (2.2) has an ergodic stationary distribution. Figure 3 confirms this.
5.2. Impact of random noises σ1,σ2,σ3,σ4 on the disease persistence
Mathematical limitations make it tough to establish sufficient conditions for disease extinction in the stochastic anthrax model [42,43]. Therefore, for a comprehensive discussion, we present numerical simulation of disease elimination with high noise. For example, to prevent the spread of anthrax, people can increase the culling rate or vaccination rate, which will effectively eliminate the spread of anthrax.
We use numerical simulations to prove that excessive environmental noise can lead to the extinction of anthrax. Let σ=σ1=σ2=σ3=σ4, σ be taken as 0.002, 0.005, and 0.01, respectively. The other parameters and initial value are identical to those in Table 1 and the simple calculations show that Rs>1. It can be concluded that the disease persists; if we let σ=0.02,0.05,0.1, studies have shown that large enough environment noise will eradicate the disease, and the numerical simulation clearly shows our conclusion in Figure 4.
5.3. The probability density function of Model (2.2)
In this part, the numerical representation of the probability density function of Model (2.2) will be our focus. From Theorem 3.1, we can calculate that if (σ1,σ2,σ3,σ4)=(0.005,0.005,0.005,0.005), the Model (2.2) has a unique stationary distribution. Moreover, through meticulous computation, it is determined that the variables' values in Table 2 are all positive. By the definition of ˉE1, we obtain that (ˉS1,ˉI1,ˉA1,ˉC1)=(24.8194,0.4094,0.00044,0.02852). According to Theorem 4.1, we know that the distribution has a probability density function around the quasi-steady state ˉE1, and the stationary distribution v(⋅) obeys a log-normal density function Φ(S,I,A,C). Its function expression is:
and the corresponding marginal density functions are :
By constructing frequency histograms and fitting curves for the variables S,I,A, and C, the marginal density functions F(S), F(I), F(A), and F(C) were plotted. The numerical findings depicted in Figure 5 validate the assertion that the fitting curves closely align with the corresponding theoretical marginal density functions.
6.
Conclusions and discussion
To explore the possibility of an ergodic stationary distribution and a representation of the probability density function, we propose the stochastic anthrax model in this study. We will discuss our primary contributions in the two areas.
By considering the effect of external environmental perturbations, we focus on the stochastic anthrax model. First, we calculate the basic reproduction number of the deterministic model R0, if R0<1, which means that the anthrax will be extinct in the animal population, while R0>1 implies that anthrax will persistence. For stochastic Model (2.2), the uniform boundedness of the model is presented. Second, we demonstrate that the stochastic anthrax model has a unique ergodic stationary distribution at the threshold Rs>1 in Lemma 2.3 and Theorem 3.1 by building appropriate Lyapunov functions and applying ergodic theory. From the similar threshold and basic reproduction number, Rs>1 can be seen as a unified criterion to ensure the persistence of disease in deterministic and stochastic models. Comparing the expressions for R0 and Rs, we find that Rs⩽R0, so Rs>1 can be viewed as a uniform measure to determine the persistence of the disease in both the model and the stochastic model. In addition, by setting up noise perturbations of different intensity, we found that the higher the noise level, the faster the disease extinction. That is, external disturbances play an important role in disease development, such as environmental elimination, culling of infected animals, and vaccination.
Understanding the statistical characteristics is crucial for managing infectious diseases, but it is challenging to ascertain the statistical properties of disease persistence within an ergodic stationary distribution. To study the dynamic behavior of disease, we construct the endemic equilibrium point, through the equivalence between the Model (4.1) and the corresponding linear Model (4.3) and deduced the log-normal accurate expression of Φ(S,I,A,C) four-dimensional density function. Furthermore, the covariance matrix Λ is determined through the use of an algebraic equation D2+ZΛ+ΛZT=0. The precision of Λ can be verified by applying the stability theory to the zero solution of the linear equation, as discussed in the previous research [39]. Furthermore, our method and theory can demonstrate that Λ is positive definite, even in cases where D is a semi-definite matrix.
Finally, there are several important themes that deserve further study. To begin, the stochastic model established in this paper only considers the effect of white noise. However, Leˊvy noise can describe the model more accurately when there is a sudden change in the environment. On the other hand, time delay is an important factor affecting the stability of ecological dynamic systems, as time delay may alter the stability of the system and lead to system fluctuations [43,44]. Therefore, considering Lvy noise and delay effects has theoretical value and practical significance. We leave these for future work.
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Acknowledgments
The research was supported by the Ningxia Natural Science Foundation (No. 2022AAC03265), and Natural Science Foundation of China (No. 12161068).
Conflict of interest
The authors declare there is no conflicts of interest.
Appendix 1 : The proof of Lemma 2.1
Proof. Denote the Lyapunov function : , for , applying It's formula
where
Let
It follows from () that there exists the positive constant such that . Hence, we can obtain that
which yields that . Integrating both sides of the equation and taking expectations leads to the following
Thus, , as a result, In addition, when , we have , which is the second moment of the solution of Model (). Now, for any , let . By Chebyshev's inequality, , which yields that . Therefore, we have . In other words, the solution of Model () is stochastically ultimately bounded.
Appendix 2 : Theory in obtaining standardized transformation matrix
Through the use of invertible linear transformations, we will derive the corresponding standardized transformation matrices of standard . The matrix of : For the algebraic equation , where and
We assume that , and . Define , which follows . Considering the following vector ,
then the corresponding strandardized transformation matrix is given by
We derive that , which means , then we have
Therefore, we can obtain that the corresponding standard matrix is , which refers to Lemma 2.5. Let and .









 DownLoad:
DownLoad: