Persistence of the heteroclinic loop under periodic perturbation

1.   Introduction

The problems in homoclinic or heteroclinic bifurcation are critical in dynamic systems because they may have some complex dynamic behavior, such as chaotic motions ^[1]. Homoclinic and heteroclinic orbits are important invariant sets. The homoclinic orbit tends asymptotically to the same hyperbolic equilibrium along stable and unstable manifolds. However, the heteroclinic orbit tends asymptotically to two different hyperbolic equilibria along the stable and unstable manifolds. A heteroclinic loop consists of two saddles connecting two heteroclinic orbits. A numerical simulation reveals that the Lorenz equation has a heteroclinic loop when $\sigma = 10$, $r\approx 40.375$ and $b\approx 2.623$ ^[2]. The heteroclinic loop is equidimensional if the two saddles have the same dimension of the unstable manifold. Otherwise, it is heterodimensional loop ^[3]. This elementary phenomenon occurs in any dimension larger than two, and is one of the primary mechanisms for non-hyperbolicity. In addition, the existence of the heteroclinic loop is often related to the traveling wave solutions of the reaction-diffusion equation.

In ^[4], Han et al. considered quadratic Hamiltonian systems with a heteroclinic loop under polynomial perturbations. Using the Melnikov function, the authors found three limit cycles near the heteroclinic loop. Later, Sun, Han, and Yang extended the theory for a heteroclinic loop with a cusp in ^[5]. Chen, Oksasoglu, and Wang considered a heteroclinic loop under periodic perturbation on the plane ^[6]. They proved three types of dynamic behavior near the heteroclinic loop under periodic perturbation. One of which with strange attractors admitting SRB measures representing chaos. More complicated dynamic behavior, such as strange attractors and horseshoes near the heteroclinic loop with periodic perturbation see, ^[7] and ^[8].

Chow, Deng, and Terman ^[9] investigated the homoclinic or periodic orbit bifurcated from a heteroclinic loop based on the method developed by Shilnikov. In 1998, Zhu and Xia ^[10] established a coordinate system in a neighborhood of a heteroclinic loop. They studied the bifurcation of the heteroclinic loop using the coordinate systems near the heteroclinic loop. Moreover, Rademacher ^[11] studied the homoclinic orbit bifurcated from a codimension 1 and 2 heteroclinic loops by Lin's method ^[12]. In ^[13], Geng, Wang, and Liu investigated the bifurcation of a heterodimensional loop using the local coordinate system. They assumed the unperturbed equation has a heteroclinic loop in $\mathbb{R}^4$ that the splitting indices of the unperturbed heteroclinic orbits are $1$ and $-1$. They obtained the persistence condition for the heterodimensional loop. For more research results regarding the bifurcation of the heteroclinic loop see ^[14].

We let $d, d\ge1,$ denote the number of the bounded solutions of the variational equation along the heteroclinic orbit. If $d = 1$, the homoclinic or heteroclinic orbit is nondegenerate; otherwise, it is degenerate ^[15], which means, along the orbit, the intersection of the spaces tangent to the stable and unstable manifolds of the equilibrium has a $d$ dimensional subspace. Hence, parameter $d$ describes the degeneration of the homoclinic or heteroclinic orbit.

The primary purpose of this paper is to extend the theory of ^[13,14] for heteroclinic loop bifurcation. We consider an autonomous ordinary differential equation that admits a heteroclinic loop in $\mathbb R^n$. The unperturbed heteroclinic loop consists of two degenerate heteroclinic orbits. Furthermore, the splitting index of the unperturbed heteroclinic orbits can be arbitrary. We investigate the bifurcation of the heterodimensional loop under periodic perturbation using the Lyapunov-Schmidt reduction method. We start with the following equation:

and its periodic perturbed equation is as follows:

where $x\in\mathbb{R}^{n}$, $\mu = (\mu_1, \mu_2)\in\mathbb{R}^2$, and we make the following assumptions:

(H1) $f\in C^{3}$.

(H2) $p_+$ and $p_-$ are the two distinct hyperbolic equilibria of Eq (1.1). Namely, $f(p_{\pm}) = 0$ and the eigenvalues of $Df(p_{\pm})$ lie off the imaginary axis, where $D$ denotes the derivative operator.

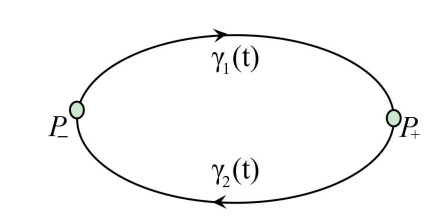
(H3) Equation (1.1) has two heteroclinic solutions $\gamma_1(t)$ and $\gamma_2(t)$, which are asymptotic to the equilibrium $p_+$ and $p_-$, respectively. That is, $\dot{\gamma_i}(t) = f(\gamma_i(t))$, $i = 1, 2$, and

(H4) $g_j\in C^{3}$, $g_j(p_{\pm}, \mu, t) = 0$, $g_j(x, 0, t) = 0$ and $g_j(x, \mu, t+2) = g_j(x, \mu, t)$.

(H5) $dim(W^{s}(p_+)) = d_{+}$ and $dim(W^{s}(p_-)) = d_{-}$, where $W^{s}(p_+)$ and $W^{s}(p_-)$ are the stable manifold of the equilibrium $p_+$ and $p_-$, respectively.

(H6)

and

where $T_{\gamma_i(0)}W^{s/u}(p_{\pm})$ is the tangent spaces of the corresponding invariant manifolds at $\gamma_i(0)$ and $d_i > 1$, $i = 1, 2$.

By $(H3)$ and $(H6)$, we know unperturbed Eq $(1.1)$ has a heteroclinic loop $\Gamma$ (see Figure 1), where

.

By $(H5)$, we know that $d_+$ and $d_-$ can be arbitrary. Thus, the unperturbed Eq $(1.1)$ has a heterodimensional loop. We provide conditions for the persistence of the heterodimensional loop under periodic perturbation. The structure of the paper is as follows. We present some background on the Lyapunov-Schmidt reduction and Lin's method in Section 2. Section 3 details the notations for the fundamental matrix of the variational equation along the heteroclinic orbit $\gamma_i(t)$ and the main result. Section 4 provides proof of the main result. The bifurcation function is obtained using the functional analytic method. We construct some solutions near the unperturbed heteroclinic loop, which can have a gap at $t = 0$, and glue those solutions at $t = 0$. Thus, the bifurcation function can be obtained. Hence, under some conditions, some solutions near the unperturbed heteroclinic loop can constitute a heteroclinic loop for a perturbed system.

2.   Preliminaries

Many problems in bifurcation theory can be changed by solving the zeros of an operator equation in some Banach space. Sometimes, the corresponding operator is not invertible, making it difficult to solve. However, this problem can equivalently transform the operator equation into an equation in a low-dimensional space using the Lyapunov-Schmidt reduction method (see ^[16]). Therefore, this method is very effective, especially in studying homoclinic or heteroclinic bifurcation.

Lin's method ^[17] is an implementation of the Lyapunov-Schmidt reduction method to construct solutions near the unperturbed heteroclinic orbit. The idea of Lin's method originated from the work by Chow, Hale, and Mallet-Paret ^[18] using the function space approach to construct piecewise continuous solutions approximating the unperturbed homoclinic orbit. The bifurcation function can be obtained using these solutions, and the zeros of the bifurcation function correspond to solutions in the homoclinic or heteroclinic bifurcation problems. Later, Palmer ^[19], Hale and Lin ^[20] extended the methods to $\mathbb R^n$ and the functional differential equation. Lin used the function space approach to construct solutions near the heteroclinic chain ^[12]. He assumed that heteroclinic orbits in the chain all have the same index. In the 1990s, Gruendler ^[21,22] generalized the method to the case of degenerate homoclinic bifurcation problems.

Next, we introduce an application of the Lyapunov-Schmidt reduction method, known as the Fredholm alternative property for linear differential equations. We consider the following nonhomogeneous linear differential equation:

where $y\in \mathbb R^n$, $A(t)$ vary continuously with $t\in \mathbb R$ and $h(t)$ is bounded and continuous on $t\in \mathbb R$. We assume that the homogeneous differential equation $\dot{y}(t) = A(t)y(t)$ has exponential dichotomies on $\mathbb R^+$ and $\mathbb R^-$, respectively. Then, $M > 0$, $K_{0} > 0$, and projections $P$ and $Q$ exist, such that

where $U(t)$ is the fundamental matrix. We define the Banach spaces as follows:

with the norm $||z||_r = \max_{0\leq j\leq r} \{\sup_{t\in \mathbb{R}}|D^jz(t)|e^{M|t|}\}$, $|D^0z(t)|$ indicates $|z(t)|$. We let the linear operator $L:\mathcal{Z}^1\to \mathcal{Z}^0$ be defined by

The adjoint operator for $L$ is

where $(A(t))^T$ denotes the transpose of matrix $A(t))$. By the definition of the linear operator $L$ and the exponential dichotomy, we know that

If $dim Ker(L^*) = d$ and $\psi_1(t), ..., \psi_{d}(t)$ are the orthonormal unit bases of $Ker (L^*)$, we define a projection operator $\Pi:\mathcal{Z}^0\rightarrow \mathcal{Z}^0$ as follows

By the method of the Lyapunov-Schmidt reduction, Eq (2.1) is equivalent to the following system

By the definition of $\Pi$, $Ran(I-\Pi) = Ran L$. We can first solve Eq $(2.6)$ for $y\in \mathcal{Z}^1$, and the bifurcation equations are obtained by Eq (2.7). That is,

Thus, Eq (2.1) has a bounded solution $y(t)$ if and only if Eq (2.8) holds.

3.   Notation and main result

The variational equation of (1.1) along the heteroclinic orbit $\gamma_i$ is:

From $(H6)$, we know that Eq (3.1) has $d_i (d_i > 1)$ linearly independent bounded solutions, $i = 1, 2$. Based on Sacker's definition ^[23], we can define the splitting index $S(\gamma_i)$ for the unperturbed heteroclinic orbit $\gamma_i$, as follows:

By $(H3)$ and the exponential dichotomy roughness theorem, we know that the variational Eq (3.1) has two-side exponential dichotomies. We let $U_i$ be the fundamental matrix of Eq (3.1). Then, $M > 0$, $K_{0} > 0$, projections $P_i$ and $Q_i$ exist, such that

where $I$ is the $n\times n$ unit matrix. We let the linear operator $L_i:\mathcal{Z}^1\to \mathcal{Z}^0$ be defined by

Further, the adjoint operator for $L_i$ is

We let $U_i^{-1}$ denote the inverse of $U_i$. Then we have $U_i^{-1}U_i = I$. Differentiating $U_i^{-1}(t)U_i(t) = I$ with respect to $t$, we obtain

hence,

Therefore, we have

We know that $(U_i^{-1})^T$ is a matrix solution of the adjoint equation of (3.1). Taking the transpose in Eq (2.2), it is apparent that the adjoint equation of (3.1) also has exponential dichotomy on $\mathbb{R}^+$ with projection $I-P_i^{T}$, and on $\mathbb{R}^-$ with projection $I- Q_i^{T}$, respectively.

By the definition of the linear operator $L_i$ and the exponential dichotomy, we know that

From the theory of homoclinic bifurcation, the linear operators $L_1$ and $L_2$ are Fredholm operators, and the index of the Fredholm operator $L_i$ is

If $dim Ker(L_i^*) = d_i^*, i = 1, 2$, then we have

In addition, if $u^i_1(t), ..., u^i_{d_i-1}(t), \dot{\gamma_i}(t)$ are the orthonormal unit bases of $Ker (L_i)$, $\varphi_1(t), ..., \varphi_{d_1-s}(t)$ are the orthonormal unit bases of $Ker (L^*_1)$ and $\psi_1(t), ..., \psi_{d_2+s}(t)$ are the orthonormal unit bases of $Ker (L^*_2)$, then define

where $i = 1, ..., d_1-s$, $p, q = 1, ..., d_1-1$, and $k = 1, 2$. Moreover,

where $j = 1, ..., d_2+s$, $m, n = 1, ..., d_2-1$, and $k = 1, 2$. Using those notations, we let $M^1:{\mathbb R}^{d_1-1}\times{\mathbb R}^{2}\times{\mathbb R}\to{\mathbb R}^{d_1-s}$ be given by

and

where $i = 1, ..., d_1-s, \beta^1 = (\beta^1_1, ..., \beta^1_{d_1-1})$.

We let $M^2:{\mathbb R}^{d_2-1}\times{\mathbb R}^{2}\times{\mathbb R}\to{\mathbb R}^{d_2+s}$ be given by

and

where $j = 1, ..., d_2+s$ and $\beta^2 = (\beta^2_1, ..., \beta^2_{d_2-1})$. Further, we let $M:{\mathbb R}^{d_1+d_2-2}\times{\mathbb R}^{2}\times{\mathbb R}^{2}\to{\mathbb R}^{d_1-s}\times{\mathbb R}^{d_2+s}$ be given by

where $\beta = (\beta^1, \beta^2), \alpha = (\alpha_1, \alpha_2)$.

We can state the main result as follows:

Theorem 1. Assume that $(H1)-(H5)$ hold. Let $M(\beta, \mu, \alpha)$ be as in Eq (3.6). If there are some points $(\beta_{0}, \mu_{0}, \alpha_{0})\in {\mathbb R}^{d_1+d_2-2}\times{\mathbb R}^{2}\times{\mathbb R}^{2}$, such that

and

is a nonsingular $(d_1+d_2)\times (d_1+d_2)$ matrix, then there exists an open interval $I$ containing origin, the $C^1$ function $\kappa_2: I\rightarrow \mathbb{R}^{2}$, and the heteroclinic solutions $x_1(\varepsilon, t)$, $x_2(\varepsilon, t)$ of the Eq (1.2) with $\mu = \varepsilon^2(\mu_0+\kappa_2(\varepsilon))$, where $\varepsilon\in I\setminus\{0\}$, $x_1(\varepsilon, t)$ and $x_2(\varepsilon, t)$ are located near the heteroclinic orbits $\gamma_1$ and $\gamma_2$, such that $x_1(\varepsilon, t)$, $x_2(\varepsilon, t)$, $p_+$ and $p_-$ can constitute a heteroclinic loop $\Gamma_{\varepsilon}$.

The proof of Theorem 1 is performed in Section 4. The heteroclinic loop $\Gamma_{\varepsilon}$ as illustrated in Figure 2.

4.   Proof of Theorem 1

By $(H2)$, we know the unperturbed Eq $(1.1)$ has a heteroclinic loop $\Gamma$. In this section, we find conditions such that the perturbed Eq $(1.2)$ have a heteroclinic loop $\Gamma_{\mu}$ with sufficiently small $\mu$. For $i = 1$ or $i = 2$, we suppose $x_i(t)$ is a solution of Eq (1.2). With the change of variable

Equation (1.2) can be transformed into

where

By direct calculation, we have

where $D_i$ and $D_{ij}$ denote the derivative of the multivariate function concerning its $i$-th and $i$ and $j$-th variables, respectively.

Because we only consider the Eq $(1.1)$ under a small periodic perturbed equation, we suppose $\mu\in \overline{B}_1(0, \delta)\subseteq\mathbb{R}^2$, where $\overline{B}_1(0, \delta)$ is a closed set with radius $\delta > 0$ centered at the origin. Moreover, we have the following property regarding the function $\widetilde{g}$.

Lemma 1. The function $\widetilde{g}(\cdot, \mu, \alpha_i): \mathcal{Z}^1\times\overline{B}_1(0, \delta)\times\mathbb{R}\mapsto\mathcal{Z}^0$.

Proof. For $i = 1$ or $i = 2$, we let $z_i\in\mathcal{Z}^1$ be given. We can choose a closed set $B$ such that $z_i(t), \gamma_i(t), z_i(t)+\gamma_i(t)$ and $p_{\pm}+z_i(t)+\gamma_i(t)$ are all $\in B$ for $t\in\mathbb{R}$. According to smoothness of $f, g_j\in C^3$ and $g_j$ is periodic about $t$. We can choose a constant $M_1$ such that

for $(x, \mu, \alpha_i)\in B\times\overline{B}_1(0, \delta)\times\mathbb{R}$. If $z_i\in\mathcal{Z}^1$, because $\gamma_i$ is a heteroclinic solution which is heteroclinic to the hyperbolic equilibrium $p_{\pm}$, we can assign a constant $M_2$ such that

We define $\sigma_1(s) = f(sz_i(t)+\gamma_i(t))-f(\gamma_i(t)):[0, 1]\mapsto\mathbb{R}^n$. By the smoothness of $f$, $\sigma_1\in C^{3}$ and for some $s^*\in(0, 1)$,

Therefore,

We define a map $\sigma_2(s):[0, 1]\mapsto\mathbb{R}^n$ by

By $(H4)$, $\sigma_2\in C^{3}$, $\sigma_2(1) = g_j(\gamma_i(t)+z_i(t), \mu, t+\alpha_i)$ and $\sigma_2(0) = g_j(p_{\pm}, \mu, t+\alpha_i) = 0$. For some $s^*\in(0, 1)$, we have

Therefore,

For any $\mu\in\mathbb{R}$, $g_j(p_{\pm}, \mu, t+\alpha_i) = 0$, thus

As a result,

that is

Thus, for any given $z_i\in\mathcal{Z}^1$, $\widetilde{g}(z_i, \mu, \alpha_i)\in\mathcal{Z}^0$. The proof is complete.

From the variable transformation of Eq $(4.1)$, if $\lim_{t\rightarrow \pm\infty}|z_i(t)| = 0$, then $x_i(t)$ is a heteroclinic solution which is heteroclinic to the hyperbolic equilibrium $p_{-}$ and $p_{+}$. Hence, the persistence of the heteroclinic loop $\Gamma$ under the periodic perturbation of Eq $(1.1)$ is equivalent to the search solution $z_i(t)$ of Eq $(4.3)$ in the Banach space $\mathcal{Z}^1$. Next, we use the method of the Lyapunov-Schmidt reduction to solve the operator equations

in the Banach space $\mathcal{Z}^1$.

We define spaces $\widetilde{\mathcal{Z}}_1$ and $\widetilde{\mathcal{Z}}_2$ which are closed linear subspaces of $\mathcal{Z}^0$, as follows

where $\varphi_1(t), ..., \varphi_{d_1-s}(t)$ are the orthonormal unit bases of $Ker (L^*_1)$ and $\psi_1(t), ..., \psi_{d_2+s}(t)$ are the orthonormal unit bases of $Ker (L^*_2)$. We define maps $\Pi_1$ and $\Pi_2:\mathcal{Z}^0\rightarrow \mathcal{Z}^0$ as follows

where $\varphi_{j}^T$ and $\psi_{j}^T$, satisfying $\langle\varphi_{i}, \varphi_{j}^T\rangle = \delta_{ij}$ and $\langle\psi_{i}, \psi_{j}^T\rangle = \delta_{ij}$, respectively. When $i = j$, $\delta_{ij} = 1$, and when $i\neq j$, $\delta_{ij} = 0$. By the definition of map $\Pi_1$, we have

For map $\Pi_2$, we can similarly obtain $(\Pi_2(z))^2(t) = \Pi_2(z)(t)$. Hence, $\Pi_1$ and $\Pi_2$ are projections. For any $z_i\in\mathcal{Z}^1$, we have

Next, we apply the Lyapunov-Schmidt reduction to solve Eq (4.2). Applying $\Pi_i$ and $(I-\Pi_i)$ on Eq (4.2), we find that Eq (4.2) is equivalent to the following system

We first solve Eq $(4.7)$ for $z_i\in \mathcal{Z}^1$. Then, the bifurcation equations are obtained by substituting the solution $z_i$ into Eq (4.8).

We can define a bounded linear map $K_i: Ran(L_i) \mapsto \mathcal{Z}^1\setminus Ker(L_i)$. Thus $K_i(h_i)$ is a solution of the linear operator equation $L_i(u) = \dot{u}(t)-Df(\gamma_i) = h_i$, when $h_i\in Ran(L_i)$. By $(H6)$, we suppose $u^i_1(t), ..., u^i_{d_i-1}(t)$ are the orthonormal unit bases of $Ker (L_i)$. Moreover, we solve Eq (4.7) for $z_i\in \mathcal{Z}^1$.

Lemma 2. Equation $(4.7)$ has a unique solution $z_i\in\mathcal{Z}^1$ such that $z_i$ satisfies

where $(\beta^i, \mu, \alpha_i)\in\mathbb{R}^{d_i-1}\times\mathbb{R}^2\times\mathbb{R}$.

Proof. We define a $C^{2}$ map: $F_i:\mathcal{Z}^1\times\mathbb{R}^{d_i-1}\times\mathbb{R}^2\times\mathbb{R}\rightarrow \mathcal{Z}^1$ as follows:

where $\beta^i = (\beta^i _{1}, ..., \beta^i _{d-1})\in{\mathbb R}^{d_i-1}$. By Eq $(4.4)$, we obtain $\widetilde{\mathcal{Z}}_i = Ran(L_i) = Ran(I-\Pi_i)$, $i = 1, 2$. By the definition of the projection operator $\Pi_i$ and Lemma 3.1, we have $(I-\Pi_i)\widetilde{g}(z_i, \mu, \alpha_i)\in Ran(L_i)$. Thus $K_i\{(I-\Pi_i)\widetilde{g}(z_i, \mu, \alpha_i)\}$ is a solution of the Eq $(4.7)$. And $u^i_j(t)\in Ker(L_i)$, then the fixed points of $F_i$ are the solutions of Eq $(4.7)$. Thus, we must demonstrate that the map $F_i$ has a unique fixed point in the space $\mathcal{Z}^1$.

We let $\overline{B}(0, \delta_1)$, $\overline{B}^i(0, \delta_2)$, and $\overline{B}_1(0, \delta_2)$ be a closed subset with radius $\delta_1 > 0$ and $\delta_2 > 0$ centered at the origins of $\mathcal{Z}^1$, $\mathbb{R}^{d_i-1}$, and $\mathbb{R}^{2}$. By $\widetilde{g}(0, 0, \alpha_i) = 0$ and the smoothness of $f, g_j$, we can set $\delta_1$ and $\delta_2$ to be sufficiently small such that

for $(z_i, \mu, \alpha_i)\in \overline{B}(0, \delta_1)\times\overline{B}_1(0, \delta_2)\times\mathbb{R}$.

Further, $u^i_j\in Ker (L_i)$, $K_i$ and $(I-\Pi_i)$ are bounded linear operators. We can set constants $M_3 > 0, M_4 > 0$ such that

for any $i = 1, 2, j = 1..., d_{i}-1$. We let $\delta_2 = \min\{\frac{\delta_1}{2M_3(d_{i}-1)}, \frac{\delta_1}{2M_4}\}$. For any $(z_i, \beta^i, \mu, \alpha_i)\in \times\overline{B}(0, \delta_1)\times\overline{B}^i(0, \delta_2)\times\overline{B}_1(0, \delta_2)\times\mathbb{R}$, we have

Thus, for any $(\beta^i, \mu, \alpha_i)\in\overline{B}^i(0, \delta_2)\times\overline{B}_1(0, \delta_2)\times\mathbb{R}$, we have

We let

Then $h(0) = 0, Dh(0) = 0$, so we can choose above $\delta_1$ to be sufficiently small such that $||Dh(z_i)||\leq \delta_2$, for $z_i\in \overline{B}(0, \delta_1)$. We select a constant $M_5 > 0$ such that $||D_1g_j(\gamma_i(t)+z_i(t), \mu, t+\alpha_i)||\leq M_5$, for $(z_i, \mu, \alpha_i)\in \times\overline{B}(0, \delta_1)\times\overline{B}_1(0, \delta_2)\times\mathbb{R}$.

By Eq $(4.3)$, we have

For $z_i^1, z_i^2\in \overline{B}(0, \delta_1)$, $(\beta^i, \mu, \alpha_i)\in \overline{B}^i(0, \delta_2)\times\overline{B}_1(0, \delta_2)\times\mathbb{R}$. From Eq $(4.3)$, we obtain the following:

for $s\in(0, 1)$. Thus,

Therefore, if we set $\delta_2 = \min\{\frac{\delta_1}{2M_3(d_{i}-1)}, \frac{\delta_1}{2M_4}, \frac{1}{2(M_4+2M_5)}\}$, then

As a result, $F_i$ is a uniform contraction in $\overline{B} (0, \delta_1)$. By the contraction mapping principle, a unique $C^1$ map $\omega_i :\overline{B}^i(0, \delta)\times\overline{B}_1(0, \delta)\times\mathbb{R}\mapsto \mathcal{Z}^1$ exists such that

Moreover, $F_i(0, 0, 0, \alpha_i) = 0$, hence, $\omega_i(0, 0, \alpha_i) = 0$, which implies the desired statement.

Substituting $\omega_i(\beta^i, \mu, \alpha_i)$ into Eq (4.8), we obtain the bifurcation function

By the definition of projection $\Pi_i$, we have

By the linear independence of $\varphi_1, ..., \varphi_{d_1-s}$ and $\psi_1, ..., \psi_{d_2+s}(t)$, Eqs (4.11) and (4.12) are equivalent to

where $i = 1, ...d_1-s, j = 1, ..., d_2+s$. We let

Therefore, by the Lyapunov-Schmidt reduction, we obtained the bifurcation function:

where $\beta = (\beta^1, \beta^2), \alpha = (\alpha_1, \alpha_2)$. If there are some parameter values $(\beta, \mu, \alpha)\in \mathbb{R}^{d_1+d_2-2}\times \mathbb{R}^2\times\mathbb{R}^2$, such that

then $z_i = \omega_i$ is a solution of Eq (4.2). Hence, the perturbed Eq (1.2) has heteroclinic solutions

and

which are asymptotic to the equilibrium $p_+$ and $p_-$, that is

and

are uniform for some $(\beta^1, \beta^2, \mu, \alpha_1, \alpha_2)$. Thus, the heteroclinic orbits $x_1(\beta^1, \mu, \alpha_1)(t)$ and $x_2(\beta^2, \mu, \alpha_2)(t)$ and the equilibria $p_+$, $p_-$ constitute a heteroclinic loop of the perturbed Eq (1.2).

Through direct calculations, the function $H(\beta, \mu, \alpha)$ has the following properties:

We define $M^1:{\mathbb R}^{d_1-1}\times{\mathbb R}^{2}\times{\mathbb R}\to{\mathbb R}^{d_1-s}$ given by

and

We define $M^2:{\mathbb R}^{d_2-1}\times{\mathbb R}^{2}\times{\mathbb R}\to{\mathbb R}^{d_2+s}$ given by

and

Thus,

Moreover, we define $M:{\mathbb R}^{d_1+d_2-2}\times{\mathbb R}^{2}\times{\mathbb R}^{2}\to{\mathbb R}^{d_1-s}\times{\mathbb R}^{d_2+s}$ given by

hence

Lemma 3. If points $(\beta_0, \mu_0, \alpha_0)\in\mathbb{R}^{d_1+d_2-2}\times\mathbb{R}^{2}\times\mathbb{R}^2$ exists such that $M(\beta_0, \mu_0, \alpha_0) = 0$, and $D_{(\beta, \mu)}M(\beta_0, \mu_0, \alpha_0)$ is a nonsingular $(d_1+d_2)\times(d_1+d_2)$ matrix, then an open interval $I\subset\mathbb{R}$ exists containing zero and differentiable functions, $\kappa_1: I\rightarrow \mathbb{R}^{d_1+d_2-2}$ and $\kappa_2: I\rightarrow \mathbb{R}^{2}$, such that $\kappa_1(0) = 0$, $\kappa_2 (0) = 0$, and $H(\varepsilon(\beta_0+\kappa_1(\varepsilon)), \varepsilon^2(\mu_{0}+\kappa_2(\varepsilon)), \alpha_0) = 0$ for $\varepsilon\in I$.

Proof. We define a $C^2$ function $N: \mathbb{R}^{d_1+d_2-2}\times\mathbb{R}^{2}\times\mathbb{R}^{2}\mapsto \mathbb{R}^{d_1+d_2}$:

It is clear that $H = 0$ if and only if $N = 0$ for $\varepsilon\neq 0$. Through direct calculations, we have $N(0, 0, 0) = 0$, and $D_{(x, y)}N(0, 0, 0) = D_{(\beta, \mu)}M(\beta_0, \mu_0, \alpha_0)$ is nonsingular matrix. Using the implicit function theorem, we know an open interval $I\subset{\mathbb {R}}$ exists containing the zero and differentiable functions, which are $\kappa_1: I\rightarrow \mathbb{R}^{d_1+d_2-2}$ and $\kappa_2: I\rightarrow \mathbb{R}^{2}$, satisfying $\kappa_1(0) = 0$ and $\kappa_2(0) = 0$, respectively, such that $N(\kappa_1(\varepsilon), \kappa_2(\varepsilon), \varepsilon) = 0$ for $\varepsilon\in I$. Hence, we obtain

The proof is complete.

Hence, the perturbed Eq (1.2) has heteroclinic orbits

and

where $\varepsilon\in I\setminus\{0\}, \beta_0 = (\beta^1_0, \beta^2_0), \kappa_1(\varepsilon) = (\kappa^1_1(\varepsilon), \kappa^2_1(\varepsilon)), \alpha_0 = (\alpha_{1, 0}, \alpha_{2, 0})$. In addition,

for some $\varepsilon\in I\setminus\{0\}$. If we let

then some solutions near the unperturbed heteroclinic loop $\Gamma$ exist which can constitute a heteroclinic loop $\Gamma_\varepsilon$ for perturbed Eq (1.2).

5.   Discussion and conclusions

In this paper, we investigated the persistence of a heteroclinic loop under periodic perturbation in $\mathbb{R}^n$. We assumed unperturbed heteroclinic loop is a heterodimensional loop and the unperturbed heteroclinic orbits are degenerate. Using the method of Lyapunov-Schmidt reduction and exponential dichotomies, we obtained the bifurcation function, which is defined by

where $\beta = (\beta^1, \beta^2), \alpha = (\alpha_1, \alpha_2)$ and $(\beta^1, \beta^2, \mu, \alpha_1, \alpha_2)\in \mathbb{R}^{d_1-1}\times\mathbb{R}^{d_2-1}\times \mathbb{R}^2\times\mathbb{R}\times\mathbb{R}$. Under the condition of Theorem 1, there exist some points such that $H(\beta, \mu, \alpha) = 0$. Hence, there exist heteroclinic solutions $x_1(\varepsilon, t)$, $x_2(\varepsilon, t)$ of the Eq (1.2) with $\mu = \varepsilon^2(\mu_0+\kappa_2(\varepsilon))$, where $\varepsilon\in I\setminus\{0\}$, $x_1(\varepsilon, t)$ and $x_2(\varepsilon, t)$ are located near the heteroclinic orbits $\gamma_1$ and $\gamma_2$, such that $x_1(\varepsilon, t)$, $x_2(\varepsilon, t)$, $p_+$ and $p_-$ can constitute a heteroclinic loop $\Gamma_{\varepsilon}$. The heteroclinic tangles is one of the primary mechanisms for non-uniformly hyperbolic dynamics. Our results extended the theory of heteroclinic loop bifurcation.

There are still many interesting and instructive issues worthy of further study. For example, the hyperbolicity of the heteroclinic solution $x_i(\varepsilon, t)$ and chaos motion near the heteroclinic loop $\Gamma_\varepsilon$.

Acknowledgments

We are grateful to the two anonymous referees for useful comments and suggestions. This work was supported by National Natural Science Foundation of China (Grant No. 11801343).

Conflict of interest

The authors declare there is no conflicts of interest.