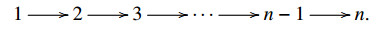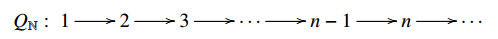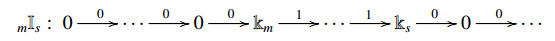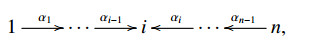1.
Introduction
W. Rump introduced Zavadskij modules in 2000 [1]. They arose from a generalization of the two-point differentiation due to A. G. Zavdaskij [2]. Such an algorithm has been used to classify tiled orders of finite representation type, abelian groups of finite rank and different types of posets [3,4,5]. The Zavadskij property was introduced for semimaximal rings to establish a Zavadskij-type reduction for orders in a finite-dimensional algebra over a complete field with respect to a discrete valuation. Thus, the known versions of Zavadskij's differentiation algorithm (for tiled orders [6], representations of posets, and vector space categories [5]) were unified and extended in this way to a part of the representation theory of general orders. In the context of quiver representations, the Zavadskij property has not been characterized; thus, we discuss this property in the general case of finite-dimensional algebras, and particularly in the special case of the cluster-tilted algebras of type A. These algebras are closely to cluster algebras and its categorification through cluster categories [7] (see also [8] for the case A). A crucial role is played by the cluster tilting objects in the cluster category, which models the clusters in the cluster algebra. The endomorphism algebras of these cluster tilting objects are called cluster-tilted algebras. Such algebras have been studied, particularly if the quiver underlying the cluster algebra, and hence the cluster category, is of Dynkin type. Actually, cluster-tilted algebras of Dynkin type can be described as quivers with relations where the possible quivers are precisely the quivers in the mutation class of the Dynkin quiver, and the quiver uniquely determines the relations in an explicit way [9]. Moreover, the quivers in the mutation classes of Dynkin quivers are explicitly known; for instance, in the case A they can be found in [10]. Moreover, the cluster-tilted algebras of type An are naturally associated with triangulations of an n+3-gon [8]. Furthermore, in [11], it was shown that there is a bijection between isomorphism classes of cluster-tilted algebras of type An and triangulations of the (n+3)-gon up to rotation. The combinatorics associated with this kind of algebras is well-understood.
Contributions
Indecomposable Zavadskij modules are uniserial modules, but the converse is not true. However, we use the mast associated with the uniserial modules to characterize in a combinatorial way the Zavadskij modules. We show that the indecomposable Zavadskij modules over a finite-dimensional algebra A are precisely the indecomposable uniserial modules whose mast is a path with multiplicity one in each vertex (Theorem 7). Furthermore, in the case of path algebras associated a tree quivers, we give a bijection between paths in the quiver and the indecomposable Zavadskij modules (Corollary 8). Thus, we establish that a direct sum of indecomposable Zavadskij modules is a Zavadskij module if and only if the support of each pairwise of direct summands is disjoint (Theorem 9). On the other hand, we use the geometric model given in [8] to establish a formula for the number of indecomposable Zavadskij modules over any cluster-tilted algebra of type A (Theorem 14). This formula is also described for the particular cases of Dynkin algebras of type A (Corollaries 16 and 17). Following [12], this formula allows us to give a categorification of the integer sequence A000217 (Theorem 18) via the indecomposable modules in the category injlf(QN) generated by the locally finite-dimensional representations of finite-length of the form
where kj=k for all m≤j≤s and 1≤m≤s<∞, over the infinite-dimensional path algebra associated with the quiver
In this setting, the representations mIs are indecomposable Zavadskij modules, and direct sums of them are Zavadskij modules if and only if each pair of different direct summands have disjunct support. Moreover, we discuss the relationship between indecomposable Zavadskij modules in certain Dynkin algebras of type A and the integer sequences A005563, A002370, and A152948 in the OEIS [13] (Proposition 19).
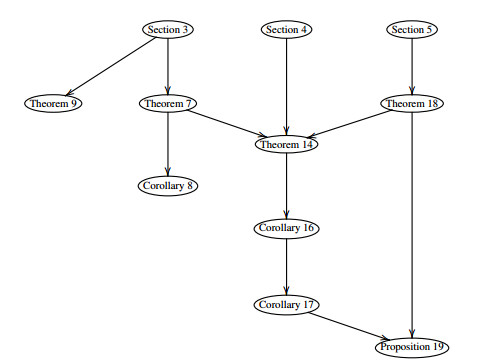
Figure 1 shows a map of the main results presented, and the connections between the different topics dealt with in this paper.
The paper is organized as follows. Section 2 deals with the modules over finite-dimensional k-algebras in the setting of the representations of quivers. As a particular case, it introduces the cluster-tilted algebras of type A. Moreover, following [1], Rump's characterization of the Zavadskij modules is described. In Section 3, we describe the results (Theorems 7 and 9, Corollary 8) about Zavadskij modules over finite-dimensional algebras. Finally, Section 4 is dedicated to giving a formula for the number of Zavadskij modules over cluster-tilted algebras of type A (Theorem 14), and Section 5 describes some integer sequences arising from Zavadskij modules.
2.
Preliminaries
Through this work, k denotes an algebraically closed field. In this section, we recall some aspects important about modules over finite-dimensional k-algebras in the language of quiver representations, cluster-tilted algebras of type A, and the characterization due Rump of Zavadskij modules.
2.1. Modules over finite-dimensional k-algebras
The use of quivers and their representations gives us the possibility to visualize very concretely the finitely generated modules over a finite-dimensional k-algebra A. We write Q0 for the set of vertices of a quiver Q, and Q1 for its set of arrows, while tα and hα denote the tail and head of an arrow tαα→hα in Q1. A vertex x∈Q0 is said to be a sink vertex (resp. source vertex) if there is no arrow α in Q1 such that tα=x (resp. hα=x). A representation V of Q assigns a finite-dimensional vector space V(x) to each x∈Q0, and to each α∈Q1 a choice to linear map V(α):V(tα)→V(hα). A subrepresentation W⊆V is a collection of subspaces (W(x)⊆V(x))x∈Q0 such that V(α)(W(tα))⊆W(hα) for all α∈Q1. The support of a representation V, written SuppV, is the set of vertices x∈Q0 such that V(x)≠0. Definitions of standard notions such as morphisms, direct sum, and indescomposability can be found in [14]. We denote by repQ the category of representations of Q over k.
There is a k-algebra defined for a quiver Q in the following way: A path of length l in Q is a sequence of arrows (α1,…,αl) of Q, of length l, such that tαi+1=hαi. A path of length 0, from a vertex x to x is denoted by ex and it is called stationary path. A path (α1,…,αl) of length l≥1 such that tα1=hαl is called an oriented cycle. The path algebra kQ of Q is defined to be the k-algebra whose underlying k-vector space has as a basis, the set of all paths (x|α1,…,αl|y) of length ≥0. The product of two basis elements is given by the concatenation of paths. The path algebras kQ are hereditary k-algebras, that is, k-algebras such that the submodules of projective modules are projective. Now, the importance of quiver representations is that if Q has no oriented cycles, is equivalent to the category modkQ of finite generated kQ-modules; moreover, each finite-dimensional hereditary k-algebra can be viewed as a path algebra ([14,Lemma 1.4 and Theorem 1.6]).
In a more general setting, a bound quiver algebra is the quotient kQ/I of a path algebra kQ by an admissible ideal I, that is, a two-sided ideal I of kQ that satisfies RmQ⊂I⊂R2Q for some integer m≥2, where RQ is two-sided ideal generated by all arrows in Q and for all l≥1, RlQ denotes the ideal of kQ generated, as a k-vector space, by the set of all paths of length ≥l. Also, the pair (Q,I) is called a bound quiver. Let Q be a finite quiver and V be a representation of Q. For any nontrivial path w=α1α2…αl from x to y in Q, the evaluation of V on the path w is the k-linear map from V(x) to V(y) defined by V(w)=V(αl)∘V(αl−1)∘⋯∘V(α1). The definition of evaluation extends to k-linear combinations of paths with a common tail and a common head which are called relations. A representation V of Q is said to be bound by I, if we have that V(ρ)=0, for all relations ρ∈I. We denote by rep(Q,I) the full subcategory of repQ, consisting of all representations of Q bounded by I. Now, the importance of bound quivers is that if A is a finite-dimensional k-algebra then the category mod A of finitely generated A-modules is equivalent to the category of representations rep(QA,IA) of an associated bound quiver (QA,IA), where A and kQA/IA are isomorphic as k-algebras ([14,Theorem 1.6]). In the sequel, finite-dimensional algebras A are identified with bound quiver algebras kQ/I and the finitely generated A-modules are identified with the representations of Q bound by I.
2.2. Cluster-tilted algebras of type A
cluster-tilted algebras of type A are a particular case of bound quiver algebras. Although these were defined as the endomorphism algebras of the well-known cluster tilting objects (see [15,16]) we recall a definition observed in [17], a cluster-tilted algebra of type A is the bound quiver algebra given by a finite connected full subquiver of the following infinite quiver (an infinite array of oriented 3-cycles attached at their vertices) modulo the relation that the composition of any two arrows in any oriented 3-cycle is zero.
Observe that a full subquiver Q containing two arrows in an oriented 3-cycle will contain the entire 3-cycle. Moreover, all 3-cycles in Q are oriented 3-cycles since the infinite quiver contains no unoriented 3-cycles.
Example 1. A cluster-tilted algebra of type A is the bound quiver algebra (Q,I), where Q is the quiver
and I is the admissible ideal defined by two length paths in the 3-cycle βγδ, that is, I={βγ,γδ,δβ}.
2.3. Zavadskij modules and the Rump's characterization
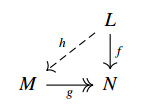
Let M be a module over a ring A. Following [1], we recall that a module L over A is said to be M-projective if for any epimorphism g:M↠N of A-modules the corresponding induced homomorphism
is surjective. In other words, if f:L⟶N is any morphism, then there exists a morphism h:L⇢M such that the diagram
commutes, that is, f=g∘h=g∗(h).
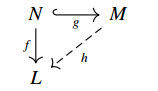
Similarly, a module L over A is said to be M-injective if for any monomorphism g:N↪M the induced homomorphism
is surjective. In other words, if f:N⟶L is any morphism, then there exists a morphism h:M⟶L such that the diagram
commutes, that is, f=h∘g=g∗(h). Moreover, if M itself is M-injective then M is said to be quasi-injective. For example, a projective (resp. injective) A-module is M-projective (resp. M-injective) for each A-module M, whereas any injective module is quasi-injective.
Definition 2. An A-module M is a Zavadskij module if all of its submodules are M-projectives and each factor module is M-injective.
In other words, this says that for each homomorphism ¯f:U⟶W from a submodule U to a factor module W of M, the diagram
can be completed by an endomorphism f of M. Thus, for M to be a Zavadskij module, it suffices to see that each submodule is M-projective and that M itself is quasi-injective.
Example 3. Let Q be the quiver 1⟶2 then the path algebra A=kQ is the matrix ring (k0kk) and the indecomposable projective A-modules are Zavadskij modules, whereas the module AA is not a Zavadskij module because it is not a quasi-injective module. Thus, a direct sum of Zavadskij modules is not a Zavadskij module.
We recall that a nonzero module M over a finite-dimensional k-algebra A is uniserial if the lattice of its submodules forms a chain, i.e., if every two submodules of M are comparable. Clearly, all subfactors of a uniserial module are again uniserial. The lattice of proper nonzero submodules of a length-finite A-module M is then a finite chain with maximal element radM and minimal element socM, where these are the notations for Jacobson radical and socle of M, respectively. Note that, if J=radA then the submodules of M are given by JlM, with l=0,…,length(M) (see [14]).
If M is a uniserial A-module with submodules 0=M0⊂M1⊂M2⊂⋯⊂Mn=M and composition factors Ui=Mi+1/Mi then there are ring homomorphisms
which are all injective if the Ui are pairwise nonisomorphic. In this case, the maps (2.2) are monomorphisms between skew fields. In this setting, we recall that a uniserial A-module M is tame if the Ui are mutually nonisomorphic and the maps (2.2) are isomorphisms.
Concerning uniserial modules, a characterization of Zavadskij modules was achieved by W. Rump. In the indecomposable case, we have the following Theorem 4.
Theorem 4. [1,Proposition 3] Let M be a module over a finite-dimensional k-algebra. M is an indecomposable Zavadskij module if and only if M is a tame uniserial module.
In the general case, we have the following Theorem 5.
Theorem 5. [1,Proposition 4 and Theorem 1] An A-module M is a Zavadskij module if and only if it satisfies one of the following conditions.
(a) The reduced part M1⊕⋯⊕Mr of M=Mn11⊕⋯⊕Mnrr is a Zavadskij module, where all of Mi are indecomposable pairwise non isomorphic.
(b) M can be decomposed into tame uniserial modules and any two indecomposable direct summands of M with a common composition factor are isomorphic.
3.
A combinatorial characterization of Zavadskij modules over finite-dimensional algebras
In this section, we prove Theorem 7, Theorem 9 and Corollary 8 to characterize finitely generated Zavadskij modules over finite-dimensional algebras using the mast associated with each indecomposable uniserial module. In the sequel of this section, A denotes a finite-dimensional k-algebra; thus, A can be identified with a bound quiver algebra A=kQ/I, where Q is a connected quiver, and I is an admissible ideal of the path algebra kQ.
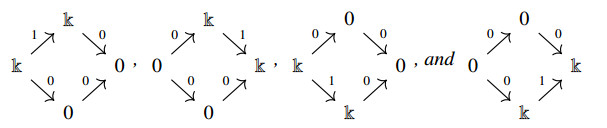
Following [19,18], we recall that a path p in the quiver Q is said to be a mast of the uniserial A-module M if length(p)=length(M)−1 and pM≠0. We let μ(x) denotes the multiplicity of a vertex x in a mast p which is the number of times that the vertex x occurs in p.
Example 6. Let A=kQ/I be a bound quiver algebra, where Q is the quiver
and I=⟨βα−δγ⟩. Then, the paths of length one α,β,γ, and δ are masts of the uniserial modules
respectively. Clearly, the simple modules S1=⟨e1⟩, S2=⟨e2⟩, S3=⟨e3⟩, and S4=⟨e4⟩ are uniserial whose masts are the stationary paths e1, e2, e3, and e4, respectively. However, neither βα nor δγ is a mast of a uniserial A-module.
According to [19,18], we have the following elementary observations:
1) Not every path in kQ with a nonzero image in A needs to occur as a mast of a uniserial module.
2) If the quiver Q has no double arrows, each uniserial A-module has a unique mast. Conversely, the uniqueness of masts in all uniserial modules implies an absence of double arrows.
3) For each uniserial A-module M with sequence (S1,…,Sl+1) of consecutive composition factors, there exists at least one path p of length l in kQ such that pM≠0 (see [18]); necessarily p passes in order through the sequence (1,…,l+1) of those vertices in Q0 which represent the simple modules Si. Moreover, 0≠socM=JlM and Jl is generated by the images of the paths of length l.
The following result gives a combinatorial characterization of indecomposable Zavadskij modules over any finite-dimensional k-algebra.
Theorem 7. An indecomposable A-module M is a Zavadskij module if and only if it is a uniserial module, and for any mast p of M it holds that μ(x)=1 for every vertex x∈Q0 that p passes through. In particular, if the algebra A is hereditary, M is a Zavadskij module if and only if it is uniserial.
Proof. First, we consider the case where A is a hereditary algebra. The necessary condition follows immediately from Theorem 4. On the other hand, we consider a uniserial representation M of the quiver Q. We suppose that the mast p has the form
thus, M is defined by the formulas
Since M is indecomposable and it has the above form, the ring of endomorphisms EndM is isomorphic to k. Moreover, the composition factors have the form Mi+1/Mi, where Mj is the subrepresentation of M such that Mj(x)=M(x) if x∈{j,…,n} and zero otherwise. Thus, Mi+1/Mi is the simple representation Si at vertex i, thus End(Mi+1/Mi) is isomorphic to k. So, the maps (2.2) are isomorphisms. Moreover, the composition factors are mutually nonisomorphic because they are simple representations in different vertices. In effect, we suppose that dimk(HomA(Pi,M))>1 for some i, where Pi denotes the indecomposable projective representation at vertex i. Since M is finitely generated then there exists a projective resolution of M of the form 0⟶P′⟶P⟶M⟶0, with P=⊕i∈Q0diPi and P′=⊕α∈Q1dtαPhα, where di denotes the dimension of M(i) and diPi stands for the direct sum of di copies of Pi. It is clear that the indecomposable projective module Pi appears once, thus dimk(HomA(Pi,M))=1. Therefore, the simple module Si at vertex i appears only once as factor composition of M. These arguments prove that M is a tame module via Theorem 4.
In the general case, let M be an indecomposable Zavadskij A-module over a bound quiver algebra A=kQ/I. Theorem 4 implies that M is a tame uniserial module of length l+1≥1, therefore, indecomposable simple modules Si, 1≤i≤l+1 in the sequence of composition factors (S1,…,Sl+1) are pairwise nonisomorphic. Additionally, if p is a mast of M then we note that p is a path in kQ of length l such that p∉I and pM≠0; necessarily p passes in order through the sequence (1,…,l+1) of those vertices in Q0 which represent the simple modules Si. Thus, μ(x)=1 for any x∈p. On the other hand, let us suppose that M is uniserial and that p is a mast of M with μ(x)=1 for any x∈p0. Therefore, factors composition of M are precisely the indecomposable simple modules Si corresponding bijectively to the set p0={1,…,l,l+1} of vertices in p, therefore Si is not isomorphic to Sj if i≠j. Since EndM≅k we have that M is a tame A-module. Thus, Theorem 4 allows to conclude that M is an indecomposable Zavadskij module. We are done.
We recall that a connected quiver with n vertices and n−1 arrows is called a tree quiver (this means that the underlying graph is a tree in the sense of graph theory). We have an easy way to determine indecomposable Zavadskij modules over path algebras of tree quivers.
Corollary 8. If Q is tree quiver then the following bijection holds
Proof. Note that each indecomposable uniserial module determines a unique mast in Q because there are no double arrows in Q. Furthermore, [20,Lemma 2.1.1] implies that each path in Q determines exactly one uniserial kQ-module because there are only finitely many paths in Q and Q does not contain a subquiver of form
Let us consider the case when the direct sum of indecomposable Zavadskij modules over a finite-dimensional algebra A is a Zavadskij module via the masts associated.
Theorem 9. A direct sum M=Mn11⊕⋯⊕Mnrr of indecomposable pairwise non isomorphic Zavadskij A-modules is a Zavadskij module if and only if for each pair i≠j the masts pi and pj associated with Mi and Mj respectively do not have common vertices.
Proof. Let pi:a0⟶a1⟶⋯⟶as and pj:a′0⟶a′1⟶⋯⟶a′t be the masts associated with the indecomposable A-modules Mi and Mj respectively, where i≠j. First, we suppose that pi and pj share the vertex a′k=ak. Since Mi(aj)=k for all 0≤j≤s, then Mj(ak)=k. Thus, the simple Sak is a composition factor of Mi and of Mj. Then Mi≅Mj which is contradiction (see Theorem 5). On the other hand, we assume that pi and pj do not have a vertex in common. Then M is of finite length because the k-algebra A is finite-dimensional, thus the reduced part ¯M=n⊕i=1Mi of M can be decomposed in tame uniserial A-modules provided that each module Mi is an indecomposable Zavadskij A-module. Now, if the simple Sa is a composition factor of Mi and Mj with i≠j, then Mi(a)≅k and Mj(a)≅k. Therefore a is a vertex in pi and pj, which contradicts the hypothesis, so Mi and Mj do not have a common composition factor. Since Theorem 5 establishes that ¯M and M are Zavadskij modules. We are done.
Example 10. Continuing Example 1, the indecomposable Zavadskij modules over the cluster-tilted algebra kQ/I correspond to: 1) the simple representations S1 to S4, whose masts associated are the stasionary paths e1 to e4 respectively. 2) the representations Mp such that p∈{α,β,γ,δ}, where Mp(x)=k if x∈{tp,hp} and zero otherwise. Moreover, M(p) is 1k whenever it is possible and zero otherwise. The masts associated with these modules are the arrows in Q. 3) the representation M such that M(x)=k if x is 1,2, or 3 and zero otherwise. Moreover M(α)=M(β)=1k. Note that the mast of M is the path αβ in Q. Direct sums as Mα⊕Mγ and M⊕S4 are Zavadskij modules while the direct sum Mβ⊕Mδ is not a Zavadskij module. Observe that in this case, the number of indecomposable Zavadskij modules is 9, and it is equal to the dimension of the algebra.
4.
The number of indecomposable Zavadskij modules over cluster-tilted algebras (An case)
This section gives a formula for the number of indecomposable Zavadskij modules over a cluster-tilted algebra A. Note that such a number equals the dimension of the algebra A. To do that, we use the geometric model for these algebras given in [8]. Moreover, we discuss the role of some integer sequences in the enumeration of indecomposable Zavadskij modules over certain families of algebras.
In [8] P. Caldero, F. Chapoton, and R. Schiffler considered regular polygons with n+3 vertices and triangulations of such polygons. A diagonal is a straight line between two non-adjacent vertices on the border of the polygon, and a triangulation is a maximal set of diagonals that do not cross. In fact, a triangulation of an (n+3)-gon consists of exactly n diagonals. In [8] the category of diagonals of such polygons was defined, and it was shown to be equivalent to the cluster category of type An; which, in greater generality, was defined simultaneously by A.B. Buan et al., [7]. They defined cluster categories as orbit categories of the bounded derived category of hereditary algebras. As an application in [8], the module category of a cluster-tilted algebra of type An is described by a category of diagonals CT, where T is a triangulation of the n+3-gon. Indeed, the cluster-tilted quivers of type An are precisely those that are associated with an arbitrary triangulation of the (n+3)-gon.
For any triangulation of the regular (n+3)-gon, we can define a quiver with n vertices in the following way. The vertices are the midpoints of the diagonals. There is an arrow between i and j if the corresponding diagonals bound a common triangle. The orientation is i→j if the diagonal corresponding to j can be obtained from the diagonal corresponding to i by rotating anticlockwise about their common vertex (see Figure 2).
It is also known from [8] that all quivers obtained in this way are quivers of cluster-tilted algebras of type An. Furthermore, in [11], it was shown that there is a bijection between isomorphism classes of cluster-tilted algebras of type An and triangulations of the (n+3)-gon up to rotation.
Define the set of relations I to be the set of all paths i→j→k such that there exists an arrow k→i. It is important to recall that every triangulation T of the (n+3)-gon gives rise to a cluster-tilted algebra kQT/I of type An and every cluster-tilted algebra is of this form. In particular, every Dynkin quiver of type An corresponds to a triangulation without internal triangles; that is, each triangle has at least one side on the boundary of the polygon.
According [21], a fan in T is a maximal subset Σv⊆T of at least two diagonals such that all the diagonals in Σv share the vertex v of Πn+3. We denote by FT the set of fans in T.
Example 11. Continuing with Example 1, we get the triangulation T (Figure 3).
Observe that the fans determined by the triangulation are FT={{2,4}, {4,3}, {1,2,3}}.
Definition 12. Let (Q,I) be a bound quiver. A path p∉I is said to be a maximal path in (Q,I) if there is no an arrow α∈Q1 such that pα∉I or αp∉I.
Lemma 13. Given a triangulation T of the (n+3)-gon and its corresponding cluster-tilted algebra kQT/I of type An, there is a bijection
Proof. Since the quiver QT is connected, then a maximal path in (QT,I) has a length of at least one. For each arrow i→j in QT, Figure 2 implies that the diagonals i and j belong to the same fan. Given a maximal path p:i1→i2→⋯→ik in (QT,I), since p∉I, p is an acycle path. If p belongs to a 3-cycle then the length of p is one because the length of two paths are zero, and we have a fan {i1,i2}, where the diagonals i1 and i2 are in a internal triangle in T. When p does not belong to a 3-cycle, we have a fan F={i1,i2,…,ik}. Indeed, the maximality of p implies the property of maximality of F. It is clear that, different fans correspond to different maximal paths in (QT,I). Finally, note that a fan F={i1,…,ik} in T, which has been drawn with blue color in Figure 4, defines a path p:i1→i2→⋯→ik (red color), which is maximal in (QT,I), because although the green arrows in Figure 4 are adjacent to p, these do not satisfy Definition 12.
We denote by tn the triangular number tn=n(n+1)2.
Theorem 14. Let FT={F1,…,Fr} be the set of fans in a triangulation T of the (n+3)-gon. The number of indecomposable Zavadskij modules over the cluster-tilted algebra kQT/I of type An is given by
where tj denotes the j-th triangular number.
Proof. Theorem 7 implies that the indecomposable Zavadskij modules over the algebra kQT/I are determined by all of the subpaths of maximal paths in (QT,I). Note that if p is a maximal path in (QT,I) and lenght(p)=r, there are exactly tr=r(r+1)2 subpaths in p, including the subpaths of length zero. Lemma 4.3 implies that each fan corresponds to a maximal path in (QT,I). Thus, the number of indecomposable Zavadskij modules over kQT/I is given by ∑F∈FTt|F|−h, where the number h corresponds to the paths of length zero which have been counted twice. In other words, h is the number of diagonals in T that belong to exactly two different fans. Since if Fi∩Fj≠∅, |Fi∩Fj|=1, we have that h=∑i≠jt|Fi∩Fj|.
Example 15. Continuing our running example, the number of indecomposable Zavadskij modules over the cluster-tilted algebra in Example 1 is (t3+t2+t2)−3=9. Note that, the cardinality of the fans {1,2,3}, {2,4}, and {4,3}, determine the summands t3=6, t2=3, and t2=3 respectively. Moreover, observe that there are three diagonals (2, 3 and 4) that belong to exactly two fans in T.
A particular case of cluster-tilted algebras of type A are the Dynkin algebras of type An, that is, path algebras associated with Dynkin quivers Q of type An, whose underlying graph ¯Q has the form given in Figure 5. In this case, the vertices 1 and n are called extreme vertices of Q. Corollary 16 provides a formula for the number of indecomposable Zavadskij modules over a Dynkin algebra of type An.
Corollary 16. Let kQ be a Dynkin algebra of type An and let FT be the set of fans in the triangulation T associated with Q. Then the number of indecomposable Zavadskij modules over kQ is given by
Proof. In the enumeration that we did in the proof of Theorem 14, h corresponds to the number of diagonals that belong to precisely two fans. In case (a), if k∈F∩F′, with F,F′∈FT, we have that k is either a non-extremal sink-vertex or a non-extremal source-vertex in Q and the number of these vertices in Q is equal to |FT|−1.
Recall that an algebra A is said to be right serial if every indecomposable projective right A-module is uniserial. An algebra A is called left serial if every indecomposable projective left A-module is uniserial. It is well known that a basic k-algebra A is right serial if and only if, for every vertex x of its ordinary quiver QA, there exists at most one arrow of source x (see [14]). Moreover, note that the path algebra A=kQ of a tree quiver Q is a right serial algebra if and only if Q has only one sink vertex i∈Q0.
Corollary 17. Let A=kQ be the right serial Dynkin algebra of type An whose unique sink vertex is i. Then the number of indecomposable Zavadskij A-modules is given by ZAi(n)=ti+tn−(i−1)−1.
Proof. Since the quiver Q has the shape 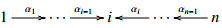 then the triangulation T of the (n+3)-gon associated with Q has two fans with i and n−(i−1) diagonals respectively. Moreover, there is exactly one diagonal (the diagonal i) that belong to the two fans. Theorem 14 implies the result.
then the triangulation T of the (n+3)-gon associated with Q has two fans with i and n−(i−1) diagonals respectively. Moreover, there is exactly one diagonal (the diagonal i) that belong to the two fans. Theorem 14 implies the result.
5.
Integer sequences arising from Zavadskij modules
As an application of the combinatorial approach studied in Sections 2 and 3, we found integer sequences arising from the number of indecomposable Zavadskij modules. In particular, a categorification of the sequence A000217 is given. In addition, we show some families of right serial path algebras whose number of indecomposable Zavadskij modules defines the integer sequences A005563, A002370, and A152948 encoded in OEIS [13].
Categorification of an integer sequence is a term coined by M. Ringel and P. Fahr [22,23] to the process for which numbers in the sequence can be seen as invariants of objects in such a way that functional relations between objects of the category can be interpreted as identities between numbers in the sequence (see [24,25] for more examples of categorifications).
Given the linearly oriented quiver Qn
of type An, all of the indecomposable modules in repQ are uniserial and each path in Q is the mast of a unique uniserial module. Then the number of indecomposable Zavadskij modules is the n-th triangular number. Now, we consider the linearly oriented infinite Dynkin quiver of type A∞, that is,
A representation V of QN is said to be of finite length if V(x)=0 for almost all vertices x∈(QN)0. Following [12], we denote by ReplfQN the category formed by locally finite dimensional representations, that is, directed unions of representations of finite length. It follows that the category ReplfQN is locally finite and replfQN consist of all objects of ReplfQN of finite length. In view of [12], each indecomposable object in replfQN is isomorphic to a finite-interval representation of the form
where kj=k for all m≤j≤s and 1≤m≤s<∞. Note that the finite-interval representations are indecomposable Zavadskij modules over the path algebra kQN and direct sums of them are Zavadskij modules if and only if each pair of different direct summands have disjunct support.
Let injlf(QN) be the full subcategory of replfQN generated by the representations 1Is, for all s≥1. The Auslander-Reiten quiver of injlf(QN) has the form
Then, we give the following categorification.
Theorem 18. The category injlf(QN) give a categorification of the integer sequence A000217 in the OEIS.
Proof. Let ϕ:injlf(QN)→N be the function given by
It associates to each indecomposable object 1Is∈injlf(QN) the triangular number ts. Note that this number is an invariant of the indecomposable objects in injlf(QN). Hence, we obtain that
This order relation of the indecomposable objects in injlf(QN) indicates that there are irreducible morphisms in injlf(QN) from 1Ik to 1Ik+1, for all k≥1.
Let A=kQn,i be a right serial Dynkin algebra of type An, where the quiver Qn,i has the form
that is, Qn,i has a unique sink vertex i. For any i≥1 fixed and N≥i={i,i+1,…}, we define the function
where ZAi(n) is the number of indecomposable Zavadskij modules over the path algebra kQn,i. Table 1 shows the values of the formula ZAi(n), for 3≤n≤10 and a unique sink at vertex i (see Corollary 17).
Proposition 19. The integer sequences A152948, A005563, and A002370 in the OEIS count indecomposable Zavadskij modules over certain right serial Dynkin algebras of type An.
Proof. For i=2, we have the sequence of quivers Q2,2=1→2, Q3,2=1→2←3, Q4,2=1→2←3←4, and so on. In this case, ZAi(2)=t2, ZAi(3)=t2+t2−t1, ZAi(4)=t2+t3−t1. In general, we have ZAi(k)=t2+tk−1−t1=(k−1)(k)2+2=k2−k+42. Replacing k by 2−n, we have the sequence a(n)=n2−3n+62 for n≥3, which is encoded by A152948 in the OEIS. Let {Qk}k≥1 be the family of Dynkin quivers of type A2k whose unique sink vertex is k+1, that is, Q1=Q2,2:1→2, Q2=Q4,3:1→2→3←4, Q3=Q6,4:1→2→3→4←5←6, and so on. Note that the number of indecomposable Zavadskij modules over the algebra kQn is given by tn+1+tn−1=(n+1)2−1, this formula corresponds to the integer sequence encoded by A005563 in OEIS. Finally, we consider the family {Qk}k≥1 of Dynkin quivers of type A2k+1 whose unique sink vertex is k+2. More precisely, the sequence of quivers is Q1=Q3,3:1→2→3, Q2=Q5,4:1→2→3→4←5, Q3=Q7,5:1→2→3→4→5←6←7, and so on. Note that the number of indecomposable Zavadskij modules over the algebra kQk is given by tk+2+tk−t1=k2+3k+2=(k+1)(k+2). If we replace k+1 by n, we obtain the sequence n(n+1) for n≥2, which is encoded by A002370 in OEIS.
6.
Concluding remarks
The indecomposable Zavadskij modules over a finite-dimensional algebra kQ/I are precisely the uniserial modules whose support is a path (called mast) in Q that does not belong to I, and this path does not pass by one same vertex twice or more. The direct sum of Zavadskij modules is a Zavadskij module when each pair of masts associated with the summands have disjoint support. The number of indecomposable Zavadskij modules is determined when the algebra is cluster-tilted of type A, in this case, there are some integer sequences associated with this formula in some particular cases. An interesting case is given when the algebra is the path algebra associated with the natural numbers, where there is an arrow from n to n+1 for each n∈N; in this case, a categorification of the integer sequence A000217 was obtained via Zavadskij modules that are finite-interval modules.
Acknowledgments
The first author has been supported by the Seminar Alexander Zavadskij on Representation of Algebras and Its Applications at Universidad Nacional de Colombia. The second and third author have been supported by Minciencias-Colombia Convocatoria fortalecimiento de vocaciones y formación en CTeI para la reactivación económica en el marco de la postpandemia 2020. No 891.
Conflict of interest
The authors declare there are no conflicts of interest.









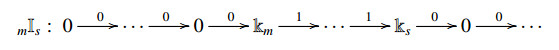
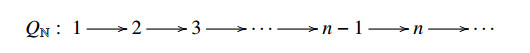
 DownLoad:
DownLoad:

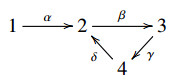
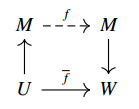

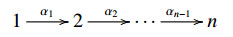




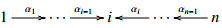 then the triangulation
then the triangulation